High-Q-Factor Tunable Silica-Based Microring Resonators
Abstract
:1. Introduction
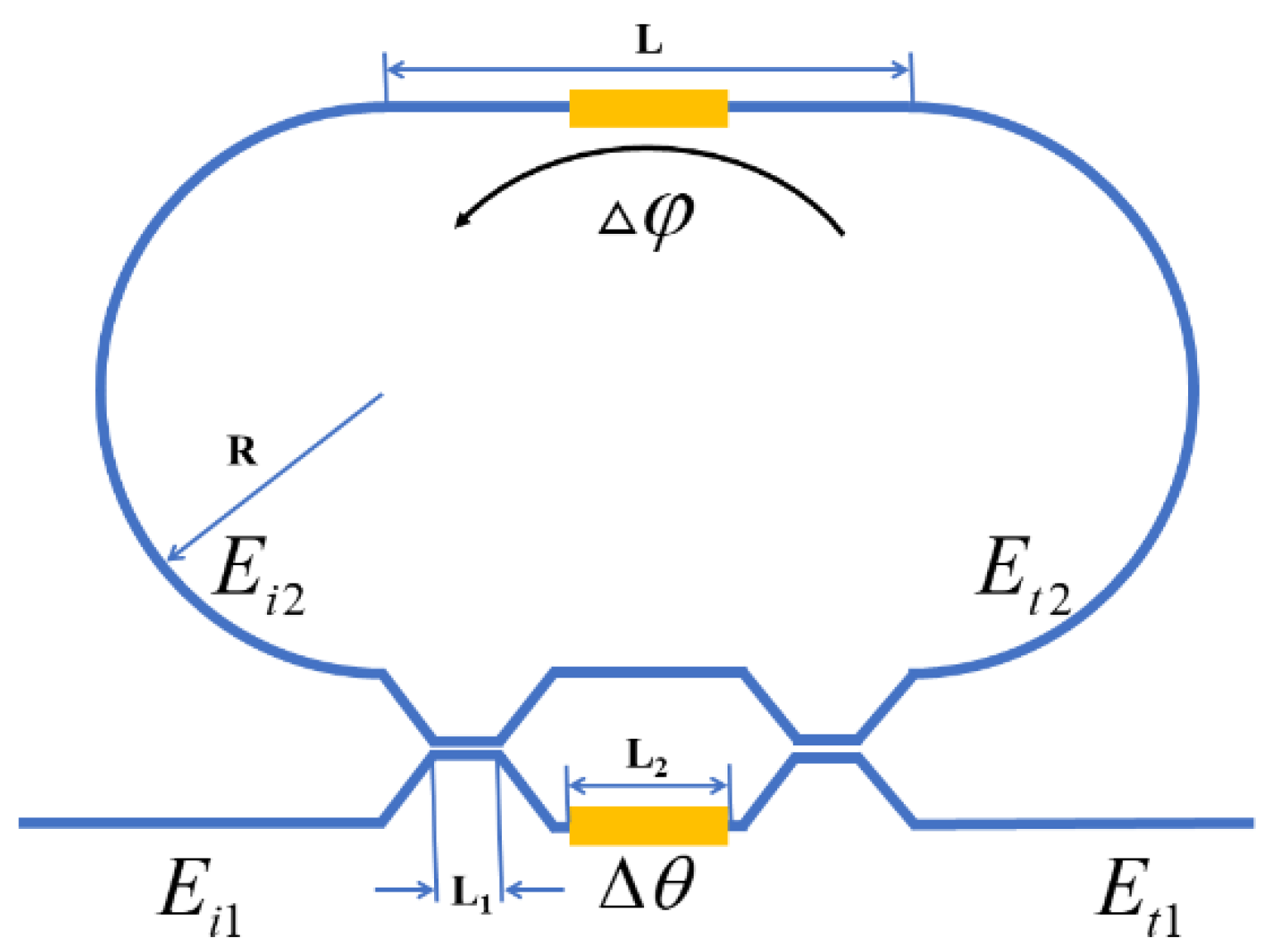
2. Structure and Design
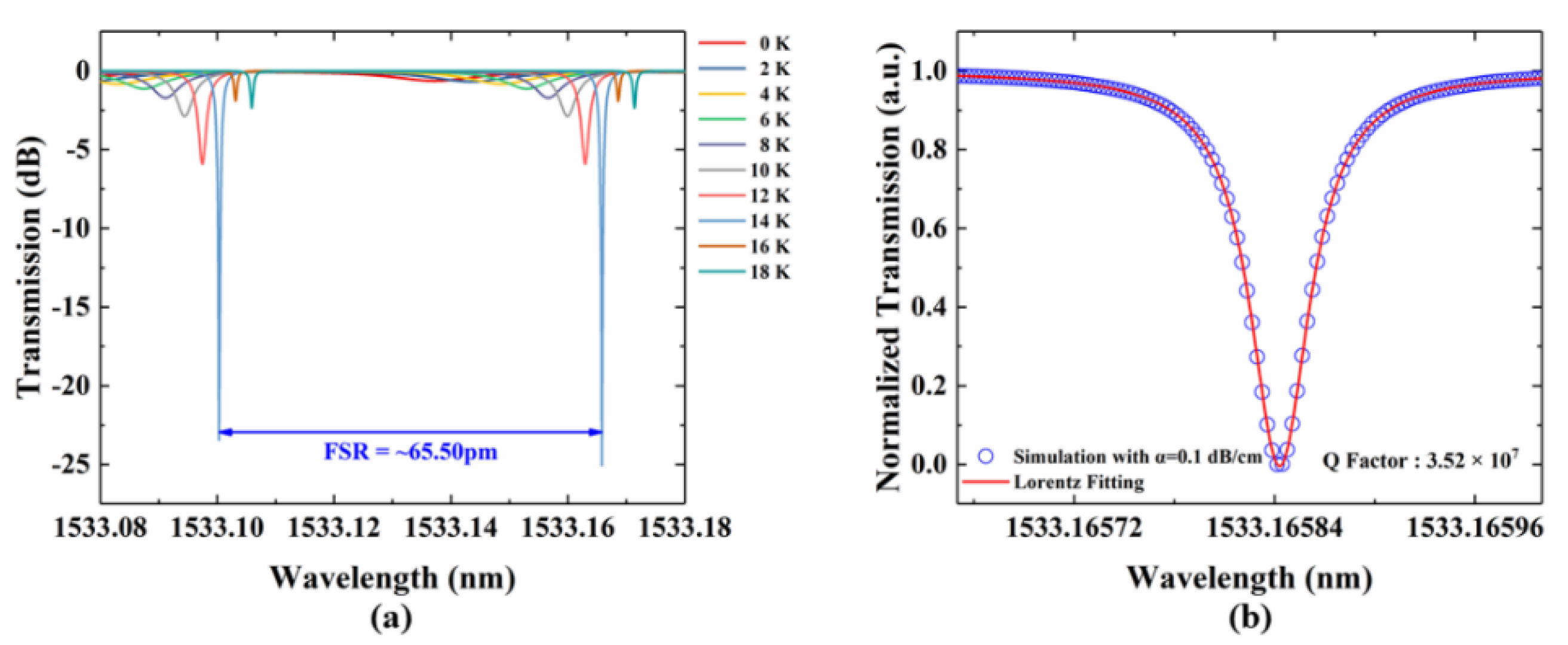
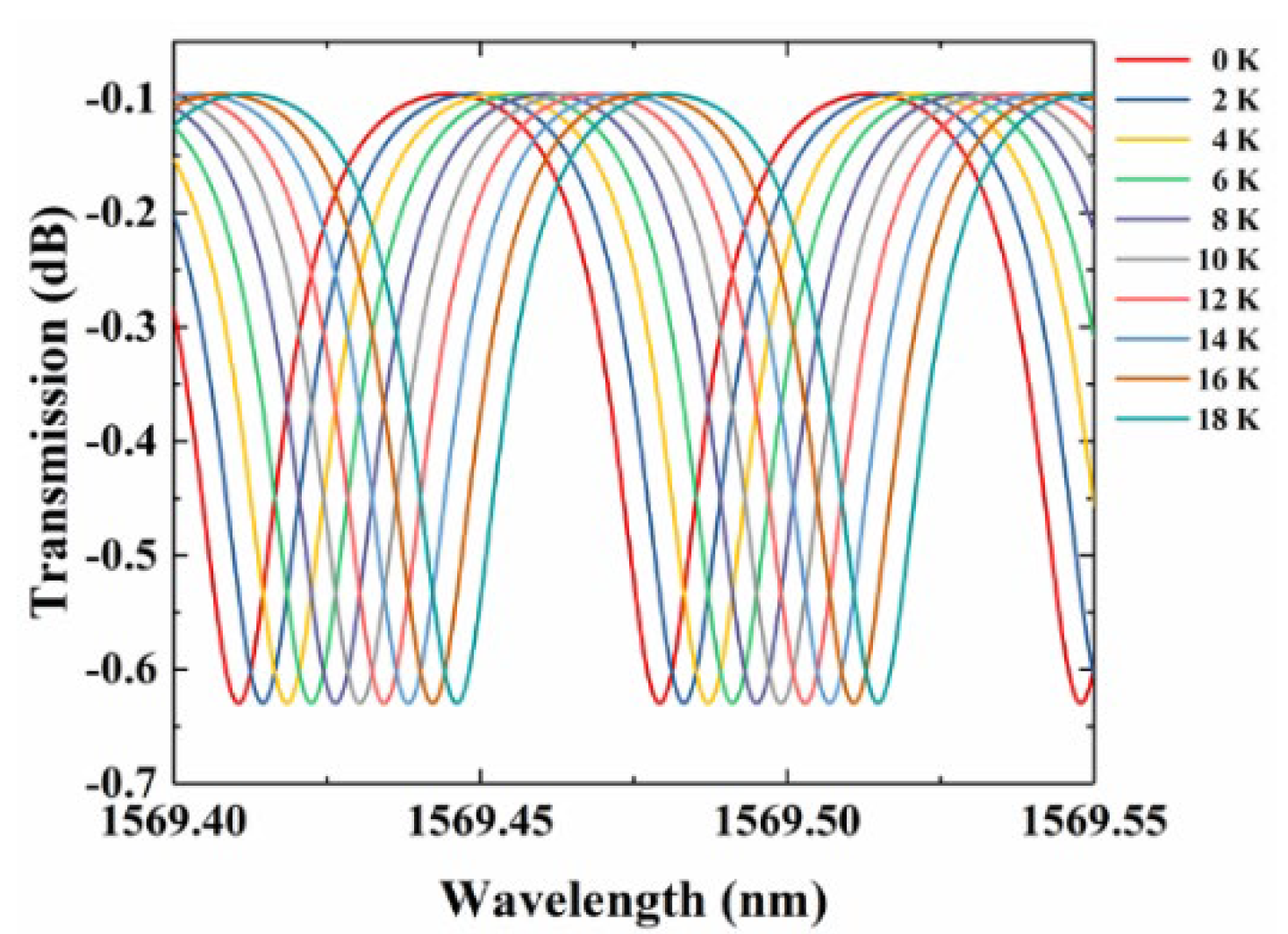
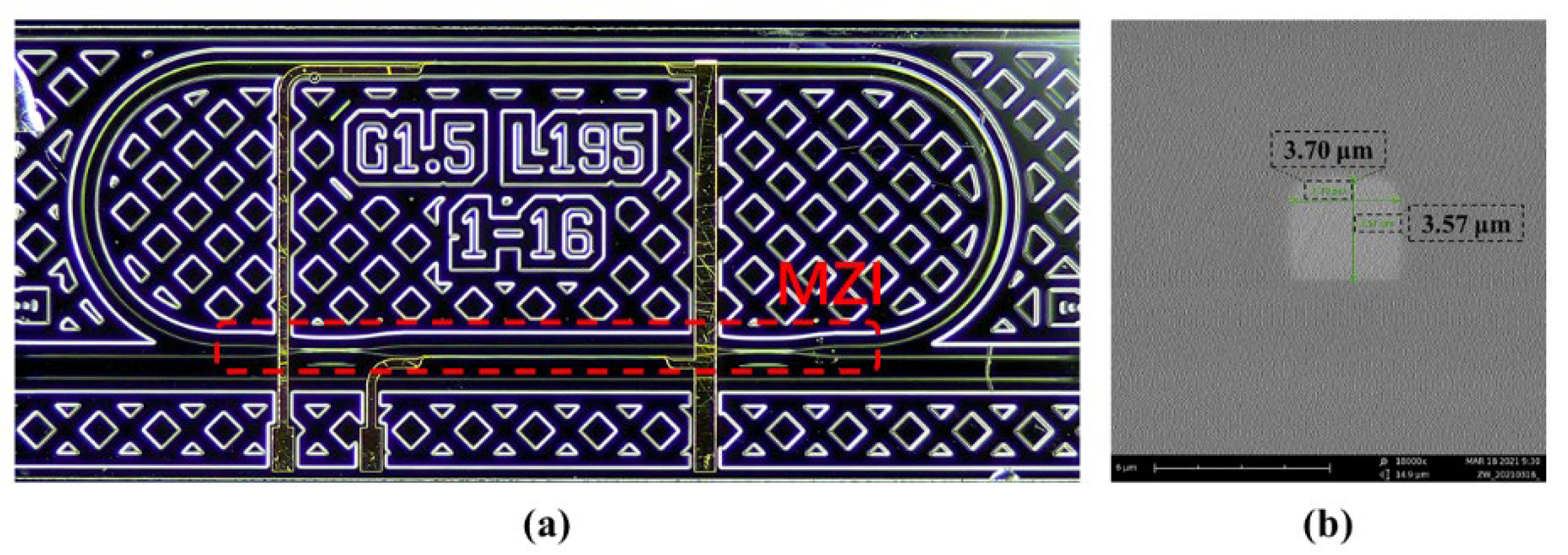
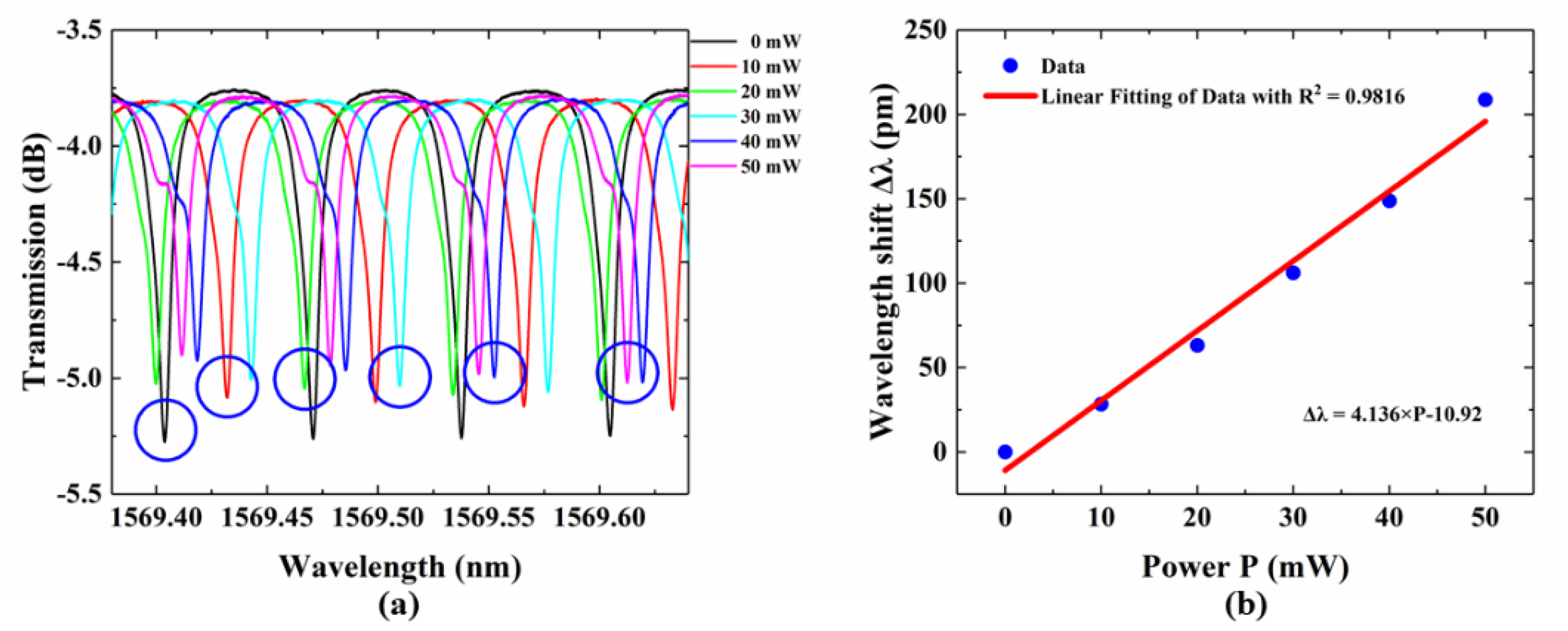
3. Fabrication and Measurement
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Mori, Y.; Honda, E.; Shiraki, R.; Suzuki, K.; Matsuura, H.; Kawashima, H.; Namiki, S.; Ikeda, K.; Sato, K.-I. Wavelength-division demultiplexing enhanced by silicon-photonic tunable filters in ultra-wideband optical-path networks. J. Lightwave Technol. 2020, 38, 1002–1009. [Google Scholar] [CrossRef]
- Dai, D. Highly sensitive digital optical sensor based on cascaded high-q ring-resonators. Opt. Express 2009, 17, 23817–23822. [Google Scholar] [CrossRef] [PubMed]
- Tu, X.; Chen, S.-L.; Song, C.; Huang, T.; Guo, L.J. Ultrahigh q polymer microring resonators for biosensing applications. IEEE Photonics J. 2019, 11, 1–10. [Google Scholar] [CrossRef]
- Ye, Z.; Fülöp, A.; Helgason, Ó.B.; Andrekson, P.A.; Torres-Company, V. Low-loss high-q silicon-rich silicon nitride microresonators for kerr nonlinear optics. Opt. Lett. 2019, 44, 4. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Miller, S.A.; Yu, M.; Ji, X.; Griffith, A.G.; Cardenas, J.; Gaeta, A.L.; Lipson, M. Low-loss silicon platform for broadband mid-infrared photonics. Optica 2017, 4, 707–712. [Google Scholar] [CrossRef]
- Gao, Y.; Lo, J.-C.; Lee, S.; Patel, R.; Zhu, L.; Nee, J.; Tsou, D.; Carney, R.; Sun, J. High-power, narrow-linewidth, miniaturized silicon photonic tunable laser with accurate frequency control. J. Lightwave Technol. 2020, 38, 265–271. [Google Scholar] [CrossRef]
- Zhang, L.; Jie, L.; Zhang, M.; Wang, Y.; Xie, Y.; Shi, Y.; Dai, D. Ultrahigh-q silicon racetrack resonators. Photonics Res. 2020, 8, 684. [Google Scholar] [CrossRef]
- Spencer, D.T.; Bauters, J.F.; Heck, M.J.R.; Bowers, J.E. Integrated waveguide coupled si3n4 resonators in the ultrahigh-q regime. Optica 2014, 1, 153–157. [Google Scholar] [CrossRef] [Green Version]
- Ji, X.; Barbosa, F.A.S.; Roberts, S.P.; Dutt, A.; Cardenas, J.; Okawachi, Y.; Bryant, A.; Gaeta, A.L.; Lipson, M. Ultra-low-loss on-chip resonators with sub-milliwatt parametric oscillation threshold. Optica 2017, 4, 619–624. [Google Scholar] [CrossRef] [Green Version]
- Puckett, M.W.; Liu, K.; Chauhan, N.; Zhao, Q.; Jin, N.; Cheng, H.; Wu, J.; Behunin, R.O.; Rakich, P.T.; Nelson, K.D.; et al. 422 million intrinsic quality factor planar integrated all-waveguide resonator with sub-mhz linewidth. Nat. Commun. 2021, 12, 934. [Google Scholar] [CrossRef] [PubMed]
- Menon, V.M.; Tong, W.; Forrest, S.R. Control of quality factor and critical coupling in microring resonators through integration of a semiconductor optical amplifier. IEEE Photonics Technol. Lett. 2004, 16, 1343–1345. [Google Scholar] [CrossRef]
- Green, W.; Lee, R.; Derose, G.; Scherer, A.; Yariv, A. Hybrid ingaasp-inp mach-zehnder racetrack resonator for thermooptic switching and coupling control. Opt. Express 2005, 13, 1651–1659. [Google Scholar] [CrossRef] [Green Version]
- Ciminelli, C.; Dell’Olio, F.; Armenise, M.N.; Soares, F.M.; Passenberg, W. High performance inp ring resonator for new generation monolithically integrated optical gyroscopes. Opt. Express 2013, 21, 556–564. [Google Scholar] [CrossRef]
- Jin, L.; Wang, J.; Fu, X.; Yang, B.; Shi, Y.; Dai, D. High-q microring resonators with 2×2 angled multimode interference couplers. IEEE Photonics Technol. Lett. 2013, 25, 612–614. [Google Scholar] [CrossRef]
- Han, X.; Wang, L.; Wang, Y.; Zou, P.; Gu, Y.; Teng, J.; Wang, J.; Jian, X.; Morthier, G.; Zhao, M. Uv-soft imprinted tunable polymer waveguide ring resonator for microwave photonic filtering. J. Lightwave Technol. 2014, 32, 3924–3932. [Google Scholar] [CrossRef]
- Yin, Y.-X.; Yin, X.-J.; Zhang, X.-P.; Yan, G.-W.; Wang, Y.; Wu, Y.-D.; An, J.-M.; Wang, L.-L.; Zhang, D.-M. High-q-factor silica-based racetrack microring resonators. Photonics 2021, 8, 43. [Google Scholar] [CrossRef]
- Wang, L.; An, J.; Wu, Y.; Zhang, J.; Wang, Y.; Li, J.; Wang, H.; Zhang, X.; Pan, P.; Zhang, L.; et al. A compact and low-loss 1 × 8 optical power splitter using silica-based plc on quartz substrate. Opt. Commun. 2014, 312, 203–209. [Google Scholar] [CrossRef]
- Li, C.; An, J.; Zhang, J.; Wang, L.; Li, J.; Wang, Y.; Yin, X.; Wang, H.; Wu, Y. 4×20 ghz silica-based awg hybrid integrated receiver optical sub-assemblies. Chin. Opt. Lett. 2018, 16. [Google Scholar] [CrossRef]
- Liu, D.; Sun, S.; Yin, X.; Sun, B.; Sun, J.; Liu, Y.; Li, W.; Zhu, N.; Li, M. Large-capacity and low-loss integrated optical buffer. Opt. Express. 2019, 27, 11585–11593. [Google Scholar] [CrossRef]
- Lee, H.; Chen, T.; Li, J.; Yang, K.Y.; Jeon, S.; Painter, O.; Vahala, K.J. Chemically etched ultrahigh-q wedge-resonator on a silicon chip. Nat. Photonics 2012, 6, 369–373. [Google Scholar] [CrossRef] [Green Version]
- Wu, L.; Wang, H.; Yang, Q.; Ji, Q.X.; Shen, B.; Bao, C.; Gao, M.; Vahala, K. Greater than one billion q factor for on-chip microresonators. Opt. Lett. 2020, 45, 5129–5131. [Google Scholar] [CrossRef] [PubMed]
- Yi, X.; Yang, Q.-F.; Yang, K.Y.; Suh, M.-G.; Vahala, K. Soliton frequency comb at microwave rates in a high-q silica microresonator. Optica 2015, 2, 1078–1085. [Google Scholar] [CrossRef]
- Yuan, Z.; Wang, H.; Wu, L.; Gao, M.; Vahala, K. Linewidth enhancement factor in a microcavity brillouin laser. Optica 2020, 7, 1150. [Google Scholar] [CrossRef]
- Perentos, A.; Cuesta-Soto, F.; Rodrigo, M.; Canciamilla, A.; Vidal, B.; Pierno, L.; Griol, A.; Losilla, N.S.; Bellieres, L.; Lopez-Royo, F.; et al. Variable carrier reduction in radio-over-fiber systems for increased modulation efficiency using a si3n4 tunable extinction ratio ring resonator. Opt. Express 2012, 20, 25478–25488. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Huang, M.; Li, S.; Xue, M.; Zhao, L.; Pan, S. Flat-top optical resonance in a single-ring resonator based on manipulation of fast- and slow-light effects. Opt. Express 2018, 26, 23215–23220. [Google Scholar] [CrossRef]
- Ma, C.-S.; Xu, Y.-Z.; Yan, X.; Qin, Z.-K.; Wang, X.-Y. Optimization and analysis of series-coupled microring resonator arrays. Opt. Commun. 2006, 262, 41–46. [Google Scholar] [CrossRef]
- Rabus, D.G. Integrated Ring Resonators; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Ren, M.-Z.; Zhang, J.-S.; An, J.-M.; Wang, Y.; Wang, L.-L.; Li, J.-G.; Wu, Y.-D.; Yin, X.; Hu, X.-W. Low power consumption 4-channel variable optical attenuator array based on planar lightwave circuit technique. Chin. Phys. B 2017, 26, 74221. [Google Scholar] [CrossRef]
- Zhang, B.; Al Qubaisi, K.; Cherchi, M.; Harjanne, M.; Ehrlichman, Y.; Khilo, A.N.; Popovic, M.A. Compact multi-million q resonators and 100 mhz passband filter bank in a thick-soi photonics platform. Opt. Lett. 2020, 45, 3005–3008. [Google Scholar] [CrossRef]


| Ref. | Platform | R (μm) | FSR (pm) | ER (dB) | Q Factor |
|---|---|---|---|---|---|
| [11] | InP-based PLC | 200 | 250 | 10 | 2.2 × 104 |
| [12] | InP-based PLC | 50 | N.A. | 18.5 | N.A. |
| [13] | InP-based PLC | 1300 | 17.8 | 7.0 | 0.97 × 106 |
| [15] | Polymer-based PLC | 500 | 130 | 18 | 8.2 × 104 |
| [7] | SOI | 29 | 900 | 4 | 1.3 × 106 |
| [29] | SOI | 2600 | 5.1 GHz | 8 | 7.5 × 107 |
| [9] | SiN | 115 | N.A. | N.A. | 3.7 × 107 |
| [10] | SiN | 11,787 | 2.713 GHz | N.A. | 4.22 × 108 |
| [16] | Silica-based PLC | 1600 | 137 | 3 | 1.83 × 106 |
| This Work | Silica-based PLC | 1600 | 64 | 13.84 | 4.47 × 106 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yin, Y.-X.; Zhang, X.-P.; Yin, X.-J.; Li, Y.; Xu, X.-R.; An, J.-M.; Wu, Y.-D.; Liu, X.-P.; Zhang, D.-M. High-Q-Factor Tunable Silica-Based Microring Resonators. Photonics 2021, 8, 256. https://doi.org/10.3390/photonics8070256
Yin Y-X, Zhang X-P, Yin X-J, Li Y, Xu X-R, An J-M, Wu Y-D, Liu X-P, Zhang D-M. High-Q-Factor Tunable Silica-Based Microring Resonators. Photonics. 2021; 8(7):256. https://doi.org/10.3390/photonics8070256
Chicago/Turabian StyleYin, Yue-Xin, Xiao-Pei Zhang, Xiao-Jie Yin, Yue Li, Xin-Ru Xu, Jun-Ming An, Yuan-Da Wu, Xiao-Ping Liu, and Da-Ming Zhang. 2021. "High-Q-Factor Tunable Silica-Based Microring Resonators" Photonics 8, no. 7: 256. https://doi.org/10.3390/photonics8070256
APA StyleYin, Y.-X., Zhang, X.-P., Yin, X.-J., Li, Y., Xu, X.-R., An, J.-M., Wu, Y.-D., Liu, X.-P., & Zhang, D.-M. (2021). High-Q-Factor Tunable Silica-Based Microring Resonators. Photonics, 8(7), 256. https://doi.org/10.3390/photonics8070256





