Research on the Coverage of Ultraviolet Communication Network in the Arbitrary Polygon Area
Abstract
:1. Introduction
2. Preliminaries
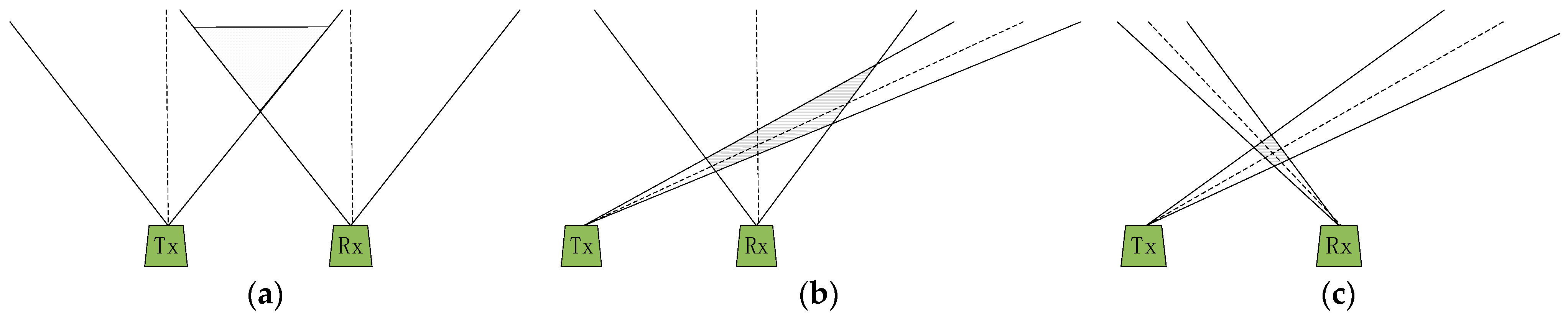
2.1. UV NLOS Scattering Model
2.2. UV Node Communication Radius
2.3. UV Network Coverage
2.3.1. Basic Concept
2.3.2. Evaluation Standard
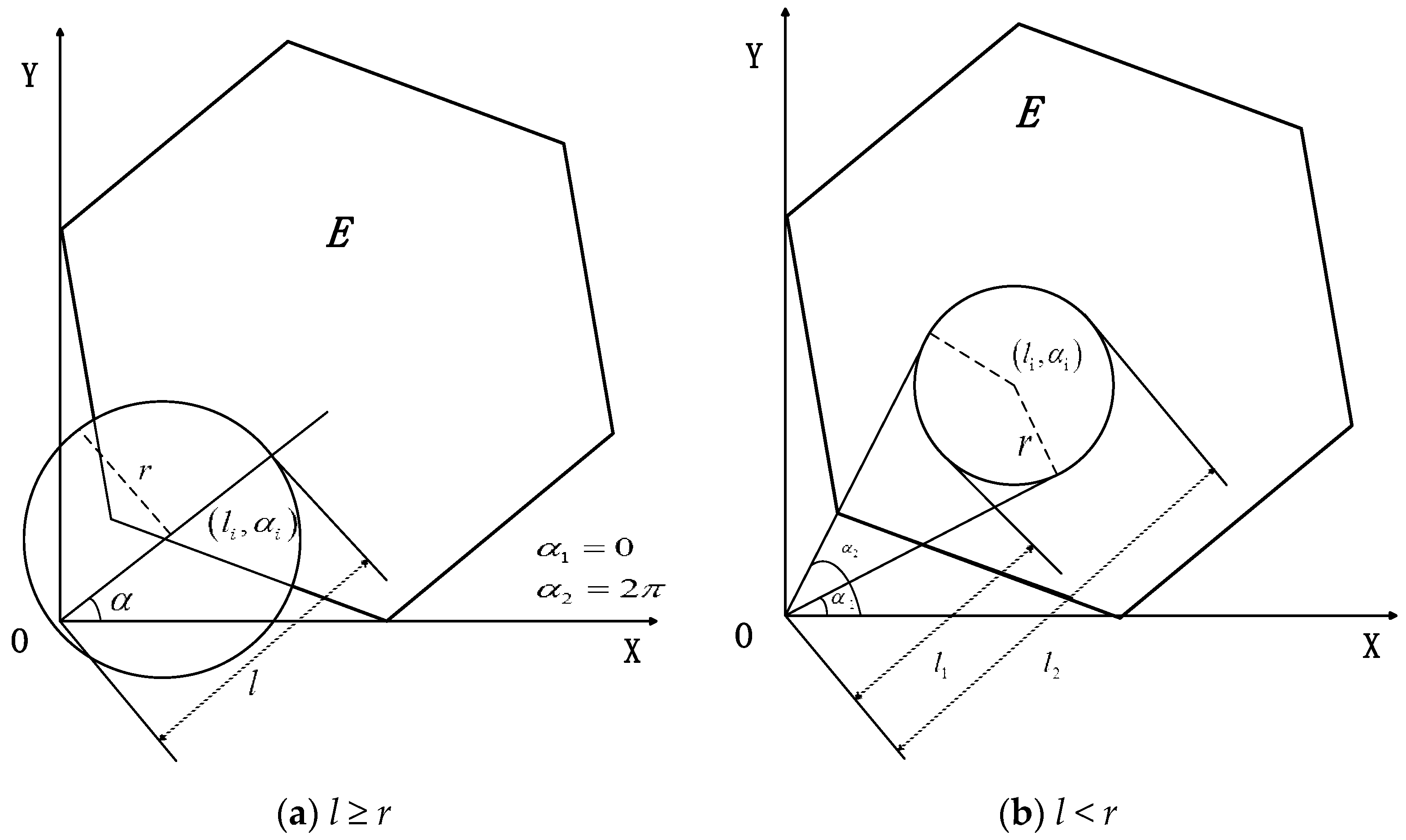
3. k-Connectivity
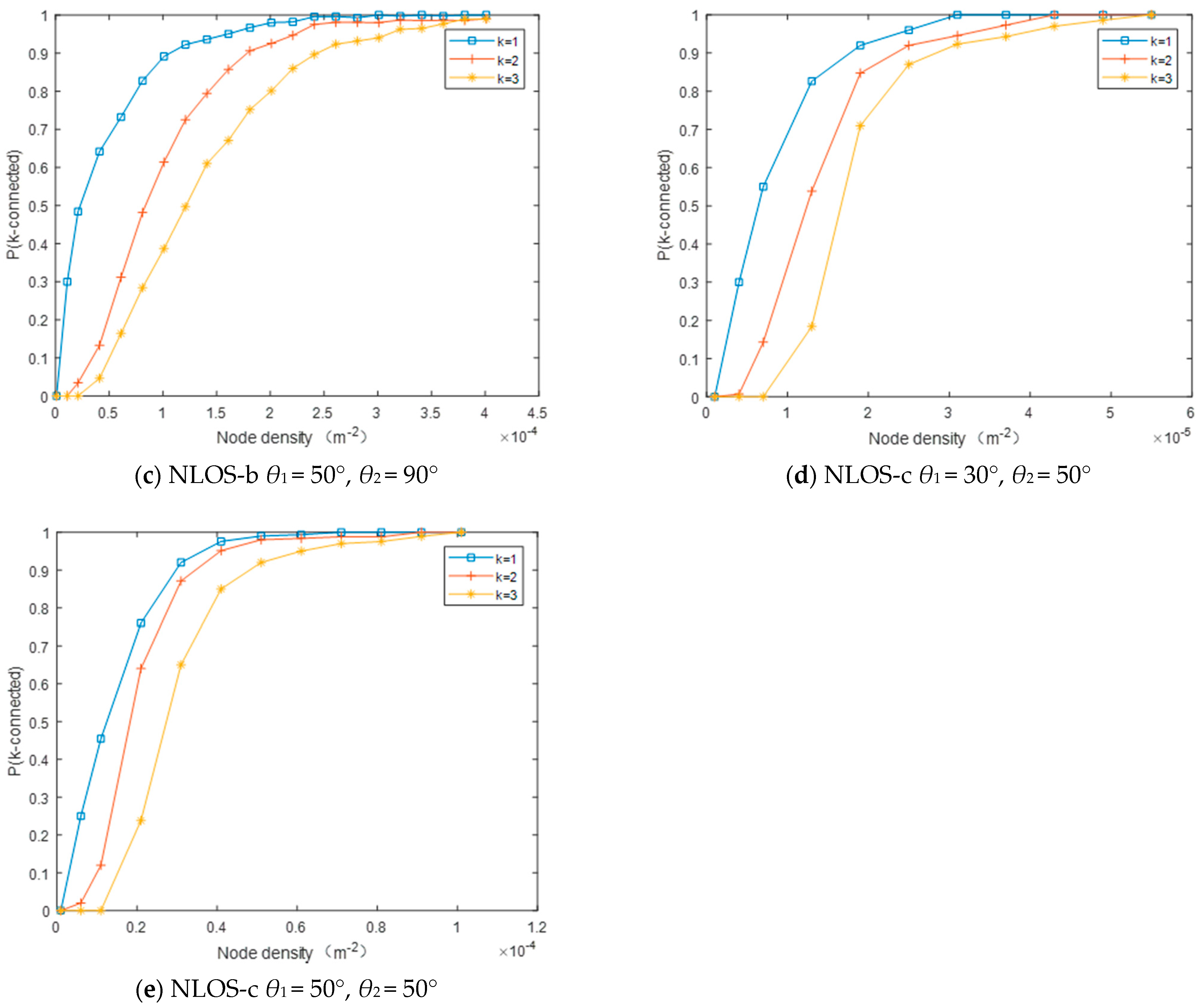
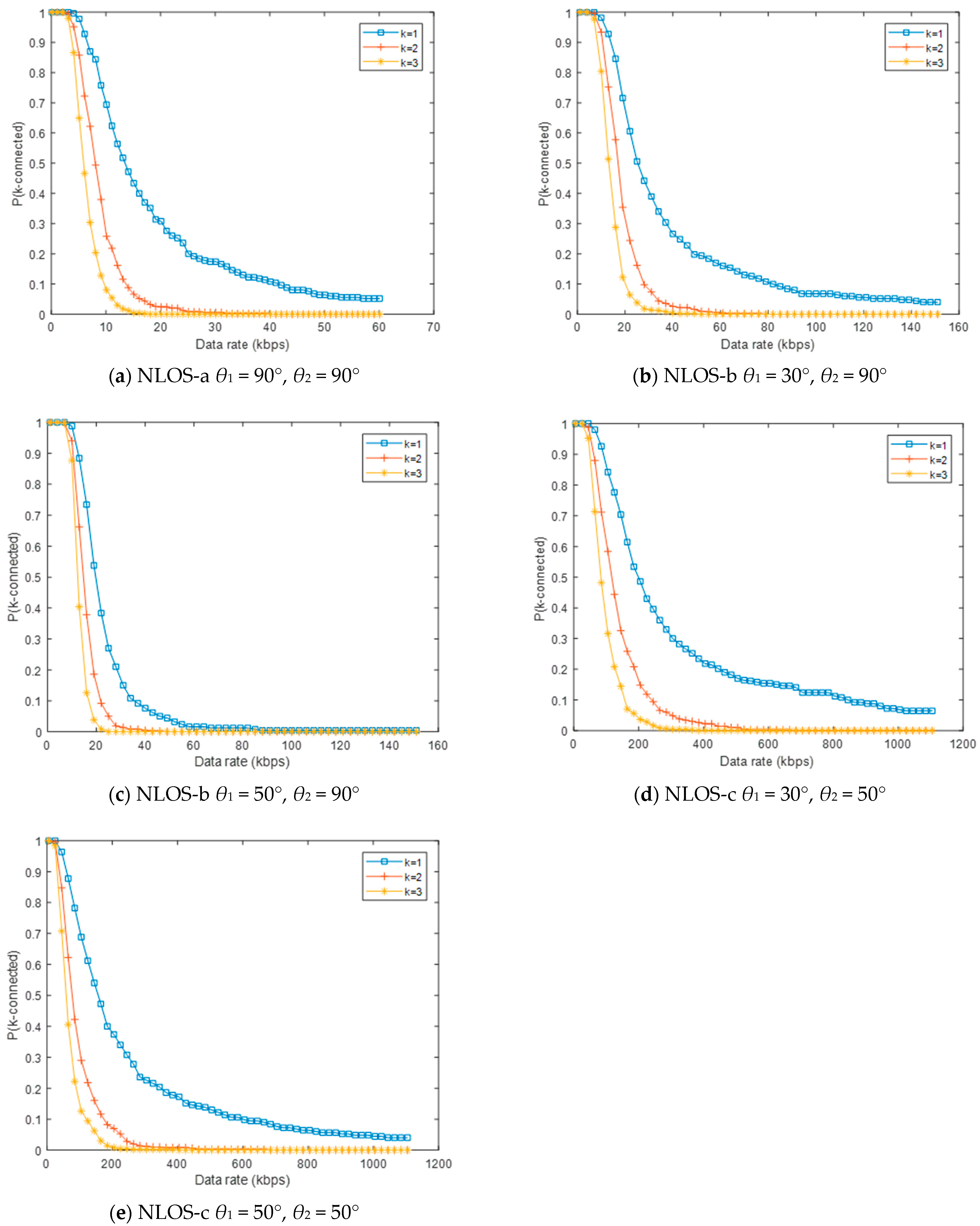
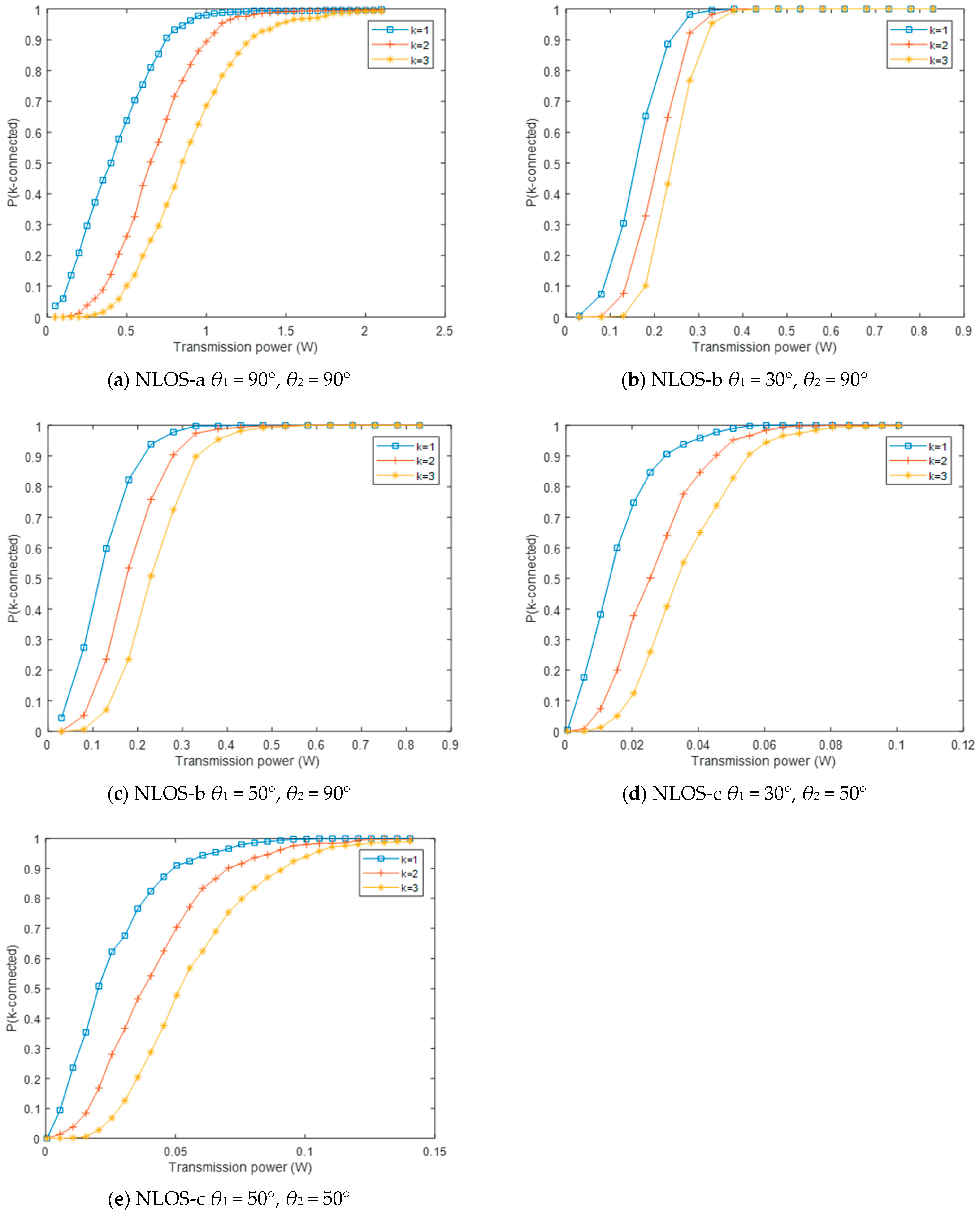
4. Simulation and Analysis
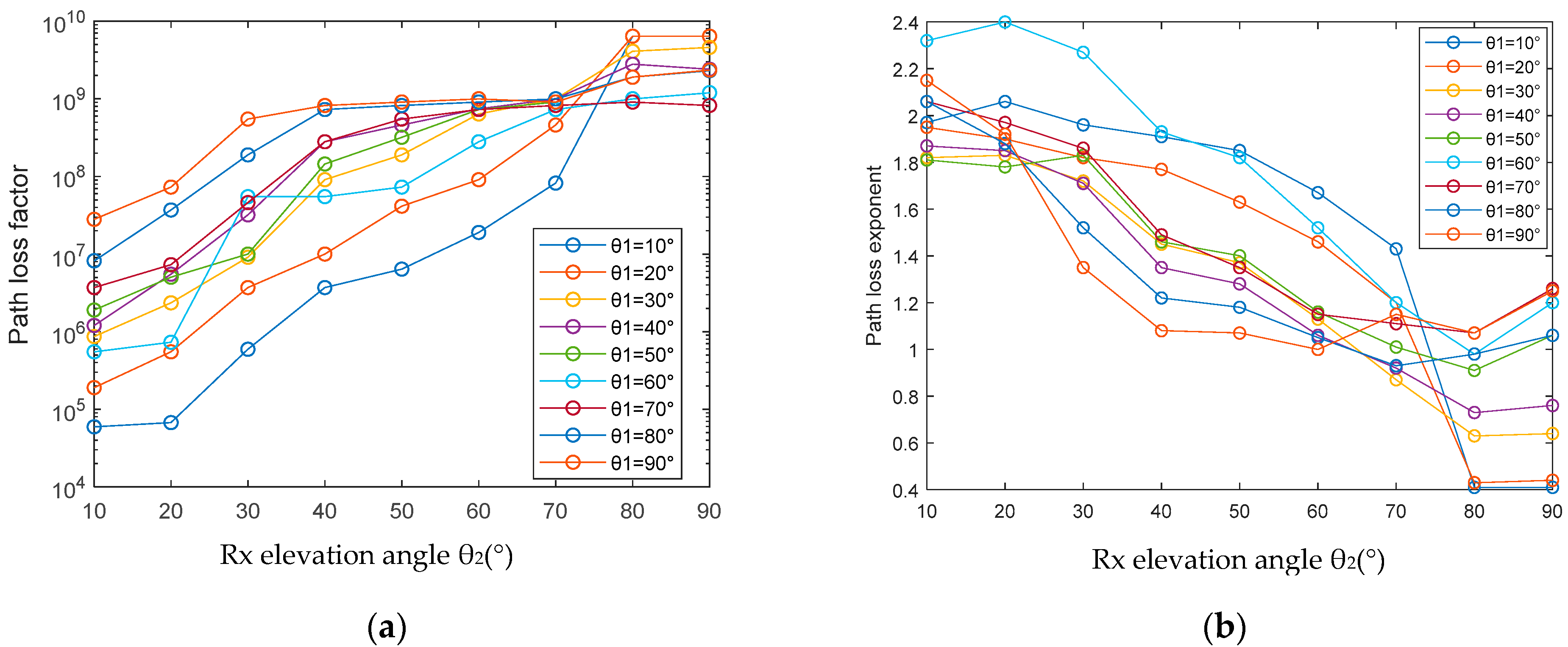
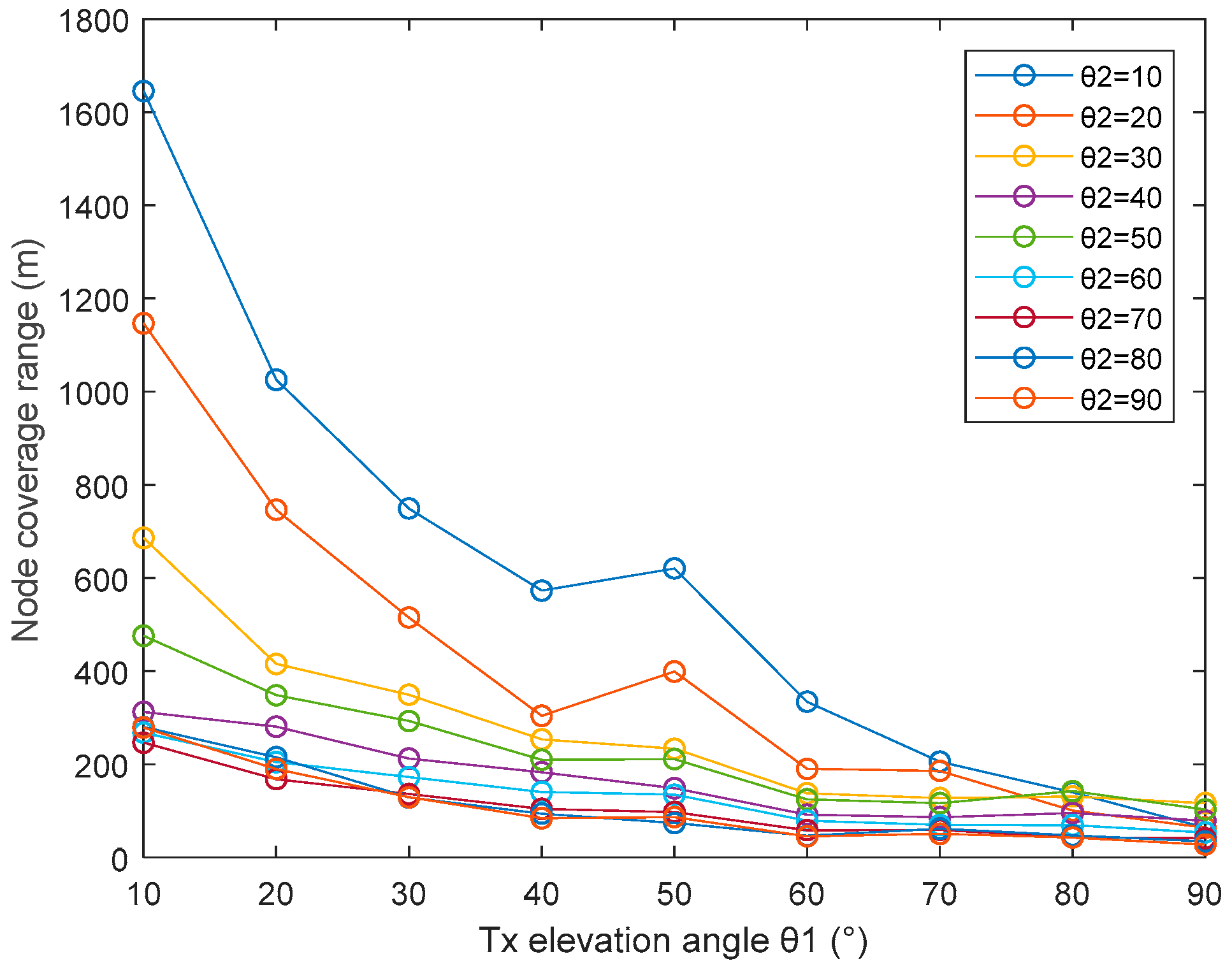
4.1. Transceiver Elevation Angles and Node Coverage Range
4.2. The Connectivity Properties
4.3. The 2-Connectivity Analysis for Two Distribution Regions
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Yong, Z.; Jian, W.; Houfei, X.; Jintong, L. Non-line-of-sight ultraviolet communication performance in atmospheric turbulence. China Commun. 2013, 10, 52–57. [Google Scholar] [CrossRef]
- Harvey, G.L. A Survey of Ultraviolet Communication Systems; Naval Research Lab: Washington, DC, USA, 1964. [Google Scholar]
- Sanderson, J.A. Optics at the naval research laboratory. Appl. Opt. 1967, 6, 2029–2043. [Google Scholar] [CrossRef]
- Shen, Z.; Ma, J.; Shan, T.; Su, P. Improved Monte Carlo Integration Models for Ultraviolet Communications. In Proceedings of the 2020 IEEE 20th International Conference on Communication Technology (ICCT), Nanning, China, 28–31 October 2020. [Google Scholar]
- Arya, S.; Chung, Y.H. A unified statistical model for Málaga distributed optical scattering communications. Opt. Commun. 2019, 463, 125402. [Google Scholar] [CrossRef]
- Kang, Y.; Zhao, J.; Wu, J.; Zhang, L.; Zhao, J.; Zhang, Y.; Zhao, Y.; Wang, X. Superior Deep-Ultraviolet Source Pumped by an Electron Beam for NLOS Communication. IEEE Trans. Electron Devices 2020, 67, 3391–3394. [Google Scholar] [CrossRef]
- Garg, K.K.; Bhatia, V. Performance Analysis of Cooperative NLOS UVC System with Receiver Diversity. In Proceedings of the 2020 National Conference on Communications (NCC), Kharagpur, India, 21–23 February 2020. [Google Scholar]
- Vavoulas, A.; Sandalidis, H.G.; Varoutas, D. Connectivity Issues for Ultraviolet UV-C Networks. IEEE/OSA J. Opt. Commun. Netw. 2011, 3, 199–205. [Google Scholar] [CrossRef]
- Zhao, T.F.; Wang, Y.; Gao, Y.Y. Connectivity performance analysis of UV-NLOS communication metwork. J. Optoelectron. Laser 2015, 26, 609–617. [Google Scholar]
- Li, C.; Li, J.; Xu, Z.; Wang, J. Study on the k-Connectivity of Ultraviolet Communication Network under Uniform Distribution of Nodes in a Circular Region. In Proceedings of the 2019 IEEE 5th International Conference on Computer and Communications (ICCC), Chengdu, China, 6–9 December 2020. [Google Scholar]
- Xu, Z. Approximate performance analysis of wireless ultraviolet links. In Proceedings of the 2007 IEEE International Conference on Acoustics, Speech and Signal Processing-ICASSP’07, Honolulu, HI, USA, 15–20 April 2007; pp. 577–580. [Google Scholar]
- Chen, G.; Xu, Z.; Ding, H.; Sadler, B.M. Path loss modeling and performance trade-off study for short-range non-line-of-sight ultraviolet communications. Opt. Express 2009, 17, 3929–3940. [Google Scholar] [CrossRef] [PubMed]
- Chen, G.; Abou-Galala, F.; Xu, Z.; Sadler, B.M. Experimental evaluation of LED-based solar blind NLOS communication links. Opt. Express 2008, 16, 15029–15068. [Google Scholar] [CrossRef]
- He, Q.; Sadler, B.M.; Xu, Z. Modulation and coding tradeoffs for non-line-of-sight ultraviolet communications. In Proceedings of the Free-Space Laser Communications IX. International Society for Optics and Photonics, San Diego, CA, USA, 2–5 August 2009; Volume 7464, pp. 74640H1–74640H12. [Google Scholar]
- Zhao, T.; Gao, Y.; Wu, P.; Xie, Y.; Song, P. A networking strategy for three-dimensional wireless ultraviolet communication network. Optik 2017, 151, 123–135. [Google Scholar] [CrossRef]
- Bettstetter, C. On the minimum node degree and connectivity of a wireless multihop network. In Proceedings of the 3rd ACM International Symposium on Mobile ad Hoc Networking & Computing, Lausanne, Switzerland, 9–11 June 2002; Volume 6, pp. 80–91. [Google Scholar]
- Bettstetter, C.; Krause, O. On border effects in modeling and simulation of wireless ad hoc networks. In Proceedings of the 3rd IEEE International Conference on Mobile and Wireless Communication Networks (WMAN), Stockholm, Sweden, 9–11 September 2001. [Google Scholar]
Short Biography of Authors



| Parameter | Value |
|---|---|
| wavelength (λ) | 250 nm |
| quantum efficiency of the filter and photodetector (η) | 0.045 |
| speed of light (c) | 3.0 × 108 m/s |
| bit error rate (Pe) | 10−6 |
| Planck constant (h) | 6.62 × 10−34 J·s |
| transmitted power (Pt) | 0.5 W |
| data rate (Rb) | 10 kbps |
| Regional Model | Circular Area | Regular Quadrilateral Area | ||||
|---|---|---|---|---|---|---|
| UV Parameters | Node Density (m−2) Rb = 10 kbps, Pt = 0.5 W | Transmission Power (W) ρ = 5.0 × 10−4 m−2, Rb = 10 kbps | Data Rate (kbps) ρ = 5.0 × 10−4 m−2, Pt = 0.5 W | Node Density (m−2) Rb = 10 kbps, Pt = 0.5 W | Transmission Power (W) ρ = 5.0 × 10−4 m−2, Rb = 10 kbps | Data Rate (kbps) ρ = 5.0 × 10−4 m−2, Pt = 0.5 W |
| NLOS-a: θ1 = 90°, θ2 = 90° | 4.51 × 10−3 | 2.24 | 3.11 | 1.71 × 10−3 | 1.12 | 4.41 |
| NLOS-b: θ1 = 30°, θ2 = 90° | 1.95 × 10−4 | 0.32 | 18.51 | 1.78 × 10−4 | 0.26 | 21.82 |
| NLOS-b: θ1 = 50°, θ2 = 90° | 4.32 × 10−4 | 0.52 | 12.83 | 2.19 × 10−4 | 0.31 | 18.11 |
| NLOS-c: θ1 = 30°, θ2 = 50° | 3.51 × 10−5 | 0.09 | 43.10 | 2.95 × 10−5 | 0.05 | 53.01 |
| NLOS-c: θ1 = 50°, θ2 = 50° | 6.50 × 10−5 | 0.31 | 18.71 | 4.32 × 10−5 | 0.28 | 33.20 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cao, F.; Li, C.; Xu, Z. Research on the Coverage of Ultraviolet Communication Network in the Arbitrary Polygon Area. Photonics 2021, 8, 240. https://doi.org/10.3390/photonics8070240
Cao F, Li C, Xu Z. Research on the Coverage of Ultraviolet Communication Network in the Arbitrary Polygon Area. Photonics. 2021; 8(7):240. https://doi.org/10.3390/photonics8070240
Chicago/Turabian StyleCao, Fang, Cheng Li, and Zhiyong Xu. 2021. "Research on the Coverage of Ultraviolet Communication Network in the Arbitrary Polygon Area" Photonics 8, no. 7: 240. https://doi.org/10.3390/photonics8070240
APA StyleCao, F., Li, C., & Xu, Z. (2021). Research on the Coverage of Ultraviolet Communication Network in the Arbitrary Polygon Area. Photonics, 8(7), 240. https://doi.org/10.3390/photonics8070240




