Photonic Nanojet Generation Using Integrated Silicon Photonic Chip with Hemispherical Structures
Abstract
:1. Introduction
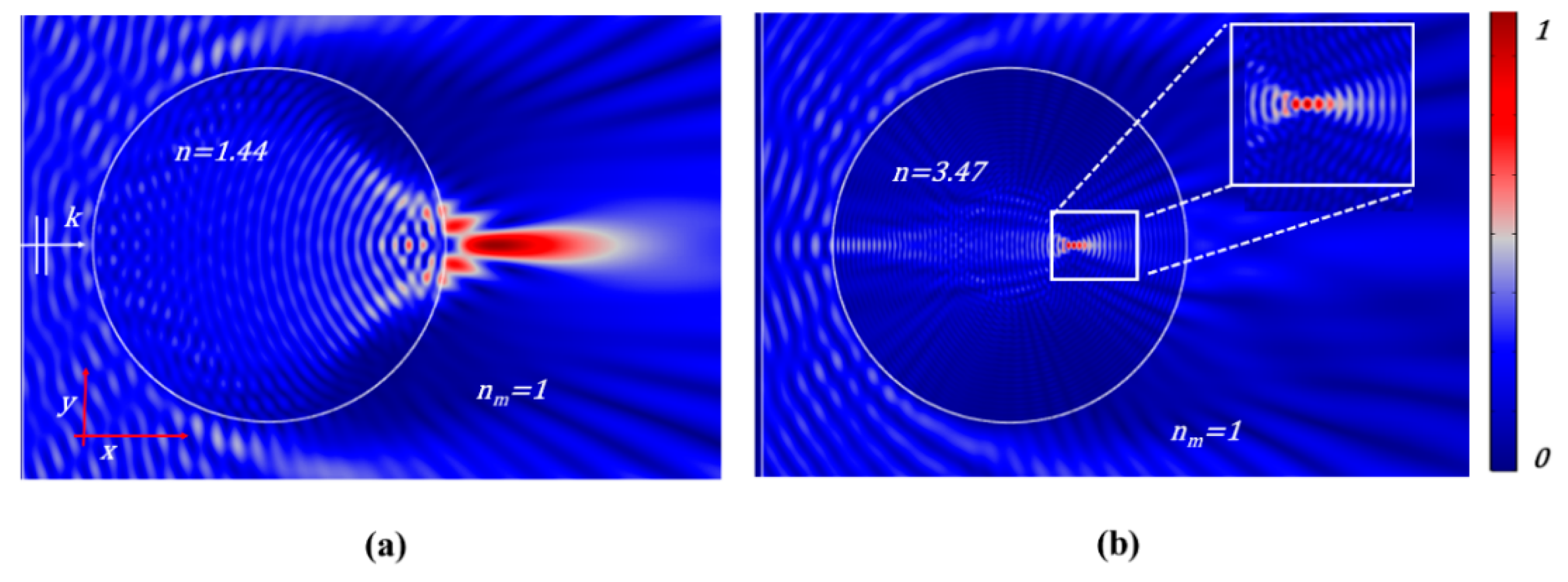
2. Modelling of Photonic Nanojet
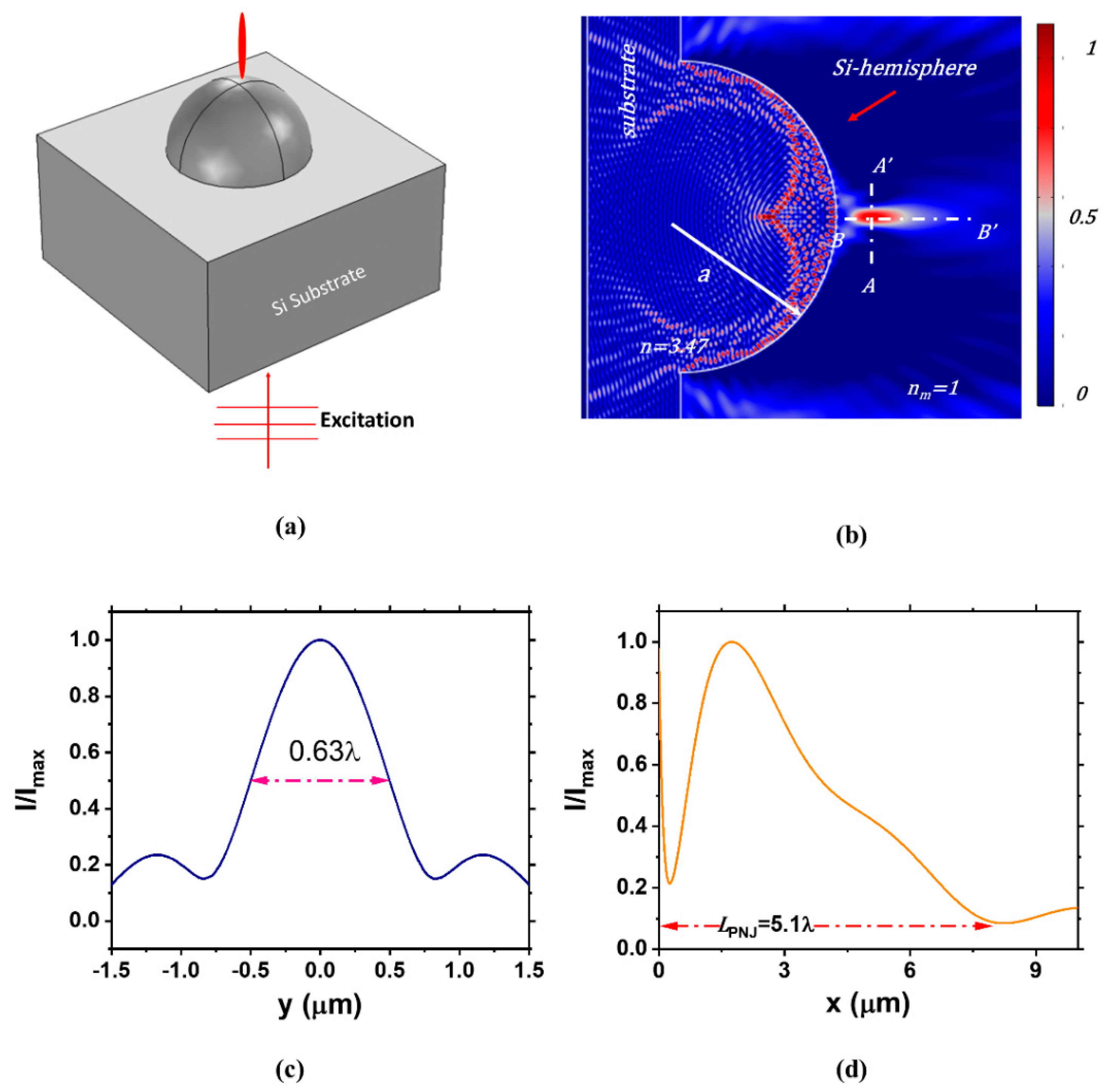
3. On-Chip Photonic Nanojet
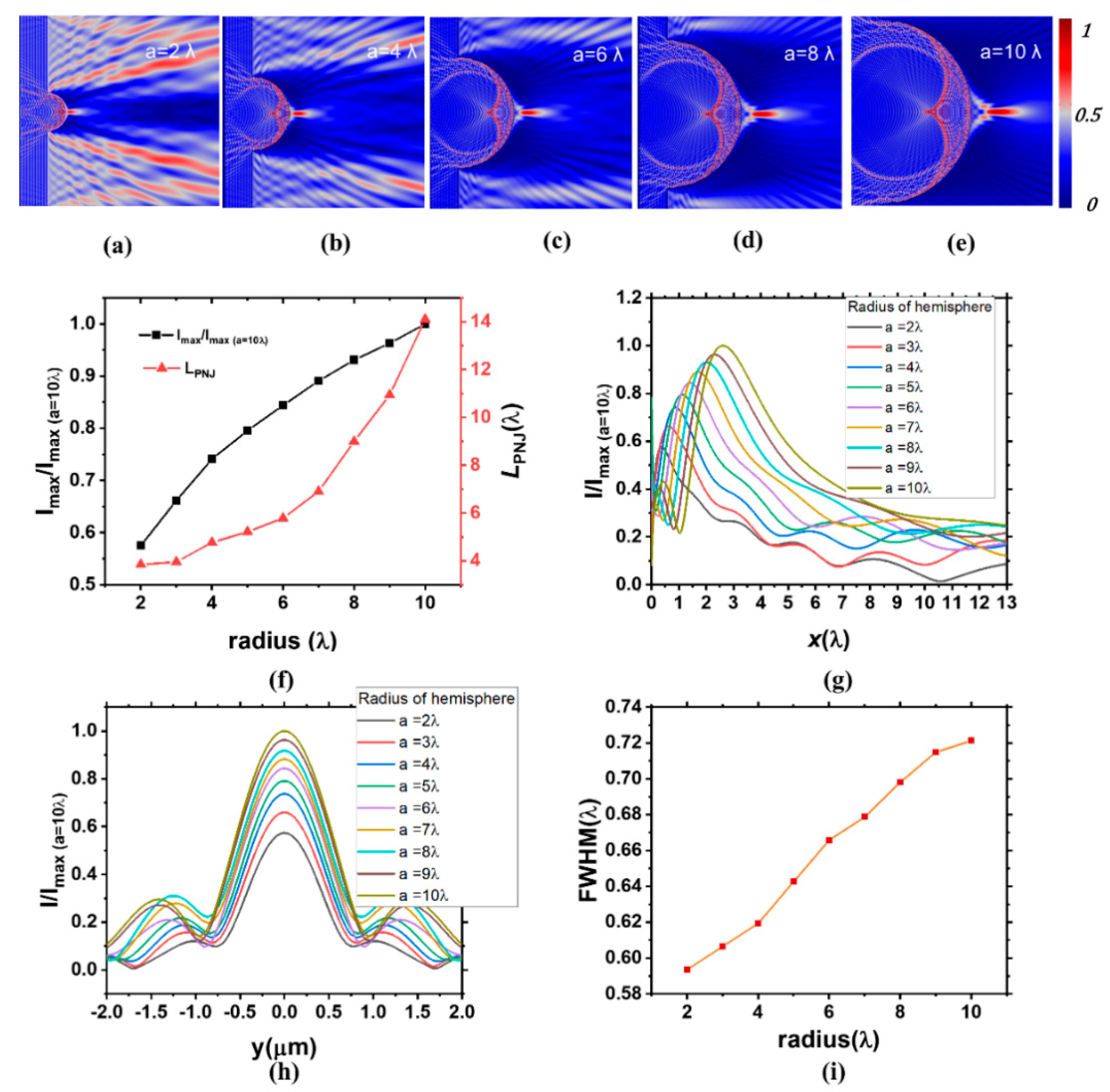
4. Effect of Radius of Dome
5. Effect of Sphericity of the Dome
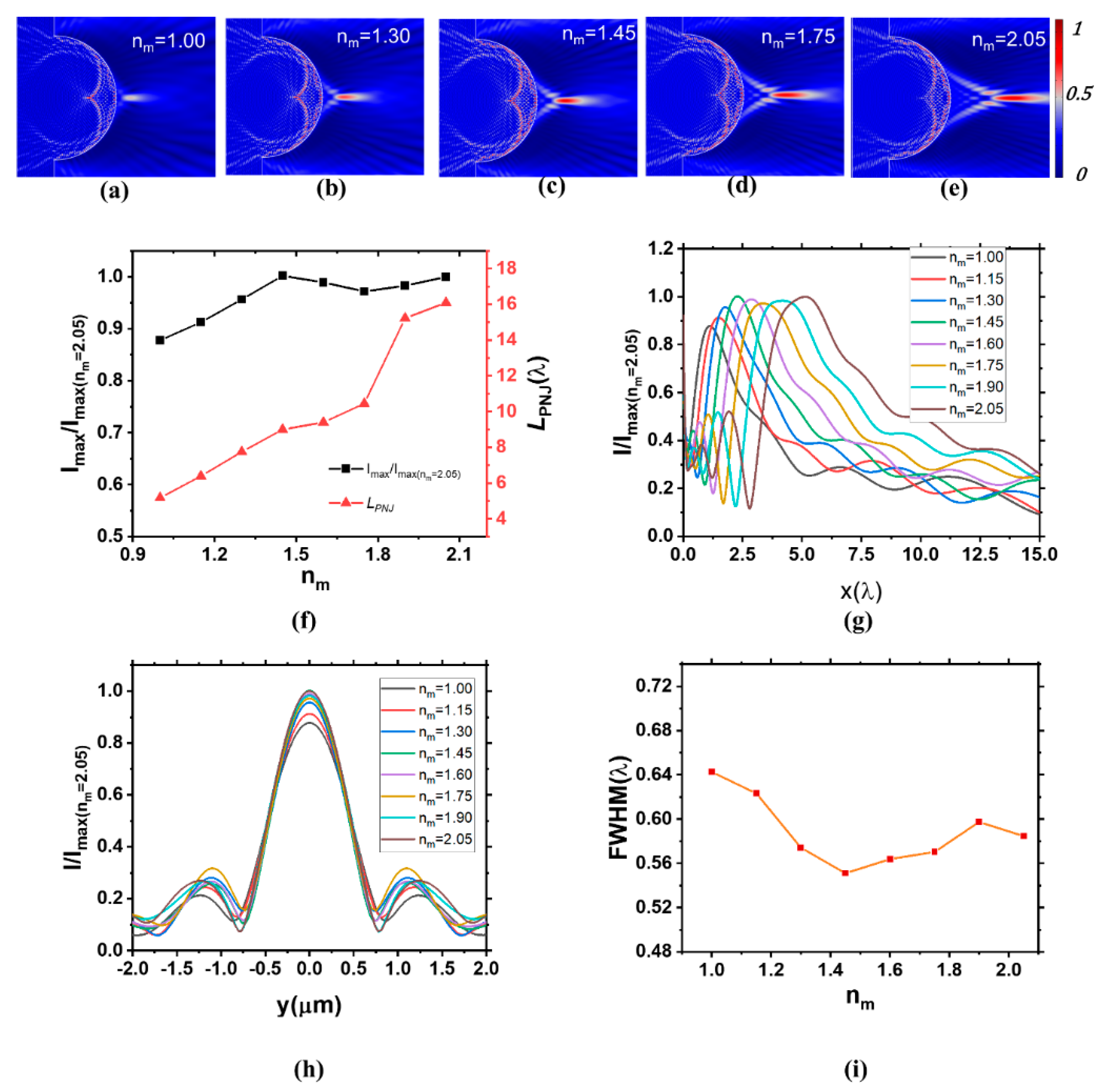
6. Significance of the Substrate and Medium Refractive Indices
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Chen, Z.; Taflove, A.; Backman, V. Photonic nanojet enhancement of backscattering of light by nanoparticles: A potential novel visible-light ultramicroscopy technique. Opt. Express 2004, 12, 1214. [Google Scholar] [CrossRef]
- Heifetz, A.; Kong, S.-C.; Sahakian, A.V.; Taflove, A.; Backman, V. Photonic nanojets. J. Comput. Theor. Nanosci. 2009, 6, 1979–1992. [Google Scholar] [CrossRef]
- Dantham, V.R.; Bisht, P.B.; Namboodiri, C.K.R. Enhancement of Raman scattering by two orders of magnitude using photonic nanojet of a microsphere. J. Appl. Phys. 2011, 109, 103103. [Google Scholar] [CrossRef]
- Veluthandath, A.V.; Bisht, P.B. Identification of Whispering Gallery Mode (WGM) coupled photoluminescence and Raman modes in complex spectra of MoS2 in Polymethyl methacrylate (PMMA) microspheres. J. Lumin. 2017, 187, 255–259. [Google Scholar] [CrossRef]
- Li, X.; Chen, Z.; Taflove, A.; Backman, V. Optical analysis of nanoparticles via enhanced backscattering facilitated by 3-D photonic nanojets. Opt. Express 2005, 13, 526. [Google Scholar] [CrossRef] [Green Version]
- Lecler, S.; Haacke, S.; Lecong, N.; Crégut, O.; Rehspringer, J.-L.; Hirlimann, C. Photonic jet driven non-linear optics: Example of two-photon fluorescence enhancement by dielectric microspheres. Opt. Express 2007, 15, 4935. [Google Scholar] [CrossRef]
- Born, B.; Geoffroy-Gagnon, S.; Krupa, J.D.A.; Hristovski, I.R.; Collier, C.M.; Holzman, J.F. Ultrafast All-Optical Switching via Subdiffractional Photonic Nanojets and Select Semiconductor Nanoparticles. ACS Photonics 2016, 3, 1095–1101. [Google Scholar] [CrossRef] [Green Version]
- Yang, H.; Trouillon, R.; Huszka, G.; Gijs, M.A.M. Super-resolution imaging of a dielectric microsphere is governed by the waist of its photonic nanojet. Nano Lett. 2016, 16, 4862–4870. [Google Scholar] [CrossRef]
- Du, B.; Zhang, H.; Xia, J.; Wu, J.; Ding, H.; Tong, G. Super-Resolution Imaging with Direct Laser Writing-Printed Microstructures. J. Phys. Chem. A 2020, 124, 7211–7216. [Google Scholar] [CrossRef]
- Darafsheh, A. Photonic nanojets and their applications. J. Phys. Photonics 2021, 3, 21. [Google Scholar] [CrossRef]
- Kim, J.; Cho, K.; Kim, I.; Kim, W.M.; Lee, T.S.; Lee, K.S. Fabrication of plasmonic nanodiscs by photonic nanojet lithography. Appl. Phys. Express 2012, 5, 3. [Google Scholar] [CrossRef]
- Jacassi, A.; Tantussi, F.; Dipalo, M.; Biagini, C.; MacCaferri, N.; Bozzola, A.; De Angelis, F. Scanning Probe Photonic Nanojet Lithography. ACS Appl. Mater. Interfaces 2017, 9, 32386–32393. [Google Scholar] [CrossRef] [Green Version]
- Wu, W.; Katsnelson, A.; Memis, O.G.; Mohseni, H. A deep sub-wavelength process for the formation of highly uniform arrays of nanoholes and nanopillars. Nanotechnology 2007, 18. [Google Scholar] [CrossRef]
- Yang, H.; Cornaglia, M.; Gijs, M.A.M. Photonic nanojet array for fast detection of single nanoparticles in a flow. Nano Lett. 2015, 15, 1730–1735. [Google Scholar] [CrossRef] [PubMed]
- Neves, A.A.R. Photonic nanojets in optical tweezers. J. Quant. Spectrosc. Radiat. Transf. 2015, 162, 122–132. [Google Scholar] [CrossRef] [Green Version]
- Li, Y.; Xin, H.; Liu, X.; Zhang, Y.; Lei, H.; Li, B. Trapping and Detection of Nanoparticles and Cells Using a Parallel Photonic Nanojet Array. ACS Nano 2016, 10, 5800–5808. [Google Scholar] [CrossRef] [PubMed]
- Gu, G.; Song, J.; Liang, H.; Zhao, M.; Chen, Y.; Qu, J. Overstepping the upper refractive index limit to form ultra-narrow photonic nanojets. Sci. Rep. 2017, 7, 5635. [Google Scholar] [CrossRef]
- Luk’yanchuk, B.S.; Paniagua-Domínguez, R.; Minin, I.; Minin, O.; Wang, Z. Refractive index less than two: Photonic nanojets yesterday, today and tomorrow [Invited]. Opt. Mater. Express 2017, 7, 1820. [Google Scholar] [CrossRef]
- Pacheco-Peña, V.; Beruete, M. Photonic nanojets with mesoscale high-index dielectric particles. J. Appl. Phys. 2019, 125. [Google Scholar] [CrossRef]
- Zhen, Z.; Huang, Y.; Feng, Y.; Shen, Y.; Li, Z. An ultranarrow photonic nanojet formed by an engineered two-layer microcylinder of high refractive-index materials. Opt. Express 2019, 27, 9178. [Google Scholar] [CrossRef]
- Geints, Y.E.; Zemlyanov, A.A.; Minin, I.V.; Minin, O.V. Overcoming refractive index limit of mesoscale light focusing by means of specular-reflection photonic nanojet. Opt. Lett. 2020, 45, 3885–3888. [Google Scholar] [CrossRef] [PubMed]
- Zhou, S.; Zhou, T. An ultra-narrow photonic nanojet generated from a high refractive-index micro-flat-ended cylinder. Appl. Phys. Express 2020, 13, 42010. [Google Scholar] [CrossRef]
- Loomis, J.; Ratnayake, D.; McKenna, C.; Walsh, K.M. Grayscale lithography—Automated mask generation for complex three-dimensional topography. J. Micro/Nanolithogr. MEMS MOEMS 2016, 15, 013511. [Google Scholar] [CrossRef]
- Lee, G.J.; Kim, H.M.; Song, Y.M. Design and fabrication of microscale, thin-film silicon solid immersion lenses for mid-infrared application. Micromachines 2020, 11, 250. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Jalali, T.; Erni, D. Highly confined photonic nanojet from elliptical particles. J. Mod. Opt. 2014, 61, 1069–1076. [Google Scholar] [CrossRef]
- Cargille Refractive Index Liquids. Available online: https://www.cargille.com/available-refractive-indices-sds-datasheets/ (accessed on 9 December 2021).

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Veluthandath, A.V.; Murugan, G.S. Photonic Nanojet Generation Using Integrated Silicon Photonic Chip with Hemispherical Structures. Photonics 2021, 8, 586. https://doi.org/10.3390/photonics8120586
Veluthandath AV, Murugan GS. Photonic Nanojet Generation Using Integrated Silicon Photonic Chip with Hemispherical Structures. Photonics. 2021; 8(12):586. https://doi.org/10.3390/photonics8120586
Chicago/Turabian StyleVeluthandath, Aneesh Vincent, and Ganapathy Senthil Murugan. 2021. "Photonic Nanojet Generation Using Integrated Silicon Photonic Chip with Hemispherical Structures" Photonics 8, no. 12: 586. https://doi.org/10.3390/photonics8120586
APA StyleVeluthandath, A. V., & Murugan, G. S. (2021). Photonic Nanojet Generation Using Integrated Silicon Photonic Chip with Hemispherical Structures. Photonics, 8(12), 586. https://doi.org/10.3390/photonics8120586






