Reduction in DC-Drift in LiNbO3-Based Electro-Optical Modulator
Abstract
:1. Introduction
2. Materials and Methods
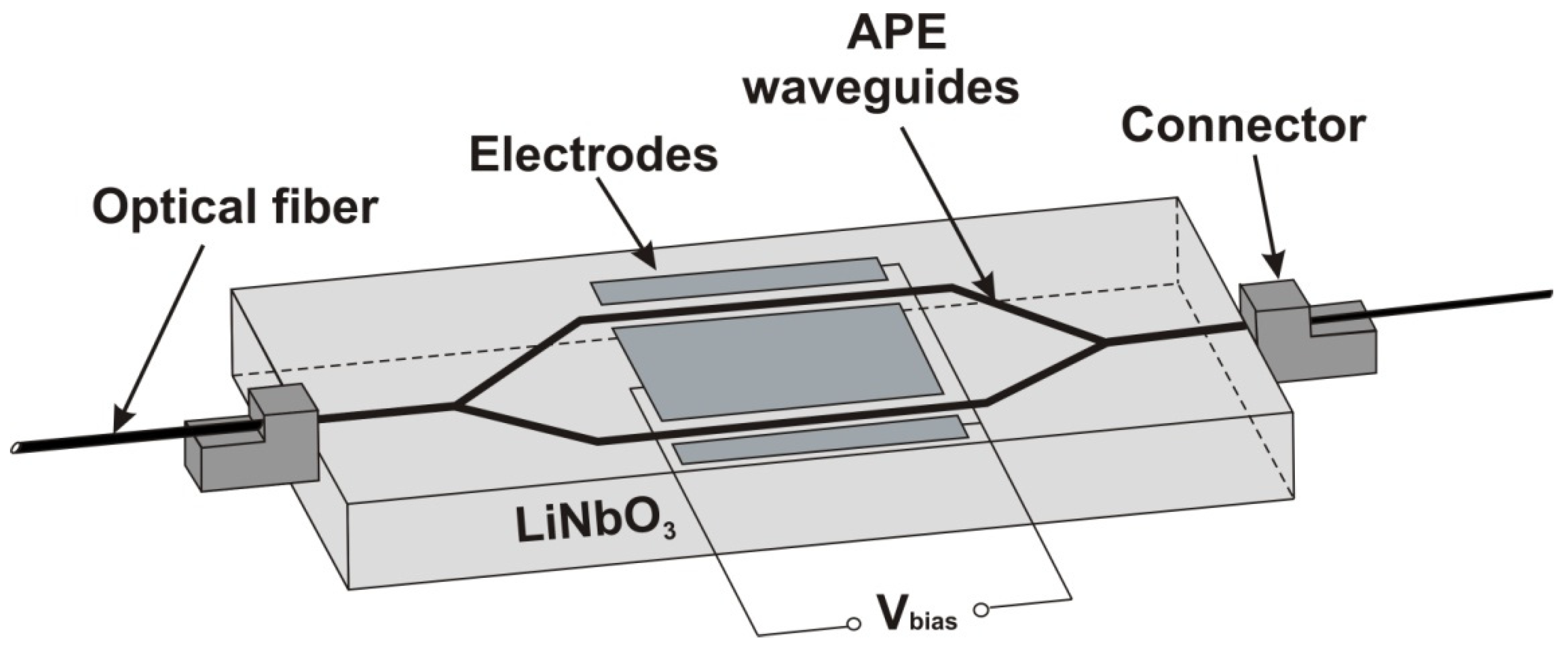
2.1. Sample Preparation
- Maximum crystal heating temperature (350 °C) is significantly lower than the temperature when the crystal composition starts to change (above 600 °C), while pyroelectric effect is characteristic of the crystal Z-cut where Li+ ions are redistributed next to two polar faces. These processes are not characteristic of the LiNbO3 X-cut, because the direction of the normal line to the crystal X-cut is nonpolar. Considering strong connections between oxygen base and Nb+5 ions, it is fair to say that the crystal composition does not change in areas where it is not subject to proton exchange when creating waveguides by described methods;
- Time of photoresist treatment is selected in such a manner that avoids interaction between acid and support structure. As far as the development process is concerned, LiNbO3 is inert to organic developers;
- UV exposure during connection of the chips and fiber can result in the generation of free electrons in LiNbO3 after photovoltaic effect, but their relaxation time is much less than the time between assembly and testing of the samples.
2.2. Optical Losses
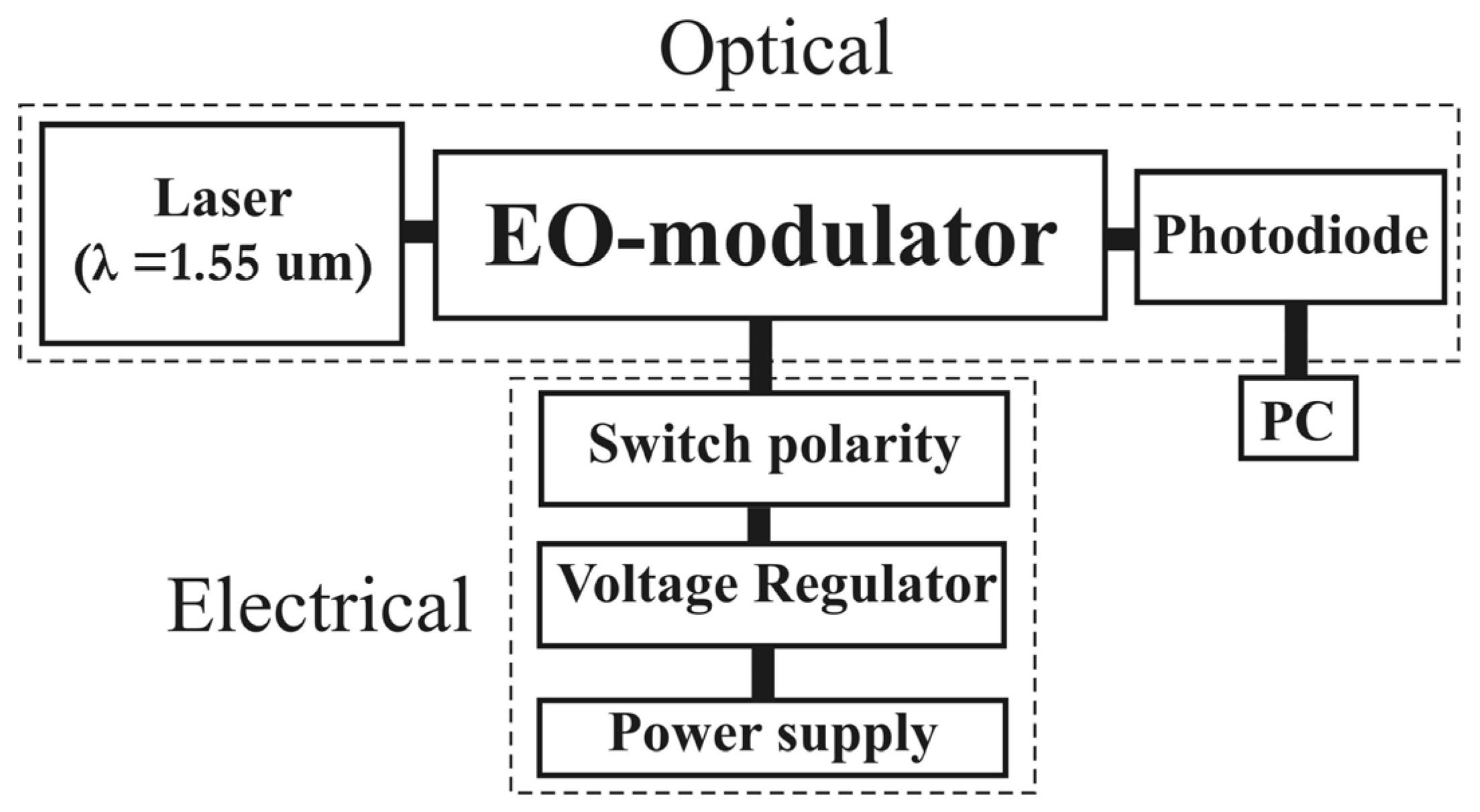
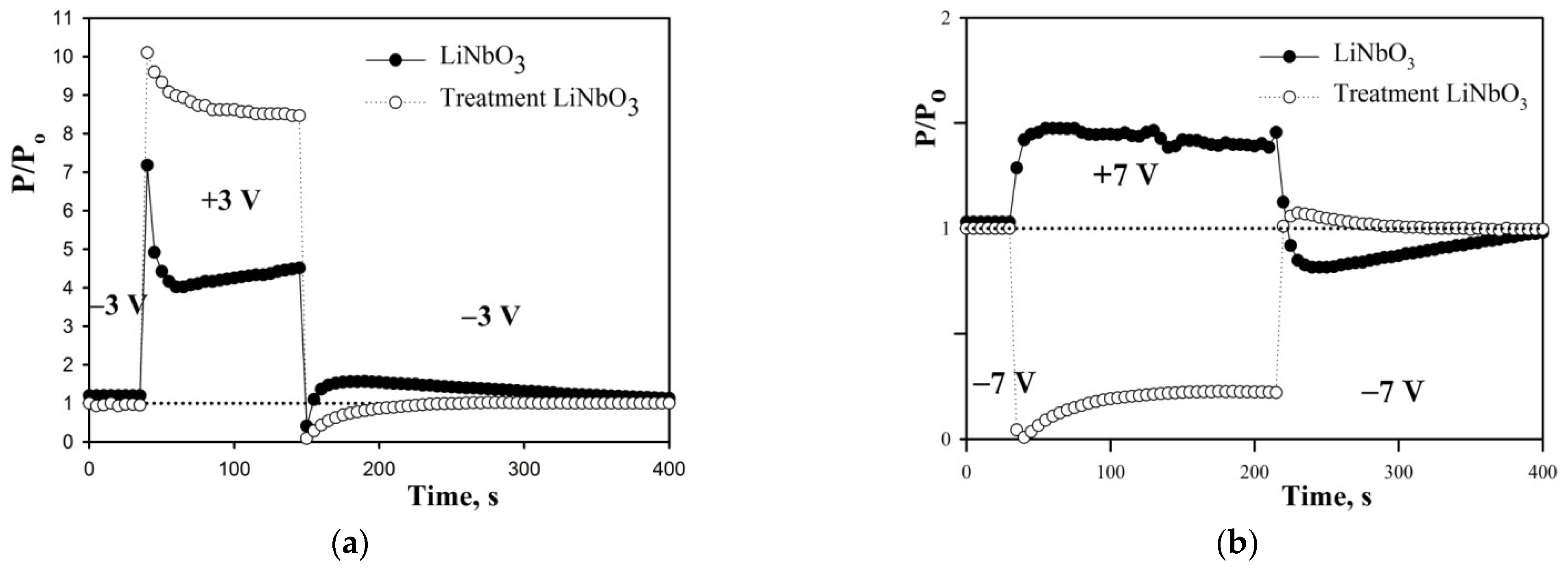
2.3. Drift of Operating Point Depending on Voltage Applied Vbias
2.4. Drift of Operating Point Depending on Temperature
3. Results and Discussion
3.1. Short-Term DC-Drift
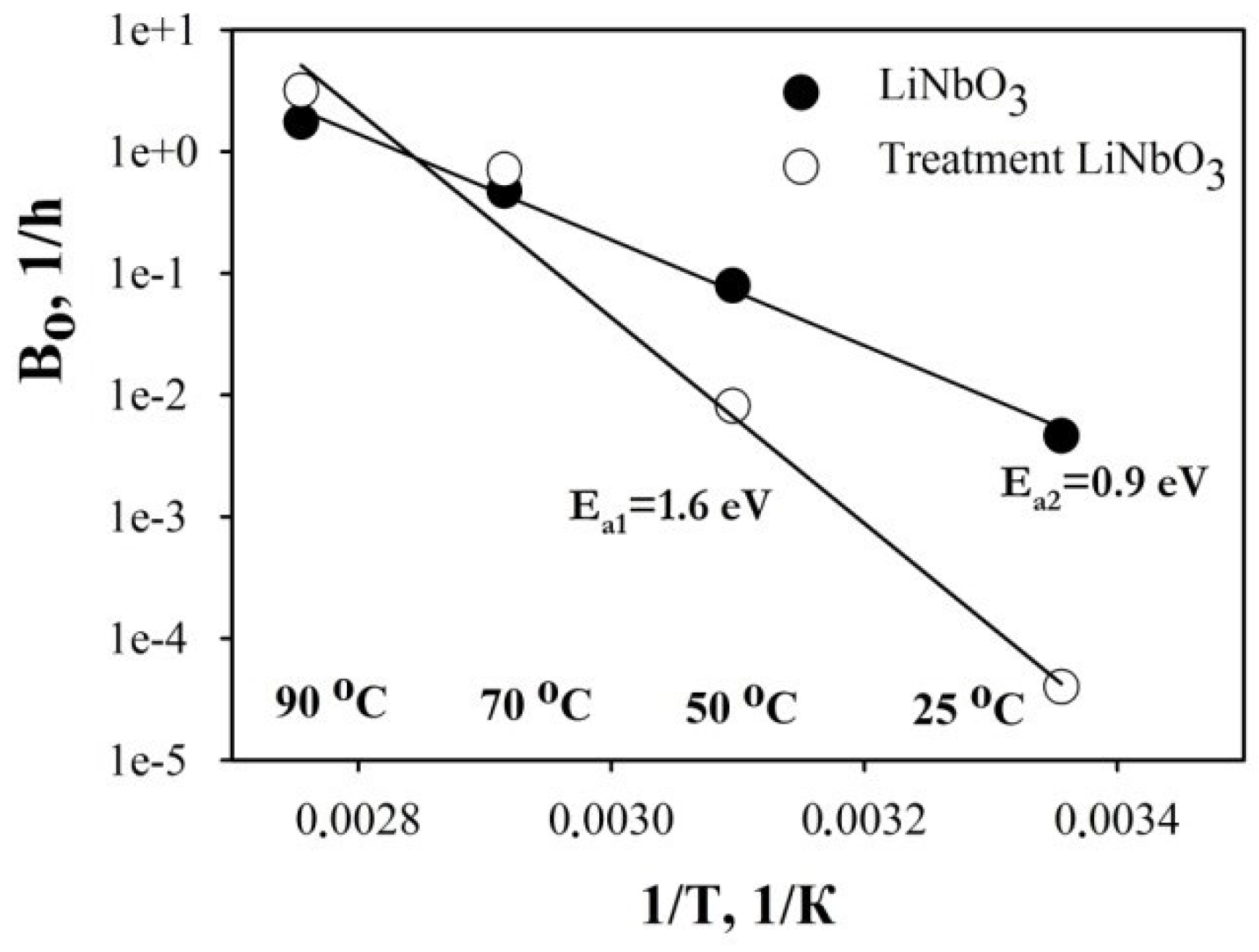
3.2. Temperature Stability of EO-Modulators (Long-Term DC-Drift)
4. Conclusions
- Relaxation time of short-term drift of operating point decreases from 286 to 116 s;
- Activation energy of operating point in EO-modulators showed growth from 0.9 to 1.6 eV.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Noguchi, K. Lithium Niobate Modulators. In Broadband Optical Modulators: Science, Technology, and Applications; Chen, A., Murphy, E.J., Eds.; CRC Press: Boca Raton, FL, USA, 2012; pp. 151–172. [Google Scholar]
- Rao, A.; Fathpour, S. Compact Lithium Niobate Electrooptic Modulators. IEEE J. Sel. Top. Quantum Electron. 2018, 24, 1–14. [Google Scholar] [CrossRef]
- Han, H.; Xiang, B. Integrated electro-optic modulators in x-cut lithium niobate thin film. Optik 2020, 212, 164691. [Google Scholar] [CrossRef]
- Wooten, E.L.; Kissa, K.M.; Yi-Yan, A.; Murphy, E.J.; Lafaw, D.A.; Hallemeier, P.F.; Maack, D.; Attanasio, D.V.; Fritz, D.J.; McBrien, G.J.; et al. Review of lithium niobate modulators for fiber-optic communications systems. IEEE J. Sel. Top. Quantum Electron. 2000, 6, 69–82. [Google Scholar] [CrossRef]
- Nagata, H. Long-term DC drift in x-cut LiNbO3 modulators without oxide buffer layer. IEE Proc.-Optoelectron. 2000, 147, 350–354. [Google Scholar] [CrossRef]
- Nagata, H.; Papasavvas, N. Bias stability of OC48 x-cut lithium-niobate optical modulators: Four years of biased aging test results. IEEE Technol. Lett. 2003, 15, 42–44. [Google Scholar] [CrossRef]
- Hofer, L.R.; Schaeffer, D.B.; Constantin, C.G.; Niemann, C. Bias Voltage Control in Pulsed Applications for Mach-Zehnder Electrooptic Intensity Modulators. IEEE Trans. Control Syst. Technol. 2017, 25, 1890–1895. [Google Scholar] [CrossRef]
- Yuan, X.; Zhang, J.; Zhang, M. Any point bias control technique for MZ modulator. Optik 2019, 178, 918–922. [Google Scholar] [CrossRef]
- Svarny, J. Analysis of quadrature bias-point drift of Mach-Zehnder electro-optic modulator. In Proceedings of the 2010 12th Biennial Baltic Electronics Conference, Tallinn, Estonia, 4–6 October 2010; pp. 231–234. [Google Scholar]
- Cho, P.S.; Khurgin, J.B.; Shpantzer, I. Closed-Loop Bias Control of Optical Quadrature Modulator. IEEE Photonic Technol. Lett. 2006, 18, 2209–2211. [Google Scholar] [CrossRef]
- Wang, L.L.; Kowalcyzk, T.A. Versatile Bias Control Technique for Any-Point Locking in Lithium Niobate Mach-Zehnder Modulators. J. Lightwave Tech. 2010, 28, 1703–1706. [Google Scholar] [CrossRef]
- Toney, J.E.; Stenger, V.E.; Pollick, A.; Sriram, S. Operating Point Correction for Mach Zehnder Interferometer-based Electro-optic E-field Sensors. In Proceedings of the Optical Sensors and Sensing Congress (ES, FTS, HISE, Sensors), San Jose, CA, USA,, 25–27 June 2019; p. SW4C.1. [Google Scholar]
- Bui, D.T.; Nguyen, C.T.; Ledoux-Rak, I.; Zyss, J.; Journet, B. Instrumentation system for determination and compensation of electro-optic modulator transfer function drift. Meas. Sci. Technol. 2011, 22, 125105. [Google Scholar] [CrossRef]
- Fu, Y.; Zhang, X.; Hraimel, B.; Liu, T.; Shen, D. Mach-Zehnder: A Review of Bias Control Techniques for Mach-Zehnder Modulators in Photonic Analog Links. IEEE Microw. Mag. 2013, 14, 102–107. [Google Scholar] [CrossRef]
- Salvestrini, J.P.; Guilbert, L.; Fontana, M.; Abarkan, M.; Gille, S. Analysis and Control of the DC Drift in LiNbO3-Based Mach-Zehnder Modulators. J. Lightwave Technol. 2011, 29, 1522–1534. [Google Scholar] [CrossRef]
- Sosunov, A.V.; Ponomarev, R.S.; Yuriev, V.A.; Volyntsev, A.B. Effect of the structure and mechanical properties of the near-surface layer of lithium niobate single crystals on the manufacture of integrated optic circuits. Optoelectron. Instrum. Data Process. 2017, 53, 82–87. [Google Scholar] [CrossRef]
- Piecha, J.; Molak, A.; Breuer, U.; Balski, M.; Szot, K. Features of surface layer of LiNbO3 as-received single crystals: Studied in situ on treatment samples modified by elevated temperature. Solid State Ion. 2016, 290, 31–39. [Google Scholar] [CrossRef]
- Sosunov, A.; Ponomarev, R.; Semenova, O.; Petukhov, I.; Volyntsev, A. Effect of pre-annealing of lithium niobate on the structure and optical characteristics of proton-exchanged waveguides. Opt. Mater. 2019, 88, 176–180. [Google Scholar] [CrossRef]
- Suchoski, P.G.; Findakly, T.K.; Leonberger, F.J. Stable low-loss proton-exchanged LiNbO3 waveguide devices with no electro-optic degradation. Opt. Lett. 1988, 13, 1050–1052. [Google Scholar] [CrossRef] [PubMed]
- Nagata, H. Activation Energy of DC-Drift of X-Cut LiNbO3. IEEE Photonic Technol. Lett. 2000, 12, 386–388. [Google Scholar] [CrossRef]
- Nagata, H.; Ishizuka, Y.; Akizuki, K. Temperature dependency of X-cut LiNbO3 modulator dc drift. Electron. Lett. 2000, 36, 1952–1953. [Google Scholar] [CrossRef]
- Nagata, H.; Li, Y.; Croston, I.; Maack, D.R.; Appleyard, A. Dc drift activation energy of LiNbO3 optical modulators based on thousands of hours of active accelerated aging tests. IEEE Photon. Technol. Lett. 2002, 14, 1076–1078. [Google Scholar] [CrossRef]


| Characteristic | APE |
|---|---|
| Waveguide loss, dB/cm | 0.13–0.17 |
| Average fiber-to-fiber loss, dB | 6.5 |
| Half-wave voltage, V | 3.5–6 |
| Sample | Temperature, °C | Coefficient, A0 | Coefficient, n | Coefficient, B0 [1/h] |
|---|---|---|---|---|
| LiNbO3 | 25 | 0.984 | 0.003 | 0.0046 |
| 50 | 0.842 | 0.068 | 0.079 | |
| 70 | 0.909 | 0.128 | 0 | |
| 90 | 1.112 | 0.191 | 1.745 | |
| Treatment LiNbO3 | 25 | 0.981 | 0.002 | 0.00004 |
| 50 | 0.783 | 0.051 | 0 | |
| 70 | 0.992 | 0.024 | 1 | |
| 90 | 1.05 | 0.043 | 3 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sosunov, A.; Ponomarev, R.; Zhuravlev, A.; Mushinsky, S.; Kuneva, M. Reduction in DC-Drift in LiNbO3-Based Electro-Optical Modulator. Photonics 2021, 8, 571. https://doi.org/10.3390/photonics8120571
Sosunov A, Ponomarev R, Zhuravlev A, Mushinsky S, Kuneva M. Reduction in DC-Drift in LiNbO3-Based Electro-Optical Modulator. Photonics. 2021; 8(12):571. https://doi.org/10.3390/photonics8120571
Chicago/Turabian StyleSosunov, Aleksei, Roman Ponomarev, Anton Zhuravlev, Sergey Mushinsky, and Mariana Kuneva. 2021. "Reduction in DC-Drift in LiNbO3-Based Electro-Optical Modulator" Photonics 8, no. 12: 571. https://doi.org/10.3390/photonics8120571
APA StyleSosunov, A., Ponomarev, R., Zhuravlev, A., Mushinsky, S., & Kuneva, M. (2021). Reduction in DC-Drift in LiNbO3-Based Electro-Optical Modulator. Photonics, 8(12), 571. https://doi.org/10.3390/photonics8120571




