Inter-Cavity Coupling Strength Control in Metal/Insulator Multilayers for Hydrogen Sensing
Abstract
:1. Introduction
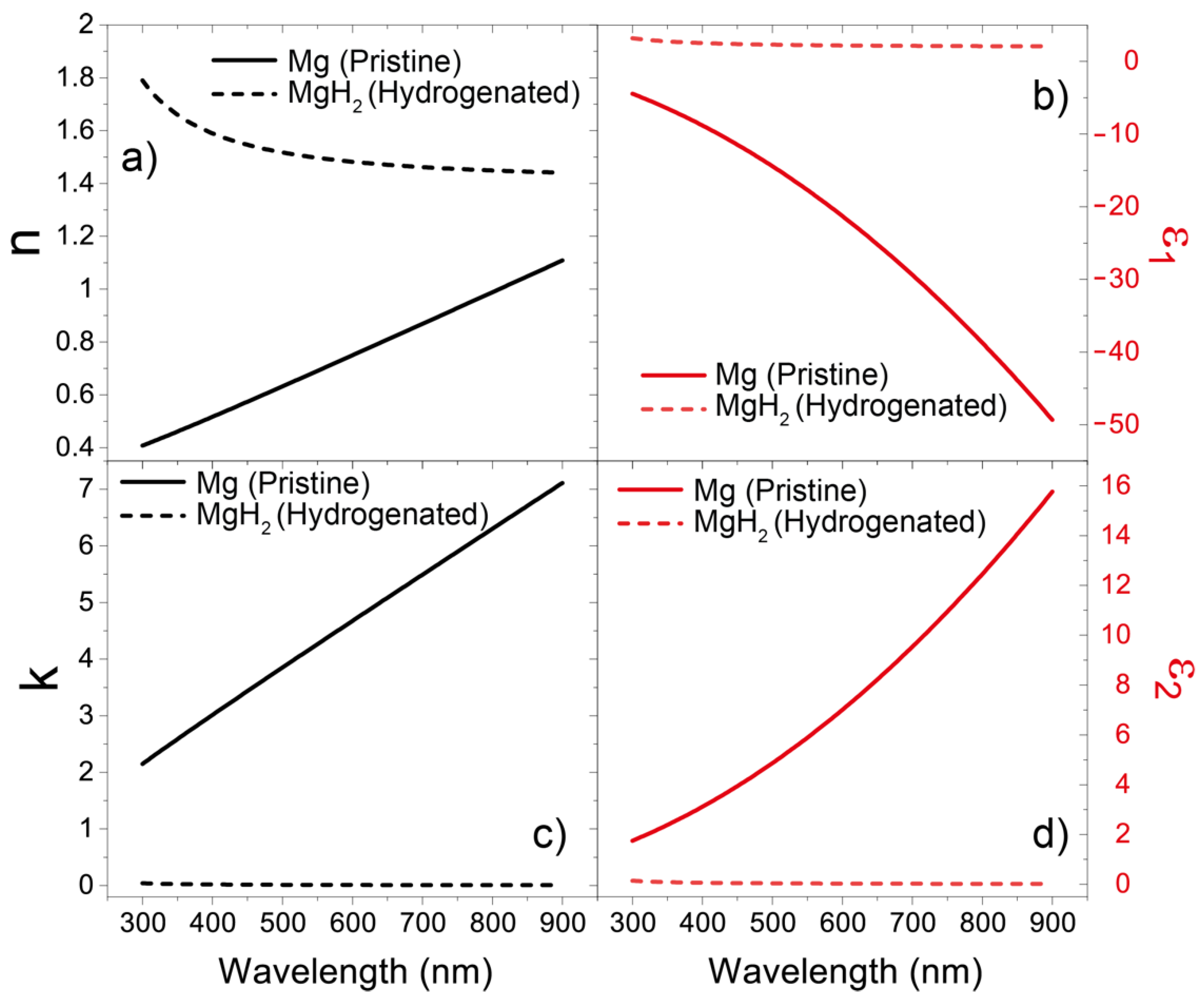
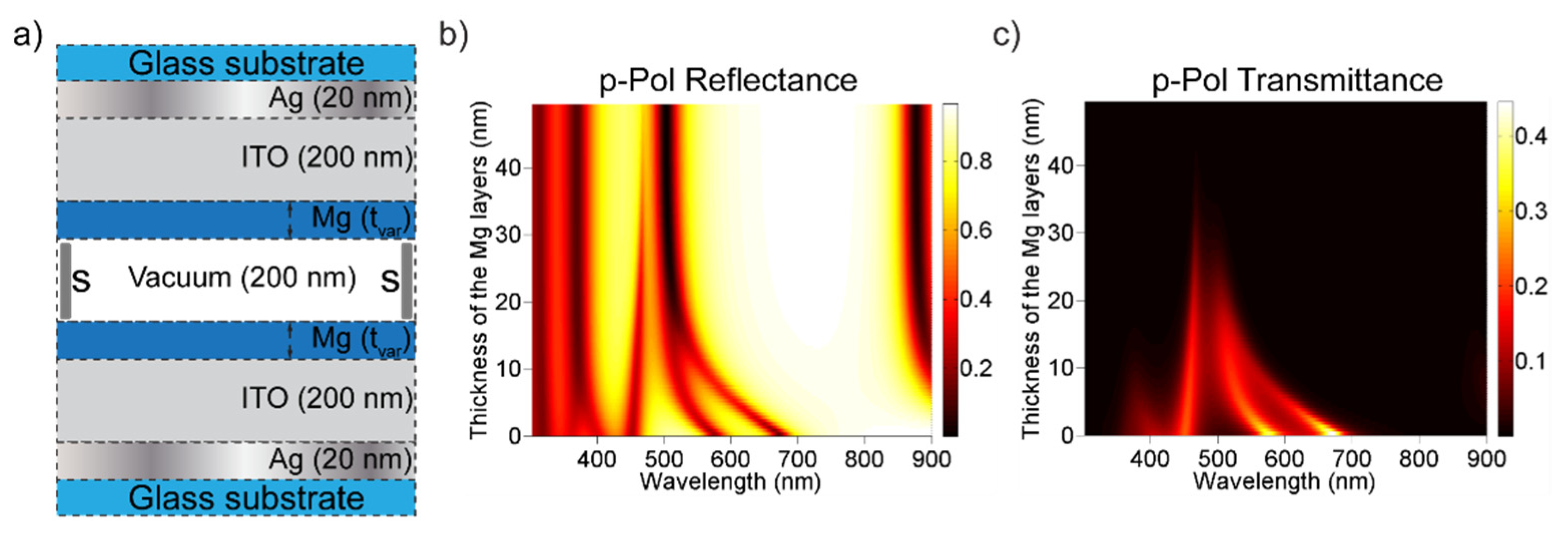
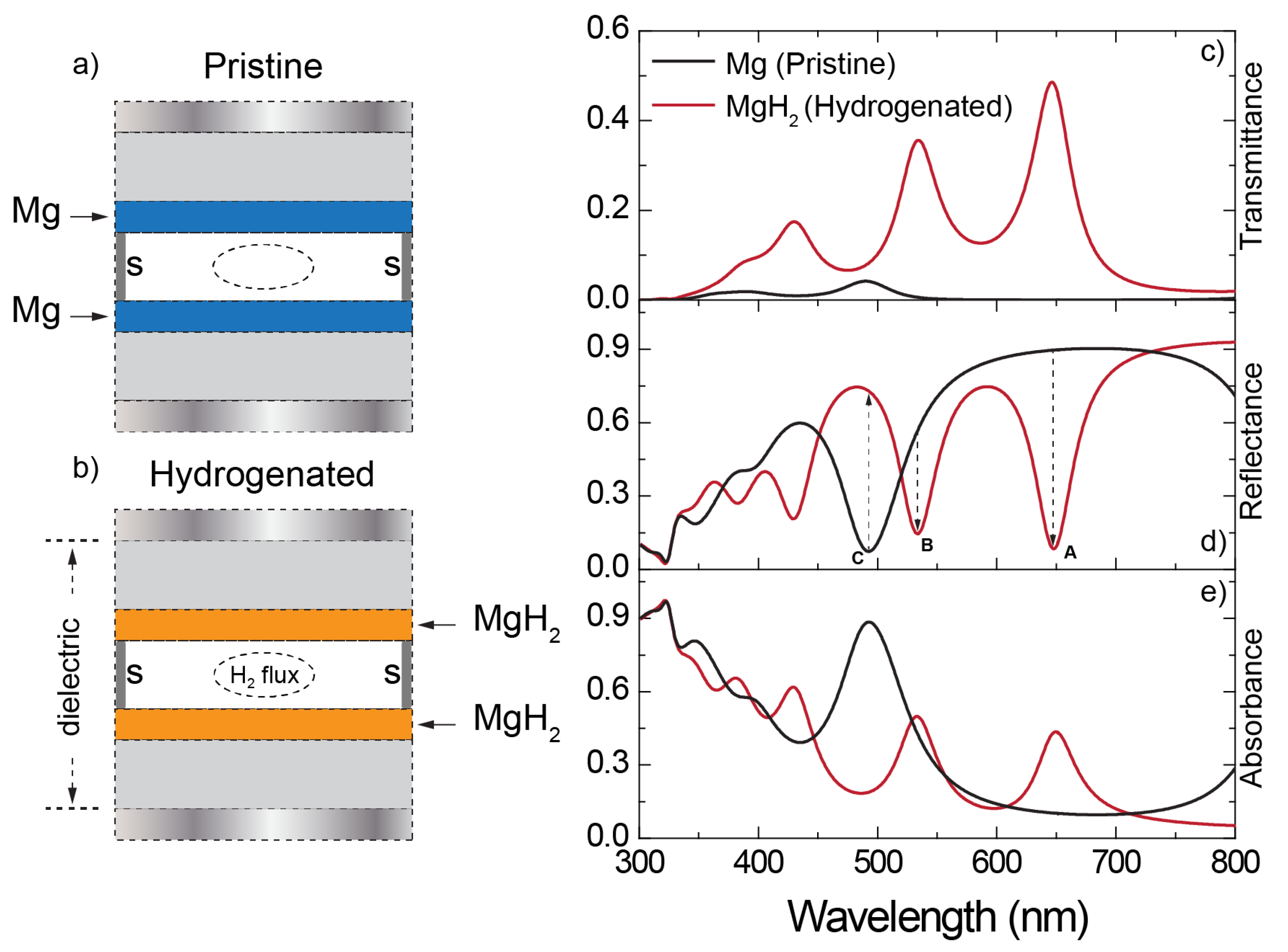
2. Results and Discussion
3. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Öztürk, S.; Kılınç, N. Pd Thin Films on Flexible Substrate for Hydrogen Sensor. J. Alloys Compd. 2016, 674, 179–184. [Google Scholar] [CrossRef]
- Kilinç, N. Resistive Hydrogen Sensors Based on Nanostructured Metals and Metal Alloys. Nanosci. Nanotechnol. Lett. 2013, 5, 825–841. [Google Scholar] [CrossRef]
- Hao, M.; Wu, S.; Zhou, H.; Ye, W.; Wei, X.; Wang, X.; Chen, Z.; Li, S. Room-Temperature and Fast Response Hydrogen Sensor Based on Annealed Nanoporous Palladium Film. J. Mater. Sci. 2016, 51, 2420–2426. [Google Scholar] [CrossRef]
- Arya, S.K.; Krishnan, S.; Silva, H.; Jean, S.; Bhansali, S. Advances in Materials for Room Temperature Hydrogen Sensors. Analyst 2012, 137, 2743–2756. [Google Scholar] [CrossRef]
- Thomas, R.C.; Hughes, R.C. Sensors for Detecting Molecular Hydrogen Based on Pd Metal Alloys. J. Electrochem. Soc. 1997, 144, 3245–3249. [Google Scholar] [CrossRef]
- Noh, J.S.; Lee, J.M.; Lee, W. Low-Dimensional Palladium Nanostructures for Fast and Reliable Hydrogen Gas Detection. Sensors 2011, 11, 825–851. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Peng, Y.; Zheng, L.; Zou, K.; Li, C. Enhancing Performances of a Resistivity-Type Hydrogen Sensor Based on Pd/SnO2/RGO Nanocomposites. Nanotechnology 2017, 28, 215501. [Google Scholar] [CrossRef]
- van Lith, J.; Lassesson, A.; Brown, S.A.; Schulze, M.; Partridge, J.G.; Ayesh, A. A Hydrogen Sensor Based on Tunneling between Palladium Clusters. Appl. Phys. Lett. 2007, 91, 181910. [Google Scholar] [CrossRef]
- Ellis, D.L.; Zakin, M.R.; Bernstein, L.S.; Rubner, M.F. Conductive Polymer Films as Ultrasensitive Chemical Sensors for Hydrazine and Monomethylhydrazine Vapor. Anal. Chem. 1996, 68, 817–822. [Google Scholar] [CrossRef]
- Huang, J.; Virji, S.; Weiller, B.H.; Kaner, R.B. Nanostructured Polyaniline Sensors. Chem.—A Eur. J. 2004, 10, 1314–1319. [Google Scholar] [CrossRef]
- Virji, S.; Kaner, R.B.; Weiller, B.H. Hydrogen Sensors Based on Conductivity Changes in Polyaniline Nanofibers. J. Phys. Chem. B 2006, 110, 22266–22270. [Google Scholar] [CrossRef] [PubMed]
- Herkert, E.; Sterl, F.; Strohfeldt, N.; Walter, R.; Giessen, H. Low-Cost Hydrogen Sensor in the Ppm Range with Purely Optical Readout. ACS Sens. 2020, 5, 978–983. [Google Scholar] [CrossRef] [PubMed]
- Mulder, D.J.; Schenning, A.P.H.J.; Bastiaansen, C.W.M. Chiral-Nematic Liquid Crystals as One Dimensional Photonic Materials in Optical Sensors. J. Mater. Chem. C 2014, 2, 6695–6705. [Google Scholar] [CrossRef] [Green Version]
- Esteves, C.; Ramou, E.; Porteira, A.R.P.; Barbosa, A.J.M.; Roque, A.C.A. Seeing the Unseen: The Role of Liquid Crystals in Gas-Sensing Technologies. Adv. Opt. Mater. 2020, 8, 1902117. [Google Scholar] [CrossRef]
- Carlton, R.J.; Hunter, J.T.; Miller, D.S.; Abbasi, R.; Mushenheim, P.C.; Tan, L.N.; Abbott, N.L. Chemical and Biological Sensing Using Liquid Crystals. Liq. Cryst. Rev. 2013, 1, 29. [Google Scholar] [CrossRef]
- De Sio, L.; Caligiuri, V.; Umeton, C. Tuneable Broadband Optical Filter Based on Soft-Composite Materials. J. Opt. 2014, 16, 065703. [Google Scholar] [CrossRef]
- Seidel, J.; Grafström, S.; Eng, L. Stimulated Emission of Surface Plasmons at the Interface between a Silver Film and an Optically Pumped Dye Solution. Phys. Rev. Lett. 2005, 94, 177401. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zaky, Z.A.; Ahmed, A.M.; Shalaby, A.S.; Aly, A.H. Refractive Index Gas Sensor Based on the Tamm State in a One-Dimensional Photonic Crystal: Theoretical Optimisation. Sci. Rep. 2020, 10, 9736. [Google Scholar] [CrossRef]
- Giessen, H.; Mai, P.; Tittl, A.; Liu, N.; Grossmann, C.; Seidel, A.; Orzekowsky, R.; Meyrath, T. Optical Hydrogen Sensing with Metallic Photonic Crystals and Plasmonic Metamaterials. In Proceedings of the IEEE Sensors, Waikoloa, HI, USA, 1–4 November 2010; pp. 2177–2178. [Google Scholar] [CrossRef]
- Goyal, A.K.; Dutta, H.S.; Pal, S. Recent Advances and Progress in Photonic Crystal-Based Gas Sensors. J. Phys. D Appl. Phys. 2017, 50, 203001. [Google Scholar] [CrossRef]
- Afsari, A.; Sarraf, M.J. Design of a Hydrogen Sulfide Gas Sensor Based on a Photonic Crystal Cavity Using Graphene. Superlattices Microstruct. 2020, 138, 106362. [Google Scholar] [CrossRef]
- Wadell, C.; Syrenova, S.; Langhammer, C. Plasmonic Hydrogen Sensing with Nanostructured Metal Hydrides. ACS Nano 2014, 8, 11925–11940. [Google Scholar] [CrossRef]
- Sil, D.; Gilroy, K.D.; Niaux, A.; Boulesbaa, A.; Neretina, S.; Borguet, E. Seeing Is Believing: Hot Electron Based Gold Nanoplasmonic Optical Hydrogen Sensor. ACS Nano 2014, 8, 7755–7762. [Google Scholar] [CrossRef]
- Langhammer, C.; Zorić, I.; Kasemo, B.; Clemens, B.M. Hydrogen Storage in Pd Nanodisks Characterized with a Novel Nanoplasmonic Sensing Scheme. Nano Lett. 2007, 7, 3122–3127. [Google Scholar] [CrossRef] [PubMed]
- Langhammer, C.; Larsson, E.M.; Zhdanov, V.P.; Zorić, I. Asymmetric Hysteresis in Nanoscopic Single-Metal Hydrides: Palladium Nanorings. J. Phys. Chem. C 2012, 116, 21201–21207. [Google Scholar] [CrossRef]
- Poyli, M.A.; Silkin, V.M.; Chernov, I.P.; Echenique, P.M.; Muiño, R.D.; Aizpurua, J. Multiscale Theoretical Modeling of Plasmonic Sensing of Hydrogen Uptake in Palladium Nanodisks. J. Phys. Chem. Lett. 2012, 3, 2556–2561. [Google Scholar] [CrossRef] [PubMed]
- Policicchio, A.; Conte, G.; Stelitano, S.; Bonaventura, C.P.; Putz, A.-M.; Ianăşi, C.; Almásy, L.; Horváth, Z.E.; Agostino, R.G. Hydrogen Storage Performances for Mesoporous Silica Synthesized with Mixed Tetraethoxysilane and Methyltriethoxysilane Precursors in Acidic Condition. Colloids Surf. A Physicochem. Eng. Asp. 2020, 601, 125040. [Google Scholar] [CrossRef]
- Pedicini, R.; Maisano, S.; Chiodo, V.; Conte, G.; Policicchio, A.; Agostino, R.G. Posidonia Oceanica and Wood Chips Activated Carbon as Interesting Materials for Hydrogen Storage. Int. J. Hydrogen Energy 2020, 45, 14038–14047. [Google Scholar] [CrossRef]
- Policicchio, A.; Putz, A.-M.; Conte, G.; Stelitano, S.; Bonaventura, C.P.; Ianăşi, C.; Almásy, L.; Wacha, A.; Horváth, Z.E.; Agostino, R.G. Hydrogen Storage Performance of Methyl-Substituted Mesoporous Silica with Tailored Textural Characteristics. J. Porous Mater. 2021, 28, 1049–1058. [Google Scholar] [CrossRef]
- Sugawa, K.; Tahara, H.; Yamashita, A.; Otsuki, J.; Sagara, T.; Harumoto, T.; Yanagida, S. Refractive Index Susceptibility of the Plasmonic Palladium Nanoparticle: Potential as the Third Plasmonic Sensing Material. ACS Nano 2015, 9, 1895–1904. [Google Scholar] [CrossRef]
- Langhammer, C.; Yuan, Z.; Zorić, I.; Kasemo, B. Plasmonic Properties of Supported Pt and Pd Nanostructures. Nano Lett. 2006, 6, 833–838. [Google Scholar] [CrossRef]
- West, P.R.; Ishii, S.; Naik, G.V.; Emani, N.K.; Shalaev, V.M.; Boltasseva, A. Searching for Better Plasmonic Materials. Laser Photonics Rev. 2010, 4, 795–808. [Google Scholar] [CrossRef] [Green Version]
- Marchi, S.D.; Núñez-Sánchez, S.; Bodelón, G.; Pérez-Juste, J.; Pastoriza-Santos, I. Pd Nanoparticles as a Plasmonic Material: Synthesis, Optical Properties and Applications. Nanoscale 2020, 12, 23424–23443. [Google Scholar] [CrossRef] [PubMed]
- Huang, X.; Tang, S.; Mu, X.; Dai, Y.; Chen, G.; Zhou, Z.; Ruan, F.; Yang, Z.; Zheng, N. Freestanding Palladium Nanosheets with Plasmonic and Catalytic Properties. Nat. Nanotechnol. 2011, 6, 28–32. [Google Scholar] [CrossRef]
- Poddubny, A.; Iorsh, I.; Belov, P.; Kivshar, Y. Hyperbolic Metamaterials. Nat. Photonics 2013, 7, 958–968. [Google Scholar] [CrossRef]
- Vasilantonakis, N.; Wurtz, G.A.; Podolskiy, V.A.; Zayats, A.V. Refractive Index Sensing with Hyperbolic Metamaterials: Strategies for Biosensing and Nonlinearity Enhancement. Opt. Express 2015, 23, 14329–14343. [Google Scholar] [CrossRef] [PubMed]
- Ferrari, L.; Wu, C.; Lepage, D.; Zhang, X.; Liu, Z. Hyperbolic Metamaterials and Their Applications. Prog. Quantum Electron. 2015, 40, 1–40. [Google Scholar] [CrossRef]
- Caligiuri, V.; Pezzi, L.; Veltri, A.; De Luca, A. Resonant Gain Singularities in 1D and 3D Metal-Dielectric Multilayered Nanostructures. ACS Nano 2017, 11, 1012–1025. [Google Scholar] [CrossRef]
- Hierro-Rodriguez, A.; Leite, I.T.; Rocha-Rodrigues, P.; Fernandes, P.; Araujo, J.P.; Jorge, P.A.S.; Santos, J.L.; Teixeira, J.M.; Guerreiro, A. Hydrogen Sensing via Anomalous Optical Absorption of Palladium-Based Metamaterials. Nanotechnology 2016, 27, 185501. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Caligiuri, V.; Palei, M.; Biffi, G.; Artyukhin, S.; Krahne, R. A Semi-Classical View on Epsilon-Near-Zero Resonant Tunneling Modes in Metal/Insulator/Metal Nanocavities. Nano Lett. 2019, 19, 3151–3160. [Google Scholar] [CrossRef]
- Isidorsson, J.; Giebels, I.A.M.E.; Arwin, H.; Griessen, R. Optical Properties of MgH2 Measured in Situ by Ellipsometry and Spectrophotometry. Phys. Rev. B 2003, 68, 115112. [Google Scholar] [CrossRef] [Green Version]
- Caligiuri, V.; Dhama, R.; Sreekanth, K.V.; Strangi, G.; De Luca, A. Dielectric Singularity in Hyperbolic Metamaterials: The Inversion Point of Coexisting Anisotropies. Sci. Rep. 2016, 6, 20002. [Google Scholar] [CrossRef]
- Caligiuri, V.; Palei, M.; Biffi, G.; Krahne, R. Hybridization of Epsilon-near-Zero Modes via Resonant Tunneling in Layered Metal-Insulator Double Nanocavities. Nanophotonics 2019, 8, 1–8. [Google Scholar] [CrossRef]
- Caligiuri, V.; Biffi, G.; Patra, A.; Pothuraju, R.D.; Luca, A.D.; Krahne, R. One-Dimensional Epsilon-Near-Zero Crystals. Adv. Photonics Res. 2021, 2, 2100053. [Google Scholar] [CrossRef]
- Caligiuri, V.; Pianelli, A.; Miscuglio, M.; Patra, A.; MacCaferri, N.; Caputo, R.; De Luca, A. Near- And Mid-Infrared Graphene-Based Photonic Architectures for Ultrafast and Low-Power Electro-Optical Switching and Ultra-High Resolution Imaging. ACS Appl. Nano Mater. 2020, 3, 12218–12230. [Google Scholar] [CrossRef]
- Kuttruff, J.; Garoli, D.; Allerbeck, J.; Krahne, R.; De Luca, A.; Brida, D.; Caligiuri, V.; Maccaferri, N. Ultrafast All-Optical Switching Enabled by Epsilon-near-Zero-Tailored Absorption in Metal-Insulator Nanocavities. Commun. Phys. 2020, 3, 1–7. [Google Scholar] [CrossRef]
- Gaponenko, S.V. Introduction to Nanophotonics; Cambridge University Press: Cambridge, UK, 2010; p. 465. ISBN 978-0-511-75050-2. [Google Scholar]
- Caligiuri, V.; Palei, M.; Imran, M.; Manna, L.; Krahne, R. Planar Double-Epsilon-Near-Zero Cavities for Spontaneous Emission and Purcell Effect Enhancement. ACS Photonics 2018, 5, 2287–2294. [Google Scholar] [CrossRef]


| (j) | |||
|---|---|---|---|
| (1) | 6.4 | 5.516 | 0.6454 |
| (2) | 6.9 | 7.689 ± 0.519 | 0.01223 ± 0.109 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Caligiuri, V.; De Luca, A. Inter-Cavity Coupling Strength Control in Metal/Insulator Multilayers for Hydrogen Sensing. Photonics 2021, 8, 537. https://doi.org/10.3390/photonics8120537
Caligiuri V, De Luca A. Inter-Cavity Coupling Strength Control in Metal/Insulator Multilayers for Hydrogen Sensing. Photonics. 2021; 8(12):537. https://doi.org/10.3390/photonics8120537
Chicago/Turabian StyleCaligiuri, Vincenzo, and Antonio De Luca. 2021. "Inter-Cavity Coupling Strength Control in Metal/Insulator Multilayers for Hydrogen Sensing" Photonics 8, no. 12: 537. https://doi.org/10.3390/photonics8120537
APA StyleCaligiuri, V., & De Luca, A. (2021). Inter-Cavity Coupling Strength Control in Metal/Insulator Multilayers for Hydrogen Sensing. Photonics, 8(12), 537. https://doi.org/10.3390/photonics8120537





