Design and Optimization of 1.55 μm AlGaInAs MQW Polarization Mode Controllers
Abstract
:1. Introduction
2. Design and Optimization
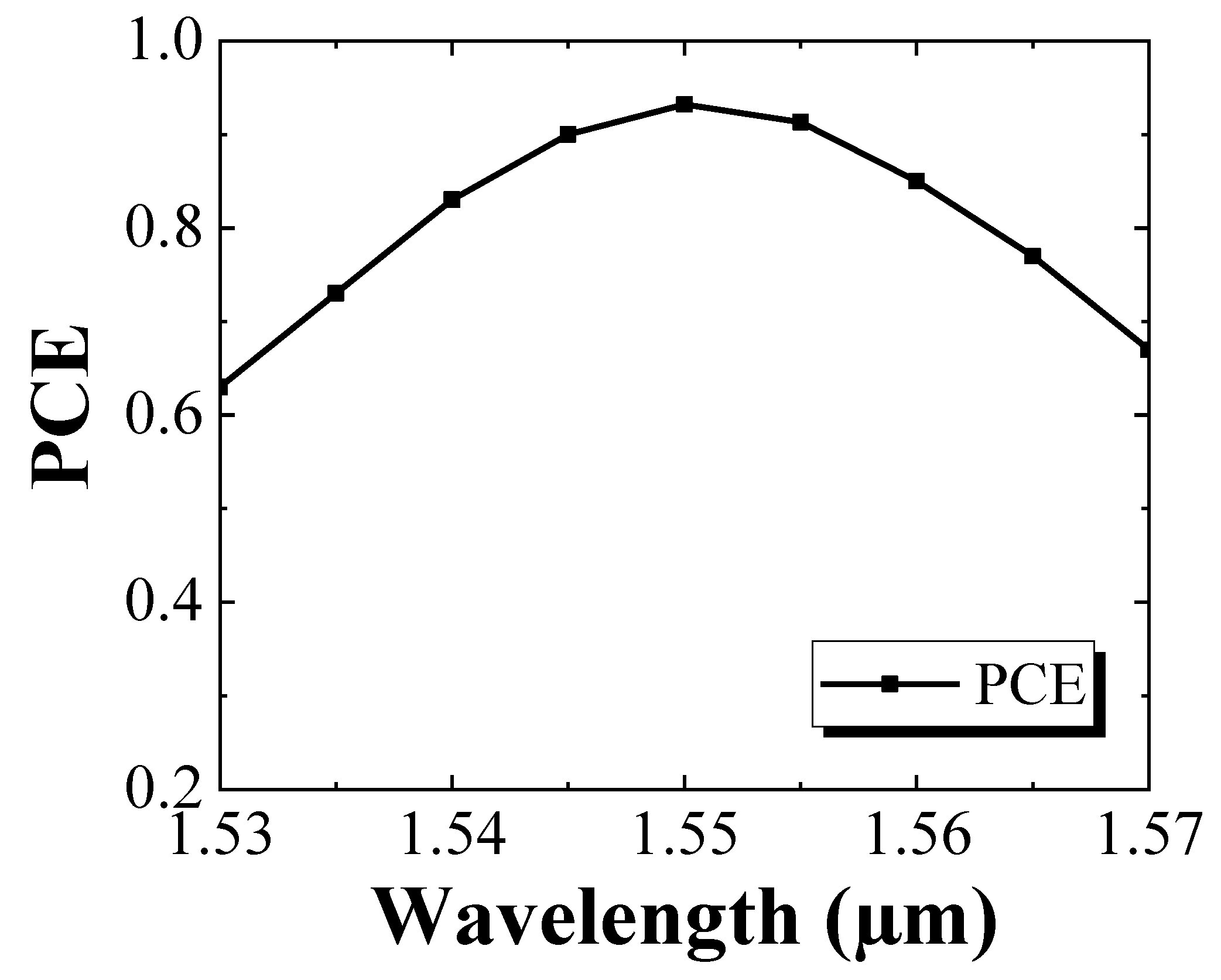
3. Simulation and Results
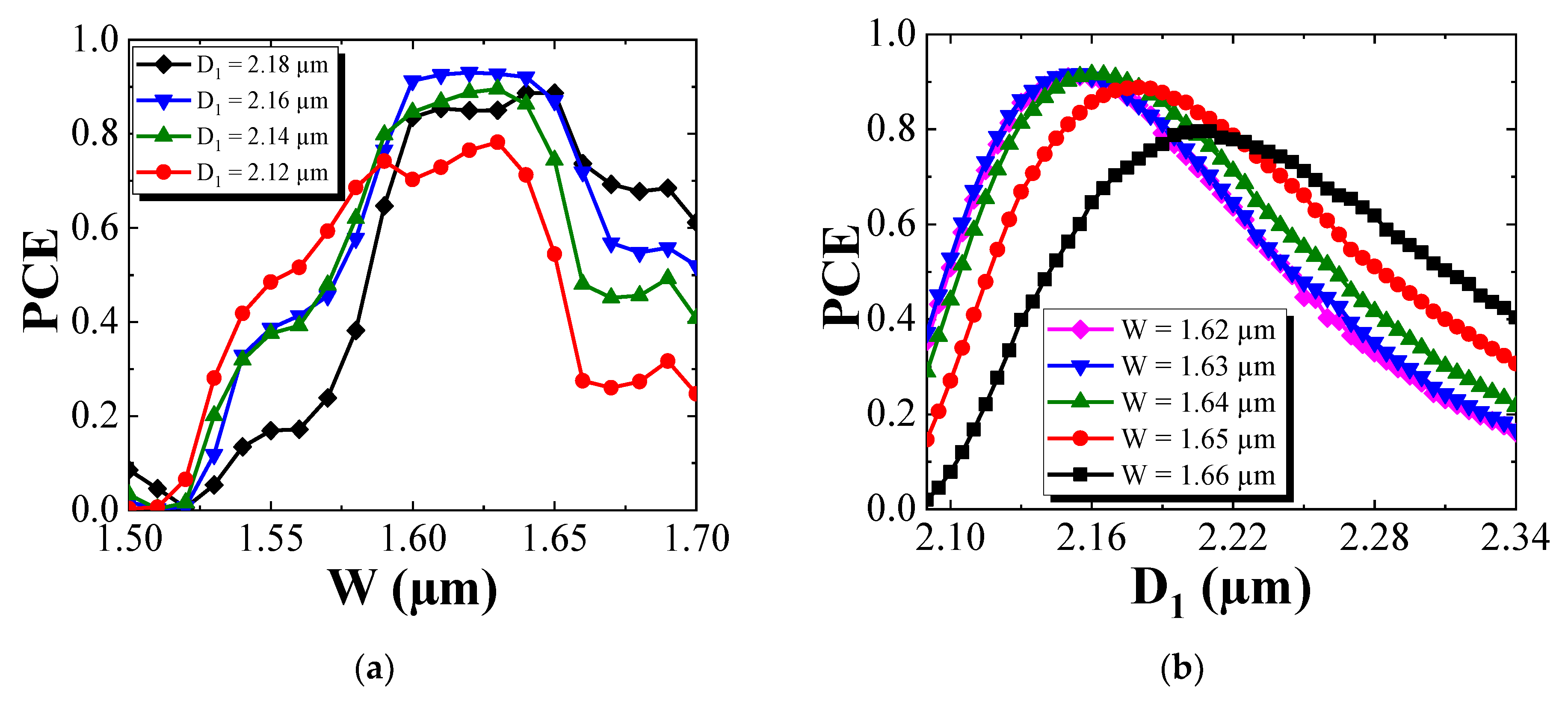
4. Tolerance Analysis
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Morsy-Osman, M.; Sowailem, M.; El-Fiky, E.; Goodwill, T.; Hoang, T.; Lessard, S.; Plant, D.V. DSP-free ‘coherent-lite’transceiver for next generation single wavelength optical intra-datacenter interconnects. Opt. Express 2018, 26, 8890–8903. [Google Scholar] [CrossRef] [PubMed]
- Bunandar, D.; Lentine, A.; Lee, C.; Cai, H.; Long, C.M.; Boynton, N.; Martinez, N.; DeRose, C.; Chen, C.; Grein, M. Metropolitan quantum key distribution with silicon photonics. Phys. Rev. X 2018, 8, 021009. [Google Scholar] [CrossRef] [Green Version]
- Elfiqi, A.E.; Kobayashi, R.; Tanomura, R.; Tanemura, T.; Nakano, Y. Fabrication-tolerant half-ridge InP/InGaAsP polarization rotator with etching-stop layer. IEEE Photonics Technol. Lett. 2020, 32, 663–666. [Google Scholar] [CrossRef]
- Holmes, B.; Hutchings, D. Realization of novel low-loss monolithically integrated passive waveguide mode converters. IEEE Photonics Technol. Lett. 2005, 18, 43–45. [Google Scholar] [CrossRef]
- Xie, S.; Andersson, S.M.; Ye, S.; Niu, Z.; Marsh, J.H.; Hou, L. Design of 2 μm Wavelength Polarization Mode Controllers. In Proceedings of the 2020 International Conference on UK-China Emerging Technologies (UCET), Glasgow, UK, 20–21 August 2020; pp. 1–3. [Google Scholar]
- Ito, M.; Okawa, K.; Suganuma, T.; Fukui, T.; Kato, E.; Tanemura, T.; Nakano, Y. Efficient InGaAsP MQW-based polarization controller without active-passive integration. Opt. Express 2021, 29, 10538–10545. [Google Scholar] [CrossRef] [PubMed]
- El-Refaei, H.; Yevick, D. An optimized InGaAsP/InP polarization converter employing asymmetric rib waveguides. J. Lightwave Technol. 2003, 21, 1544–1548. [Google Scholar] [CrossRef]
- Huan, Z.; Scarmozzino, R.; Nagy, G.; Steel, J.; Osgood, R. Realization of a compact and single-mode optical passive polarization converter. IEEE Photonics Technol. Lett. 2000, 12, 317–319. [Google Scholar] [CrossRef]
- Hou, L.; Haji, M.; Dylewicz, R.; Qiu, B.; Bryce, A.C. Monolithic 45-GHz mode-locked surface-etched DBR laser using quantum-well intermixing technology. IEEE Photonics Technol. Lett. 2010, 22, 1039–1041. [Google Scholar] [CrossRef]
- Suzuki, Y.; Iwamura, H.; Miyazawa, T.; Wakatsuki, A.; Mikami, O. Polarization-dependent refractive-index change induced by superlattice disordering. IEEE J. Quantum Electron. 1996, 32, 1922–1931. [Google Scholar] [CrossRef]
- Skogen, E.J.; Raring, J.W.; Barton, J.S.; DenBaars, S.P.; Coldren, L.A. Postgrowth control of the quantum-well band edge for the monolithic integration of widely tunable lasers and electroabsorption modulators. IEEE J. Sel. Top. Quantum Electron. 2003, 9, 1183–1190. [Google Scholar] [CrossRef]
- Walker, R. Simple and accurate loss measurement technique for semiconductor optical waveguides. Electron. Lett. 1985, 21, 581–583. [Google Scholar] [CrossRef]
- Hou, L.; Haji, M.; Akbar, J.; Marsh, J.H.; Bryce, A.C. AlGaInAs/InP monolithically integrated DFB laser array. IEEE J. Quantum Electron. 2011, 48, 137–143. [Google Scholar] [CrossRef]
- Hou, L.; Stolarz, P.; Javaloyes, J.; Green, R.P.; Ironside, C.N.; Sorel, M.; Bryce, A.C. Subpicosecond Pulse Generation at Quasi-40-GHz Using a Passively Mode-Locked AlGaInAs–InP 1.55-μm Strained Quantum-Well Laser. IEEE Photonics Technol. Lett. 2009, 21, 1731–1733. [Google Scholar]
- Zaitsu, M.; Tanemura, T.; Nakano, Y. Numerical study on fabrication tolerance of half-ridge InP polarization converters. IEICE Trans. Electron. 2014, 97, 731–735. [Google Scholar] [CrossRef] [Green Version]
- Hutchings, D.C.; Holmes, B.M. A waveguide polarization toolset design based on mode beating. IEEE Photonics J. 2011, 3, 450–461. [Google Scholar] [CrossRef]
- El-Refaei, H.; Yevick, D.; Jones, T. Slanted-rib waveguide InGaAsP-InP polarization converters. J. Lightwave Technol. 2004, 22, 1352. [Google Scholar] [CrossRef]
- Tanemura, T.; Amemiya, T.; Takeda, K.; Higo, A.; Nakano, Y. Simple and compact InP polarization converter for polarization-multiplexed photonic integrated circuits. In Proceedings of the 2009 IEEE LEOS Annual Meeting Conference Proceedings, Belek-Antalya, Turkey, 4–8 October 2009; pp. 436–437. [Google Scholar]
- Groen, F.; Zhu, Y.; Van Der Tol, J. Compact polarisation converter on InP/InGaAsP using an asymmetrical waveguide. In Proceedings of the 11th European Conference on Integrated Optics (ECIO 2003), Prague, Czech Republic, 2–4 April 2003. [Google Scholar]


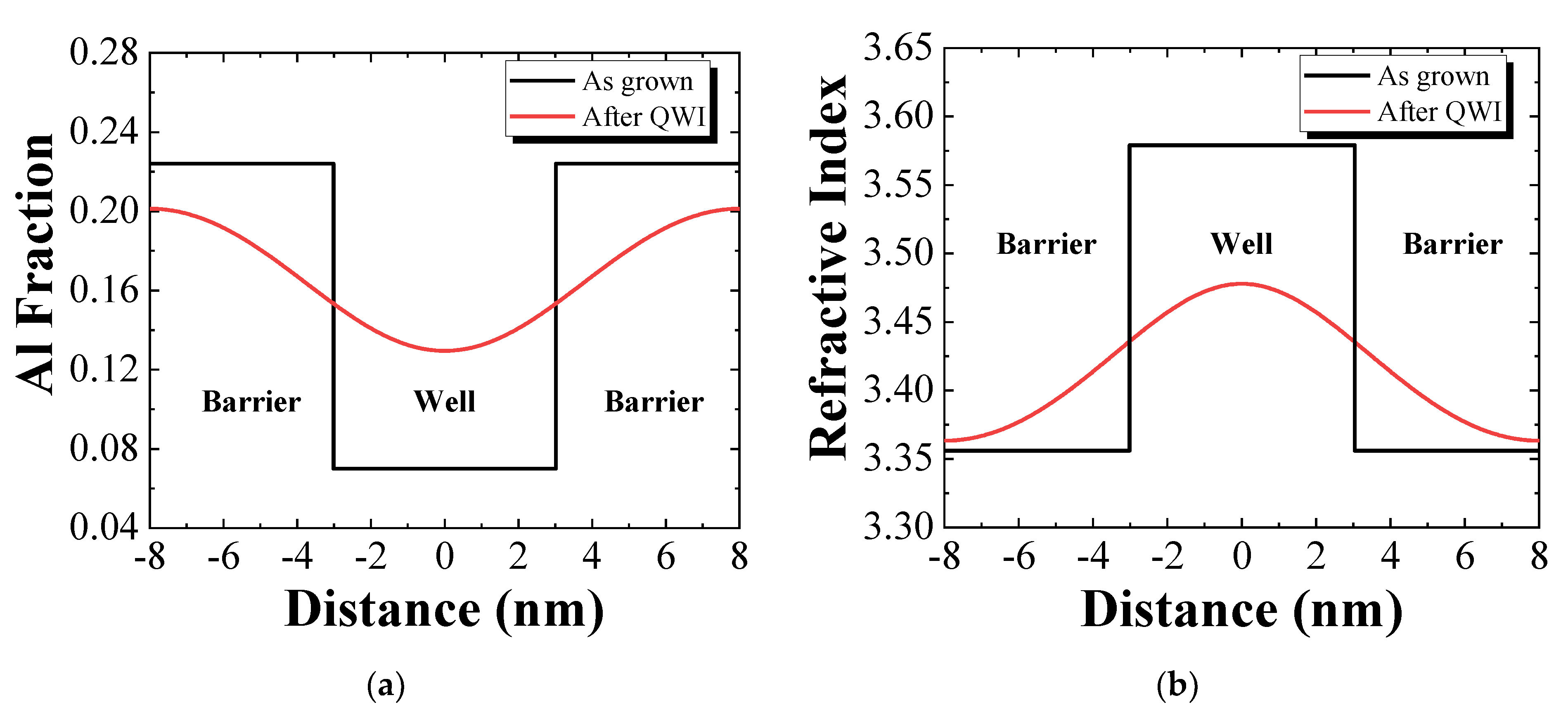
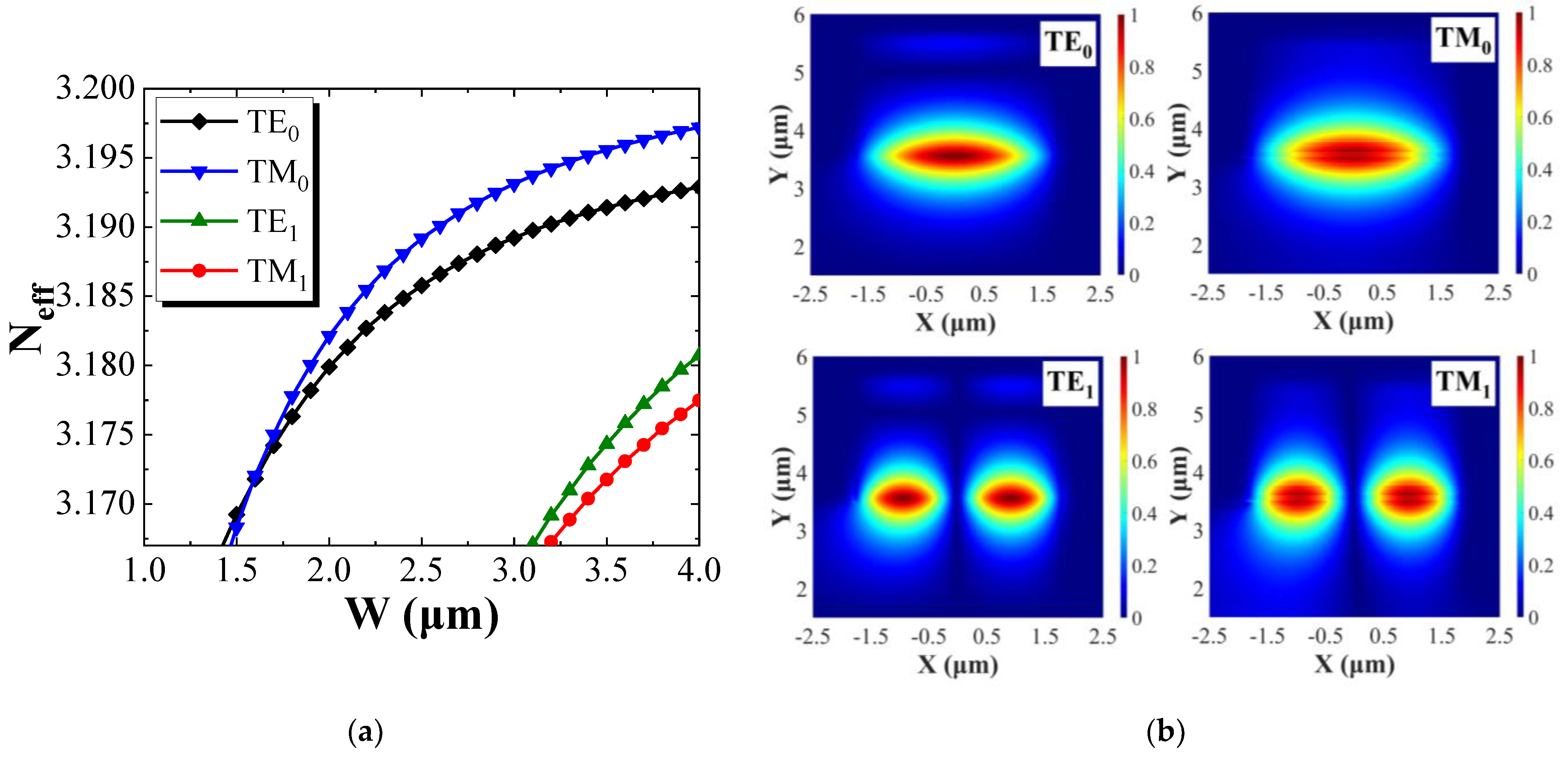



| Specifications | PL Wavelength (nm) | Al Fraction | Refractive Index | Diffusion Length |
|---|---|---|---|---|
| As-grown | 1530 | 0.07 (QW), 0.224 (QB) | 3.579 (QW), 3.356 (QB) | |
| After QWI | 1330 | 0.166 (average) | 3.4168 (average) | 2.45 nm |
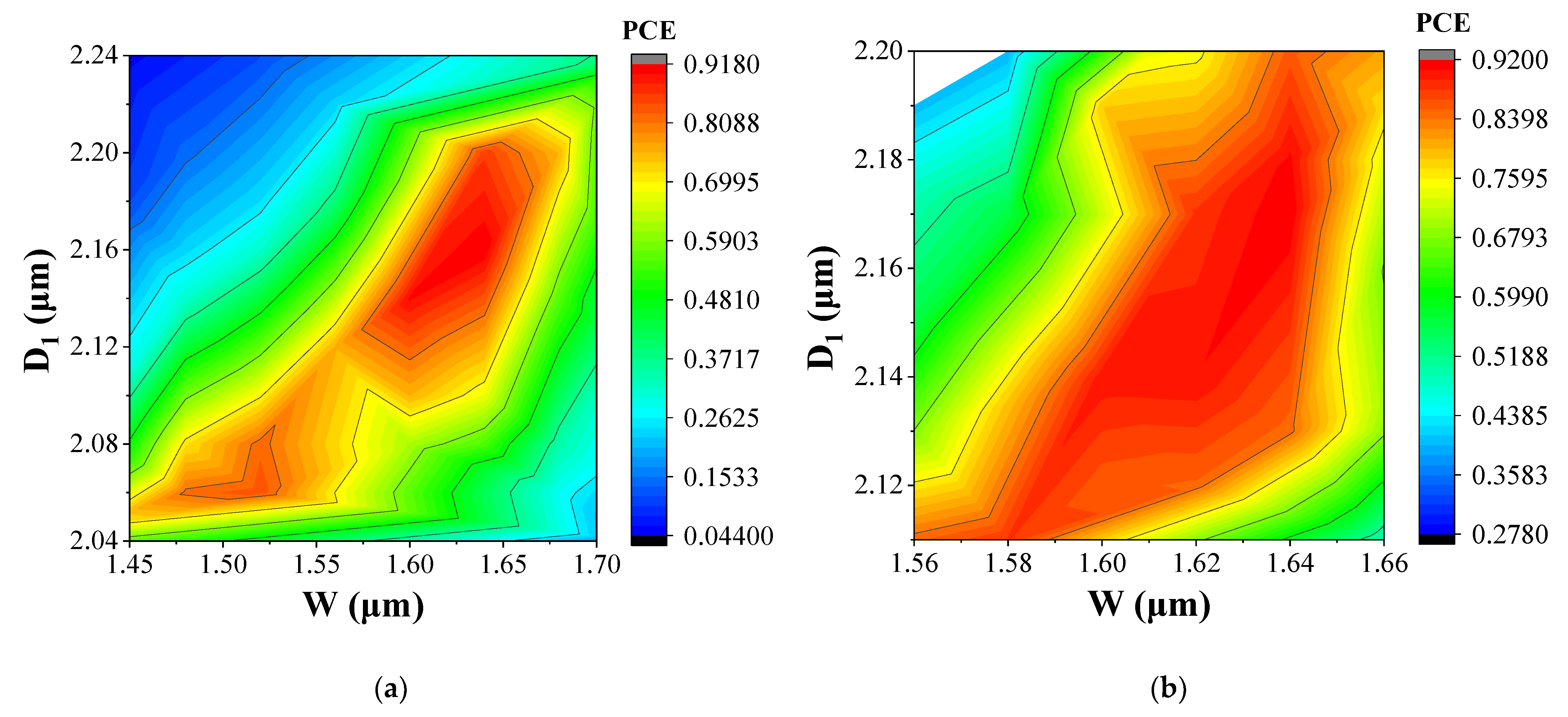
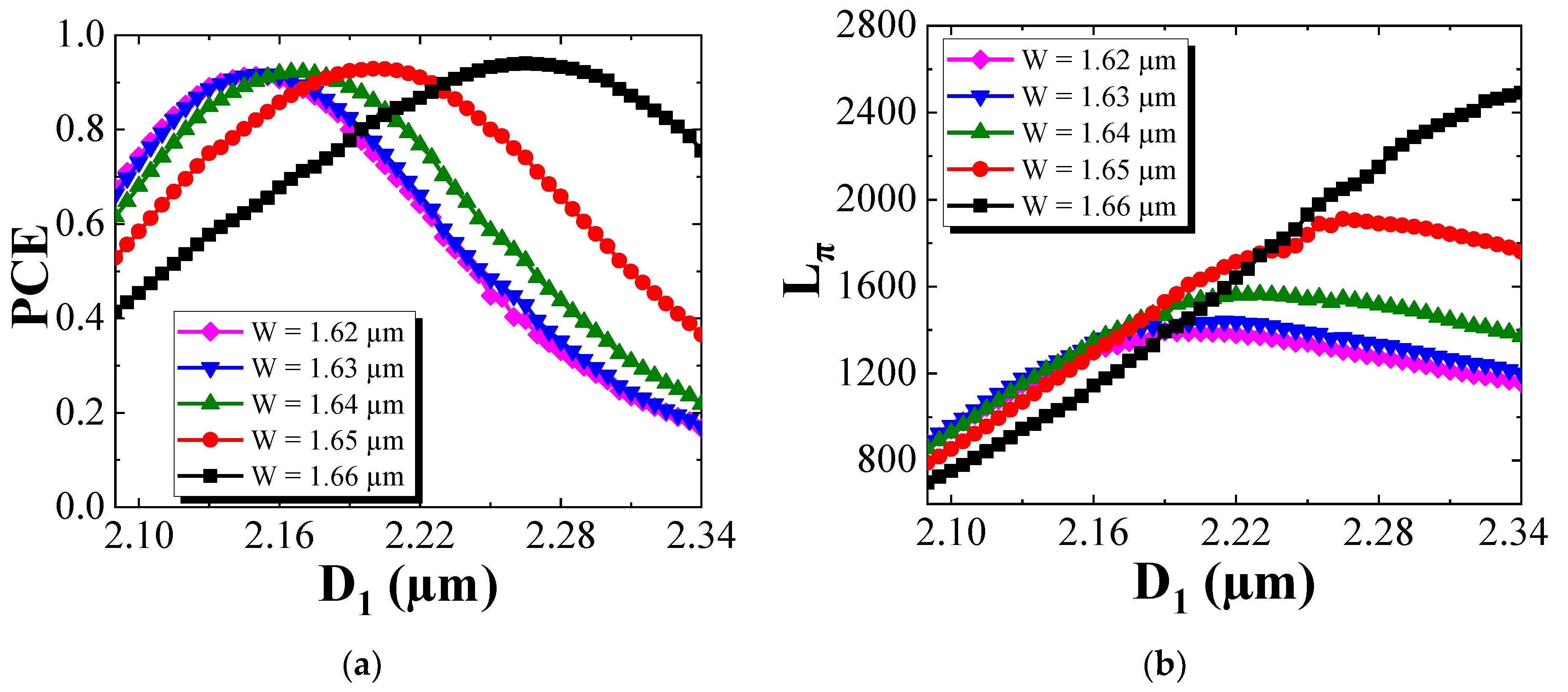
| Profiles | W | D1 | D2 | Lπ | MAX PCE |
|---|---|---|---|---|---|
| 1 | 1.58 μm | 2.10 μm | 3.64 μm | 970 μm | 90.2% |
| 2 | 1.62 μm | 2.16 μm | 3.64 μm | 1250 μm | 92.5% |
| 3 | 1.66 μm | 2.24 μm | 3.64 μm | 1870 μm | 94.0% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, X.; Ye, S.; Qiu, B.; Xiong, J.; Liu, X.; Marsh, J.; Hou, L. Design and Optimization of 1.55 μm AlGaInAs MQW Polarization Mode Controllers. Photonics 2021, 8, 422. https://doi.org/10.3390/photonics8100422
Sun X, Ye S, Qiu B, Xiong J, Liu X, Marsh J, Hou L. Design and Optimization of 1.55 μm AlGaInAs MQW Polarization Mode Controllers. Photonics. 2021; 8(10):422. https://doi.org/10.3390/photonics8100422
Chicago/Turabian StyleSun, Xiao, Shengwei Ye, Bocang Qiu, Jichuan Xiong, Xuefeng Liu, John Marsh, and Lianping Hou. 2021. "Design and Optimization of 1.55 μm AlGaInAs MQW Polarization Mode Controllers" Photonics 8, no. 10: 422. https://doi.org/10.3390/photonics8100422
APA StyleSun, X., Ye, S., Qiu, B., Xiong, J., Liu, X., Marsh, J., & Hou, L. (2021). Design and Optimization of 1.55 μm AlGaInAs MQW Polarization Mode Controllers. Photonics, 8(10), 422. https://doi.org/10.3390/photonics8100422





