Irregular Shifting of RF Driving Signal Phase to Overcome Dispersion Power Fading
Abstract
:1. Introduction
- A new method for dealing with dispersion power fading by using irregular θ.
- Generating a simple RoF link with standard DD-MZM as an E/O converter that can overcome dispersion power fading at all m. This link:
- (a)
- Can be used in any fm, L, and λc without having to re-adjust the transmitter.
- (b)
- Has Xrec(t) set at the same frequency as that of XTX(t), thereby removing any additional work.
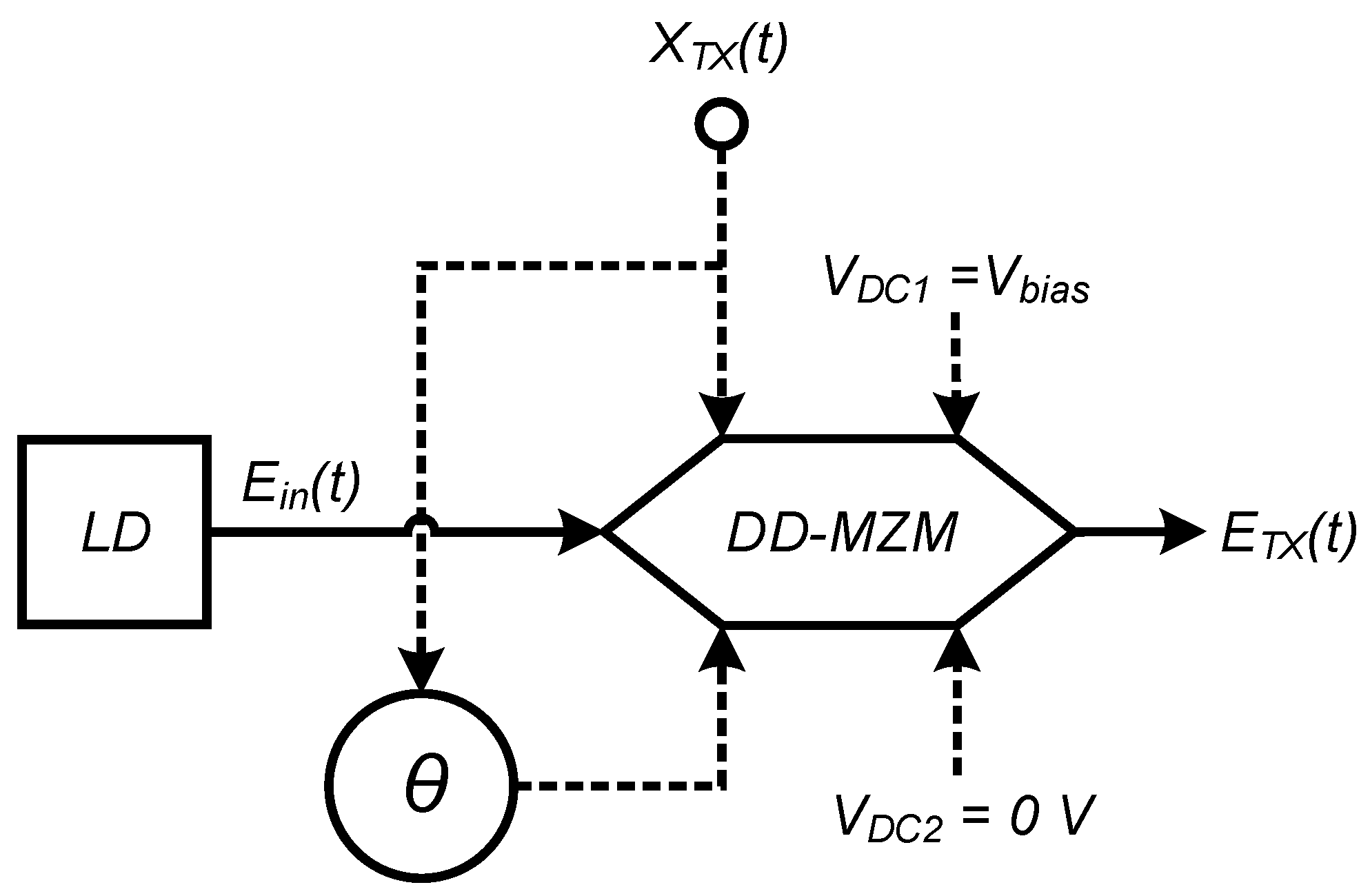
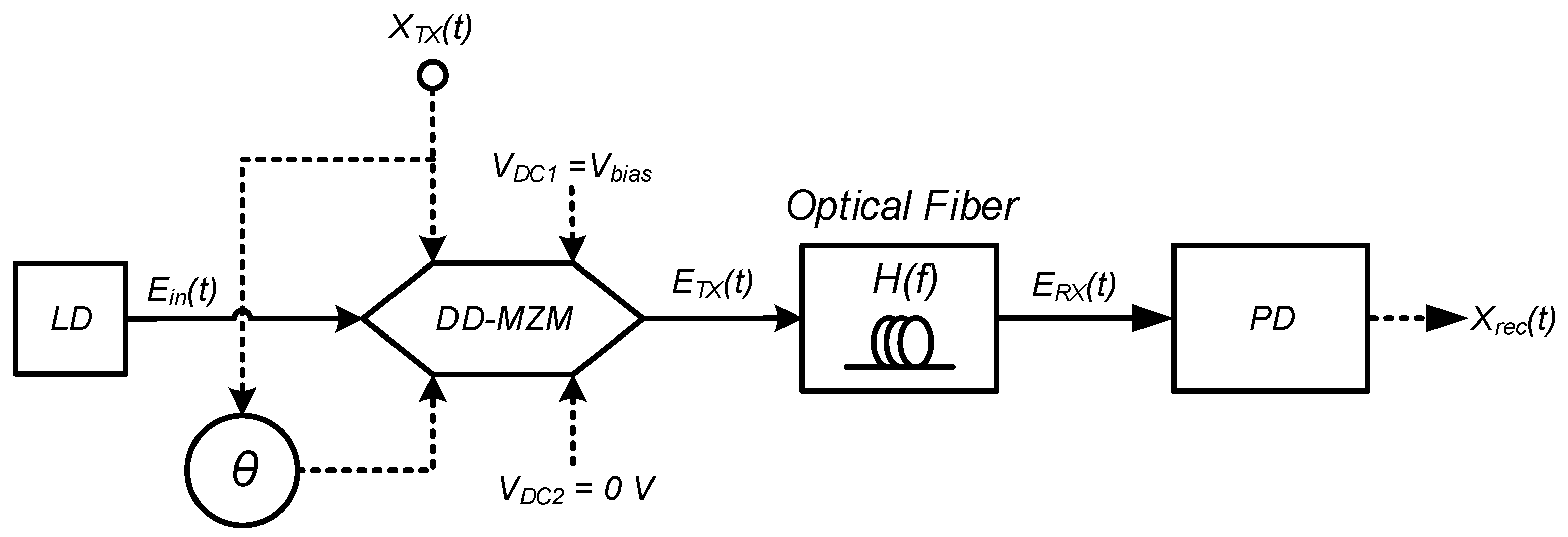
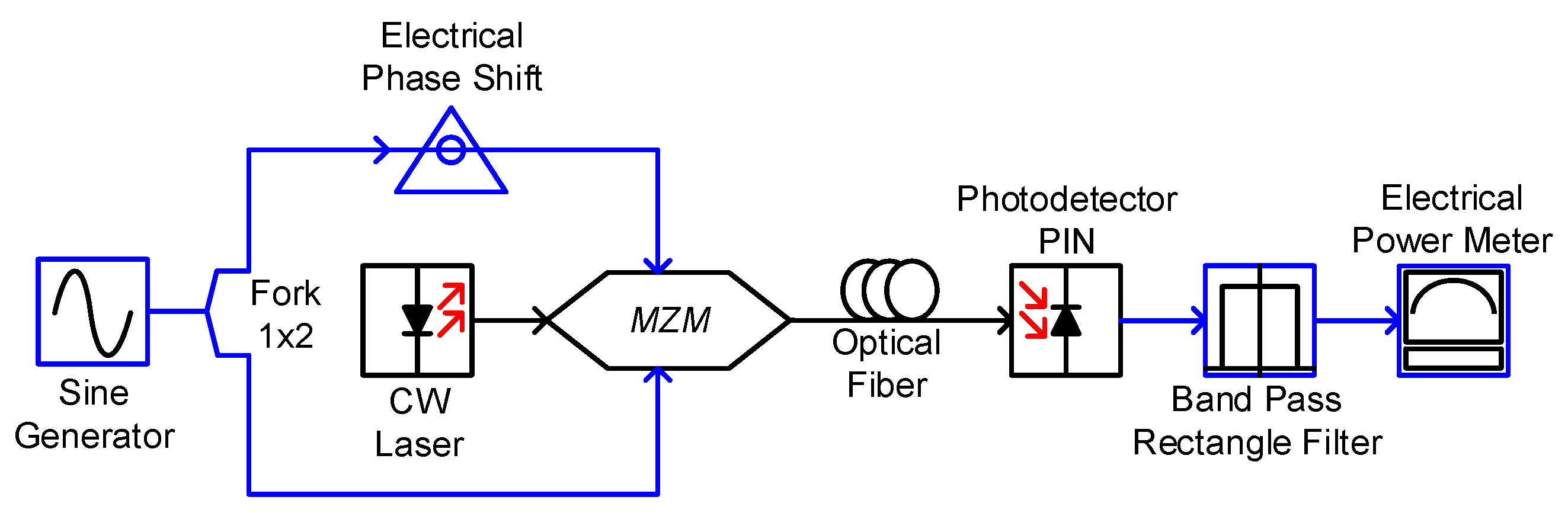
2. Principles of DD-MZM
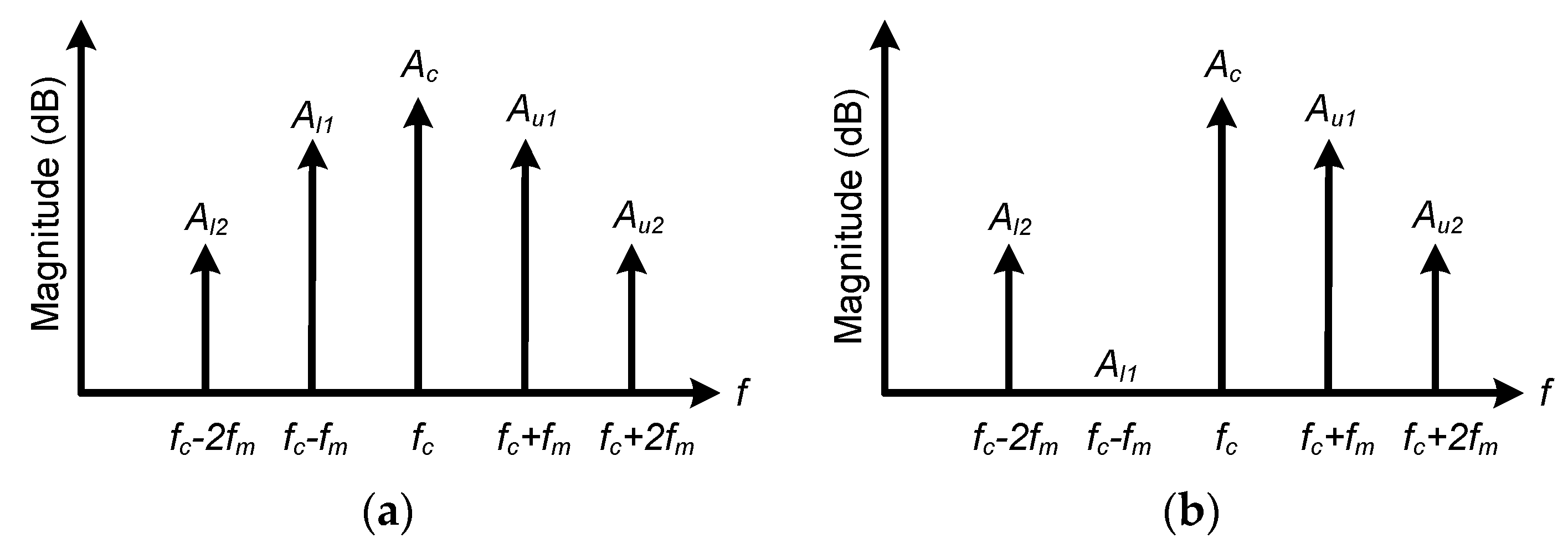
- For and (QBP, which is ), an ODSB modulation scheme is produced. The spectrum of this modulated signal is shown in Figure 2a.
3. Modeling of Recovered RF Signal Power
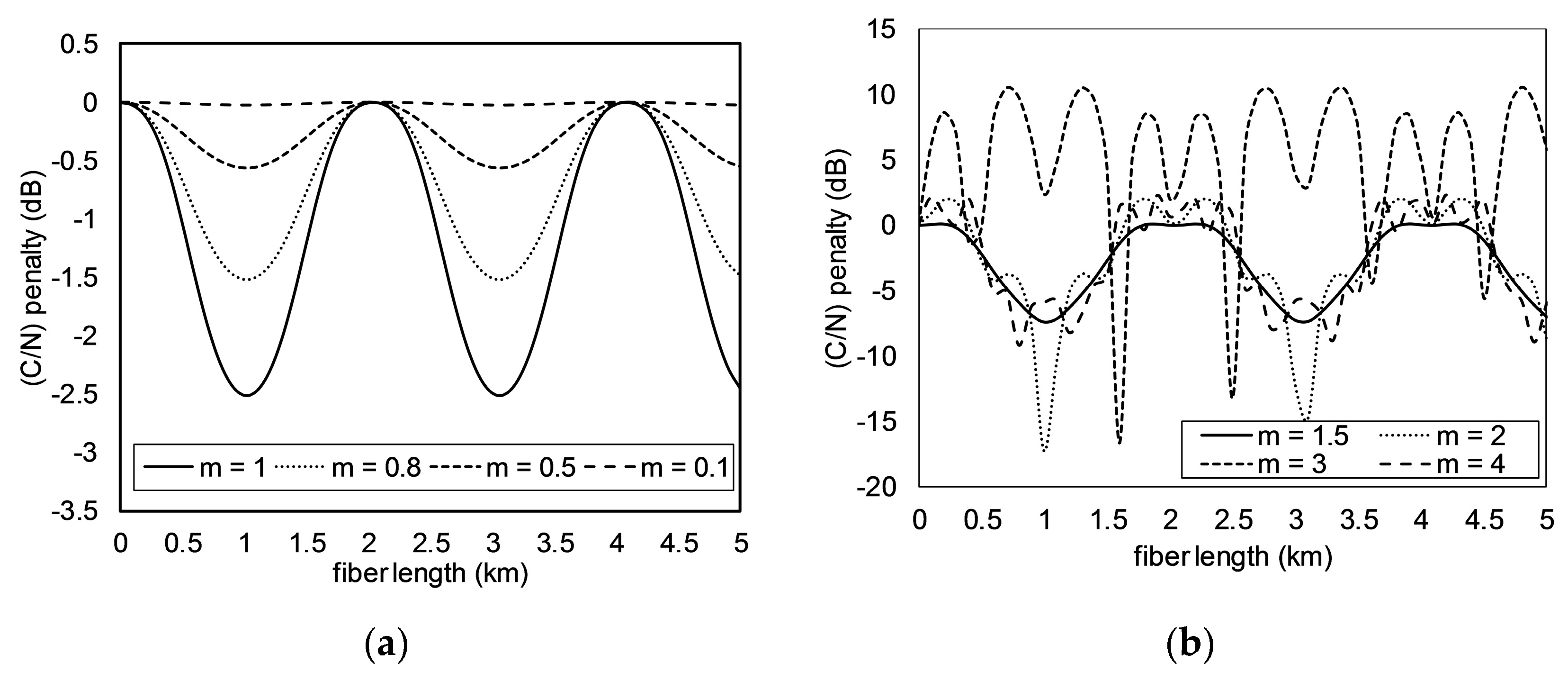
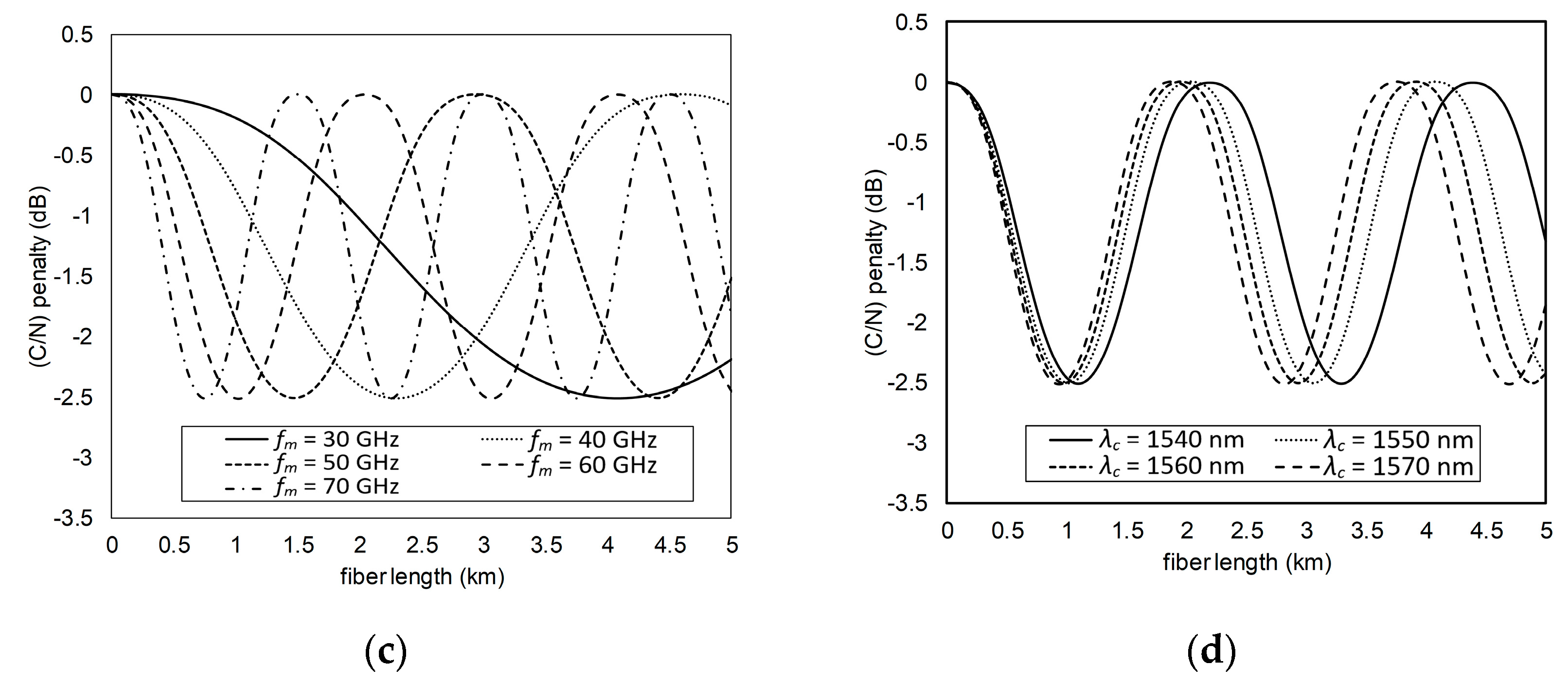
3.1. (C/N) Penalty on ODSB
3.2. (C/N) Penalty on OSSB
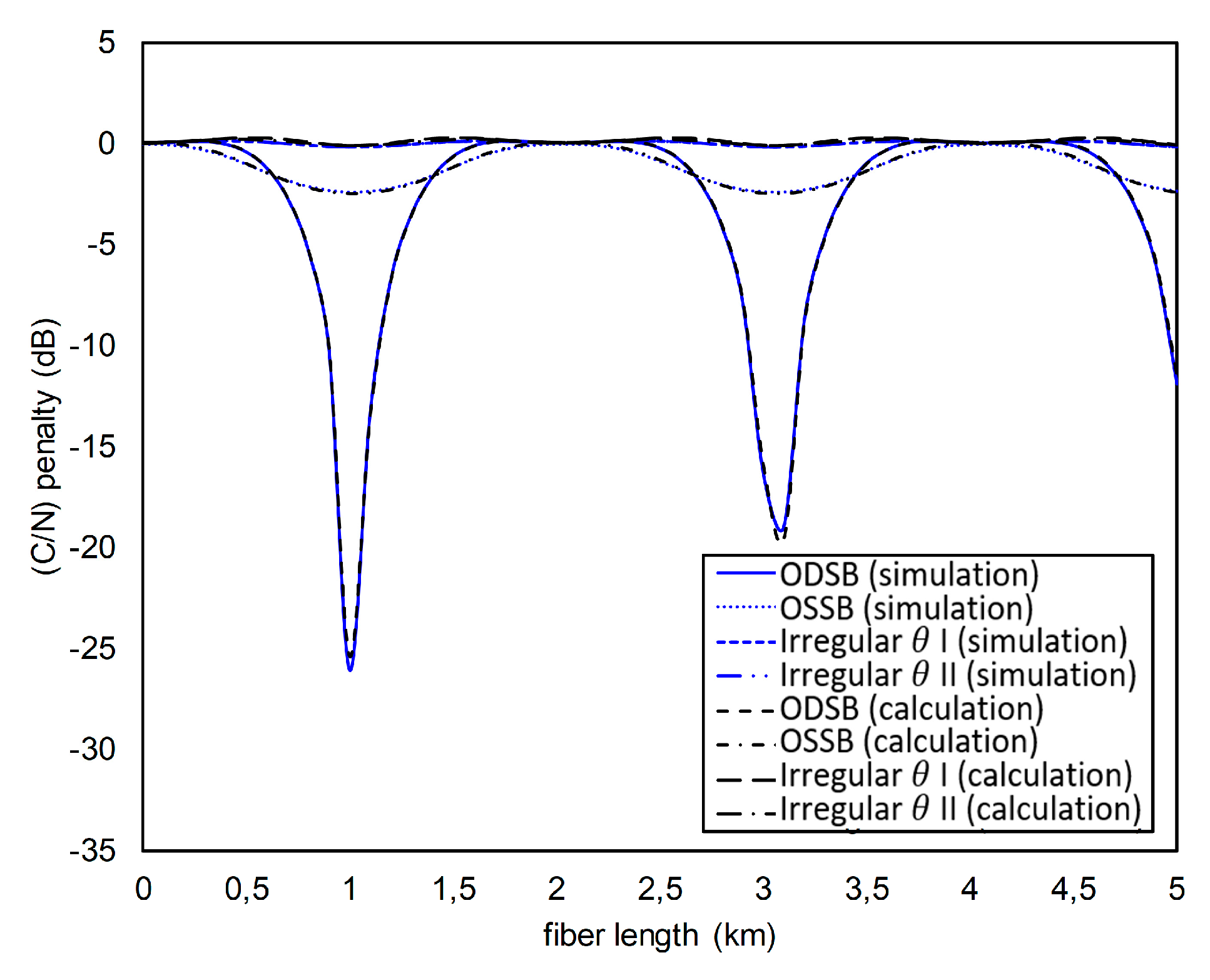
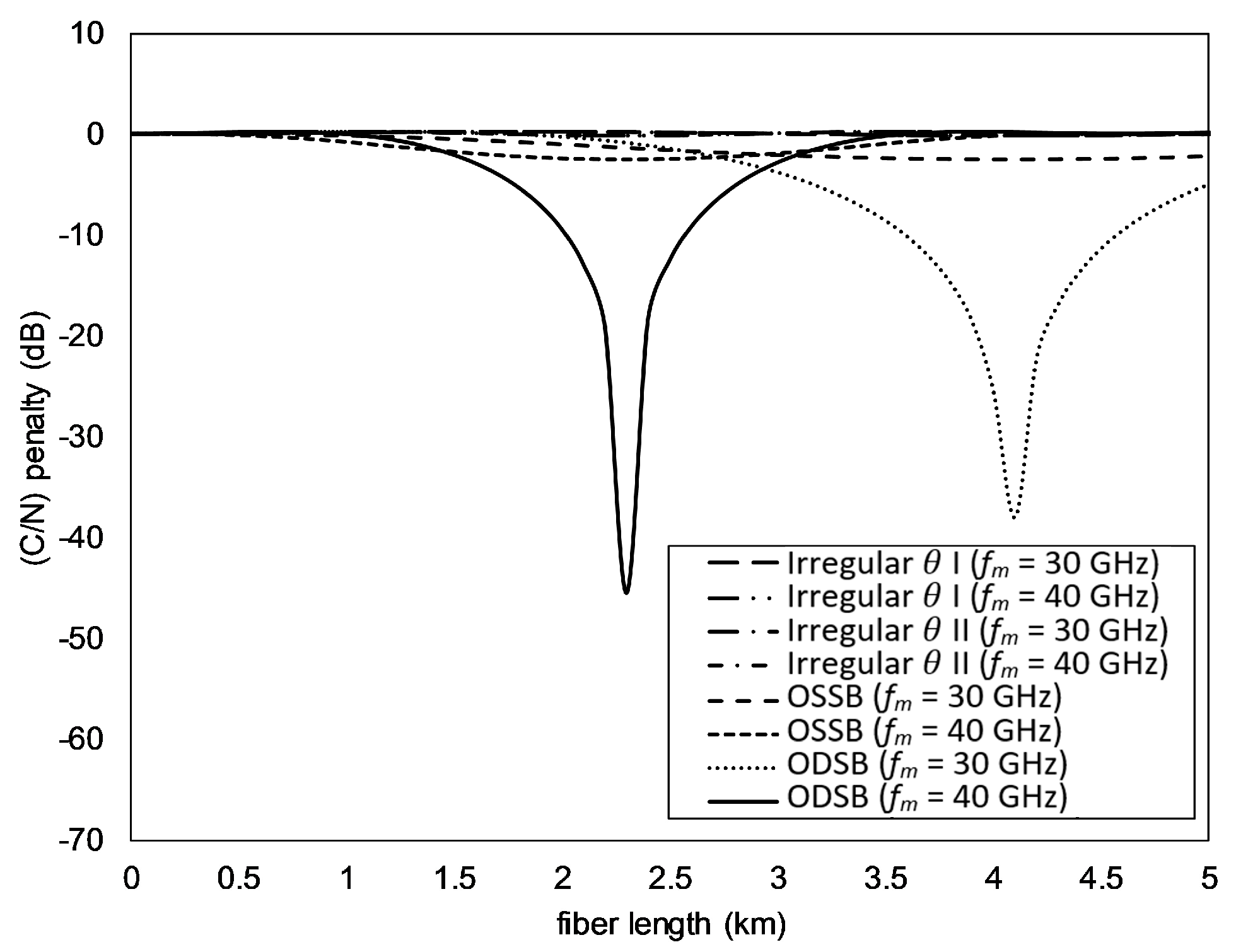
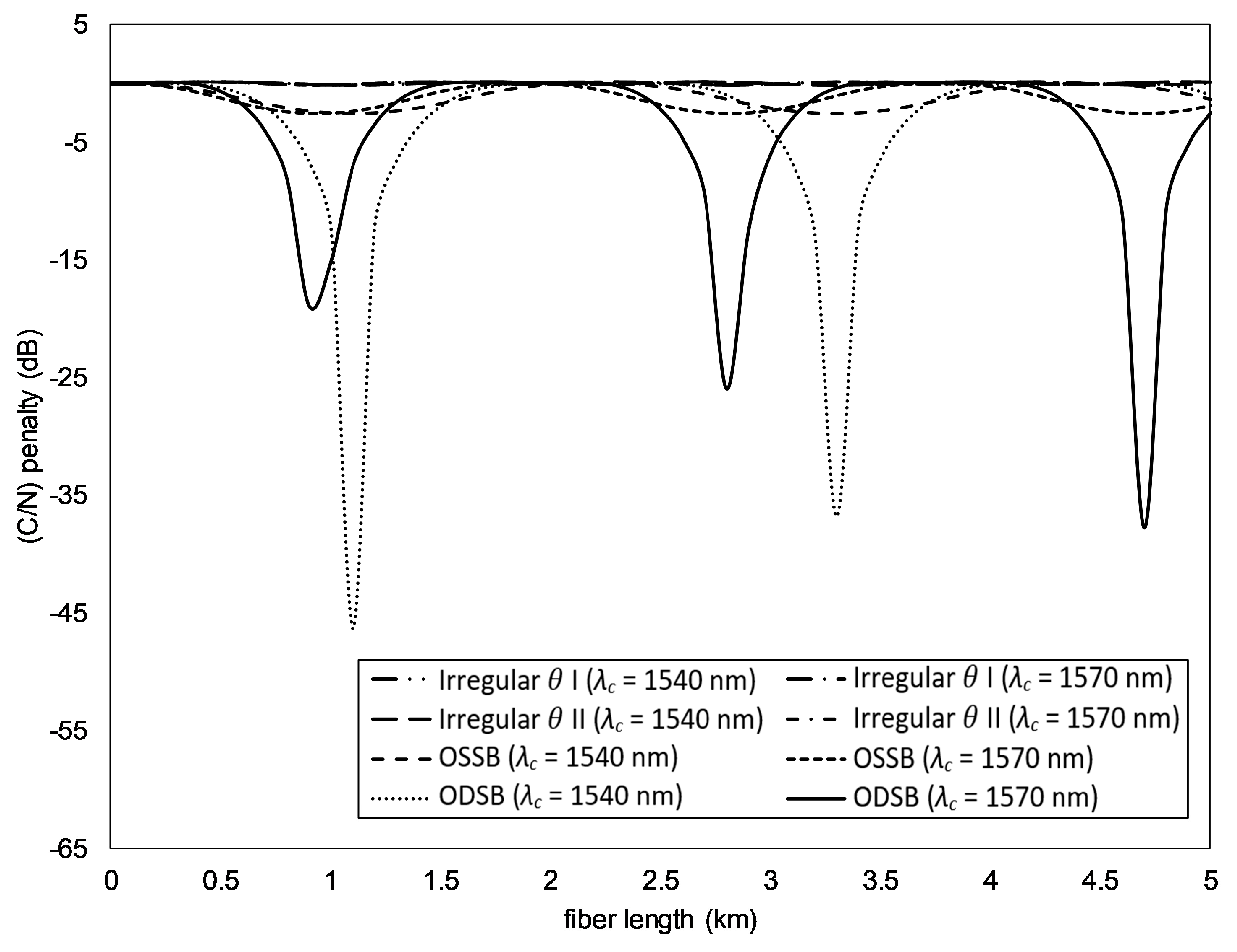
4. Irregular Phase Shifted
- (a)
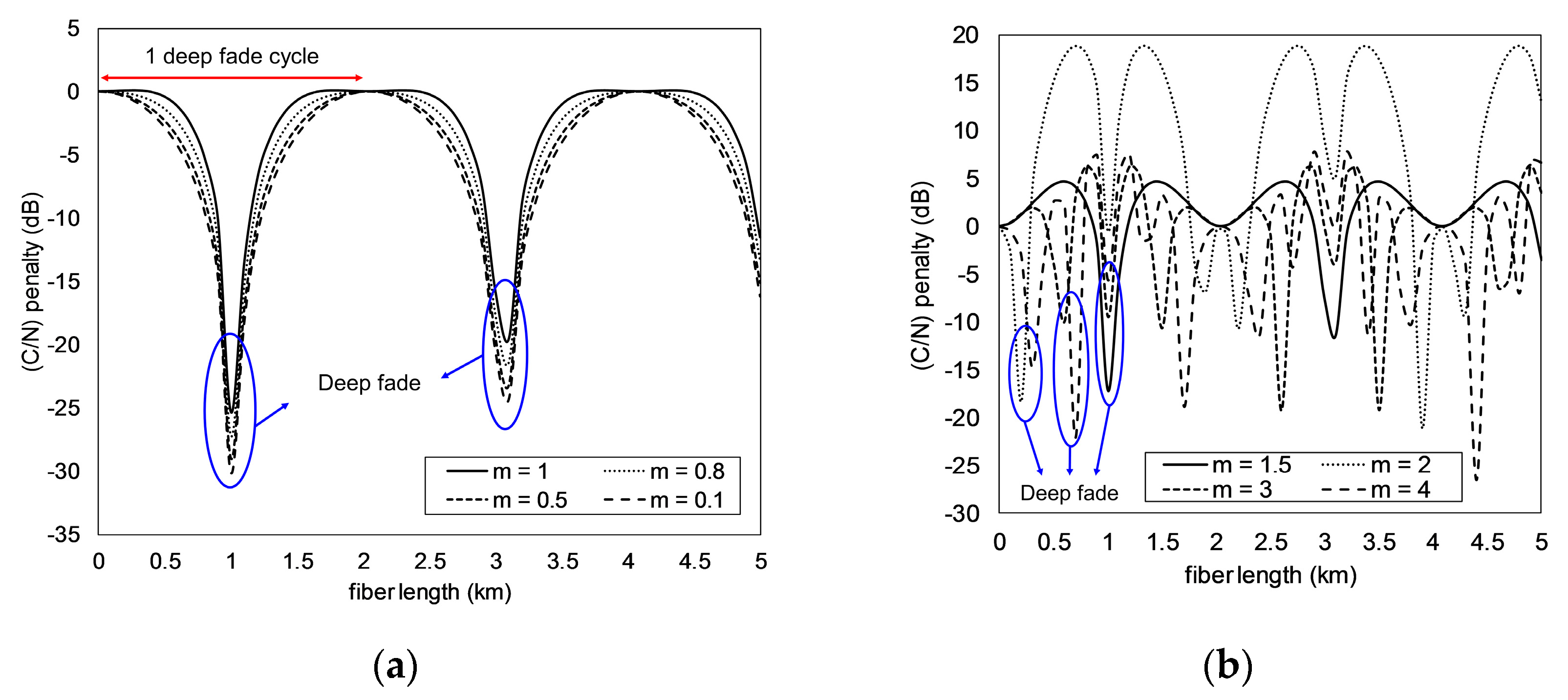
- Calculate Prec(L) using (24) with n = 10, m = 0.1, θ = 0 rad, λc = 1550 nm (D = 17 ps/(nm km)), and fm = 60 GHz. The Prec(L) is calculated at 0 ≤ L ≤ 5 km with step 0.1 km.
- (b)
- From the obtained Prec(L) in a), calculate the (C/N) penalty using (26).
- (c)
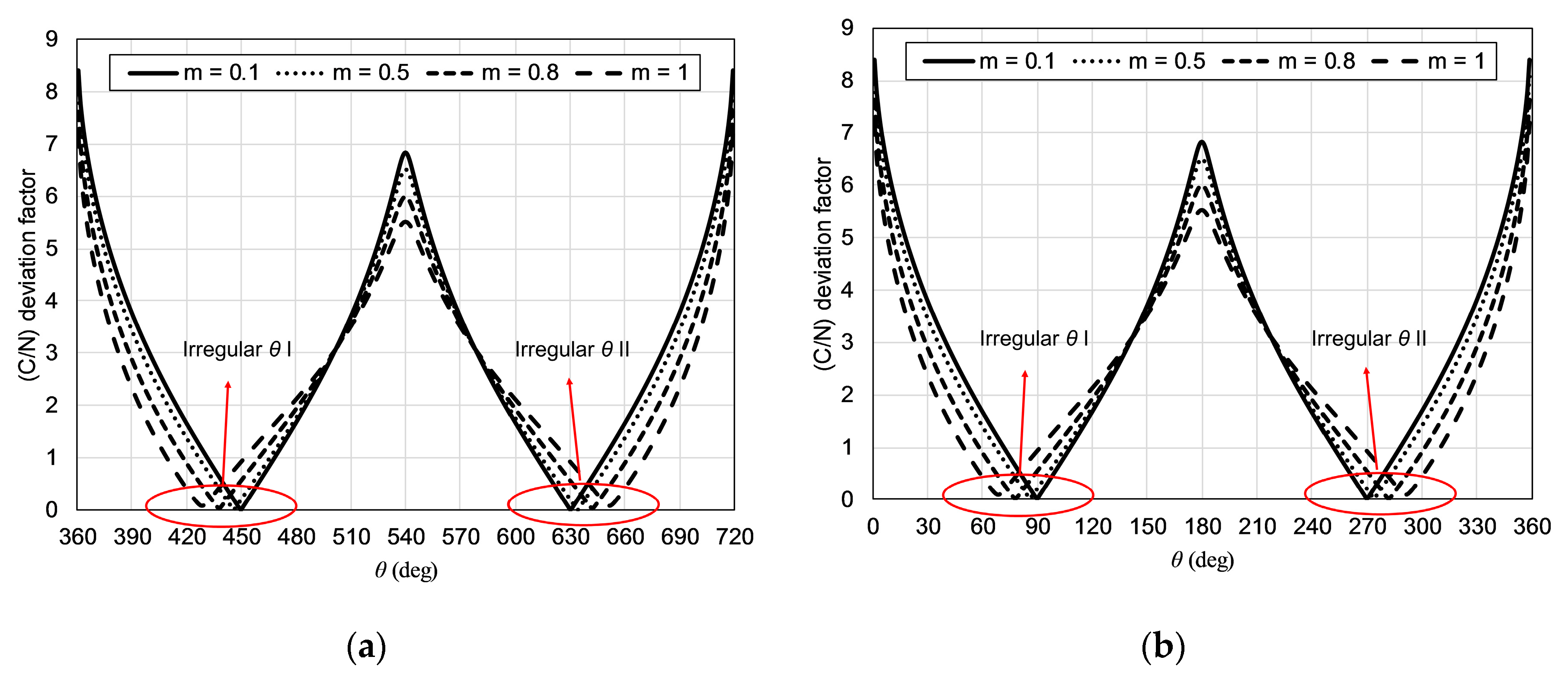
- Calculate the (C/N) deviation factor using (27) of all (C/N) penalties in b).
- (d)
- Repeat steps a) to c) for the value 0° ≤ θ ≤ 360° with step 1°.
- (e)
- Find θ in step d) which produces the smallest (C/N) deviation factor.
- (f)
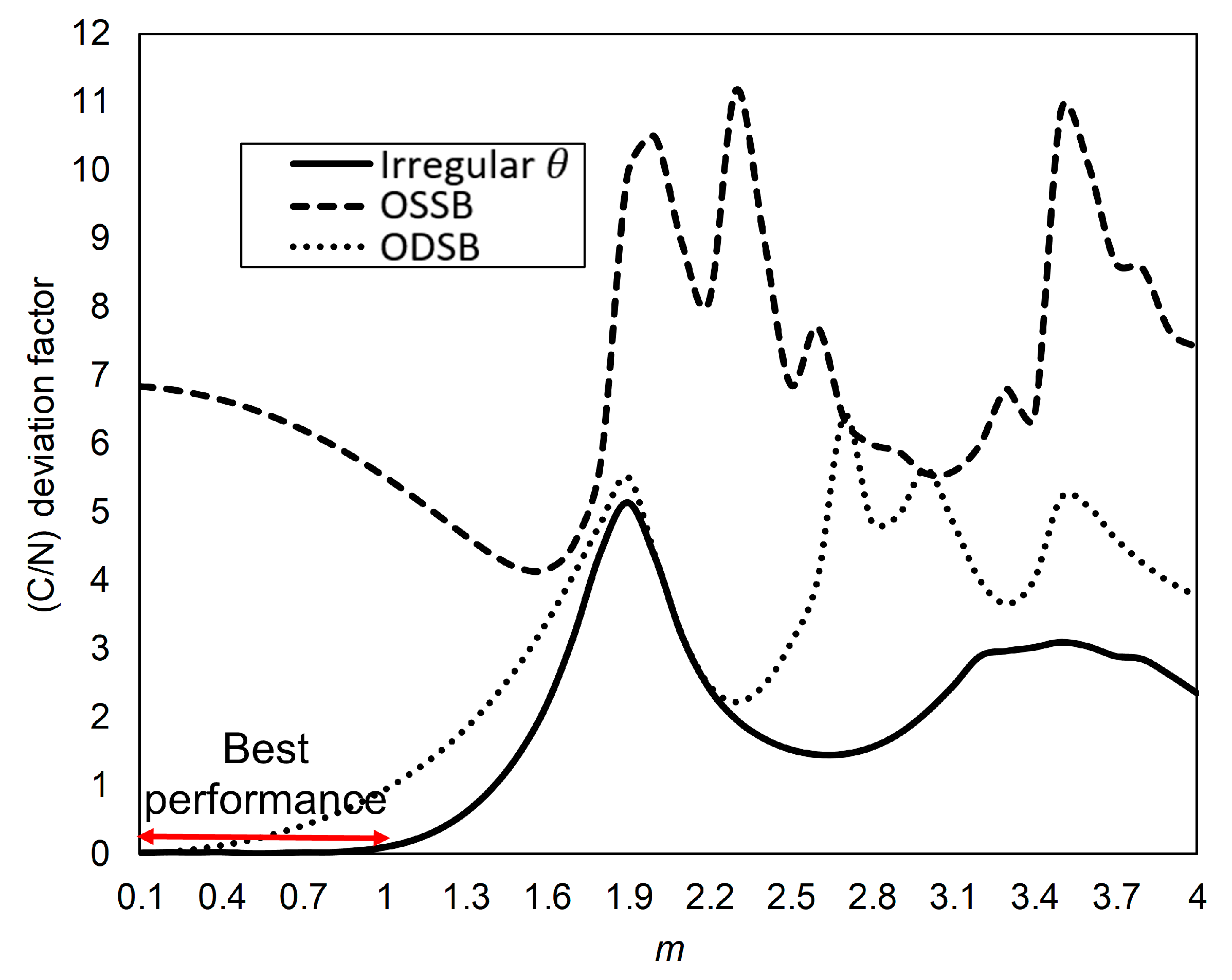
- Repeat steps a) to e) for 0.1 ≤ m ≤ 4 with step 0.1. The value of m is limited to 4 since only in this condition can the sidebands of >10 order be ignored.
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Fernando, X.N. Radio over Fiber for Wireless Communications: From Fundamentals to Advanced Topics; John Wiley & Sons: Hoboken, NJ, USA, 2014. [Google Scholar]
- Insua, I.G. Optical Generation of mm-Wave Signals for Use in Broadband Radio over Fiber Systems. Ph.D. Thesis, Technische Universität Dresden, Dresden, Germany, 2010. [Google Scholar]
- Elwan, H.H.; Poette, J.; Cabon, B. Simplified Chromatic Dispersion Model Applied to Ultra-Wide Optical Spectra for 60 GHz Radio-Over-Fiber Systems. J. Lightwave Technol. 2019, 37, 5115–5121. [Google Scholar] [CrossRef]
- Rzaigui, H.; Poette, J.; Cabon, B.; Brendel, F.; Khayatzadeh, R. Optical heterodyning for reduction of chromatic dispersion sensitivity in 60 GHz mode-locked laser systems. J. Lightwave Technol. 2013, 31, 2955–2960. [Google Scholar] [CrossRef]
- Brendel, F.; Poette, J.; Cabon, B.; Zwick, T.; van Dijk, F.; Lelarge, F.; Accard, A. Chromatic dispersion in 60 GHz radio-over-fiber networks based on mode-locked lasers. J. Lightwave Technol. 2011, 29, 3810–3816. [Google Scholar] [CrossRef]
- Hu, B.-N.; Wang, J.; Wang, W.; Zhao, R.-W. Analysis on Dispersion Compensation with DCF based on Optisystem. In Proceedings of the 2010 2nd International Conference on Industrial and Information Systems, Dalian, China, 10–11 July 2010. [Google Scholar] [CrossRef]
- Li, S.; Zheng, X.; Zhang, H.; Zhou, B. Compensation of dispersion-induced power fading for highly linear radio-over-fiber link using carrier phase-shifted double sideband modulation. Opt. Lett. 2011, 36, 546–548. [Google Scholar] [CrossRef] [PubMed]
- Liu, J.; Wu, X.; Huang, C.; Tsang, H.K.; Shu, C. Compensation of Dispersion-Induced Power Fading in Analog Photonic Links by Gain-Transparent SBS. IEEE Photonics Technol. Lett. 2018, 30, 688–691. [Google Scholar] [CrossRef]
- Gao, Y.; Wen, A.; Liu, L.; Tian, S.; Xiang, S.; Wang, Y. Compensation of the dispersion-induced power fading in an analog photonic link based on PM–IM conversion in a Sagnac loop. J. Lightwave Technol. 2015, 33, 2899–2904. [Google Scholar] [CrossRef]
- Chen, Z.; Yan, L.; Jiang, H.; Pan, W.; Luo, B.; Zou, X. Dispersion compensation in analog photonic link utilizing a phase modulator. J. Lightwave Technol. 2014, 32, 4642–4647. [Google Scholar] [CrossRef]
- Gao, Y.; Wen, A.; Wu, X.; Wang, Y.; Zhang, H. Efficient photonic microwave mixer with compensation of the chromatic dispersion-induced power fading. J. Lightwave Technol. 2016, 34, 3440–3448. [Google Scholar] [CrossRef]
- Yin, C.; Li, J.; Li, B.; Lv, Q.; Dai, J.; Yin, F.; Dai, Y.; Xu, K. Microwave Photonic Frequency Up-Convertor with Frequency Doubling and Compensation of Chromatic-Dispersion-Induced Power Fading. IEEE Photonics J. 2017, 9, 1–7. [Google Scholar] [CrossRef]
- Tian, Y.; Lee, K.-L.; Lim, C.; Nirmalathas, A. 60 GHz Analog Radio-Over-Fiber Fronthaul Investigations. J. Lightwave Technol. 2017, 35, 4304–4310. [Google Scholar] [CrossRef]
- Dong, Z. 64 QAM vector radio-frequency signal generation based on phase precoding and optical carrier suppression modulation. IEEE Photonics J. 2016, 8, 1–7. [Google Scholar] [CrossRef]
- Tian, Y.; Lee, K.-L.; Lim, C.; Nirmalathas, A. Experimental comparison of DSB-SC & OSSB based 60 GHz radio-over-fiber fronthaul links. In Proceedings of the 2016 IEEE International Topical Meeting on Microwave Photonics (MWP), Long Beach, CA, USA, 31 October–3 November 2016. [Google Scholar] [CrossRef]
- Ma, J.; Yu, J.; Yu, C.; Xin, X.; Zeng, J.; Chen, L. Fiber dispersion influence on transmission of the optical millimeter-waves generated using LN-MZM intensity modulation. J. Lightwave Technol. 2007, 25, 3244–3256. [Google Scholar] [CrossRef]
- Yu, J.; Jia, Z.; Yi, L.; Su, Y.; Chang, G.-K.; Wang, T. Optical millimeter-wave generation or up-conversion using external modulators. IEEE Photonics Technol. Lett. 2006, 18, 265–267. [Google Scholar] [CrossRef]
- Wang, K.; Zheng, X.; Zhang, H.; Guo, Y. A radio-over-fiber downstream link employing carrier-suppressed modulation scheme to regenerate and transmit vector signals. IEEE Photonics Technol. Lett. 2007, 19, 1365–1367. [Google Scholar] [CrossRef]
- Al-Shareefi, N.A.; Hassan, S.; Malek, F.; Ngah, R.; Aljunid, S.A.; Abbas, S.; Anida, N. A study in OCS millimeter-wave generation using two parallel DD-MZMs. In Proceedings of the 2013 IEEE 11th Malaysia International Conference on Communications (MICC), Kuala Lumpur, Malaysia, 26–28 November 2013. [Google Scholar] [CrossRef]
- Lim, C.; Tian, Y.; Lee, K.-L.; Nirmalathas, A. Transport schemes for fiber-based fronthaul for transporting 60 GHz wireless signals. In Proceedings of the 2017 19th International Conference on Transparent Optical Networks (ICTON), Girona, Spain, 2–6 July 2017. [Google Scholar] [CrossRef]
- Basha, S.A.H.; Ghosh, D.; Sahu, P. 4-QAM and 16-QAM modulation employing single dual drive Mach-Zehnder modulator and FBG for OFDM-radio-over-fiber system. In Proceedings of the 2017 International Conference on Communication and Signal Processing (ICCSP), Chennai, India, 6–8 April 2017. [Google Scholar] [CrossRef]
- Park, M.; Song, J.-I. All-optical frequency upconversion of a quasi optical single sideband signal utilizing a nonlinear semiconductor optical amplifier for radio-over-fiber applications. Opt. Express 2011, 19, 24499–24506. [Google Scholar] [CrossRef] [PubMed]
- Paloi, F.; Haxha, S. Analysis of the carrier suppressed single sideband modulation for long distance optical communication systems. Optik 2018, 161, 230–243. [Google Scholar] [CrossRef]
- Xie, M.; Zhao, M.; Lei, M.; Wu, Y.; Liu, Y.; Gao, X.; Huang, S. Anti-dispersion phase-tunable microwave mixer based on a dual-drive dual-parallel Mach-Zehnder modulator. Opt. Express 2018, 26, 454–462. [Google Scholar] [CrossRef]
- Krishna, V.R.; Singhal, R. Dual carrier multiplexed M-QAM signals modulated optical SSB transmission over fiber. In Proceedings of the 2016 Thirteenth International Conference on Wireless and Optical Communications Networks (WOCN), Hyderabad, India, 21–23 July 2016. [Google Scholar] [CrossRef]
- Ma, J. Dual-tone QPSK optical millimeter-wave signal generation by frequency nonupling the RF signal without phase precoding. IEEE Photonics J. 2016, 8, 1–7. [Google Scholar] [CrossRef]
- Chen, X.; Yao, J. A High Spectral Efficiency Coherent RoF System Based on OSSB Modulation with Low-Cost Free-Running Laser Sources for UDWDM-PONs. J. Lightwave Technol. 2016, 34, 2789–2795. [Google Scholar] [CrossRef]
- Lim, C.; Nirmalathas, A.; Lee, K.-L.; Novak, D.; Waterhouse, R. Intermodulation distortion improvement for fiber–radio applications incorporating OSSB+ C modulation in an optical integrated-access environment. J. Lightwave Technol. 2007, 25, 1602–1612. [Google Scholar] [CrossRef]
- Peng, Z.; Wen, A.; Gao, Y.; Tu, Z.; Chen, W.; Zhang, W. A novel method to realize optical single sideband modulation with tunable optical carrier to sideband ratio. Microw. Opt. Technol. Lett. 2017, 59, 1740–1743. [Google Scholar] [CrossRef]
- Zheng, Z.; Peng, M.; Zhou, H.; Chen, M.; Jiang, L.; Tan, L.; Dai, X.; Xiang, Y. Optical single sideband millimeter-wave signal generation and transmission using 120° hybrid coupler. Opt. Commun. 2018, 411, 21–26. [Google Scholar] [CrossRef]
- Shen, Y.; Zhang, X.; Chen, K. Optical single sideband modulation of 11-GHz RoF system using stimulated Brillouin scattering. IEEE Photonics Technol. Lett. 2005, 17, 1277–1279. [Google Scholar] [CrossRef]
- Sung, H.-K.; Lau, E.K.; Wu, M.C. Optical single sideband modulation using strong optical injection-locked semiconductor lasers. IEEE Photonics Technol. Lett. 2007, 19, 1005–1007. [Google Scholar] [CrossRef]
- Wu, B.; Wang, M.; Tang, Y.; Sun, J.; Zhang, J.; Yan, F.; Jian, S. Optical single sideband modulation with tunable optical carrier-to-sideband ratio using a modulator in a Sagnac loop. Opt. Laser Technol. 2017, 91, 98–102. [Google Scholar] [CrossRef]
- Thomas, V.A.; El-Hajjar, M.; Hanzo, L. Optical single sideband signal generation relying on a single-drive Mach–Zehnder modulator for radio over fibre communications. IET Commun. 2016, 10, 534–539. [Google Scholar] [CrossRef]
- Lee, U.-S.; Jung, H.-D.; Han, S.-K. Optical single sideband signal generation using phase modulation of semiconductor optical amplifier. IEEE Photonics Technol. Lett. 2004, 16, 1373–1375. [Google Scholar] [CrossRef]
- Li, Z.; Chi, H.; Zhang, X.; Yao, J. Optical single-sideband modulation using a fiber-Bragg-grating-based optical Hilbert transformer. IEEE Photonics Technol. Lett. 2011, 23, 558–560. [Google Scholar] [CrossRef]
- Xue, M.; Pan, S.; Zhao, Y. Optical spectral response measurement based on optical single-sideband modulation with doubled measurement range. Electron. Lett. 2016, 52, 852–853. [Google Scholar] [CrossRef]
- Chang, C.-H.; Chang, J.-H. Optical SSB modulation scheme based on phase-modulator and vertical-cavity-surface-emitting laser. In Proceedings of the 2014 Conference on Lasers and Electro-Optics (CLEO)—Laser Science to Photonic Applications, San Jose, CA, USA, 8–13 June 2014. [Google Scholar] [CrossRef]
- Parajuli, H.N.; Udvary, E. Optimization of optical carrier to sideband ratio for the concatenated AM-PM based optical single sideband radio over fiber system. In Proceedings of the 2016 21st European Conference on Networks and Optical Communications (NOC), Lisbon, Portugal, 1–3 June 2016. [Google Scholar] [CrossRef]
- Smith, G.H.; Novak, D.; Ahmed, Z. Overcoming chromatic-dispersion effects in fiber-wireless systems incorporating external modulators. IEEE Trans. Microw. Theory Tech. 1997, 45, 1410–1415. [Google Scholar] [CrossRef]
- Patel, D.; Singh, V.K.; Dalal, U. Performance analysis of optical double sideband and optical single sideband generation using mach zehnder modulator chirp and extinction ratio over different fiber link. In Proceedings of the 2017 2nd International Conference for Convergence in Technology (I2CT), Mumbai, India, 7–9 April 2017. [Google Scholar] [CrossRef]
- Shimotsu, S.; Oikawa, S.; Saitou, T.; Mitsugi, N.; Kubodera, K.; Kawanishi, T.; Izutsu, M. Single side-band modulation performance of a LiNbO 3 integrated modulator consisting of four-phase modulator waveguides. IEEE Photonics Technol. Lett. 2001, 13, 364–366. [Google Scholar] [CrossRef]
- Hervé, D.; Corral, J.L.; Fuster, J.M.; Herrera, J.; Martinez, A.; Polo, V.; Ramos, F.; Vourc’h, E.; Marti, J. Techniques for the compensation for chromatic-dispersion effects in fiber-wireless systems. Ursi Radio Sci. Bull. 2004, 2004, 26–37. [Google Scholar] [CrossRef]
- Xue, M.; Pan, S.; Zhao, Y. Optical single-sideband modulation based on a dual-drive MZM and a 120 hybrid coupler. J. Lightwave Technol. 2014, 32, 3317–3323. [Google Scholar] [CrossRef]
- Ujang, F.; Wibisono, G. A Single DD-MZM for Generating Vestigial Sideband Modulation Scheme in Radio over Fiber Systems. In Proceedings of the 2018 2nd International Conference on Electrical Engineering and Informatics (ICon EEI), Batam, Indonesi, 16–17 October 2018. [Google Scholar] [CrossRef]
- Colton, D.; Kress, R. Inverse Acoustic and Electromagnetic Scattering Theory, 3rd ed.; Springer Science & Business Media: Heidelberg, Germany, 1998; p. 32. [Google Scholar]
- Davies, R.J. Optical Single Sideband for Broadband and Subcarrier Systems; University of Alberta: Edmonton, AB, Canada, 1999. [Google Scholar]
- Meslener, G. Chromatic dispersion induced distortion of modulated monochromatic light employing direct detection. IEEE J. Quantum Electron. 1984, 20, 1208–1216. [Google Scholar] [CrossRef]


| m | Irregular θ I (Degrees) | Irregular θ II (Degrees) | m | Irregular θ I (Degrees) | Irregular θ II (Degrees) |
|---|---|---|---|---|---|
| 0.1 | 90 | 270 | 2.1 | 88 | 272 |
| 0.2 | 89 | 271 | 2.2 | 85 | 275 |
| 0.3 | 89 | 271 | 2.3 | 79 | 281 |
| 0.4 | 88 | 272 | 2.4 | 73 | 287 |
| 0.5 | 86 | 274 | 2.5 | 68 | 292 |
| 0.6 | 84 | 276 | 2.6 | 65 | 295 |
| 0.7 | 81 | 279 | 2.7 | 62 | 298 |
| 0.8 | 78 | 282 | 2.8 | 60 | 300 |
| 0.9 | 74 | 286 | 2.9 | 59 | 301 |
| 1.0 | 69 | 291 | 3.0 | 58 | 302 |
| 1.1 | 63 | 297 | 3.1 | 18 | 342 |
| 1.2 | 56 | 304 | 3.2 | 15 | 345 |
| 1.3 | 50 | 310 | 3.3 | 105 | 255 |
| 1.4 | 44 | 316 | 3.4 | 107 | 256 |
| 1.5 | 38 | 322 | 3.5 | 108 | 252 |
| 1.6 | 32 | 328 | 3.6 | 107 | 253 |
| 1.7 | 28 | 332 | 3.7 | 105 | 255 |
| 1.8 | 145 | 215 | 3.8 | 103 | 257 |
| 1.9 | 142 | 218 | 3.9 | 47 | 313 |
| 2.0 | 86 | 274 | 4.0 | 45 | 315 |
| Parameter | Value | Units |
|---|---|---|
| Extinction ratio | 20 | dB |
| Switching bias voltage | 4 | V |
| Switching radio frequency (RF) voltage | 4 | V |
| Insertion loss | 0 | dB |
| Normalize electrical signal | unchecked | - |
| Bias voltage1 | 0 | V |
| Bias voltage2 | 2 | V |
| Parameter | Value | Units |
|---|---|---|
| User-defined reference wavelength | Checked | - |
| Reference wavelength | 1550 | nm |
| Length | 0–5 | km |
| Attenuation effect | Unchecked | - |
| Group velocity dispersion | Checked | - |
| Third-order dispersion | Unchecked | - |
| Frequency domain parameter | Unchecked | - |
| Dispersion | 17 | ps/nm/km |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ujang, F.; Firmansyah, T.; Priambodo, P.S.; Wibisono, G. Irregular Shifting of RF Driving Signal Phase to Overcome Dispersion Power Fading. Photonics 2019, 6, 104. https://doi.org/10.3390/photonics6040104
Ujang F, Firmansyah T, Priambodo PS, Wibisono G. Irregular Shifting of RF Driving Signal Phase to Overcome Dispersion Power Fading. Photonics. 2019; 6(4):104. https://doi.org/10.3390/photonics6040104
Chicago/Turabian StyleUjang, Febrizal, Teguh Firmansyah, Purnomo S. Priambodo, and Gunawan Wibisono. 2019. "Irregular Shifting of RF Driving Signal Phase to Overcome Dispersion Power Fading" Photonics 6, no. 4: 104. https://doi.org/10.3390/photonics6040104
APA StyleUjang, F., Firmansyah, T., Priambodo, P. S., & Wibisono, G. (2019). Irregular Shifting of RF Driving Signal Phase to Overcome Dispersion Power Fading. Photonics, 6(4), 104. https://doi.org/10.3390/photonics6040104






