Bunimovich Stadium-Like Resonator for Randomized Fiber Laser Operation
Abstract
1. Introduction
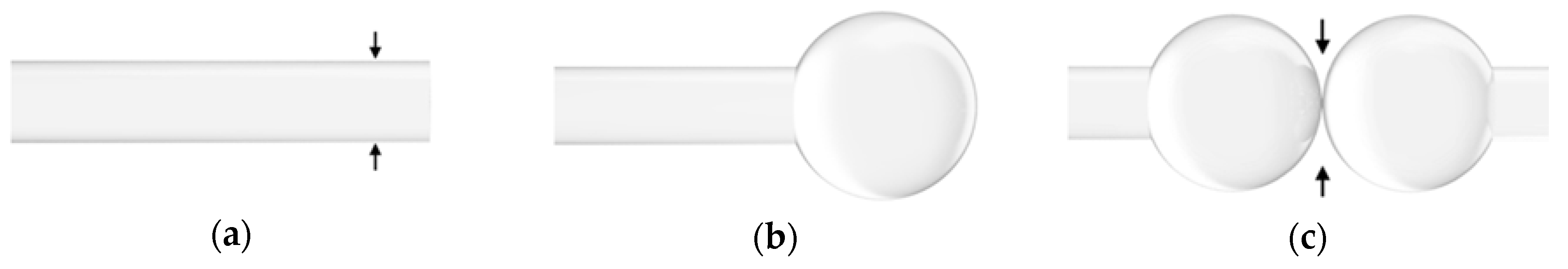
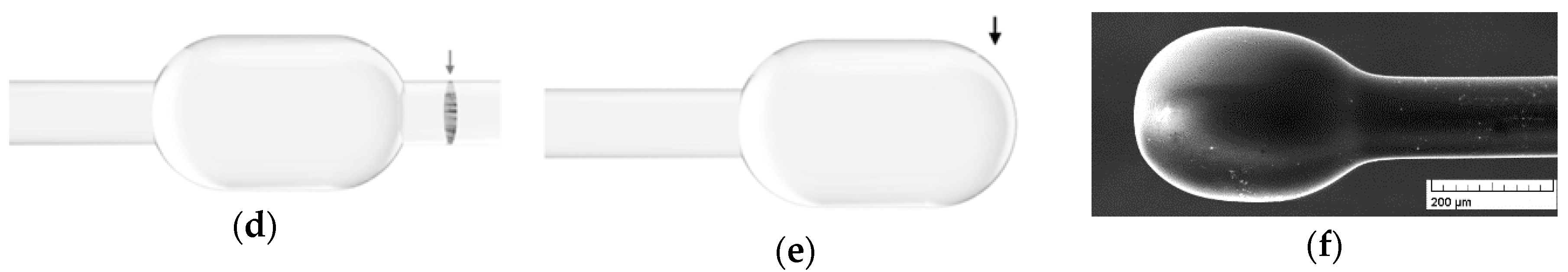
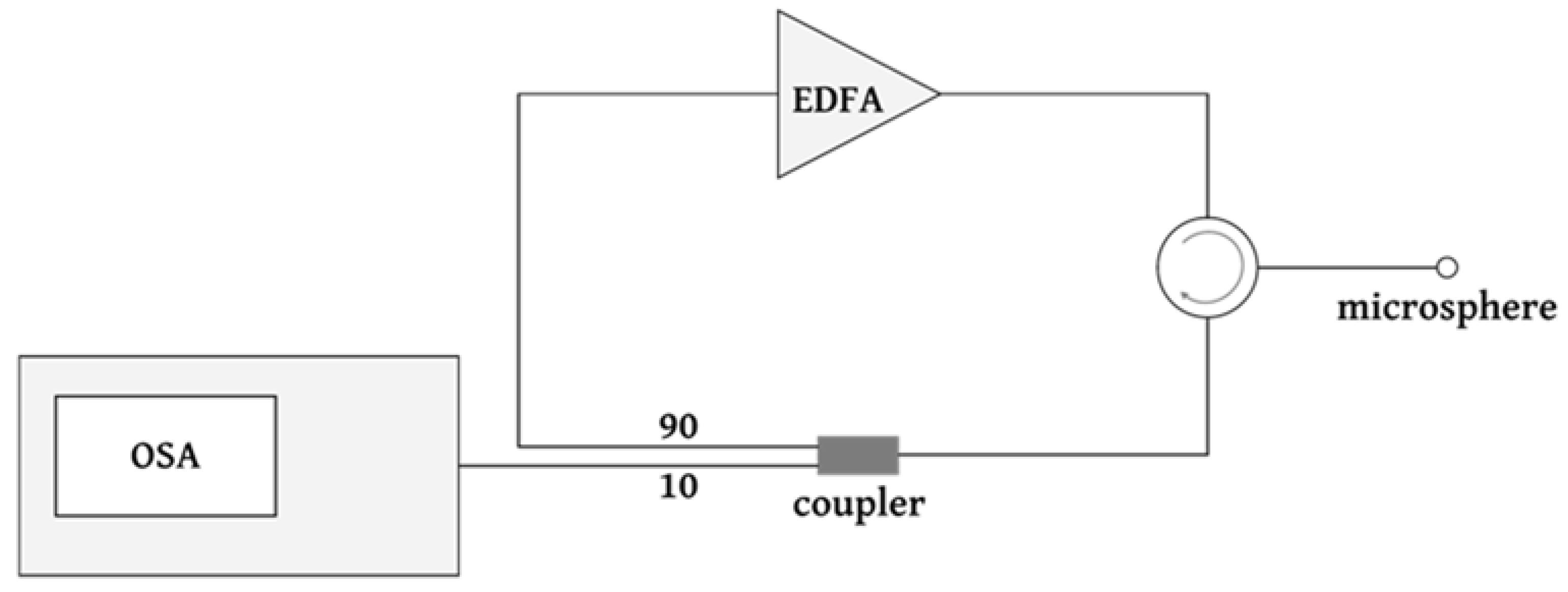
2. Materials and Methods
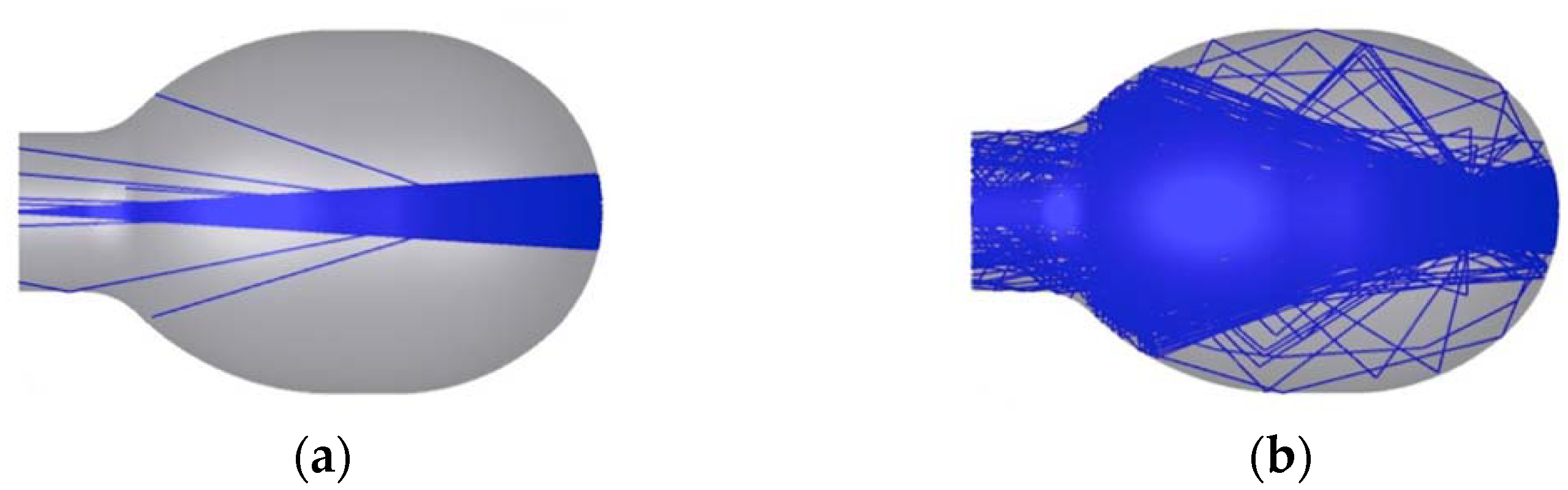
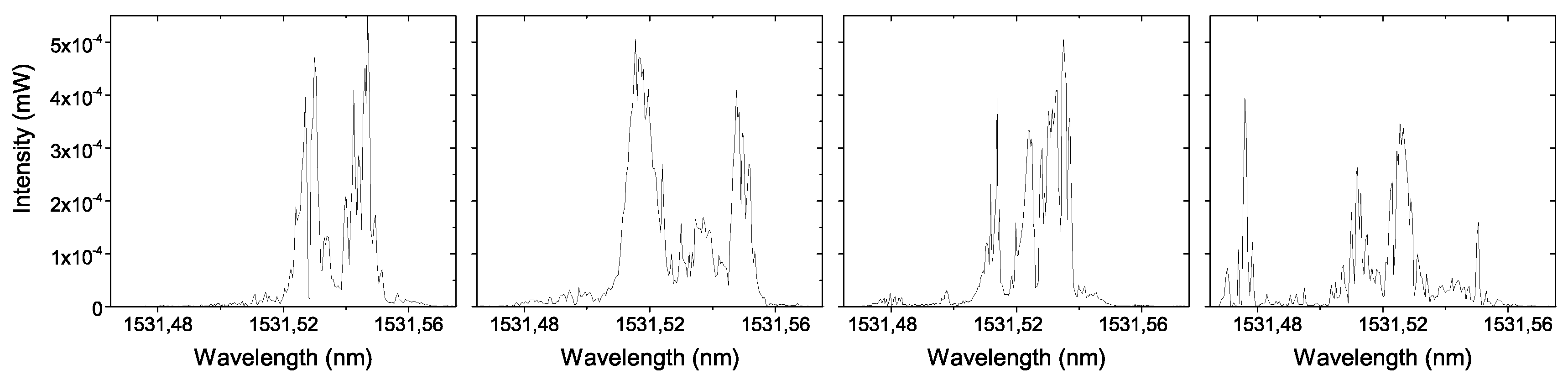
3. Results
4. Discussion and Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Letokhov, V.S. Generation of light by a scattering medium with negative resonance absorption. Zh. Eksp. Teor. Fiz. 1968, 53, 1442–1447. [Google Scholar]
- Andreasen, J.; Asatryan, A.A.; Botten, L.C.; Byrne, M.A.; Cao, H.; Ge, L.; Labonté, L.; Sebbah, P.; Stone, A.D.; Türeci, H.E.; et al. Modes of random lasers. Adv. Opt. Photonics 2011, 3, 88–127. [Google Scholar] [CrossRef]
- Wiersma, D.S. The physics and applications of random lasers. Nat. Phys. 2008, 4, 359–367. [Google Scholar] [CrossRef]
- Cao, H. Review on latest developments in random lasers with coherent feedback. J. Phys. A Math. Gen. 2005, 38, 10497. [Google Scholar] [CrossRef]
- Yang, L.; Yang, J.; Yang, Y.; Zhang, Z.; Wang, J.; Zhang, Z.; Xue, P.; Gong, Y.; Copner, N. Optical sensors using chaotic correlation fiber loop ring down. Opt. Express 2017, 25, 2031–2037. [Google Scholar] [CrossRef] [PubMed]
- Turitsyn, S.K.; Babin, S.A.; Churkin, D.V.; Vatnik, I.D.; Nikulin, M.; Podivilov, E.V. Random distributed feedback fibre lasers. Phys. Rep. 2014, 542, 133–193. [Google Scholar] [CrossRef]
- Churkin, D.V.; Sugavanam, S.; Vatnik, I.D.; Wang, Z.; Podivilov, E.V.; Babin, S.A.; Rao, Y.; Turitsyn, S.K. Recent advances in fundamentals and applications of random fiber lasers. Adv. Opt. Photonics 2015, 7, 516–569. [Google Scholar] [CrossRef]
- Argyris, A.; Syvridis, D.; Larger, L.; Annovazzi-Lodi, V.; Colet, P.; Fischer, I.; García-Ojalvo, J.; Mirasso, C.R.; Pesquera, L.; Shore, K.A. Chaos-based communications at high bit rates using commercial fibre-optic links. Nature 2005, 438, 343–346. [Google Scholar] [CrossRef] [PubMed]
- Uchida, A.; Amano, K.; Inoue, M.; Hirano, K.; Naito, S.; Someya, H.; Oowada, I.; Kurashige, T.; Shiki, M.; Yoshimori, S.; et al. Fast physical random bit generation with chaotic semiconductor lasers. Nat. Photonics 2008, 2, 728–732. [Google Scholar] [CrossRef]
- Redding, B.; Choma, M.A.; Cao, H. Spatial coherence of random laser emission. Opt. Lett. 2011, 36, 3404–3406. [Google Scholar] [CrossRef] [PubMed]
- Redding, B.; Choma, M.A.; Cao, H. Speckle-free laser imaging using random laser illumination. Nat. Photonics 2012, 6, 355–359. [Google Scholar] [CrossRef] [PubMed]
- Lahoz, F.; Martín, I.R.; Urgellés, M.; Marrero-Alonso, J.; Marín, R.; Saavedra, C.J.; Boto, A.; Díaz, M. Random laser in biological tissues impregnated with a fluorescent anticancer drug. Laser Phys. Lett. 2015, 12, 045805. [Google Scholar] [CrossRef]
- Markushev, V.M.; Zolin, V.F.; Briskina, C.M. Luminescence and stimulated emission of neodymium in sodium lanthanum molybdate powders. Quantum Electron. 1986, 16, 281–283. [Google Scholar] [CrossRef]
- Cao, H.; Zhao, Y.G.; Ho, S.T.; Seelig, E.W.; Wang, Q.H.; Chang, R.P.H. Random laser action in semiconductor powder. Phys. Rev. Lett. 1999, 82, 2278–2281. [Google Scholar] [CrossRef]
- Baudouin, Q.; Mercadier, N.; Guarrera, V.; Guerin, W.; Kaiser, R. A cold-atom random laser. Nat. Phys. 2013, 9, 357–360. [Google Scholar] [CrossRef]
- Doya, V.; Legrand, O.; Mortessagne, F.; Miniatura, C. Light scarring in an optical fiber. Phys. Rev. Lett. 2002, 88, 014102. [Google Scholar] [CrossRef] [PubMed]
- Lizárraga, N.; Puente, N.P.; Chaikina, E.I.; Leskova, T.A.; Méndez, E.R. Single-mode Er-doped fiber random laser with distributed Bragg grating feedback. Opt. Express 2009, 17, 395–404. [Google Scholar] [CrossRef] [PubMed]
- Quochi, F.; Cordella, F.; Mura, A.; Bongiovanni, G. Gain amplification and lasing properties of individual organic nanofibers. Appl. Phys. Lett. 2006, 88, 041106. [Google Scholar] [CrossRef]
- Bunimovich, L.A. On the ergodic properties of nowhere dispersing billiards. Commun. Math. Phys. 1979, 65, 295–312. [Google Scholar] [CrossRef]
- Shinohara, S.; Fukushima, T.; Harayama, T. Light emission patterns from stadium-shaped semiconductor microcavity lasers. Phys. Rev. A 2008, 77, 033807. [Google Scholar] [CrossRef]
- Lee, S.-B.; Lee, J.-H.; Chang, J.-S.; Moon, H.-J.; Kim, S.W.; An, K. Observation of Scarred Modes in Asymmetrically Deformed Microcylinder Lasers. Phys. Rev. Lett. 2002, 88, 033903. [Google Scholar] [CrossRef] [PubMed]
- Schwefel, H.G.L.; Rex, N.B.; Tureci, H.E.; Chang, R.K.; Stone, A.D.; Ben-Messaoud, T.; Zyss, J. Dramatic shape sensitivity of directional emission patterns from similarly deformed cylindrical polymer lasers. J. Opt. Soc. Am. B 2004, 21, 923–934. [Google Scholar] [CrossRef]
- Fukushima, T.; Harayama, T.; Davis, P.; Vaccaro, P.O.; Nishimura, T.; Aida, T. Quasi-stadium laser diodes with an unstable resonator condition. Opt. Lett. 2003, 28, 408–410. [Google Scholar] [CrossRef] [PubMed]
- Fukushima, T.; Harayama, T. Stadium and quasi-stadium laser diodes. IEEE Sel. Top. Quantum Electron. 2004, 10, 1039–1051. [Google Scholar]
- Chern, G.D.; Tureci, H.E.; Stone, A.D.; Chang, R.K.; Kneissl, M.; Johnson, N.M. Unidirectional lasing from InGaN multiple-quantum-well spiral-shaped micropillars. Appl. Phys. Lett. 2003, 83, 1710–1712. [Google Scholar] [CrossRef]
- Wiersig, J.; Hentschel, M. Unidirectional light emission from high-Q modes in optical microcavities. Phys. Rev. A 2006, 73, 031802. [Google Scholar] [CrossRef]
- Wiersig, J.; Hentschel, M. Combining directional light output and ultralow loss in deformed microdisks. Phys. Rev. Lett. 2008, 100, 033901. [Google Scholar] [CrossRef] [PubMed]
- Kurdoglyan, M.S.; Lee, S.Y.; Rim, S.; Kim, C.-M. Unidirectional lasing from a microcavity with a rounded isoceles triangle shape. Opt. Lett. 2004, 29, 2758–2760. [Google Scholar] [CrossRef] [PubMed]
- Kim, M.W.; Yi, C.H.; Rim, S.; Kim, C.M.; Kim, J.H.; Oh, K.R. Directional single mode emission in a microcavity laser. Opt. Express 2012, 20, 13651–13656. [Google Scholar] [CrossRef] [PubMed]
- Fang, W.; Cao, H.; Solomon, G.S. Control of lasing in fully chaotic open microcavities by tailoring the shape factor. Appl. Phys. Lett. 2007, 90, 081108. [Google Scholar] [CrossRef]
- Park, H.G.; Qian, F.; Barrelet, C.J.; Li, Y. Microstadium single-nanowire laser. Appl. Phys. Lett. 2007, 91, 251115. [Google Scholar]
- Antenucci, F.; Crisanti, A.; Leuzzi, L. The glassy random laser: Replica symmetry breaking in the intensity fluctuations of emission spectra. Sci. Rep. 2015, 5, 16792. [Google Scholar] [PubMed]

© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Silveira, B.; Gomes, A.; Becker, M.; Schneidewind, H.; Frazão, O. Bunimovich Stadium-Like Resonator for Randomized Fiber Laser Operation. Photonics 2018, 5, 17. https://doi.org/10.3390/photonics5030017
Silveira B, Gomes A, Becker M, Schneidewind H, Frazão O. Bunimovich Stadium-Like Resonator for Randomized Fiber Laser Operation. Photonics. 2018; 5(3):17. https://doi.org/10.3390/photonics5030017
Chicago/Turabian StyleSilveira, Beatriz, André Gomes, Martin Becker, Henrik Schneidewind, and Orlando Frazão. 2018. "Bunimovich Stadium-Like Resonator for Randomized Fiber Laser Operation" Photonics 5, no. 3: 17. https://doi.org/10.3390/photonics5030017
APA StyleSilveira, B., Gomes, A., Becker, M., Schneidewind, H., & Frazão, O. (2018). Bunimovich Stadium-Like Resonator for Randomized Fiber Laser Operation. Photonics, 5(3), 17. https://doi.org/10.3390/photonics5030017





