Graphene Plasmonic Waveguides for Mid-Infrared Supercontinuum Generation on a Chip
Centre for Photonics and Photonic Materials, Department of Physics, University of Bath, Bath BA27AY, UK
Photonics 2015, 2(3), 825-837; https://doi.org/10.3390/photonics2030825
Received: 1 July 2015 / Revised: 17 July 2015 / Accepted: 20 July 2015 / Published: 23 July 2015
(This article belongs to the Special Issue New Frontiers in Plasmonics and Metamaterials)
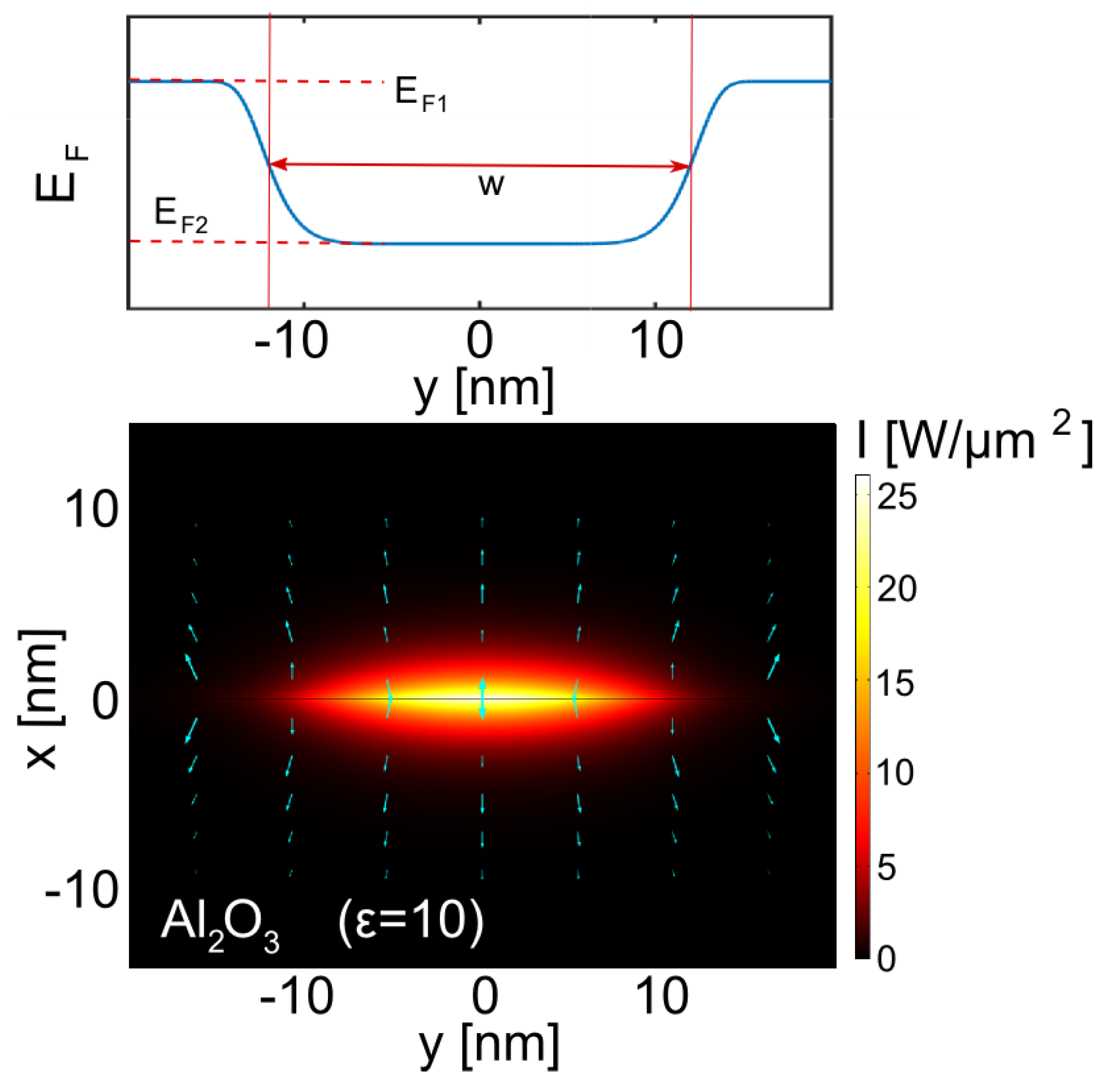
Using perturbation expansion of Maxwell equations with the nonlinear boundary condition, a generic propagation equation is derived to describe nonlinear effects, including spectral broadening of pulses, in graphene surface plasmon (GSP) waveguides. A considerable spectral broadening of an initial 100 fs pulse with 0.5 mW peak power in a 25 nm wide and 150 nm long waveguide is demonstrated. The generated supercontinuum covers the spectral range from 6 μm to 13 μm .
View Full-Text
Keywords:
graphene plasmonics; nonlinear waveguides; frequency conversion; optical supercontinuum; surface plasmon polaritons
▼
Show Figures
Figure 1
This is an open access article distributed under the Creative Commons Attribution License which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited
- Supplementary File 1:
Supplementary Materials (ZIP, 2129 KiB)
MDPI and ACS Style
Gorbach, A.V. Graphene Plasmonic Waveguides for Mid-Infrared Supercontinuum Generation on a Chip. Photonics 2015, 2, 825-837.
AMA Style
Gorbach AV. Graphene Plasmonic Waveguides for Mid-Infrared Supercontinuum Generation on a Chip. Photonics. 2015; 2(3):825-837.
Chicago/Turabian StyleGorbach, Andrey V. 2015. "Graphene Plasmonic Waveguides for Mid-Infrared Supercontinuum Generation on a Chip" Photonics 2, no. 3: 825-837.
Find Other Styles
Search more from Scilit
