Photo-Ionization of Noble Gases: A Demonstration of Hybrid Coupled Channels Approach
Abstract
:1. Introduction
2. Hybrid Coupled Channels Method
3. Results
3.1. One- and Two-Photon Cross-Sections of Neon

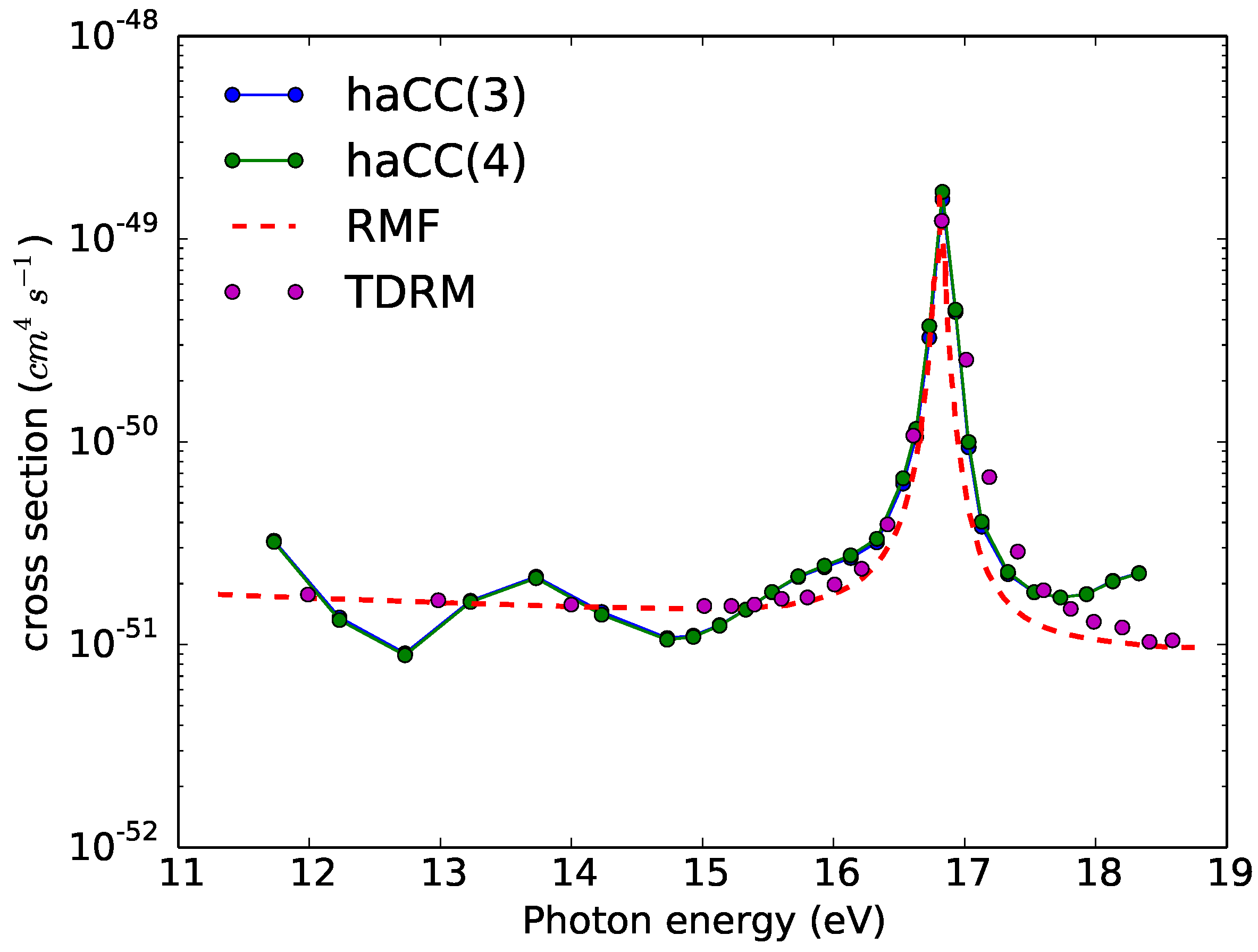
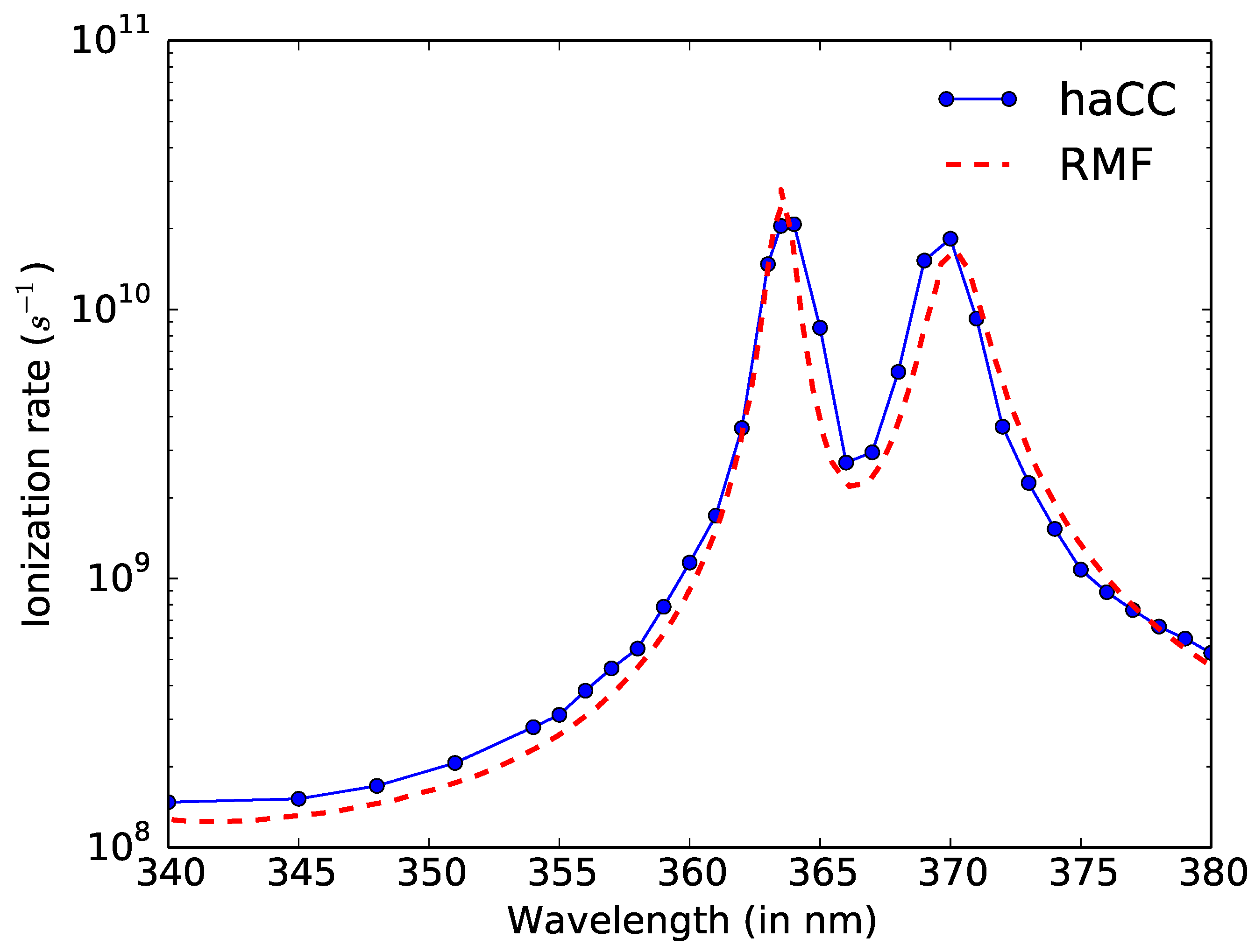
3.2. Five-Photon Ionization Rates from Argon
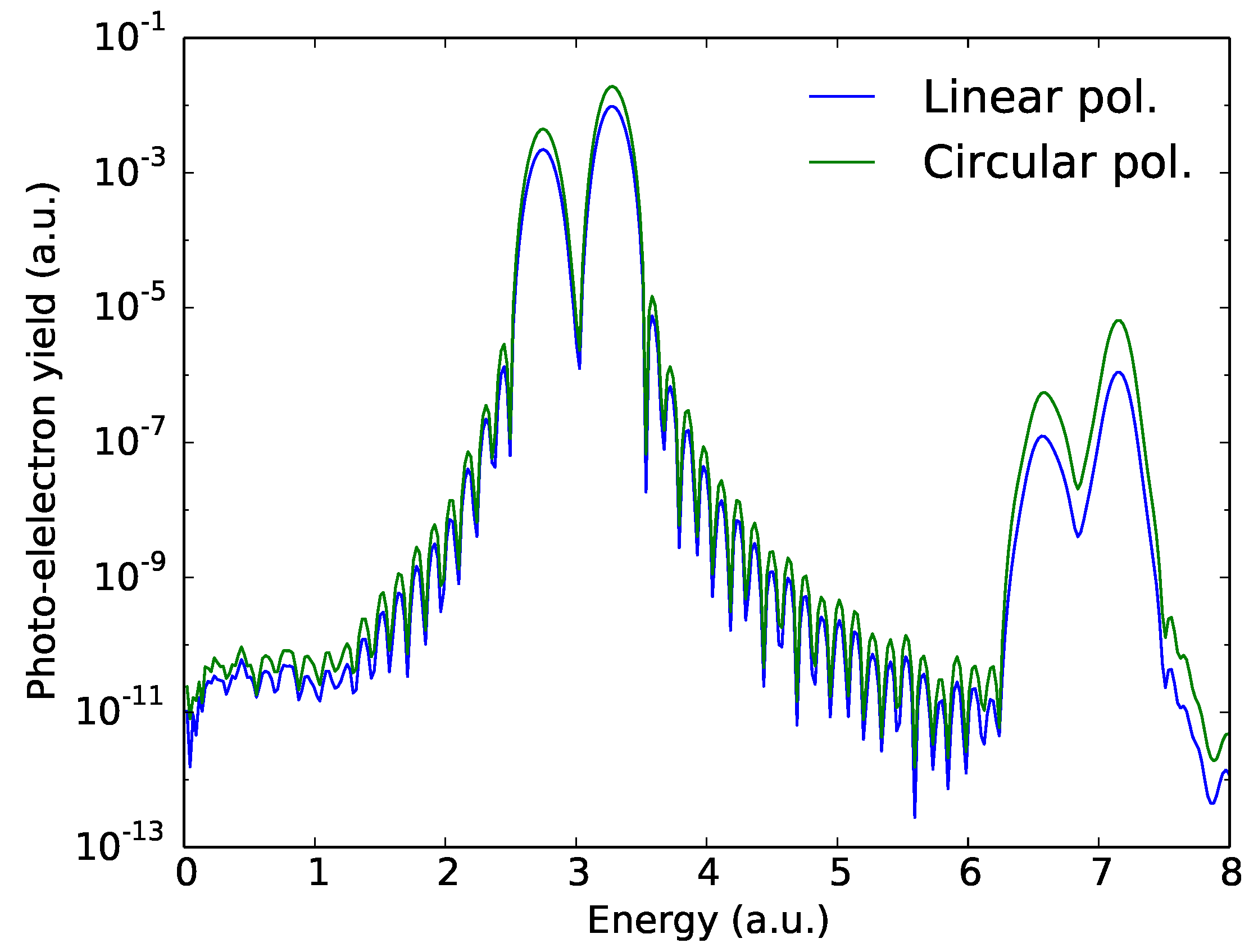
3.3. Photo-Electron Spectra from Argon with 12 nm Wavelength Laser Fields
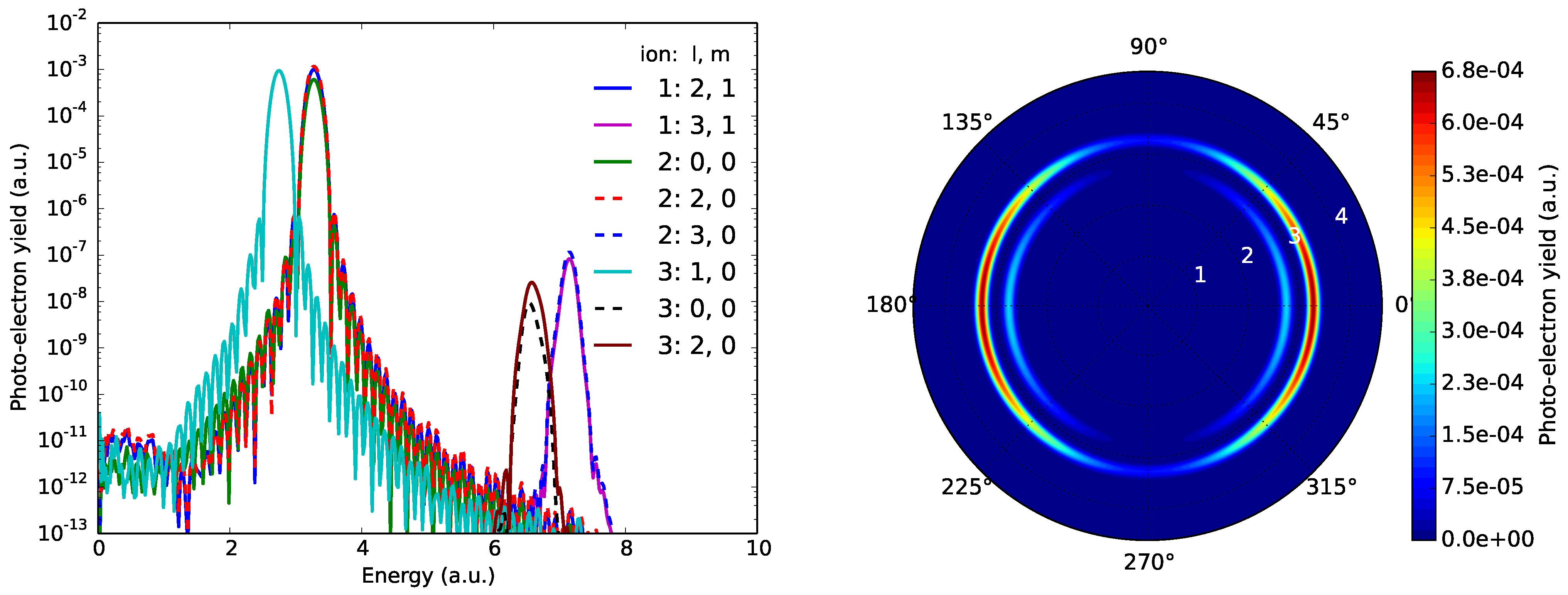
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Feldhaus, J.; Krikunova, M.; Meyer, M.; Möller, T.; Moshammer, R.; Rudenko, A.; Tschentscher, T.; Ullrich, J. AMO science at the FLASH and European XFEL free-electron laser facilities. J. Phys. B Atomic Mol. Opt. Phys. 2013, 46, 164002. [Google Scholar] [CrossRef]
- Goulielmakis, E.; Loh, Z.H.; Wirth, A.; Santra, R.; Rohringer, N.; Yakovlev, V.S.; Zherebtsov, S.; Pfeifer, T.; Azzeer, A.M.; Kling, M.F.; et al. Real-time observation of valence electron motion. Nature 2010, 466, 739–743. [Google Scholar] [CrossRef] [PubMed]
- Wörner, H.; Niikura, H.; Bertrand, J.; Corkum, P.; Villeneuve, D. Observation of Electronic Structure Minima in High-Harmonic Generation. Phys. Rev. Lett. 2009, 102, 103901. [Google Scholar] [CrossRef] [PubMed]
- Bhardwaj, S.; Son, S.K.; Hong, K.H.; Lai, C.J.; Kärtner, F.; Santra, R. Recombination-amplitude calculations of noble gases, in both length and acceleration forms, beyond the strong-field approximation. Phys. Rev. A 2013, 88, 053405. [Google Scholar] [CrossRef]
- Schultze, M.; Fieß, M.; Karpowicz, N.; Gagnon, J.; Korbman, M.; Hofstetter, M.; Neppl, S.; Cavalieri, A.L.; Komninos, Y.; Mercouris, T.; et al. Delay in Photoemission. Science 2010, 328, 1658–1662. [Google Scholar] [CrossRef] [PubMed]
- Moore, L.; Lysaght, M.; Parker, J.; van der Hart, H.; Taylor, K. Time delay between photoemission from the 2p and 2s subshells of neon. Phys. Rev. A 2011, 84, 061404. [Google Scholar] [CrossRef]
- Saenz, A.; Lambropoulos, P. Theoretical two-, three- and four-photon ionization cross sections of helium in the XUV range. J. Phys. B Atomic Mol. Opt. Phys. 1999, 32, 5629. [Google Scholar] [CrossRef]
- Van der Hart, H.W.; Bingham, P. Two- and three-photon ionization of He between 1013 and 1014 W/cm2. J. Phys. B Atomic Mol. Opt. Phys. 2005, 38, 207. [Google Scholar] [CrossRef]
- Caillat, J.; Zanghellini, J.; Kitzler, M.; Koch, O.; Kreuzer, W.; Scrinzi, A. Correlated multielectron systems in strong laser fields: A multiconfiguration time-dependent Hartree-Fock approach. Phys. Rev. A 2005, 71, 012712. [Google Scholar] [CrossRef]
- Greenman, L.; Ho, P.J.; Pabst, S.; Kamarchik, E.; Mazziotti, D.A.; Santra, R. Implementation of the time-dependent configuration-interaction singles method for atomic strong-field processes. Phys. Rev. A 2010, 82, 023406. [Google Scholar] [CrossRef]
- Hochstuhl, D.; Bonitz, M. Time-dependent restricted-active-space configuration-interaction method for the photoionization of many-electron atoms. Phys. Rev. A 2012, 86, 053424. [Google Scholar] [CrossRef]
- Lysaght, M.A.; van der Hart, H.W.; Burke, P.G. Time-dependent R-matrix theory for ultrafast atomic processes. Phys. Rev. A 2009, 79, 053411. [Google Scholar] [CrossRef]
- Spanner, M.; Patchkovskii, S. One-electron ionization of multielectron systems in strong nonresonant laser fields. Phys. Rev. A 2009, 80, 063411. [Google Scholar] [CrossRef]
- Van der Hart, H.W. Ionization rates for He, Ne, and Ar subjected to laser light with wavelengths between 248.6 and 390 nm. Phys. Rev. A 2006, 73, 023417. [Google Scholar] [CrossRef]
- Van der Hart, H.W.; Lysaght, M.A.; Burke, P.G. Time-dependent multielectron dynamics of Ar in intense short laser pulses. Phys. Rev. A 2007, 76, 043405. [Google Scholar] [CrossRef]
- Majety, V.P.; Zielinski, A.; Scrinzi, A. Photoionization of few electron systems with a hybrid Coupled Channels approach. 2014. [Google Scholar] [CrossRef]
- Tao, L.; Scrinzi, A. Photo-electron momentum spectra from minimal volumes: The time-dependent surface flux method. New J. Phys. 2012, 14, 013021. [Google Scholar] [CrossRef]
- Scrinzi, A. t -SURFF: Fully differential two-electron photo-emission spectra. New J. Phys. 2012, 14, 085008. [Google Scholar] [CrossRef]
- Lischka, H.; Müller, T.; Szalay, P.G.; Shavitt, I.; Pitzer, R.M.; Shepard, R. Columbus—A program system for advanced multireference theory calculations. WIREs Comput. Mol. Sci. 2011, 1, 191–199. [Google Scholar] [CrossRef]
- Majety, V.P.; Zielinski, A.; Scrinzi, A. Mixed gauge in strong laser-matter interaction. J. Phys. B Atomic Mol. Opt. Phys. 2015, 48, 025601. [Google Scholar] [CrossRef]
- Scrinzi, A. Infinite-range exterior complex scaling as a perfect absorber in time-dependent problems. Phys. Rev. A 2010, 81, 053845. [Google Scholar] [CrossRef]
- Samson, J.; Stolte, W. Precision measurements of the total photoionization cross-sections of He, Ne, Ar, Kr, and Xe. J. Electron Spectrosc. Relat. Phenom. 2002, 123, 265–276. [Google Scholar] [CrossRef]
- Karamatskou, A.; Pabst, S.; Chen, Y.J.; Santra, R. Calculation of photoelectron spectra within the time-dependent configuration-interaction singles scheme. Phys. Rev. A 2014, 89, 033415. [Google Scholar] [CrossRef]
© 2015 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Majety, V.P.; Scrinzi, A. Photo-Ionization of Noble Gases: A Demonstration of Hybrid Coupled Channels Approach. Photonics 2015, 2, 93-103. https://doi.org/10.3390/photonics2010093
Majety VP, Scrinzi A. Photo-Ionization of Noble Gases: A Demonstration of Hybrid Coupled Channels Approach. Photonics. 2015; 2(1):93-103. https://doi.org/10.3390/photonics2010093
Chicago/Turabian StyleMajety, Vinay Pramod, and Armin Scrinzi. 2015. "Photo-Ionization of Noble Gases: A Demonstration of Hybrid Coupled Channels Approach" Photonics 2, no. 1: 93-103. https://doi.org/10.3390/photonics2010093
APA StyleMajety, V. P., & Scrinzi, A. (2015). Photo-Ionization of Noble Gases: A Demonstration of Hybrid Coupled Channels Approach. Photonics, 2(1), 93-103. https://doi.org/10.3390/photonics2010093



