Ultra-Wideband Cross-Polarization Converter Using Metasurface Operating in the X- and K-Band
Abstract
1. Introduction
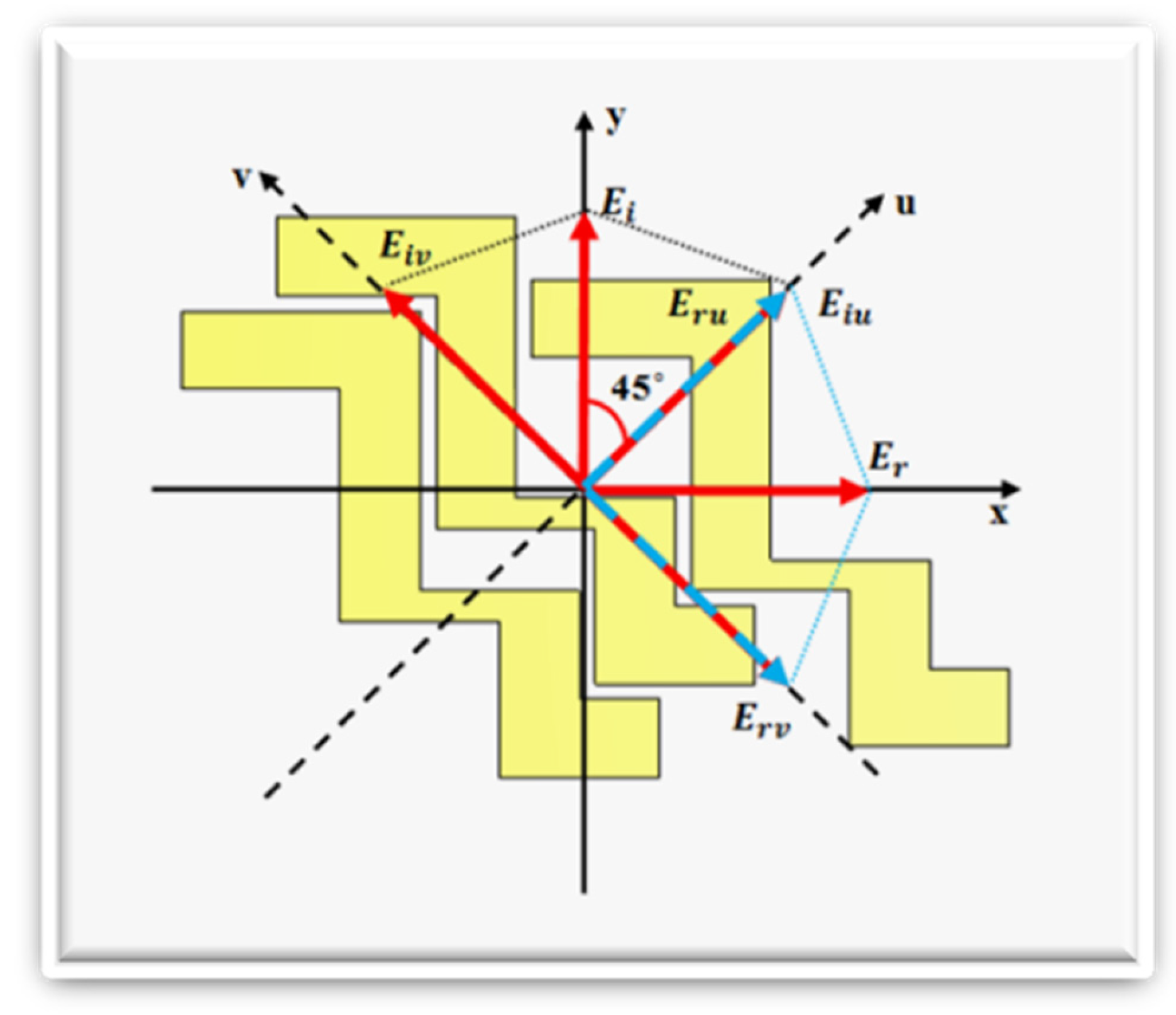
2. Basic Theory
Design and Simulation
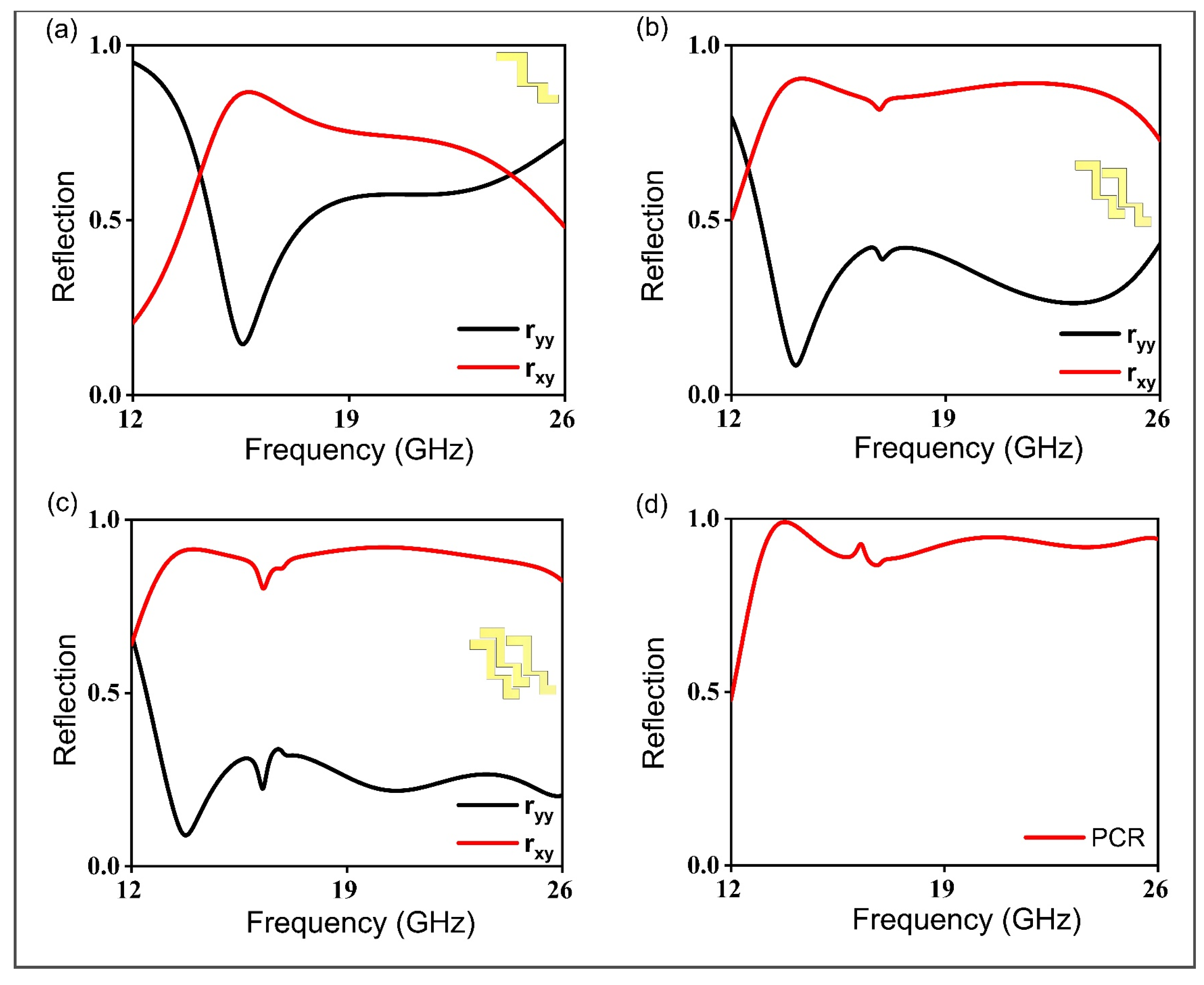
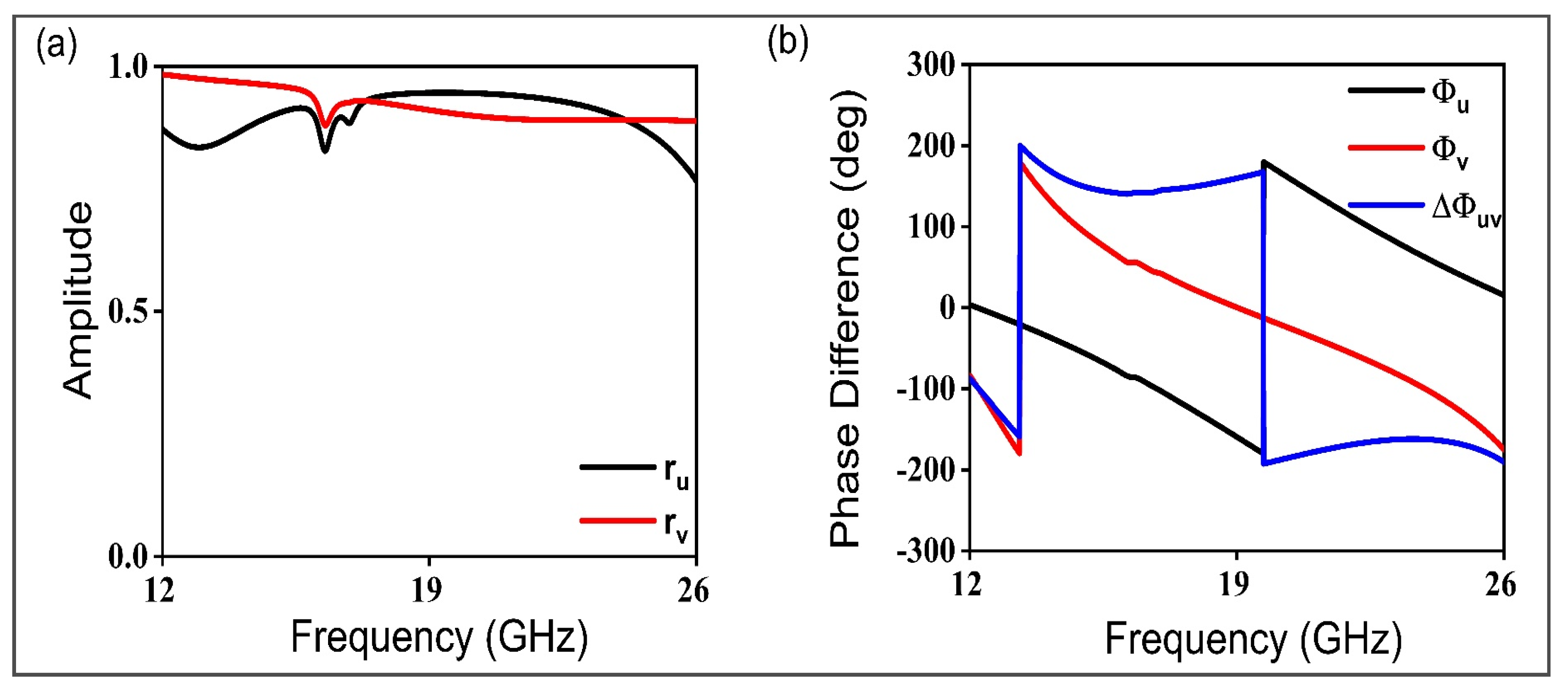
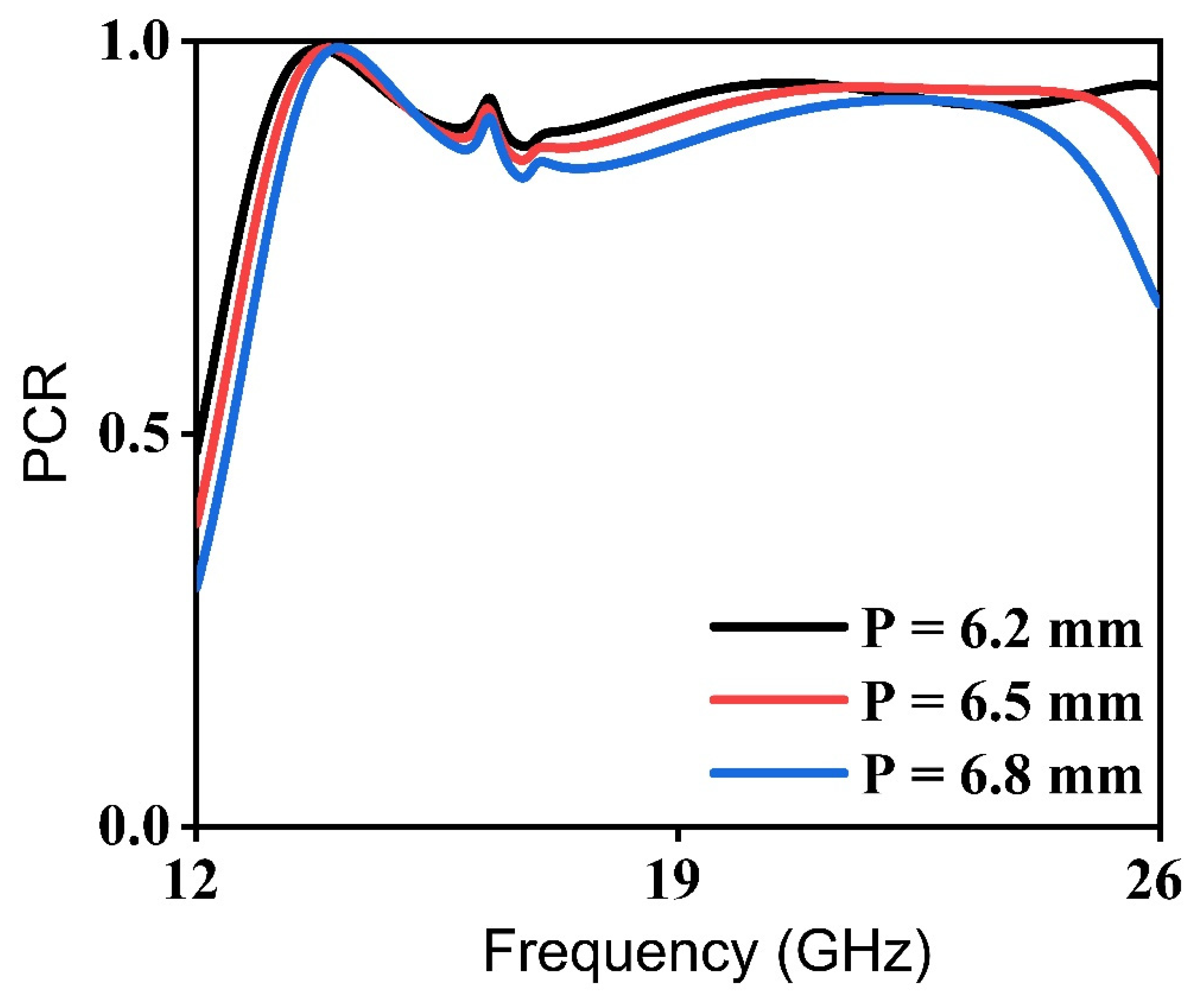
3. Results and Discussion
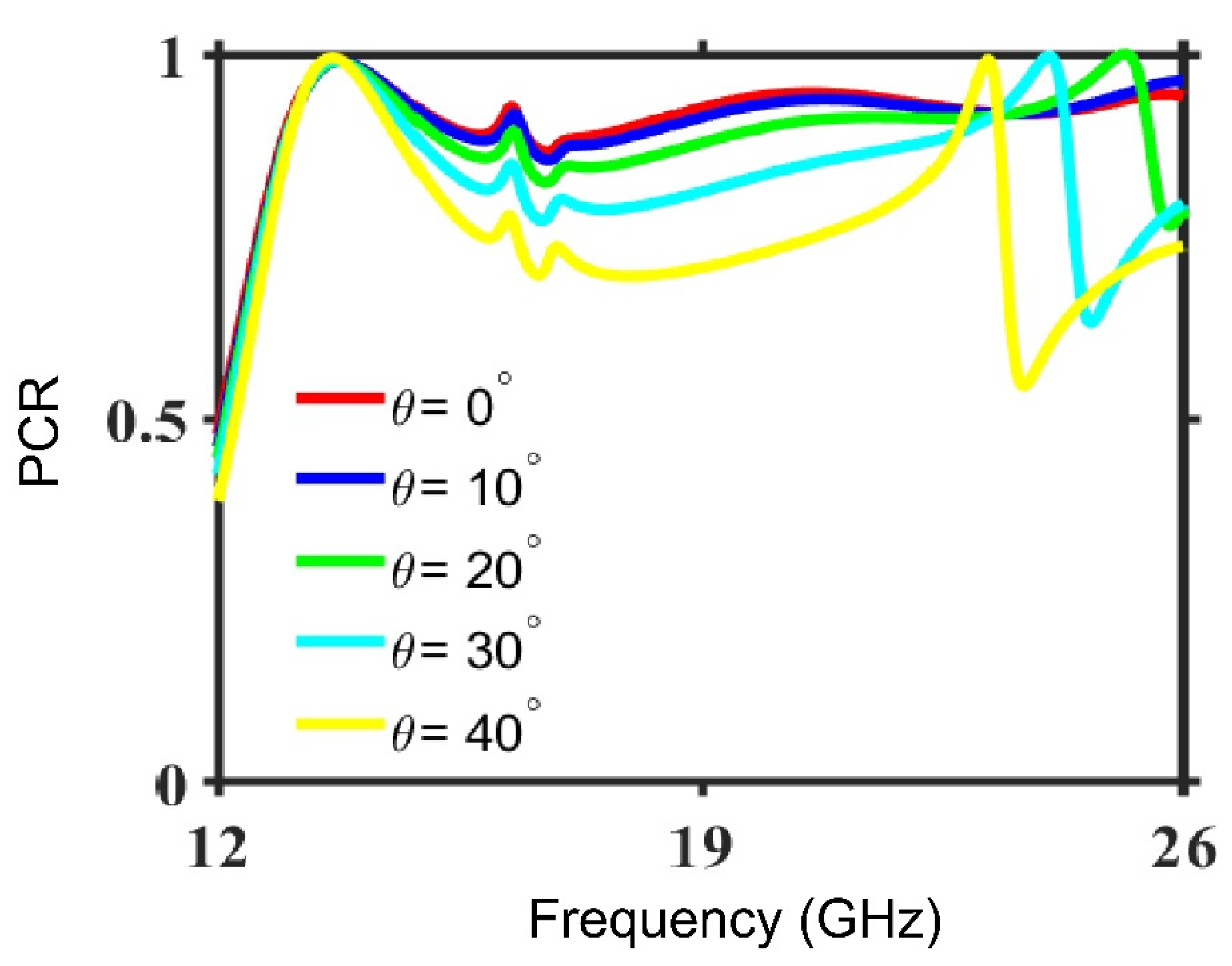
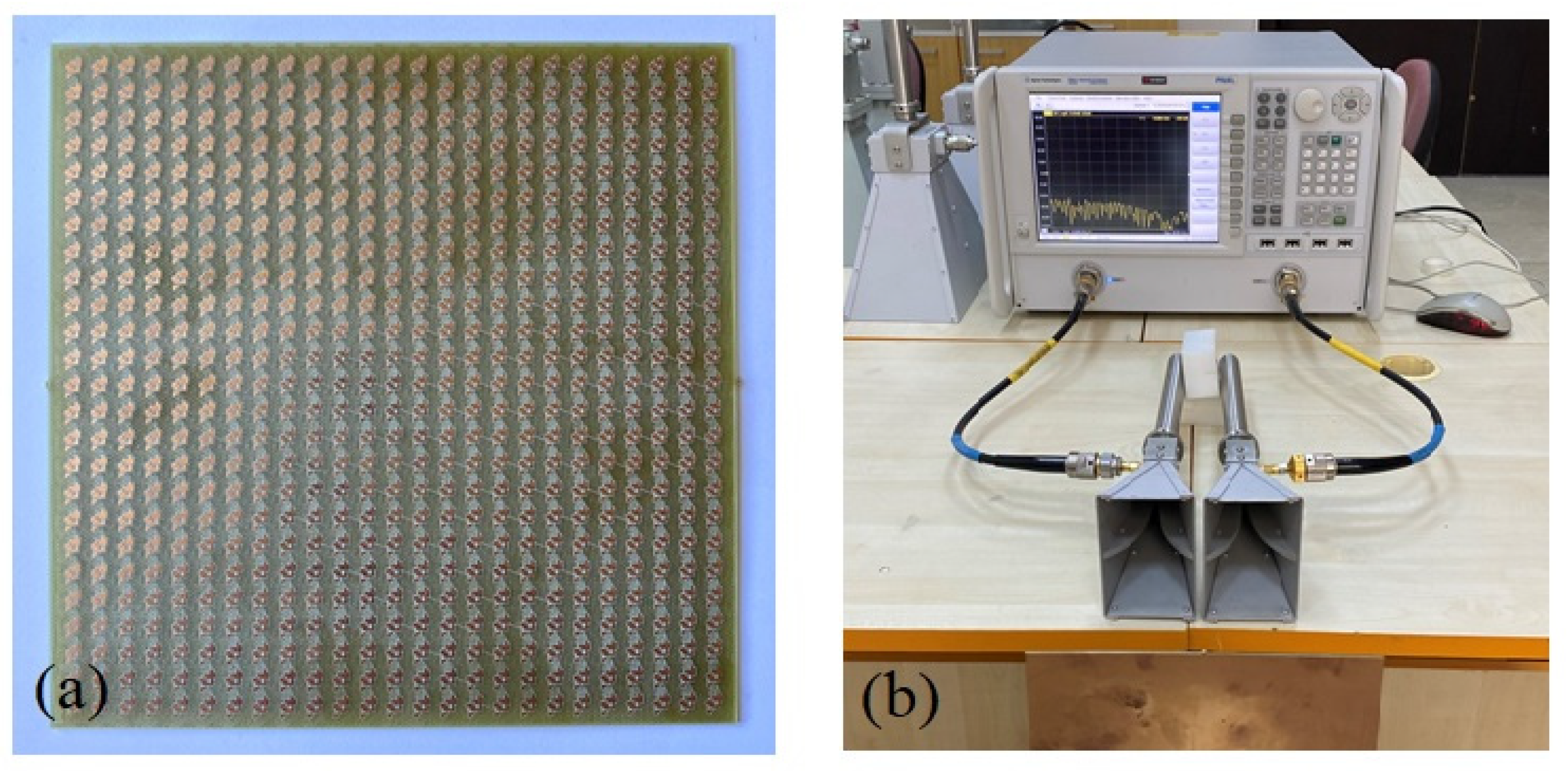
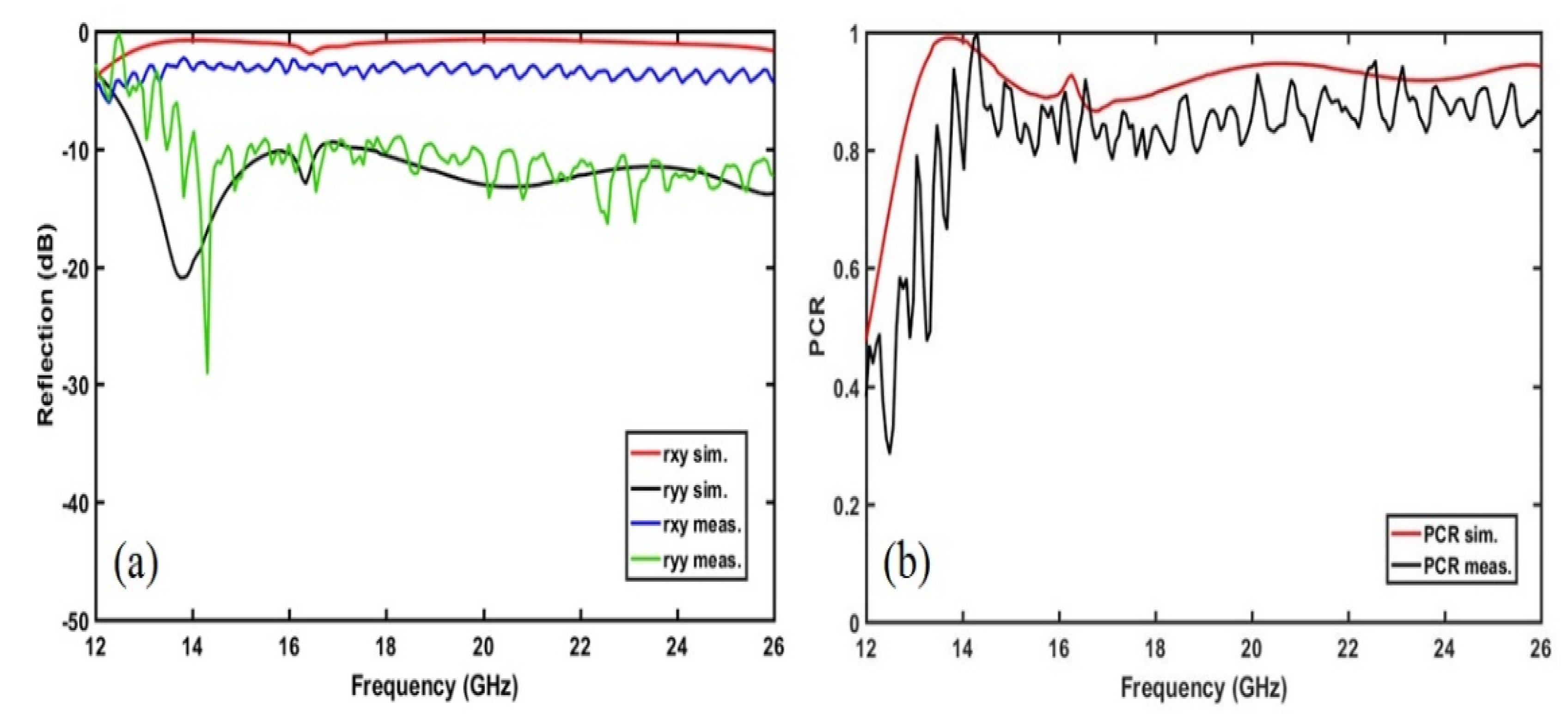
4. Experimental Results
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Nicholls, L.H.; Rodríguez-Fortuño, F.J.; Nasir, M.E.; Córdova-Castro, R.M.; Olivier, N.; Wurtz, G.A.; Zayats, A.V. Ultrafast synthesis and switching of light polarization in nonlinear anisotropic metamaterials. Nat. Photonics 2017, 11, 628–633. [Google Scholar] [CrossRef]
- Arismar, C.S.; Da Costa, I.; Pinna, S.; Melo, S.; Laghezza, F.; Scotti, F.; Ghelfi, P.; Spadoti, D.; Bogoni, A. A novel dual-polarization and dual-band slotted waveguide antenna array for dual-use radars. In Proceedings of the 2016 10th European Conference on Antennas and Propagation (EuCAP), Davos, Switzerland, 10–15 April 2016; IEEE: New York, NY, USA, 2016; pp. 1–4. [Google Scholar]
- Wu, Z.; Li, L.; Li, Y.; Chen, X. Metasurface superstrate antenna with wideband circular polarization for satellite communication application. IEEE Antennas Wirel. Propag. Lett. 2015, 15, 374–377. [Google Scholar] [CrossRef]
- Qi, Y.; Zhang, B.; Liu, C.; Deng, X. Ultra-broadband polarization conversion meta-surface and its application in polarization converter and rcs reduction. IEEE Access 2020, 8, 116675–116684. [Google Scholar] [CrossRef]
- Beruete, M.; Navarro-Cía, M.; Sorolla, M.; Campillo, I. Polarization selection with stacked hole array metamaterial. J. Appl. Phys. 2008, 103, 053102. [Google Scholar] [CrossRef]
- Pors, A.; Nielsen, M.G.; Bozhevolnyi, S.I. Broadband plasmonic half-wave plates in reflection. Opt. Lett. 2013, 38, 513–515. [Google Scholar] [CrossRef]
- Ren, L.-S.; Jiao, Y.-C.; Li, F.; Zhao, J.-J.; Zhao, G. A dual-layer t-shaped element for broadband circularly polarized reflect array with linearly polarized feed. IEEE Antennas Wirel. Propag. Lett. 2011, 10, 407–410. [Google Scholar]
- Vasić, B.; Zografopoulos, D.C.; Isić, G.; Beccherelli, R.; Gajić, R. Electrically tunable terahertz polarization converter based on overcoupled metal-isolator-metal metamaterials infiltrated with liquid crystals. Nanotechnology 2017, 28, 124002. [Google Scholar] [CrossRef]
- Chen, P.; Ji, W.; Wei, B.-Y.; Hu, W.; Chigrinov, V.; Lu, Y.-Q. Generation of arbitrary vector beams with liquid crystal polarization converters and vector-photoaligned q-plates. Appl. Phys. Lett. 2015, 107, 241102. [Google Scholar] [CrossRef]
- Li, L.; Cui, T.J.; Ji, W.; Liu, S.; Ding, J.; Wan, X.; Li, Y.B.; Jiang, M.; Qiu, C.-W.; Zhang, S. Electromagnetic reprogrammable coding-metasurface holograms. Nat. Commun. 2017, 8, 197. [Google Scholar] [CrossRef]
- Yu, N.; Capasso, F. Flat optics with designer metasurfaces. Nat. Mater. 2014, 13, 139–150. [Google Scholar] [CrossRef]
- Akram, M.R.; Ding, G.; Chen, K.; Feng, Y.; Zhu, W. Ultrathin single layer metasurfaces with ultra-wideband operation for both transmission and reflection. Adv. Mater. 2020, 32, 1907308. [Google Scholar] [CrossRef] [PubMed]
- Zhang, J.; Wei, X.; Rukhlenko, I.D.; Chen, H.-T.; Zhu, W. Electrically tunable metasurface with independent frequency and amplitude modulations. ACS Photonics 2019, 7, 265–271. [Google Scholar] [CrossRef]
- Liu, B.; Zhu, W.; Gunapala, S.D.; Stockman, M.I.; Premaratne, M. Open resonator electric spaser. ACS Nano 2017, 11, 12573–12582. [Google Scholar] [CrossRef]
- Lin, D.; Fan, P.; Hasman, E.; Brongersma, M.L. Dielectric gradient metasurface optical elements. Science 2014, 345, 298–302. [Google Scholar] [CrossRef]
- High, A.A.; Devlin, R.C.; Dibos, A.; Polking, M.; Wild, D.S.; Perczel, J.; De Leon, N.P.; Lukin, M.D.; Park, H. Visible-frequency hyperbolic metasurface. Nature 2015, 522, 192–196. [Google Scholar] [CrossRef]
- West, P.R.; Stewart, J.L.; Kildishev, A.V.; Shalaev, V.M.; Shkunov, V.V.; Strohkendl, F.; Zakharenkov, Y.A.; Dodds, R.K.; Byren, R. All-dielectric subwavelength metasurface focusing lens. Opt. Express 2014, 22, 26212–26221. [Google Scholar] [CrossRef]
- Wen, J.; Chen, L.; Yu, B.; Nieder, J.B.; Zhuang, S.; Zhang, D.; Lei, D. All-dielectric synthetic-phase metasurfaces generating practical airy beams. ACS Nano 2021, 15, 1030–1038. [Google Scholar] [CrossRef]
- Tang, T.; Kanwal, S.; Lu, Y.; Li, Y.; Wu, S.; Chen, L.; Qian, Z.; Xie, Z.; Wen, J.; Zhang, D. 3D nano-printed geometric phase metasurfaces for generating accelerating beams with complex amplitude manipulation. Sci. China Phys. Mech. Astron. 2024, 67, 264211. [Google Scholar] [CrossRef]
- Wen, J.; Chen, L.; Chen, X.; Kanwal, S.; Zhang, L.; Zhuang, S.; Zhang, D.; Lei, D. Use of dielectric metasurfaces to generate deep-subwavelength nondiffractive bessel-like beams with arbitrary trajectories and ultralarge deflection. Laser Photonics Rev. 2021, 15, 2000487. [Google Scholar] [CrossRef]
- Lei, C.; Kanwal, S.; Yu, B.; Feng, J.; Tao, C.; Wen, J.; Zhang, D. Generation of high-uniformity and high-resolution Bessel beam arrays through all-dielectric metasurfaces. Nanophotonics 2022, 11, 967–977. [Google Scholar]
- Lin, B.-Q.; Guo, J.-X.; Huang, B.-G.; Fang, L.-B.; Chu, P.; Liu, X.-W. Wideband linear-to-circular polarization conversion realized by a transmissive anisotropic metasurface. Chin. Phys. B 2018, 27, 054204. [Google Scholar] [CrossRef]
- Chin, J.Y.; Lu, M.; Cui, T.J. Metamaterial polarizers by electric-field-coupled resonators. Appl. Phys. Lett. 2008, 93, 251903. [Google Scholar] [CrossRef]
- Singh, R.; Plum, E.; Menzel, C.; Rockstuhl, C.; Azad, A.; Cheville, R.; Lederer, F.; Zhang, W.; Zheludev, N. Terahertz metamaterial with asymmetric transmission. Phys. Rev. B 2009, 80, 153104. [Google Scholar] [CrossRef]
- Menzel, C.; Helgert, C.; Rockstuhl, C.; Kley, E.-B.; Tünnermann, A.; Pertsch, T.; Lederer, F. Asymmetric transmission of linearly polarized light at optical metamaterials. Phys. Rev. Lett. 2010, 104, 253902. [Google Scholar] [CrossRef] [PubMed]
- Ding, X.; Monticone, F.; Zhang, K.; Zhang, L.; Gao, D.; Burokur, S.N.; de Lustrac, A.; Wu, Q.; Qiu, C.-W.; Alù, A. Ultrathin pancharatnam–berry metasurface with maximal cross-polarization efficiency. Adv. Mater. 2015, 27, 1195–1200. [Google Scholar] [CrossRef]
- Ye, Y.; He, S. 90° polarization rotator using a bilayered chiral metamaterial with giant optical activity. Phys. Rev. Lett. 2010, 96, 203501. [Google Scholar] [CrossRef]
- Shi, H.; Zhang, A.; Zheng, S.; Li, J.; Jiang, Y. Dual-band polarization angle independent 90 polarization rotator using twisted electric-field-coupled resonators. Phys. Rev. Lett. 2014, 104, 034102. [Google Scholar] [CrossRef]
- Wang, S.-Y.; Liu, W.; Geyi, W. Dual-band transmission polarization converter based on planar-dipole pair frequency selective surface. Sci. Rep. 2018, 8, 3791. [Google Scholar] [CrossRef]
- Liu, Y.; Xia, S.; Shi, H.; Zhang, A.; Xu, Z. Dual-band and high-efficiency polarization converter based on metasurfaces at microwave frequencies. Appl. Phys. B 2016, 122, 178. [Google Scholar] [CrossRef]
- Huang, X.; Yang, D.; Yang, H. Multiple-band reflective polarization converter using u-shaped metamaterial. J. Appl. Phys. 2014, 115, 103505. [Google Scholar] [CrossRef]
- Jia, Y.; Liu, Y.; Guo, Y.J.; Li, K.; Gong, S.-X. Broadband polarization rotation reflective surfaces and their applications to rcs reduction. IEEE Trans. Antennas Propag. 2015, 64, 179–188. [Google Scholar] [CrossRef]
- Gao, X.; Yang, W.L.; Ma, H.F.; Cheng, Q.; Yu, X.H.; Cui, T.J. A reconfigurable broadband polarization converter based on an active metasurface. IEEE Trans. Antennas Propag. 2018, 66, 6086–6095. [Google Scholar] [CrossRef]
- Shi, H.; Li, J.; Zhang, A.; Wang, J.; Xu, Z. Broadband cross polarization converter using plasmon hybridizations in a ring/disk cavity. Opt. Express 2014, 22, 20973–20981. [Google Scholar] [CrossRef] [PubMed]
- Gao, X.; Han, X.; Cao, W.-P.; Li, H.O.; Ma, H.F.; Cui, T.J. Ultrawideband and high-efficiency linear polarization converter based on double v-shaped metasurface. IEEE Trans. Antennas Propag. 2015, 63, 3522–3530. [Google Scholar] [CrossRef]
- Mei, Z.L.; Ma, X.M.; Lu, C.; Zhao, Y.D. High-efficiency and wide-bandwidth linear polarization converter based on double u-shaped metasurface. AIP Adv. 2017, 7, 125323. [Google Scholar] [CrossRef]
- Yang, J.J.; Cheng, Y.Z.; Ge, C.C.; Gong, R.Z. Broadband polarization conversion metasurface based on metal cut-wire structure for radar cross section reduction. Materials 2018, 11, 626. [Google Scholar] [CrossRef]
- Rubin, N.A.; Zaidi, A.; Dorrah, A.H.; Shi, Z.; Capasso, F. Jones matrix holography with metasurfaces. Sci. Adv. 2021, 7, eabg7488. [Google Scholar] [CrossRef]
- Zheng, C.; Liu, J.; Li, H.; Wang, M.; Zang, H.; Zhang, Y.; Yao, J. Terahertz metasurface polarization detection employing vortex pattern recognition. Photonics Res. 2023, 11, 2256–2263. [Google Scholar] [CrossRef]
- Zheng, C.; Li, H.; Liu, J.; Wang, M.; Zang, H.; Zhang, Y.; Yao, J. Full-Stokes metasurface polarimetry requiring only a single measurement. Photonics Res. 2024, 12, 514–521. [Google Scholar] [CrossRef]
- Sui, S.; Ma, H.; Wang, J.; Feng, M.; Pang, Y.; Xia, S.; Xu, Z.; Qu, S. Symmetry-based coding method and synthesis topology optimization design of ultra-wideband polarization conversion metasurfaces. Phys. Rev. Lett. 2016, 109, 014104. [Google Scholar] [CrossRef]
- Zhou, Y.; Kravchenko, I.I.; Wang, H.; Zheng, H.; Gu, G.; Valentine, J. Multifunctional metaoptics based on bilayer metasurfaces. Light Sci. Appl. 2019, 8, 80. [Google Scholar] [CrossRef] [PubMed]
- Ori, A.; Almeida, E.; Prior, Y.; Ellenbogen, T. Composite functional metasurfaces for multispectral achromatic optics. Nat. Commun. 2017, 8, 14992. [Google Scholar]
- Mansouree, M.; Kwon, H.; Arbabi, E.; McClung, A.; Faraon, A.; Arbabi, A. Multifunctional 2.5 D metastructures enabled by adjoint optimization. Optica 2020, 7, 77–84. [Google Scholar] [CrossRef]
- Tauqirm, A.; Rahim, A.A.; Bilal, R.M.H.; Noor, A.; Maab, H.; Naveed, M.A.; Madni, A.; Ali, M.M.; Saeed, M.A. Ultrawideband cross-polarization converter using anisotropic reflective metasurface. Electronics 2022, 11, 487. [Google Scholar] [CrossRef]
- Nguyen, T.M.; Phan, H.L.; Vu, D.L.; Nguyen, T.Q.H.; Kim, J.-M. Ultra-wideband and high-efficiency cross-polarization conversion using a double split ring shaped metasurface for C, X, and Ku-band applications. AIP Adv. 2022, 12, 115002. [Google Scholar] [CrossRef]
- Babar, K.; Chen, J.; Yingzeng, Y.; Ren, J.; Ullah, S.; Khan, W.U.R. High efficiency and ultra-wideband polarization converter based on an L-shaped metasurface. Opt. Mater. Express 2021, 11, 1343–1352. [Google Scholar]
- Yu, H.; Wang, X.; Su, J.; Qu, M.; Guo, Q.; Li, Z.; Song, J. Ultrawideband and high-efficient polarization conversion metasurface based on multi-resonant element and interference theory. Opt. Express 2021, 29, 35938–35950. [Google Scholar] [CrossRef]


| Ref. | Unit Cell Size (mm) | Thickness (mm) | Operating Bandwidth (GHz) | Polarization Conversion | PCR |
|---|---|---|---|---|---|
| [35] | 6.4 | 1.6 | 12.4–27.96 | LP to LP | PCR > 0.9 |
| [45] | 7 | 1.6 | 13–26 | LP to LP | PCR > 0.9 |
| [46] | 14 | 0.8 | 4–14 | LP to LP | PCR > 0.93 |
| [47] | 8 | 3 | 8.6–22 | LP to LP, LP to CP | PCR > 0.9 |
| [48] | 10 | 3.5 | 3.37–22.07 | LP to LP | PCR > 0.9 |
| Our work | 6.2 | 1.6 | 12.94–16.54, 17.54–26 | LP to LP | PCR > 0.9 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abbas, M.B.; Raza, F.; Baqir, M.A.; Altintas, O.; Abbas, M.; KaraaSlan, M.; Naqvi, Q.A. Ultra-Wideband Cross-Polarization Converter Using Metasurface Operating in the X- and K-Band. Photonics 2024, 11, 863. https://doi.org/10.3390/photonics11090863
Abbas MB, Raza F, Baqir MA, Altintas O, Abbas M, KaraaSlan M, Naqvi QA. Ultra-Wideband Cross-Polarization Converter Using Metasurface Operating in the X- and K-Band. Photonics. 2024; 11(9):863. https://doi.org/10.3390/photonics11090863
Chicago/Turabian StyleAbbas, Muhammad Basir, Faizan Raza, Muhammad Abuzar Baqir, Olcay Altintas, Musarat Abbas, Muharrem KaraaSlan, and Qaisar Abbas Naqvi. 2024. "Ultra-Wideband Cross-Polarization Converter Using Metasurface Operating in the X- and K-Band" Photonics 11, no. 9: 863. https://doi.org/10.3390/photonics11090863
APA StyleAbbas, M. B., Raza, F., Baqir, M. A., Altintas, O., Abbas, M., KaraaSlan, M., & Naqvi, Q. A. (2024). Ultra-Wideband Cross-Polarization Converter Using Metasurface Operating in the X- and K-Band. Photonics, 11(9), 863. https://doi.org/10.3390/photonics11090863








