Channel Correlation-Based Adaptive Power Transmission for Free-Space Optical Communications
Abstract
1. Introduction
2. Theoretical Analysis
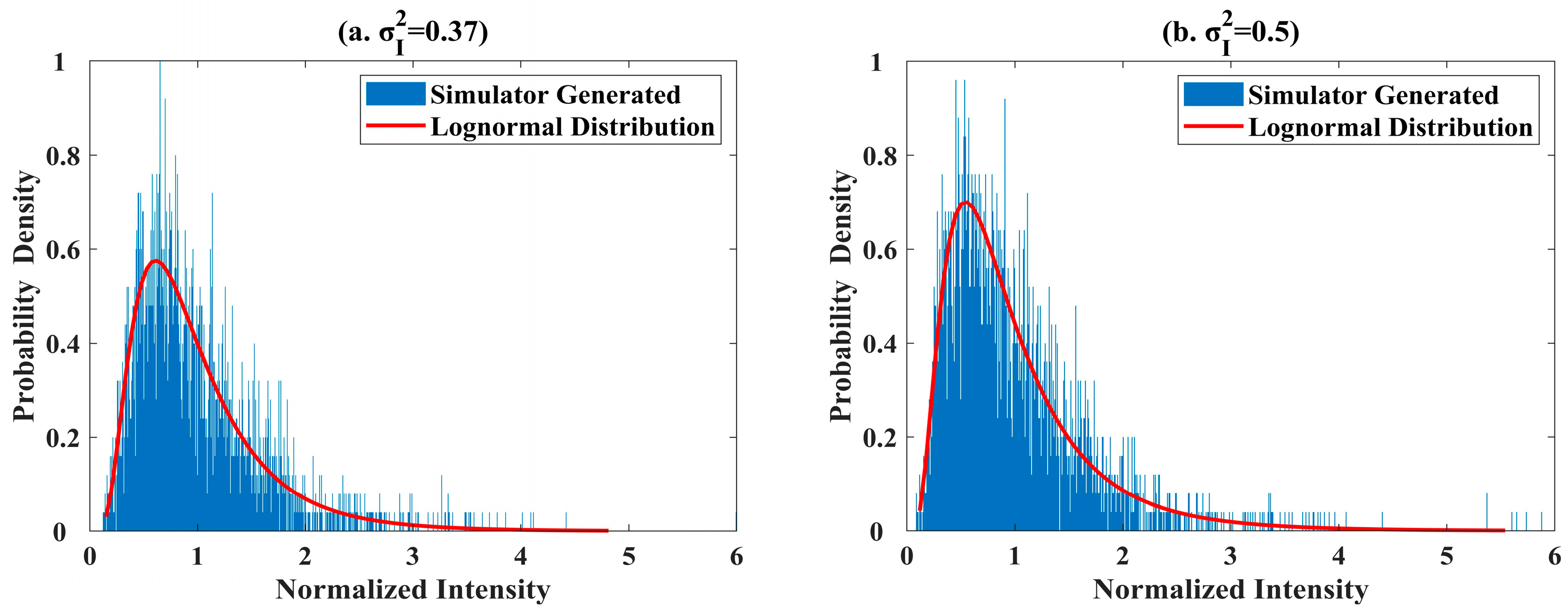
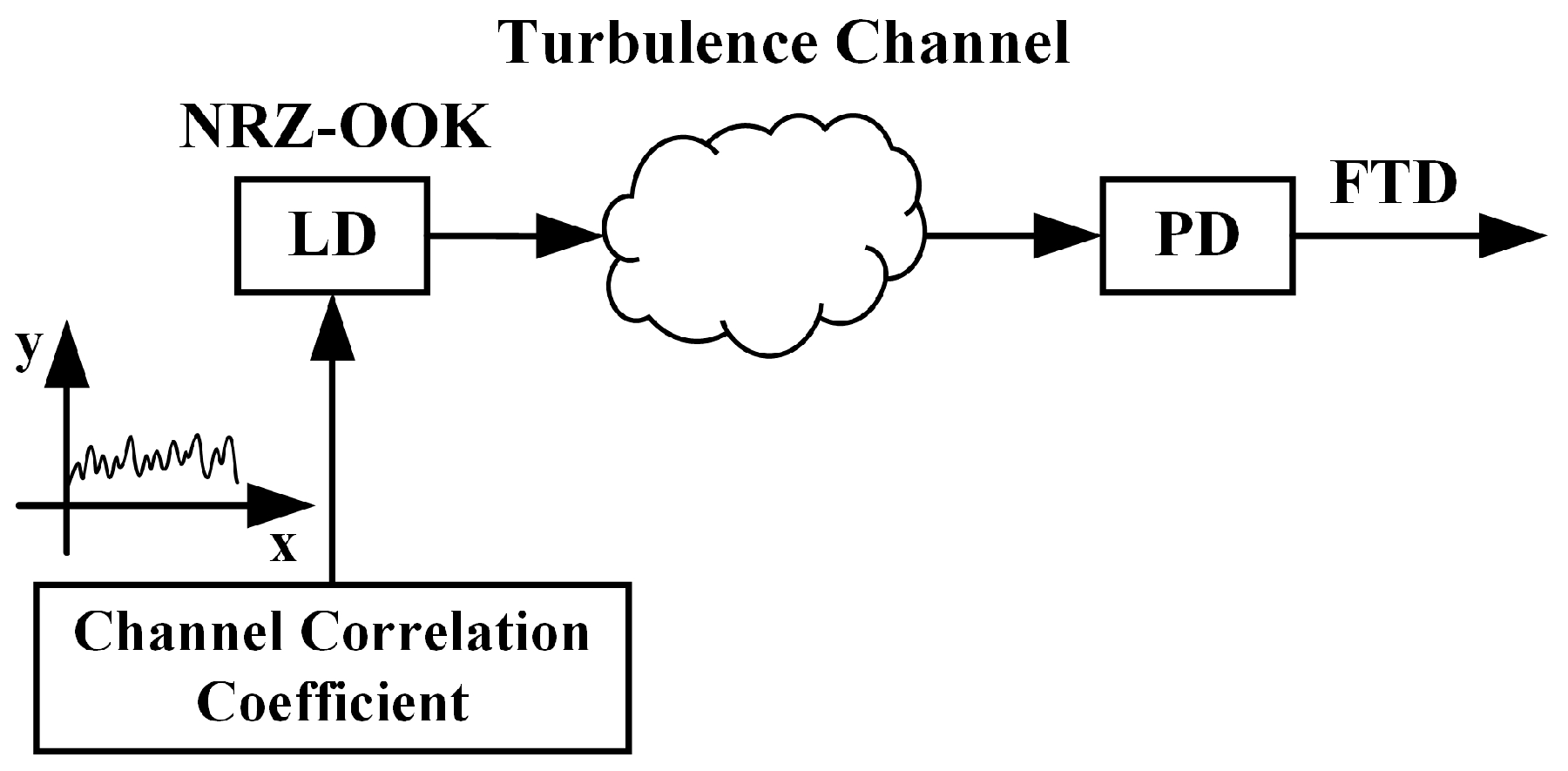
2.1. Channel Model
2.2. Operation Principle
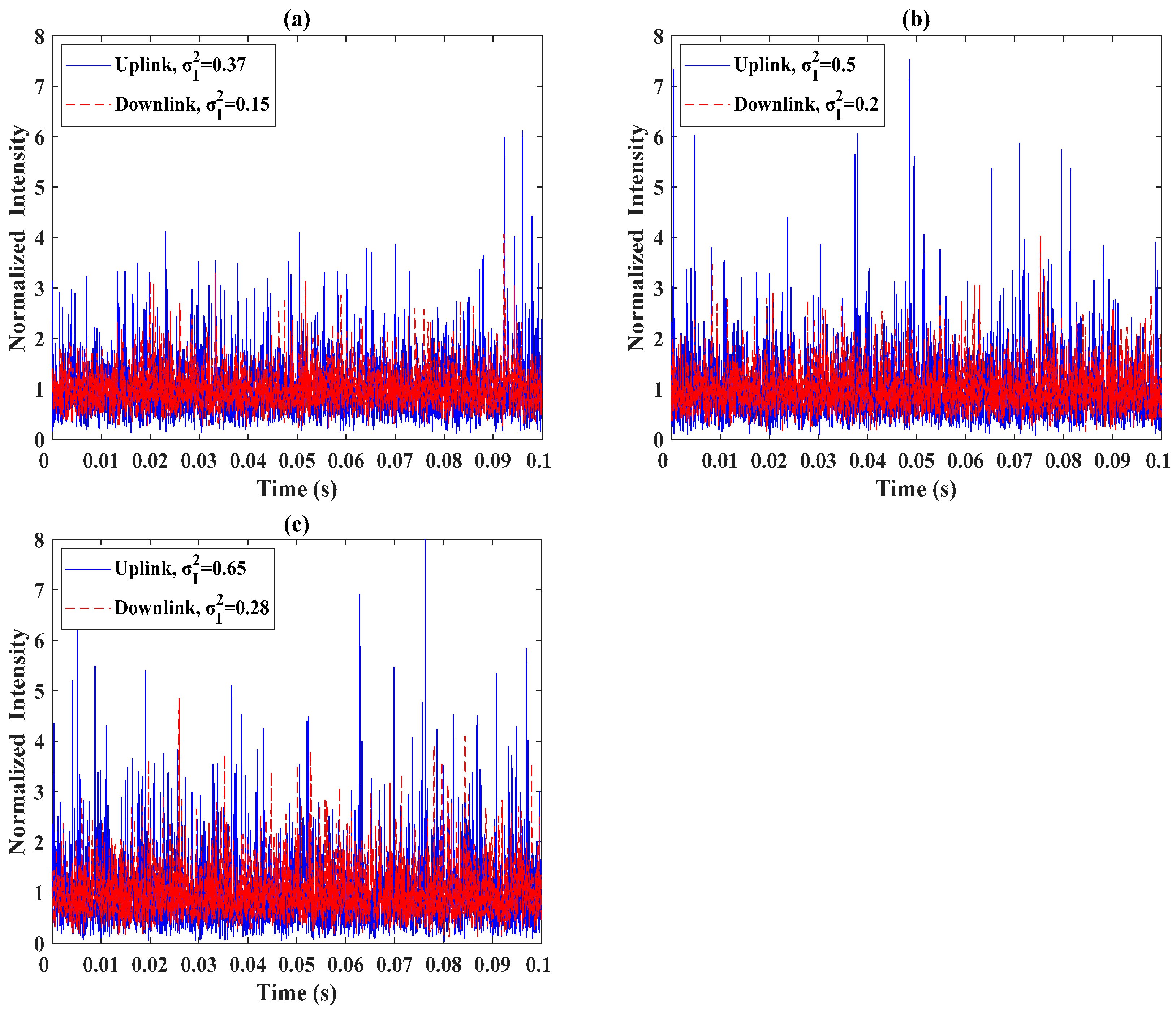
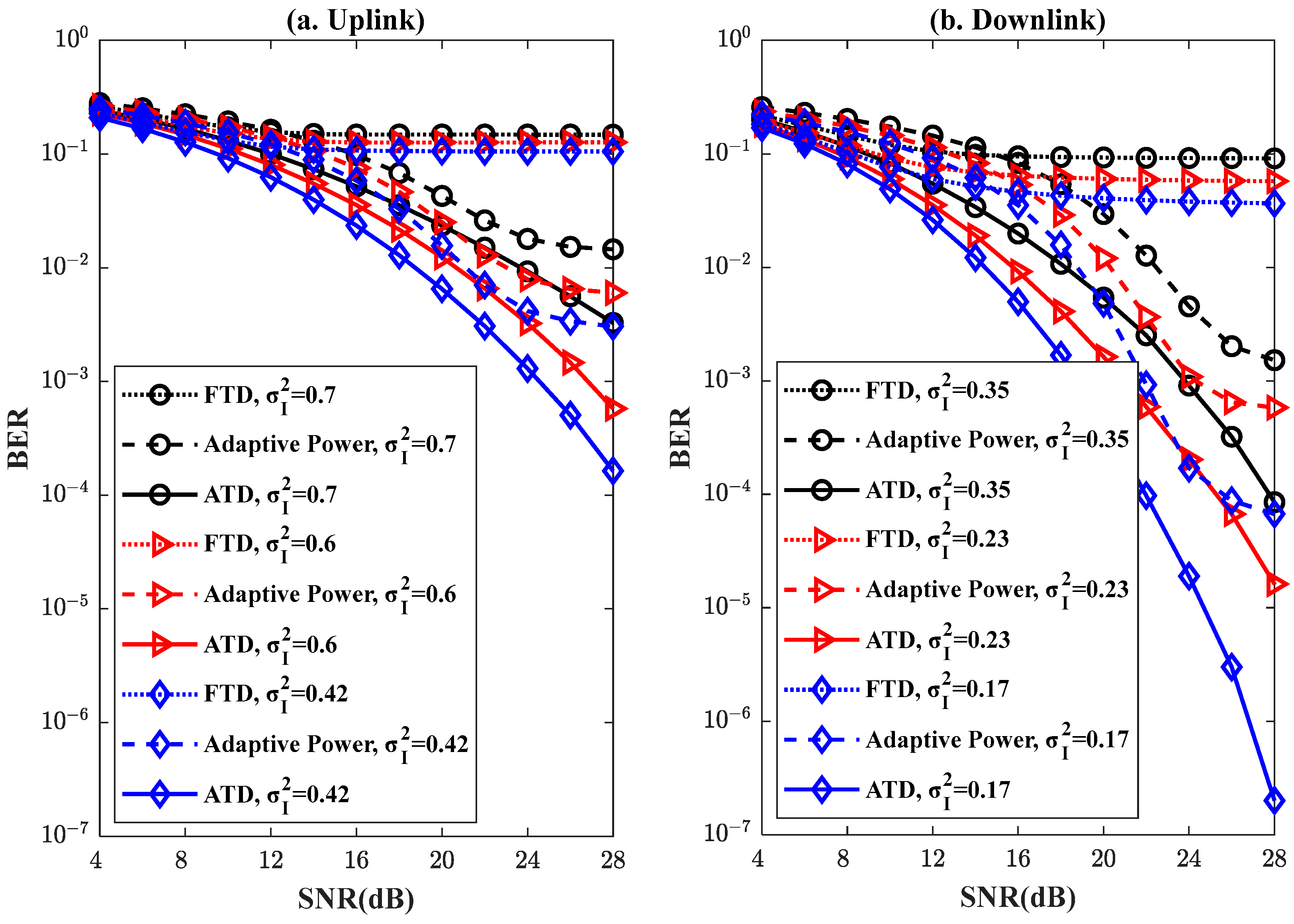
3. Simulations and Results
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Al-Gailani, S.A.; Salleh, M.F.M.; Salem, A.A.; Shaddad, R.Q.; Sheikh, U.U.; Algeelani, N.A.; Almohamad, T.A. A Survey of Free Space Optics (FSO) Communication Systems, Links, and Networks. IEEE Access 2021, 9, 7353–7373. [Google Scholar] [CrossRef]
- Samy, R.; Yang, H.-C.; Rakia, T.; Alouini, M.-S. Space-Air-Ground FSO Networks for High-Throughput Satellite Communications. IEEE Commun. Mag. 2022, 60, 82–87. [Google Scholar] [CrossRef]
- Esmail, M.A. Experimental performance evaluation of weak turbulence channel models for FSO links. Opt. Commun. 2021, 486, 126776. [Google Scholar] [CrossRef]
- Kaushal, H.; Kaddoum, G. Optical communication in space: Challenges and mitigation techniques. IEEE Commun. Surv. Tutor. 2017, 19, 57–96. [Google Scholar] [CrossRef]
- Sharma, P.K.; Bansal, A.; Garg, P.; Tsiftsis, T.; Barrios, R. Relayed FSO communication with aperture averaging receivers and misalignment errors. IET Commun. 2017, 11, 45–52. [Google Scholar] [CrossRef]
- Israr, A.; Israr, A.; Khan, F.; Khan, F. Optimal modulation technique for MIMO FSO link. Wirel. Pers. Commun. 2019, 109, 695–714. [Google Scholar] [CrossRef]
- Hasan, S.M.A.; Ahmed, S.; Islam, A.K.M.N. Simulation of A Massive MIMO FSO System Under Atmospheric Turbulence. In Proceedings of the 2021 5th International Conference on Electrical Engineering and Information Communication Technology (ICEEICT), Dhaka, Bangladesh, 18–20 November 2021. [Google Scholar]
- Darusalam, U.; Priambodo, P.S.; Rahardjo, E.T. SNR and BER performance enhancement on FSO induced by atmospheric turbulence using optical spatial filter. Int. J. Opt. Appl. 2015, 5, 51–57. [Google Scholar]
- Mao, H.; Zhong, J.; Yu, S.; Xiao, P.; Yang, X.; Lu, G. An Atmospheric Turbulence Compensation Algorithm Based on FSM-DM Cascaded AO Architecture for FSO Communications. Network 2022, 2, 270–287. [Google Scholar] [CrossRef]
- Nguyen, D.-N.; Zvanovec, S.; Ghassemlooy, Z. Mitigation of dispersion and turbulence in a hybrid optical fibre and free-space optics link using electronic equalisation. Optik 2019, 196, 163154. [Google Scholar] [CrossRef]
- Choi, J.-Y.; Lee, J.-W.; Han, S.-K. Stochastic Approximation Aided Adaptive Thresholding for Optical Detection in PAM4 Based FSO Transmission. IEEE Access 2021, 9, 106451–106458. [Google Scholar] [CrossRef]
- Jing, Q.-W.; Hong, Y.-Q.; Liu, M.-F. RZ-PPM impulse tone-based CSI estimation for adaptive threshold decision in FSO communications. Microw. Opt. Technol. Lett. 2024, 66, e34239. [Google Scholar] [CrossRef]
- Chen, Z.; Yu, S.; Wang, T.; Wu, G.; Wang, S.; Gu, W. Channel correlation in aperture receiver diversity systems for free-space optical communication. J. Opt. 2012, 14, 125710. [Google Scholar] [CrossRef]
- Priyadarshani, R.; Jaiswal, A.; Bhatnagar, M.R.; Bohata, J.; Zvanovec, S.; Ghassemlooy, Z. Impact of Channel Correlation on Different Performance Metrics of OSSK-Based FSO Systems. IEEE Trans. Wirel. Commun. 2020, 19, 1593–1609. [Google Scholar] [CrossRef]
- Choi, I.-Y.; Shin, W.-H.; Han, S.-K. CSI estimation with pilot tone for scintillation effects mitigation on satellite optical communication. Opt. Commun. 2019, 435, 88–92. [Google Scholar] [CrossRef]
- Lv, P.; Hong, Y.; Jing, Q.; Liu, W. Average SNR Based Adaptive Power Transmission Technique in FSO Links. J. Nav. Aviat. Univ. 2024, 39, 235–240. [Google Scholar]
- Hong, Y.-Q.; Li, G.; Liu, Z.-Y. Optical adaptive power transmission using APC-EDFA for turbulence-tolerant FSO communications. Opt. Express 2021, 29, 23777–23785. [Google Scholar] [CrossRef] [PubMed]
- Liu, W.; Chen, X.; Liu, M.; Hong, Y. Bidirectional Atmospheric Channel Reciprocity-Based Adaptive Power Transmission. Photonics 2023, 10, 1067. [Google Scholar] [CrossRef]
- Navidpour, S.M.; Uysal, M.; Kavehrad, M. BER Performance of Free-Space Optical Transmission with Spatial Diversity. IEEE Trans. Wirel. Commun. 2007, 6, 2813–2819. [Google Scholar] [CrossRef]
- Kwon, D.-H.; Han, S.-K. Optical wireless channel reciprocity-based temporal power allocation technique for FSO communications. Opt. Commun. 2023, 529, 129089. [Google Scholar] [CrossRef]
- Maharjan, N.; Devkota, N.; Kim, B.W. Atmospheric Effects on Satellite–Ground Free Space Uplink and Downlink Optical Transmissions. Appl. Sci. 2022, 12, 10944. [Google Scholar] [CrossRef]

| Parameters | Values |
|---|---|
| Wavelength | 1550 nm |
| Output power | 20 dBm |
| Link Distance | ||||
|---|---|---|---|---|
| 30 km | 0.37 | 0.15 | 0.6384 | 0.6404 |
| 30 km | 0.5 | 0.2 | 0.5984 | 0.5979 |
| 30 km | 0.65 | 0.28 | 0.5218 | 0.5225 |
| 40 km | 0.42 | 0.17 | 0.6342 | 0.6338 |
| 40 km | 0.6 | 0.23 | 0.5792 | 0.5808 |
| 40 km | 0.7 | 0.35 | 0.4847 | 0.4871 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, B.-S.; Lv, P.-F.; Hong, Y.-Q. Channel Correlation-Based Adaptive Power Transmission for Free-Space Optical Communications. Photonics 2024, 11, 859. https://doi.org/10.3390/photonics11090859
Zhao B-S, Lv P-F, Hong Y-Q. Channel Correlation-Based Adaptive Power Transmission for Free-Space Optical Communications. Photonics. 2024; 11(9):859. https://doi.org/10.3390/photonics11090859
Chicago/Turabian StyleZhao, Bai-Shan, Peng-Fei Lv, and Yan-Qing Hong. 2024. "Channel Correlation-Based Adaptive Power Transmission for Free-Space Optical Communications" Photonics 11, no. 9: 859. https://doi.org/10.3390/photonics11090859
APA StyleZhao, B.-S., Lv, P.-F., & Hong, Y.-Q. (2024). Channel Correlation-Based Adaptive Power Transmission for Free-Space Optical Communications. Photonics, 11(9), 859. https://doi.org/10.3390/photonics11090859






