The Method and Experiment of Micro-Crack Identification Using OFDR Strain Measurement Technology
Abstract
1. Introduction
2. Principles
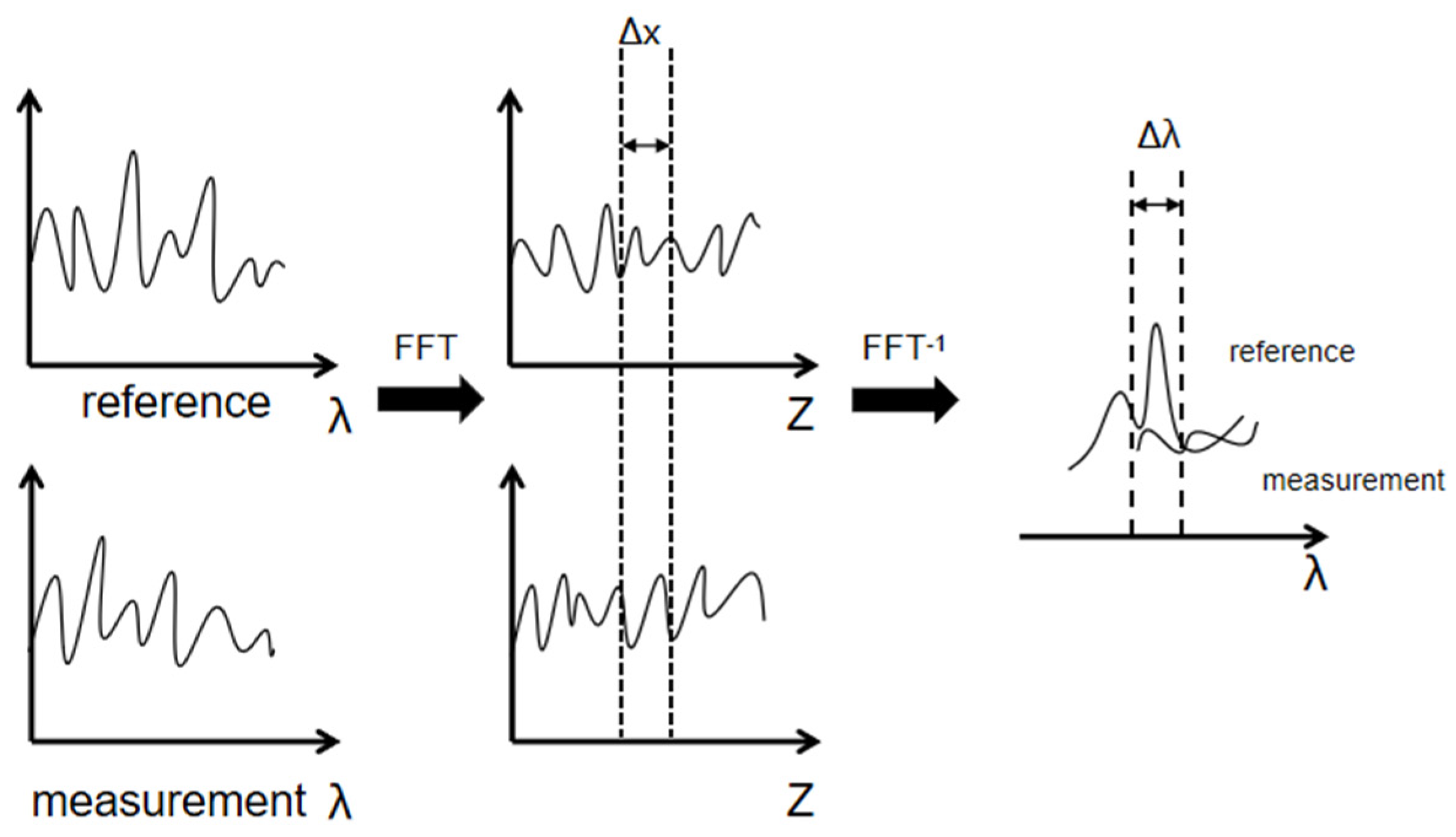
2.1. The Micro-Crack Localization Principle Based on OFDR
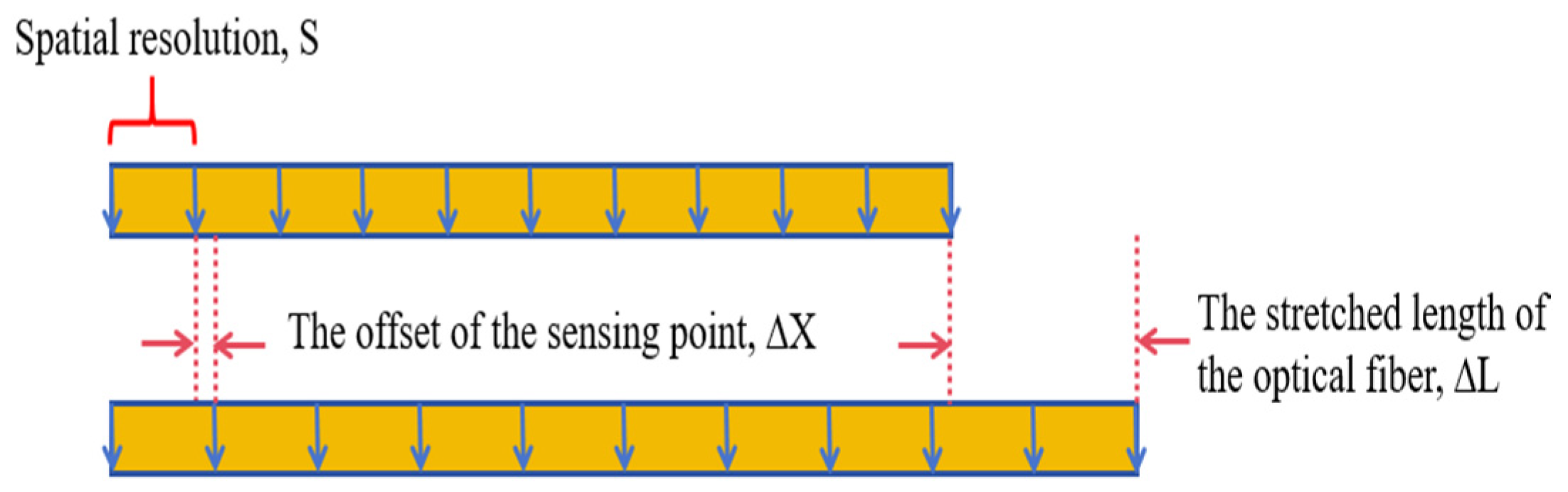
2.2. Calculation Method for Micro-Crack Width
3. Experimental Preparation
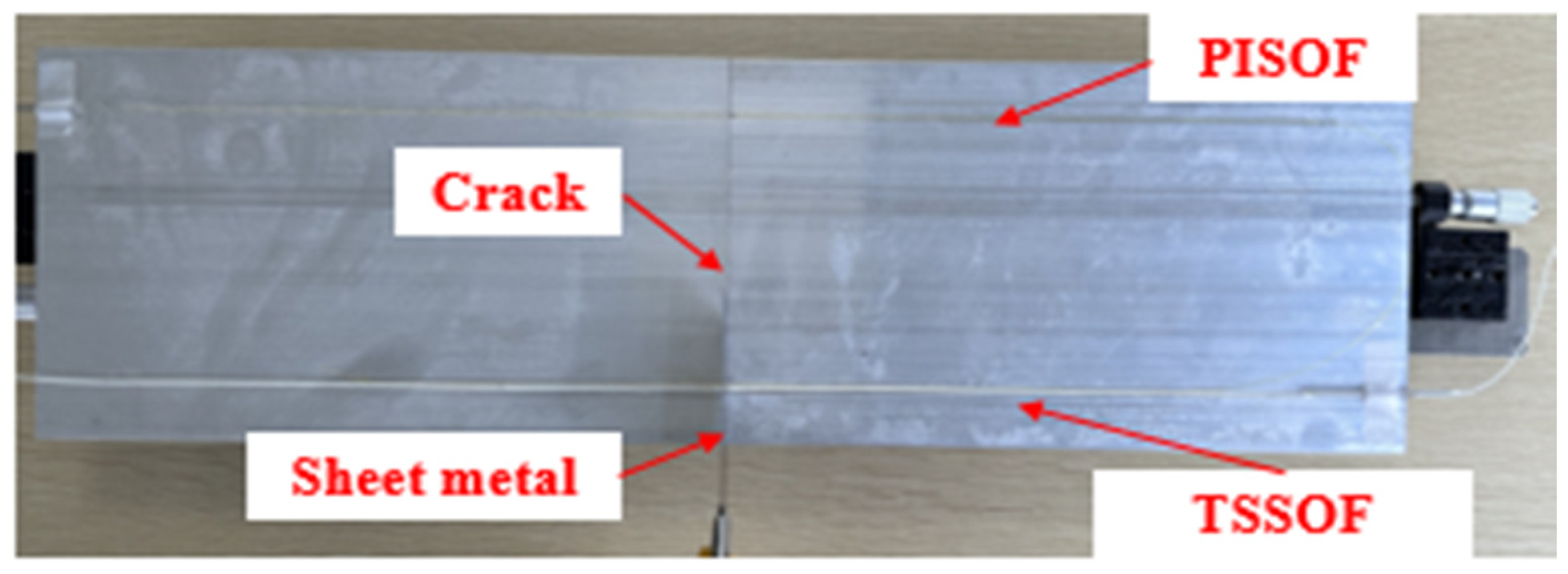
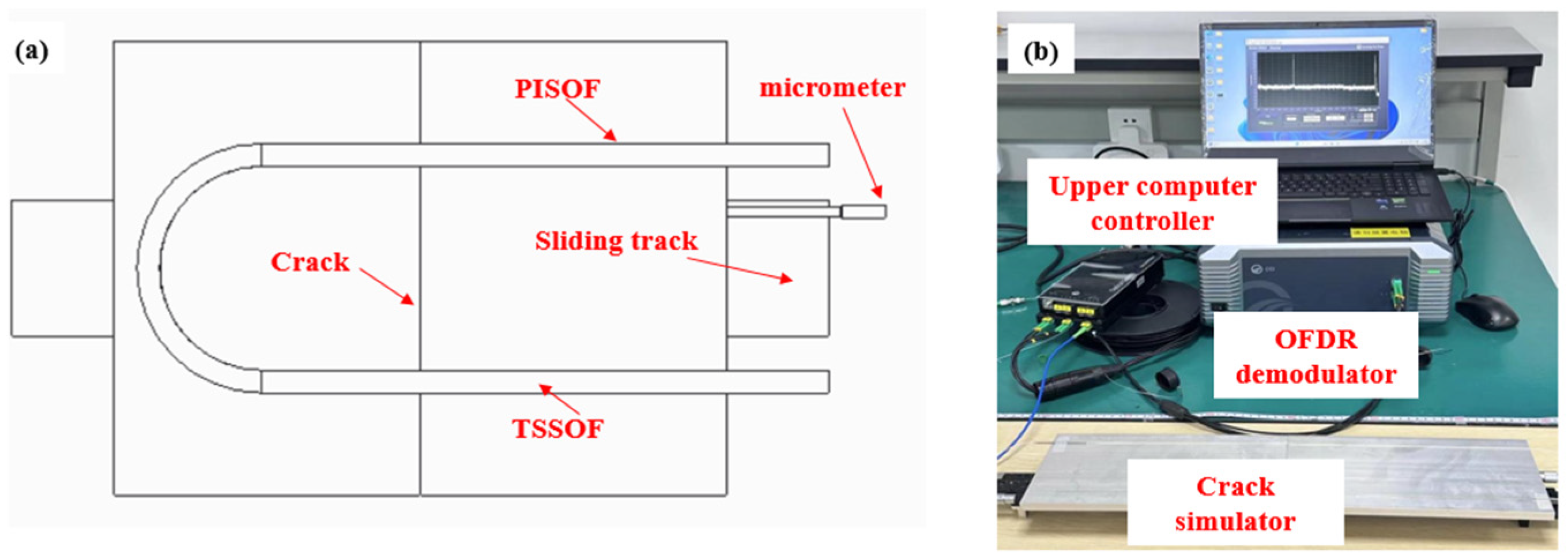
3.1. Experimental Setup
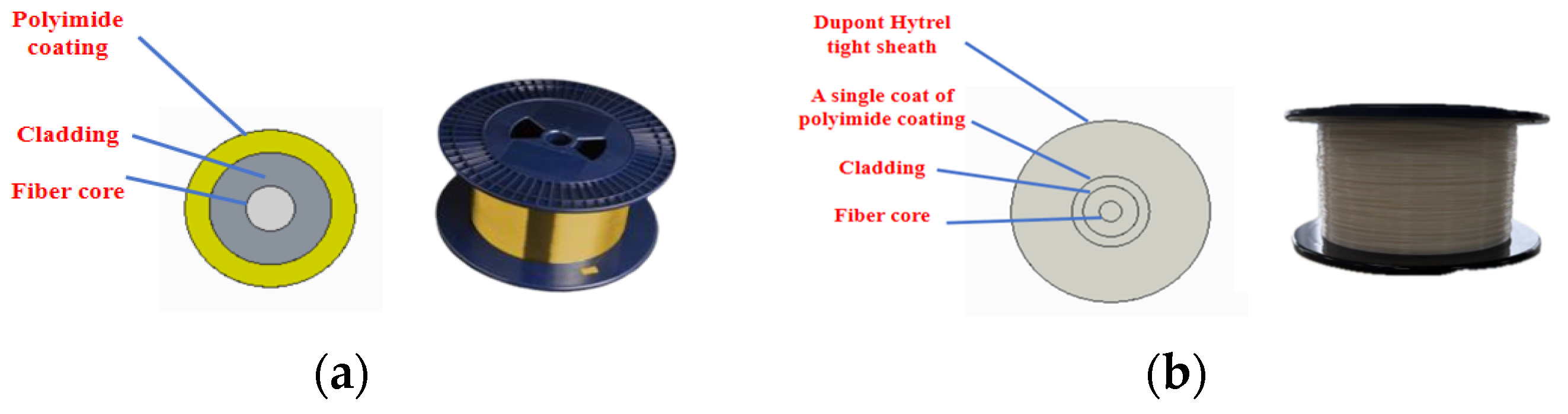
3.2. Two Types of Fiber Optic Strain Sensors
4. Results and Discussion
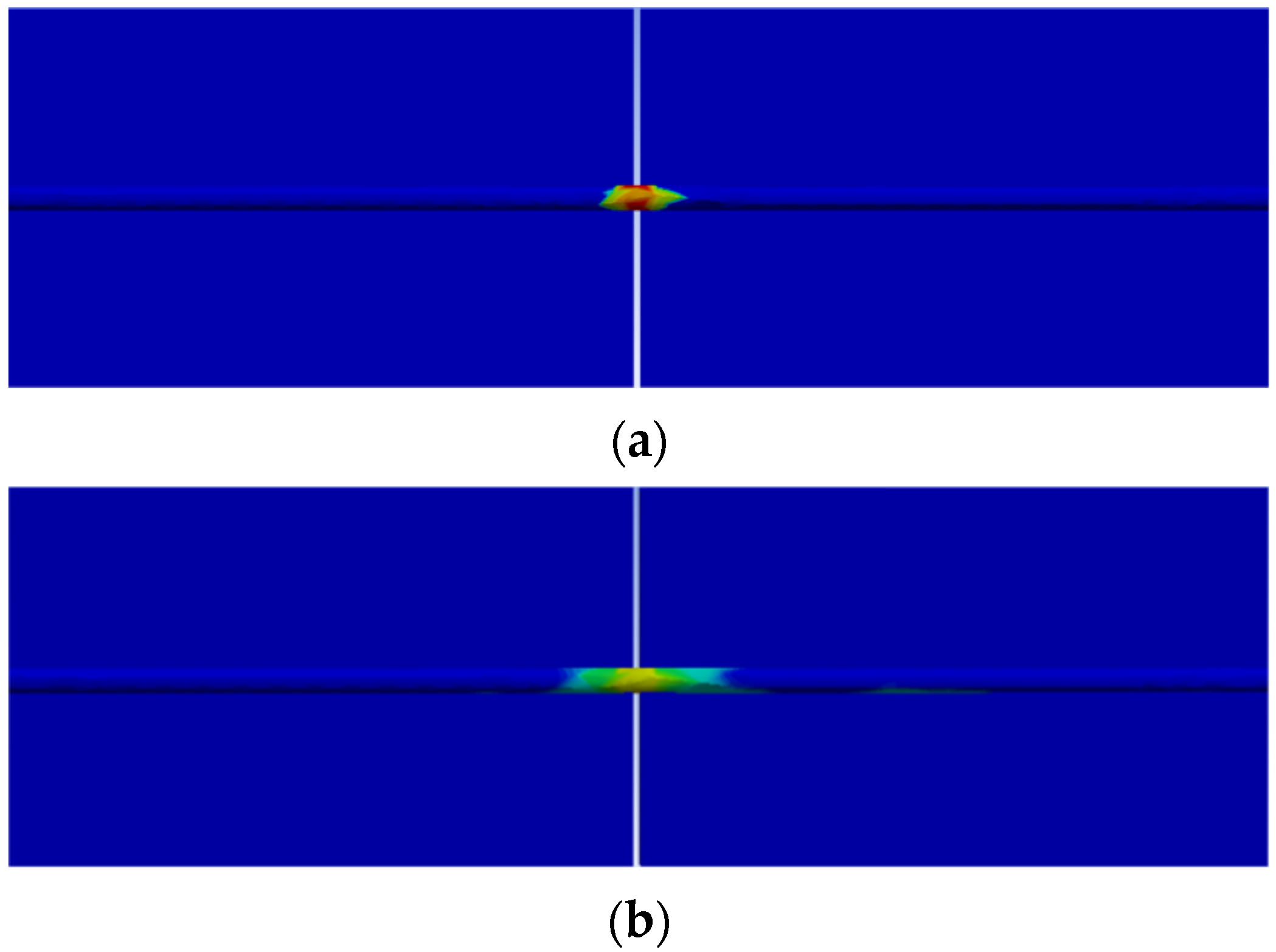
4.1. Analysis of Location Results of Micro-Cracks
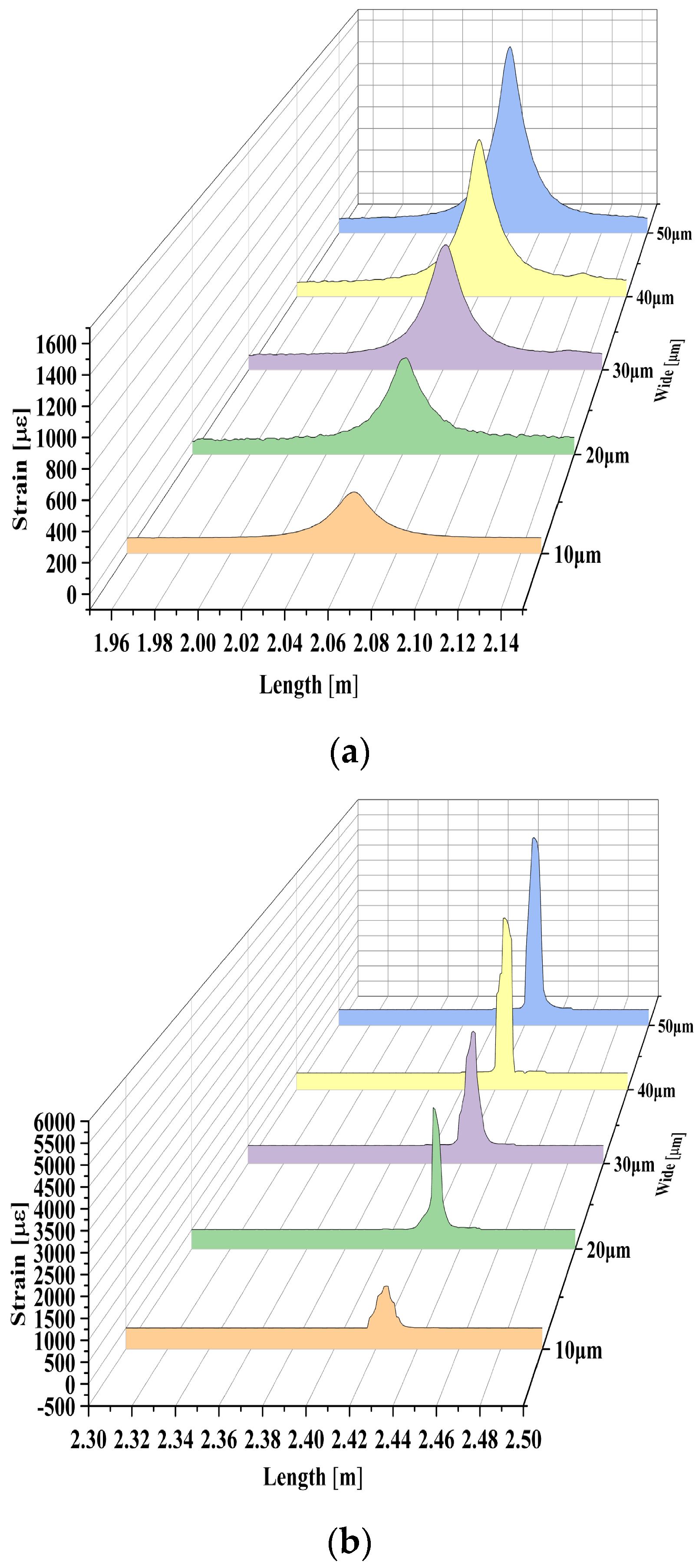
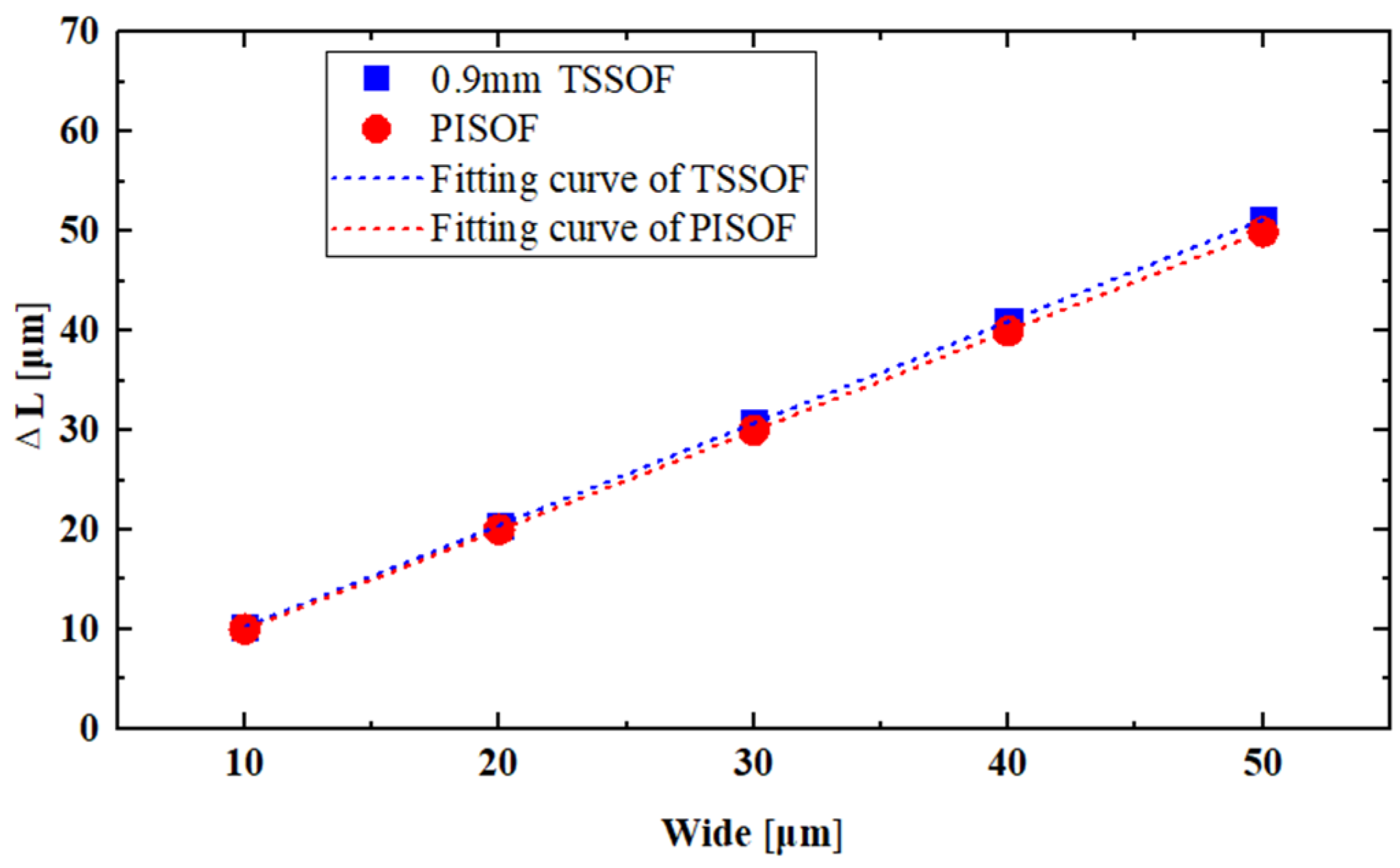
4.2. Analysis of Micro-Crack Width Measurement Results
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Jing, A. Application of Laser welding Technology in aviation industry machinery manufacturing. Mod. Ind. Econ. Inf. Technol. 2024, 14, 121–123. [Google Scholar]
- Molaro, J.L.; Byrne, S.; Langer, S.A. Grain-scale thermoclastic stresses and spatiotemporal temperature gradients on airless bodies, implications for rock breakdown. J. Geophys. Res. Planets 2015, 120, 255–277. [Google Scholar] [CrossRef]
- Li, J.; Wang, Q.; Zan, Y.; Ju, L.; Jing, C.; Zhang, Y. Research on wing crack propagation of closed crack under uniaxial compression based on peridynamics. Eng. Anal. Bound. Elem. 2024, 158, 121–138. [Google Scholar] [CrossRef]
- Wang, Y.; Han, S.; Yu, Y.; Qi, X.; Zhang, Y.; Lian, Y.; Bai, Z.; Wang, Y.; Lv, Z. Numerical Simulation of Metal Defect Detection Based on Laser Ultrasound. IEEE Photonics J. 2021, 13, 6800609. [Google Scholar] [CrossRef]
- Zhang, R.; Sun, Y.; Ma, J. Understanding thermal–mechanical fatigue crack growth: A microscopic mechanical model. Mater. Sci. Eng. A 2005, 398, 142–145. [Google Scholar] [CrossRef]
- Esmaeili, A.; Mohammadi, B.; Yousefi, A. Investigation of T-stress and tensile strength effect on crack tip conditions and crack initiation angle in off-axis laminate composite. Theor. Appl. Fract. Mech. 2024, 130, 104283. [Google Scholar] [CrossRef]
- Yan, X.; Wang, H.; Fan, X. Research Progress in Nonlinear Ultrasonic Testing for Early Damage in Metal Materials. Materials 2023, 16, 2161. [Google Scholar] [CrossRef] [PubMed]
- Bi, C.; Yuan, Y.; Zhang, R.; Xiang, Y.; Wang, Y.; Zhang, J. A Dynamic Mode Decomposition Based Edge Detection Method for Art Images. IEEE Photonics J. 2017, 9, 7803813. [Google Scholar] [CrossRef]
- Ogura, K.; Nishikawa, I. Fatigue Crack Closure Monitoring at Elevated Temperature Using Laser Interferometric Displacement Gage. Proceedings of the KSME/JSME THERMAL and FLUID Engineering Conference, 1990. [Google Scholar]
- Dietzel, W.; Schwalbe, K.H. Monitoring stable crack growth using a combined AC/DC potential drop technique. Mater. Test. 1986, 28, 368–372. [Google Scholar] [CrossRef]
- Swartz, S.E.; Jones, G.L.; Hu, K.K. Compliance Monitoring of Crack Growth in Concrete. J. Eng. Mech. Div. 1978, 104, 789–800. [Google Scholar] [CrossRef]
- Dumoulin, C.; Deraemaeker, A. Real-time fast ultrasonic monitoring of concrete cracking using embedded piezoelectric transducers. Smart Mater. Struct. 2017, 26, 104006. [Google Scholar] [CrossRef]
- Zhang, G.Q.; Wang, J.W. A New Method for Inspecting Crack of Concrete Bridges Using Image Processing Technique. Adv. Mater. Res. 2010, 1037, 2704–2708. [Google Scholar]
- Yi, D.; Kong, L.; Xie, J.; Huang, C. Online Crack Detection of Highly Curved Cylindrical Coils. IEEE Trans. Ind. Inform. 2024, 20, 703–712. [Google Scholar] [CrossRef]
- Yin, S.; Xiao, H.; Xu, C.; Wang, J.; Deng, M.; Kundu, T. Microcrack localization using nonlinear Lamb waves and cross-shaped sensun clusters. Ultrasonics 2022, 124, 106770. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.; Xu, C.; Hu, N.; Deng, M. Microcrack localization based on static component induced by a primary A0 Lamb wave in a thin plate. JASA Express Lett. 2022, 2, 024001. [Google Scholar] [CrossRef] [PubMed]
- Leffers, L.; Locmelis, J.; Bremer, K.; Roth, B.; Overmeyer, L. Optical Bend Sensor Based on Eccentrically Micro-Structured Multimode Polymer Optical Fibers. IEEE Photonics J. 2021, 13, 6800307. [Google Scholar] [CrossRef]
- Ying, Y.; Morgese, M.; Ansari, F.; Gao, Z. Prediction of crack opening in steel beam based on strains measured from distributed optical fiber sensor. Meas. Sci. Technol. 2024, 35, 015101. [Google Scholar] [CrossRef]
- Ma, S.; Ji, Q.; Zhao, X.; Qin, Z.; Liu, Z.; Xu, Y. Humidity Sensing Using a Multimode Fiber Ring Laser with Thermal Compensation. Photonics 2024, 11, 484. [Google Scholar] [CrossRef]
- Liu, X.; Tang, Y.; Zhang, Z.; Yang, S.; Hu, Z.; Xu, Y. A Pattern Recognition Method for Filter Bags in Bag Dust Collectors Based on Φ-Optical Time-Domain Reflectometry. Photonics 2024, 11, 152. [Google Scholar] [CrossRef]
- Liu, K.; Yin, G.; Zhang, Z.; Yang, P.; Lu, H.; Li, D.; Zhu, T. High-Resolution and High-Precision Φ-OFDR Strain Sensing Scheme Based on Adaptive Phase Unwrapping and Wavelet Packet Denoising. J. Light. Technol. 2024, 42, 891–897. [Google Scholar] [CrossRef]
- Li, Y.; Fan, H.; Zhang, L.; Liu, Z.; Wang, L.; Wu, J.; Wang, S. Single-Mode Input Fiber Combined with Multimode Sensing Fiber Used in Brillouin Optical Time-Domain Reflectometry. Photonics 2022, 9, 398. [Google Scholar] [CrossRef]
- Li, Y.N.; Cao, X.Y.; Xie, T.; Zhao, Z.G.; Zhou, Y.P.; Li, C.; Liu, H.; Su, J.K.; Li, L.M.; Zhang, Z.L. Strain and Cracks of Tunnel Lining Surveyed by Fiber Bragg Grating Strain Sensors and Ultrasonic Tester. Appl. Mech. Mater. 2013, 2453, 472–478. [Google Scholar] [CrossRef]
- Fu, C.; Meng, Y.; Chen, L.; Zhong, H.; Du, C.; He, J.; Weng, X.; Liu, L.; Qu, J.; Wang, Y. High-spatial-resolution φ-OFDR shape sensor based on multicore optical fiber with femtosecond-laser-induced permanent scatter arrays. Opt. Lett. 2023, 48, 3219–3222. [Google Scholar] [CrossRef] [PubMed]
- Chen, B.; Yang, J.; Zhang, D.; Li, J.; Wang, Z.; Shi, G.; Zhang, M. Double-Wire-Based Single Distributed Optical Fiber Strain Sensing Method in High-Temperature Environment. IEEE Trans. Instrum. Meas. 2023, 26, 7000710. [Google Scholar] [CrossRef]
- Chakrabarti, K.; Shiwa, M. Simulation Study of Strains Obtained by Two Different Scattering Processes in Optical Fiber Sensors. Int. J. Comput. Sci. Eng. 2016, 4, 1–9. [Google Scholar]


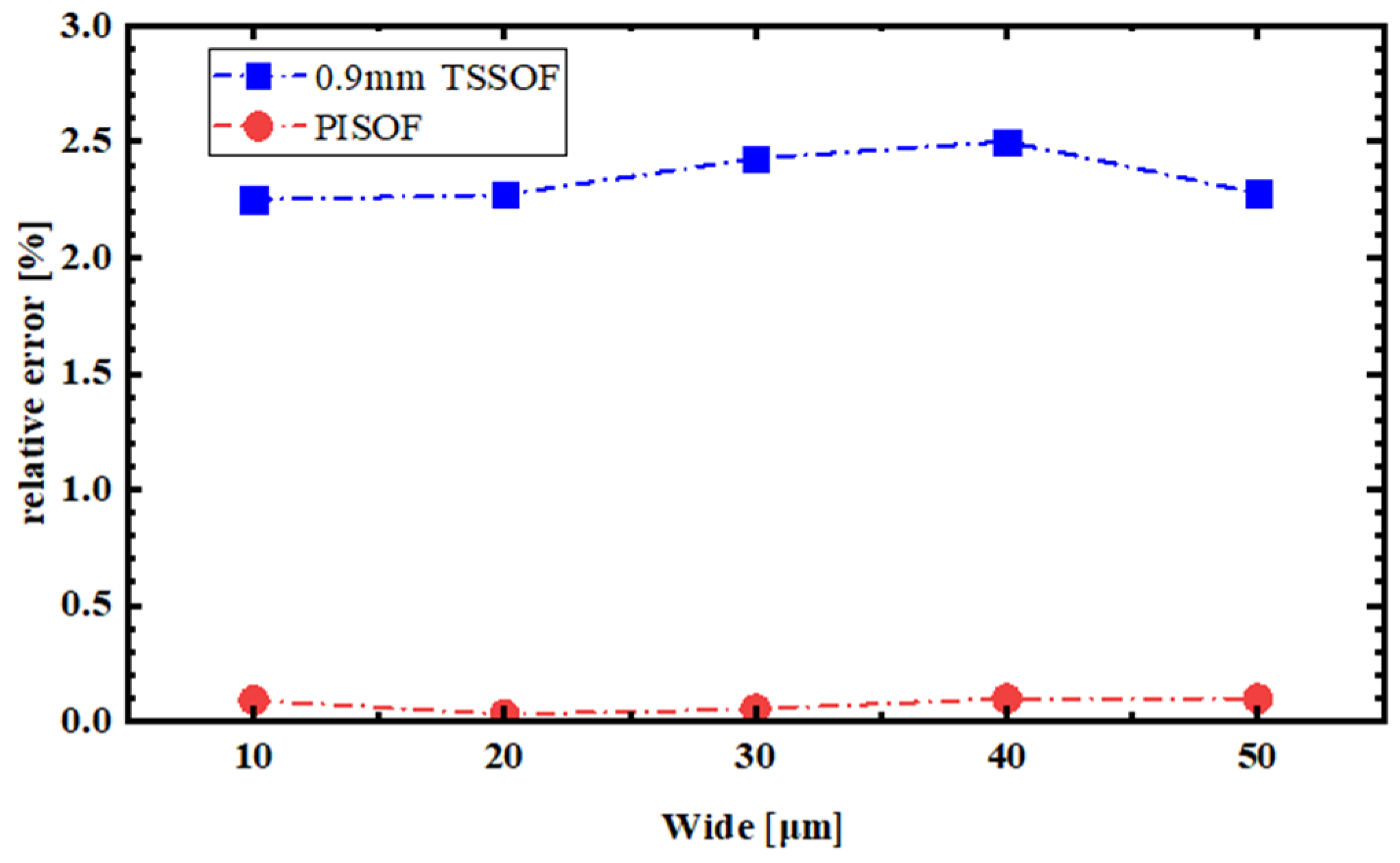
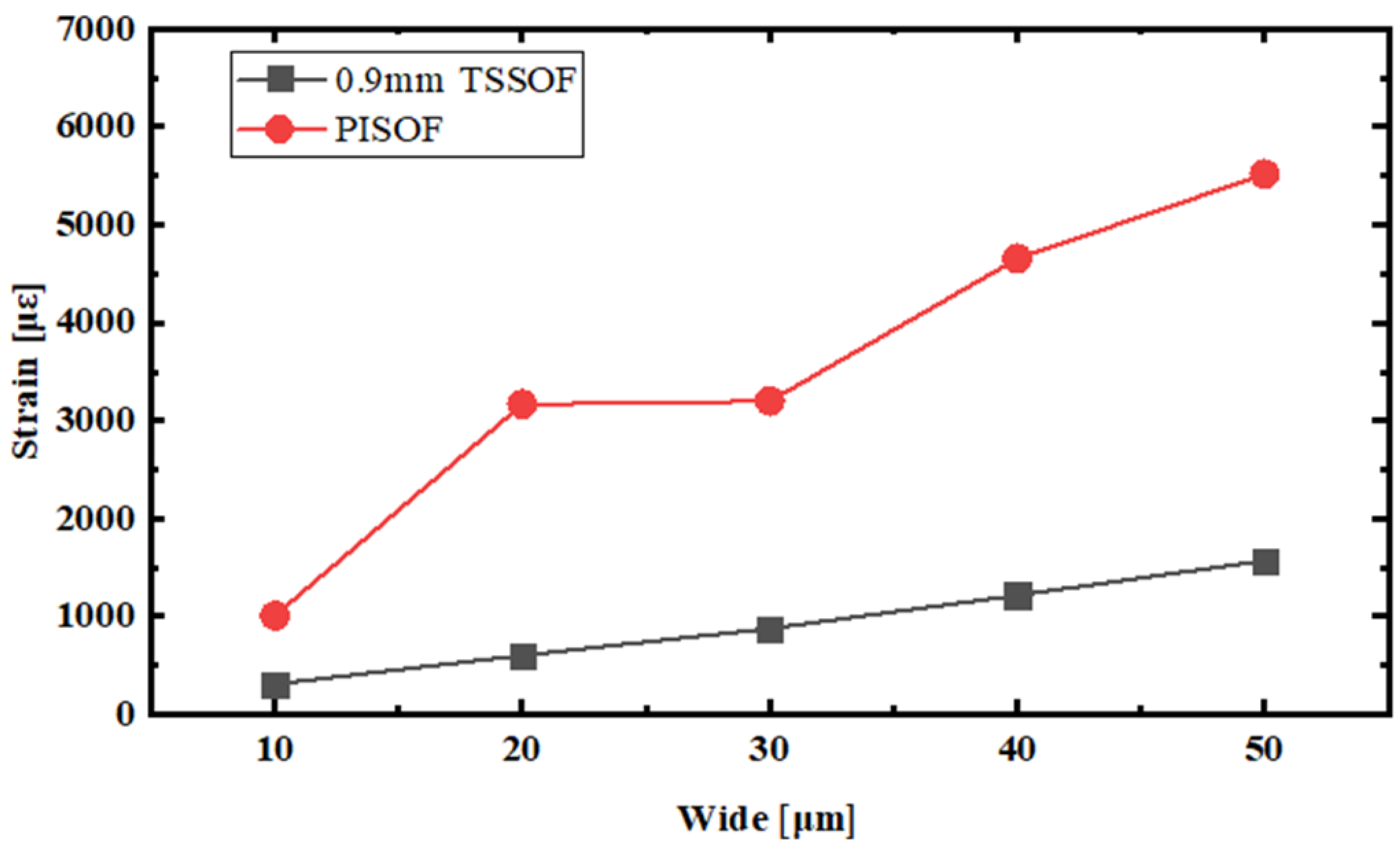
| Types of Optical Fiber Sensors | Crack Width | ||||
|---|---|---|---|---|---|
| 10 μm | 20 μm | 30 μm | 40 μm | 50 μm | |
| 0.9mm-TSSOF | 10.225 μm | 20.454 μm | 30.728 μm | 41.022 μm | 51.138 μm |
| PISOF | 9.991 μm | 20.006 μm | 29.984 μm | 39.961 μm | 49.951 μm |
| Types of Optical Fiber Sensors | Crack Width | ||||
|---|---|---|---|---|---|
| 10 μm | 20 μm | 30 μm | 40 μm | 50 μm | |
| 0.9mm TSSOF | 310.2 με | 601.9 με | 876.4 με | 1217.5 με | 1569.4 με |
| PISOF | 1006.6 με | 3166.7 με | 3197.8 με | 4652.1 με | 5515.6 με |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, B.; Yang, J.; Zhang, D.; Liu, W.; Li, J.; Zhang, M. The Method and Experiment of Micro-Crack Identification Using OFDR Strain Measurement Technology. Photonics 2024, 11, 755. https://doi.org/10.3390/photonics11080755
Chen B, Yang J, Zhang D, Liu W, Li J, Zhang M. The Method and Experiment of Micro-Crack Identification Using OFDR Strain Measurement Technology. Photonics. 2024; 11(8):755. https://doi.org/10.3390/photonics11080755
Chicago/Turabian StyleChen, Bin, Jun Yang, Dezhi Zhang, Wenxiang Liu, Jin Li, and Min Zhang. 2024. "The Method and Experiment of Micro-Crack Identification Using OFDR Strain Measurement Technology" Photonics 11, no. 8: 755. https://doi.org/10.3390/photonics11080755
APA StyleChen, B., Yang, J., Zhang, D., Liu, W., Li, J., & Zhang, M. (2024). The Method and Experiment of Micro-Crack Identification Using OFDR Strain Measurement Technology. Photonics, 11(8), 755. https://doi.org/10.3390/photonics11080755





