Broadband Spin-Selective Wavefront Manipulations with Generalized Pancharatnam–Berry Phase Metasurface
Abstract
1. Introduction
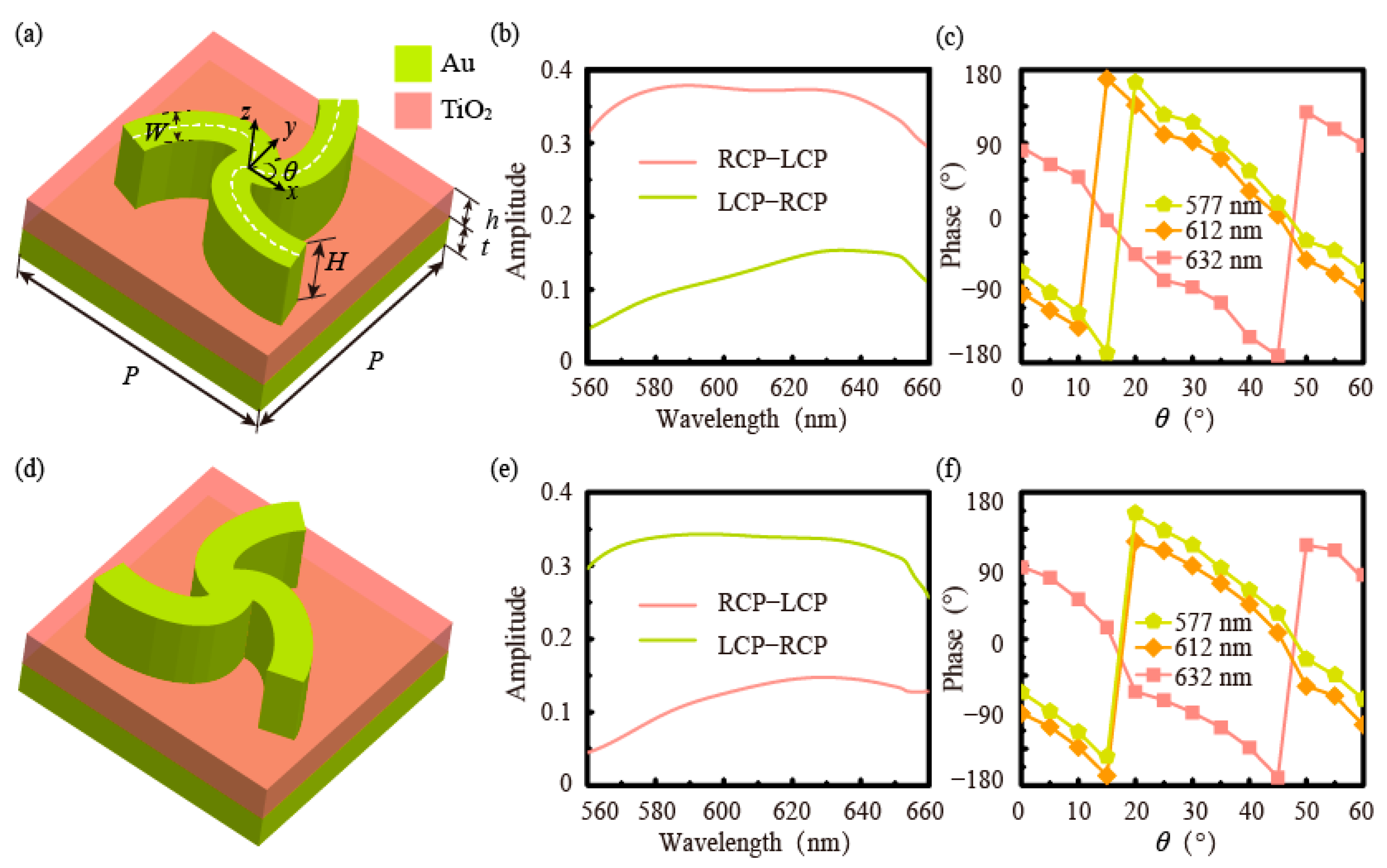
2. Concept and Design
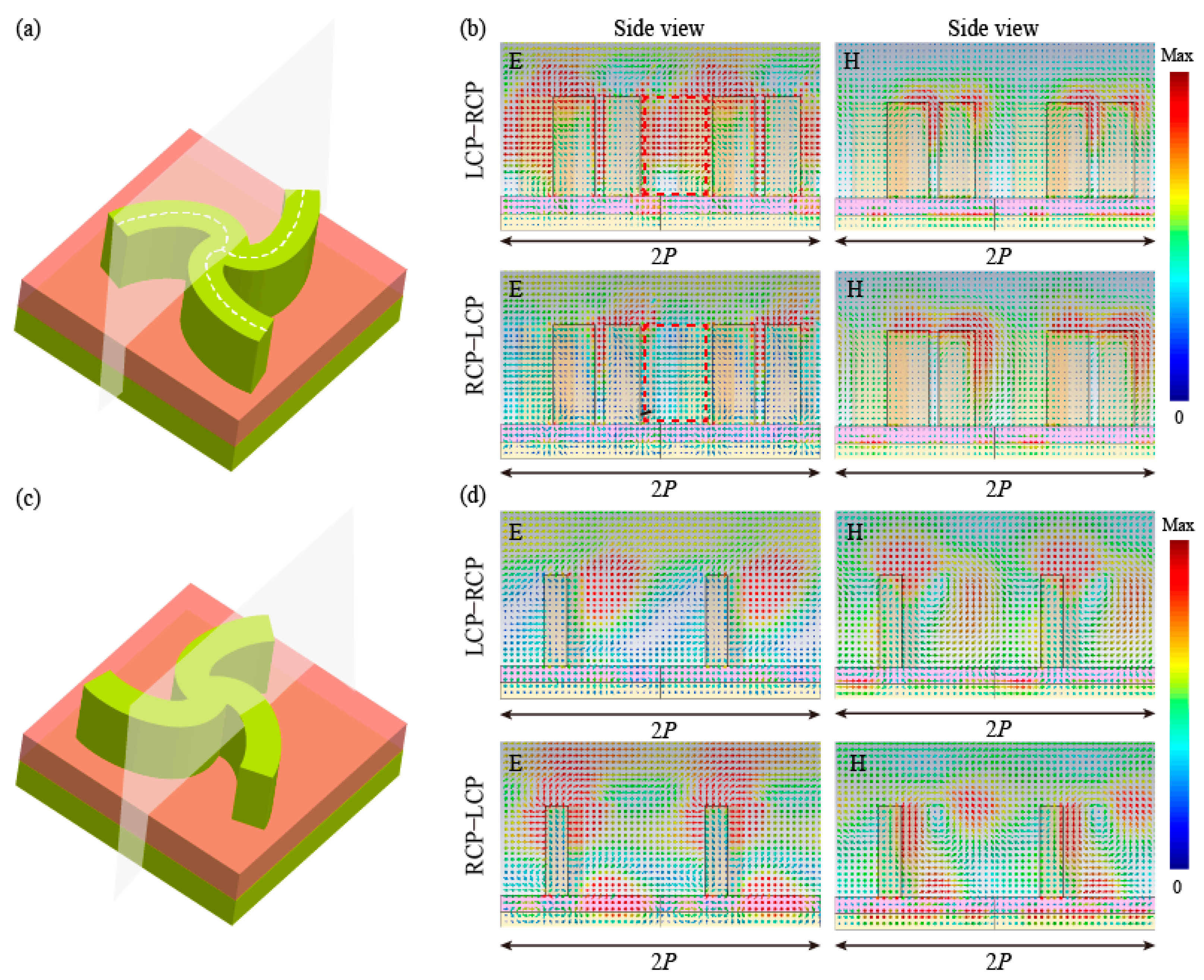
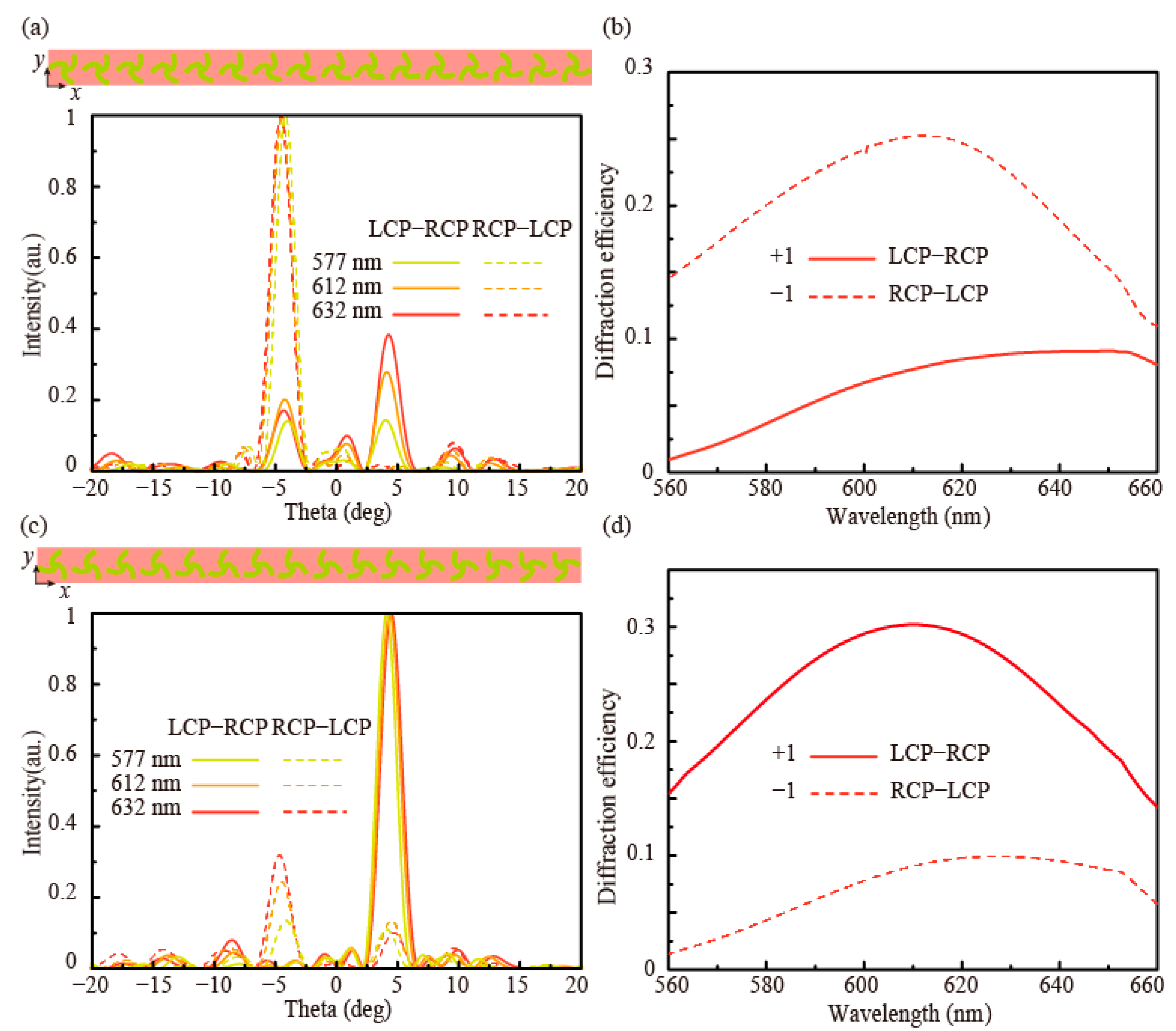
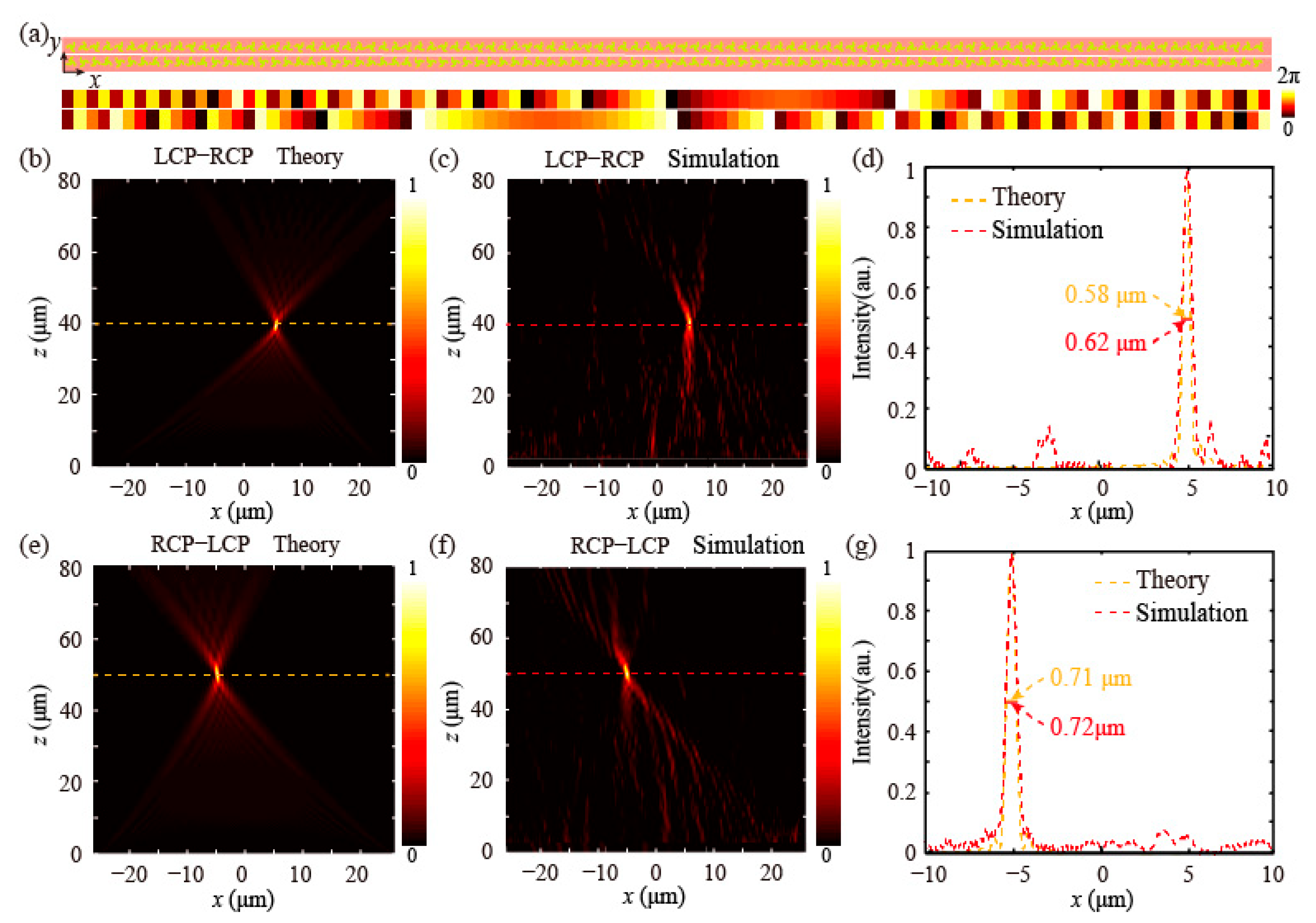
3. Spin-Selective Metadevices
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Yu, N.; Genevet, P.; Kats, M.A.; Aieta, F.; Tetienne, J.P.; Capasso, F.; Gaburro, Z. Light propagation with phase discontinuities: Generalized laws of reflection and refraction. Science 2011, 334, 333–337. [Google Scholar] [CrossRef]
- Zaidi, A.; Rubin, N.A.; Meretska, M.L.; Li, L.W.; Dorrah, A.H.; Park, J.S.; Capasso, F. Metasurface-enabled single-shot and complete mueller matrix imaging. Nat. Photonics 2024, 18, 704–712. [Google Scholar] [CrossRef]
- Kim, M.; Lee, D.; Yang, Y.; Kim, Y.; Rho, J. Reaching the highest efficiency of spin hall effect of light in the near-infrared using all-dielectric metasurfaces. Nat. Commun. 2022, 13, 2036. [Google Scholar] [CrossRef] [PubMed]
- Sell, D.; Yang, J.; Wang, E.W.; Phan, T.; Doshay, S.; Fan, J.A. Ultra-high-efficiency anomalous refraction with dielectric metasurfaces. ACS Photonics 2018, 5, 2402–2407. [Google Scholar] [CrossRef]
- Xu, H.X.; Hu, G.; Wang, Y.; Wang, C.; Wang, M.; Wang, S.; Huang, Y.; Genevet, P.; Huang, W.; Qiu, C.-W. Polarization-insensitive 3d conformal-skin metasurface cloak. Light Sci. Appl. 2021, 10, 75. [Google Scholar] [CrossRef] [PubMed]
- Siegel, J.; Kim, S.; Fortman, M.; Wan, C.; Kats, M.A.; Hon, P.W.C.; Sweatlock, L.; Jang, M.S.; Brar, V.W. Electrostatic steering of thermal emission with active metasurface control of delocalized modes. Nat. Commun. 2024, 15, 3376. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.; Yang, X.; Gao, J. Spin-controlled wavefront shaping with plasmonic chiral geometric metasurfaces. Light Sci. Appl. 2018, 7, 84. [Google Scholar] [CrossRef]
- Huang, Y.; Xiao, T.; Xie, Z.; Zheng, J.; Su, Y.; Chen, W.; Liu, K.; Tang, M.; Müller-Buschbaum, P.; Li, L. Single-layered reflective metasurface achieving simultaneous spin-selective perfect absorption and efficient wavefront manipulation. Adv. Opt. Mater. 2021, 9, 2001663. [Google Scholar] [CrossRef]
- Li, T.; Chen, Y.; Fu, B.; Liu, M.; Wang, J.; Gao, H.; Wang, S.; Zhu, S. Spin-selective trifunctional metasurfaces for deforming versatile nondiffractive beams along the optical trajectory. Laser Photonics Rev. 2024, 2024, 2301372. [Google Scholar] [CrossRef]
- Huang, Y.; Xiao, T.; Chen, S.; Xie, Z.; Zheng, J.; Zhu, J.; Su, Y.; Chen, W.; Liu, K.; Tang, M.; et al. All-optical controlled-not logic gate achieving directional asymmetric transmission based on metasurface doublet. Opto-Electron. Adv. 2023, 6, 220073. [Google Scholar] [CrossRef]
- Zheng, G.; Mühlenbernd, H.; Kenney, M.; Li, G.; Zentgraf, T.; Zhang, S. Metasurface holograms reaching 80% efficiency. Nat. Nanotechnol. 2015, 10, 308–312. [Google Scholar] [CrossRef]
- Ming, Y.; Intaravanne, Y.; Ahmed, H.; Kenney, M.; Lu, Y.Q.; Chen, X. Creating composite vortex beams with a single geometric metasurface. Adv. Mater. 2022, 34, 2109714. [Google Scholar] [CrossRef] [PubMed]
- Fang, B.; Shu, F.; Wang, Z.; Ji, J.; Jin, Z.; Hong, Z.; Shen, C.; Cheng, Q.; Li, T. On-chip non-uniform geometric metasurface for multi-channel wavefront manipulations. Opt. Lett. 2023, 48, 3119–3122. [Google Scholar] [CrossRef]
- Gou, Y.; Ma, H.F.; Wu, L.W.; Wang, Z.X.; Xu, P.; Cui, T.J. Broadband spin-selective wavefront manipulations based on pancharatnam–berry coding metasurfaces. ACS Omega 2021, 6, 30019–30026. [Google Scholar] [CrossRef] [PubMed]
- Xiao, S.; Wang, J.; Liu, F.; Zhang, S.; Yin, X.; Li, J. Spin-dependent optics with metasurfaces. Nanophotonics 2017, 6, 215–234. [Google Scholar] [CrossRef]
- Zhang, M.; Pu, M.; Zhang, F.; Guo, Y.; He, Q.; Ma, X.; Huang, Y.; Li, X.; Yu, H.; Luo, X. Plasmonic metasurfaces for switchable photonic spin–orbit interactions based on phase change materials. Adv. Sci. 2018, 5, 1800835. [Google Scholar] [CrossRef] [PubMed]
- Balthasar Mueller, J.P.; Rubin, N.A.; Devlin, R.C.; Groever, B.; Capasso, F. Metasurface polarization optics: Independent phase control of arbitrary orthogonal states of polarization. Phys. Rev. Lett. 2017, 118, 113901. [Google Scholar] [CrossRef]
- Zhang, F.; Guo, Y.; Pu, M.; Li, X.; Ma, X.; Luo, X. Metasurfaces enabled by asymmetric photonic spin-orbit interactions. Opto-Electron. Eng. 2020, 47, 200366. [Google Scholar]
- Wu, X.; Feng, Y.; Zhang, C.; Liu, H.L. Three-dimensional chiral metasurfaces for circular-polarized anomalous beam steering. Opt. Lett. 2022, 47, 1794–1797. [Google Scholar] [CrossRef]
- He, C.; Sun, T.; Guo, J.; Cao, M.; Xia, J.; Hu, J.; Yan, Y.; Wang, C. Chiral metalens of circular polarization dichroism with helical surface arrays in mid-infrared region. Adv. Opt. Mater. 2019, 7, 1901129. [Google Scholar] [CrossRef]
- Ma, Z.; Li, Y.; Li, Y.; Gong, Y.; Maier, S.A.; Hong, M. All-dielectric planar chiral metasurface with gradient geometric phase. Opt. Express 2018, 26, 6067–6078. [Google Scholar] [CrossRef]
- Zhu, A.Y.; Chen, W.T.; Zaidi, A.; Huang, Y.W.; Khorasaninejad, M.; Sanjeev, V.; Qiu, C.W.; Capasso, F. Giant intrinsic chiro-optical activity in planar dielectric nanostructures. Light Sci. Appl. 2018, 7, 17158. [Google Scholar] [CrossRef] [PubMed]
- Kim, D.; Yu, J.; Hwang, I.; Park, S.; Demmerle, F.; Boehm, G.; Amann, M.C.; Belkin, M.A.; Lee, J. Giant nonlinear circular dichroism from intersubband polaritonic metasurfaces. Nano Lett. 2020, 20, 8032–8039. [Google Scholar] [CrossRef]
- Faraz, F.; Li, Z.; Zhang, Z.; Rehman Abbasi, T.U.; Wang, X.; Rukhlenko, I.D.; Zhu, W. High-efficiency geometric phase metasurface with multifold rotationally symmetric resonators. ACS Appl. Opt. Mater. 2023, 1, 173–178. [Google Scholar] [CrossRef]
- Liu, B.; Sain, B.; Reineke, B.; Zhao, R.; Meier, C.; Huang, L.; Jiang, Y.; Zentgraf, T. Nonlinear wavefront control by geometric-phase dielectric metasurfaces: Influence of mode field and rotational symmetry. Adv. Opt. Mater. 2020, 8, 1902050. [Google Scholar] [CrossRef]
- Liu, B.; Geromel, R.; Su, Z.; Guo, K.; Wang, Y.; Guo, Z.; Huang, L.; Zentgraf, T. Nonlinear dielectric geometric-phase metasurface with simultaneous structure and lattice symmetry design. ACS Photonics 2023, 10, 4357–4366. [Google Scholar] [CrossRef]
- Li, G.; Chen, S.; Pholchai, N.; Reineke, B.; Wong, P.W.H.; Pun Edwin, Y.B.; Cheah, K.W.; Zentgraf, T.; Zhang, S. Continuous control of the nonlinearity phase for harmonic generations. Nat. Mater. 2015, 14, 607–612. [Google Scholar] [CrossRef]
- Chen, S.; Li, G.; Zeuner, F.; Wong, W.H.; Pun, E.Y.B.; Zentgraf, T.; Cheah, K.W.; Zhang, S. Symmetry-selective third-harmonic generation from plasmonic metacrystals. Phys. Rev. Lett. 2014, 113, 033901. [Google Scholar] [CrossRef] [PubMed]
- Li, G.; Zhang, S.; Zentgraf, T. Nonlinear photonic metasurfaces. Nat. Rev. Mater. 2017, 2, 17010. [Google Scholar]
- Xie, X.; Pu, M.B.; Jin, J.J.; Xu, M.; Guo, Y.; Li, X.; Gao, P.; Ma, X.; Luo, X. Generalized pancharatnam-berry phase in rotationally symmetric meta-atoms. Phys. Rev. Lett. 2021, 126, 183902. [Google Scholar] [CrossRef] [PubMed]
- Yuan, Y.; Zhang, K.; Ratni, B.; Song, Q.; Ding, X.; Wu, Q.; Burokur, S.N.; Genevet, P. Independent phase modulation for quadruplex polarization channels enabled by chirality-assisted geometric-phase metasurfaces. Nat. Commun. 2020, 11, 4186. [Google Scholar] [CrossRef]
- Song, Q.; Baroni, A.; Sawant, R.; Ni, P.; Brandli, V.; Chenot, S.; Vézian, S.; Damilano, B.; de Mierry, P.; Khadir, S.; et al. Ptychography retrieval of fully polarized holograms from geometric-phase metasurfaces. Nat. Commun. 2020, 11, 2651. [Google Scholar] [CrossRef] [PubMed]
- Deng, Q.; Yang, J.; Lan, X.; Zhang, W.; Cui, H.; Xie, Z.; Li, L.; Huang, Y. Investigations of generalized pancharatnam-berry phase in all-dielectric metasurfaces. Results Phys. 2023, 51, 106730. [Google Scholar] [CrossRef]
- Cai, J.; Zhang, F.; Pu, M.; Chen, Y.; Guo, Y.; Xie, T.; Feng, X.; Ma, X.; Li, X.; Yu, H.; et al. All-metallic high-efficiency generalized pancharatnam–berry phase metasurface with chiral meta-atoms. Nanophotonics 2022, 11, 1961–1968. [Google Scholar] [CrossRef]
- Palik, E.D. Handbook of Optical Constants of Solids; Boston Academic Press: Boston, MA, USA, 1991; pp. 286–395. [Google Scholar]
- Zhang, F.; Xie, X.; Pu, M.; Guo, Y.; Ma, X.; Li, X.; Luo, J.; He, Q.; Yu, H.; Luo, X. Multistate switching of photonic angular momentum coupling in phase-change metadevices. Adv. Mater. 2020, 32, 1908194. [Google Scholar] [CrossRef] [PubMed]
- Wang, L.; Guo, X.; Zhang, Y.; Zhou, X.; Yuan, L.; Zhang, P.; Liang, S.; Lan, F.; Zeng, H.; Zhang, T.; et al. Enhanced thz eit resonance based on the coupled electric field dropping effect within the undulated meta-surface. Nanophotonics 2019, 8, 1071–1078. [Google Scholar] [CrossRef]
- Xu, Z.; Ni, C.; Cheng, Y.; Dong, L.; Wu, L. Photo-excited metasurface for tunable terahertz reflective circular polarization conversion and anomalous beam deflection at two frequencies independently. Nanomaterials 2023, 13, 1846. [Google Scholar] [CrossRef]
- Asadchy, V.S.; Albooyeh, M.; Tcvetkova, S.N.; Díaz-Rubio, A.; Ra’di, Y.; Tretyakov, S.A. Perfect control of reflection and refraction using spatially dispersive metasurfaces. Phys. Rev. B 2016, 94, 075142. [Google Scholar] [CrossRef]
- Cai, J.; Yu, H. Full-space wavefront manipulation enabled by asymmetric photonic spin-orbit interactions. Opt. Express 2023, 31, 1409–1419. [Google Scholar] [CrossRef]
- Zhang, Z.; Luo, J.; Song, M.; Yu, H. Polarization filtering and phase controlling metasurfaces based on a metal-insulator-metal grating. Plasmonics 2017, 12, 1797–1803. [Google Scholar] [CrossRef]
- Zhao, M.; Chen, M.K.; Zhuang, Z.P.; Zhang, Y.; Chen, A.; Chen, Q.; Liu, W.; Wang, J.; Chen, Z.M.; Wang, B.; et al. Phase characterisation of metalenses. Light Sci. Appl. 2021, 10, 52. [Google Scholar] [CrossRef] [PubMed]
- Luo, X. Catenary Optics; Springer: Berlin/Heidelberg, Germany, 2019; pp. 377–419. [Google Scholar]
- Pu, M.; Li, X.; Ma, X.; Wang, Y.; Zhao, Z.; Wang, C.; Hu, C.; Gao, P.; Huang, C.; Ren, H.; et al. Catenary optics for achromatic generation of perfect optical angular momentum. Sci. Adv. 2015, 1, e1500396. [Google Scholar] [CrossRef] [PubMed]
- Cai, J.; Zhang, F.; Pu, M.; Xie, T.; Feng, X.; Yu, H.; Luo, X. Broadband and high-efficiency photonic spin-hall effect with all-metallic metasurfaces. Opt. Express 2022, 30, 14938–14947. [Google Scholar] [CrossRef]
- Paniagua-Domínguez, R.; Yu, Y.F.; Khaidarov, E.; Choi, S.; Leong, V.; Bakker, R.M.; Liang, X.; Fu, Y.H.; Valuckas, V.; Krivitsky, L.A.; et al. A metalens with a near-unity numerical aperture. Nano Lett. 2018, 18, 2124–2132. [Google Scholar] [CrossRef]
- Qiao, M.; Yan, J.; Jiang, L. Direction controllable nano-patterning of titanium by ultrafast laser for surface coloring and optical encryption. Adv. Opt. Mater. 2022, 10, 2101673. [Google Scholar] [CrossRef]
- Zhuang, Z.P.; Chen, R.; Fan, Z.B.; Pang, X.N.; Dong, J.W. High focusing efficiency in subdiffraction focusing metalens. Nanophotonics 2019, 8, 1279–1289. [Google Scholar] [CrossRef]
- Cai, J.; Zhang, F.; Zhang, M.; Ou, Y.; Yu, H. Simultaneous polarization filtering and wavefront shaping enabled by localized polarization-selective interference. Sci. Rep. 2020, 10, 14477. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gan, S.; Zhao, T.; Mei, X.; Zhang, T.; Wang, Z.; Gao, H.; Yang, G.; Cai, J.; Bai, F. Broadband Spin-Selective Wavefront Manipulations with Generalized Pancharatnam–Berry Phase Metasurface. Photonics 2024, 11, 690. https://doi.org/10.3390/photonics11080690
Gan S, Zhao T, Mei X, Zhang T, Wang Z, Gao H, Yang G, Cai J, Bai F. Broadband Spin-Selective Wavefront Manipulations with Generalized Pancharatnam–Berry Phase Metasurface. Photonics. 2024; 11(8):690. https://doi.org/10.3390/photonics11080690
Chicago/Turabian StyleGan, Shiming, Tianci Zhao, Xiuzhuang Mei, Tingting Zhang, Zhiqi Wang, Hongyu Gao, Gensen Yang, Jixiang Cai, and Fuzhong Bai. 2024. "Broadband Spin-Selective Wavefront Manipulations with Generalized Pancharatnam–Berry Phase Metasurface" Photonics 11, no. 8: 690. https://doi.org/10.3390/photonics11080690
APA StyleGan, S., Zhao, T., Mei, X., Zhang, T., Wang, Z., Gao, H., Yang, G., Cai, J., & Bai, F. (2024). Broadband Spin-Selective Wavefront Manipulations with Generalized Pancharatnam–Berry Phase Metasurface. Photonics, 11(8), 690. https://doi.org/10.3390/photonics11080690




