Characterization of Single Frequency Fiber-Laser-Based Ultrasound Sensor
Abstract
1. Introduction
2. Simulation and Theoretical Analysis
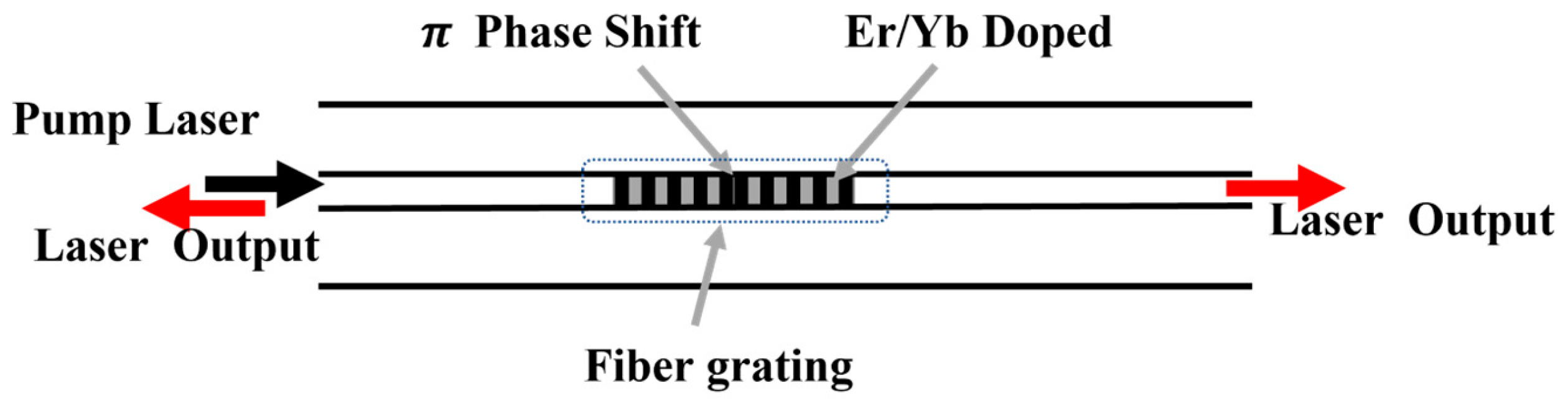
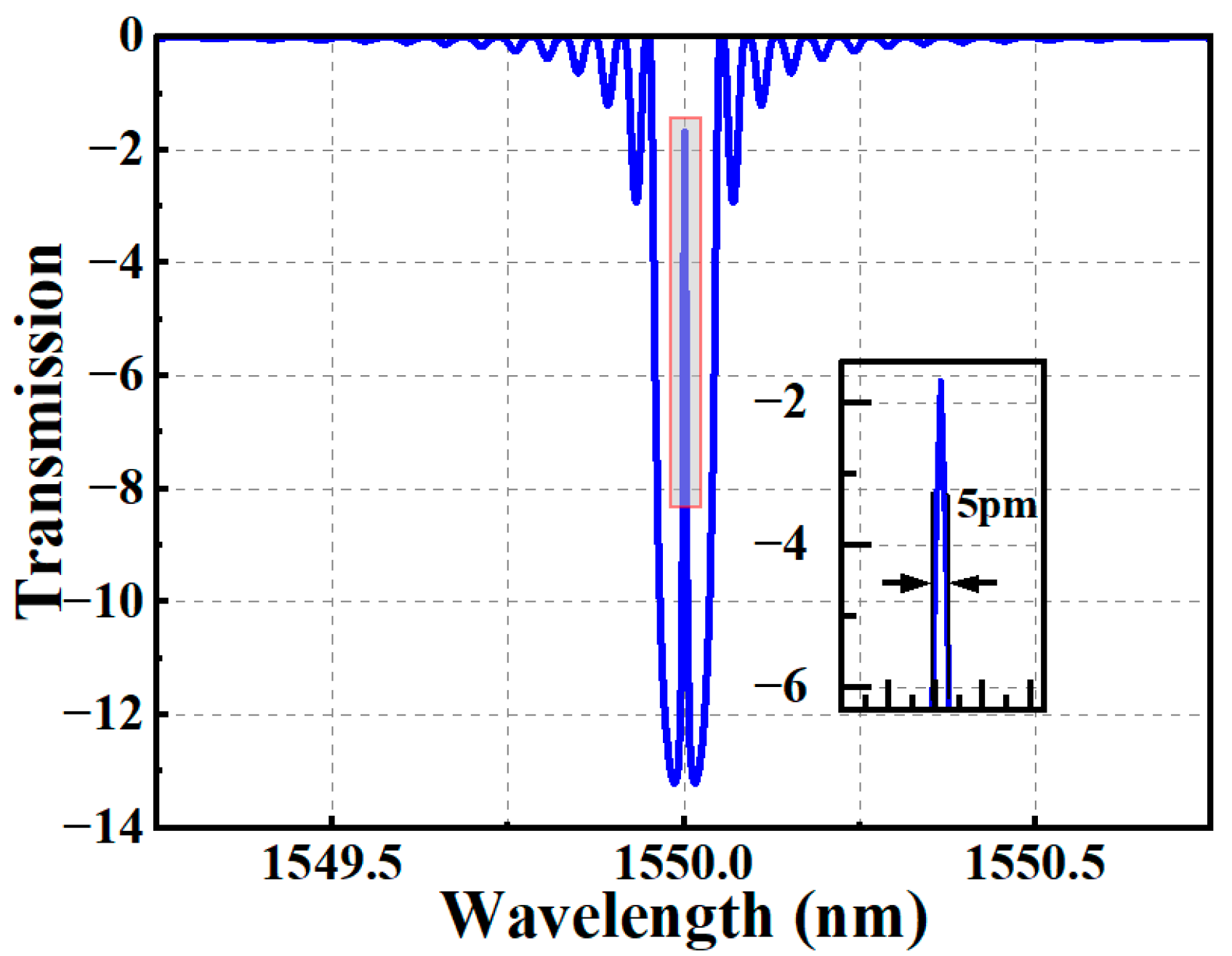
2.1. Simulation of the π-FBG
2.2. Sensing Method
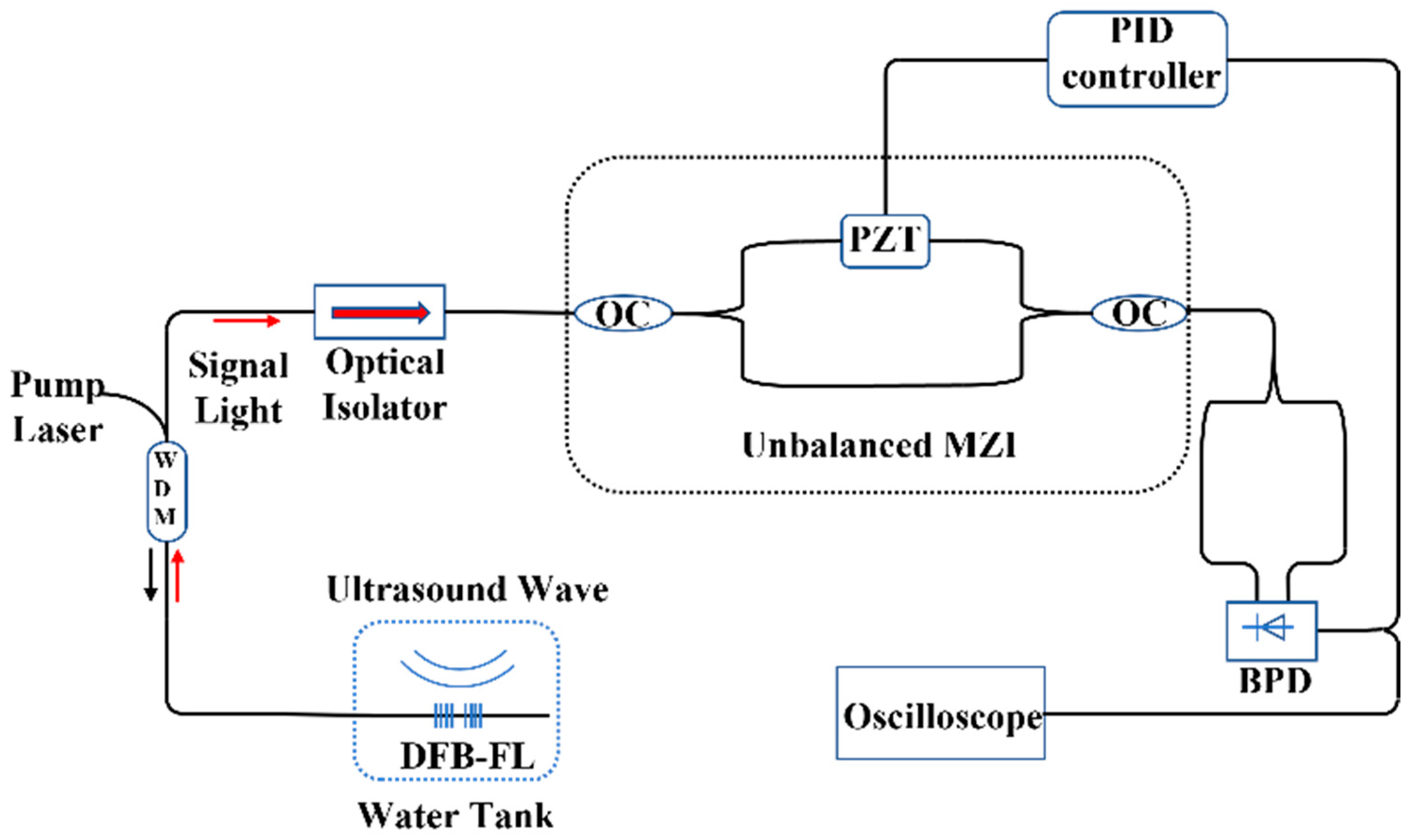
2.3. Demodulation Method
3. Experiment and Results
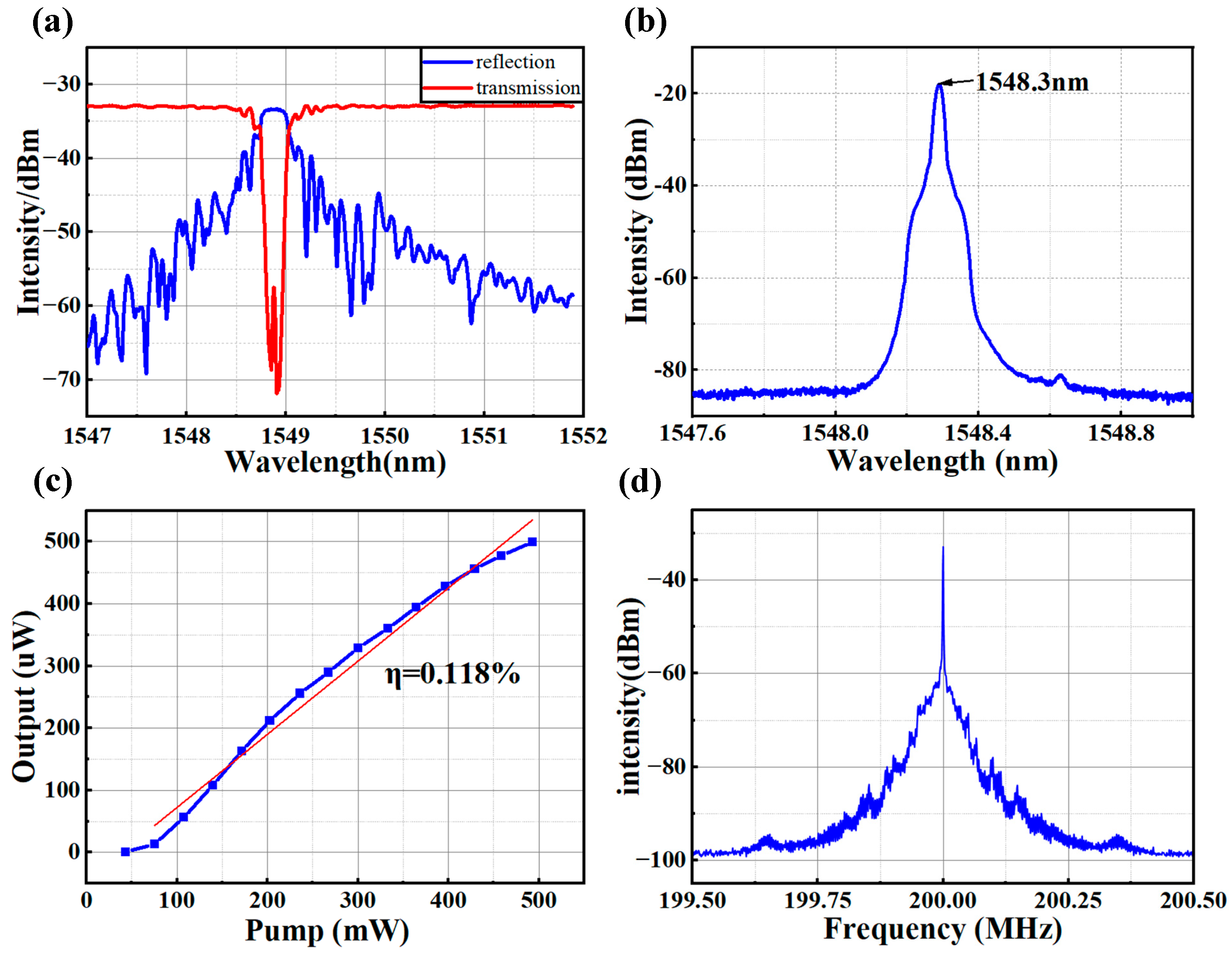
3.1. Laser Output Measurement
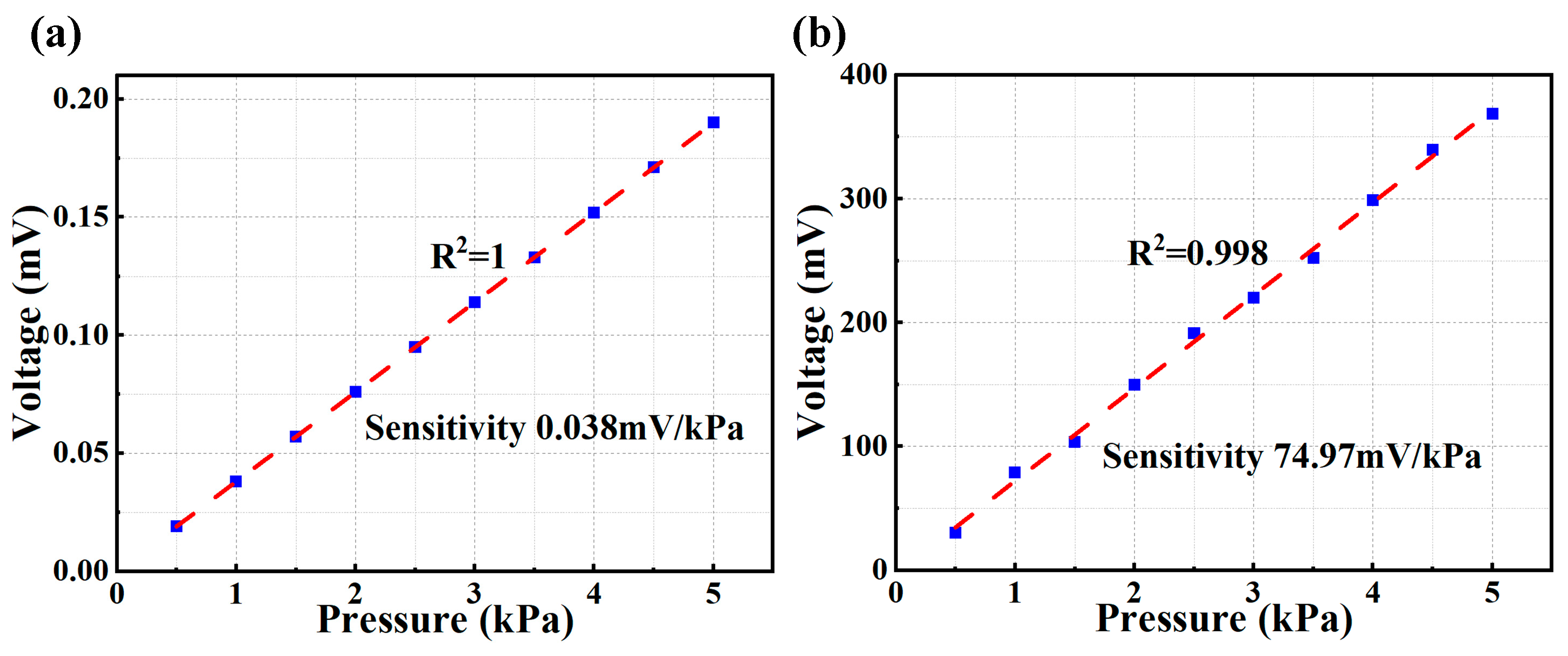
3.2. Ultrasound Sensing Setup and Result
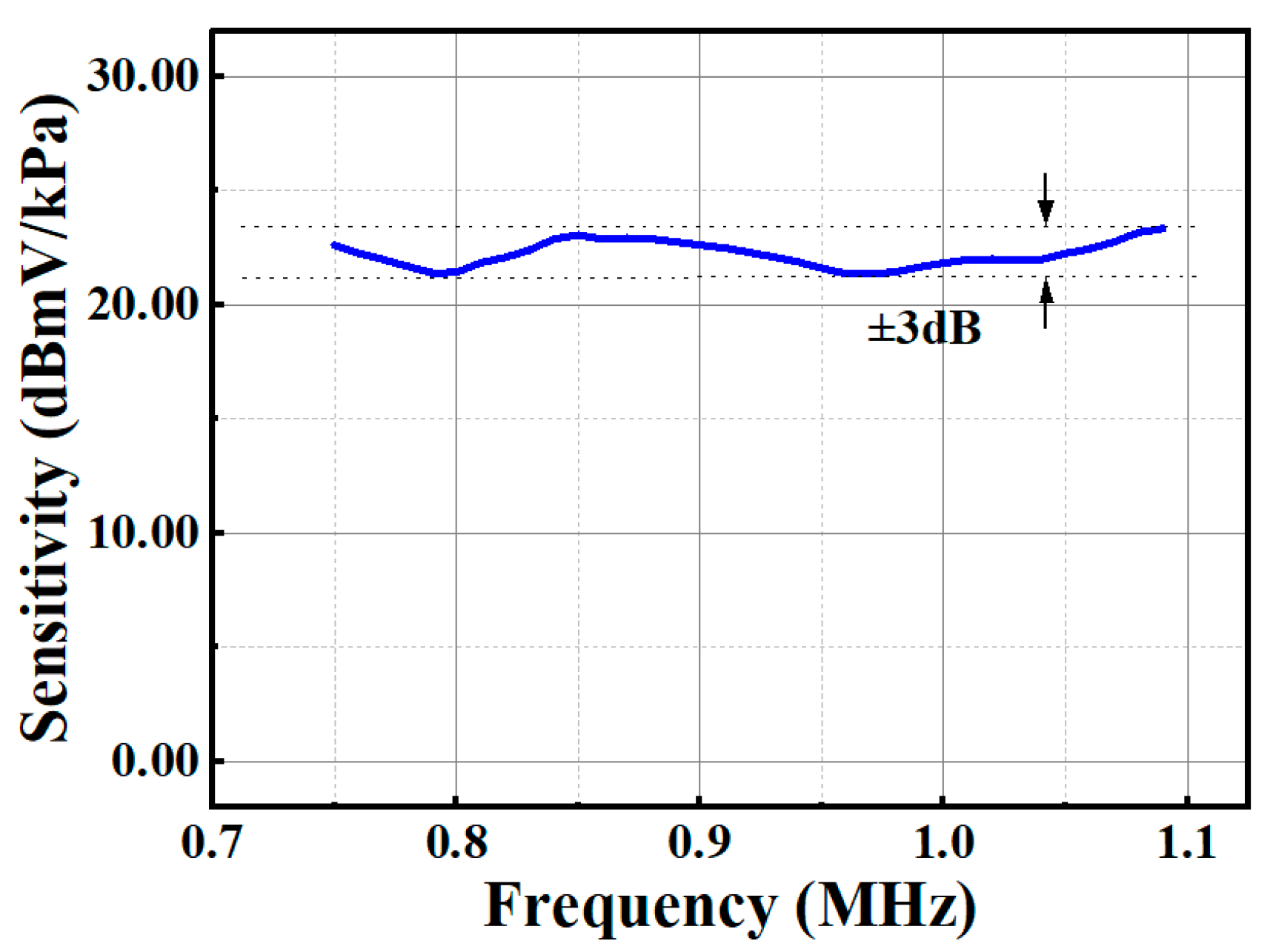
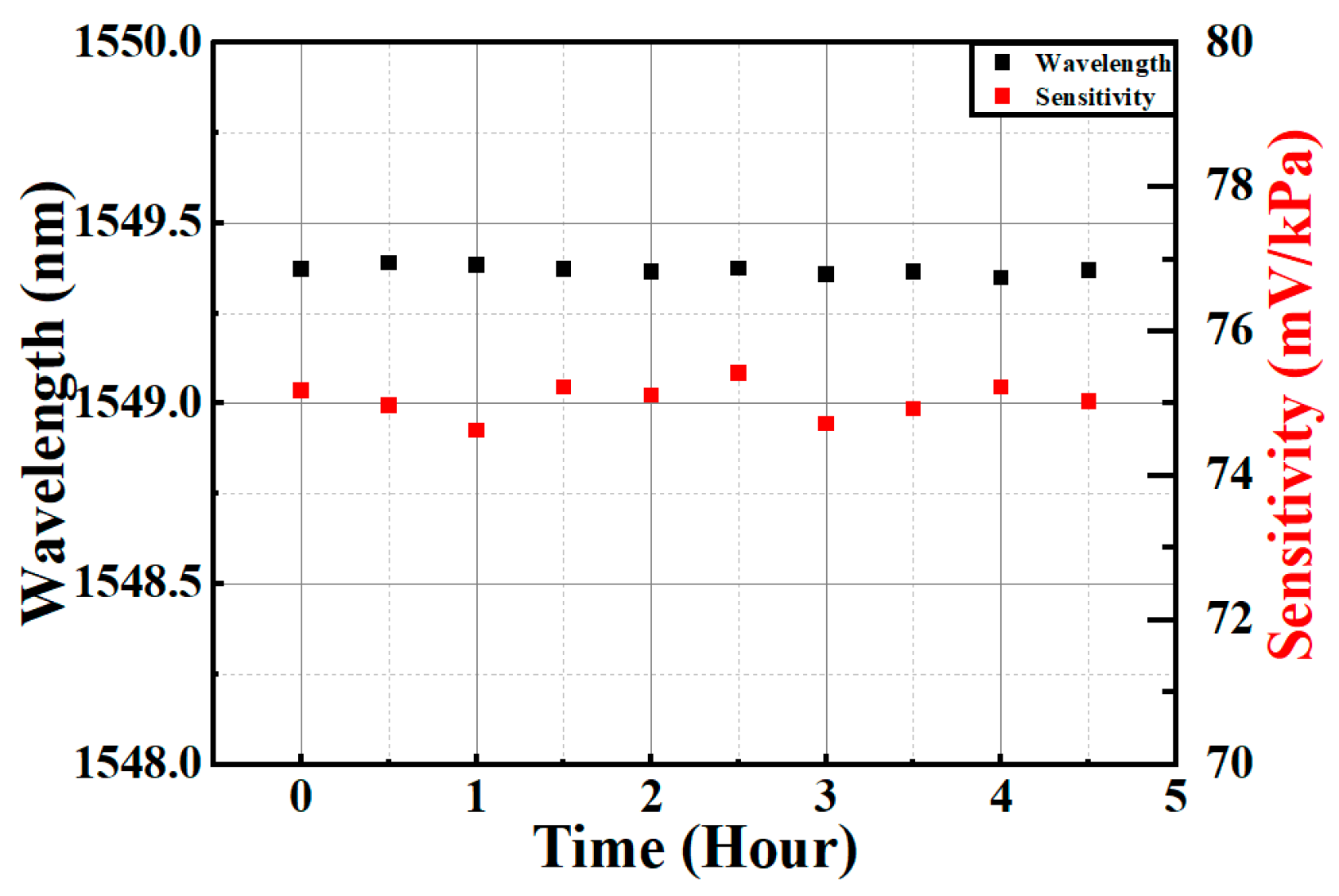
3.3. Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Taruttis, A.; Ntziachristos, V. Advances in real-time multispectral optoacoustic imaging and its applications. Nat. Photonics 2015, 9, 219–227. [Google Scholar] [CrossRef]
- Qiu, Y.; Gigliotti, J.V.; Wallace, M.; Griggio, F.; Demore, C.E.; Cochran, S.; Trolier-McKinstry, S. Piezoelectric micromachined ultrasound transducer (PMUT) arrays for integrated sensing, actuation and imaging. Sensors 2015, 15, 8020–8041. [Google Scholar] [CrossRef] [PubMed]
- Rosenthal, A.; Razansky, D.; Ntziachristos, V. High-sensitivity compact ultrasonic detector based on a pi-phase-shifted fiber Bragg grating. Opt. Lett. 2011, 36, 1833–1835. [Google Scholar] [CrossRef] [PubMed]
- Guggenheim, J.A.; Li, J.; Allen, T.J.; Colchester, R.J.; Noimark, S.; Ogunlade, O.; Parkin, I.P.; Papakonstantinou, I.; Desjardins, A.E.; Zhang, E.Z. Ultrasensitive plano-concave optical microresonators for ultrasound sensing. Nat. Photonics 2017, 11, 714–719. [Google Scholar] [CrossRef]
- Ma, X.D.; Cai, Y.Q.; Fu, B.; Xu, L.J.; Ma, J.G. Fiber optic-based laser interferometry array for three-dimensional ultrasound sensing. Opt. Lett. 2019, 44, 5852–5855. [Google Scholar] [CrossRef]
- Wang, X.X.; Jiang, Y.H.; Li, Z.Y.; Wang, W.; Li, Z.B. Sensitivity Characteristics of Microfiber Fabry-Perot Interferometric Photoacoustic Sensors. J. Light. Technol. 2019, 37, 4229–4235. [Google Scholar] [CrossRef]
- Bai, X.; Qi, Y.; Liang, Y.; Ma, J.; Jin, L.; Guan, B.-o. Photoacoustic computed tomography with lens-free focused fiber-laser ultrasound sensor. Biomed. Opt. Express 2019, 10, 2504–2512. [Google Scholar] [CrossRef]
- Nuster, R.; Gratt, S.; Passler, K.; Grün, H.; Berer, T.; Burgholzer, P.; Paltauf, G. Comparison of optical and piezoelectric integrating line detectors. In Proceedings of the Photons Plus Ultrasound: Imaging and Sensing, San Jose, CA, USA, 24–29 January 2009; pp. 220–227. [Google Scholar]
- Liang, Y.; Jin, L.; Wang, L.; Bai, X.; Cheng, L.; Guan, B.-O. Fiber-laser-based ultrasound sensor for photoacoustic imaging. Sci. Rep. 2017, 7, 40849. [Google Scholar] [CrossRef]
- Zhu, Y.; Hu, L.; Liu, Z.; Han, M. Ultrasensitive ultrasound detection using an intracavity phase-shifted fiber Bragg grating in a self-injection-locked diode laser. Opt. Lett. 2019, 44, 5525–5528. [Google Scholar] [CrossRef]
- Pang, Y.; Ma, S.; Zhao, X.; Qin, Z.; Liu, Z.; Xu, Y. Broadband high-sensitivity acoustic sensing based on Brillouin random fiber laser. Opt. Laser Technol. 2023, 161, 109195. [Google Scholar] [CrossRef]
- Yan, L.S.; Yi, A.; Pan, W.; Luo, B. A simple demodulation method for FBG temperature sensors using a narrow band wavelength tunable DFB laser. IEEE Photonics Technol. Lett. 2010, 22, 1391–1393. [Google Scholar] [CrossRef]
- Foster, S.B.; Cranch, G.A.; Harrison, J.; Tikhomirov, A.E.; Miller, G.A. Distributed feedback fiber laser strain sensor technology. J. Light. Technol. 2017, 35, 3514–3530. [Google Scholar] [CrossRef]
- Muanenda, Y.; Oton, C.J.; Faralli, S.; Di Pasquale, F. A cost-effective distributed acoustic sensor using a commercial off-the-shelf DFB laser and direct detection phase-OTDR. IEEE Photonics J. 2015, 8, 6800210. [Google Scholar] [CrossRef]
- Dandridge, A.; Tveten, A.B.; Giallorenzi, T.G. Homodyne demodulation scheme for fiber optic sensors using phase generated carrier. IEEE Trans. Microw. Theory Tech. 1982, 30, 1635–1641. [Google Scholar] [CrossRef]
- Leung, I.; Brodzeli, Z.; Whitbread, T.; Chen, X.B.; Peng, G.D. A distributed-feedback fiber-laser-based optical fiber hydrophone system with very high sensitivity. In Proceedings of the Photonics Asia, Beijing, China, 8–11 November 2004; pp. 434–443. [Google Scholar]
- Hansen, L.V.; Kullander, F. Modelling of hydrophone based on a DFB fiber laser. In Proceedings of the 21st International Congress of Theoretical and Applied Mechanics, Warsaw, Poland, 15–21 August 2004. [Google Scholar]
- Lovseth, S.W.; Stepanov, D.Y. Analysis of multiple wavelength DFB fiber lasers. IEEE J. Quantum Electron. 2001, 37, 770–780. [Google Scholar] [CrossRef]
- Lovseth, S.W.; Stepanov, D.Y. Dynamic analysis of multiple wavelength DFB fiber lasers. IEEE J. Quantum Electron. 2001, 37, 1237–1245. [Google Scholar] [CrossRef]
- Foster, S.; Tikhomirov, A.; Milnes, M.; Van Velzen, J.; Hardy, G. A fiber laser hydrophone. In Proceedings of the 17th International Conference on Optical Fibre Sensors, Bruges, Belgium, 23–27 May 2005; pp. 627–630. [Google Scholar]
- Goodman, S.; Tikhomirov, A.; Foster, S. Pressure compensated distributed feedback fibre laser hydrophone. In Proceedings of the 19th International Conference on Optical Fibre Sensors, Perth, WA, Australia, 14–18 April 2008; pp. 320–323. [Google Scholar]
- Vivek, K.; Rajesh, R.; Sreehari, C.V.; Sham Kumar, S.; Shajahan, K.; Praveen, T.V.; Santhanakrishnan, T.; Moosad, K.P.B. A new approach of large diameter polymer-coated fiber laser hydrophone. J. Light. Technol. 2017, 35, 4097–4104. [Google Scholar] [CrossRef]
- Ma, L.; Hu, Y.; Luo, H.; Hu, Z. DFB fiber laser hydrophone with flat frequency response and enhanced acoustic pressure sensitivity. IEEE Photonics Technol. Lett. 2009, 21, 1280–1282. [Google Scholar] [CrossRef]
- Yang, L.; Li, Y.; Fang, F.; Li, L.; Yan, Z.; Zhang, L.; Sun, Q. Highly sensitive and miniature microfiber-based ultrasound sensor for photoacoustic tomography. Opto-Electron. Adv. 2022, 5, 200076-1–200076-8. [Google Scholar] [CrossRef]
- Erdogan, T. Fiber grating spectra. J. Light. Technol. 1997, 15, 1277–1294. [Google Scholar] [CrossRef]
- Xiong, S.D. Fibre Optic Vector Hydrophone Research. Ph.D. Thesis, National University of Defense Technology, Changsha, China, 2004. [Google Scholar]
- Liu, Y.; Lin, H.; Dai, Y.; Zhou, A.; Yuan, L. Humidity sensor based on an in-fiber integrated Mach–Zehnder interferometer. IEEE Photonics Technol. Lett. 2019, 31, 393–396. [Google Scholar] [CrossRef]
- Cui, M.; Huang, J.; Yang, X. Review on Methods for Laser Linewidth Measurement. Laser Optoelectron. Prog. 2021, 58, 0900005. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, W.; Lu, Q.; Yang, B.; Tian, R.; Luo, H.; Cai, C.; Yan, Z.; Zhao, L. Characterization of Single Frequency Fiber-Laser-Based Ultrasound Sensor. Photonics 2024, 11, 654. https://doi.org/10.3390/photonics11070654
Zhu W, Lu Q, Yang B, Tian R, Luo H, Cai C, Yan Z, Zhao L. Characterization of Single Frequency Fiber-Laser-Based Ultrasound Sensor. Photonics. 2024; 11(7):654. https://doi.org/10.3390/photonics11070654
Chicago/Turabian StyleZhu, Wei, Qiang Lu, Bo Yang, Rui Tian, Hao Luo, Chao Cai, Zhijun Yan, and Luming Zhao. 2024. "Characterization of Single Frequency Fiber-Laser-Based Ultrasound Sensor" Photonics 11, no. 7: 654. https://doi.org/10.3390/photonics11070654
APA StyleZhu, W., Lu, Q., Yang, B., Tian, R., Luo, H., Cai, C., Yan, Z., & Zhao, L. (2024). Characterization of Single Frequency Fiber-Laser-Based Ultrasound Sensor. Photonics, 11(7), 654. https://doi.org/10.3390/photonics11070654





