Single-Source VLCP System Based on Solar Cell Array Receiver and Right-Angled Tetrahedron Trilateration VLP (RATT-VLP) Algorithm
Abstract
1. Introduction
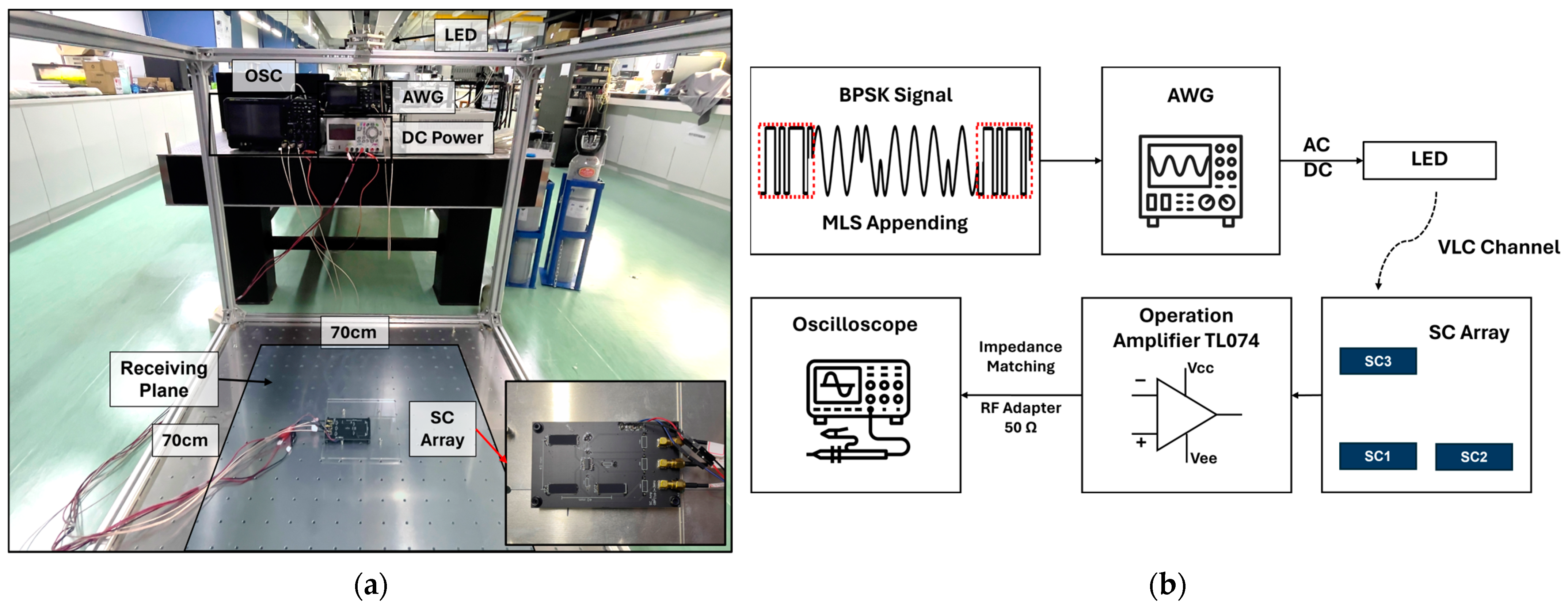
2. System Mechanism and Setup
2.1. VLCP System Mechanism
2.2. Experimental Setup
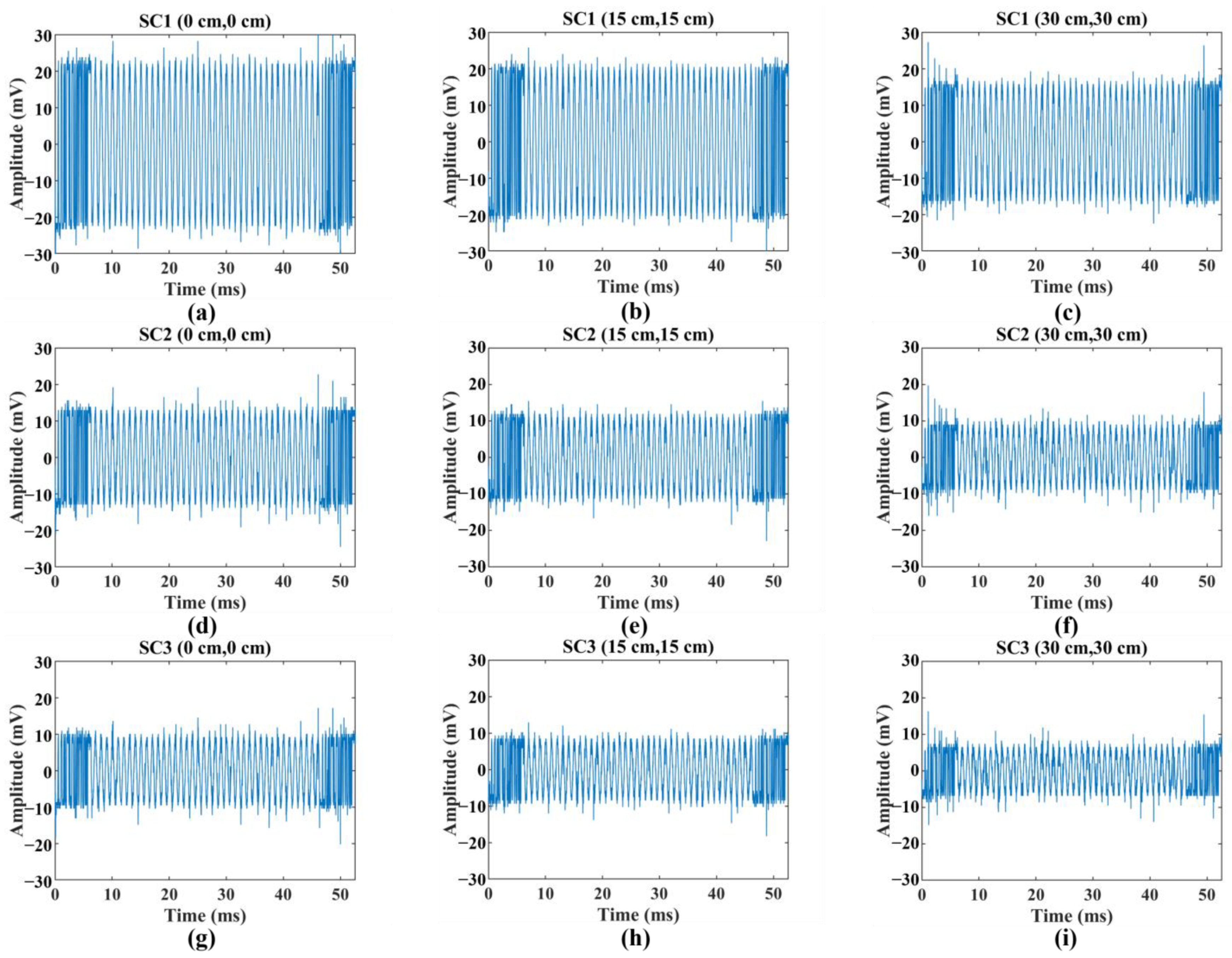
3. Results and Discussion
4. Future Work
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Pathak, P.H.; Feng, X.; Hu, P.; Mohapatra, P. Visible light communication, networking, and sensing: A survey, potential and challenges. IEEE Commun. Surv. Tutor. 2015, 17, 2047–2077. [Google Scholar] [CrossRef]
- Karunatilaka, D.; Zafar, F.; Kalavally, V.; Parthiban, R. LED based indoor visible light communications: State of the art. IEEE Commun. Surv. Tutor. 2015, 17, 1649–1678. [Google Scholar] [CrossRef]
- Lee, S.H.; Jung, S.Y.; Kwon, J.K. Modulation and coding for dimmable visible light communication. IEEE Commun. Mag. 2015, 53, 136–143. [Google Scholar] [CrossRef]
- Lou, P.; Zhang, H.; Zhang, X.; Yao, M.; Xu, Z. Fundamental analysis for indoor visible light positioning system. In Proceedings of the 2012 1st IEEE International Conference on Communications in China Workshops (ICCC), Beijing, China, 15–17 August 2012; pp. 59–63. [Google Scholar] [CrossRef]
- Komine, T.; Nakagawa, M. Integrated system of white LED visible-light communication and power-line communication. IEEE Trans. Consum. Electron. 2003, 49, 71–79. [Google Scholar] [CrossRef]
- Liu, Z.; Yu, C. Multi-user visible light communication and positioning system based on dual-domain multiplexing scheme. Photonics 2023, 10, 306. [Google Scholar] [CrossRef]
- Yu, C.; You, X.; Liu, Z.; Chen, J. Conceptual design for indoor visible light communication and positioning cooperative systems. In Proceedings of the 22nd International Conference on Transparent Optical Networks (ICTON) 2020, Bari, Italy, 19–23 July 2020. [Google Scholar] [CrossRef]
- Zhang, Y.; Wei, Z.; Liu, Z.; Cheng, C.; Wang, Z.; Tang, X.; Yang, Y.; Fu, H.Y. Optical communication and positioning convergence for flexible underwater wireless sensor network. J. Light. Technol. 2023, 41, 5321–5327. [Google Scholar] [CrossRef]
- Shao, S.; Salustri, A.; Khreishah, A.; Xu, C.; Ma, S. R-VLCP: Channel Modeling and Simulation in Retroreflective Visible Light Communication and Positioning Systems. IEEE Internet Things J. 2023, 10, 11429–11439. [Google Scholar] [CrossRef]
- Yang, H.; Chen, C.; Zhong, W.D.; Alphones, A.; Zhang, S.; Du, P. Demonstration of a Quasi-Gapless Integrated Visible Light Communication and Positioning System. IEEE Photonics Technol. Lett. 2018, 30, 2001–2004. [Google Scholar] [CrossRef]
- Kim, S.M.; Won, J.S.; Nahm, S.H. Simultaneous reception of solar power and visible light communication using a solar cell. Opt. Eng. 2014, 53, 046103. [Google Scholar] [CrossRef]
- Wang, Z.; Tsonev, D.; Videv, S.; Haas, H. On the design of a solar-panel receiver for optical wireless communications with simultaneous energy harvesting. IEEE J. Sel. Areas Commun. 2015, 33, 1612–1623. [Google Scholar] [CrossRef]
- Shin, W.H.; Yang, S.H.; Kwon, D.H.; Han, S.K. Self-reverse-biased solar panel optical receiver for simultaneous visible light communication and energy harvesting. Opt. Express 2016, 24, A1300–A1305. [Google Scholar] [CrossRef] [PubMed]
- Diamantoulakis, P.D.; Karagiannidis, G.K.; Ding, Z. Simultaneous lightwave information and power transfer (SLIPT). IEEE Trans. Green Commun. Netw. 2018, 2, 764–773. [Google Scholar] [CrossRef]
- Wang, Z.; Tsonev, D.; Videv, S.; Haas, H. Towards self-powered solar panel receiver for optical wireless communication. In Proceedings of the 2014 IEEE International Conference on Communications (ICC), Sydney, NSW, Australia, 10–14 June 2014; pp. 3348–3353. [Google Scholar] [CrossRef]
- Lorrière, N.; Bialic, E.; Pasquinelli, M.; Chabriel, G.; Barrere, J.; Escoubas, L.; Simon, J.J. An OFDM testbed for LiFi performance characterization of photovoltaic modules. In Proceedings of the 2018 Global LIFI Congress (GLC), Paris, France, 8–9 February 2018; pp. 1–5. [Google Scholar] [CrossRef]
- Sepehrvand, S.; Theagarajan, L.N.; Hranilovic, S. Rate-power trade-off in simultaneous lightwave information and power transfer systems. IEEE Commun. Lett. 2020, 25, 1249–1253. [Google Scholar] [CrossRef]
- Fakidis, J.; Helmers, H.; Haas, H. Simultaneous wireless data and power transfer for a 1-Gb/s GaAs VCSEL and photovoltaic link. IEEE Photonics Technol. Lett. 2020, 32, 1277–1280. [Google Scholar] [CrossRef]
- Das, S.; Sparks, A.; Poves, E.; Videv, S.; Fakidis, J.; Haas, H. Effect of sunlight on photovoltaics as optical wireless communication receivers. J. Light. Technol. 2021, 39, 6182–6190. [Google Scholar] [CrossRef]
- Hsu, C.W.; Wu, J.T.; Wang, H.Y.; Chow, C.W.; Lee, C.H.; Chu, M.T.; Yeh, C.H. Visible light positioning and lighting based on identity positioning and RF carrier allocation technique using a solar cell receiver. IEEE Photonics J. 2016, 8, 7905507. [Google Scholar] [CrossRef]
- Wang, Z.; Zhong, W.-D.; Yu, C.; Chen, J.; Francois, C.P.S.; Chen, W. Performance of dimming control scheme in visible light communication system. Opt. Express 2012, 20, 18861–18868. [Google Scholar] [CrossRef] [PubMed]
- Neokosmidis, I.; Kamalakis, T.; Walewski, J.W.; Inan, B.; Sphicopoulos, T. Impact of Nonlinear LED Transfer Function on Discrete Multitone Modulation: Analytical Approach. J. Light. Technol. 2009, 27, 4970–4978. [Google Scholar] [CrossRef]
- Komine, T.; Nakagawa, M. Fundamental analysis for visible-light communication system using LED lights. IEEE Trans. Consum. Electron. 2004, 50, 100–107. [Google Scholar] [CrossRef]
- Raes, W.; Knudde, N.; De Bruycker, J.; Dhaene, T.; Stevens, N. Experimental Evaluation of Machine Learning Methods for Robust Received Signal Strength-Based Visible Light Positioning. Sensors 2020, 20, 6109. [Google Scholar] [CrossRef]
- Dos Santos, D.R.; Julien-Vergonjanne, A.; Dkhil, S.B.; Parmentier, M.; Combeau, P.; Sahuguède, S.; Bouclé, J. Toward Indoor Simulations of OPV Cells for Visible Light Communication and Energy Harvesting. IEEE Access 2024, 12, 41027–41041. [Google Scholar] [CrossRef]
- Chen, S.; Liu, L.; Chen, L.K. Passive Nonlinear Compensation Circuits for Photovoltaic Visible Light Communications under Low Illuminance. In Proceedings of the Optical Fiber Communication Conference (OFC) 2022, San Diego, CA, USA, 6–10 March 2022; p. W2A.32. [Google Scholar]
- Fu, Y.; Zhang, L.; Wangzhou, Y.; Zheng, S.; Sun, Y.; Liu, J.; Zhang, Y.; Li, S.; Hu, Z.; Yu, B.; et al. Wavelength division multiplexing visible light communication with wide incident angle enabled by perovskite quantum dots. Opt. Commun. 2024, 559, 130427. [Google Scholar] [CrossRef]






| Ref | Illumination | Receiver Type | Rate | Positioning Error | Note |
|---|---|---|---|---|---|
| This work | Single white LED | Monocrystalline Si SC Array | 1.21 kbps | Narrow-range 3.40 cm; wide-range 10.49 cm | First single-source SC-VLCP |
| [12] | Single white LED | α-Si SC Panel | 11.84 Mbps (OFDM) | N/A | VLC |
| [24] | 4 White LEDs | Si PD | N/A | 4 cm | VLP |
| [10] | 3 White LEDs | APD | N/A | 6.08 cm | VLP |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xie, D.; Liu, Z.; Yu, C. Single-Source VLCP System Based on Solar Cell Array Receiver and Right-Angled Tetrahedron Trilateration VLP (RATT-VLP) Algorithm. Photonics 2024, 11, 536. https://doi.org/10.3390/photonics11060536
Xie D, Liu Z, Yu C. Single-Source VLCP System Based on Solar Cell Array Receiver and Right-Angled Tetrahedron Trilateration VLP (RATT-VLP) Algorithm. Photonics. 2024; 11(6):536. https://doi.org/10.3390/photonics11060536
Chicago/Turabian StyleXie, Dawei, Zhongxu Liu, and Changyuan Yu. 2024. "Single-Source VLCP System Based on Solar Cell Array Receiver and Right-Angled Tetrahedron Trilateration VLP (RATT-VLP) Algorithm" Photonics 11, no. 6: 536. https://doi.org/10.3390/photonics11060536
APA StyleXie, D., Liu, Z., & Yu, C. (2024). Single-Source VLCP System Based on Solar Cell Array Receiver and Right-Angled Tetrahedron Trilateration VLP (RATT-VLP) Algorithm. Photonics, 11(6), 536. https://doi.org/10.3390/photonics11060536







