A 930 m/180 Gbps*User Underwater Coherent Optical Code-Division Multiple-Access Network Based on Hybrid 256-Differential Pulse Position Modulation and Weighted Modified Prime Code Sequence
Abstract
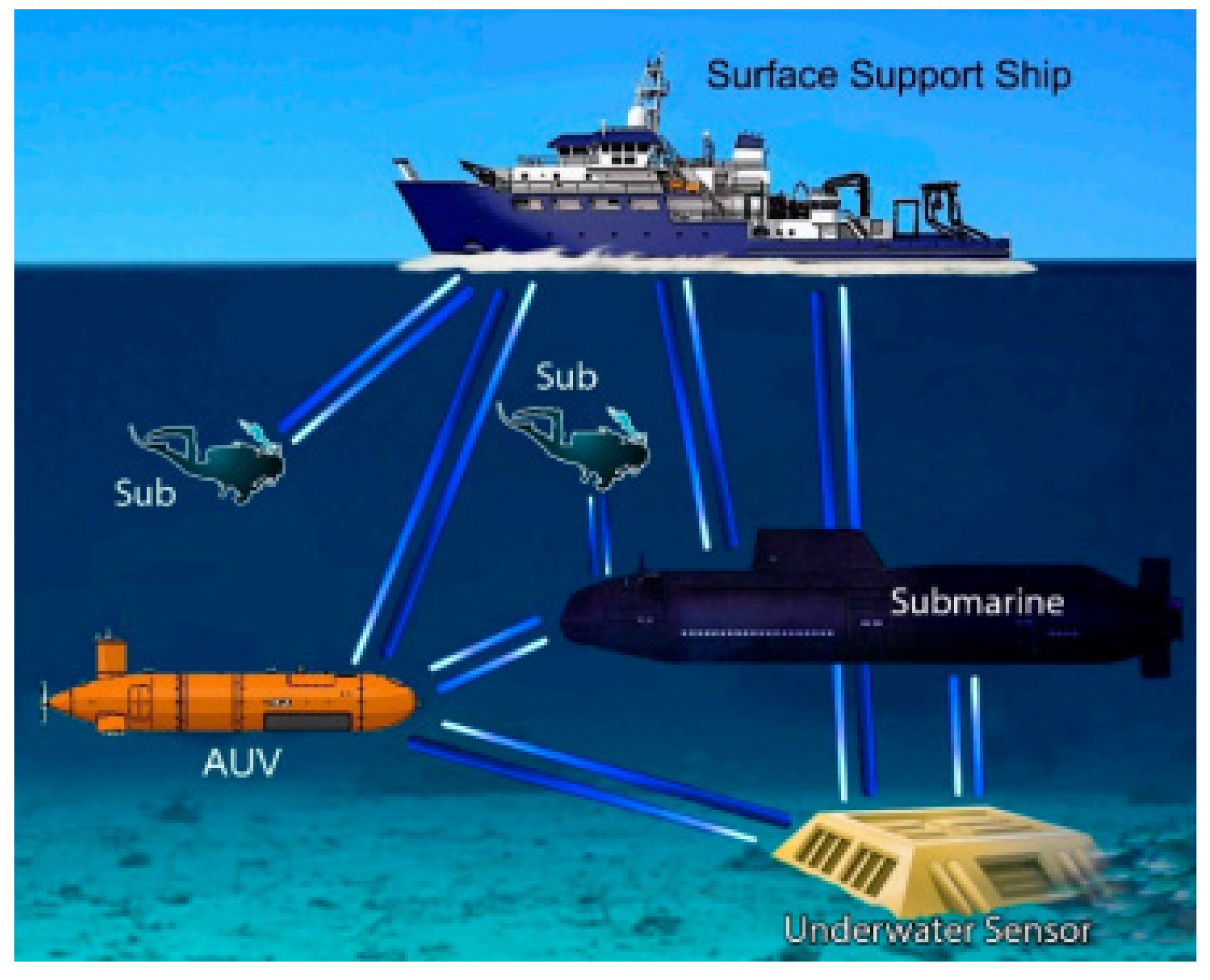
1. Introduction
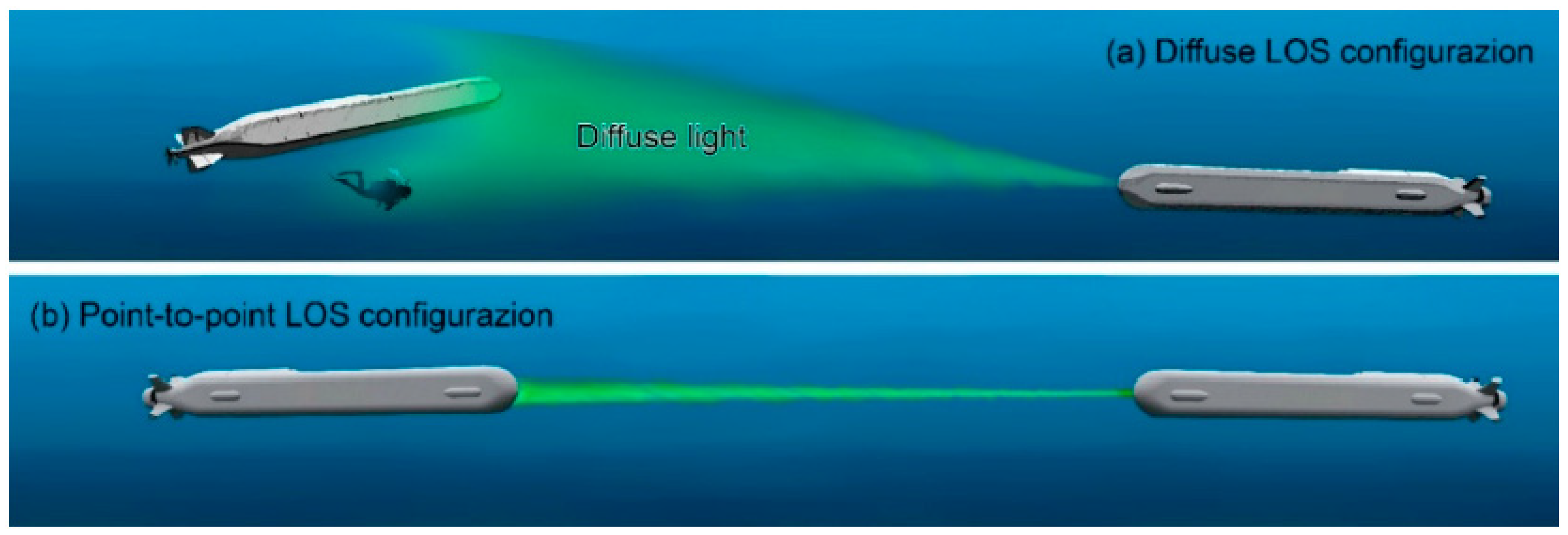
2. Literature Review
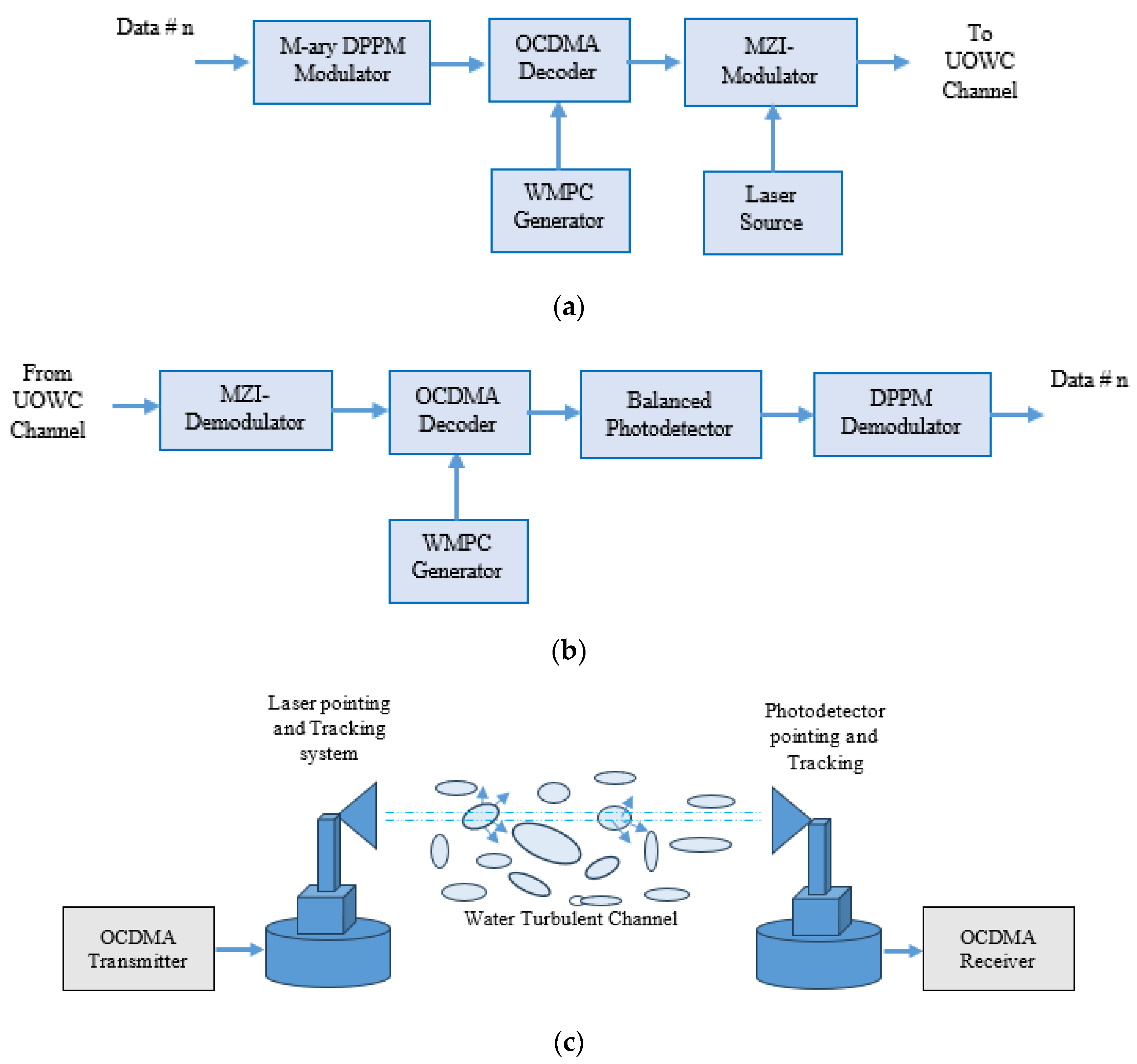
3. Proposed System Model
4. WMPC Sequence Generation
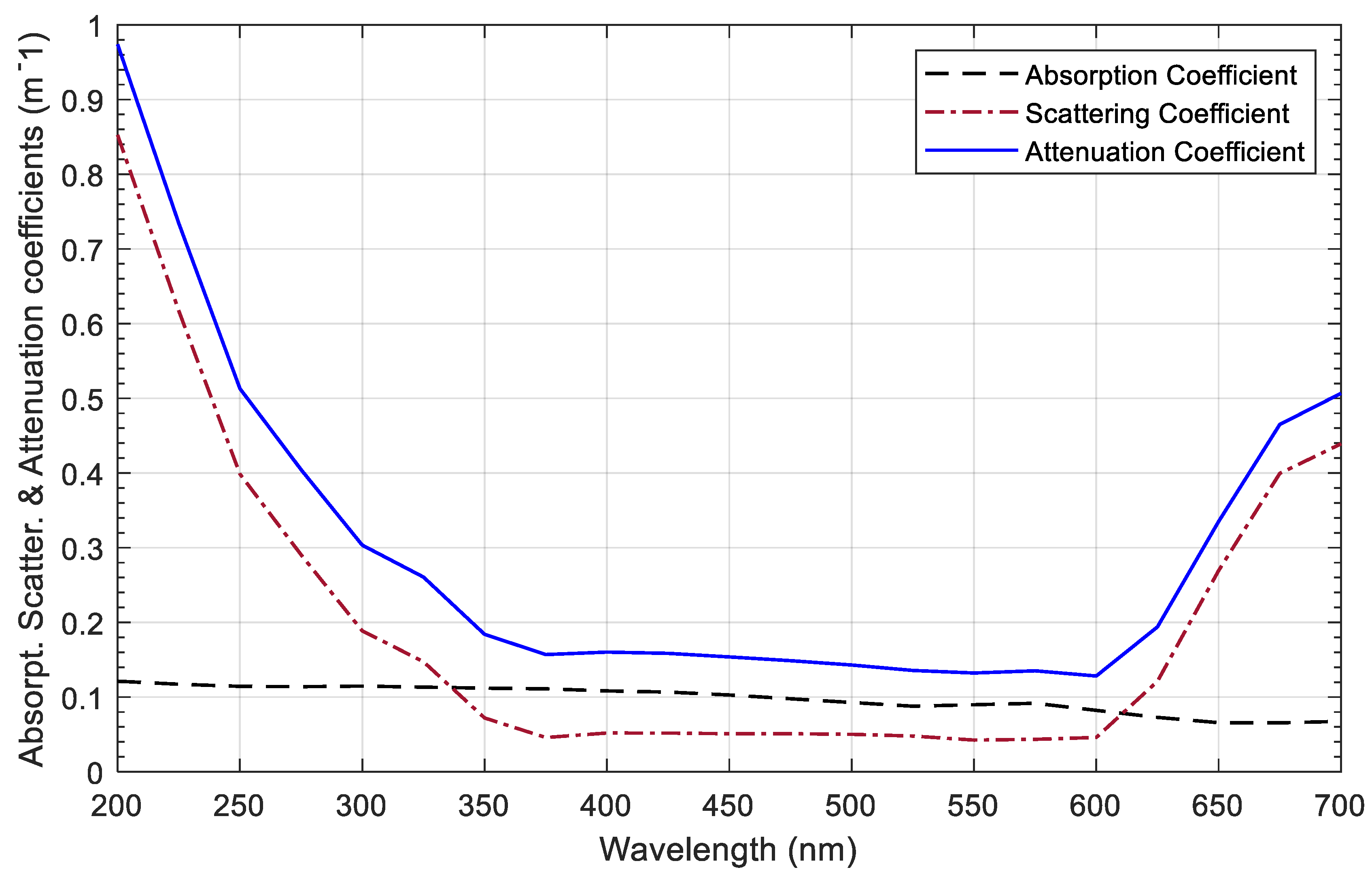
5. Performance Analysis
6. EVM Analysis
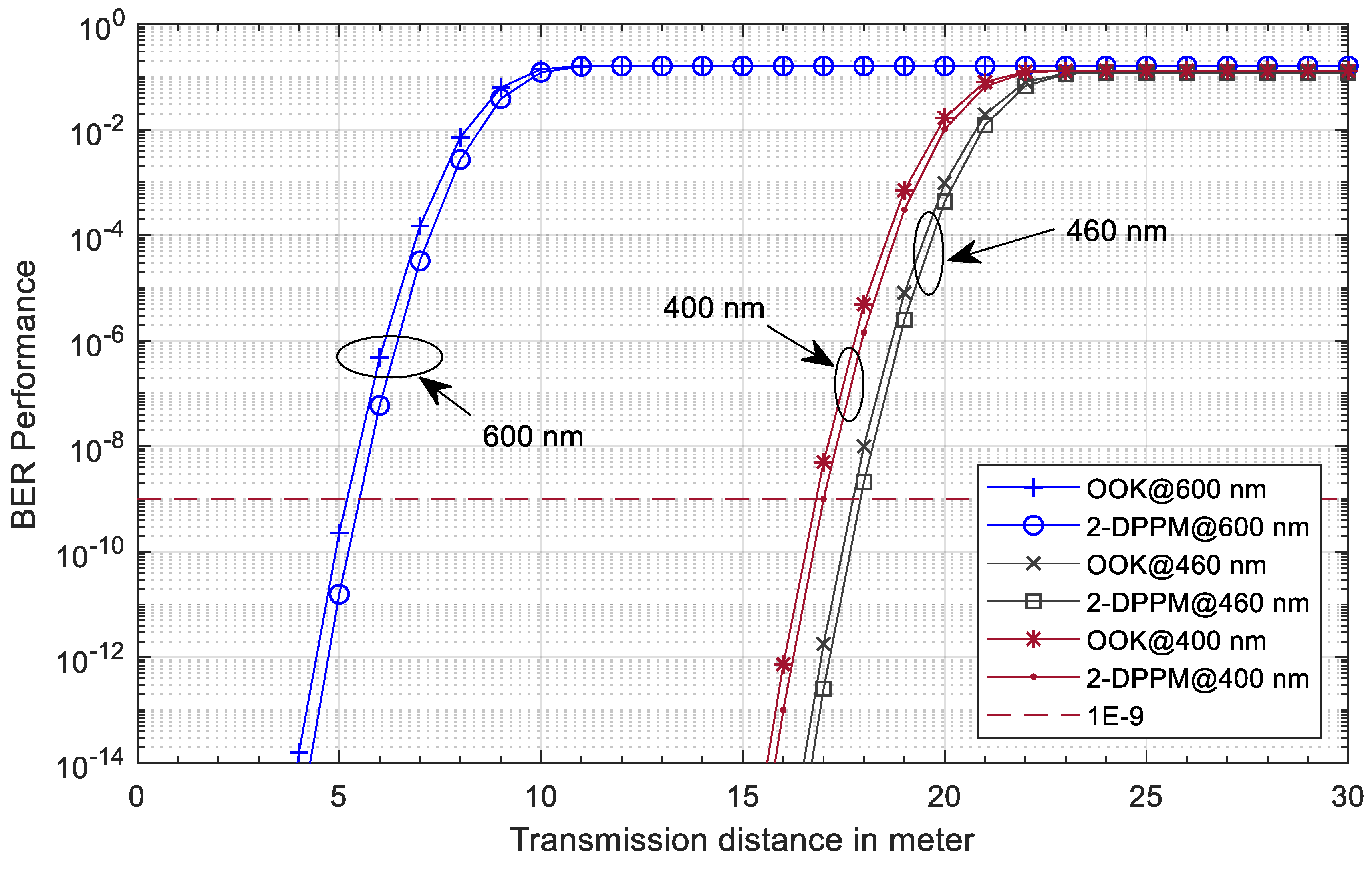
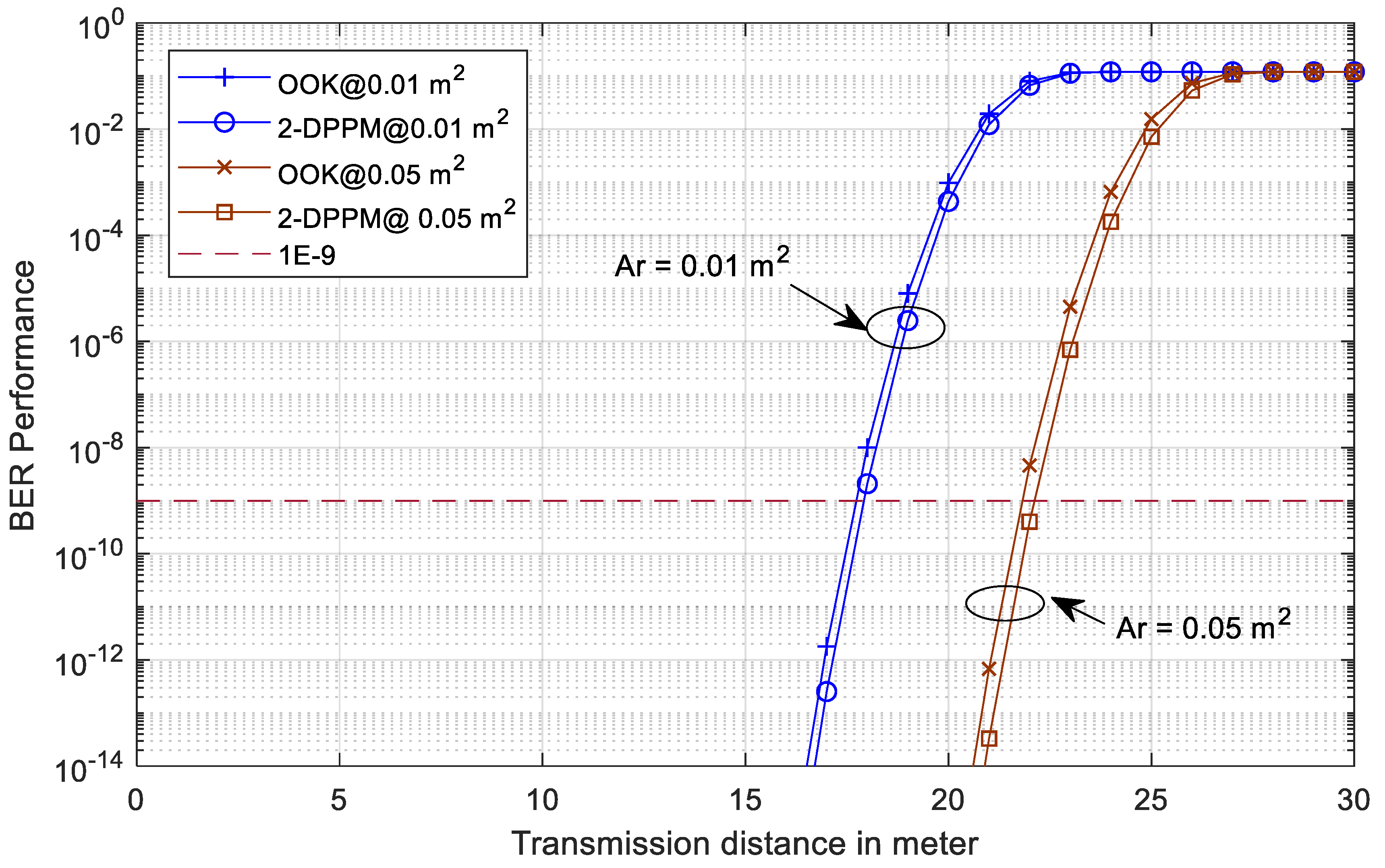
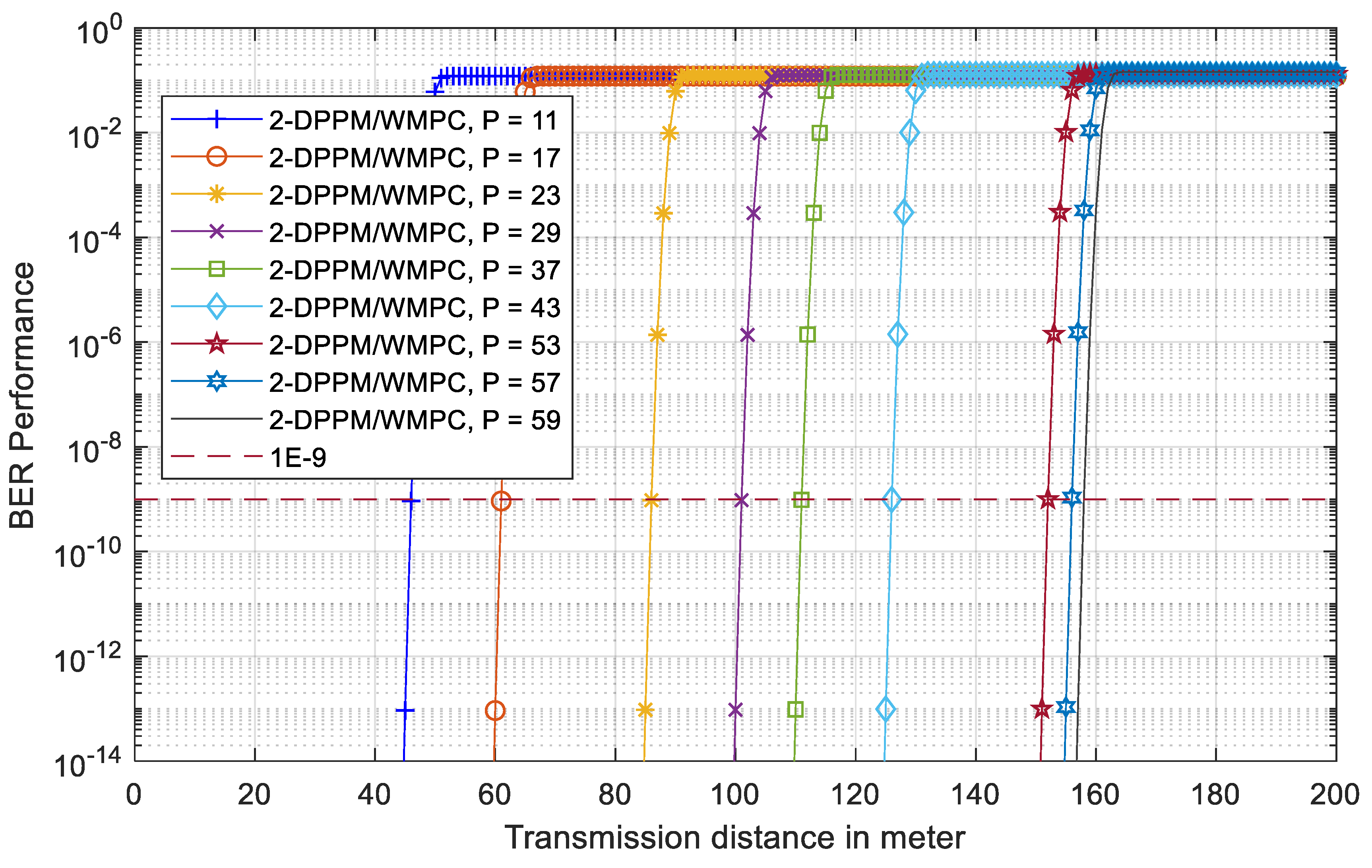
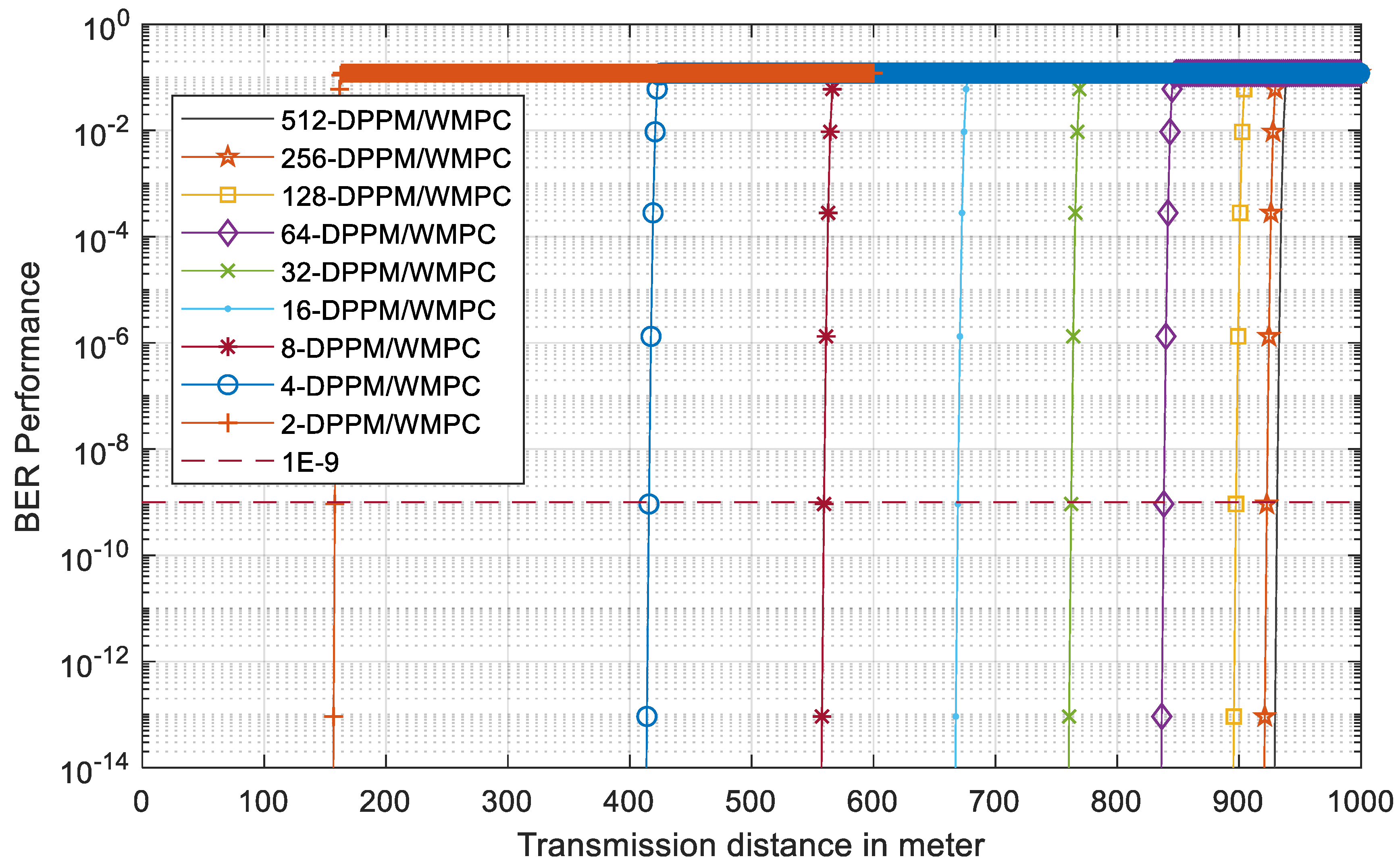
7. Results and Discussion
8. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Álvarez-Roa, C.; Álvarez-Roa, M.; Raddo, T.R.; Jurado-Navas, A.; Castillo-Vázquez, M. Cooperative Terrestrial–Underwater FSO System: Design and Performance Analysis. Photonics 2024, 11, 58. [Google Scholar] [CrossRef]
- Shen, C.; Guo, Y.; Oubei, H.M.; Ng, T.K.; Liu, G.; Park, K.-H.; Ho, K.-T.; Alouini, M.-S.; Ooi, B.S. 20-meter underwater wireless optical communication link with 1.5 Gbps data rate. Opt. Expr. 2016, 24, 25502–25509. [Google Scholar] [CrossRef] [PubMed]
- Lu, C.; Wang, J.; Li, S.; Xu, Z. 60 m/2.5 Gbps underwater optical wireless communication with NRZ-OOK modulation and digital nonlinear equalization. In Proceedings of the Conference on Lasers and Electro-Optics (CLEO), San Jose, CA, USA, 5–10 May 2019; IEEE: Piscataway, NJ, USA, 2019; pp. 1–2. [Google Scholar] [CrossRef]
- Du, J.; Wang, Y.; Fei, C.; Chen, R.; Zhang, G.; Hong, X.; He, S. Experimental demonstration of 50-m/5-Gbps underwater optical wireless communication with low-complexity chaotic encryption. Opt. Expr. 2021, 29, 783–796. [Google Scholar] [CrossRef] [PubMed]
- Hassan, W.H.W.; Sabril, M.S.; Jasman, F.; Idrus, S.M. Experimental study of light wave propagation for underwater optical wireless communication. J. Commun. 2022, 17, 23–29. [Google Scholar] [CrossRef]
- Baykal, Y.; Ata, Y.; Gökçe, M.C. Underwater turbulence, its effects on optical wireless communication and imaging: A review. Opt. Laser Technol. 2022, 156, 108624. [Google Scholar] [CrossRef]
- Kou, L.; Zhang, J.; Zhang, P.; Yang, Y.; He, F. Composite channel modeling for underwater optical wireless communication and analysis of multiple scattering characteristics. Opt. Express 2023, 31, 11320–11334. [Google Scholar] [CrossRef] [PubMed]
- Liu, X.; Yi, S.; Zhou, X.; Fang, Z.; Qiu, Z.-J.; Hu, L.; Cong, C.; Zheng, L.; Liu, R.; Tian, P. 345 m underwater optical wireless communication with 270 Gbps data rate based on a green laser diode with NRZ-OOK modulation. Opt. Express 2017, 25, 27937–27947. [Google Scholar] [CrossRef] [PubMed]
- Liu, W.; Zhang, L.; Huang, N.; Xu, Z. Wide dynamic range signal detection for underwater optical wireless communication using a PMT detector. Opt. Express 2023, 31, 25267–25279. [Google Scholar] [CrossRef]
- Wang, J.; Lu, C.; Li, S.; Xu, Z. 100 m/500 Mbps underwater optical wireless communication using an NRZ-OOK modulated 520 nm laser diode. Opt. Express 2019, 27, 12171–12181. [Google Scholar] [CrossRef]
- Mohammed Salim, O.N.; Adnan, S.A.; Mutlag, A.H. Underwater optical wireless communication system performance improvement using convolutional neural networks. AIP Adv. 2023, 13, 045302. [Google Scholar] [CrossRef]
- Zhang, W.; Wang, L.; Wu, X.; Fei, L.; Peng, H.; Wen, K.; Zhao, Y. Performance Evaluation of Maximum Ratio Combining Diversity Technology and Traditional System Based on Comprehensive Noise Analysis in Underwater Wireless Optical Communication. Photonics 2023, 10, 1388. [Google Scholar] [CrossRef]
- Hu, S.; Mi, L.; Zhou, T.; Chen, W. 3588 attenuation lengths and 332 bits/photon underwater optical wireless communication based on photon-counting receiver with 256-PPM. Opt. Express 2018, 26, 21685–21699. [Google Scholar] [CrossRef] [PubMed]
- Shen, T.; Guo, J.; Liang, H.; Li, Y.; Li, K.; Dai, Y.; Ai, Y. Research on a Blue–Green LED Communication System Based on an Underwater Mobile Robot. Photonics 2023, 10, 1238. [Google Scholar] [CrossRef]
- Chen, Y.; Kong, M.; Ali, T.; Wang, J.; Sarwar, R.; Han, J.; Guo, C.; Sun, B.; Deng, N.; Xu, J. 26 m/55 Gbps air-water optical wireless communication based on an OFDM-modulated 520-nm laser diode. Opt. Express 2017, 25, 14760–14765. [Google Scholar] [CrossRef]
- Chen, D.; Wang, J.; Li, S.; Xu, Z. Effects of air bubbles on underwater optical wireless communication [Invited]. Chin. Opt. Lett. 2019, 17, 100008. [Google Scholar] [CrossRef]
- Rong, Y.; Nordholm, S.; Duncan, A. On the capacity of underwater optical wireless communication systems. In Proceedings of the 2021 Fifth Underwater Communications and Networking Conference (UComms), Lerici, Italy, 31 August–2 September 2021; IEEE: Piscataway, NJ, USA, 2021; pp. 1–4. [Google Scholar]
- Geldard, C.T.; Guler, E.; Hamilton, A.; Popoola, W.O. An empirical comparison of modulation schemes in turbulent underwater optical wireless communications. J. Light. Technol. 2022, 40, 2000–2007. [Google Scholar] [CrossRef]
- Huang, X.; Yang, F.; Song, J. Hybrid LD and LED-based underwater optical communication: State-of-the-art, opportunities, challenges, and trends. Chin. Opt. Lett. 2019, 17, 100002. [Google Scholar] [CrossRef]
- Gabriel, C.; Khalighi, M.-A.; Bourennane, S.; Léon, P.; Rigaud, V. Monte-Carlo-based channel characterization for underwater optical communication systems. J. Opt. Commun. Netw. 2013, 5, 1–12. [Google Scholar] [CrossRef]
- Saeed, N.; Celik, A.; Al-Naffouri, T.Y.; Alouini, M.S. Underwater optical wireless communications, networking, and localization: A survey. Ad Hoc Netw. 2019, 94, 101935. [Google Scholar] [CrossRef]
- Han, S.; Noh, Y.; Liang, R.; Chen, R.; Cheng, Y.-J.; Gerla, M. Evaluation of underwater optical-acoustic hybrid network. China Commun. 2014, 11, 49–59. [Google Scholar] [CrossRef]
- Kaushal, H.; Kaddoum, G. Underwater optical wireless communication. IEEE Access 2016, 4, 1518–1547. [Google Scholar] [CrossRef]
- Farr, N.; Bowen, A.; Ware, J.; Pontbriand, C.; Tivey, M. An integrated, underwater optical/acoustic communications system. In Proceedings of the OCEANS’10 IEEE SYDNEY, Sydney, NSW, Australia, 24–27 May 2010; IEEE: Piscataway, NJ, USA, 2010; pp. 1–6. [Google Scholar]
- Willner, A.E.; Zhao, Z.; Ren, Y.; Li, L.; Xie, G.; Song, H.; Liu, C.; Zhang, R.; Bao, C.; Pang, K. Underwater optical communications using orbital angular momentum-based spatial division multiplexing. Opt. Commun. 2018, 408, 21–25. [Google Scholar] [CrossRef]
- Al-Zhrani, S.; Bedaiwi, N.M.; El-Ramli, I.F.; Barasheed, A.Z.; Abduldaiem, A.; Al-Hadeethi, Y.; Umar, A. Underwater optical communications: A brief overview and recent developments. Eng. Sci. 2021, 16, 146–186. [Google Scholar] [CrossRef]
- Lu, H.; Jiang, M.; Cheng, J. Deep learning aided robust joint channel classification, channel estimation, and signal detection for underwater optical communication. IEEE Trans. Commun. 2020, 69, 2290–2303. [Google Scholar] [CrossRef]
- Zhu, S.; Chen, X.; Liu, X.; Zhang, G.; Tian, P. Recent progress in and perspectives of underwater wireless optical communication. Prog. Quantum Electron. 2020, 73, 100274. [Google Scholar] [CrossRef]
- Zhao, Y.; Wang, A.; Zhu, L.; Lv, W.; Xu, J.; Li, S.; Wang, J. Performance evaluation of underwater optical communications using spatial modes subjected to bubbles and obstructions. Opt. Lett. 2017, 42, 4699–4702. [Google Scholar] [CrossRef] [PubMed]
- Zhou, H.; Zhang, M.; Wang, X.; Ren, X. Design and implementation of more than 50m real-time underwater wireless optical communication system. J. Light. Technol. 2022, 40, 3654–3668. [Google Scholar] [CrossRef]
- Nguyen, C.T.; Nguyen, M.T.; Mai, V.V. Underwater optical wireless communication-based IoUT networks: MAC performance analysis and improvement. Opt. Switch. Netw. 2020, 37, 100570. [Google Scholar] [CrossRef]
- Ismail, M.A.M.; Saleh, K. Performance analysis toward 880 m/4.255 Gbps underwater optical wireless communication CDMA network based on hybrid M-ary differential pulse position modulation and double length modified prime code. Opt. Quantum Electron. 2024, 56, 668. [Google Scholar] [CrossRef]
- Xu, J. Underwater wireless optical communication: Why, what, and how? Chin. Opt. Lett. 2019, 17, 100007. [Google Scholar] [CrossRef]
- Spagnolo, G.S.; Cozzella, L.; Leccese, F. Underwater optical wireless communications: Overview. Sensors 2020, 20, 2261. [Google Scholar] [CrossRef] [PubMed]
- Morsy, M.A.; Alsayyar, A.S. Performance analysis of OCDMA wireless communication system based on double length modified prime code for security improvement. IET Commun. 2020, 14, 1139–1146. [Google Scholar] [CrossRef]
- Morsy, M.A.; Alsayyari, A.S. Performance analysis of coherent BPSK-OCDMA wireless communication system. Wirel. Netw. 2020, 26, 4491–4505. [Google Scholar] [CrossRef]
- Morsy, M.A. Analysis and design of weighted MPC in incoherent synchronous OCDMA network. Opt. Quantum Electron. 2018, 50, 387. [Google Scholar] [CrossRef]
- Morsy, M.A.; Alsayyari, A.S. Multi-rate OCDMA system BER performance evaluations for different ML-code sequences. Opt. Quantum Electron. 2019, 51, 198. [Google Scholar] [CrossRef]
- Morsy, M.A.; Alsayyari, A.S. Performance analysis of incoherent PPM-OCDMA networks based on optimised modified prime code for multimedia applications. IET Commun. 2020, 14, 4014–4021. [Google Scholar] [CrossRef]
- Morsy, M.A. Coherent OCDMA network based on a new BER performance equalization technique for multimedia applications. Opt. Quantum Electron. 2023, 55, 167. [Google Scholar] [CrossRef]
- Ismail, M.A.M.; Alsayyari, A.; Galal, O.H. Performance analysis of optical code division multiple access networks for multimedia applications using multilength weighted modified prime codes. Opt. Eng. 2019, 58, 035101. [Google Scholar] [CrossRef]
- Chen, L.-K.; Shao, Y.; Di, Y. Underwater and water-air optical wireless communication. J. Light. Technol. 2021, 40, 1440–1452. [Google Scholar] [CrossRef]
- Adnan, S.A.; Hassan, H.A.; Alchalaby, A.; Kadhim, A.C. Experimental study of underwater wireless optical communication from clean water to turbid harbor under various conditions. Int. J. Des. Nat. Ecodynamics 2021, 16, 219–226. [Google Scholar] [CrossRef]
- Kumari, M. Performance analysis of high speed hybrid PON-VLC for long-reach land-to-underwater applications. Wirel. Netw. 2023, 29, 1721–1735. [Google Scholar] [CrossRef]
- Sabhapathy, U.; Wilson, L.A. Implementation of underground water for wireless optical communication system using CDMA. Int. Innov. Res. J. Eng. Technol. 2021, 6, 10–20. [Google Scholar] [CrossRef]
- Joseph, D.; Karthikeyan, A.; Kuppusamy, P.G.; Prabhu, V. Investigations on hybrid wavelength-mode-orthogonal frequency-division multiplexing scheme based free space optical transmission system under varying atmospheric conditions. Opt. Quantum Electron. 2022, 54, 64. [Google Scholar] [CrossRef]
- Sabbagh, A.G.; Miandehi, F.Z. Performance analysis of unequal-mark-power optical CDMA systems. IEEE Trans. Commun. 2020, 68, 3696–3705. [Google Scholar] [CrossRef]
- Zedini, E.; Kammoun, A.; Soury, H.; Hamdi, M.; Alouini, M.-S. Performance analysis of dual-hop underwater wireless optical communication systems over mixture exponential-generalized gamma turbulence channels. IEEE Trans. Commun. 2020, 68, 5718–5731. [Google Scholar] [CrossRef]
- Al Hammadi, M.M.; Islam, M.J. Performance evaluation of underwater wireless optical CDMA system for different water types. Photonic Netw. Commun. 2020, 39, 246–254. [Google Scholar] [CrossRef]
- Kandouci, C. Investigation of Jervol water types properties effects on underwater optical wireless OCDMA system performances for different modulation techniques. Opt. Quantum Electron. 2022, 54, 3. [Google Scholar] [CrossRef]
- Kandouci, C. Performances enhancement of underwater wireless optical communications (UWOC) using pulse position modulation. J. Opt. Commun. 2022, 43, 289–294. [Google Scholar] [CrossRef]
- Shiu, D.S.; Kahn, J.M. Differential pulse-position modulation for power-efficient optical communication. IEEE Trans. Commun. 1999, 47, 1201–1210. [Google Scholar] [CrossRef]
- Morsy, M.A.; Aly, M.H. A new hybrid prime code for OCDMA network multimedia applications. Electronics 2021, 10, 2705. [Google Scholar] [CrossRef]
- Morsy, M.A.; Hadeel, S.A.R.; Al-Obaidan, H.M. Performance of Passive OCDMA Networks for Different Encoder/Decoder Delay Lines. Int. J. Opt. Appl. 2013, 3, 19–26. [Google Scholar]
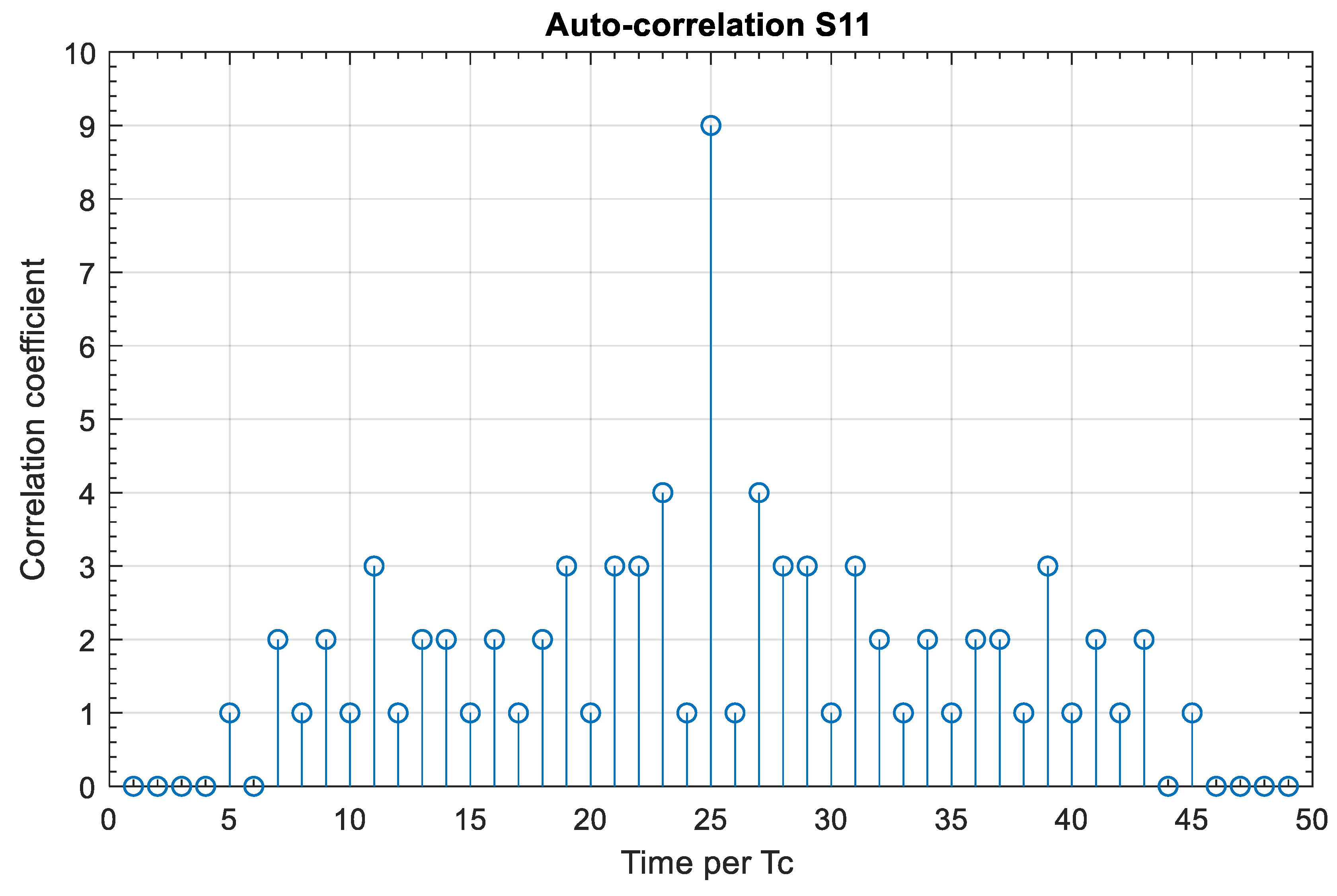
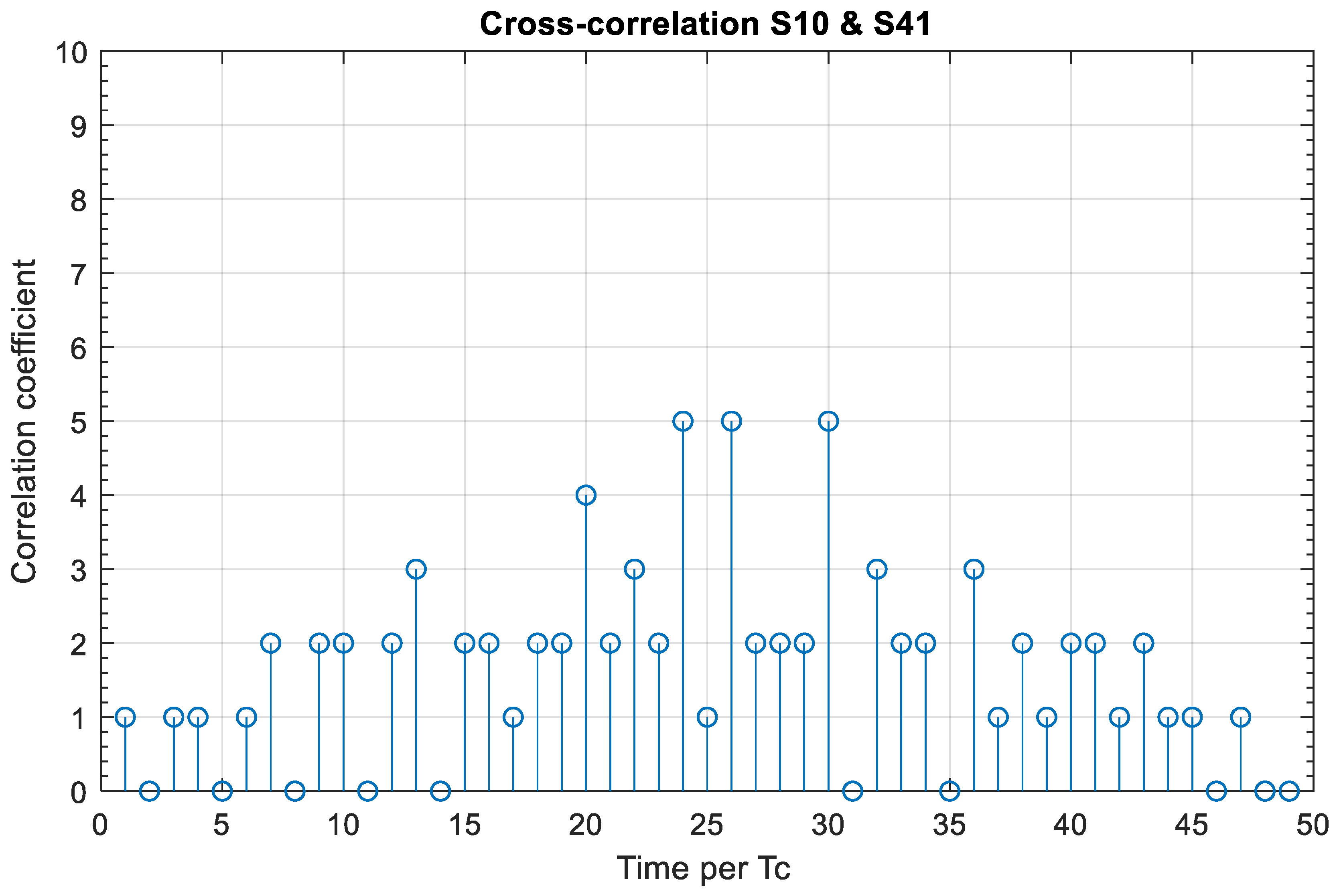




| Ref. | Parameters | Optical | RF Waves | Acoustic Waves |
|---|---|---|---|---|
| [14] | Attenuation | 0.39 dB/m (ocean)–11 dB/m (turbid) | - | - |
| Speed (m/s) | 2.26 × 108 | |||
| Data rate | ~Gbps | |||
| Latency | Low | |||
| Distance | 10–100 m | |||
| [13] | Attenuation | - | Frequency band and conductivity dependent (3.5–5) dB/m | - |
| Speed (m/s) | 2.26 × 108 | |||
| Data rate | ~Mbps | |||
| Latency | Moderate | |||
| Distance | Up to 10 m | |||
| [12] | Attenuation | Distance- and frequency-dependent (0.1–4) dB/km | ||
| Speed (m/s) | 1500 | |||
| Data rate | ~Kbps | |||
| Latency | High | |||
| Distance | Up to Kms | |||
| [8] | Bandwidth | 10–150 MHz | About MHz | Distance dependent 1000 km and 1 kHz 1–10 km and 10 kHz Less than 100 m and 100 kHz |
| Frequency band | 1012–1015 Hz | 30–300 Hz for direct underwater communication system or MHz for buoyant communication system | 10–15 kHz | |
| Transmission power | Few Watts | Few mW to hundreds of Watts | Tens of Watts | |
| Antenna size | 0.1 m | 0.5 m | 0.1 m | |
| Efficiency | 30,000 bits/J | - | 100 bits/J | |
| Performance parameters | Absorption and organic matter | Conductivity and permittivity | Temperature and pressure | |
| [29,30,31] | Code length | 121–289 Chip | 121–289 Chip | 121–289 Chip |
| BER performance | Less than 10−20 | Less than 10−15 | Less than 10−10 | |
| Number of users | 110–180 | 90–100 | 30–50 | |
| Throughput performance | 1 Tbps*user | 10 Gbps*user | Less than Mbps*user | |
| [43,44,45] | Number of orthogonal frequencies | Less than 32 | Less than 16 | - |
| Number of users | 20–32 | 1–16 | ||
| BER performance | Less than 10−12 | Less than 10−9 | ||
| Throughput performance | Approximately 100 Gbps*user | Approximately 1 Gbps*user |
| Group x | i 0 1 2 3 4 | Sequence | MPC Part | WMPC Part |
|---|---|---|---|---|
| 1 | 0 1 2 3 4 | S1,0 | 10000–01000–00100–00010–00001 | 10010–01001–00100–00101–01010 A 01001–10010–00100–01010–00101 B |
| 4 0 1 2 3 | S1,1 | 00001–10000–01000–00100–00010 | 00101–10010–01000–01010–10100 A 10010–00101–01000–10100–01010 B | |
| 3 4 0 1 2 | S1,2 | 00010–00001–10000–01000–00100 | 01010–00101–10000–10100–01001 A 00101–01010–10000–01001–10100 B | |
| 2 3 4 0 1 | S1,3 | 00100–00010–00001–10000–01000 | 10100–01010–00001–01001–10010 A 01010–10100–00001–10010–01001 B | |
| 1 2 3 4 0 | S1,4 | 01000–00100–00010–00001–10000 | 01001–10100–00010–10010–00101 A 10100–01001–00010–00101–10010 B | |
| . . | . . | . . | . . | . . |
| 4 | 0 4 3 2 1 | S4,0 | 10000–00001–00010–00100–01000 | 10100–01001–00010–01010–00101 A 01001–10100–00010–00101–01010 B |
| 1 0 4 3 2 | S4,1 | 01000–10000–00001–00010–00100 | 01010–10100–00001–00101–10010 A 10100–01010–00001–10010–00101 B | |
| 2 1 0 4 3 | S4,2 | 00100–01000–10000–00001–00010 | 00101–01010–10000–10010–01001 A 01010–00101–10000–01001–10010 B | |
| 3 2 1 0 4 | S4,3 | 00010–00100–01000–10000–00001 | 10010–00101–01000–01001–10100 A 00101–10010–01000–10100–01001 B | |
| 4 3 2 1 0 | S4,4 | 00001–00010–00100–01000–10000 | 01001–10010–00100–10100–01010 A 10010–01001–00100–01010–10100 B |
| Parameter | Definition | Value |
|---|---|---|
| Received photocurrent depends on the PIN photodetector responsivity and optical power received | A | |
| PIN photo-detector responsivity | 0.8 A/W | |
| Receiver noise figure | 1.2 | |
| Gain of preamplifier receiver | 5 | |
| PIN dark current | 10 nA | |
| Receiver electrical bandwidth | 3.2 GHz | |
| Boltzmann constant | 1.38 × 10−23 J/K | |
| Receiver temperature | 298 K | |
| Receiver load resistance | 70 KΩ | |
| Instantaneous SNR | Ratio |
| No. | Name | Symbol | Value |
|---|---|---|---|
| 1 | Laser peak transmitted power | 20 dBmW | |
| 4 | Receiver aperture area | 0.01 m2 and 0.05 m2 | |
| 5 | Transmitter efficiency | 0.9 | |
| 6 | Receiver efficiency | 0.9 | |
| 8 | Divergence angle of laser beam | 60° | |
| 9 | Angle between perpendicular of transmitter–receiver planes | 5° | |
| 10 | Operating wavelength | λ | 400–600 nm |
| 11 | Prime number of WMPC | P | 11, 23, 31, 47, 53, 61 |
| 12 | Chip time duration | 2.6 ps |
| Parameter | Description |
|---|---|
| Maximal number of users out of where is the WMPC prime number | |
| Random variable representing active user # , where and | |
| Random variable representing the number of active users in the first group, | |
| Realization variable of | |
| Probability distribution function of the active users in all groups, | |
| Random vector defining the amount of interference, , is a random variable representing the number of chips that interfere with time slot i. | |
| w | Vector realizing , w = (w0, w1, …, wM−1)U |
| Probability of the random vector l, |
| Ref. | Modulation Scheme | Transmission Distance (m) | Throughput (Gbps*User) | Single/Multi-User |
|---|---|---|---|---|
| [1] | 4-PAM | 130 | 1 | Single user |
| [2] | IM | 20 | 1.5 | Single user |
| [3] | NRZ-OOK | 60 | 2.5 | Single user |
| [4] | DFT-S DMT | 50 | 5 | Single user |
| [10] | NRZ-OOK | 100 | 5 | Single user |
| [12] | IM | 10 | 10 | Single user |
| [13] | NRZ-OOK | 35 | 27 | Single user |
| [14] | 256-PPM | 36 | 3.32 | Single user |
| [15] | OFDM | 26 | 5.5 | Multi-user |
| This work | 256-DPPM/WMPC | 930 | 180 | Multi-user |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ismail, M.A.M.; Saleh, K. A 930 m/180 Gbps*User Underwater Coherent Optical Code-Division Multiple-Access Network Based on Hybrid 256-Differential Pulse Position Modulation and Weighted Modified Prime Code Sequence. Photonics 2024, 11, 368. https://doi.org/10.3390/photonics11040368
Ismail MAM, Saleh K. A 930 m/180 Gbps*User Underwater Coherent Optical Code-Division Multiple-Access Network Based on Hybrid 256-Differential Pulse Position Modulation and Weighted Modified Prime Code Sequence. Photonics. 2024; 11(4):368. https://doi.org/10.3390/photonics11040368
Chicago/Turabian StyleIsmail, Morsy Ahmed Morsy, and Khalid Saleh. 2024. "A 930 m/180 Gbps*User Underwater Coherent Optical Code-Division Multiple-Access Network Based on Hybrid 256-Differential Pulse Position Modulation and Weighted Modified Prime Code Sequence" Photonics 11, no. 4: 368. https://doi.org/10.3390/photonics11040368
APA StyleIsmail, M. A. M., & Saleh, K. (2024). A 930 m/180 Gbps*User Underwater Coherent Optical Code-Division Multiple-Access Network Based on Hybrid 256-Differential Pulse Position Modulation and Weighted Modified Prime Code Sequence. Photonics, 11(4), 368. https://doi.org/10.3390/photonics11040368





