Development of Cryogenic Systems for Astronomical Research
Abstract
1. Introduction
2. Classification of Cooling Systems for Astronomy
2.1. Frequency Range of Astronomical Instruments and Their Cooling Problems
2.2. Temperature Levels of Cooling for Astronomical Receivers
2.3. Main Types of Cryosystems for Astronomy
2.3.1. Dewars or Cryo-Accumulators and Liquid Nitrogen Cooling Solutions
- gaseous agent (a cold flow of N2, H2 and He gases);
- solid agent (carbon dioxide); and
- liquefied gas (nitrogen < 77 K, hydrogen < 20 K, helium < 4 K).
2.3.2. Hydrogen Level Refrigerators for Astronomy and Telecommunications
2.3.3. Cooling Systems of the Helium Level
2.3.4. Cryosorption Cryostats
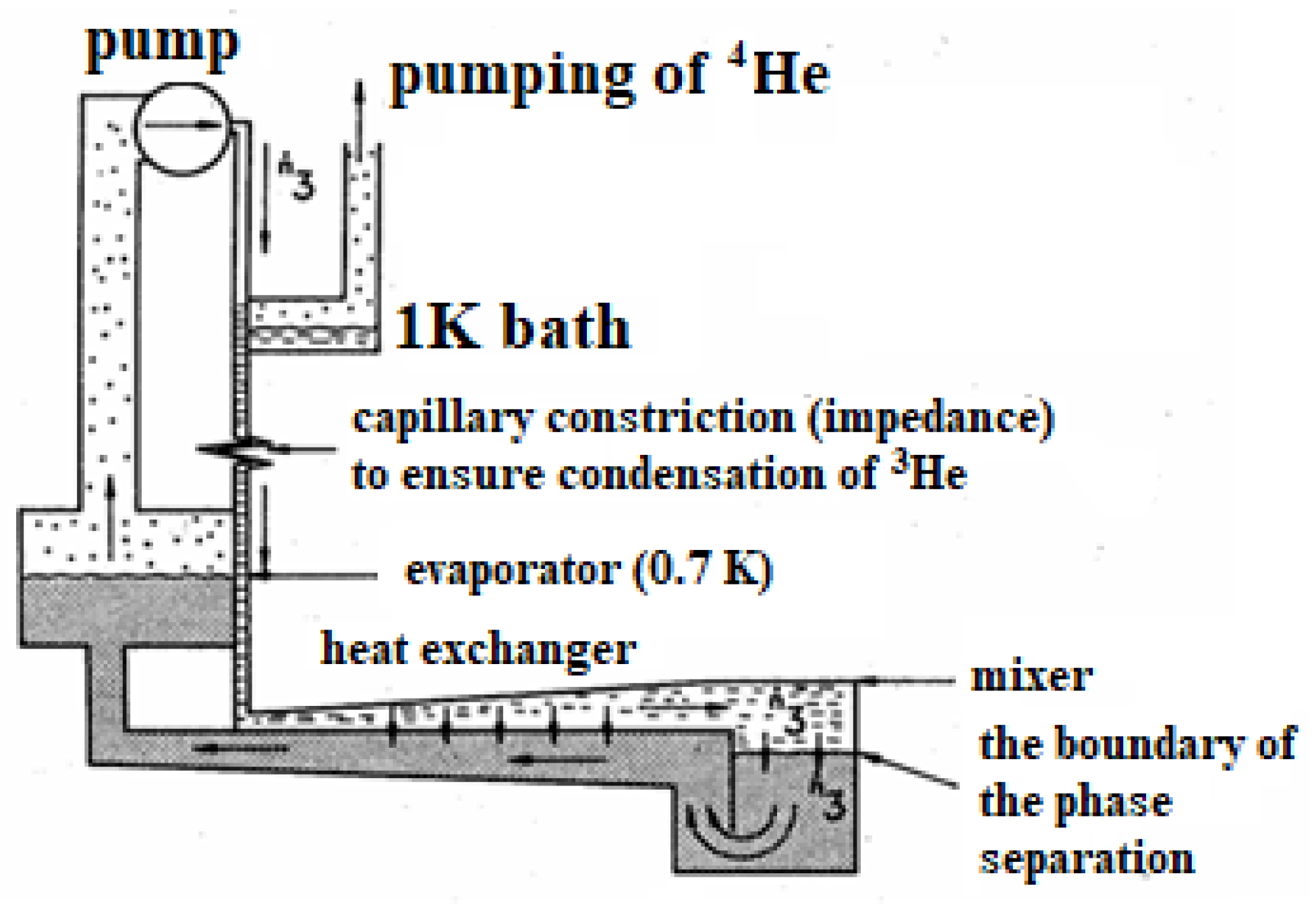
2.3.5. Dilution Cryostats
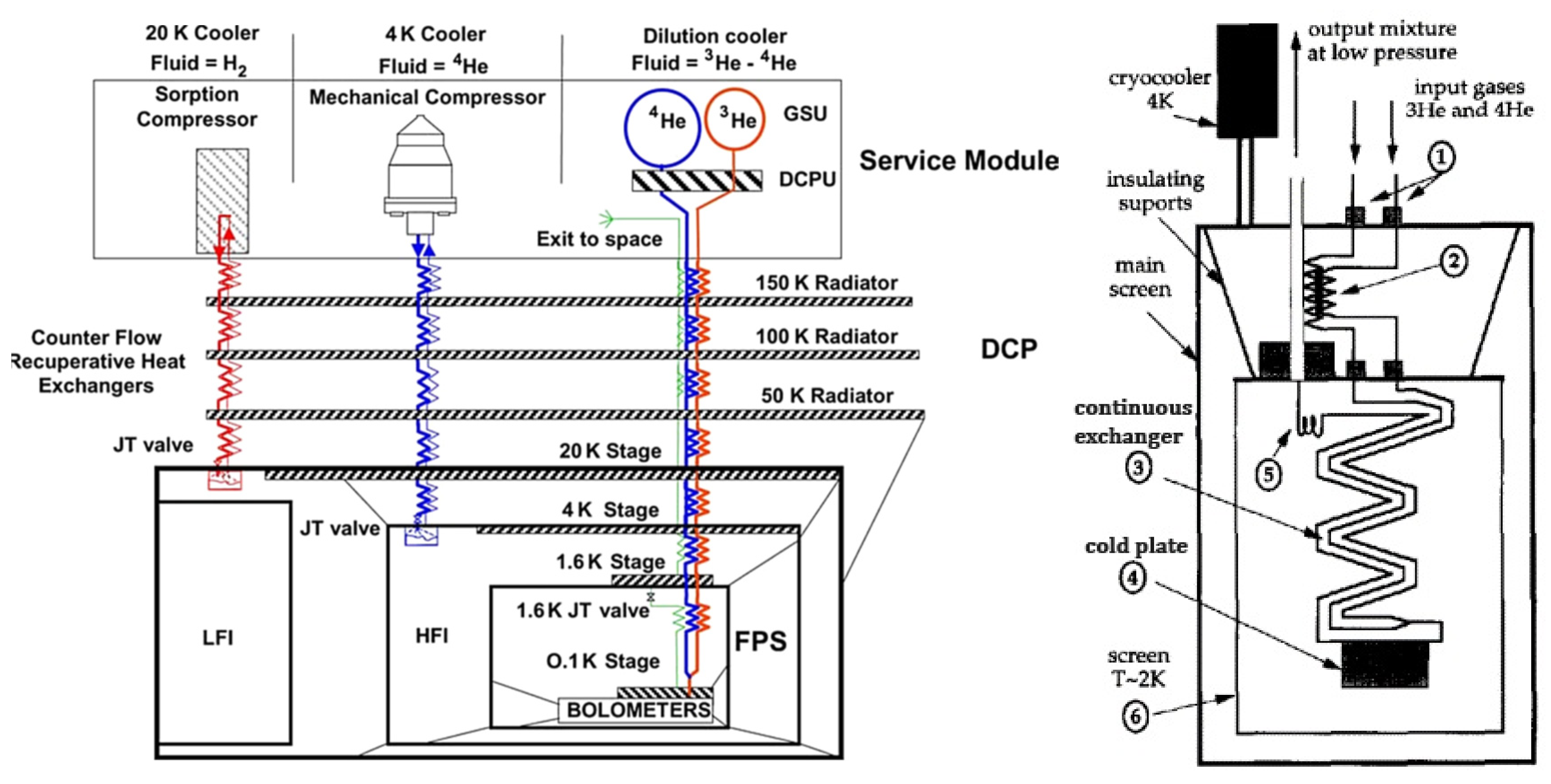
2.3.6. SubK Systems for Balloon Missions
3. Specific Features of Cryogenic System Technology for Astronomical Instruments
3.1. Cryogenic Interface and the Basic Elements of the Cryo Design Calculation
3.2. Vacuum Interface
3.3. Mechanical Interface
3.4. Optical Interface
3.5. DC, RF and Digital Interfaces
4. Development of Cryogenic Systems for Astronomy: Technical Solutions of Combined Optical and Radiophysical Problems
4.1. 4 K Cryostating Systems
- Operating temperature: 4 K ± 0.1 K; with a load: 0.8–1.5 W
- Vacuum level: 10−4 mbar; flange KF D25 for pumping
- 2 flanges for installation of optical windows with a diameter of 25 mm;
- Input of electrical and RF signals
- Size of the working cavity: diameter 185 mm, height 70 mm, overall dimensions not more than 1600 mm; the diameter is not less than 700 mm; there are several options
- A built-in interface for reducing the influence of temperature fluctuations with the possibility of observations when MCS is disabled
- Availability of optical windows
- The system should operate being installed on an antivibration rack
- -
- Resolution in full-step mode: 0.64 μm;
- -
- Resolution in microstep mode (practically achievable): 0.3 mm;
- -
- Range of movement: ±3 mm;
- -
- Repeatability error: from 0.2 to 1.55 μm (0.8 μm, on average) in a range of 150 μm;
- -
- Minimum consumption power (continuous operation): 600 μW (T = 8...10 K);
- -
- Minimum consumption power when moving: 1 μm through 4 μW·s (mJ) (T = 8...10 K).
- 2.
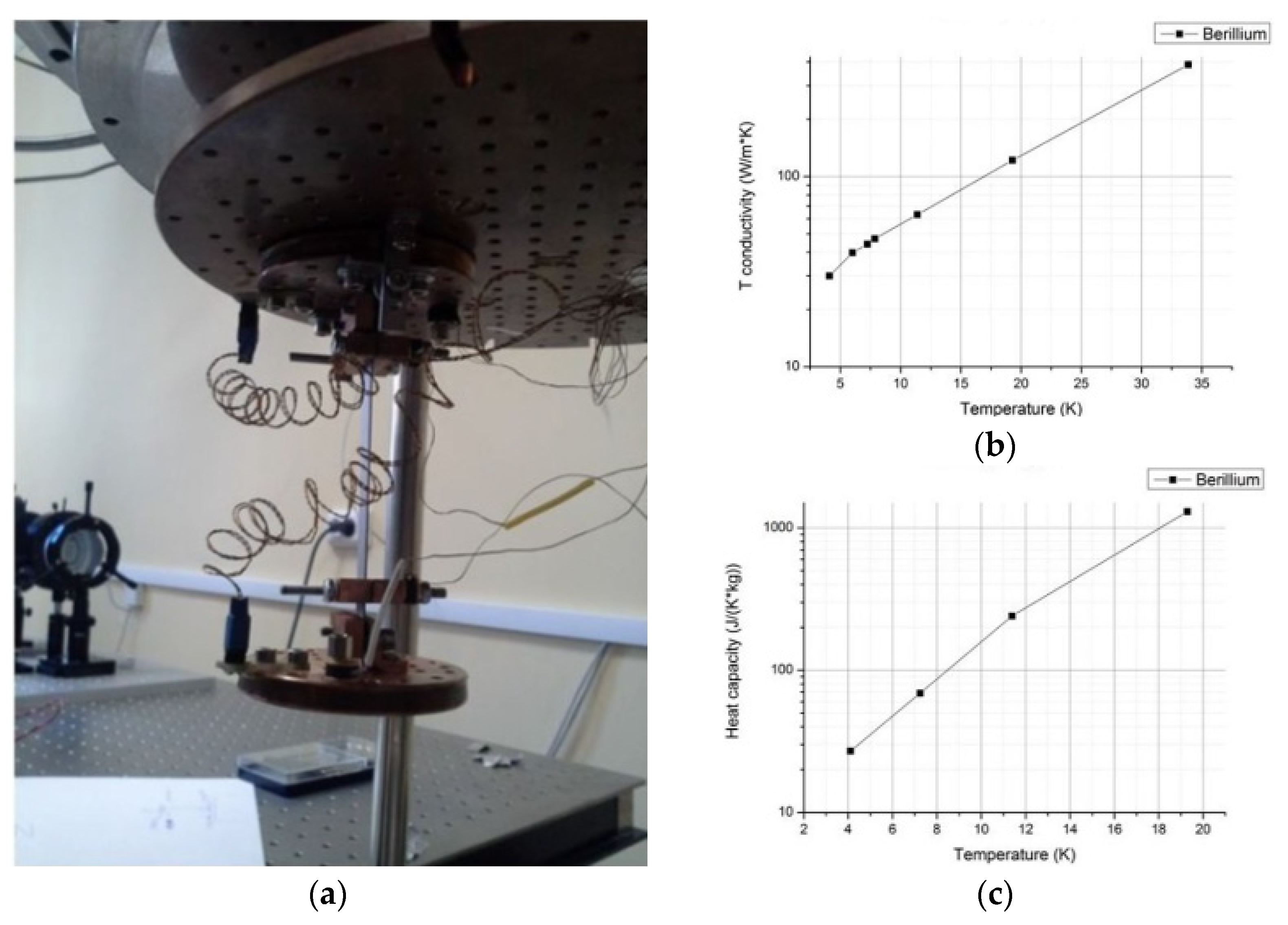
- Measurements of the thermal conductivity and heat capacity of beryllium samples intended for the manufacture of the switching mirror of the space cryogenic telescope “Millimetron” were carried out.
4.1.1. Solving the Problem of Vibrations and Temperature Fluctuations in Cryogenic Refrigerators
- Accelerometers B&K 4371-2
- Charge amplifiers B&K 2651-2
- Power supply B&K 2805
- 2-channel ADC M-AUDIO Transit
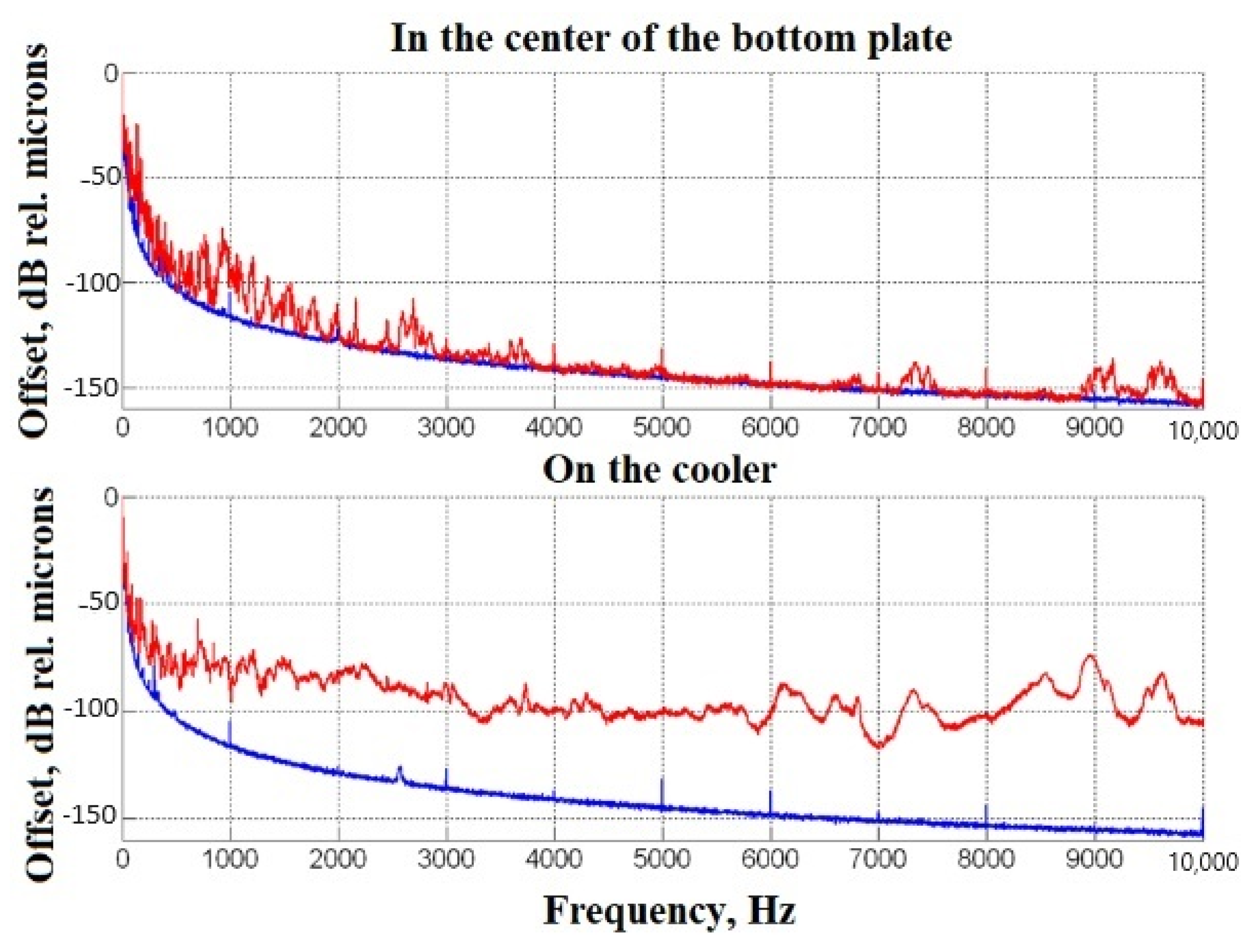
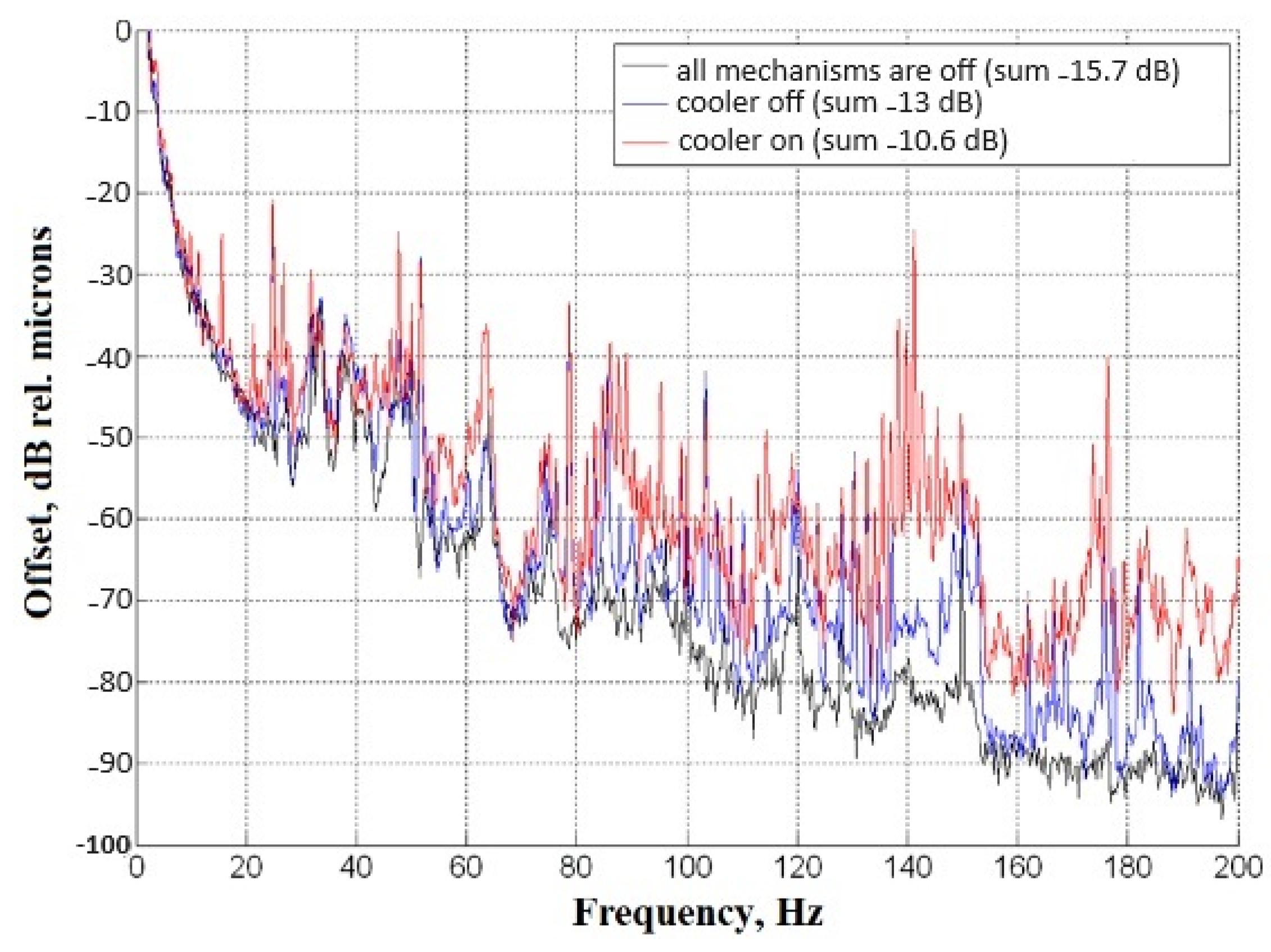
- All mechanisms were disabled (recording duration ~60 s).
- All main mechanisms (the pumps and compressors) were turned on; the cooler was turned off (recording duration ~60 s).
- All main mechanisms (the pumps and compressors) were “on”; the cooler was “on” (recording duration ~30 s).
- The vibrations in the working area were determined by the periodic action of the cooler, and the width of the discrete components of the vibrations was obviously <0.1 Hz.
- The vibration displacement levels of the narrow-band components at the control points on the lower plate in the working area of the installation did not exceed 0.22 μm (<0.1 μm in the center of the plate). The integral level of vibration displacement in the 8–200 Hz band ranged from 0.3 μm to 0.6 μm at different points of the plate.
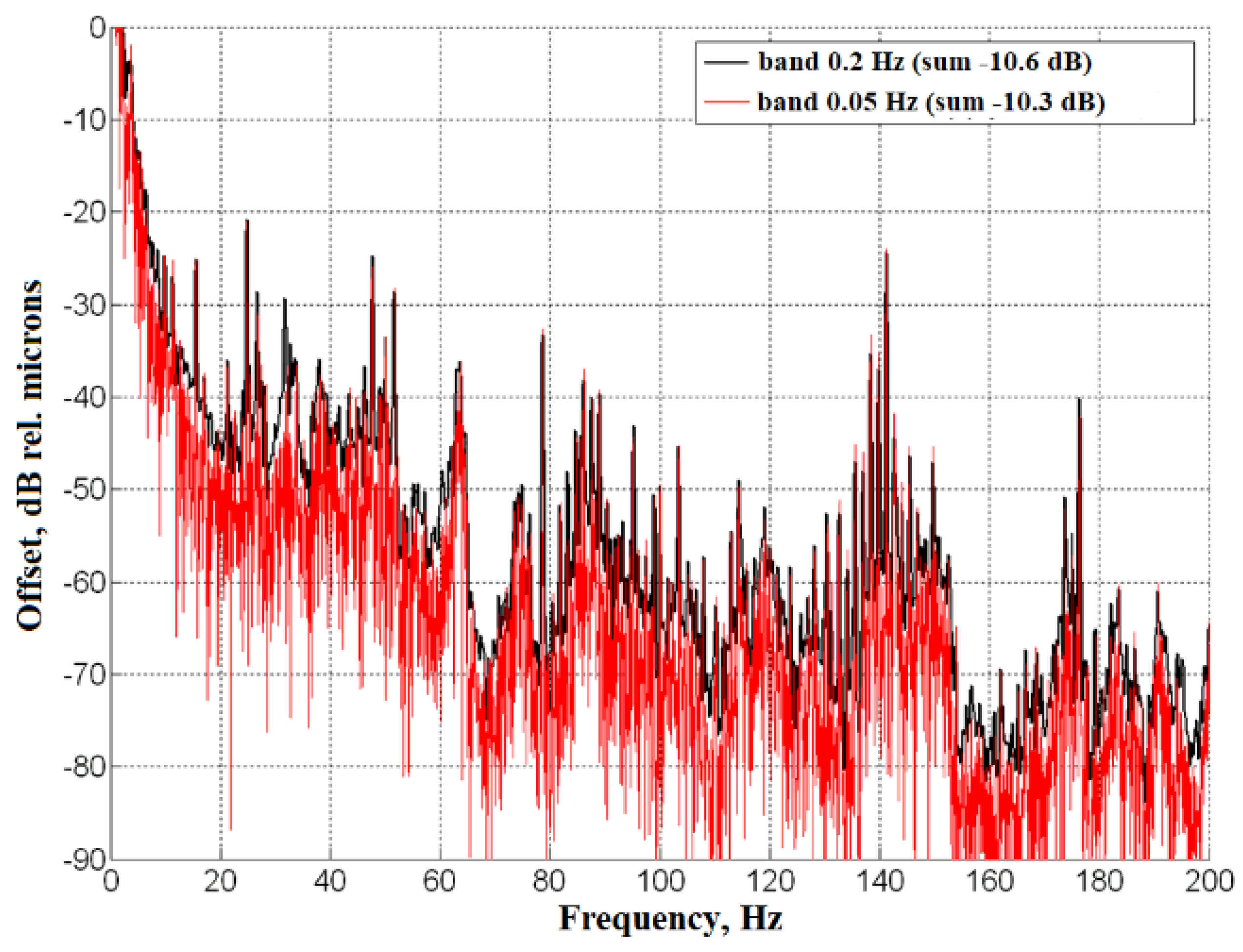
- To provide measurements in the frequency range of 0.5 Hz, it is recommended to use a laser vibrometer. It is also recommended to evaluate its measuring capability at the operating temperatures. The measurable levels achievable by using standard laser vibrometers will be no worse than 100 nm up to zero frequencies.
- To develop recommendations for reducing the vibration level of the structure in the working area of the installation, a more detailed measurement of the acoustic vibration characteristics of the stand (transmission coefficients from the source to the working area) should be carried out, and measures should be taken to ensure vibration isolation of the installation as a whole. For the following measurements, a measuring path with a lower noise level should be used.
- Of course, for the final verification of the data obtained, it is useful to conduct a test during cooling; for this, it is necessary to provide thermal and vacuum isolation of the measuring equipment, since the individual parameters, in particular, heat capacity, thermal conductivity, sound propagation velocity in the medium can vary depending on the temperature.
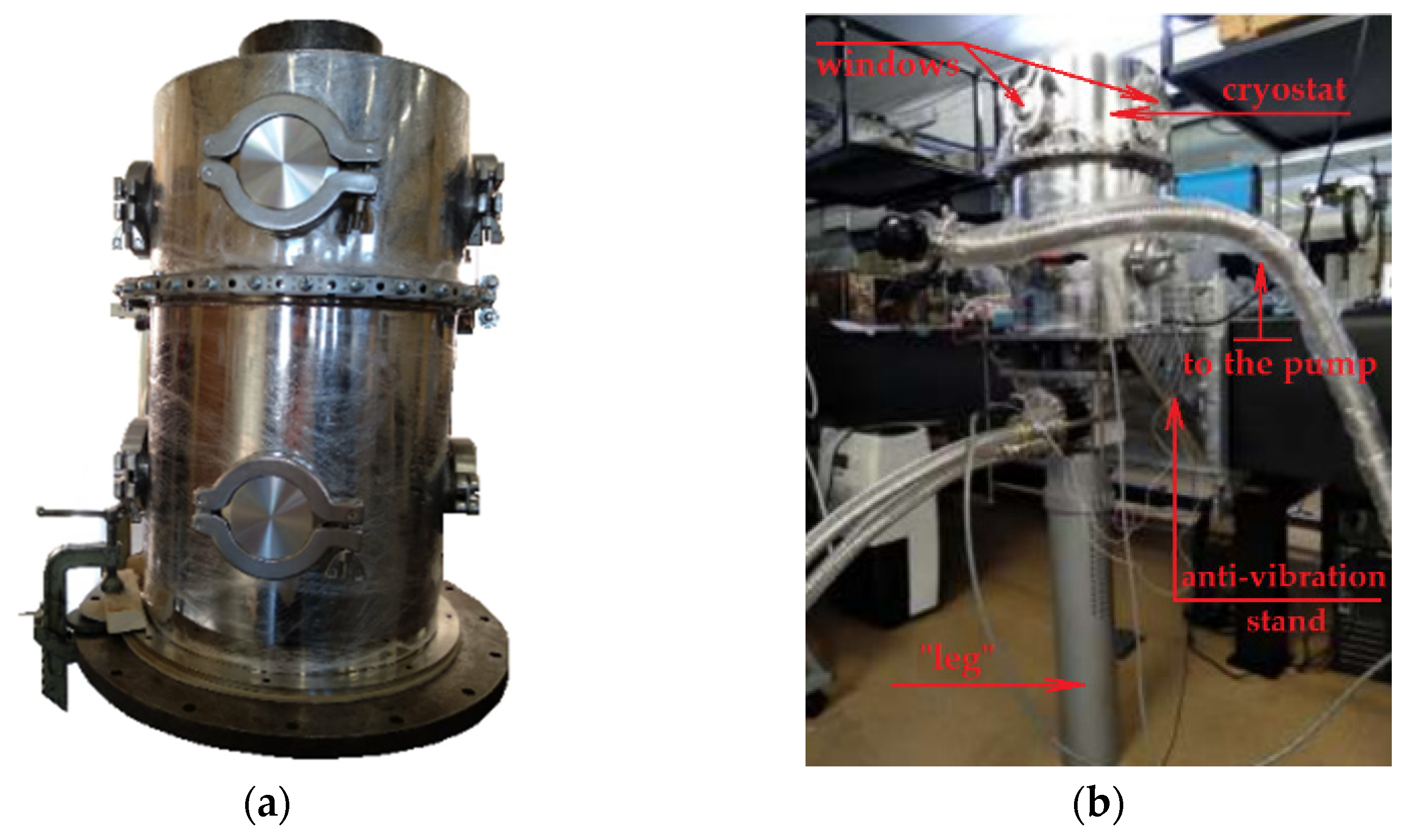
4.1.2. Antivibration 4 K Cryostating System
- -
- Operating temperature: 4 K ± 0.1 K; with heat load: 1 W;
- -
- Vacuum level: 10−4 mbar;
- -
- Vibration displacement of the 4 K plate in the horizontal plane: no more than 10 μm;
- -
- close-cycle MCS RDK-408D2 (SHI), mounting of MCS: cold head up
- -
- 4 flanges KF D50 for mounting of optical windows with a diameter of 50 mm (optional), 3 flanges KF D50 or the mounting of electrical and RF connectors;
- -
- Working cavity size: diameter 200 mm, height 90 mm;
- -
- Overall dimensions of the product: diameter 400 mm, height 800 mm.
- -
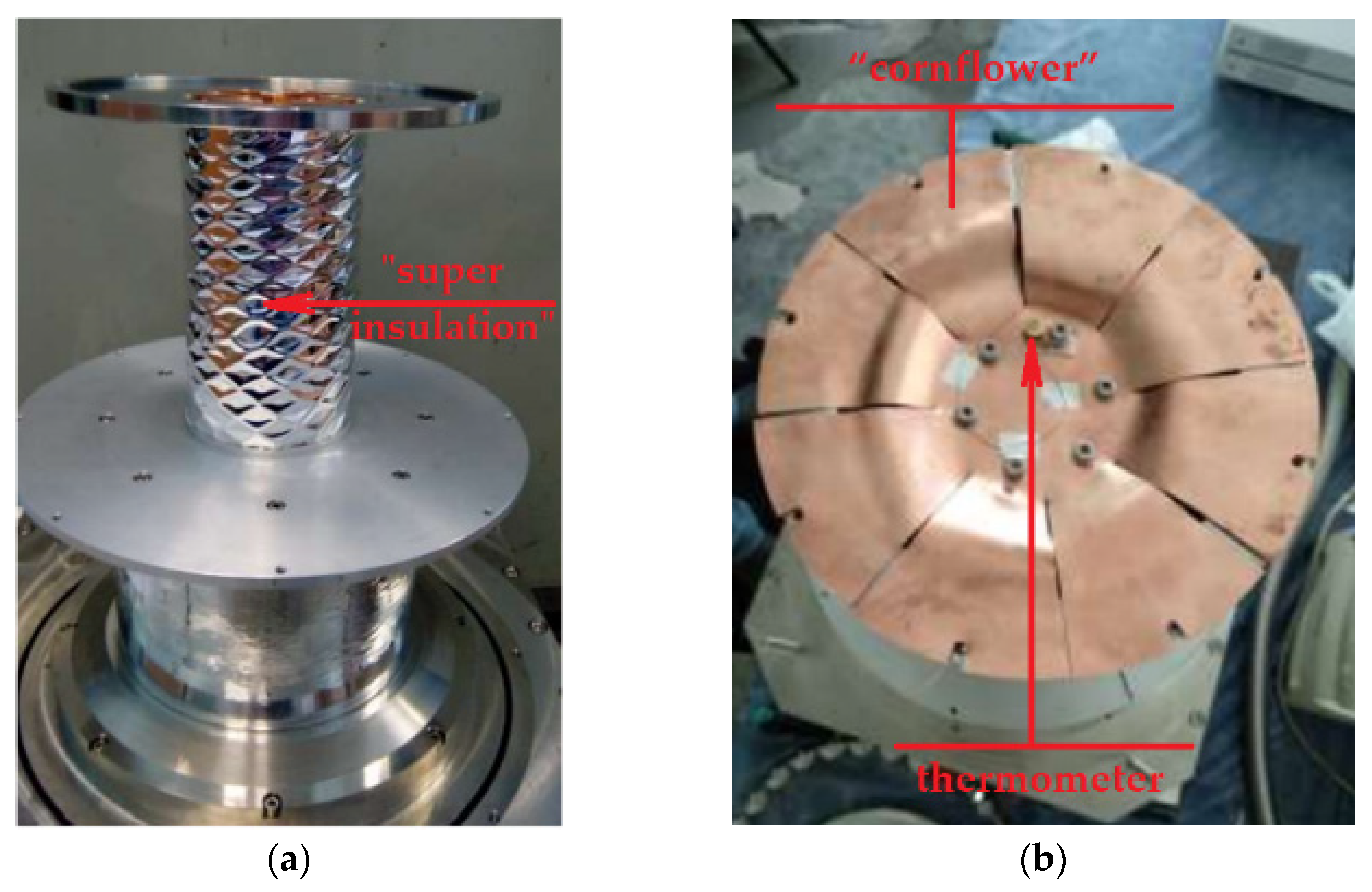
- To reduce the 4 K radiation losses, the support was covered with superinsulation (Figure 22a)—an additional cooling line made of a spring ring of soft annealed copper was installed under the 4 K panel and on the cold finger of the cryomachine to improve heat transfer between them (“cornflower”) (Figure 22b). A great deal of this work regarding this element of the process has been carried out on microphonics and their treatment.
4.1.3. The Low-Vibration 4 K Cryostating System for Studying Thermal Deformations of the Panels of the Main Mirror of the Millimetron Space Mission at Cryogenic Temperatures
- Operating temperature: 4 K ± 0.5 K
- Residual pressure inside the cryostat: 10–5 mbar
- The level of vibration displacements on a cold plate: no more than ±0.5 μm in the frequency range up to 300 Hz
- Vacuum inlets: 32 fiber optic, 2 KF25, KF16, and an optical window
- Overall dimensions: height 1450 mm, diameter 830 mm
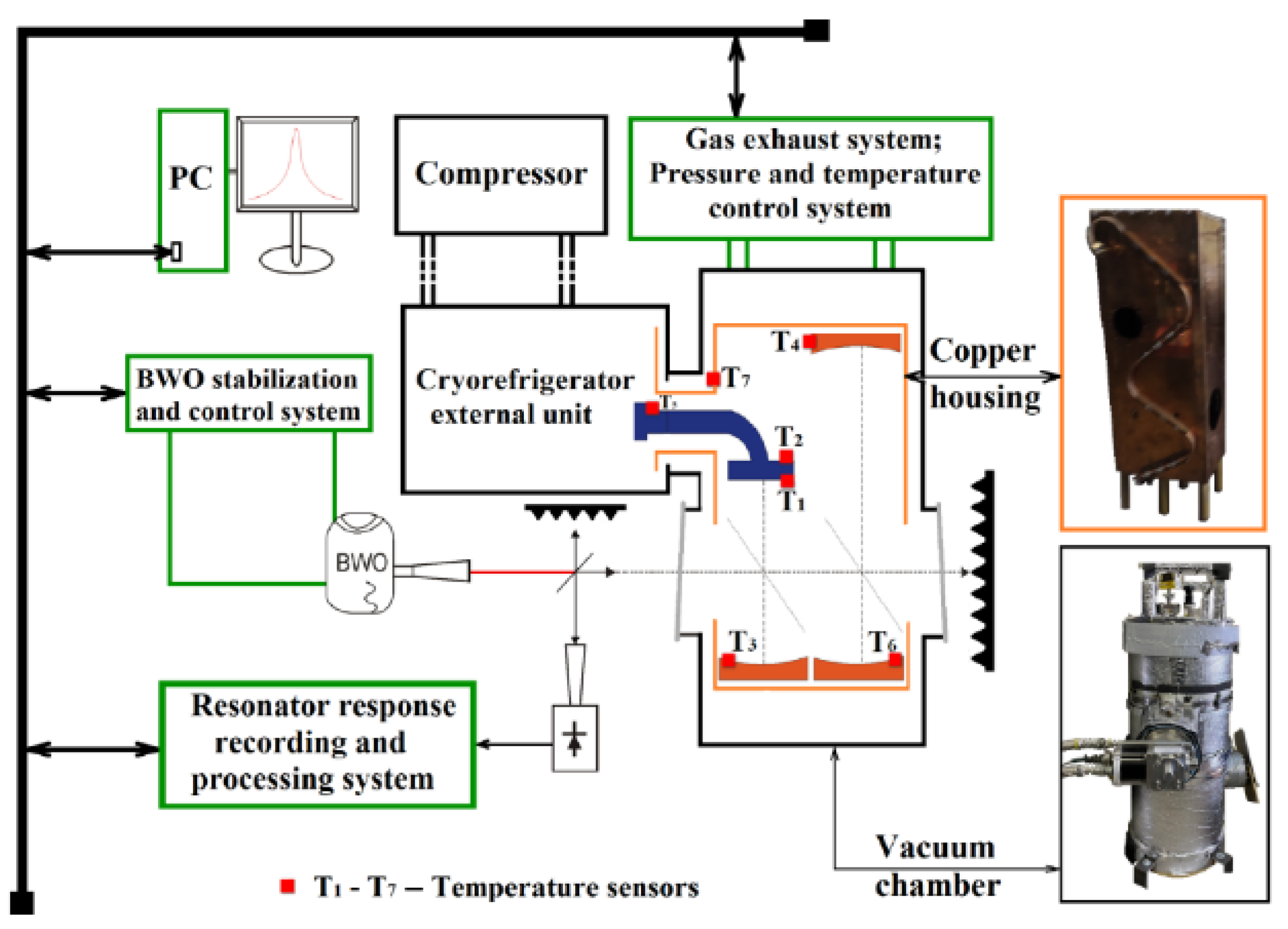
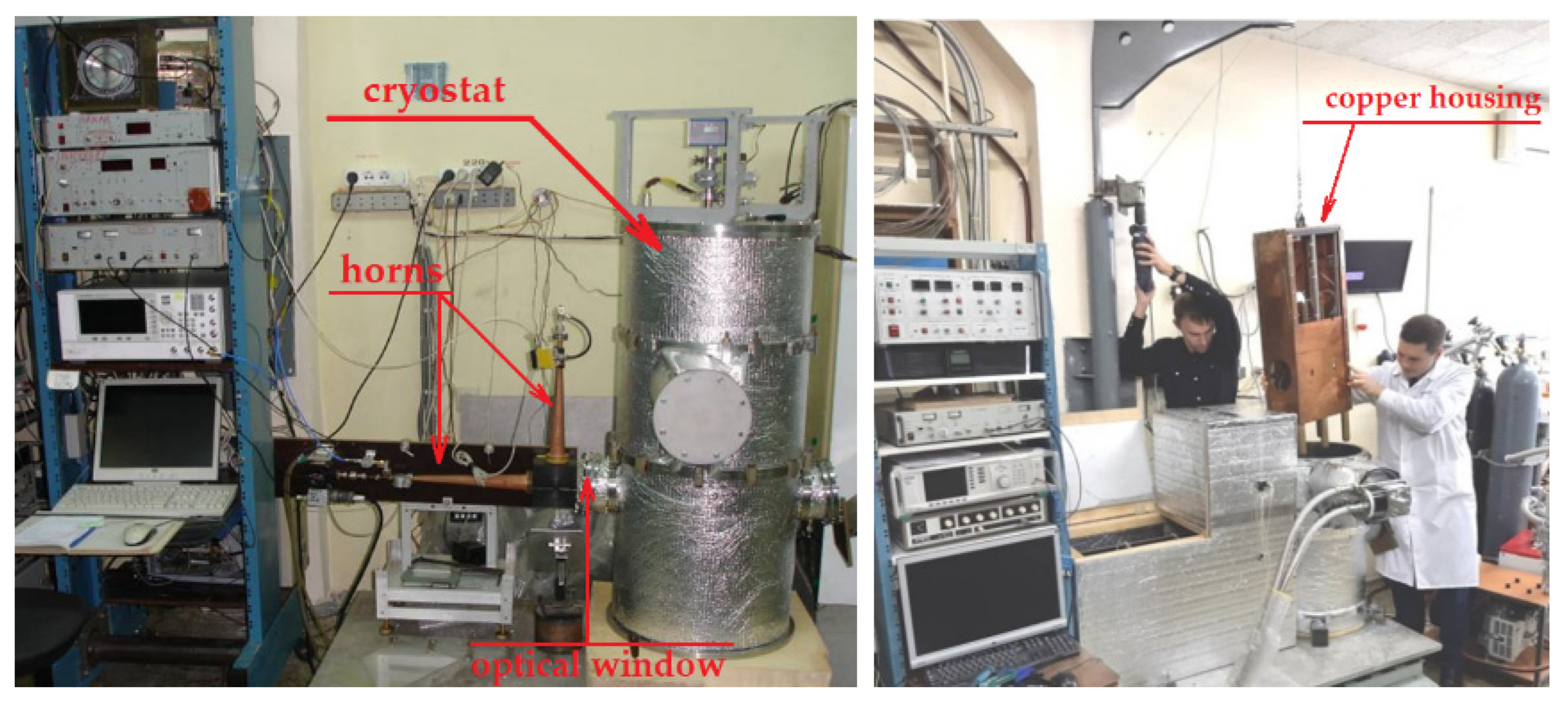
4.1.4. Cryovacuum Resonator Complex
- Frequency range: 36 ÷ 520 GHz
- Temperature range for gases: within 220–370 K with the possibility of long term stabilization at any temperature, within 10–220 K without temperature stabilization, and 4 K–900 K for dielectrics and metals
- Gas pressure: 0–1500 Torr
- Sensitivity to changes in absorption in gas: ~0.001 dB/km (4 × 10−9 cm−1)
- The range of measured values of the refractive index: 1–10 with a relative error up to 10−4
- Measured thickness of the dielectric plane-parallel plates: 0.002–30 mm with an accuracy of up to 10−4
- Minimum diameter of the solid sample under study: ~12 mm (on 140 GHz)
- Range of measured values (tgδ): 10−2 ÷ 10−7 with a relative error of up to 5%
- Range of measured values of reflection losses: 10−1 ÷ 10−4 with an average relative measurement error ~5% at the level of reflection losses ~10−3
4.2. CCD Matrix Cooling Systems
4.3. Components of Cryogenic Astronomical Receivers That Are out of the State of Thermodynamic Equilibrium
4.3.1. Physical Temperature and Noise of a Sealed Window
4.3.2. Dissipative Transmission Line at the Temperature Drop
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ladu, A.; Schirru, L.; Gaudiomonte, F.; Marongiu, P.; Angius, G.; Perini, F.; Vargiu, G.P. Upgrading of the L-P Band Cryogenic Receiver of the Sardinia Radio Telescope: A Feasibility Study. Sensors 2022, 22, 4261. [Google Scholar] [CrossRef]
- Murzin, V.A.; Markelov, S.V.; Ardilanov, V.I.; Afanaseva, T.; Borisenko, A.; Ivashchenko, N.; Pritychenko, M.; Mitiani, G.; Vdovin, V. Astronomical CCD systems for the 6-meter telescop BTA (a review). Appl. Phys. 2016, 4, 500–506. (In Russian) [Google Scholar]
- Vdovin, V.F. Problems of cryogenic cooling of superconductor and semiconductor receivers in the range 0.1–1 THz. Radiophys. Quantum Electron. 2005, 48, 779–791. [Google Scholar] [CrossRef]
- Ulbricht, G.; De Lucia, M.; Baldwin, E. Applications for Microwave Kinetic Induction Detectors in Advanced Instrumentation. Appl. Sci. 2021, 11, 2671. [Google Scholar] [CrossRef]
- Alzhanov, B.; Shafiee, M.; Kazykenov, Z.; Bekbolanova, M.; Smoot, G.; Grossan, B. The cryogenic detector for cosmology observation. IOP Conf. Ser. Mater. Sci. Eng. 2019, 502, 012060. [Google Scholar] [CrossRef]
- 27th International Cryogenics Engineering Conference and International Cryogenic Materials Conference 2018 (ICEC-ICMC 2018) 3–7 September 2018, Oxford, United Kingdom. Available online: https://iopscience.iop.org/article/10.1088/1757-899X/502/1/011001/pdf (accessed on 24 November 2023).
- Han, Y.; Zhang, A. Cryogenic technology for infrared detection in space. Sci. Rep. 2022, 12, 2349. [Google Scholar] [CrossRef] [PubMed]
- Benford, D.J.; Moseley, S.H. Cryogenic Detectors for Infrared Astronomy: The Single Aperture Far-InfraRed (SAFIR) Observatory; NASA/GSFC, Infrared Astrophysics, Code 685: Greenbelt, MD, USA, 2004.
- Archer, J.W. High-performance, 2.5-K cryostat incorporating a 100–120-GHz dual polarization receiver. Sci. Instrum. 1985, 56, 449–458. [Google Scholar] [CrossRef]
- Lang, A.; De Bernardis, P.; De Petris, M.; Masi, S.; Melchiorri, F.; Aquilini, E.; Martinis, L.; Scaramuzzi, F.; Melchiorri, B.; Boscaleri, A.; et al. The BOOMERANG experiment. Space Sci. Rev. 1995, 74, 145–150. [Google Scholar] [CrossRef]
- Little, W.A. Microminiature refrigeration. Rev. Sci. Instrum. 1984, 55, 661–680. [Google Scholar] [CrossRef]
- Edelman, V.S. Near the Absolute Zero; Science: Moscow, Russia, 1983; 176p. (In Russian) [Google Scholar]
- Space Cryogenics Workshop. 2021. Available online: https://www.sciencedirect.com/journal/cryogenics/special-issue/10KMQLLLNS1 (accessed on 24 November 2023).
- Astronomical Journal. Available online: https://journals.aas.org/astronomical-journal/ (accessed on 24 November 2023).
- Messenger, G.C. Cooling of Microwave Crystal Mixers and Antennas. Trans. Microw. Theory Tech. 1957, 5, 62–63. [Google Scholar] [CrossRef]
- Timmerhaus, K.D. Advances in Cryogenic Engineering. In Proceedings of the 1959 Cryogenic Engineering Conference, Berkeley, CA, USA, 2–4 September 1959. [Google Scholar]
- White, G.K. Experimental Techniques in Low-Temperature Physics, 3rd ed.; Clarendon Press: Oxford, UK, 1979; 331p. [Google Scholar]
- Barron, R.F. Cryogenic Systems, 2nd ed.; Oxford University Press: Oxford, UK, 1985; 507p. [Google Scholar]
- Köhler, J.W.L.; Jonkers, C.O. Fundamentals of the gas refrigeration machine. Philips Tech. Rev. 1954, 16, 69–78. [Google Scholar]
- 33rd International Symposium on Space Terahertz Technology (Coming). Available online: https://web.cvent.com/event/ed54f48e-ce7d-4361-8d7f-7c2e997215a6/summary (accessed on 24 November 2023).
- 32nd International Symposium on Space Terahertz Technology (The Last). Available online: https://www.showsbee.com/fairs/IEEE-ISSTT.html (accessed on 24 November 2023).
- 30th Space Cryogenics Workshop. Available online: https://spacecryogenicsworkshop.org (accessed on 24 November 2023).
- Wang, C. Cryogenic Engineering and Technologies. Principles and Applications of Cryogen-Free Systems; Zhao, Z., Ed.; Taylor & Francis Group, LLC.: Abingdon, UK, 2020; ISBN 978-1-4987-6576-3. [Google Scholar]
- Jha, A.R. Cryogenic Technology and Applications; Elsevier: Amsterdam, The Netherlands, 2006; ISBN 13: 978-07506-7887-2. ISBN 10: 0-7506-7887-9. [Google Scholar]
- Ventura, G.; Risegari, L. The Art of Cryogenics: Low-Temperature Experimental Techniques; Elsevier: Amsterdam, The Netherlands, 2008; ISBN 978-0-08-0444796. [Google Scholar]
- Cryogenic materials. In Handbook of Brookhaven National Laboratory; Brookhaven National Laboratory: New York, NY, USA, 1966; p. 11973.
- Malkov, M.P.; Danilov, I.B.; Zeldovich, A.G.; Fradkov, A.B. Handbook on the Physical and Technical Foundations of Cryogenics, 3rd ed.; Energoatomizdat: Moscow, Russia, 1985; p. 432.
- Martiyanov, K.; Makhalov, V.; Turlapov, A. Observation of a Two-Dimensional Fermi Gas of Atoms. Phys. Rev. Lett. 2010, 105, 030404. [Google Scholar] [CrossRef] [PubMed]
- Quinn, T.J. Temperature, 2nd ed.; Academic Press: Cambridge, MA, USA, 1990; ISBN 0-12-569681-7. [Google Scholar]
- Hill, K.D.; Steele, A.G. The International Temperature Scale: Past, Present, and Future. J. Meas. Sci. 2014, 9, 60–67. [Google Scholar] [CrossRef]
- Nicastro, A.J. Dalton’s temperature scale. Phys. Teach. 1983, 21, 6. [Google Scholar] [CrossRef]
- Masuda, T.; Hiramoto, A.; Ang, D.G.; Meisenhelder, C.; Panda, C.D.; Sasao, N.; Uetake, S.; DeMille, D.P.; Doyle, J.M.; Gabrielse, G.; et al. High-sensitivity low-noise photodetector using a large-area silicon photomultiplier. Opt. Express. 2023, 31, 1943–1957. [Google Scholar] [CrossRef]
- Tan, G.H. Atacama Large Millimeter Array. In Proceedings of the 3rd ESA Workshop on Millimeter Wave Technology and Applications: Circuit, Systems, and Meas-urement Technique, Espoo, Finland, 21–23 May 2003; p. 107. [Google Scholar]
- Vdovin, V.F.; Grachev, V.G.; Dryagin, S.Y.; Eliseev, A.I.; Kamaletdinov, R.K.; Korotaev, D.V.; Lesnov, I.V.; Mansfeld, M.A.; Pevzner, E.L.; Perminov, V.G.; et al. Cryogenically cooled low-noise amplifier for radio-astronomical observations and centimeter-wave deep-space communications systems. Astrophys. Bull. 2016, 71, 134–138. [Google Scholar] [CrossRef]
- Raisanen, A.V. Experimental Studies Cooled Millimeter Wave Mixers; Acta Polytechnica Scandinavica, Electrical Engineering Series 46; Helsinki University of Technology: Espoo, Finland, 1980. [Google Scholar]
- Weiner, S.; Pospieszalsky, M.R.; Norrod, R. Cryogenic HEMT low-noise receiver for 1.3–43 GHz. IEEE Trans. Microw. Theory Tech. 1987, MTT-35, 1067–1069. [Google Scholar]
- Zimmuidzinas, J.; Richards, P.I. Superconducting Detectors and Mixers for Millimeter and Submillimeter. Proc. IEEE 2004, 92, 1597–2006. [Google Scholar] [CrossRef]
- Rudakov, K.I.; Khudchenko, A.V.; Richards, P.I.; Filippenko, L.V.; Paramonov, M.E.; Hesper, R.; Ronsó da Costa Lima, D.D.; Baryshev, A.M.; Koshelets, V.P. THz Range Low-Noise SIS Receivers for Space and Ground-Based Radio Astronomy. Appl. Sci. 2021, 11, 10087. [Google Scholar] [CrossRef]
- Cryogenic Technologies for the Stratospheric Observatory for Infrared Astronomy (SOFIA) Science Instruments. Available online: https://ntrs.nasa.gov/api/citations/20205008777/downloads/20205008777.pdf (accessed on 24 November 2023).
- Balega, Y.; Bolshakov, O.; Chernikov, A.; Edelman, V.; Eliseev, A.; Emelyanov, E.; Gunbina, A.; Krasilnikov, A.; Lesnov, I.; Mansfeld, M.; et al. Cryogenic Systems for Astronomical Research in the Special Astrophysical Observatory of the Russian Academy of Science. Photonics 2023, 10, 1263. [Google Scholar] [CrossRef]
- About ALMA, at First Glance. Available online: https://www.almaobservatory.org/en/about-alma/ (accessed on 24 November 2023).
- May, A.J. Sub-Kelvin Cryogenics for Experimental Cosmology. In Proceedings of the 28th International Cryogenic Engineering Conference and International Cryogenic Materials Conference 2022—ICEC28-ICMC 2022, Hangzhou, China, 25–29 April 2022; Volume 70, pp. 240–248. [Google Scholar]
- Vystavkin, A.N.; Shitov, S.V.; Bankov, S.E.; Kovalenko, A.G.; Pestriakov, A.V.; Cohn, I.A.; Uvarov, A.V.; Vdovin, V.F.; Perminov, V.G.; Trofimov, V.N.; et al. High sensitive 0.13–0.38 THz TES array radiometer for the big telescope azimuthal of Special Astrophysical Observatory of Russian Academy of Sciences. In Proceedings of the 2007 Joint 32nd International Conference on Infrared and Millimeter Waves and the 15th International Conference on Terahertz Electronics, Cardiff, UK, 2–9 September 2007. [Google Scholar]
- Chernikov, A.N.; Trofimov, V.N. Helium-3 adsorption refrigerator cooled with a closed-cycle cryocooler. J. Surf. Investig. X-ray Synchrotron Neutron Tech. 2014, 8, 956–960. [Google Scholar] [CrossRef]
- Camus, P.; Vermeulen, G.; Volpe, A.; Triqueneaux, S.; Benoit, A.; Butterworth, J.; d’Escrivan, S.; Tirolien, T. Status of the Closed-Cycle Dilution Refrigerator Development for Space Astrophysics. J. Low Temp. Phys. 2013, 176, 5–6. [Google Scholar] [CrossRef]
- Frossati, G. Experimental techniques: Methods for cooling below 300 mK. J. Low Temp. Phys. 1992, 87, 595–663. [Google Scholar] [CrossRef]
- Benoit, A.; Pujol, S. A dilution refrigerator insensitive to gravity. Phys. B Condens. Matter 1991, 169, 457–458. [Google Scholar] [CrossRef]
- Benoit, A.; Caussignac, M.; Pujol, S. New types of dilution refrigerator and space applications. Phys. B 1994, 197, 48–53. [Google Scholar] [CrossRef]
- Sirbi, A.; Pouilloux, B.; Benoit, A.; Lamarre, J.-M. Influence of the astrophysical requirements on dilution refrigerator design. Cryogenics 1999, 39, 665–669. [Google Scholar] [CrossRef]
- Volpe, A. Development of a Closed Cycle Dilution Refrigerator for Astrophysical Experiments in Space. Ph.D. Thesis, Universite de Grenoble, Saint-Martin-d’Hères, France, 2014. [Google Scholar]
- Chaudhry, G.; Volpe, A.; Camus, P.; Triqueneaux, S.; Vermeulen, G.-M. A closed-cycle dilution refrigerator for space applications. Cryogenics 2012, 52, 471–477. [Google Scholar] [CrossRef][Green Version]
- Haziot, A.; Vermeulen, G. A New Type of Mixing Chamber for a Zero Gravity Dilution Refrigerator; CEC-ICMC и: Hartford, CT, USA, 2019. [Google Scholar]
- Oxford Instruments Group/Cryogenics. Available online: https://nanoscience.oxinst.com/cryogenics (accessed on 24 November 2023).
- Blueforce. Available online: https://bluefors.com (accessed on 24 November 2023).
- Masi, S.; de Bernardis, P.; De Troia, G.; Giacometti, M.; Iacoangeli, A.; Piacentini, F.; Polenta, G.; Ade, P.A.R.; Mauskopf, P.D.; Bock, J.J.; et al. The BOOMERanG experiment and the curvature of the universe. Prog. Part. Nucl. Phys. 2002, 48, 243–261. [Google Scholar] [CrossRef]
- Nati, F.; Ade, P.; Boscaleri, A.; Brienza, D.; Calvo, M.; Colafrancesco, S.; Conversi, L.; de Bernardis, P.; De Petris, M.; Delbart, A.; et al. The OLIMPO experiment. New Astron. Rev. 2007, 51, 385–389. [Google Scholar] [CrossRef]
- Masi, S.; de Bernardis, P.; Paiella, A.; Piacentini, F.; Lamagna, L.; Coppolecchia, A.; Ade, P.A.R.; Battistelli, E.S.; Castellano, M.G.; Colantoni, I.; et al. Kinetic Inductance Detectors for the OLIMPO experiment: In-flight operation and performance. J. Cosmol. Astropart. Phys. 2019, 2019. [Google Scholar] [CrossRef]
- Nati, L.; Calvo de Bernardis, P.; Fiadino, P.; Lamagna, L.; Masi, S.; Piacentini, R.; Rispoli, R. Cryogenic systems for Long Duration Balloon experiments. Mem. Della Soc. Astron. Ital. 2008, 79, 878–882. [Google Scholar]
- Cold Facts Issued by the Cryogenic Society of America, Inc. Available online: https://www.cryogenicsociety.org/cold-facts#:~:text=Cold%20Facts%2C%20an%20internationally%20recognized,%20every%20member%20of%20the%20CSA (accessed on 24 November 2023).
- Yusov, A.V.; Kozlov, S.A.; Ustinova, E.A.; Arkhipov, M.Y.; Kostrov, E.A.; Smirnov, A.V.; Kuznetsov, I.V.; Mansfeld, M.A.; Tyatushkin, N.V.; Kovalev, F.N.; et al. Testing high-precision electromechanical actuators used for adjustment of deployable antennas of astronomy space missions. Cryogenics 2021, 118, 103346. [Google Scholar] [CrossRef]
- Parshin, V.V.; Serov, E.A.; Bubnov, G.M.; Vdovin, V.F.; Koshelev, M.A.; Tretyakov, M.Y. Cryogenic resonator complex. Radiophys. Quantum Electron. 2014, 56, 554–560. [Google Scholar] [CrossRef]
- Gunbina, A.A.; Serov, E.A.; Mineev, K.V.; Parshin, V.V.; Vdovin, V.F.; Chekushkin, A.M.; Khan, F.V.; Koshelets, V.P. Experimental Study of the Reflectivity of Superconducting Nb-Based Films in the Subterahertz Frequency Band. Radiophys. Quantum Electron. 2022, 65, 471–480. [Google Scholar] [CrossRef]
- Serov, E.A.; Parshin, V.V.; Bubnov, G.M. Reflectivity of metals in the millimeter wavelength range at cryogenic temperatures. IEEE Trans. Microw. Theory Tech. 2016, 64, 3828–3838. [Google Scholar] [CrossRef]
- Parshin, V.V.; Serov, E.A.; Bubnov, G.M.; Vdovin, V.F.; Nikolenko, A.S.; Lesnov, I.V.; Gunbina, A.A.; Smirnov, A.V.; Malginov, V.A.; Dolzenko, D.E.; et al. Terahertz Reflectivity of YBa2Cu3O7-δ at Cryogenic Temperatures. IEEE Trans. Appl. Supercond. 2020, 30, 9001705. [Google Scholar] [CrossRef]
- Gardner, D.V. Does Your CCD Camera Need Cooling? Available online: https://www.photonics.com/Articles/Does_Your_CCD_Camera_Need_Cooling/a12810 (accessed on 24 November 2023).
- Cease, H.; DePoy, D.; Derylo, G.; Diehl, H.T.; Estrada, J.; Flaugher, B.; Kuk, K.; Kuhlmann, S.; Lathrop, A.; Schultz, K.; et al. Cooling the dark energy camera CCD array using a closed-loop two-phase liquid nitrogen system. In Modern Technologies in Space and Ground-Base Telescopes and Instrumentation; SPIE: Bellingham, DC, USA, 2010; p. 77393N. [Google Scholar]
- Afanasieva, I.; Murzin, V.; Ardilanov, V.; Ivaschenko, N.; Pritchenko, M.; Moiseev, A.; Shablovinskaya, E.; Malginov, E. Astronomical Camera Based on a CCD261-84 Detector with Increased Sensitivity in the Near-Infrared. Photonics 2023, 10, 774. [Google Scholar] [CrossRef]
- Vdovin, V.F.; Korotaev, D.V.; Lapkin, I.V. Collection of Abstracts of the 11th International School of Microwave Radiophysics and RF Electrodynamics; IAP PAS: Nizhniy Novgorod, Russia, 1993; p. 21. [Google Scholar]
- Lamb, W. Miscellaneous data on materials for millimetre and submillimetre optics. Int. J. Infrared Millim. Wave 1993, 14, 951. [Google Scholar] [CrossRef]
- Siegel, R.; Howell, J.R. Thermal Radiation. Heat Transfer; McGraw-Hill Book Company: New-York, NY, USA, 1972. [Google Scholar]
- Marchiori, G.; Rampini, F.; Tordi, M.; Spinola, M.; Bressan, R. Towards the Eurasian Submillimeter Telescope (ESMT): Telescope concept outline and first results. In Proceedings of the Ground-Based Astronomy in Russia. 21st Century; Romanyuk, I.I., Yakunin, I.A., Valeev, A.F., Kudryavtsev, D.O., Eds.; Special Astrophysical Observatory: Nizhnii Arkhyz, Russia, 2020; pp. 378–383. [Google Scholar]
- Hojaev, A.; Shanin, G.I.; Artyomenko, Y.N. Suffa Radio Observatory in Uzbekistan: Progress and radio-seeing research plans. Proc. Int. Astron. Union 2007, 5, 177–182. [Google Scholar] [CrossRef]
- Wang, N. Xinjiang Qitai 110 m radio telescope. Sci. Sin. Phys. Mech. Astron. 2014, 44, 783–794. [Google Scholar] [CrossRef]
- Doeleman, S.S.; Barrett, J.; Blackburn, L.; Bouman, K.; Broderick, A.E.; Chaves, R.; Fish, V.L.; Fitzpatrick, G.; Fuentes, A.; Freeman, M.; et al. Reference Array and Design Consideration for the next-generation Event Horizon Telescope. arXiv 2023, arXiv:2306.08787. [Google Scholar] [CrossRef]

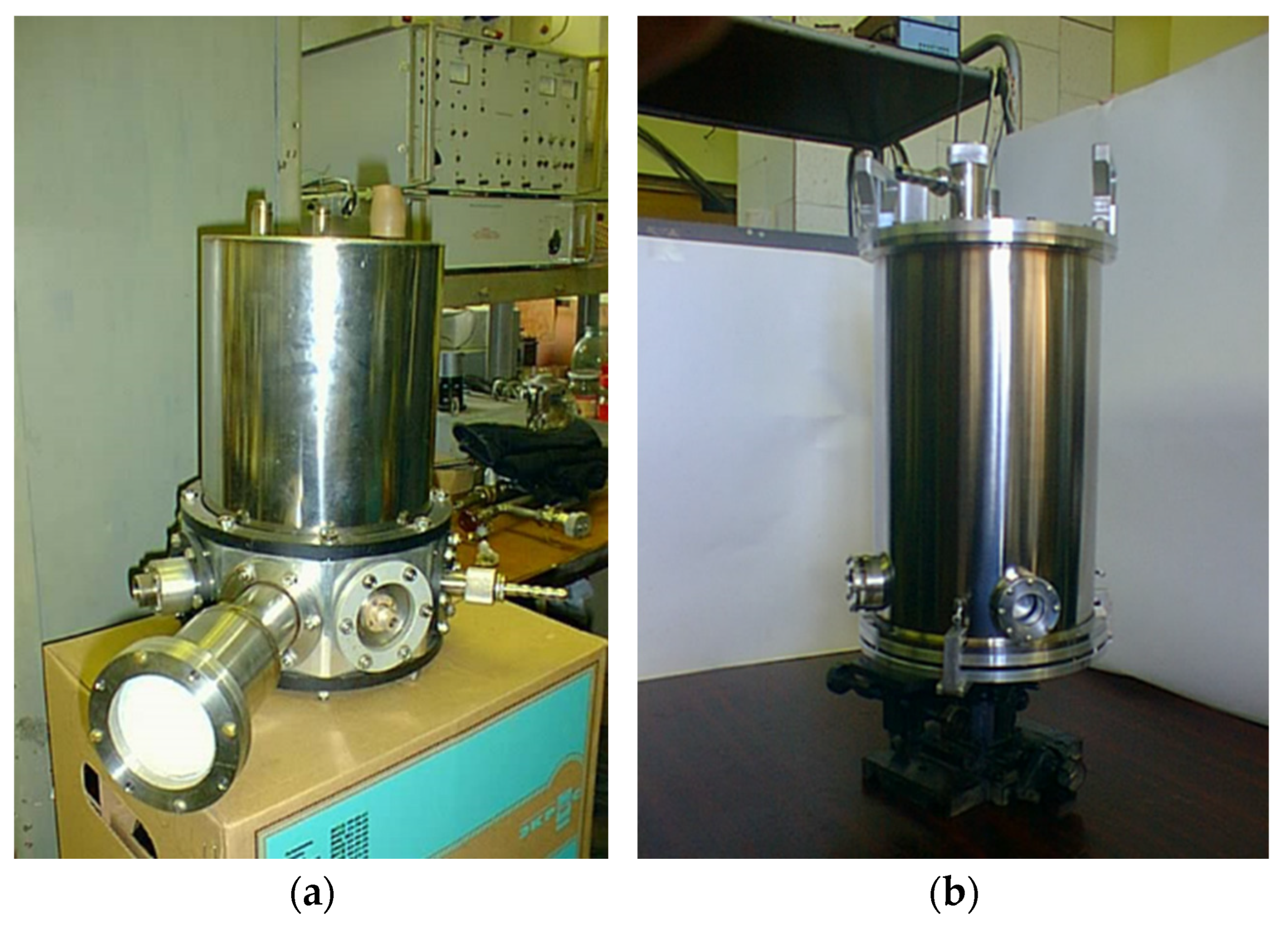



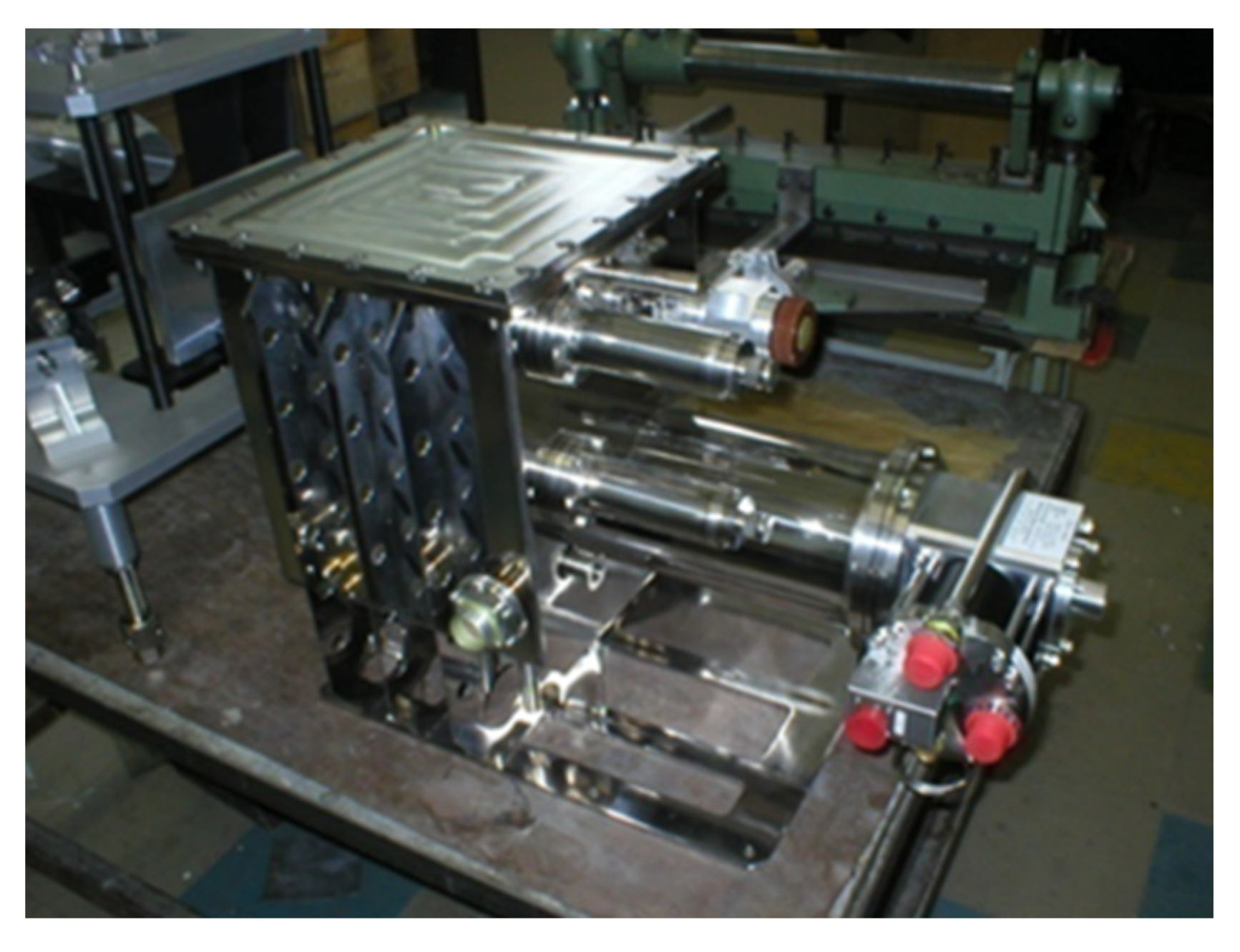











| Group Name | Temperature Range | Applications |
|---|---|---|
| Non-cryogenic temperatures | T > 100 (120) K |
|
| High cryogenic temperatures | 100 K |
|
| Nitrogen level | ~77 K |
|
| Hydrogen level | ~20 K |
|
| Helium level | ~4 K (up to 1.6 K with pumping) |
|
| Sub-Kelvin cooling | <1 K |
|
| Extremely low temperatures reached | ~10−9 K |
|
| Photo | Characteristic | Advantages |
|---|---|---|
| Chamber with remote cooling | ||
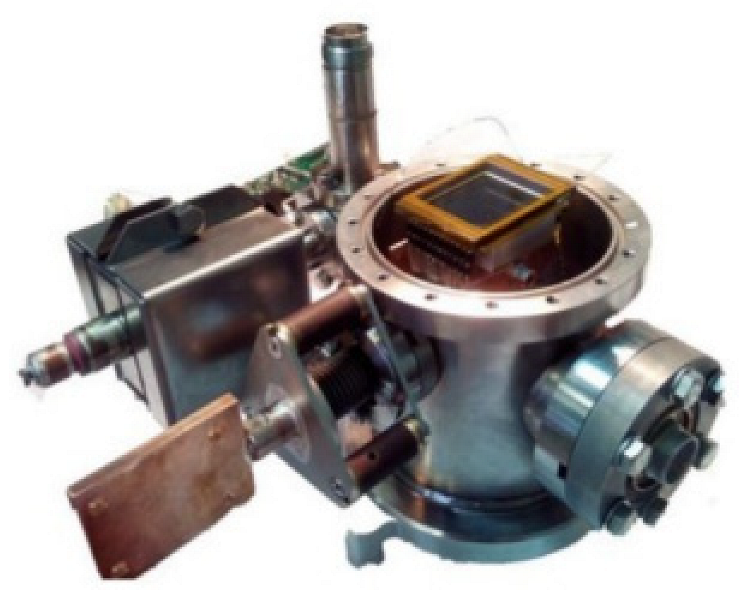 |
|
|
| Cryogenic transport system | ||
 |
|
|
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Balega, Y.; Bolshakov, O.; Chernikov, A.; Gunbina, A.; Edelman, V.; Efimova, M.; Eliseev, A.; Krasilnikov, A.; Lapkin, I.; Lesnov, I.; et al. Development of Cryogenic Systems for Astronomical Research. Photonics 2024, 11, 257. https://doi.org/10.3390/photonics11030257
Balega Y, Bolshakov O, Chernikov A, Gunbina A, Edelman V, Efimova M, Eliseev A, Krasilnikov A, Lapkin I, Lesnov I, et al. Development of Cryogenic Systems for Astronomical Research. Photonics. 2024; 11(3):257. https://doi.org/10.3390/photonics11030257
Chicago/Turabian StyleBalega, Yuri, Oleg Bolshakov, Aleksandr Chernikov, Aleksandra Gunbina, Valerian Edelman, Mariya Efimova, Aleksandr Eliseev, Artem Krasilnikov, Igor Lapkin, Ilya Lesnov, and et al. 2024. "Development of Cryogenic Systems for Astronomical Research" Photonics 11, no. 3: 257. https://doi.org/10.3390/photonics11030257
APA StyleBalega, Y., Bolshakov, O., Chernikov, A., Gunbina, A., Edelman, V., Efimova, M., Eliseev, A., Krasilnikov, A., Lapkin, I., Lesnov, I., Mansfeld, M., Markina, M., Pevzner, E., Shitov, S., Smirnov, A., Tarasov, M., Tyatushkin, N., Vdovin, A., & Vdovin, V. (2024). Development of Cryogenic Systems for Astronomical Research. Photonics, 11(3), 257. https://doi.org/10.3390/photonics11030257





