Fabrication of Micro/Nano Dual Needle Structures with Morphological Gradient Based on Two-Photon Polymerization Laser Direct Writing with Proactive Focus Compensation
Abstract
1. Introduction
2. Methods
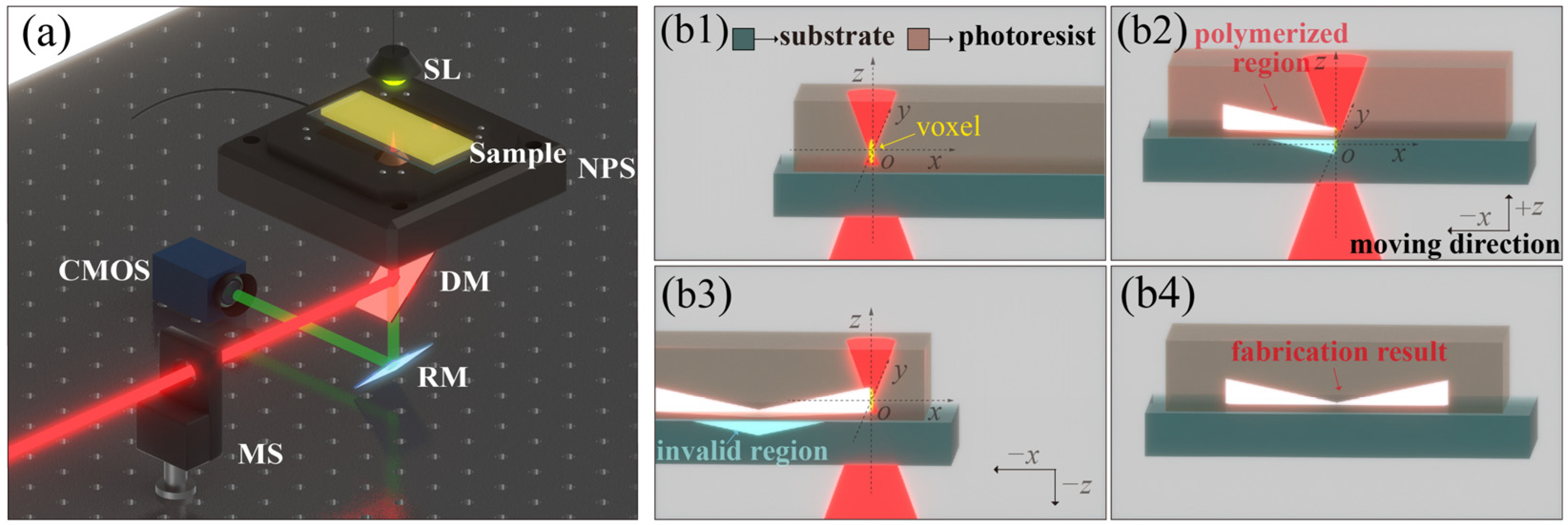
2.1. Setup and Fabrication Process
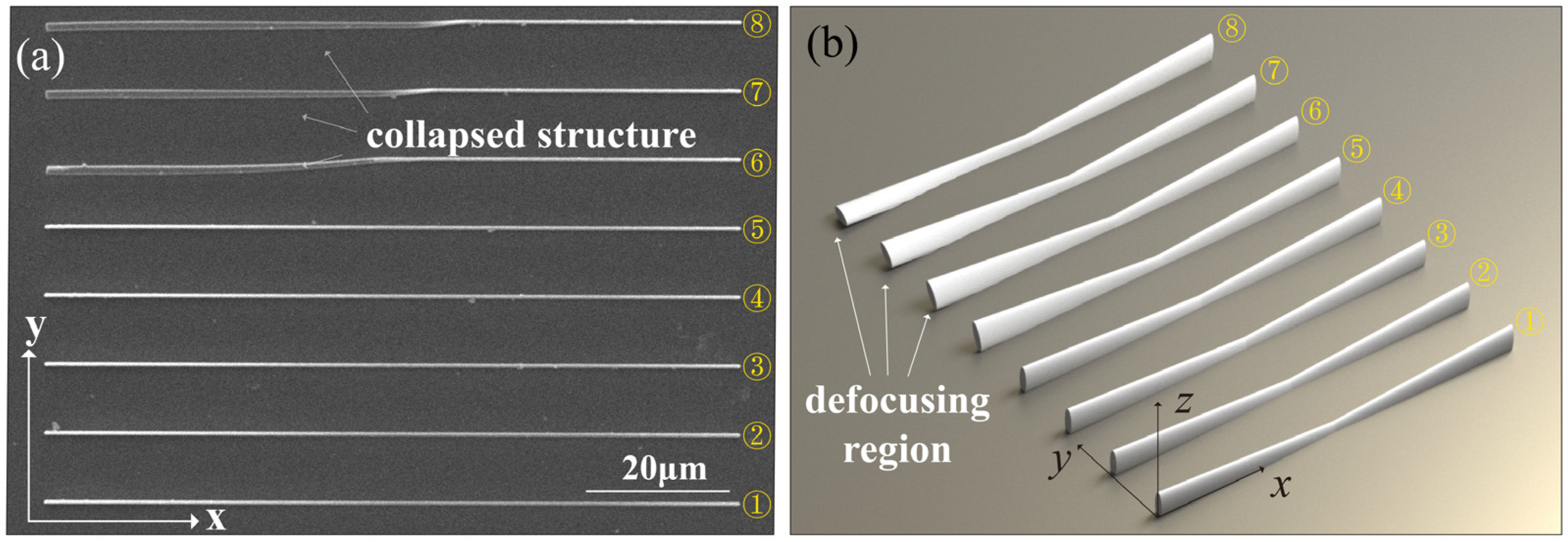
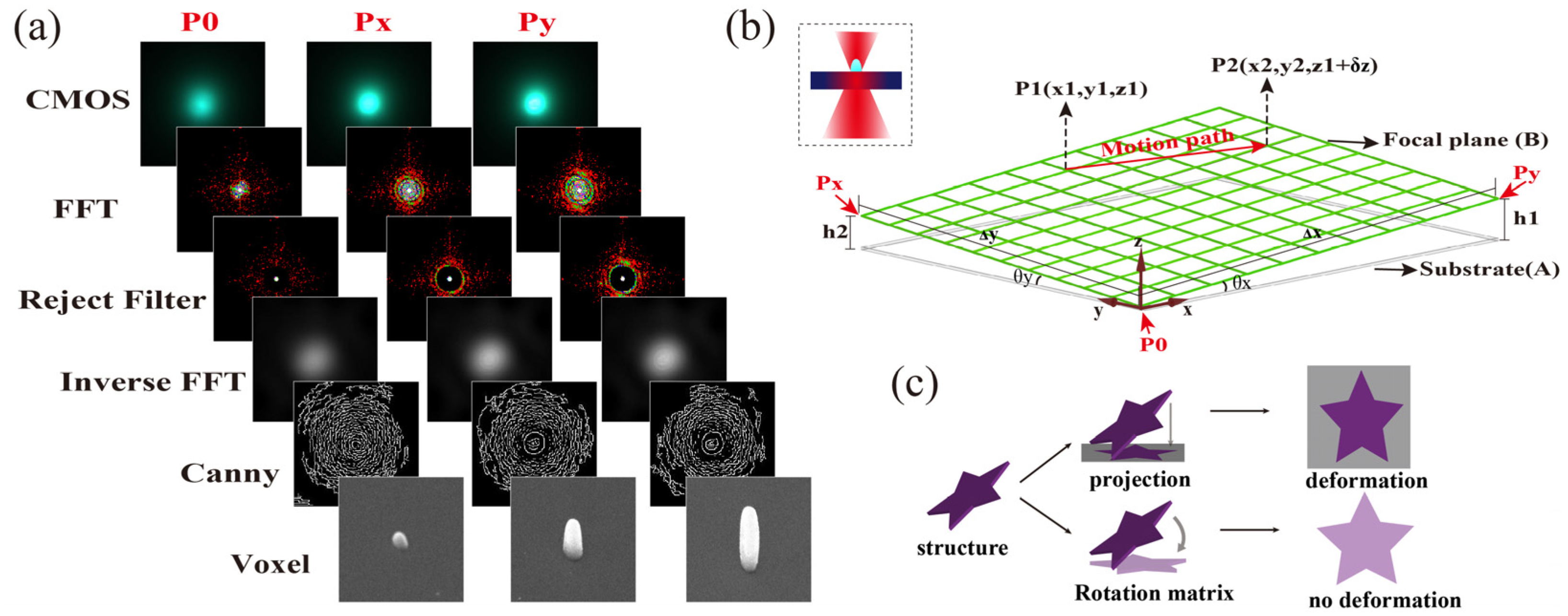
2.2. Focus Self Compensation
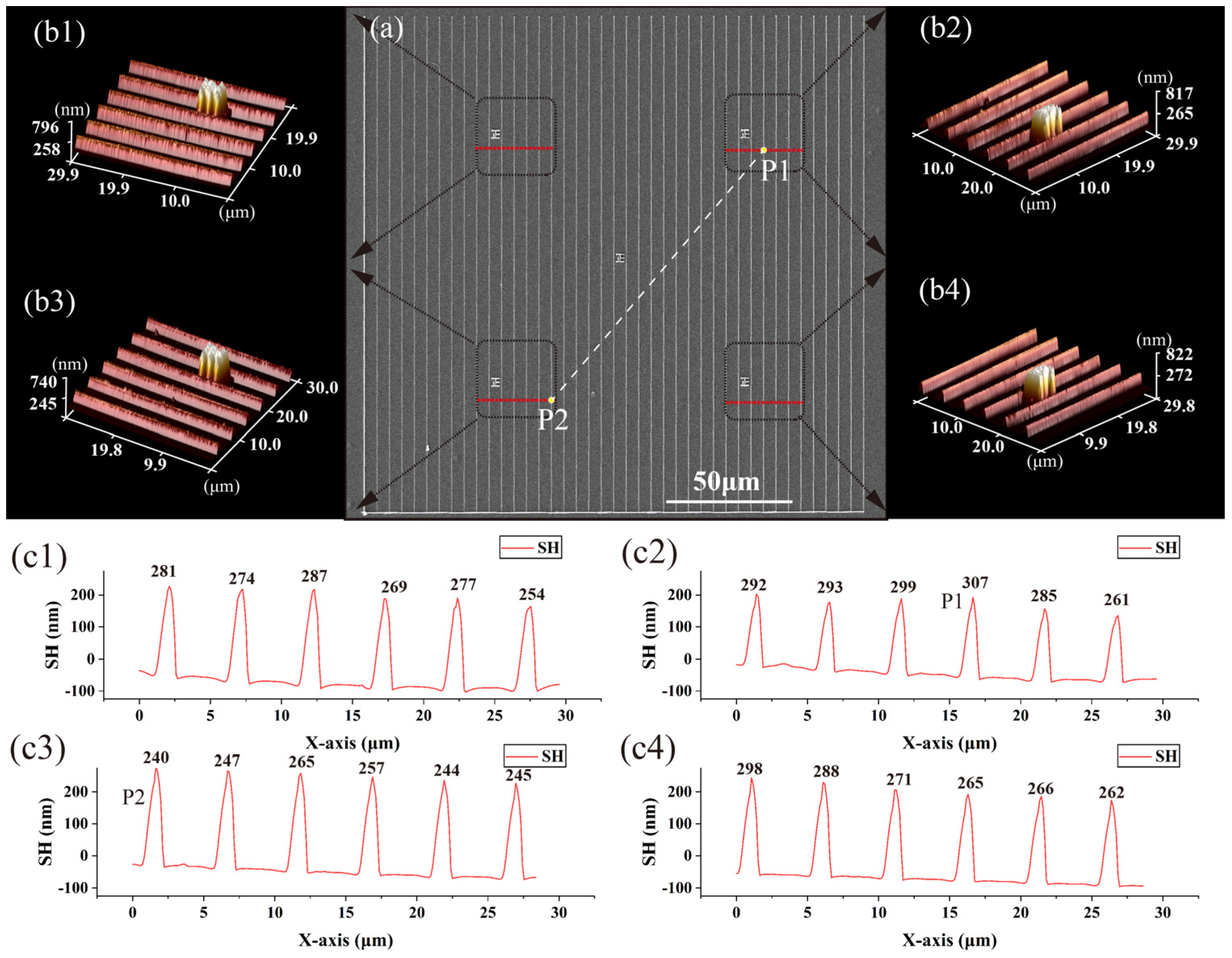
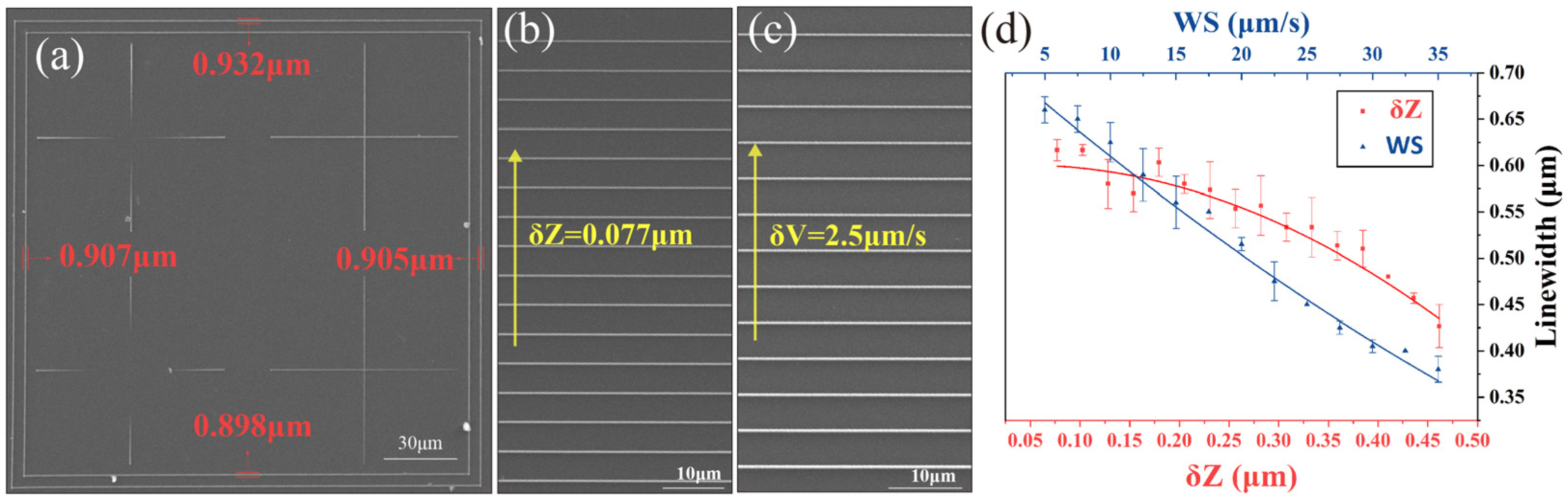
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Duleba, D.; Johnson, R.P. Sensing with ion current rectifying solid-state nanopores. Curr. Opin. Electrochem. 2022, 34, 100989. [Google Scholar] [CrossRef]
- Zhou, Y.; Liao, X.; Han, J.; Chen, T.; Wang, C. Ionic current rectification in asymmetric nanofluidic devices. Chin. Chem. Lett. 2020, 31, 2414–2422. [Google Scholar] [CrossRef]
- Tu, Y.-M.; Samineni, L.; Ren, T.; Schantz, A.B.; Song, W.; Sharma, S.; Kumar, M. Prospective applications of nanometer-scale pore size biomimetic and bioinspired membranes. J. Membr. Sci. 2021, 620, 118968. [Google Scholar] [CrossRef]
- Chen, T.; Wang, D.; Chen, X.; Qiu, M.; Fan, Y. Three-dimensional printing of high-flux ceramic membranes with an asymmetric structure via digital light processing. Ceram. Int. 2022, 48, 304–312. [Google Scholar] [CrossRef]
- Ko, J.; Fredj, N.B.; Adhawiyah, R.E.; Lee, J. Nozzle-based precision patterning with micro-/nano fluidics integrated cantilevers. J. Mech. Sci. Technol. 2023, 37, 887–900. [Google Scholar] [CrossRef]
- Wang, D.; Abbas, Z.; Lu, L.; Liang, S.; Zhao, X.; Xu, P.; Zhao, K.; Suo, L.; Cui, Y.; Yin, P. Simulation of cone-jet and micro-drip regimes and printing of micro-scale patterns on PET substrate. Polymers 2022, 14, 2683. [Google Scholar] [CrossRef] [PubMed]
- Li, J.; Guo, Z. Spontaneous directional transportations of water droplets on surfaces driven by gradient structures. Nanoscale 2018, 10, 13814–13831. [Google Scholar] [CrossRef] [PubMed]
- Wen, T.; Zhang, C.; Gong, Y.; Liu, Z.; Zhao, W.; Zhan, Y.; Zhang, C.; Wang, K.; Bai, J. High-durability photothermal slippery surfaces for droplet manipulation based on ultraviolet lithography. Polymers 2023, 15, 1132. [Google Scholar] [CrossRef]
- Liu, Y.; Zhou, B.; Wang, W.; Shen, J.; Kou, W.; Li, Z.; Zhang, D.; Guo, L.; Lau, C.; Lu, J. Insertable, scabbarded, and nanoetched silver needle sensor for hazardous element depth profiling by laser-induced breakdown spectroscopy. ACS Sens. 2022, 7, 1381–1389. [Google Scholar] [CrossRef]
- Downs, A.M.; Bolotsky, A.; Weaver, B.M.; Bennett, H.; Wolff, N.; Polsky, R.; Miller, P.R. Microneedle electrochemical aptamer-based sensing: Real-time small molecule measurements using sensor-embedded, commercially-available stainless steel microneedles. Biosens. Bioelectron. 2023, 236, 115408. [Google Scholar] [CrossRef]
- Zhang, W.; Li, W.; Zhang, T.; Zheng, Z.; Chi, Z.; Jiang, Y.; Wu, N. A large-size and polarization-independent two dimensional grating fabricated by scanned reactive-ion-beam etching. Nanophotonics 2022, 11, 4649–4657. [Google Scholar] [CrossRef]
- Desbiolles, B.; Bertsch, A.; Renaud, P. Ion beam etching redeposition for 3D multimaterial nanostructure manufacturing. Microsyst. Nanoeng. 2019, 5, 11. [Google Scholar] [CrossRef] [PubMed]
- Saifullah, M.S.; Asbahi, M.; Neo, D.C.; Mahfoud, Z.; Tan, H.R.; Ha, S.T.; Dwivedi, N.; Dutta, T.; bin Dolmanan, S.; Aabdin, Z. Patterning at the resolution limit of commercial electron beam lithography. Nano Lett. 2022, 22, 7432–7440. [Google Scholar] [CrossRef] [PubMed]
- Lewis, S.M.; DeRose, G.A.; Alty, H.R.; Hunt, M.S.; Lee, N.; Mann, J.A.; Grindell, R.; Wertheim, A.; De Rose, L.; Fernandez, A. Tuning the performance of negative tone electron beam resists for the next generation lithography. Adv. Funct. Mater. 2022, 32, 2202710. [Google Scholar] [CrossRef]
- Tian, Y.; Polzer, F.B.; Zhang, H.V.; Kiick, K.L.; Saven, J.G.; Pochan, D.J. Nanotubes, plates, and needles: Pathway-dependent self-assembly of computationally designed peptides. Biomacromolecules 2018, 19, 4286–4298. [Google Scholar] [CrossRef] [PubMed]
- Ghosh, D.; Farahani, A.D.; Martin, A.D.; Thordarson, P.; Damodaran, K.K. Unraveling the self-assembly modes in multicomponent supramolecular gels using single-crystal X-ray diffraction. Chem. Mater. 2020, 32, 3517–3527. [Google Scholar] [CrossRef]
- Huang, S.; Zhang, Q.; Xin, P.; Zhang, J.; Chen, Q.; Fu, J.; Jin, Z.; Wang, Q.; Hu, Z. Construction of Fe-doped NiS–NiS2 heterostructured microspheres via etching prussian blue analogues for efficient water-urea splitting. Small 2022, 18, 2106841. [Google Scholar] [CrossRef]
- Khanna, S.; Paneliya, S.; Ray, A.; Mukhopadhyay, I.; Banerjee, R. Controlled etching of silica nanospheres monolayer for template application: A systematic study. Appl. Surf. Sci. 2020, 500, 144050. [Google Scholar]
- Chen, S.-T.; Huang, C.-T.; Zheng, M.-Y.; Yen, H.-Y. Co-shaft in-situ rolling-imprinting technique for printing of silver micro-nanowire array. J. Mater. Process. Technol. 2022, 299, 117387. [Google Scholar] [CrossRef]
- Cox, L.M.; Martinez, A.M.; Blevins, A.K.; Sowan, N.; Ding, Y.; Bowman, C.N. Nanoimprint lithography: Emergent materials and methods of actuation. Nano Today 2020, 31, 100838. [Google Scholar] [CrossRef]
- Ding, H.; Zhang, Q.; Gu, Z.; Gu, M. 3D computer-aided nanoprinting for solid-state nanopores. Nanoscale Horiz. 2018, 3, 312–316. [Google Scholar] [CrossRef] [PubMed]
- Cao, Q.; Shin, M.; Lavrik, N.V.; Venton, B.J. 3D-printed carbon nanoelectrodes for in vivo neurotransmitter sensing. Nano Lett. 2020, 20, 6831–6836. [Google Scholar] [CrossRef] [PubMed]
- Faraji Rad, Z.; Nordon, R.E.; Anthony, C.J.; Bilston, L.; Prewett, P.D.; Arns, J.-Y.; Arns, C.H.; Zhang, L.; Davies, G.J. High-fidelity replication of thermoplastic microneedles with open microfluidic channels. Microsyst. Nanoeng. 2017, 3, 1–11. [Google Scholar] [CrossRef] [PubMed]
- Cordeiro, A.S.; Tekko, I.A.; Jomaa, M.H.; Vora, L.; McAlister, E.; Volpe-Zanutto, F.; Nethery, M.; Baine, P.T.; Mitchell, N.; McNeill, D.W. Two-photon polymerisation 3D printing of microneedle array templates with versatile designs: Application in the development of polymeric drug delivery systems. Pharm. Res. 2020, 37, 1–15. [Google Scholar] [CrossRef] [PubMed]
- Plamadeala, C.; Gosain, S.R.; Hischen, F.; Buchroithner, B.; Puthukodan, S.; Jacak, J.; Bocchino, A.; Whelan, D.; O’Mahony, C.; Baumgartner, W. Bio-inspired microneedle design for efficient drug/vaccine coating. Biomed. Microdevices 2020, 22, 1–9. [Google Scholar] [CrossRef] [PubMed]
- Zhang, C.; Hou, J.Q.; Lin, J.Q.; Li, K.; Fan, L.B.; Zhang, C.; Wang, K.G.; Bai, J.T. Investigation on fabricating continuous gradient micro/nano needle structure by single Femtosecond laser voxel (Invited). Acta Photonica Sinica 2022, 51, 1014001. [Google Scholar]
- Thiel, M.; Fischer, J.; Von Freymann, G.; Wegener, M. Direct laser writing of three-dimensional submicron structures using a continuous-wave laser at 532 nm. Appl. Phys. Lett. 2010, 97, 221102. [Google Scholar] [CrossRef]
- Sun, H.-B.; Takada, K.; Kim, M.-S.; Lee, K.-S.; Kawata, S. Scaling laws of voxels in two-photon photopolymerization nanofabrication. Appl. Phys. Lett. 2003, 83, 1104–1106. [Google Scholar] [CrossRef]
- Cao, B.X.; Le, P.H.; Ahn, S.; Kang, H.; Kim, J.; Noh, J. Automatic real-time focus control system for laser processing using dynamic focusing optical system. Opt. Express 2017, 25, 28427–28441. [Google Scholar] [CrossRef]
- Zhang, C.; Hou, J.; Zeng, Y.; Dai, L.; Zhao, W.; Jing, G.; Sun, D.; Cao, Y.; Zhang, C. An optically fabricated gradient nanochannel array to access the translocation dynamics of T4-phage DNA through nanoconfinement. Lab Chip 2023, 23, 3811–3819. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, C.; Zhang, C.; Zhao, W.; Liu, Y.; Li, Z.; Wang, Z.; Lu, B.; Wang, K.; Bai, J. Fabrication of Micro/Nano Dual Needle Structures with Morphological Gradient Based on Two-Photon Polymerization Laser Direct Writing with Proactive Focus Compensation. Photonics 2024, 11, 187. https://doi.org/10.3390/photonics11020187
Xu C, Zhang C, Zhao W, Liu Y, Li Z, Wang Z, Lu B, Wang K, Bai J. Fabrication of Micro/Nano Dual Needle Structures with Morphological Gradient Based on Two-Photon Polymerization Laser Direct Writing with Proactive Focus Compensation. Photonics. 2024; 11(2):187. https://doi.org/10.3390/photonics11020187
Chicago/Turabian StyleXu, Chenxi, Chen Zhang, Wei Zhao, Yining Liu, Ziyu Li, Zeyu Wang, Baole Lu, Kaige Wang, and Jintao Bai. 2024. "Fabrication of Micro/Nano Dual Needle Structures with Morphological Gradient Based on Two-Photon Polymerization Laser Direct Writing with Proactive Focus Compensation" Photonics 11, no. 2: 187. https://doi.org/10.3390/photonics11020187
APA StyleXu, C., Zhang, C., Zhao, W., Liu, Y., Li, Z., Wang, Z., Lu, B., Wang, K., & Bai, J. (2024). Fabrication of Micro/Nano Dual Needle Structures with Morphological Gradient Based on Two-Photon Polymerization Laser Direct Writing with Proactive Focus Compensation. Photonics, 11(2), 187. https://doi.org/10.3390/photonics11020187





