Research on the Fabrication and Parameters of a Flexible Fiber Optic Pressure Sensor with High Sensitivity
Abstract
1. Introduction
2. Theory and Methods
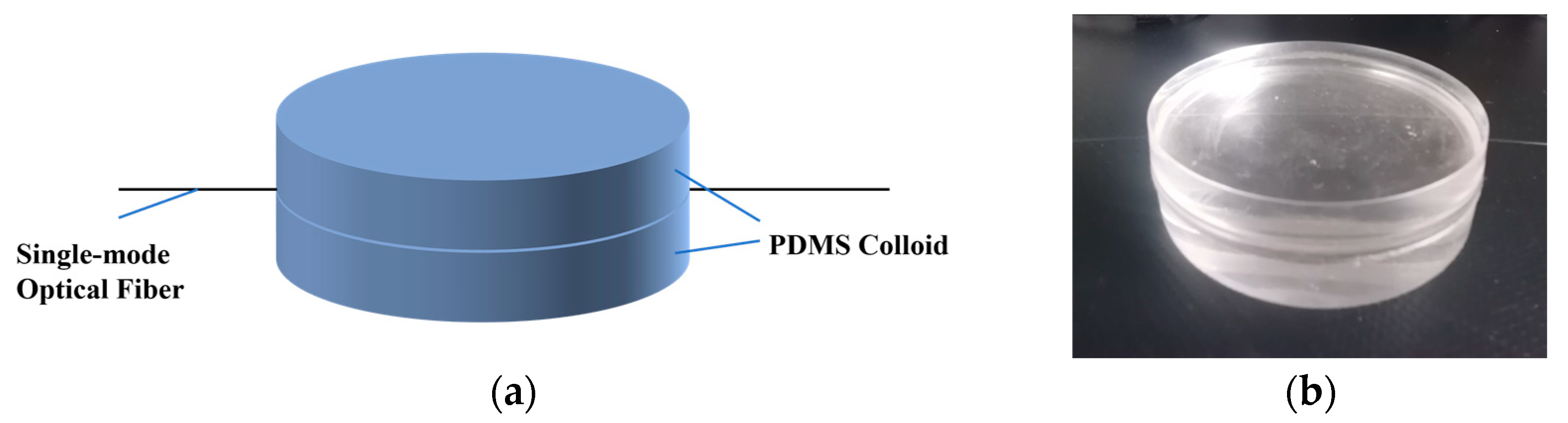
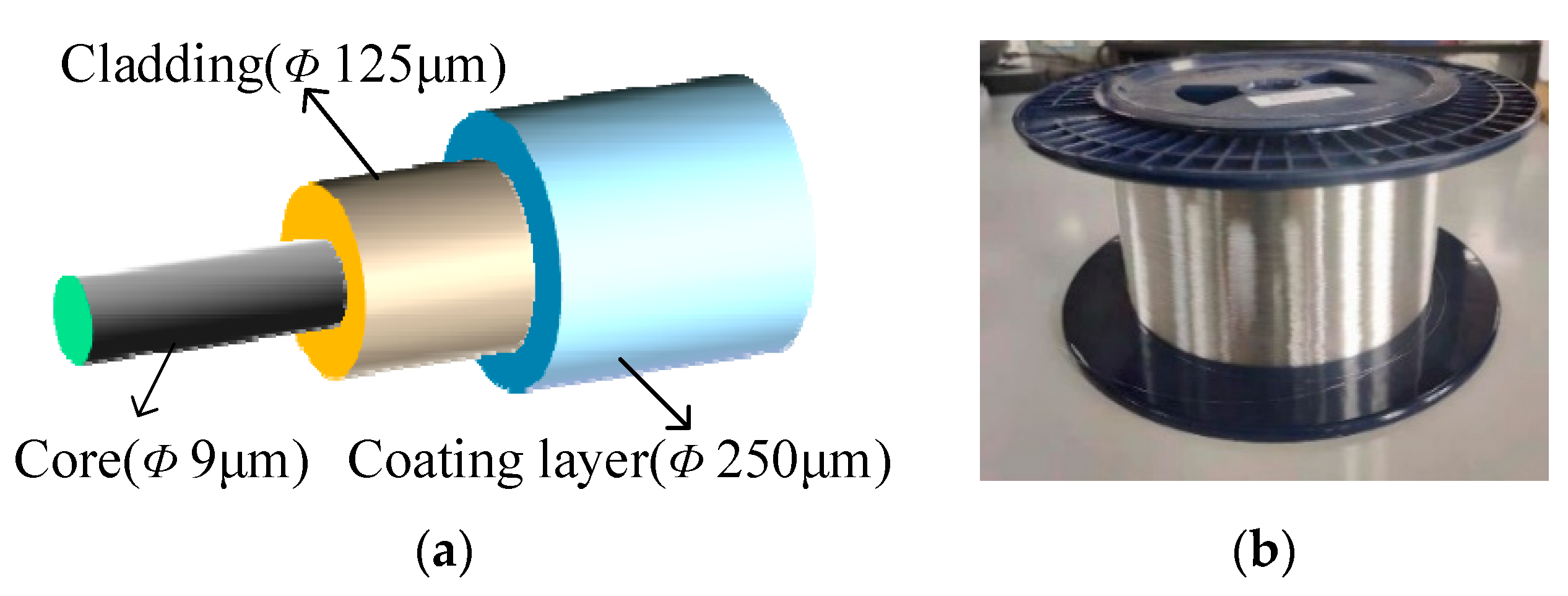
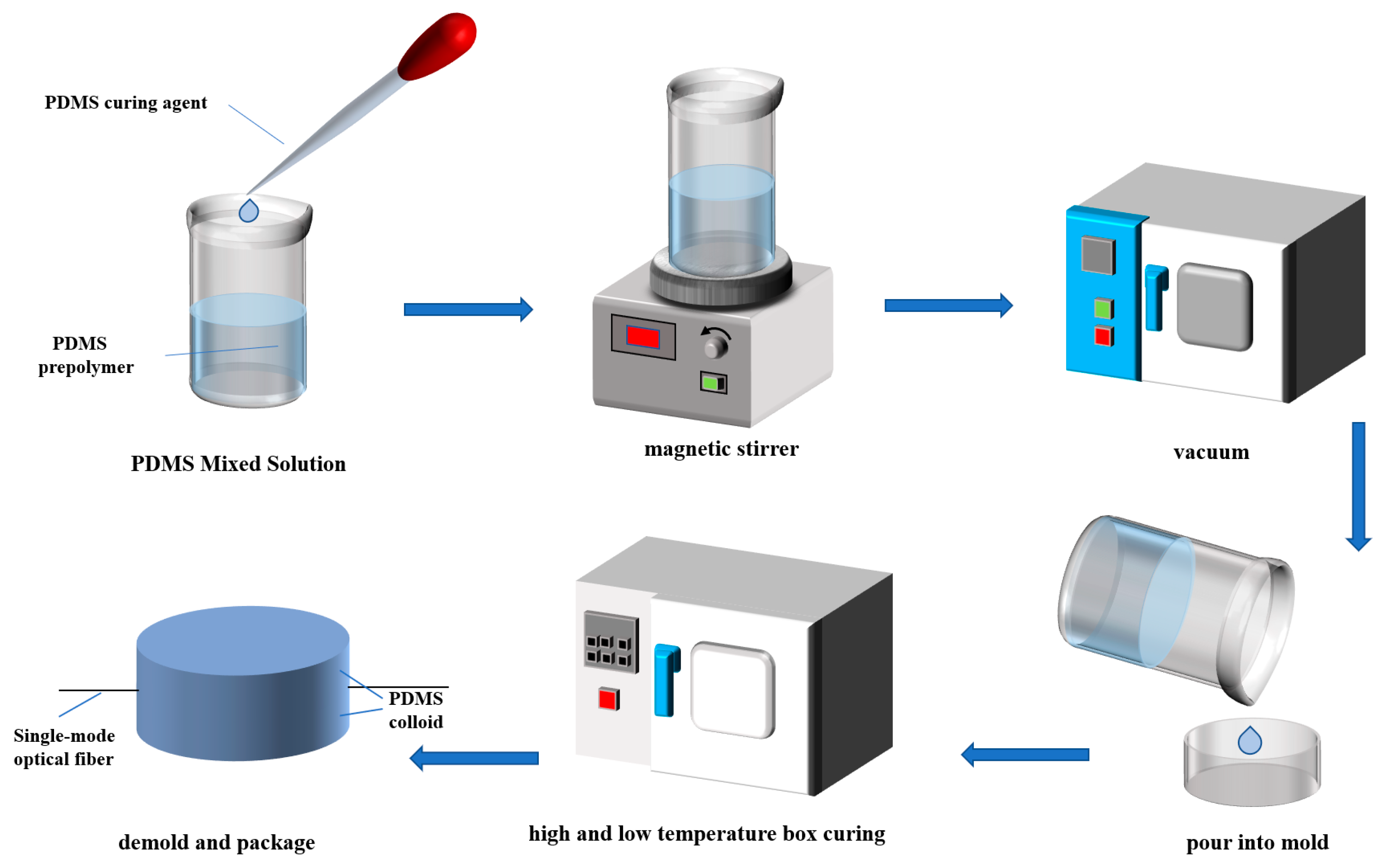
2.1. Sensor Structure Design
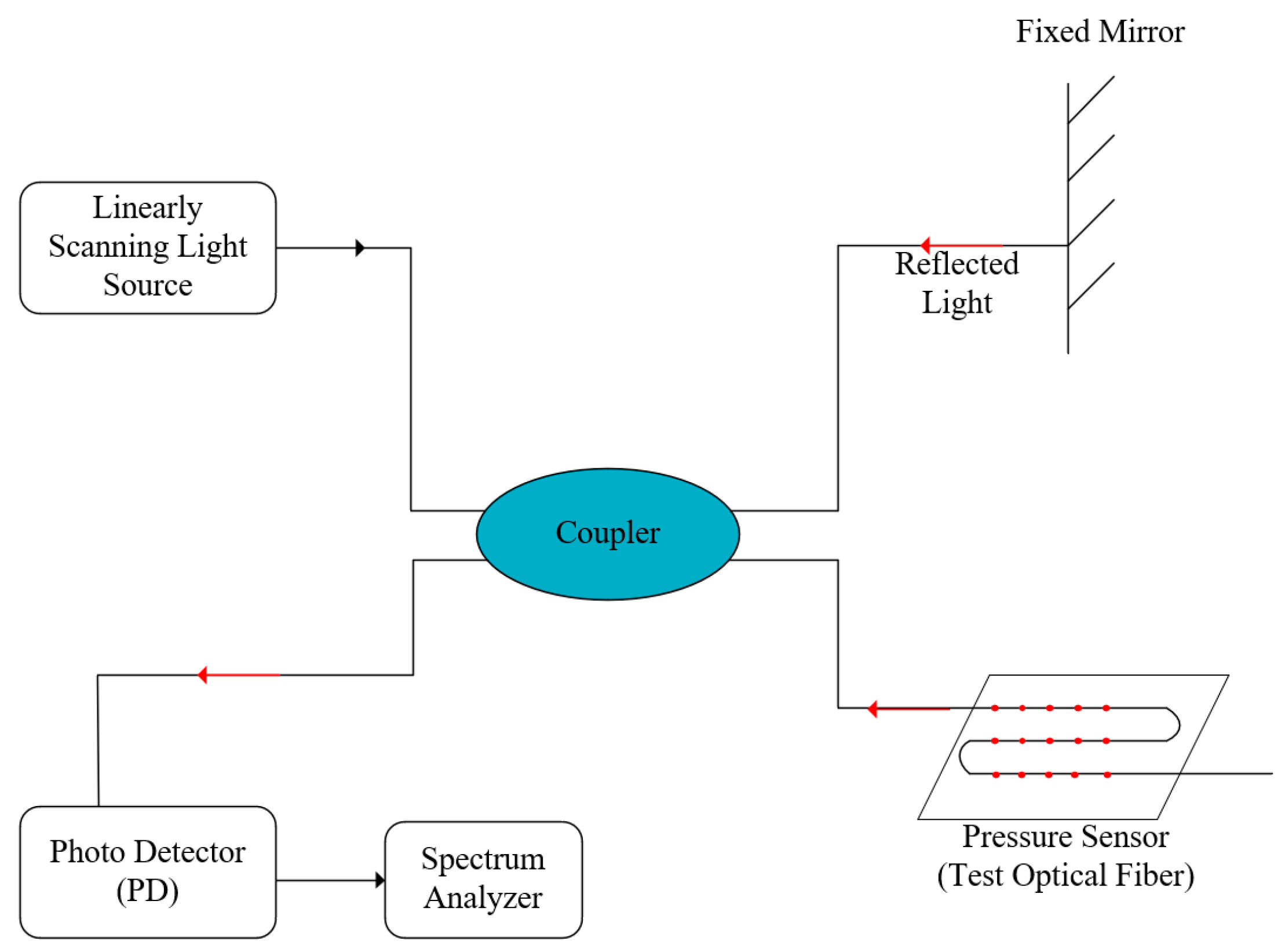
2.2. Theory of the Sensor Measurement
3. Experimental Testing and Results
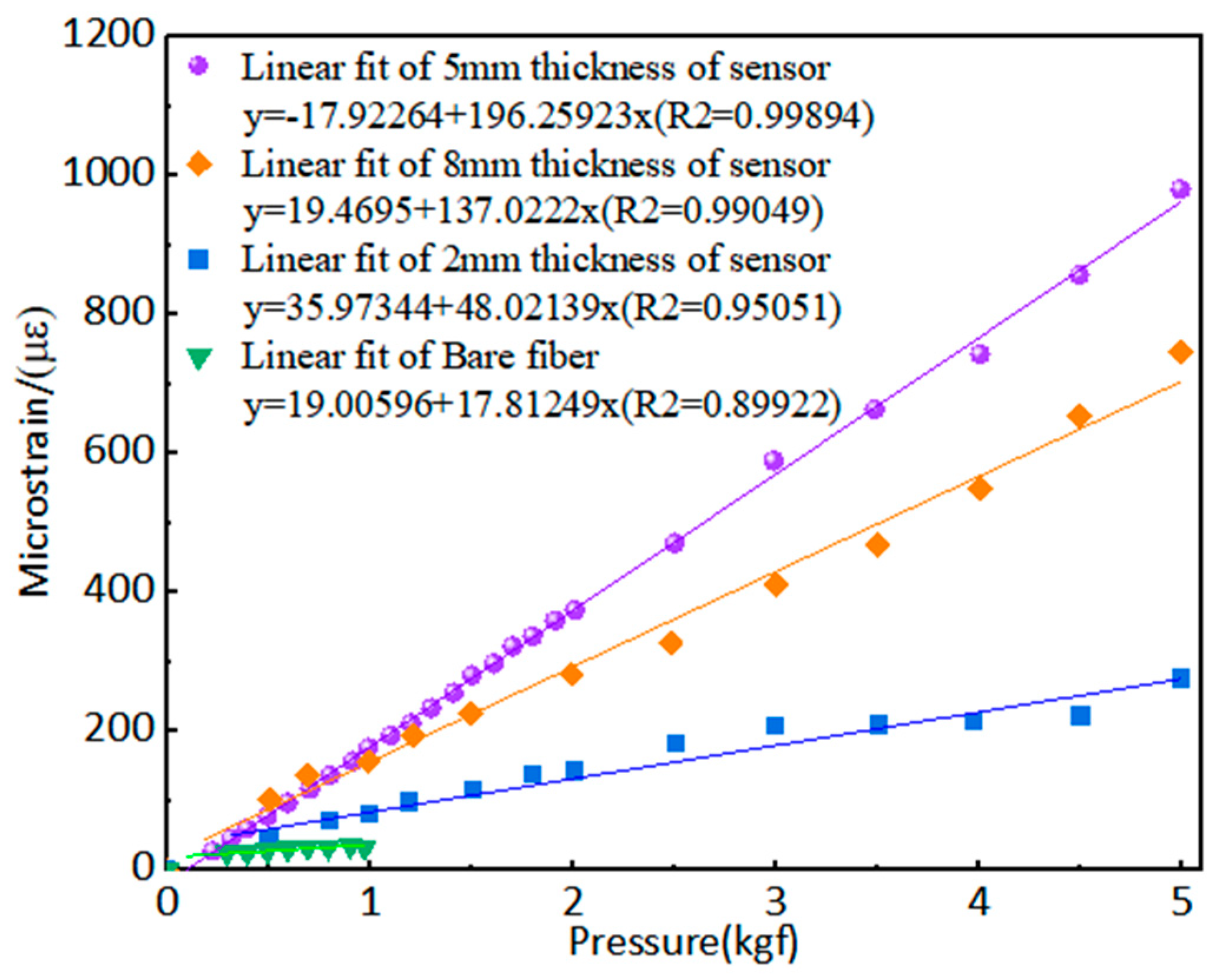
3.1. Effect of Different Thickness of PDMS Substrate on Sensor Sensitivity
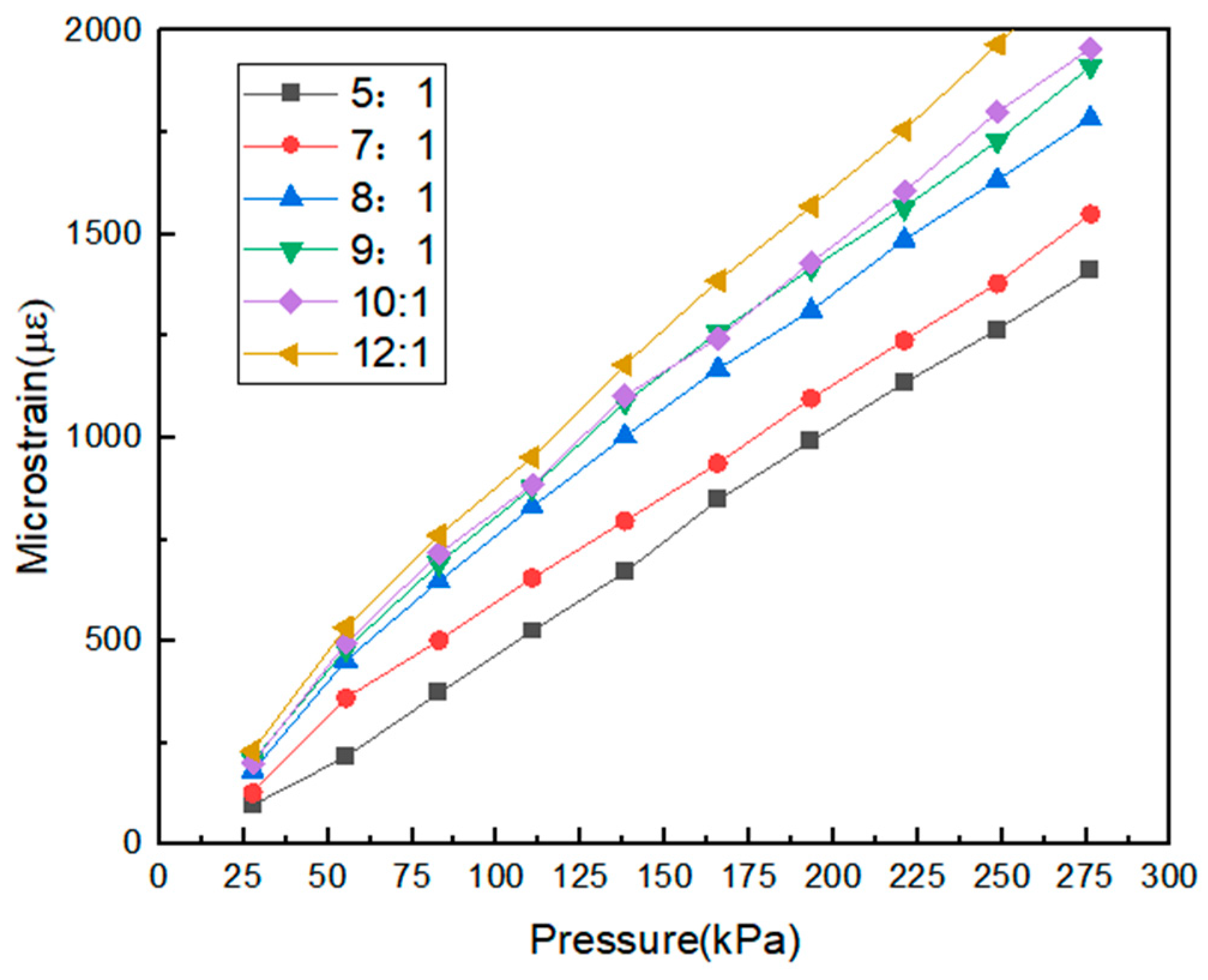
3.2. Effect of Different Ratio of PDMS on Sensor Sensitivity
3.3. Effect of Different Curing Time on Sensor Sensitivity
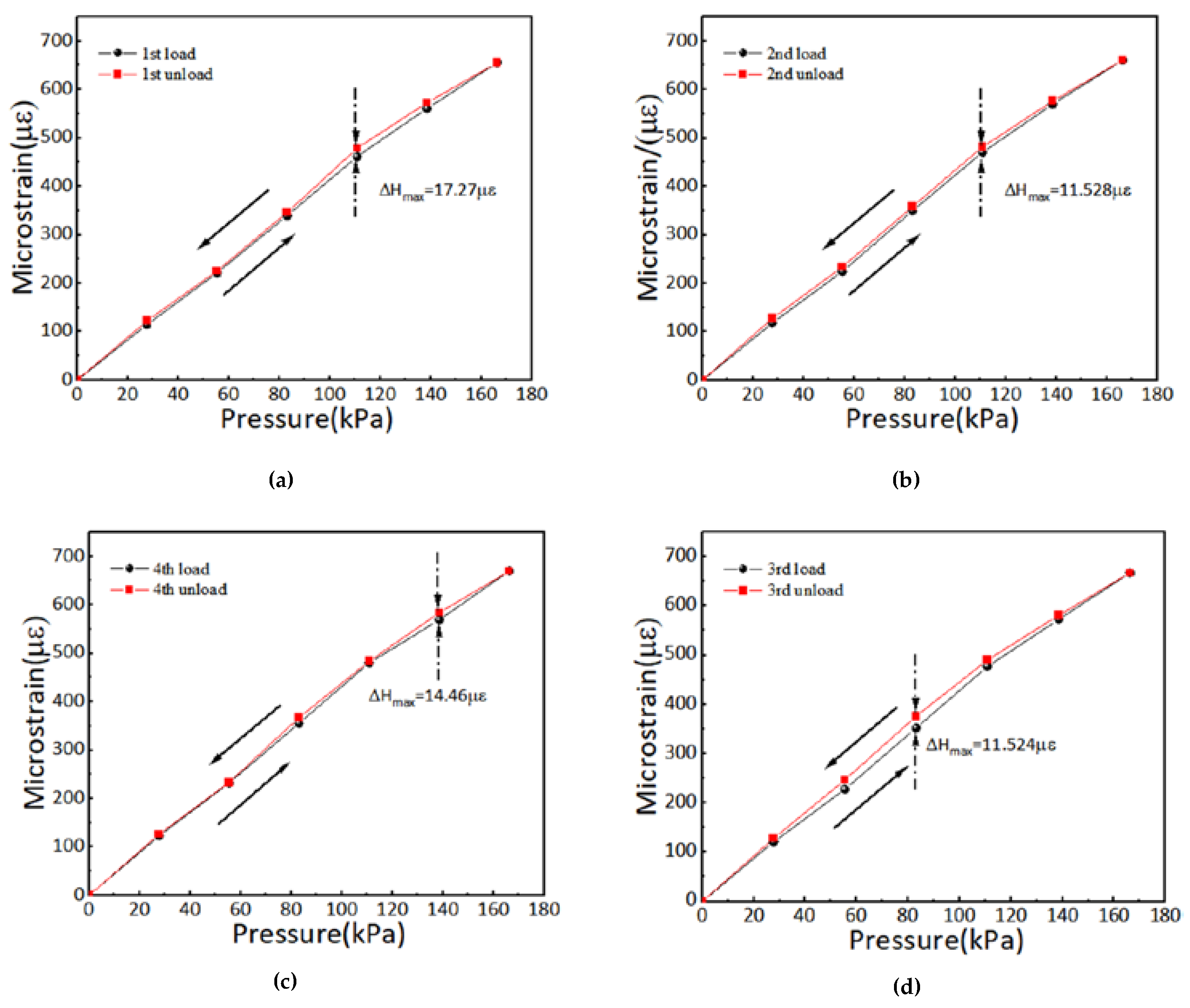
3.4. Reepeatability and Hysteresis of Flexible Fiber Optic Pressure Sensors
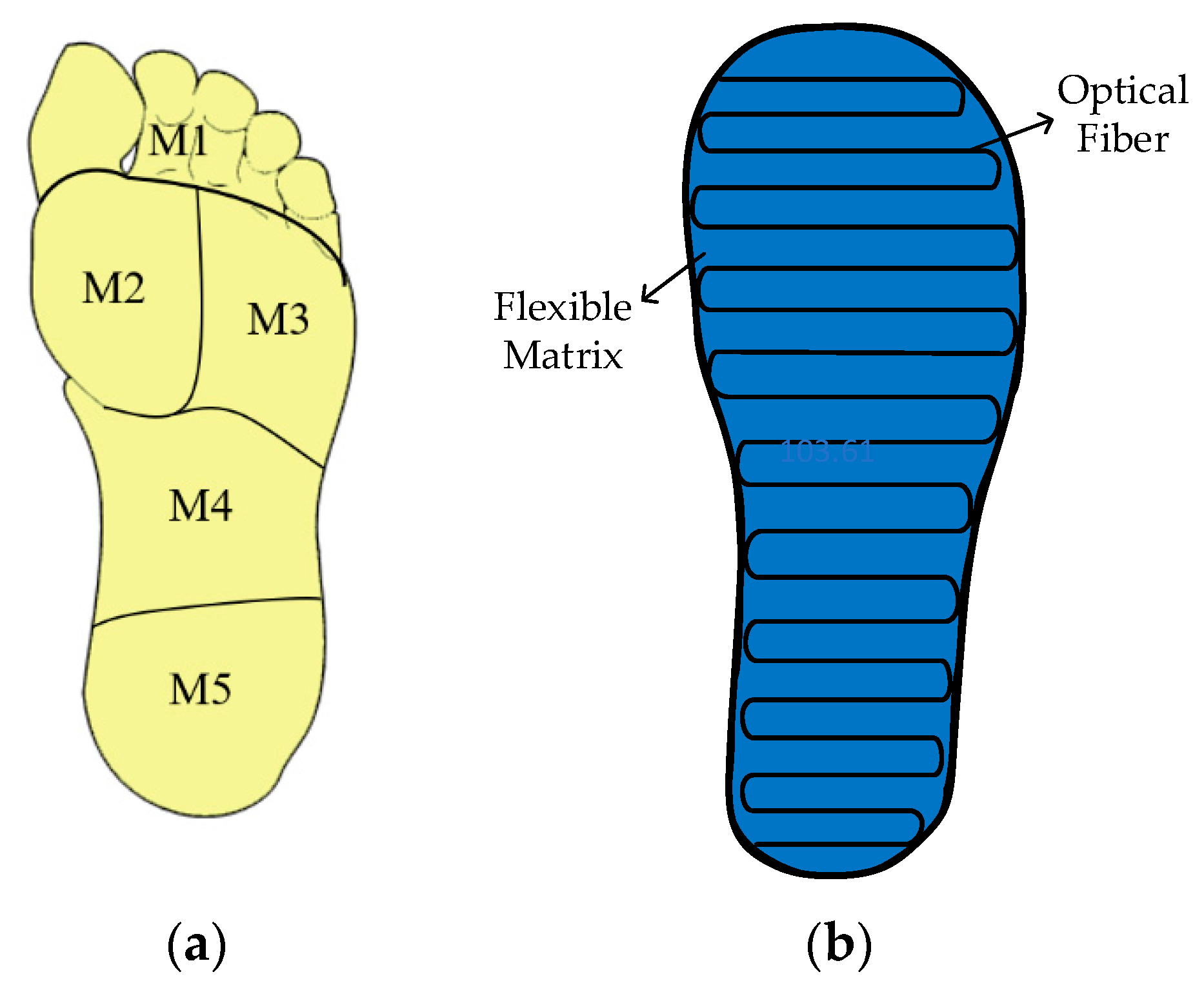
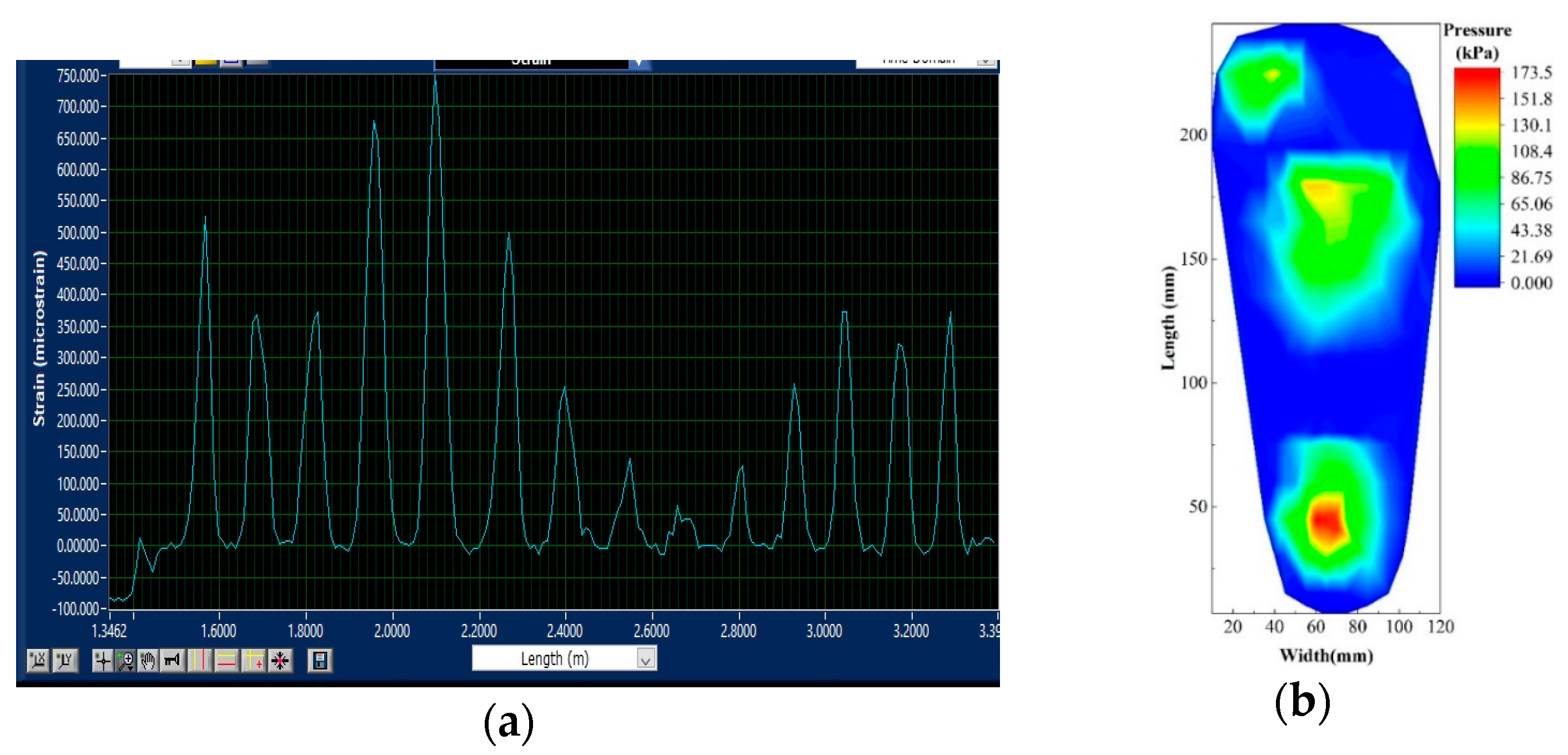
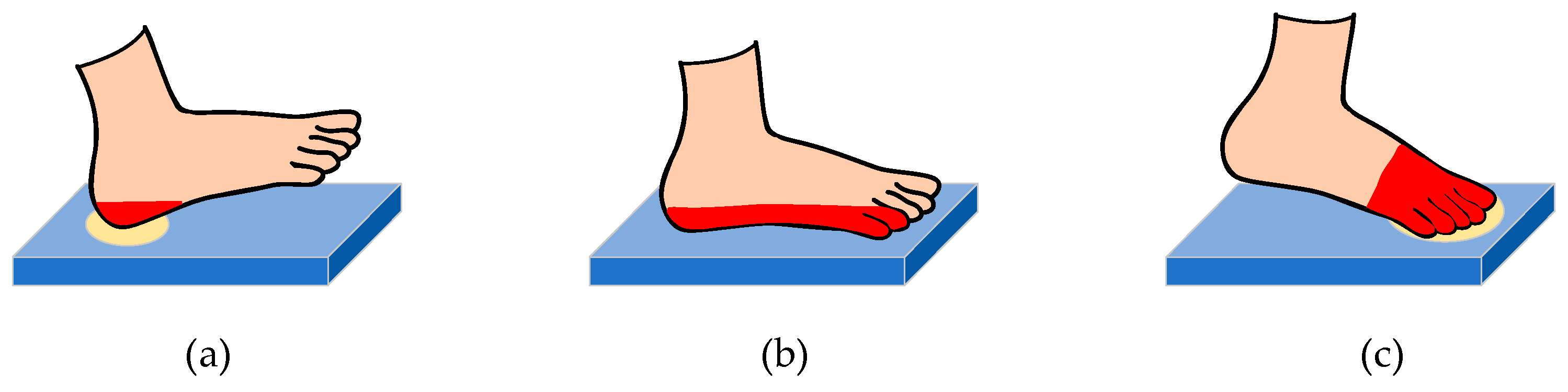
3.5. Plantar Distributed Pressure Measurement
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wan, Y.; Wang, Y.; Guo, C.F. Recent progresses on flexible tactile sensors. Mater. Today Phys. 2017, 1, 61–73. [Google Scholar] [CrossRef]
- Andrews, J.; Cardenas, J.; Mullett, J.; Franklin, A.D. Fully printed and flexible carbon nanotube transistors designed for environmental pressure sensing and aimed at smart tire applications. In Proceedings of the IEEE SENSORS, Glasgow, UK, 29 October–1 November 2017; pp. 1–3. [Google Scholar]
- Yang, T.; Xie, D.; Li, Z.; Zhu, H. Recent advances in wearable tactile sensors: Materials, sensing mechanisms, and device performance. Mater. Sci. Eng. R: Rep. 2017, 115, 1–37. [Google Scholar] [CrossRef]
- Schwartz, G.; Tee, B.C.-K.; Mei, J.; Appleton, A.L.; Kim, D.H.; Wang, H.; Bao, Z. Flexible polymer transistors with high pressure sensitivity for application in electronic skin and health monitoring. Nat. Commun. 2013, 4, 1859. [Google Scholar] [CrossRef]
- Wang, K.; Lou, Z.; Wang, L.; Zhao, L.; Zhao, S.; Wang, D.; Han, W.; Jiang, K.; Shen, G. Bioinspired Interlocked Structure-Induced High Deformability for Two-Dimensional Titanium Carbide (MXene)/Natural Microcapsule-Based Flexible Pressure Sensors. ACS Nano 2019, 13, 9139–9147. [Google Scholar] [CrossRef]
- Qiu, Z.; Wan, Y.; Zhou, W.; Yang, J.; Yang, J.; Huang, J.; Zhang, J.; Liu, Q.; Huang, S.; Bai, N.; et al. Ionic Skin with Biomimetic Dielectric Layer Templated from Calathea Zebrine Leaf. Adv. Funct. Mater. 2018, 28, 1802343. [Google Scholar] [CrossRef]
- Chen, J.; Zheng, J.; Gao, Q.; Zhang, J.; Zhang, J.; Omisore, O.M.; Wang, L.; Li, H. Polydimethylsiloxane (PDMS)-Based Flexible Resistive Strain Sensors for Wearable Applications. Appl. Sci. 2018, 8, 345. [Google Scholar] [CrossRef]
- Zhang, Y.; Ye, J.; Lin, Z.; Huang, S.; Wang, H.; Wu, H. A Piezoresistive Tactile Sensor for a Large Area Employing Neural Network. Sensors 2018, 19, 27. [Google Scholar] [CrossRef]
- Larson, C.; Peele, B.; Li, S.; Robinson, S.; Totaro, M.; Beccai, L.; Mazzolai, B.; Shepherd, R. Highly stretchable electroluminescent skin for optical signaling and tactile sensing. Science 2016, 351, 1071–1074. [Google Scholar] [CrossRef]
- Lee, H.; Park, K.; Kim, Y.; Kim, J. Durable and Repairable Soft Tactile Skin for Physical Human Robot Interaction. In Proceedings of the Companion of the ACM/IEEE International Conference on Human-Robot Interaction, Vienna, Austria, 6–9 March 2017; ACM: New York, NY, USA, 2017; pp. 183–184. [Google Scholar]
- Park, Y.-L.; Ryu, S.C.; Black, R.J.; Chau, K.K.; Moslehi, B.; Cutkosky, M.R. Exoskeletal Force-Sensing End-Effectors with Embedded Optical Fiber-Bragg-Grating Sensors. IEEE Trans. Robot. 2009, 25, 1319–1331. [Google Scholar] [CrossRef]
- Saccomandi, P.; Caponero, M.; Polimadei, A.; Francomano, M.; Formica, D.; Accoto, D.; Tamilia, E.; Taffoni, F.; Taffoni, F.; Di Pino, G.; et al. An MR-compatible force sensor based on FBG technology for biomedical application. In Proceedings of the 2014 36th Annual International Conference of the IEEE Engineering in Medicine and Biology Society, Chicago, IL, USA, 26–30 August 2014; pp. 5731–5734. [Google Scholar]
- Chen, L.; Smith, M.R.; Hui, Y. Comparing the performance of two feedforward neural network training algorithms in MRI: Reconstruction. In Proceedings of the 1996 Canadian Conference on Electrical and Computer Engineering, Calgary, AB, Canada, 26–29 May 1996; Volume 1, pp. 362–364. [Google Scholar]
- Mingxin, J.I.A. Research on fiber-optic tactile sensors and their performance. J. Harbin Eng. Univ. 2002, 2, 78–81. [Google Scholar]
- Li, M. Research on Pressure Sensing Technology Based on Multimode Fiber Spot Analysis. Master’s Thesis, Beijing Jiaotong University, Beijing, China, 2020. [Google Scholar]
- Yu, X.; Zhang, S.; Olivo, M.; Li, N. Micro- and nano-fiber probes for optical sensing, imaging, and stimulation in biomedical applications. Photonics Res. 2020, 8, 1703–1724. [Google Scholar] [CrossRef]
- Giurgiutiu, V. Fiber-Optic Sensors. In Structural Health Monitoring of Aerospace Composites; Elsevier: Amsterdam, The Netherlands, 2016; pp. 249–296. [Google Scholar]
- Gianluca, G.; Mario, S.; Pietro, F.; Chehura, E.; Tatam, R.P.; Gangopadhyay, T.K.; Ballard, N.; Paz-Soldan, D.; Barnes, J.A.; Loock, H.P.; et al. Optical fiber sensing based on reflection laser spectroscopy. Sensors 2010, 10, 1823–1845. [Google Scholar] [CrossRef] [PubMed]
- Xu, Z.; Kai, C. Research on OFDR Pressure Sensor Based on PDMS. In Advances in Precision Instruments and Optical Engineering; Liu, G., Cen, F., Eds.; Springer Proceedings in Physics; Springer: Singapore, 2022; Volume 270. [Google Scholar]
- Yao, N.; Wang, X.; Ma, S.; Song, X.; Wang, S.; Shi, Z.; Pan, J.; Wang, S.; Xiao, J.; Liu, H.; et al. Single optical microfiber enabled tactile sensor for simultaneous temperature and pressure measurement. Photon. Res. 2022, 10, 2040–2046. [Google Scholar] [CrossRef]
- Li, L.; Sheng, S.; Liu, Y.; Wen, J.; Song, C.; Chen, Z.; Xu, W.; Zhang, Z.; Fan, W.; Chen, C.; et al. Automatic and continuous blood pressure monitoring via an optical-fiber-sensor-assisted smartwatch. PhotoniX 2023, 4. [Google Scholar] [CrossRef]
- Hou, L.; Jiang, T.; Yu, T.; Cao, C.; Tu, X.; Zhang, J.; Pan, J.; Wang, S.; Zhou, N.; Yao, N.; et al. Tapered Optical Fiber Enabled Distributed Sensors with High Spatial Resolution by Deep Learning. Adv. Opt. Mater. 2024, 12, 2303118. [Google Scholar] [CrossRef]
- Domingues, M.F.; Rodriguez, C.A.; Martins, J.; Tavares, C.; Marques, C.; Alberto, N.; André, P.; Antunes, P. Cost-effective optical fiber pressure sensor based on intrinsic Fabry-Perot interferometric micro-cavities—ScienceDirect. Opt. Fiber Technol. 2018, 42, 56–62. [Google Scholar] [CrossRef]
- Huang, R.; Lele, S.P.; Anand, L. Non-linear mechanical behavior of polydimethylsiloxane (PDMS): Application to the manufacture of microfluidic devices. Innov. Manuf. Syst. Technol. IMST 2004. Available online: http://hdl.handle.net/1721.1/3913 (accessed on 15 August 2024).
- Cui, K.; Hong, Y.; Sui, D.; Wenyi, L.; Huixin, Z. A Lossless Fiber Pressure Sensor Based on PDMS. IEEE Access 2020, 8, 189036–189042. [Google Scholar]
- Zhao, L.; Tang, F.; You, R.; Li, H.-N. A silicone rubber packaged distributed optical fiber sensing tape for strain-crack monitoring based on OFDR technique. Sens. Actuators A Phys. 2024, 372, 115383. [Google Scholar] [CrossRef]
- Huang, J.; Zhou, Z.; Wen, X.; Zhang, D. A diaphragm-type fiber Bragg grating pressure sensor with temperature compensation. Measurement 2013, 46, 1041–1046. [Google Scholar] [CrossRef]
- Li, J.; Gan, J.; Zhang, Z.; Heng, X.; Yang, C.; Qian, Q.; Xu, S.; Yang, Z. High spatial resolution distributed fiber strain sensor based on phase-OFDR. Opt. Express 2017, 25, 27913–27922. [Google Scholar] [CrossRef] [PubMed]
- Froggatt, M.; Gifford, D. Rayleigh Backscattering Signatures of Optical Fibers—Their Properties and Applications. In Proceedings of the Optical Fiber Communication Conference, Anaheim, CA, USA, 17–21 March 2013; pp. 1–3. [Google Scholar]
- Wang, C.Q.; Wu, L.W.; Liu, Y. Research progress of distributed optical fiber temperature and strain sensing system. Transducer Microsyst. Technol. 2017, 36, 1–4. [Google Scholar]
- Luo, M.; Liu, J.; Tang, C.; Wang, X.; Lan, T.; Kan, B. 0.5 mm spatial resolution distributed fiber temperature and strain sensor with position-deviation compensation based on OFDR. Opt. Express 2019, 27, 35823–35829. [Google Scholar] [CrossRef] [PubMed]
- Wada, D.; Igawa, H.; Murayama, H. Simultaneous distributed measurement of the strain and temperature for a four-point bending test using polarization-maintaining fiber Bragg grating interrogated by optical frequency domain reflectometry. Measurement 2016, 94, 745–752. [Google Scholar] [CrossRef]
- Yang, D.; Liu, T.; Ding, Z.; Han, Q.; Liu, K.; Jiang, J.; Chen, Q.; Feng, B. Cryogenic Temperature Measurement Using Rayleigh Backscattering Spectra Shift by OFDR. IEEE Photonics Technol. Lett. 2014, 26, 1150–1153. [Google Scholar] [CrossRef]





| Name | Radius/(μm) | Elastic Modulus/(Mpa) | Poisson’s Ratio/(V) | Coefficient of Thermal Expansion/() |
|---|---|---|---|---|
| Core | 4.5 | 72,000 | 0.17 | 0.55 |
| Cladding | 62.5 | 7200 | 0.17 | 0.55 |
| Coating layer | 125 | 1200 | 0.4995 | 150 |
| Parameter | Value |
|---|---|
| Height (cm) | 161 |
| Weight (kg) | 53 ± 2 |
| Foot length (mm) | 245 ± 5 |
| Foot width (mm) | 95 ± 5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, H.; Wu, J.; Gao, C. Research on the Fabrication and Parameters of a Flexible Fiber Optic Pressure Sensor with High Sensitivity. Photonics 2024, 11, 919. https://doi.org/10.3390/photonics11100919
Zhang H, Wu J, Gao C. Research on the Fabrication and Parameters of a Flexible Fiber Optic Pressure Sensor with High Sensitivity. Photonics. 2024; 11(10):919. https://doi.org/10.3390/photonics11100919
Chicago/Turabian StyleZhang, Huixin, Jing Wu, and Chencheng Gao. 2024. "Research on the Fabrication and Parameters of a Flexible Fiber Optic Pressure Sensor with High Sensitivity" Photonics 11, no. 10: 919. https://doi.org/10.3390/photonics11100919
APA StyleZhang, H., Wu, J., & Gao, C. (2024). Research on the Fabrication and Parameters of a Flexible Fiber Optic Pressure Sensor with High Sensitivity. Photonics, 11(10), 919. https://doi.org/10.3390/photonics11100919




