Tilted Wire Metamaterials Enabling Ultra-Broadband Absorption from Middle to Very Long Infrared Regimes
Abstract
1. Introduction
2. Structural Design and Simulation Methods
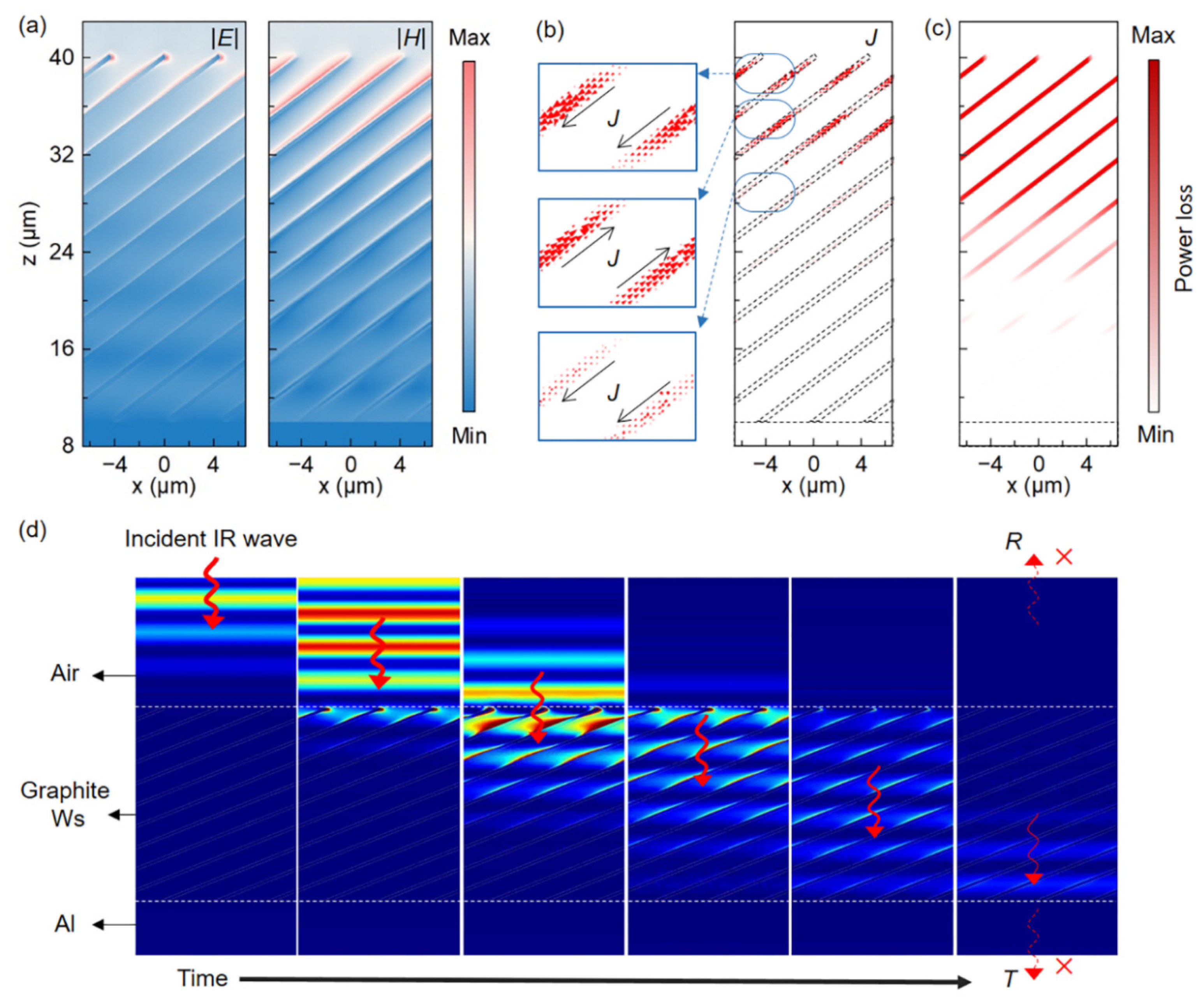
3. Results and Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhao, X.; Li, T.; Xie, H.; Liu, H.; Wang, L.; Qu, Y.; Li, S.C.; Liu, S.; Brozena, A.H.; Yu, Z.; et al. A solution-processed radiative cooling glass. Science 2023, 382, 684–691. [Google Scholar] [CrossRef] [PubMed]
- Huang, G.; Yengannagari, A.R.; Matsumori, K.; Patel, P.; Datla, A.; Trindade, K.; Amarsanaa, E.; Zhao, T.; Köhler, U.; Busko, D.; et al. Radiative cooling and indoor light management enabled by a transparent and self-cleaning polymer-based metamaterial. Nat. Commun. 2024, 15, 3798. [Google Scholar] [CrossRef] [PubMed]
- Chen, Z.; Zhu, L.; Li, W.; Fan, S. Simultaneously and Synergistically Harvest Energy from the Sun and Outer Space. Joule 2019, 3, 101–110. [Google Scholar] [CrossRef]
- Raman, A.P.; Li, W.; Fan, S.H. Generating Light from Darkness. Joule 2019, 3, 2679–2686. [Google Scholar] [CrossRef]
- Suen, J.Y.; Fan, K.B.; Montoya, J.; Bingham, C.; Stenger, V.; Sriram, S.; Padilla, W.J. Multifunctional metamaterial pyroelectric infrared detectors. Optica 2017, 4, 276–279. [Google Scholar] [CrossRef]
- Xue, X.M.; Hao, Q.; Chen, M.L. Very long wave infrared quantum dot photodetector up to 18 μm. Light-Sci. Appl. 2024, 13, 89. [Google Scholar] [CrossRef]
- Maier, T.; Brueckl, H. Multispectral microbolometers for the midinfrared. Opt. Lett. 2010, 35, 3766–3768. [Google Scholar] [CrossRef]
- Maier, T.; Brückl, H. Wavelength-tunable microbolometers with metamaterial absorbers. Opt. Lett. 2009, 34, 3012–3014. [Google Scholar] [CrossRef]
- Cortés, E.; Wendisch, F.J.; Sortino, L.; Mancini, A.; Ezendam, S.; Saris, S.; de S. Menezes, L.; Tittl, A.; Ren, H.; Maier, S.A. Optical Metasurfaces for Energy Conversion. Chem. Rev. 2022, 122, 15082–15176. [Google Scholar] [CrossRef]
- Liu, X.; Wang, P.; Xiao, C.; Fu, L.; Zhou, H.; Fan, T.; Zhang, D. A Bioinspired Bilevel Metamaterial for Multispectral Manipulation toward Visible, Multi-Wavelength Detection Lasers and Mid-Infrared Selective Radiation. Adv. Mater. 2023, 35, 2302844. [Google Scholar] [CrossRef]
- Zhai, Y.; Ma, Y.; David, S.N.; Zhao, D.; Lou, R.; Tan, G.; Yang, R.; Yin, X. Scalable-manufactured randomized glass-polymer hybrid metamaterial for daytime radiative cooling. Science 2017, 355, 1062–1066. [Google Scholar] [CrossRef] [PubMed]
- Kim, S.; Jang, M.S.; Brar, V.W.; Mauser, K.W.; Kim, L.; Atwater, H.A. Electronically Tunable Perfect Absorption in Graphene. Nano Lett. 2018, 18, 971–979. [Google Scholar] [CrossRef] [PubMed]
- Nefedov, I.S.; Valagiannopoulos, C.A.; Melnikov, L.A. Perfect absorption in graphene multilayers. J. Opt. 2013, 15, 114003. [Google Scholar] [CrossRef]
- Haddadan, F.; Soroosh, M.; Alaei-Sheini, N. Designing an electro-optical encoder based on photonic crystals using the graphene–Al2O3 stacks. Appl. Opt. 2020, 59, 2179–2185. [Google Scholar] [CrossRef] [PubMed]
- Soroosh, M.; Shahbaznia, M.; Maleki, M.J.; Ganji, J. Designing a compact photonic crystal decoder using graphene-SiO2 stack. Opt. Quantum Electron. 2024, 56, 828. [Google Scholar] [CrossRef]
- Maas, R.; Parsons, J.; Engheta, N.; Polman, A. Experimental realization of an epsilon-near-zero metamaterial at visible wavelengths. Nat. Photonics 2013, 7, 907–912. [Google Scholar] [CrossRef]
- Tittl, A.; Harats, M.G.; Walter, R.; Yin, X.; Schaferling, M.; Liu, N.; Rapaport, R.; Giessen, H. Quantitative angle-resolved small-spot reflectance measurements on plasmonic perfect absorbers: Impedance matching and disorder effects. ACS Nano 2014, 8, 10885–10892. [Google Scholar] [CrossRef]
- Yun, S.; Jiang, Z.H.; Xu, Q.; Liu, Z.; Werner, D.H.; Mayer, T.S. Low-loss impedance-matched optical metamaterials with zero-phase delay. ACS Nano 2012, 6, 4475–4482. [Google Scholar] [CrossRef]
- Xu, J.; Mandal, J.; Raman, A.P. Broadband directional control of thermal emission. Science 2021, 372, 393–397. [Google Scholar] [CrossRef]
- McSherry, S.; Lenert, A. Design of a gradient epsilon-near-zero refractory metamaterial with temperature-insensitive broadband directional emission. Appl. Phys. Lett. 2022, 121, 191702. [Google Scholar] [CrossRef]
- Hendrickson, J.R.; Vangala, S.; Dass, C.; Gibson, R.; Goldsmith, J.; Leedy, K.; Walker, D.E.; Cleary, J.W.; Kim, W.; Guo, J. Coupling of Epsilon-Near-Zero Mode to Gap Plasmon Mode for Flat-Top Wideband Perfect Light Absorption. ACS Photonics 2018, 5, 776–781. [Google Scholar] [CrossRef]
- Ji, C.; Lee, K.T.; Xu, T.; Zhou, J.; Park, H.J.; Guo, L.J. Engineering Light at the Nanoscale: Structural Color Filters and Broadband Perfect Absorbers. Adv. Opt. Mater. 2017, 5, 1700368. [Google Scholar] [CrossRef]
- Taylor, S.; Yang, Y.; Wang, L. Vanadium dioxide based Fabry-Perot emitter for dynamic radiative cooling applications. J. Quant. Spectrosc. Ra. 2017, 197, 76–83. [Google Scholar] [CrossRef]
- Sun, K.; Riedel, C.A.; Wang, Y.; Urbani, A.; Simeoni, M.; Mengali, S.; Zalkovskij, M.; Bilenberg, B.; de Groot, C.H.; Muskens, O.L. Metasurface Optical Solar Reflectors Using AZO Transparent Conducting Oxides for Radiative Cooling of Spacecraft. ACS Photonics 2017, 5, 495–501. [Google Scholar] [CrossRef]
- Zhou, Y.; Qin, Z.; Liang, Z.; Meng, D.; Xu, H.; Smith, D.R.; Liu, Y. Ultra-broadband metamaterial absorbers from long to very long infrared regime. Light Sci. Appl. 2021, 10, 138. [Google Scholar] [CrossRef]
- Lei, L.; Li, S.; Huang, H.; Tao, K.; Xu, P. Ultra-broadband absorber from visible to near-infrared using plasmonic metamaterial. Opt. Express 2018, 26, 5686–5693. [Google Scholar] [CrossRef]
- Yu, P.; Besteiro, L.V.; Huang, Y.; Wu, J.; Fu, L.; Tan, H.H.; Jagadish, C.; Wiederrecht, G.P.; Govorov, A.O.; Wang, Z. Broadband Metamaterial Absorbers. Adv. Opt. Mater. 2018, 7, 1800995. [Google Scholar] [CrossRef]
- Zhou, Y.; Liang, Z.; Qin, Z.; Hou, E.; Shi, X.; Zhang, Y.; Xiong, Y.; Tang, Y.; Fan, Y.; Yang, F.; et al. Small–sized long wavelength infrared absorber with perfect ultra–broadband absorptivity. Opt. Express 2020, 28, 1279–1290. [Google Scholar] [CrossRef]
- Mayer, A.; Bi, H.; Griesse-Nascimento, S.; Hackens, B.; Loicq, J.; Mazur, E.; Deparis, O.; Lobet, M. Genetic-algorithm-aided ultra-broadband perfect absorbers using plasmonic metamaterials. Opt. Express 2022, 30, 1167–1181. [Google Scholar] [CrossRef]
- Chen, Z.; Cai, P.; Wen, Q.; Chen, H.; Tang, Y.; Yi, Z.; Wei, K.; Li, G.; Tang, B.; Yi, Y. Graphene Multi-Frequency Broadband and Ultra-Broadband Terahertz Absorber Based on Surface Plasmon Resonance. Electronics 2023, 12, 2655. [Google Scholar] [CrossRef]
- Jin, Y.; Liang, J.; Wu, S.; Zhang, Y.; Zhou, L.; Wang, Q.J.; Liu, H.; Zhu, J. Electrical Dynamic Switching of Magnetic Plasmon Resonance Based on Selective Lithium Deposition. Adv. Mater. 2020, 32, 2000058. [Google Scholar] [CrossRef] [PubMed]
- Long, L.; Taylor, S.; Wang, L. Enhanced Infrared Emission by Thermally Switching the Excitation of Magnetic Polariton with Scalable Microstructured VO2 Metasurfaces. ACS Photonics 2020, 7, 2219–2227. [Google Scholar] [CrossRef]
- Lim, J.-S.; Lee, N.; Kim, T.; Chang, I.; Nam, J.; Cho, H.H. Multiresonant Selective Emitter with Enhanced Thermal Management for Infrared Camouflage. ACS Appl. Mater. 2024, 16, 15416–15425. [Google Scholar] [CrossRef] [PubMed]
- Guo, W.; Liu, Y.; Han, T. Ultra-broadband infrared metasurface absorber. Opt. Express 2016, 24, 20586–20592. [Google Scholar] [CrossRef] [PubMed]
- Yue, S.; Hou, M.J.; Wang, R.; Guo, H.F.; Hou, Y.; Li, M.; Zhang, Z.; Wang, Y.; Zhang, Z.C. Ultra-broadband metamaterial absorber from ultraviolet to long-wave infrared based on CMOS-compatible materials. Opt. Express 2020, 28, 31844–31861. [Google Scholar] [CrossRef]
- Shrestha, S.; Wang, Y.; Overvig, A.C.; Lu, M.; Stein, A.; Negro, L.D.; Yu, N. Indium Tin Oxide Broadband Metasurface Absorber. ACS Photonics 2018, 5, 3526–3533. [Google Scholar] [CrossRef]
- Zhou, Y.; Liang, Z.Z.; Qin, Z.; Shi, X.Y.; Meng, D.J.; Zhang, L.C.; Wang, X.Y. Broadband long wavelength infrared metamaterial absorbers. Results Phys. 2020, 19, 103566. [Google Scholar] [CrossRef]
- Zhu, Y.; Tang, B.; Jiang, C. Tunable ultra-broadband anisotropic absorbers based on multi-layer black phosphorus ribbons. Appl. Phys. Express 2019, 12, 032009. [Google Scholar] [CrossRef]
- Sang, T.; Qi, H.L.; Wang, X.; Yin, X.; Li, G.Q.; Niu, X.S.; Ma, B.; Jiao, H.F. Ultrabroadband Absorption Enhancement via Hybridization of Localized and Propagating Surface Plasmons. Nanomaterials 2020, 10, 1625. [Google Scholar] [CrossRef]
- Kenney, M.; Grant, J.; Shah, Y.D.; Escorcia-Carranza, I.; Humphreys, M.; Cumming, D.R.S. Octave-Spanning Broadband Absorption of Terahertz Light Using Metasurface Fractal-Cross Absorbers. ACS Photonics 2017, 4, 2604–2612. [Google Scholar] [CrossRef]
- Wang, B.X.; Xu, C.; Duan, G.; Xu, W.; Pi, F. Review of Broadband Metamaterial Absorbers: From Principles, Design Strategies, and Tunable Properties to Functional Applications. Adv. Funct. Mater. 2023, 33, 2213818. [Google Scholar] [CrossRef]
- Kan, Y.H.; Zhao, C.Y.; Fang, X.; Wang, B.X. Designing ultrabroadband absorbers based on Bloch theorem and optical topological transition. Opt. Lett. 2017, 42, 1879–1882. [Google Scholar] [CrossRef] [PubMed]
- West, P.R.; Ishii, S.; Naik, G.V.; Emani, N.K.; Shalaev, V.M.; Boltasseva, A. Searching for better plasmonic materials. Laser. Photonics Rev. 2010, 4, 795–808. [Google Scholar] [CrossRef]
- Naik, G.V.; Shalaev, V.M.; Boltasseva, A. Alternative Plasmonic Materials: Beyond Gold and Silver. Adv. Mater. 2013, 25, 3264–3294. [Google Scholar] [CrossRef]
- Yildirim, D.U.; Ghobadi, A.; Soydan, M.C.; Atesal, O.; Toprak, A.; Caliskan, M.D.; Ozbay, E. Disordered and Densely Packed ITO Nanorods as an Excellent Lithography-Free Optical Solar Reflector Metasurface. ACS Photonics 2019, 6, 1812–1822. [Google Scholar] [CrossRef]
- Borah, D.; Bhattacharyya, N.S. Design and Development of Expanded Graphite-Based Non-metallic and Flexible Metamaterial Absorber for X-band Applications. J. Electron. Mater. 2016, 46, 226–232. [Google Scholar] [CrossRef]
- Makhloufi, M.; Salah, H. Graphite based metamaterial for high frequency applications. J. Magn. Magn. Mater. 2022, 557, 169410. [Google Scholar] [CrossRef]
- Rani, N.; Saha, S. Graphite based metal-free and polarization-insensitive multiband THz absorber with wide incident angle. Optik 2022, 266, 169601. [Google Scholar] [CrossRef]
- Singhal, S. Wide angle insensitive and polarization independent graphite based superwideband absorber. Opt. Quant. Electron. 2022, 54, 671. [Google Scholar] [CrossRef]
- Chen, X.; Jia, X.; Wu, Z.; Tang, Z.; Zeng, Y.; Wang, X.; Fu, X.; Zou, Y. A Graphite-Based Metamaterial Microwave Absorber. IEEE Antenn. Wirel. Pract. 2019, 18, 1016–1020. [Google Scholar] [CrossRef]
- Simovski, C.R.; Belov, P.A.; Atrashchenko, A.V.; Kivshar, Y.S. Wire metamaterials: Physics and applications. Adv. Mater. 2012, 24, 4229–4248. [Google Scholar] [CrossRef] [PubMed]
- Wurtz, G.A.; Pollard, R.; Hendren, W.; Wiederrecht, G.P.; Gosztola, D.J.; Podolskiy, V.A.; Zayats, A.V. Designed ultrafast optical nonlinearity in a plasmonic nanorod metamaterial enhanced by nonlocality. Nat. Nanotechnol. 2011, 6, 107–111. [Google Scholar] [CrossRef] [PubMed]
- Gao, W.L.; Fang, F.Z.; Liu, Y.M.; Zhang, S. Chiral surface waves supported by biaxial hyperbolic metamaterials. Light-Sci. Appl. 2015, 4, e328. [Google Scholar] [CrossRef]
- Huang, J.; Wang, X.; Hogan, N.L.; Wu, S.; Lu, P.; Fan, Z.; Dai, Y.; Zeng, B.; Starko-Bowes, R.; Jian, J.; et al. Nanoscale Artificial Plasmonic Lattice in Self-Assembled Vertically Aligned Nitride-Metal Hybrid Metamaterials. Adv. Sci. 2018, 5, 1800416. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.; Wang, H.; Jian, J.; Rutherford, B.X.; Gao, X.; Xu, X.; Zhang, X.; Wang, H. Metal-Free Oxide-Nitride Heterostructure as a Tunable Hyperbolic Metamaterial Platform. Nano Lett. 2020, 20, 6614–6622. [Google Scholar] [CrossRef]
- Hashemi, S.M.; Nefedov, I.S. Wideband perfect absorption in arrays of tilted carbon nanotubes. Phys. Rev. B 2012, 86, 195411. [Google Scholar] [CrossRef]
- Nefedov, I.S.; Valagiannopoulos, C.A.; Hashemi, S.M.; Nefedov, E.I. Total absorption in asymmetric hyperbolic media. Sci. Rep. 2013, 3, 2662. [Google Scholar] [CrossRef]
- Ma, Z.; Fan, H.; Zhou, H.; Huang, M.; Luo, J. Broadband perfect transparency-to-absorption switching in tilted anisotropic metamaterials based on the anomalous Brewster effect. Opt. Express 2021, 29, 39186–39199. [Google Scholar] [CrossRef]
- Zhang, H.; Ly, K.C.S.; Liu, X.; Chen, Z.; Yan, M.; Wu, Z.; Wang, X.; Zheng, Y.; Zhou, H.; Fan, T. Biologically inspired flexible photonic films for efficient passive radiative cooling. Proc. Natl. Acad. Sci. USA 2020, 117, 14657–14666. [Google Scholar] [CrossRef]
- Fan, S.H.; Li, W. Photonics and thermodynamics concepts in radiative cooling. Nat. Photonics 2022, 16, 182–190. [Google Scholar] [CrossRef]
- Wang, Y.; Li, X.; Wu, S.; Hu, C.; Liu, Y. Design of metamaterial perfect absorbers in the long-wave infrared region. Phys. Chem. Chem. Phys. 2024, 26, 551–557. [Google Scholar] [CrossRef] [PubMed]
- Zhao, D.; Aili, A.; Zhai, Y.; Xu, S.; Tan, G.; Yin, X.; Yang, R. Radiative sky cooling: Fundamental principles, materials, and applications. Appl. Phys. Rev. 2019, 6, 021306. [Google Scholar] [CrossRef]
- Wang, S.; Jiang, T.; Meng, Y.; Yang, R.; Tan, G.; Long, Y. Scalable thermochromic smart windows with passive radiative cooling regulation. Science 2021, 374, 1501–1504. [Google Scholar] [CrossRef] [PubMed]
- Wu, S.-H.; Chen, M.; Barako, M.T.; Jankovic, V.; Hon, P.W.C.; Sweatlock, L.A.; Povinelli, M.L. Thermal homeostasis using microstructured phase-change materials. Optica 2017, 4, 1390. [Google Scholar] [CrossRef]
- Liu, X.; Xiao, C.; Wang, P.; Yan, M.; Wang, H.; Xie, P.; Liu, G.; Zhou, H.; Zhang, D.; Fan, T. Biomimetic Photonic Multiform Composite for High-Performance Radiative Cooling. Adv. Opt. Mater. 2021, 9, 2101151. [Google Scholar] [CrossRef]
- Zhang, Q.; Lv, Y.; Wang, Y.; Yu, S.; Li, C.; Ma, R.; Chen, Y. Temperature-dependent dual-mode thermal management device with net zero energy for year-round energy saving. Nat. Commun. 2022, 13, 4874. [Google Scholar] [CrossRef]
- Levola, T.; Laakkonen, P. Replicated slanted gratings with a high refractive index material for in and outcoupling of light. Opt. Express 2007, 15, 2067–2074. [Google Scholar] [CrossRef]
- Jeong, H.E.; Lee, J.K.; Kim, H.N.; Moon, S.H.; Suh, K.Y. A nontransferring dry adhesive with hierarchical polymer nanohairs. Proc. Natl. Acad. Sci. USA 2009, 106, 5639–5644. [Google Scholar] [CrossRef]
- Kustandi, T.S.; Low, H.Y.; Teng, J.H.; Rodriguez, I.; Yin, R. Mimicking domino-like photonic nanostructures on butterfly wings. Small 2009, 5, 574–578. [Google Scholar] [CrossRef]
- Wang, Z.Z. Slanted Functional Gradient Micropillars for Optimal Bioinspired Dry Adhesion. Acs Nano 2018, 12, 1273–1284. [Google Scholar] [CrossRef]
- Chen, X.; Grzegorczyk, T.M.; Wu, B.I.; Pacheco, J., Jr.; Kong, J.A. Robust method to retrieve the constitutive effective parameters of metamaterials. Phys. Rev. E 2004, 70, 016608. [Google Scholar] [CrossRef]
- Wang, Y.; Li, H.; Ji, L.; Zhao, F.; Li, J.; Liu, X.; Wu, Y.; Lv, Y.; Fu, Y.; Zhou, H.; et al. Study on the microstructure and properties of graphite-like carbon films deposited by unbalanced magnetron sputtering. P. I. Mech. Eng. J. Eng. 2012, 226, 714–721. [Google Scholar] [CrossRef]
- Huang, M.; Zhang, X.; Ke, P.; Wang, A. Graphite-like carbon films by high power impulse magnetron sputtering. Appl. Surf. Sci. 2013, 283, 321–326. [Google Scholar] [CrossRef]
- Schelz, S.; Richmond, T.; Kania, P.; Oelhafen, P.; Guntherodt, H.J. Electronic and atomic structure of evaporated carbon films. Surf. Sci. 1996, 359, 227–236. [Google Scholar] [CrossRef]
- Blackstock, J.J.; Rostami, A.A.; Nowak, A.M.; McCreery, R.L.; Freeman, M.R.; McDermott, M.T. Ultraflat Carbon Film Electrodes Prepared by Electron Beam Evaporation. Anal. Chem. 2004, 76, 2544–2552. [Google Scholar] [CrossRef]
- Brus, V.V.; Ilashchuk, M.I.; Orletskyi, I.G.; Solovan, M.M.; Parkhomenko, G.P.; Babichuk, I.S.; Schopp, N.; Andrushchak, G.O.; Mostovyi, A.I.; Maryanchuk, P.D. Coupling between structural properties and charge transport in nano-crystalline and amorphous graphitic carbon films, deposited by electron-beam evaporation. Nanotechnology 2020, 31, 109601. [Google Scholar] [CrossRef]
- Zhang, Z.; Ding, M.; Cheng, T.; Qiao, R.; Zhao, M.; Luo, M.; Wang, E.; Sun, Y.; Zhang, S.; Li, X.; et al. Continuous epitaxy of single-crystal graphite films by isothermal carbon diffusion through nickel. Nat. Nanotechnol. 2022, 17, 1258–1264. [Google Scholar] [CrossRef]
- Sun, M.; Wang, X.; Ye, Z.; Chen, X.; Xue, Y.; Yang, G. Highly Thermal Conductive Graphite Films Derived from the Graphitization of Chemically Imidized Polyimide Films. Nanomaterials 2022, 12, 367. [Google Scholar] [CrossRef]
- Weng, M.; Luo, X.; Jian, L.; Liang, J.; Hu, J.; Liu, Y.; Zhang, J.; Feng, X.; Min, Y. Lutidine catalyzed highly thermal conductive graphite polyimide films via controlling grain size. Appl. Surf. Sci. 2022, 578. [Google Scholar] [CrossRef]
- Gao, J.; He, Z.; Dong, S.; Xie, T.; Yang, Y.; Zhuang, S.; Xu, K. Sidewall Kink Elimination of Slanted Gratings Utilizing a Twice-Etching Method. In Proceedings of the 2024 Conference of Science and Technology for Integrated Circuits (CSTIC), Shanghai, China, 17–18 March 2024. [Google Scholar]
- Choi, S.; Park, H.; Lee, S.; Koh, K.H. Fabrication of graphite nanopillars and nanocones by reactive ion etching. Thin. Solid Film. 2006, 513, 31–35. [Google Scholar] [CrossRef]
- Wang, S.; Du, J.; Chi, Z.; Cong, H.; Wang, B. Ultra-broadband metamaterial absorber in the far infrared. Mater. Lett. 2024, 355, 135518. [Google Scholar] [CrossRef]
- Zhang, W.; Chao, M.; Liu, Q.; Zhuang, L.; Cheng, B.; Jiang, B.; Song, G.; Liu, J. Broadband ultra-thin Long-Wave InfraRed metamaterial absorber based on trapezoidal pyramid array. Results Phys. 2023, 52, 106813. [Google Scholar] [CrossRef]
- Pan, Y.Z.; Li, Y.C.; Chen, F.; Yang, W.X.; Wang, B.Y. A Perfect Absorber for Ultra-long-wave Infrared Based on a Cross-Shaped Resonator Structure. Plasmonics 2023, 19, 2077–2086. [Google Scholar] [CrossRef]
- Huang, X.; Zhou, Z.; Cao, M.; Li, R.; Sun, C.; Li, X. Ultra-Broadband Mid-Infrared Metamaterial Absorber Based on Multi-Sized Resonators. Materials 2022, 15, 5411. [Google Scholar] [CrossRef] [PubMed]
- Liang, Y.; Liu, X.; Xin, J.; Zhang, X.; Wang, Y.; Song, Y. Ultra-broadband long-wave infrared metasurface absorber based on Peano fractal curve. Results Phys. 2022, 33, 105169. [Google Scholar] [CrossRef]
- Luo, Y.; Liang, Z.; Meng, D.; Tao, J.; Liang, J.; Chen, C.; Lai, J.; Qin, Y.; Lv, J.; Zhang, Y. Ultra-broadband and high absorbance metamaterial absorber in long wavelength Infrared based on hybridization of embedded cavity modes. Opt. Commun. 2019, 448, 1–9. [Google Scholar] [CrossRef]
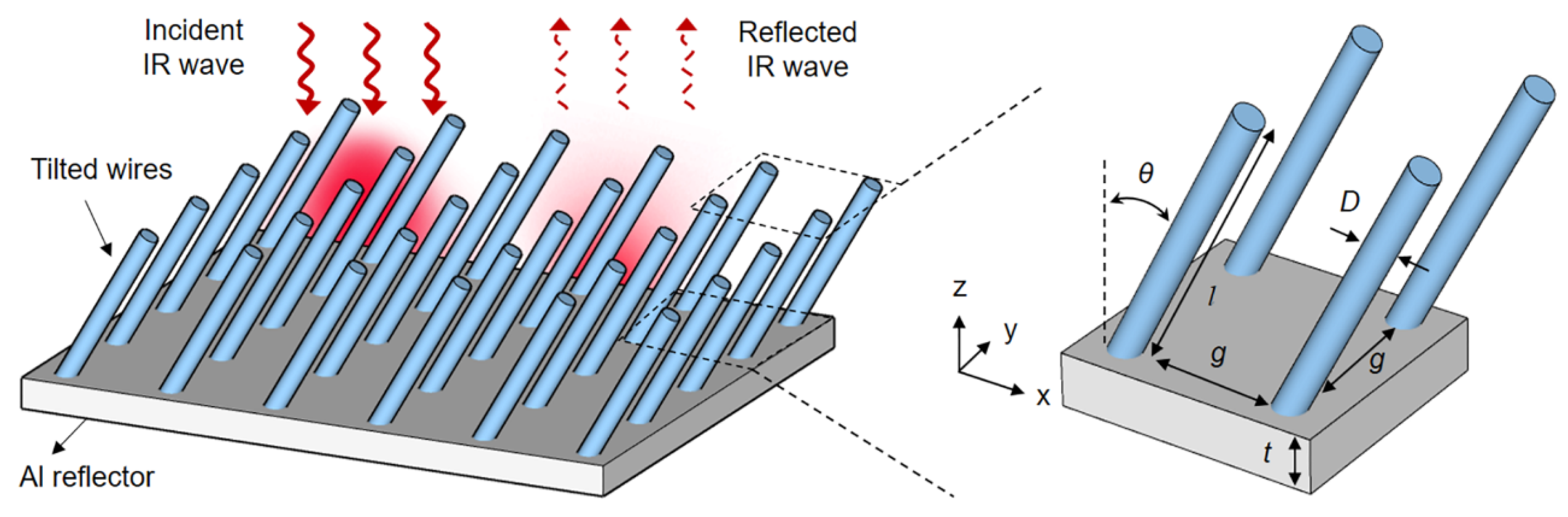
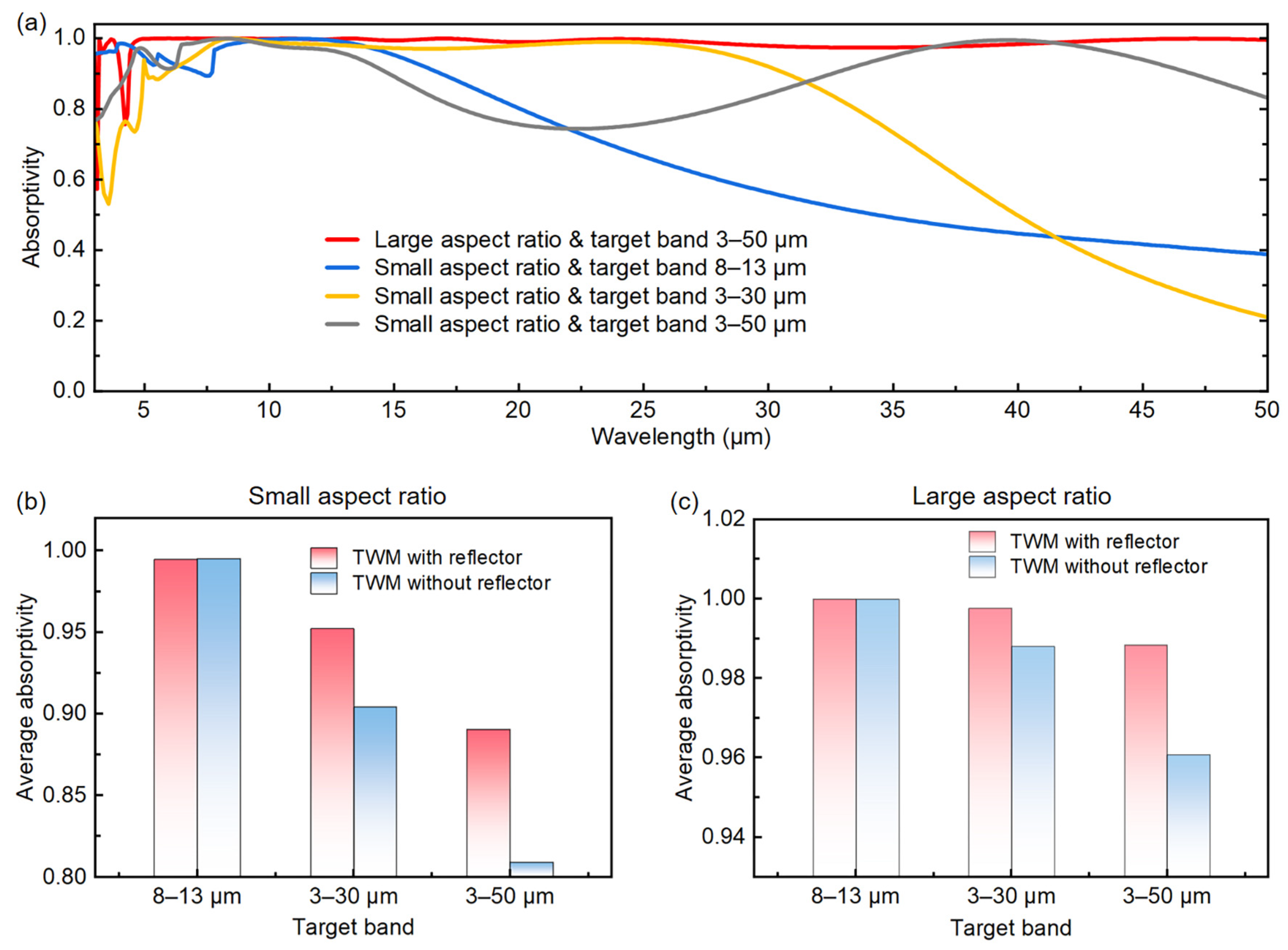
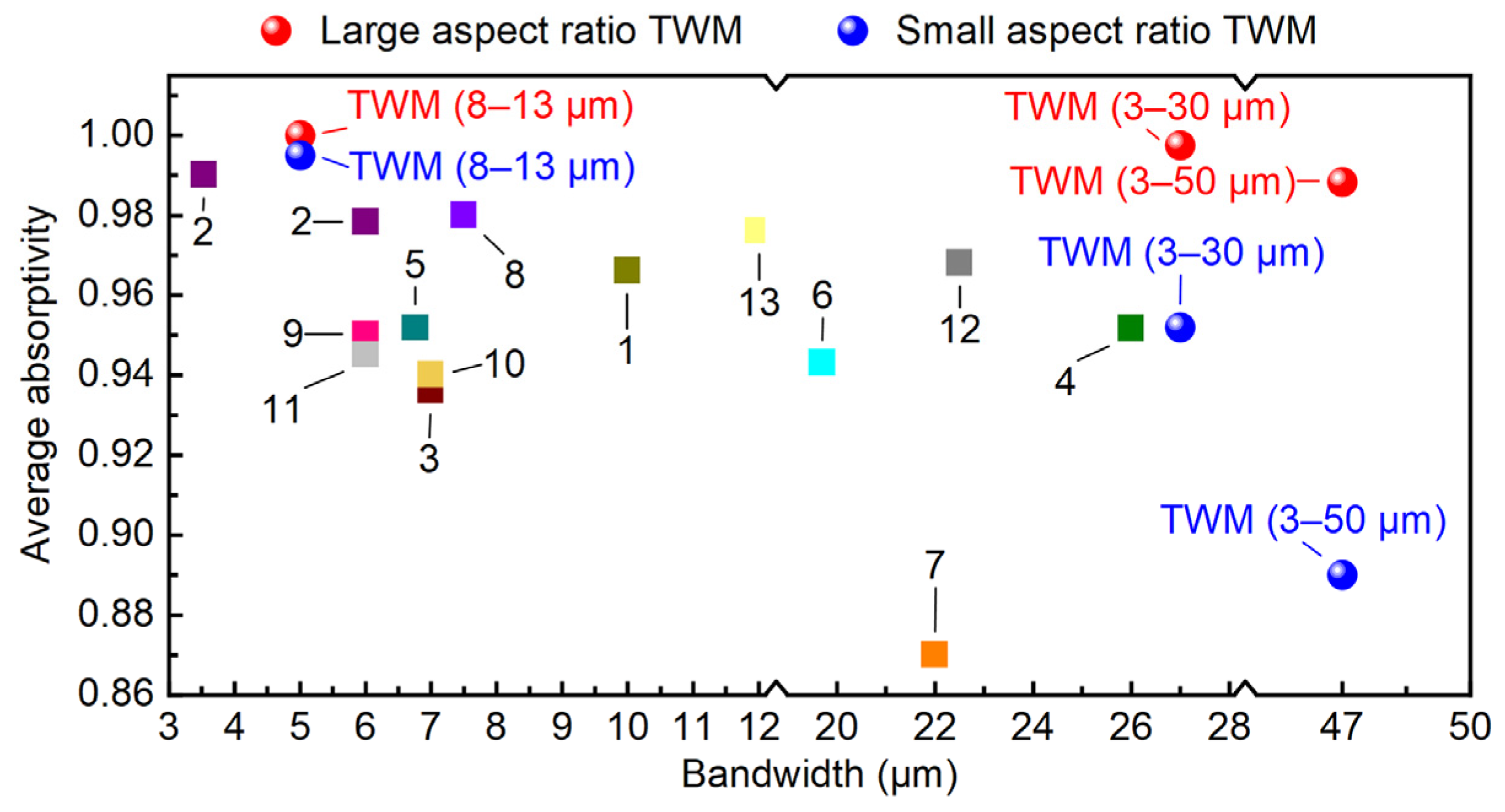

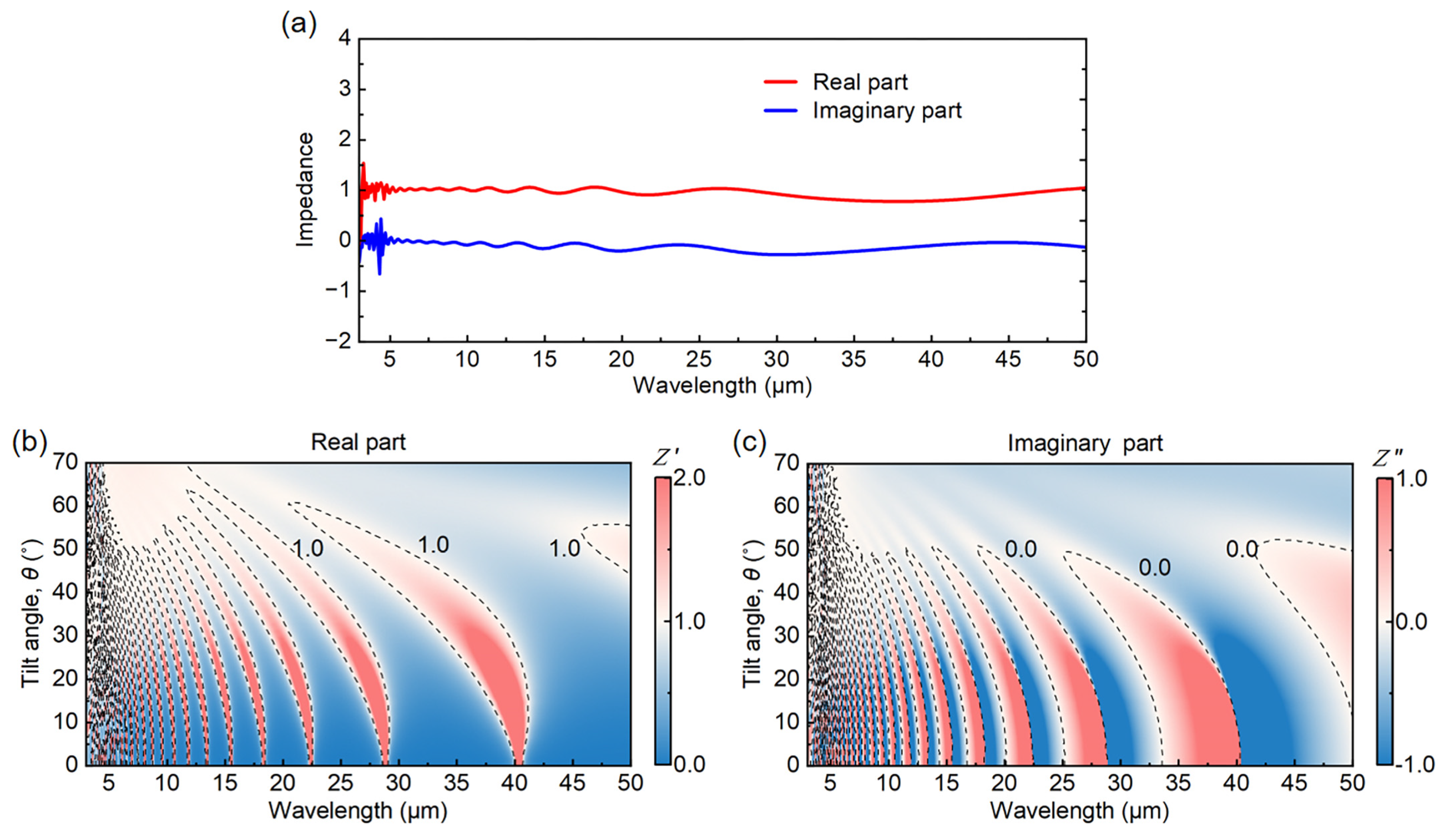

| Target Band (μm) | t (μm) | g (μm) | θ (°) | D (μm) | l (μm) | l/D | Average α |
|---|---|---|---|---|---|---|---|
| 8–13 | 0 | 2.62 | 49.0 | 4.58 | 10 | 2.18 | 0.995 |
| 0.3 | 2.06 | 42.6 | 5.71 | 9.9 | 1.74 | 0.995 | |
| 3–30 | 0 | 2.66 | 47.6 | 5.18 | 9.9 | 1.91 | 0.904 |
| 0.3 | 3.94 | 56.4 | 1 | 10 | 10.0 | 0.952 | |
| 3–50 | 0 | 2.02 | 42.2 | 4.92 | 10 | 2.03 | 0.81 |
| 0.3 | 3.29 | 50 | 2.06 | 10 | 3.27 | 0.89 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, P.; Xiao, C.; Chen, S.; Zhang, M.; Sun, Y.; Wang, H.; Zhang, J.; Zhou, H. Tilted Wire Metamaterials Enabling Ultra-Broadband Absorption from Middle to Very Long Infrared Regimes. Photonics 2024, 11, 899. https://doi.org/10.3390/photonics11100899
Wang P, Xiao C, Chen S, Zhang M, Sun Y, Wang H, Zhang J, Zhou H. Tilted Wire Metamaterials Enabling Ultra-Broadband Absorption from Middle to Very Long Infrared Regimes. Photonics. 2024; 11(10):899. https://doi.org/10.3390/photonics11100899
Chicago/Turabian StyleWang, Pan, Chengyu Xiao, Shaowen Chen, Mengqi Zhang, Ya Sun, Haoyu Wang, Jin Zhang, and Han Zhou. 2024. "Tilted Wire Metamaterials Enabling Ultra-Broadband Absorption from Middle to Very Long Infrared Regimes" Photonics 11, no. 10: 899. https://doi.org/10.3390/photonics11100899
APA StyleWang, P., Xiao, C., Chen, S., Zhang, M., Sun, Y., Wang, H., Zhang, J., & Zhou, H. (2024). Tilted Wire Metamaterials Enabling Ultra-Broadband Absorption from Middle to Very Long Infrared Regimes. Photonics, 11(10), 899. https://doi.org/10.3390/photonics11100899





