Designing a Long Optical Path Direct-Injection-Integrated Cavity for Laser Absorption Spectroscopy
Abstract
:1. Introduction
2. Materials and Methods
2.1. Principle of Direct-Injection Integrated Cavity Output Spectroscopy
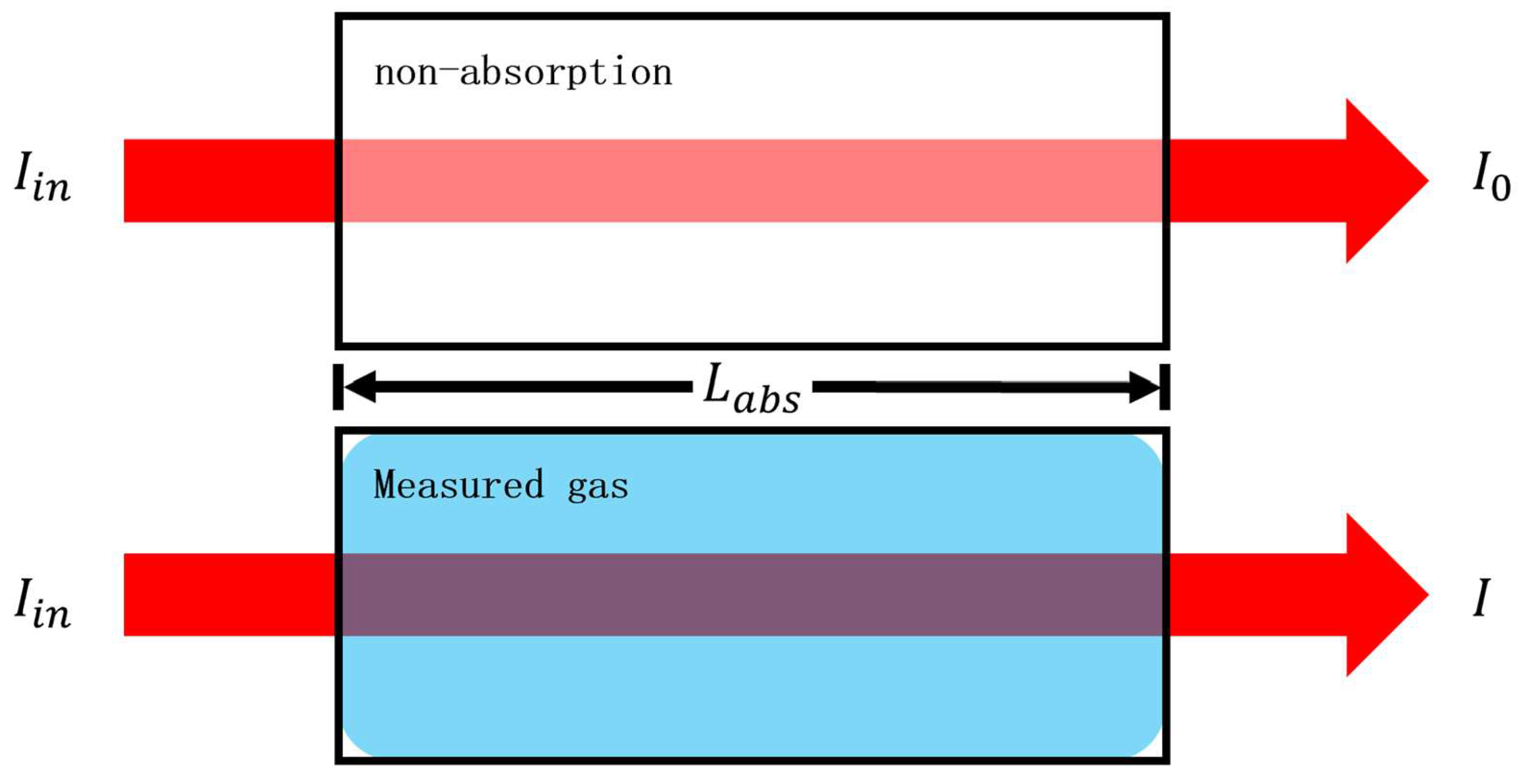
2.1.1. Lambert–Beer Law
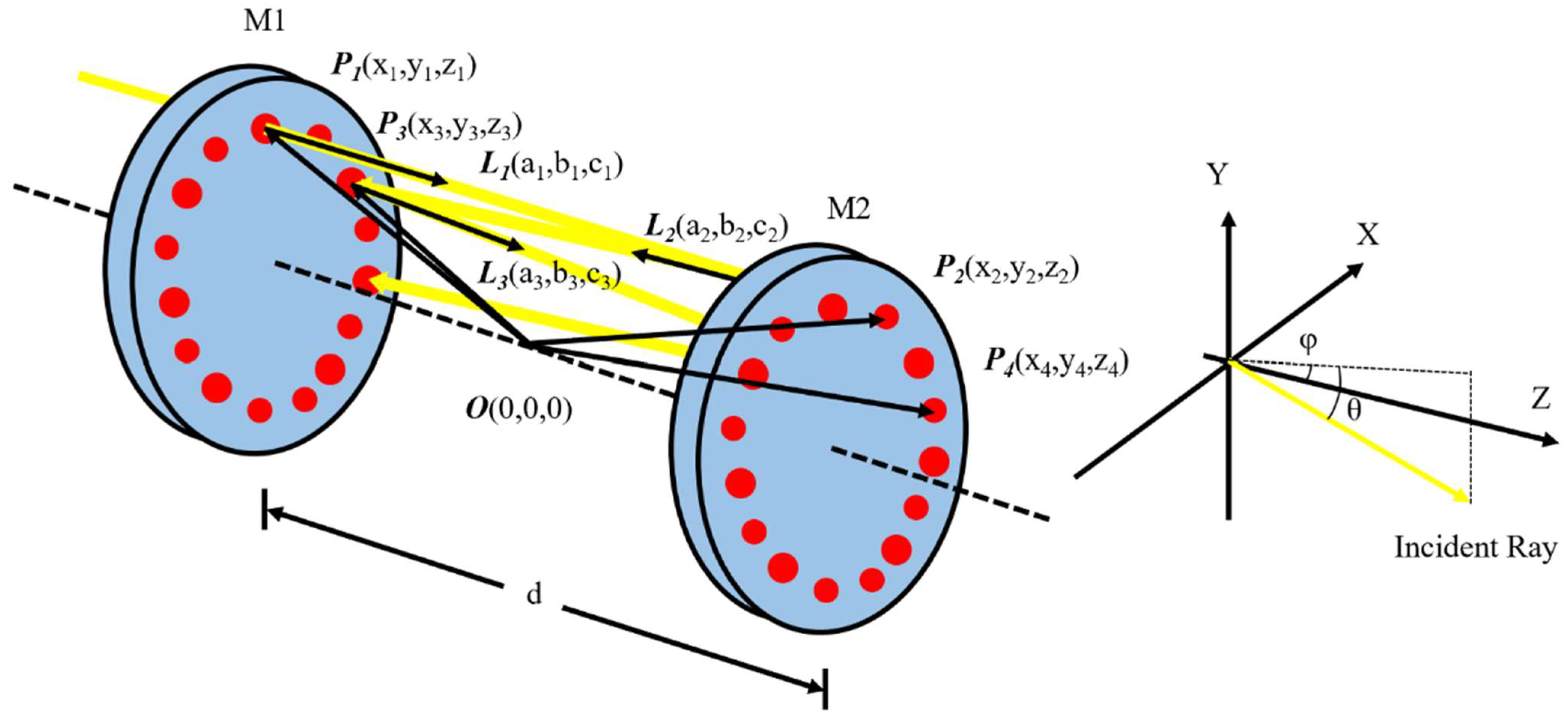
2.1.2. Modeling of an Integrated Cavity with Two Spherical Mirrors
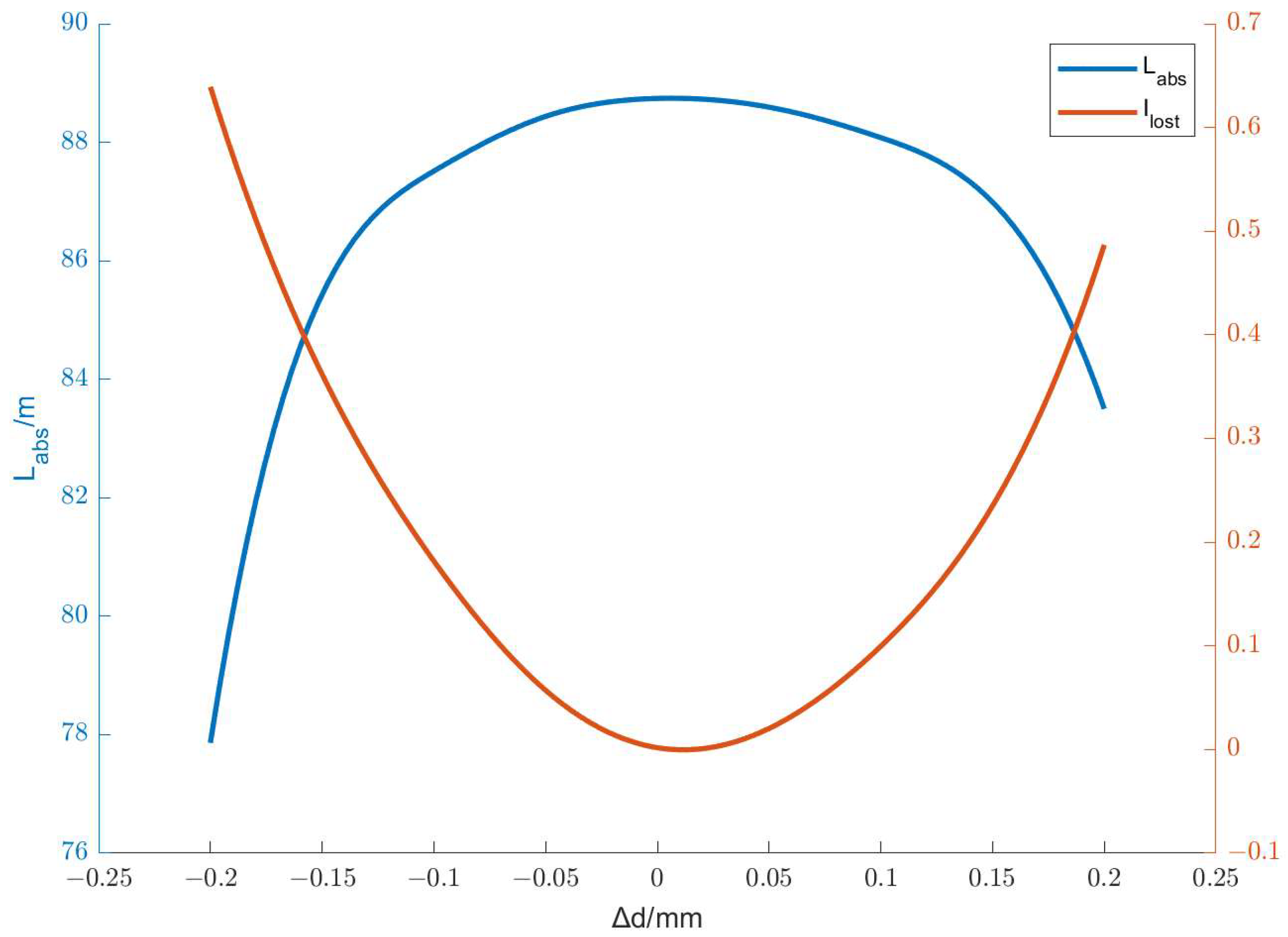
2.2. Characteristics of Spot Deformation after Multiple Reflections
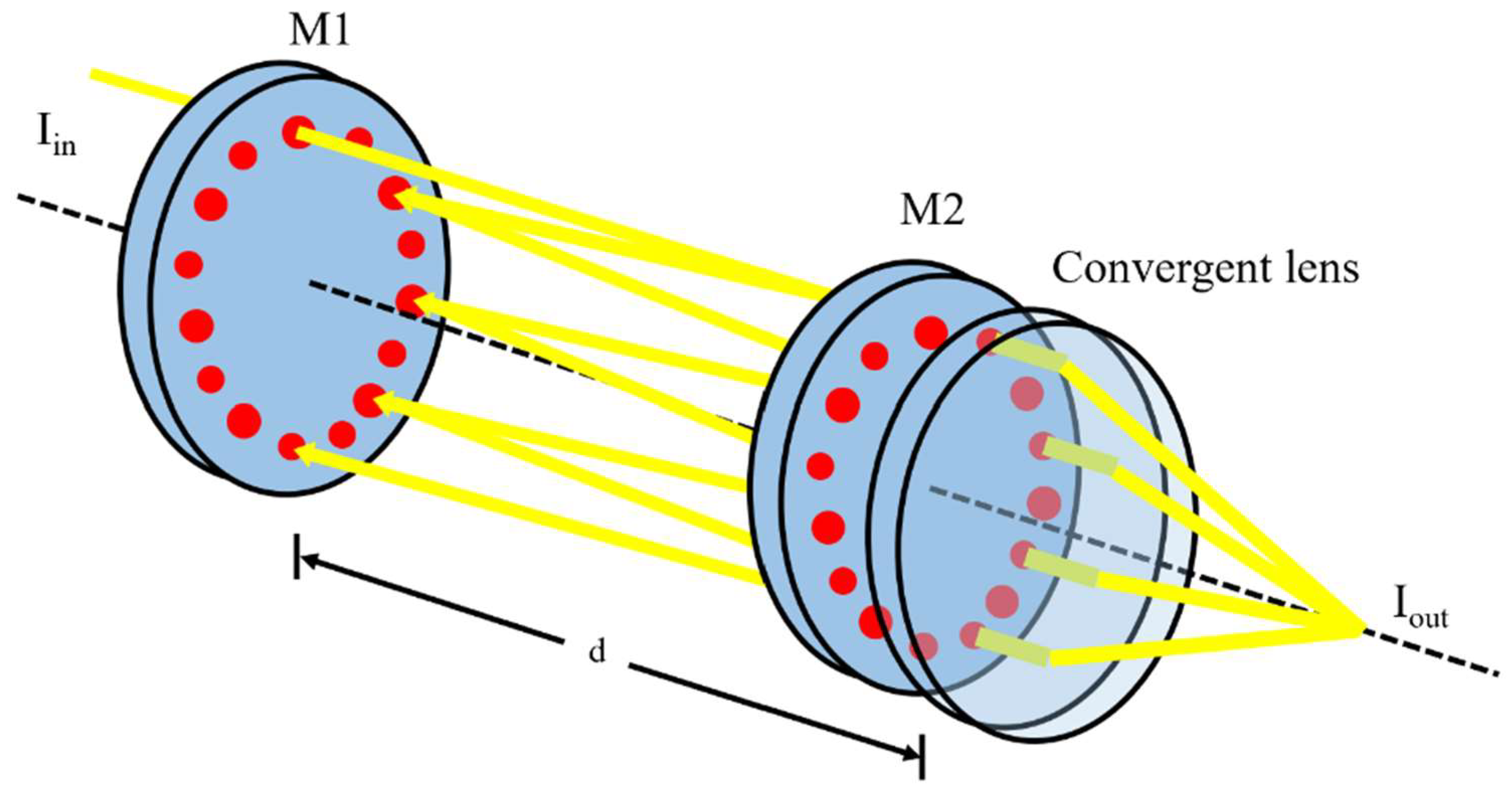
2.3. Design of Direct-Injection Integrated Cavity
- ①
- The number of reflections required for the light to return to the incident hole should be appropriate.
- ②
- When the light returns to the incident hole each time, the overlap area of the light spot with the incident hole should be as small as possible.
- ③
- The size of the incident hole should be as small as possible to prevent light rays from escaping the incident hole untimely during the entire reflection process.
3. Results
4. Discussion
4.1. Comparison with Off-Axis Integrated Cavity
4.2. Stability of Direct-Injection Integrated Cavity
4.3. Further Development of Direct-Injection Integrated Cavity
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- O’Keefe, A. Integrated cavity output analysis of ultra-weak absorption. Chem. Phys. Lett. 1998, 293, 331–336. [Google Scholar] [CrossRef]
- O’Keefe, A.; Scherer, J.J. Cwintegrated Cavity Output Spectroscopy; Optica Publishing Group: Washington, DC, USA, 2000. [Google Scholar]
- Li, Y.; Zhan, L.; Zhang, J.; Chen, L. Equilibrator-based measurements of dissolved methane in the surface ocean using an integrated cavity output laser absorption spectrometer. Acta Oceanol. Sin. 2015, 34, 34–41. [Google Scholar] [CrossRef]
- Mahesh, P.; Sreenivas, G.; Rao, P.V.N.; Dadhwal, V.K.; Sai Krishna, S.V.S.; Mallikarjun, K. High-precision surface-level CO2 and CH4 using off-axis integrated cavity output spectroscopy (OA-ICOS) over Shadnagar, India. Int. J. Remote Sens. 2015, 36, 5754–5765. [Google Scholar] [CrossRef]
- Li, L.; Duo, L.; Gong, D.; Ma, Y.; Zhang, Z.; Wang, Y.; Zhou, D.; Jin, Y. Studies of Cavity Enhanced Absorption Spectroscopy for Weak Absorption Gas Measurements. In Proceedings of the International Symposium on High Power Laser Systems and Applications, Gmunden, Austria, 5–9 September 2017. [Google Scholar]
- Tian, C.; Wang, L.; Novick, K.A. Water vapor δ2 H, δ18 O and δ17 O measurements using an off-axis integrated cavity output spectrometer—Sensitivity to water vapor concentration, delta value and averaging-time. Rapid Commun. Mass Spectrom. 2016, 30, 2077–2086. [Google Scholar] [CrossRef] [PubMed]
- Nasir, E.F.; Farooq, A. Cavity-enhanced absorption sensor for carbon monoxide in a rapid compression machine. Proc. Combust. Inst. 2019, 37, 1297–1304. [Google Scholar] [CrossRef]
- Lang, N.; Macherius, U.; Zimmermann, H.; Glitsch, S.; Wiese, M.; Röpcke, J.; Van Helden, J.-P.H. RES-Q-Trace: A Mobile CEAS-Based Demonstrator for Multi-Component Trace Gas Detection in the MIR. Sensors 2018, 18, 2058. [Google Scholar] [CrossRef] [PubMed]
- Alquaity, A.B.S.; Kc, U.; Popov, A.; Farooq, A. Detection of shock-heated hydrogen peroxide (H2O2) by off-axis cavity-enhanced absorption spectroscopy (OA-CEAS). Appl. Phys. B 2017, 123, 280. [Google Scholar] [CrossRef]
- Sprenger, M.; Tetzlaff, D.; Soulsby, C. No influence of CO2 on stable isotope analyses of soil waters with off-axis integrated cavity output spectroscopy (OA-ICOS). Rapid Comm. Mass Spectrom. 2017, 31, 430–436. [Google Scholar] [CrossRef] [PubMed]
- Mering, J.A.; Barker, S.L.L. Precise Measurement of the Hydrogen Isotope Composition of Phyllosilicates by Continuous Flow Off-Axis Integrated Cavity Output Spectroscopy. Anal. Chem. 2018, 90, 2852–2859. [Google Scholar] [CrossRef] [PubMed]
- Gupta, A.; Singh, P.J.; Gaikwad, D.Y.; Udupa, D.V.; Topkar, A.; Sahoo, N.K. Instrumentation and signal processing for the detection of heavy water using off axis–integrated cavity output spectroscopy technique. Rev. Sci. Instrum. 2018, 89, 023110. [Google Scholar] [CrossRef] [PubMed]
- Azhar, M.; Mandon, J.; Neerincx, A.H.; Liu, Z.; Mink, J.; Merkus, P.J.F.M.; Cristescu, S.M.; Harren, F.J.M. A widely tunable, near-infrared laser-based trace gas sensor for hydrogen cyanide (HCN) detection in exhaled breath. Appl. Phys. B 2017, 123, 268. [Google Scholar] [CrossRef]
- Miller, K.E.; Bajzer, Ž.; Hein, S.S.; Phillips, J.E.; Syed, S.; Wright, A.M.; Cipriani, G.; Gibbons, S.J.; Szurszewski, J.H.; Farrugia, G.; et al. High temporal resolution gastric emptying breath tests in mice. Neurogastroenterol. Motil. 2018, 30, e13333. [Google Scholar] [CrossRef] [PubMed]
- Bayrakli, I. Tunable double-mode sensor for multi-gas detection based on the external-cavity diode laser. Appl. Opt. 2018, 57, 4039. [Google Scholar] [CrossRef] [PubMed]
- Shen, G.; Chao, X.; Sun, K. Modeling the optical field in off-axis integrated-cavity-output spectroscopy using the decentered Gaussian beam model. Appl. Opt. 2018, 57, 2947. [Google Scholar] [CrossRef] [PubMed]
- Nadeem, F.; Postma, B.R.; Postma, G.; Cristescu, S.M.; Mandon, J.; Harren, F.J.M. Comprehensive three-dimensional ray tracing model for three-mirror cavity-enhanced spectroscopy. Appl. Opt. 2018, 57, 154. [Google Scholar] [CrossRef] [PubMed]
- Paul, J.B.; Lapson, L.; Anderson, J.G. Ultrasensitive absorption spectroscopy with a high-finesse optical cavity and off-axis alignment. Appl. Opt. 2001, 40, 4904. [Google Scholar] [CrossRef] [PubMed]
- Leen, J.B.; O’Keefe, A. Optical re-injection in cavity-enhanced absorption spectroscopy. Rev. Sci. Instrum. 2014, 85, 093101. [Google Scholar] [CrossRef] [PubMed]
- Wu, T.; Xu, D.; He, X.; Lai, R.; Cheng, T. Off-axis integrating cavity output spectroscopy based on wavelength modulation. Acta Opt. Sin. 2017, 37, 389–398. [Google Scholar]


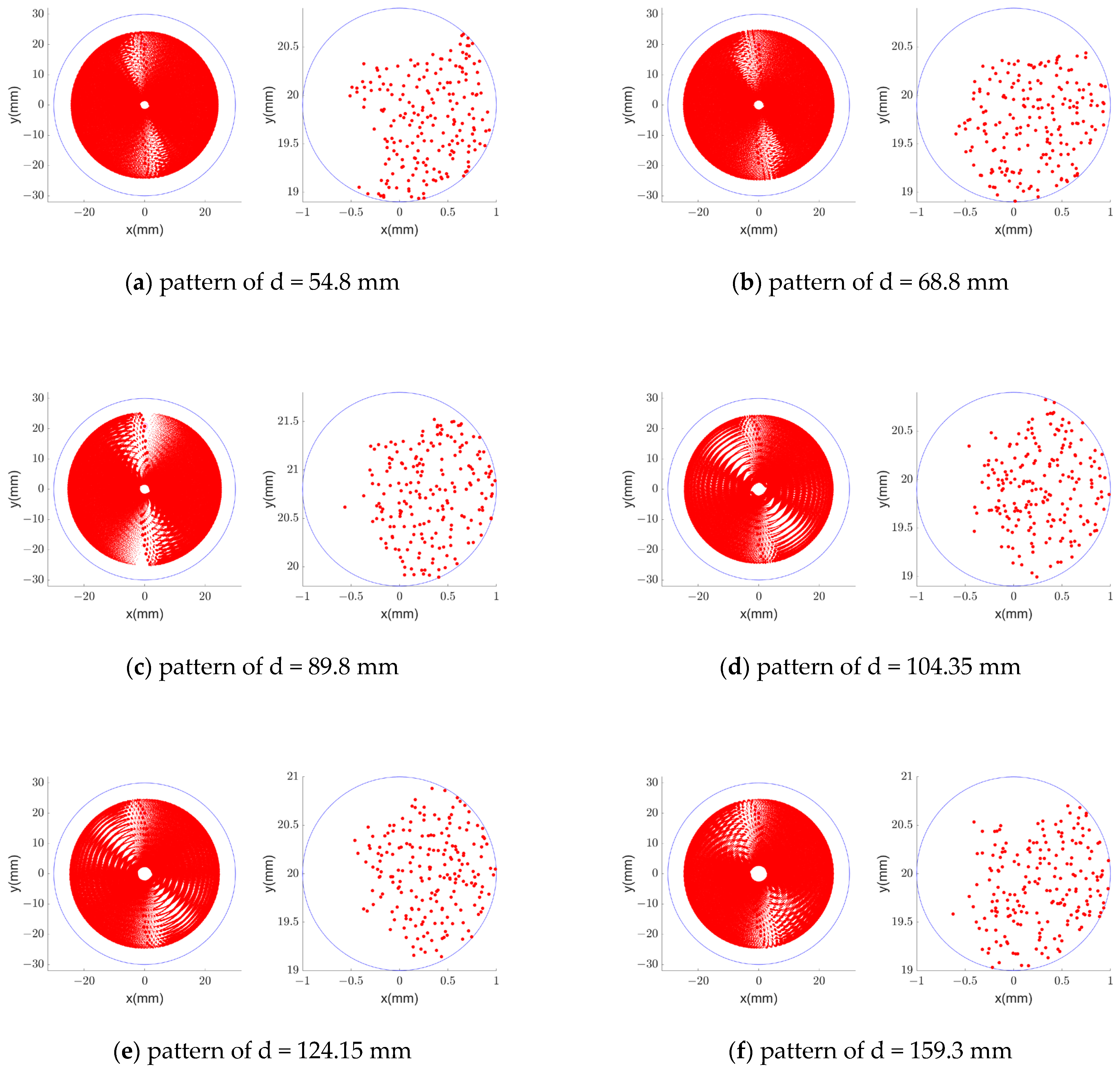
| R/mm | d/mm | N | y0/mm | θ/deg | φ/deg | ||||
|---|---|---|---|---|---|---|---|---|---|
| Figure 5a | 500 | 54.8 | 2892 | 19.9 | 8.75 | 1.0 | 0.0034 | 53.7109 | 26.7773 |
| Figure 5b | 500 | 68.8 | 2930 | 19.9 | 8.45 | 1.0 | 0.0034 | 67.4098 | 33.6056 |
| Figure 5c | 500 | 89.8 | 3257 | 20.8 | 7.55 | 0.85 | 0.0017 | 88.7411 | 44.3178 |
| Figure 5d | 500 | 104.35 | 2900 | 19.9 | 7.0 | 1.0 | 0.0035 | 102.1624 | 50.9271 |
| Figure 5e | 500 | 124.15 | 2837 | 20.0 | 6.6 | 1.0 | 0.0037 | 121.4269 | 60.5202 |
| Figure 5f | 500 | 159.3 | 2806 | 20.0 | 6.0 | 1.0 | 0.0038 | 155.6582 | 77.5713 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gao, Q.; Zheng, Y.; Wang, L.; Li, C.; Zhang, J.; Wu, H.; Han, Y.; Wang, S. Designing a Long Optical Path Direct-Injection-Integrated Cavity for Laser Absorption Spectroscopy. Photonics 2024, 11, 36. https://doi.org/10.3390/photonics11010036
Gao Q, Zheng Y, Wang L, Li C, Zhang J, Wu H, Han Y, Wang S. Designing a Long Optical Path Direct-Injection-Integrated Cavity for Laser Absorption Spectroscopy. Photonics. 2024; 11(1):36. https://doi.org/10.3390/photonics11010036
Chicago/Turabian StyleGao, Quyouyang, Yuquan Zheng, Long Wang, Chengliang Li, Jialun Zhang, Hao Wu, Yanxue Han, and Shuo Wang. 2024. "Designing a Long Optical Path Direct-Injection-Integrated Cavity for Laser Absorption Spectroscopy" Photonics 11, no. 1: 36. https://doi.org/10.3390/photonics11010036
APA StyleGao, Q., Zheng, Y., Wang, L., Li, C., Zhang, J., Wu, H., Han, Y., & Wang, S. (2024). Designing a Long Optical Path Direct-Injection-Integrated Cavity for Laser Absorption Spectroscopy. Photonics, 11(1), 36. https://doi.org/10.3390/photonics11010036





