Nonparaxial Focusing of Partially Coherent Gaussian Schell-Model and Bessel-Correlated Beams in Free Space
Abstract
:1. Introduction
2. Focusing of Gaussian Schell-Model Beam
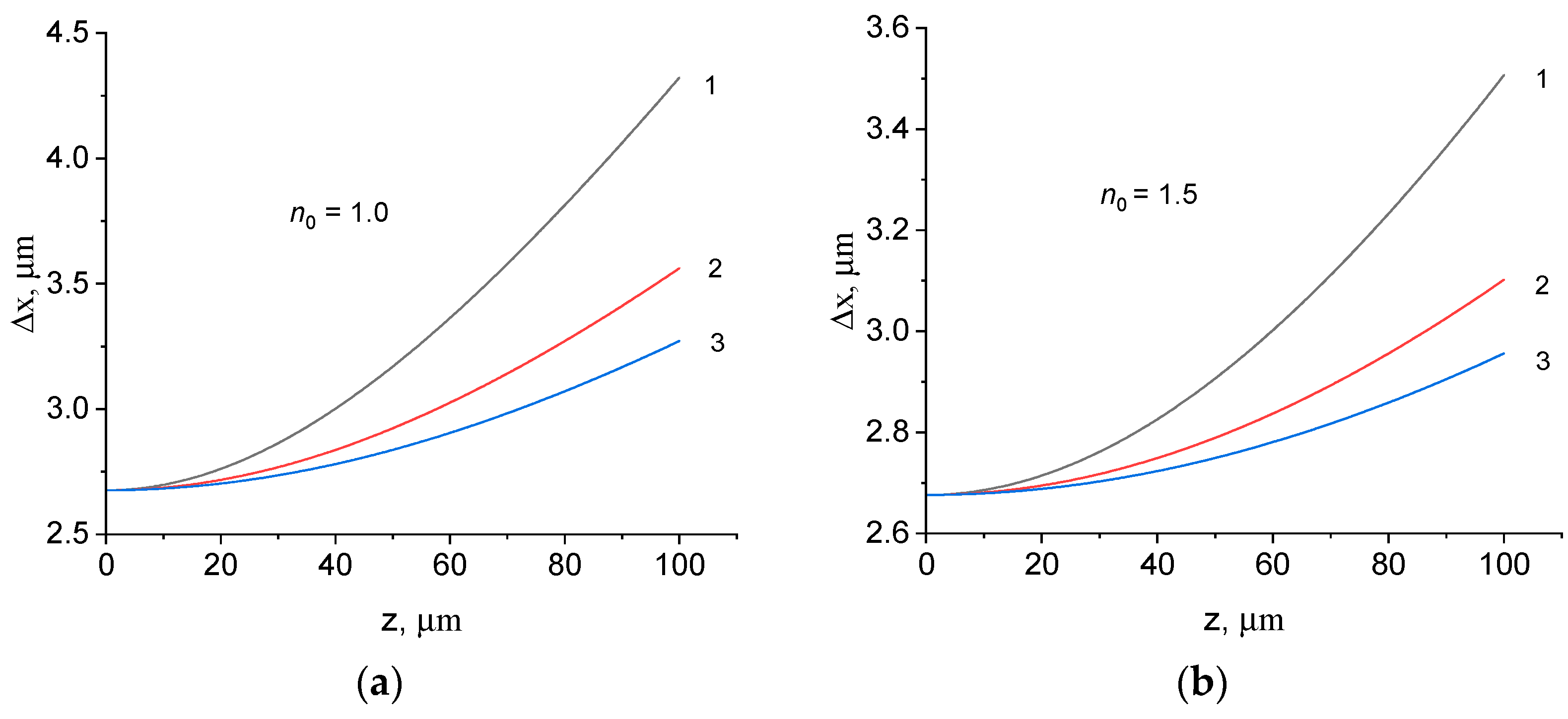
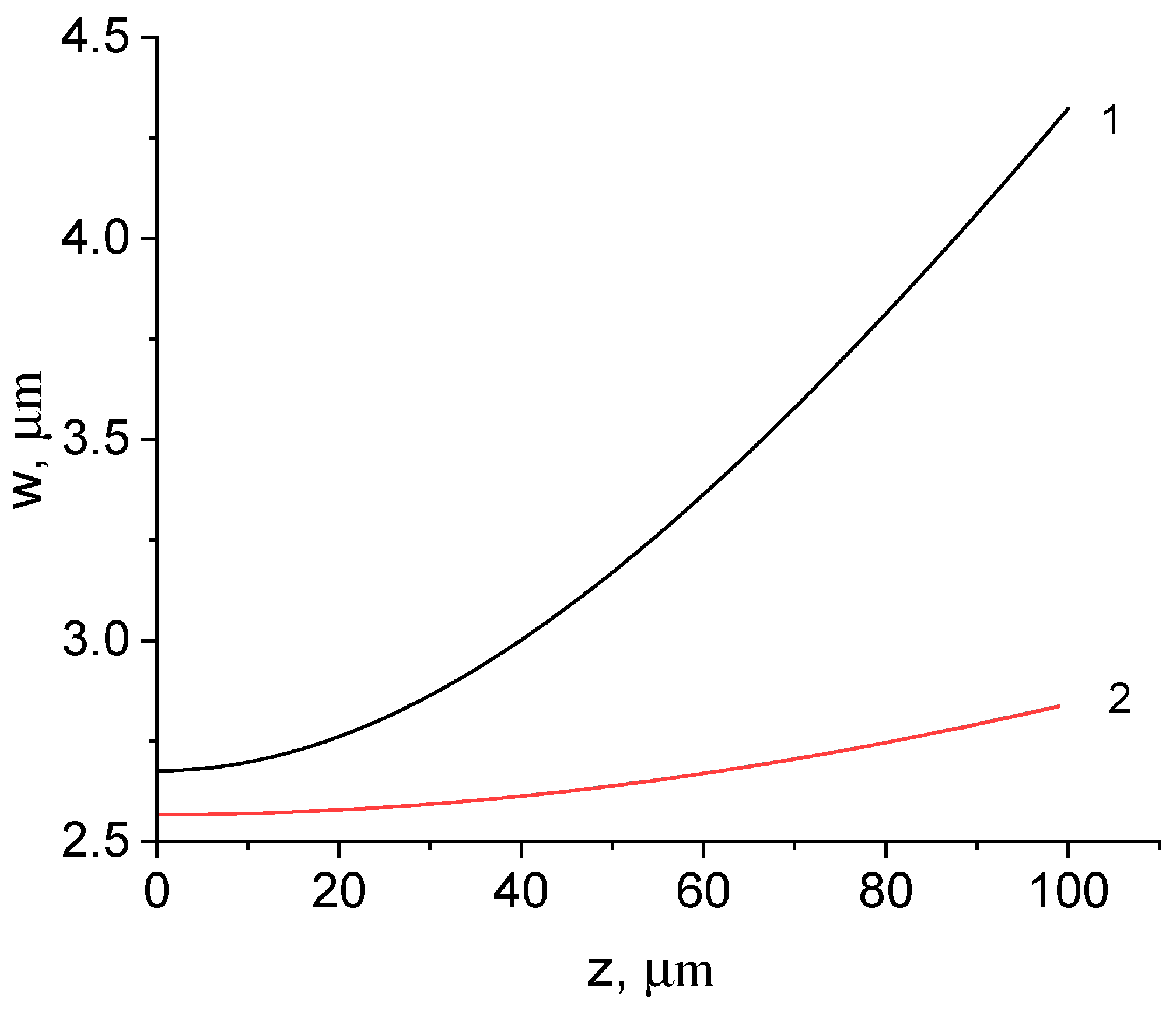
2.1. Beam Width
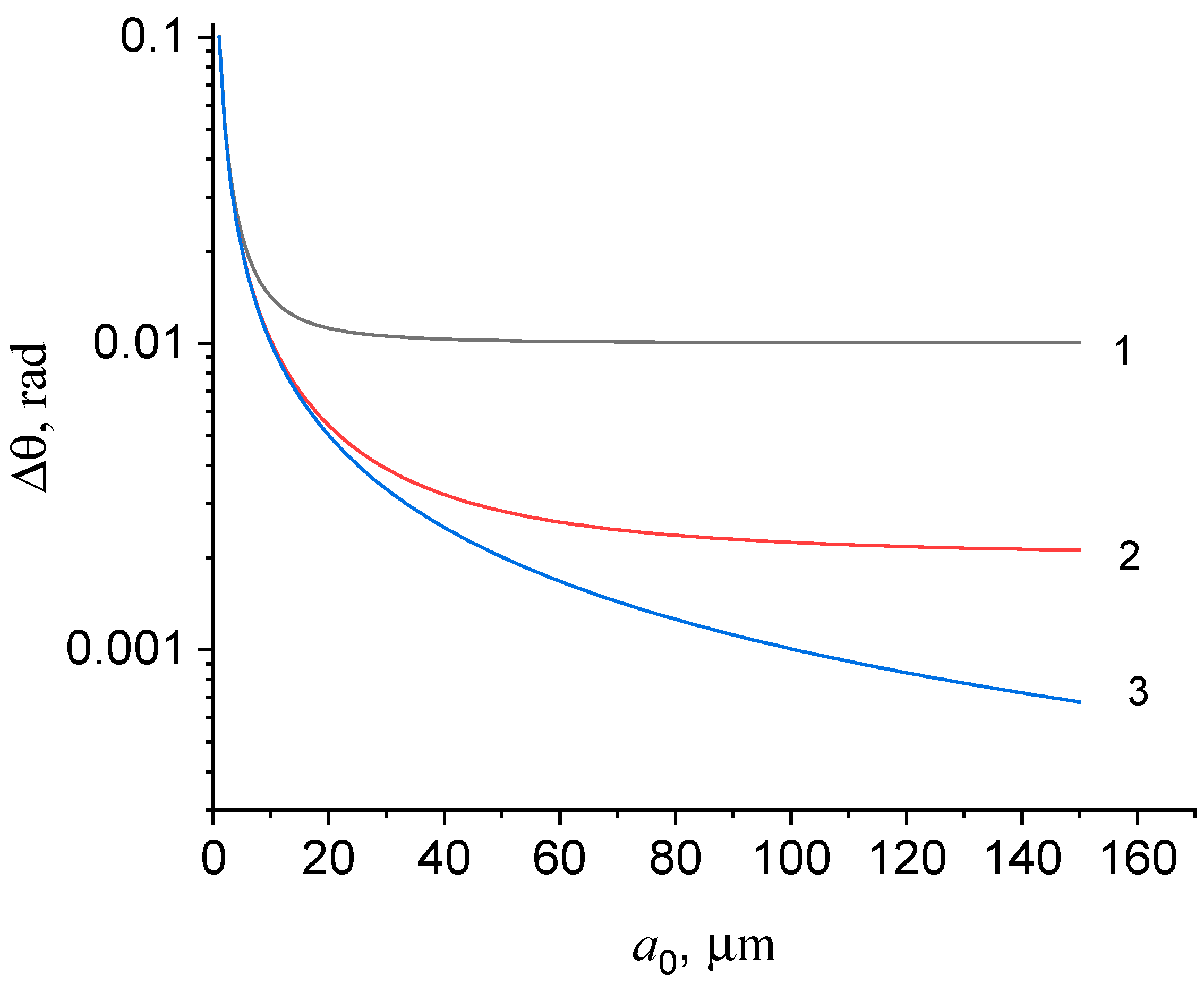
2.2. Angular Divergence
2.3. Uncertainty Relationship
3. Focusing of Bessel-Correlated Beams
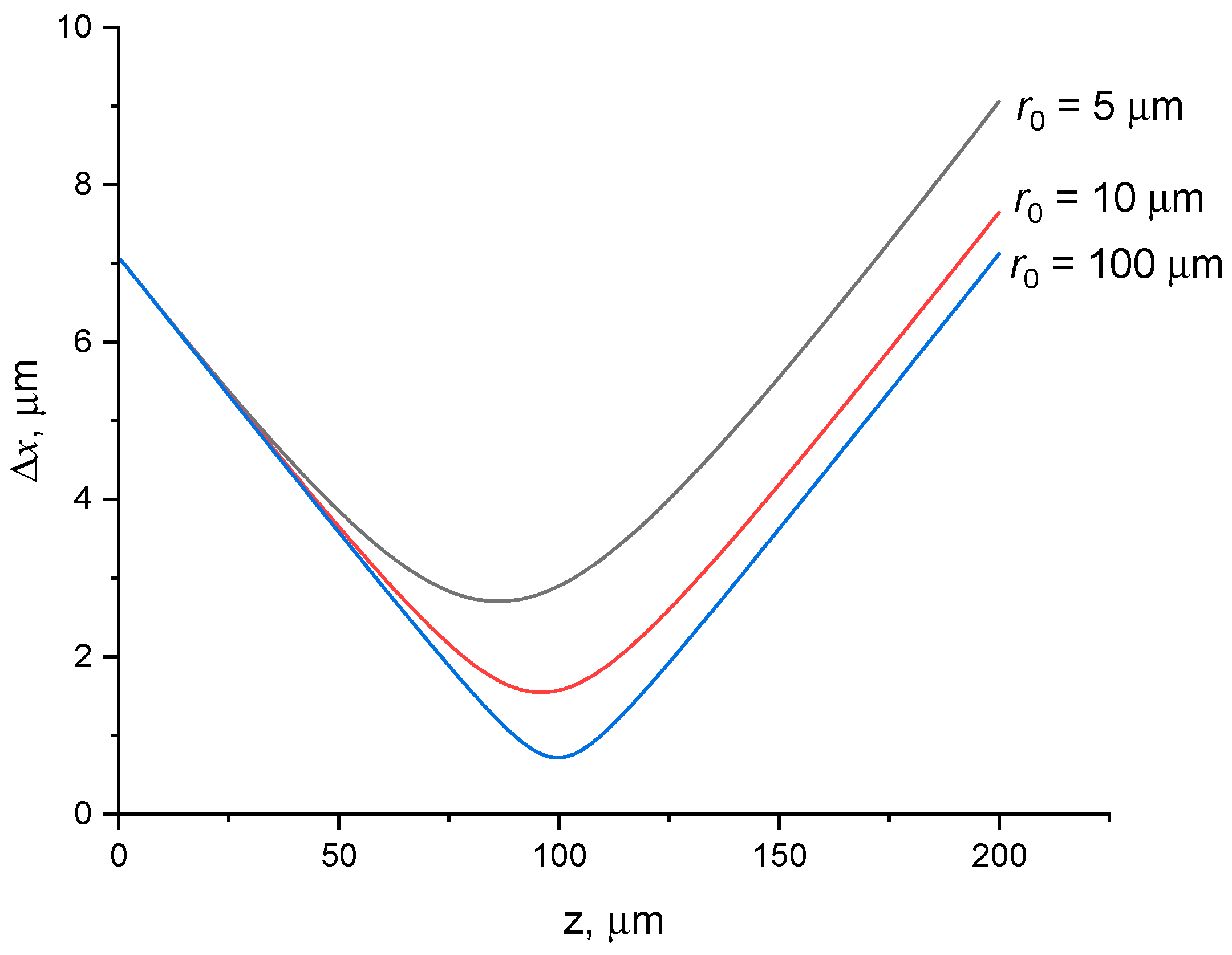
3.1. Average Beam Radius
3.2. Diffraction Spreading
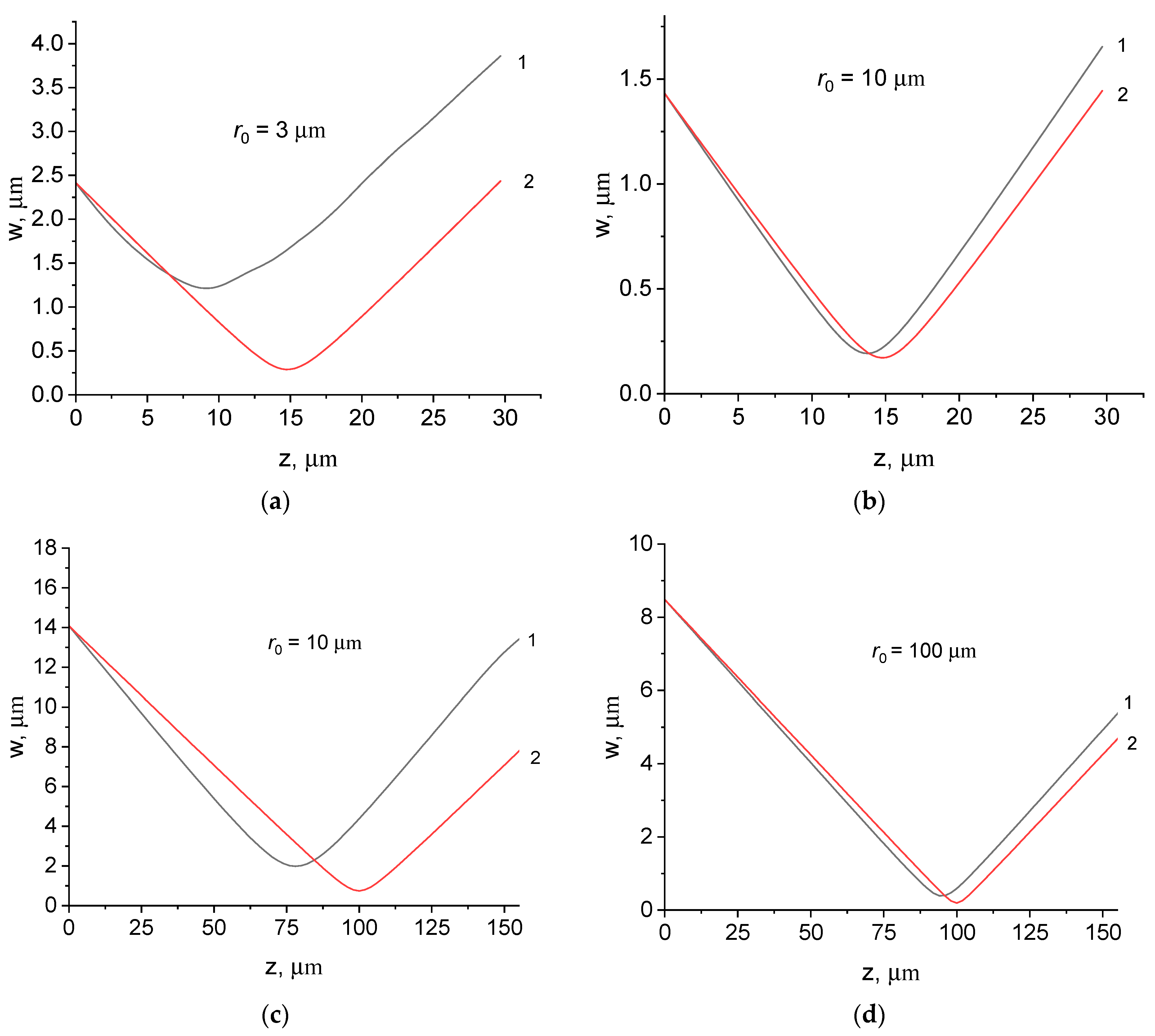
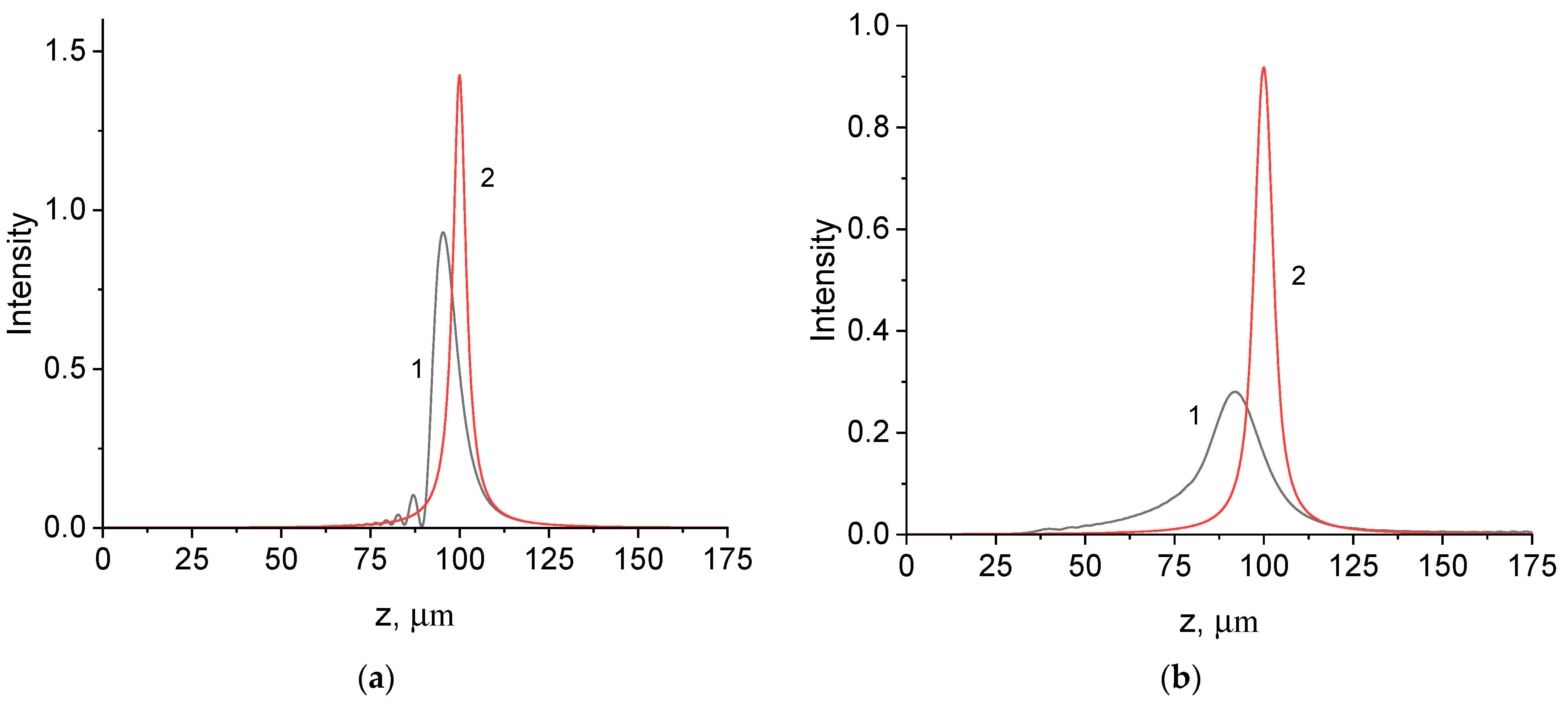
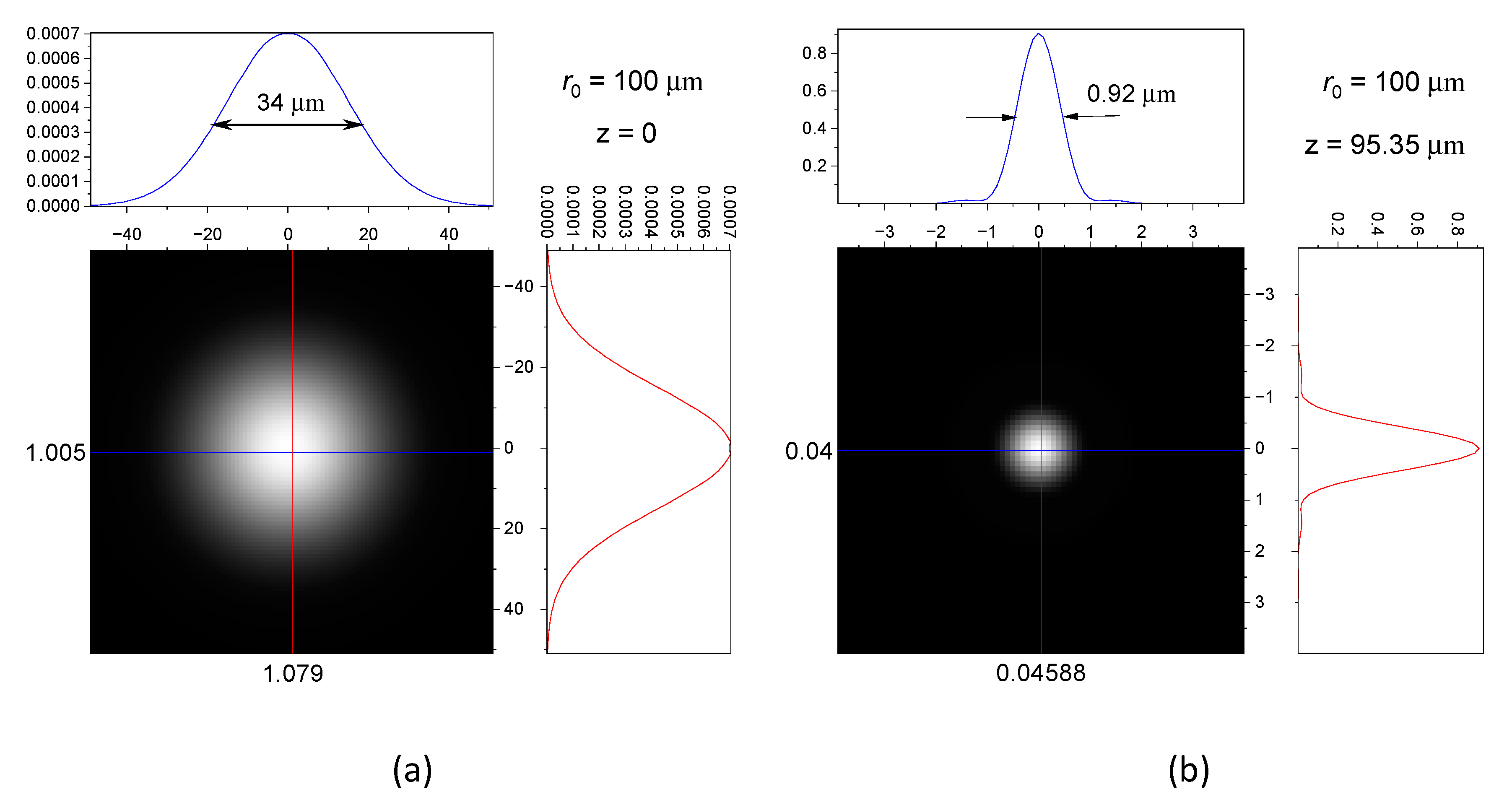
3.3. Beam Intensity Profile
4. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wolf, E. Introduction to the Theory of Coherence and Polarization of Light; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar]
- Mandel, L.; Wolf, E. Optical Coherence and Quantum Optics; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Wolf, E. Unified theory of coherence and polarization of random electromagnetic beams. Phys. Lett. A 2003, 312, 263–267. [Google Scholar] [CrossRef]
- Tervo, J.; Setala, T.; Friberg, A.T. Theory of partially coherent electromagnetic fields in the space-frequency domain. J. Opt. Soc. Am. A 2004, 21, 2205–2215. [Google Scholar] [CrossRef]
- Korotkova, O.; Hoover, B.G.; Gamiz, V.L.; Wolf, E. Coherence and polarization properties of far fields generated by quasi-homogeneous planar electromagnetic sources. J. Opt. Soc. Am. A 2005, 22, 2547–2556. [Google Scholar] [CrossRef]
- Liu, Z.; Zeng, J.; Cai, Y. Review on vortex beams with low spatial coherence. Adv. Phys. 2019, 4, 1626766. [Google Scholar] [CrossRef] [Green Version]
- Zeng, J.; Lin, R.; Liu, X.; Zhao, C.; Cai, Y. Review on partially coherent vortex beams. Front. Optoelectron. 2019, 12, 229–248. [Google Scholar] [CrossRef]
- Dong, M.; Zhao, C.; Cai, Y.; Yang, Y. Partially coherent vortex beams: Fundamentals and applications. Sci. China Phys. Mech. Astron. 2021, 64, 224201. [Google Scholar] [CrossRef]
- Zhang, H.; Lu, X.; Wang, Z.; Konijnenberg, A.P.; Wang, H.; Zhao, C.; Cai, Y. Generation and Propagation of Partially Coherent Power-Exponent-Phase Vortex Beam. Front. Phys. 2021, 9, 781688. [Google Scholar] [CrossRef]
- Chen, Y.; Wang, F.; Cai, Y. Partially coherent light beam shaping via complex spatial coherence structure engineering. Adv. Phys. X 2022, 7, 2009742. [Google Scholar] [CrossRef]
- Krivoshlykov, S.G.; Petrov, N.I.; Sisakyan, I.N. Spatial coherence of optical fields in longitudinally inhomogeneous media with the square-law index profile. Sov. J. Quantum Electron. 1985, 15, 330–338. [Google Scholar] [CrossRef]
- Petrov, N.I.; Petrova, G.N. Diffraction of partially coherent light beams by micro-lens arrays. Opt. Express 2017, 25, 22545–22564. [Google Scholar] [CrossRef] [PubMed]
- Petrov, N.I. Holographic diffuser with controlled scattering indicatrix. Comp. Opt. 2017, 41, 831–836. [Google Scholar] [CrossRef]
- Gori, F.; Palma, C. Partially coherent sources which give rise to highly directional light beams. Opt. Commun. 1978, 27, 185–188. [Google Scholar] [CrossRef]
- Ponomarenko, S.A. A class of partially coherent beams carrying optical vortices. J. Opt. Soc. Am. A 2001, 18, 150–156. [Google Scholar] [CrossRef]
- Marasinghe, M.L.; Premaratne, M.; Paganin, D.M.; Alonso, M.A. Coherence vortices in Mie scattered nonparaxial partially coherent beams. Opt. Expr. 2012, 20, 2858–2875. [Google Scholar] [CrossRef]
- Alieva, T.; Rodrigo, J.A.; Camara, A.; Abramochkin, E. Partially coherent stable and spiral beams. J. Opt. Soc. Am. A 2013, 30, 2237–2243. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ostrovsky, A.S.; García-García, J.; Rickenstorff-Parrao, C.; Olvera-Santamaria, M.A. Partially coherent diffraction-free vortex beams with a Bessel-mode structure. Opt. Lett. 2017, 42, 5182–5185. [Google Scholar] [CrossRef]
- Santamaría, M.A.O.; Garciapiña, J.L.R.; García, J.G.; Ostrovsky, A.S. Generation of a partially coherent secondary source with Bessel-mode vortex structure by liquid crystal spatial light modulator. Opt. Commun. 2019, 439, 312–316. [Google Scholar] [CrossRef]
- Chen, X.; Li, J.; Rafsanjani, S.M.H.; Korotkova, O. Synthesis of Im-Bessel correlated beams via coherent modes. Opt. Lett. 2018, 43, 3590–3593. [Google Scholar] [CrossRef]
- Xu, Z.; Li, X.; Liu, X.; Ponomarenko, S.A.; Cai, Y.; Liang, C. Vortex preserving statistical optical beams. Opt. Exp. 2020, 28, 8475–8483. [Google Scholar] [CrossRef]
- Ferlic, N.A.; van Iersel, M.; Davis, C.C. Simulation of Im-Bessel beam propagation through time-correlated atmospheric turbulence. Proc. SPIE 2021, 11834, 118340L. [Google Scholar]
- Petrov, N.I. Nonparaxial Propagation of Bessel Correlated Vortex Beams in Free Space. Micromachines 2023, 14, 38. [Google Scholar] [CrossRef]
- Fischer, D.G.; Visser, T.D. Spatial correlation properties of focused partially coherent light. J. Opt. Soc. Am. A 2004, 21, 2097–2102. [Google Scholar] [CrossRef]
- Foreman, M.R.; Torok, P. Focusing of spatially inhomogeneous partially coherent, partially polarized electromagnetic fields. J. Opt. Soc. Am. A 2009, 26, 2470–2479. [Google Scholar] [CrossRef] [Green Version]
- Chen, B.; Zhang, Z.; Pu, J. Tight focusing of partially coherent and circularly polarized vortex beams. J. Opt. Soc. Am A 2009, 26, 862–869. [Google Scholar] [CrossRef] [PubMed]
- Wang, F.; Zhu, S.; Cai, Y. Experimental study of the focusing properties of a Gaussian Schell-model vortex beam. Opt. Lett. 2011, 36, 3281–3283. [Google Scholar] [CrossRef] [PubMed]
- Xu, H.F.; Zhang, Z.; Qu, J.; Huang, W. The tight focusing properties of Laguerre–Gaussian-correlated Schell-model beams. J. Mod. Opt. 2016, 63, 1429–1437. [Google Scholar] [CrossRef]
- Ping, C.; Liang, C.H.; Wang, F.; Cai, Y.J. Radially polarized multi-Gaussian Schell-model beam and its tight focusing properties. Opt. Express 2017, 25, 32475–32490. [Google Scholar] [CrossRef]
- Lin, H.; Zhou, X.; Chen, Z.; Sasaki, O.; Li, Y.; Pu, J. Tight focusing properties of a circular partially coherent Gaussian beam. J. Opt. Soc. Am. A 2018, 35, 1974–1980. [Google Scholar] [CrossRef]
- Zhang, Z.; Pu, J.; Wang, X. Focusing of partially coherent Bessel–Gaussian beams through a high numerical-aperture objective. Opt. Lett. 2008, 33, 49–51. [Google Scholar] [CrossRef]
- Stamnes, J.J. Hybrid integration technique for efficient and accurate computation of diffraction integrals. J. Opt. Soc. Am. A 1989, 6, 1330–1342. [Google Scholar] [CrossRef]
- Eide, H.A.; Stamnes, J.J. Exact and approximate solutions for focusing of two-dimensional waves. J. Opt. Soc. Am. A 1998, 15, 1285–1319. [Google Scholar]
- Gori, F.; Santarsiero, M.; Simon, R.; Piquero, G.; Borghi, R.; Guattari, G. Coherent-mode decomposition of partially polarized, partially coherent sources. J. Opt. Soc. Am. A 2003, 20, 78–84. [Google Scholar] [CrossRef]
- Krivoshlykov, S.G.; Sisakyan, I.N. Coherent States and Light Propagation in Inhomogeneous Media. Sov. J. Quantum Electron. 1980, 10, 312–318. [Google Scholar] [CrossRef]
- Krivoshlykov, S.G.; Petrov, N.I.; Sisakyan, I.N. Correlated coherent states and propagation of arbitrary Gaussian beams in longitudinally homogeneous quadratic media exhibiting absorption or amplification. Sov. J. Quantum Electron. 1986, 16, 933–941. [Google Scholar] [CrossRef]
- Krivoshlykov, S.G.; Petrov, N.I.; Sisakyan, I.N. Mode excitation in waveguides with a quadratic refractive-index profile by partially coherent radiation sources. Sov. Tech. Phys. 1985, 30, 1031–1036. [Google Scholar]
- Krivoshlykov, S.G.; Petrov, N.I.; Sisakyan, I.N. Density-matrix formalism for partially coherent optical fields propagating in slightly inhomogeneous media. Opt. Quant. Electr. 1986, 18, 253–264. [Google Scholar] [CrossRef]
- Petrov, N.I. Nonparaxial focusing of wave beams in a graded-index medium. Rus. J. Quant. Electron. 1999, 29, 249–255. [Google Scholar] [CrossRef]
- Petrov, N.I. Focusing of beams into subwavelength area in an inhomogeneous medium. Opt. Exp. 2001, 9, 658–673. [Google Scholar] [CrossRef]
- Yuan, Y.; Du, S.; Dong, Y.; Wang, F.; Zhao, C.; Cai, Y. Nonparaxial propagation properties of a vector partially coherent dark hollow beam. J. Opt. Soc. Am. A 2013, 30, 1358–1372. [Google Scholar] [CrossRef]
- Petrov, N.I. Macroscopic quantum effects for classical light. Phys. Rev. A 2014, 90, 043814. [Google Scholar] [CrossRef] [Green Version]
- Petrov, N.I. Evanescent and propagating fields of a strongly focused beam. J. Opt. Soc. Am. A 2003, 20, 2385–2389. [Google Scholar] [CrossRef]
- Petrov, N.I. Reflection and transmission of strongly focused light beams at a dielectric interface. J. Mod. Opt. 2005, 52, 1545–1556. [Google Scholar] [CrossRef]
- Zhang, Z.; Fan, H.; Xu, H.F.; Qu, J.; Huang, W. Three-dimensional focus shaping of partially coherent circularly polarized vortex beams using a binary optic. J. Opt. 2015, 17, 065611. [Google Scholar] [CrossRef] [Green Version]
- Petrov, N.I. Depolarization of Light in Optical Fibers: Effects of Diffraction and Spin-Orbit Interaction. Fibers 2021, 9, 34. [Google Scholar] [CrossRef]
- Petrov, N.I. Depolarization of vector light beams on propagation in free space. Photonics 2022, 9, 162. [Google Scholar] [CrossRef]
- Li, H.; Ma, C.; Wang, J.; Tang, M.; Li, X. Spin-orbit Hall effect in the tight focusing of a radially polarized vortex beam. Opt. Exp. 2021, 29, 39419–39427. [Google Scholar] [CrossRef]
- Dev, V.; Reddy, A.N.K.; Ustinov, A.V.; Khonina, S.N.; Pal, V. Autofocusing and Self-Healing Properties of Aberration Laser Beams in a Turbulent Media. Phys. Rev. Appl. 2021, 16, 014061. [Google Scholar] [CrossRef]
- Zeng, Z.; Zhao, D. Partially coherent dual nonparaxial accelerating beams. Ann. Phys. 2020, 533, 2000438. [Google Scholar] [CrossRef]
- Hyde, M.W., IV; Korotkova, O.; Spencer, M.F. Partially coherent sources whose coherent modes are spatiotemporal optical vortex beams. J. Opt. 2023, 25, 035606. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Petrov, N.I. Nonparaxial Focusing of Partially Coherent Gaussian Schell-Model and Bessel-Correlated Beams in Free Space. Photonics 2023, 10, 857. https://doi.org/10.3390/photonics10070857
Petrov NI. Nonparaxial Focusing of Partially Coherent Gaussian Schell-Model and Bessel-Correlated Beams in Free Space. Photonics. 2023; 10(7):857. https://doi.org/10.3390/photonics10070857
Chicago/Turabian StylePetrov, Nikolai I. 2023. "Nonparaxial Focusing of Partially Coherent Gaussian Schell-Model and Bessel-Correlated Beams in Free Space" Photonics 10, no. 7: 857. https://doi.org/10.3390/photonics10070857
APA StylePetrov, N. I. (2023). Nonparaxial Focusing of Partially Coherent Gaussian Schell-Model and Bessel-Correlated Beams in Free Space. Photonics, 10(7), 857. https://doi.org/10.3390/photonics10070857




