A Brief Review on Nonlinear Photonic Crystals Induced by Direct Femtosecond Laser Writing
Abstract
1. Introduction
2. -Erasing Technique Using Femtosecond Laser
3. -Poling Technique by Femtosecond Laser
3.1. Primary Domain Inversion
3.2. Secondary Domain Inversion
3.3. Two Types of Domain Inversion Simultaneously Occurring
4. Laser Writing Parameters
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| 1D | One-dimensional |
| 2D | Two-dimensional |
| 3D | Three-dimensional |
| SHG | Secpnd harmonic generation |
| DFG | Difference-frequency generation |
| SFG | Sum-frequency generation |
| OPO | Optical parametric generation |
| SH | Second harmonic |
| BPM | Birefringence Phase Matching |
| QPM | Quasi-phase matching |
| RVL | reciprocal vector |
| PPLN | Periodically poled lithium noibate |
| NPC | Nonlinear photonic crystal |
| PC | Photonic crystal |
| UV | Ultraviolet |
| SHM | erenkov SH microscopy |
References
- Wu, A.; Xu, J.; Zheng, Y.; Liang, X. Crystal growth and application of large size YCOB crystal for high power laser. Opt. Mater. 2014, 36, 2000–2003. [Google Scholar] [CrossRef]
- Li, Y.; Huang, X.; Mao, W.; Xu, J.; Duan, Y.; Zhu, H. Compact 589 nm yellow source generated by frequency-doubling of passively Q-switched Nd:YVO4 Ramen laser. Microw. Opt. Technol. Lett. 2023, 65, 1122. [Google Scholar] [CrossRef]
- Mazumder, N.; Balla, N.K.; Zhuo, G.; Kistenev, Y.V.; Kumar, R.; Kao, F.; Brasselet, S.; Nikolaev, V.V.; Krivova, N.A. Label-free nonlinear multimodal optical microscopy–basics, development, and applications. Front. Phys. 2019, 7, 170. [Google Scholar] [CrossRef]
- Zheng, Z.; Zhou, N.; Zhou, R. Enhancing Resolution of Quantitative Phase Microscopy through Second-Harmonic Generation and Structured Illumination. In Proceedings of the TENCON 2022–2022 IEEE Region 10 Conference (TENCON), Hong Kong, China, 1–4 November 2022; pp. 1–3. [Google Scholar]
- Gibson, G.; Courtial, J.; Padgett, M.J.; Vasnetsov, M.; Pas’ko, V.; Barnett, S.M.; Franke-Arnold, S. Free-space information transfer using light beams carrying orbital angular momentum. Opt. Express 2019, 12, 5448–5456. [Google Scholar] [CrossRef]
- Li, S.; Bao, J.; Deng, Q.; Chen, L.; Wang, H. Frequency Conversion Interface towards Quantum Network: From Atomic Transition Line to Fiber Optical Communication Band. Appl. Sci. 2022, 12, 6522. [Google Scholar] [CrossRef]
- Hong, X.H.; Yang, B.; Zhang, C.; Qin, Y.Q.; Zhu, Y.Y. Nonlinear volume holography for wave-front engineering. Phys. Rev. Lett. 2014, 113, 163902. [Google Scholar] [CrossRef]
- Bowman, P.M.; Bowman, R. Tweezers with a twist. Nat. Photonics 2003, 5, 343–348. [Google Scholar]
- Grier, D.G. A revolution in optical manipulation. Nature 2003, 424, 810–816. [Google Scholar] [CrossRef]
- Pryamikov, A.; Hadzievski, L.; Fedoruk, M.; Turitsyn, S.; Aceves, A. Optical vortices in waveguides with discrete and continuous rotational symmetry. J. Eur. Opt. Soc.-Rapid Public 2021, 17, 23. [Google Scholar] [CrossRef]
- Adcock, J.C.; Ding, Y. Quantum prospects for hybrid thin-film lithium niobate on silicon photonics. Front. Optoelectron. 2022, 15, 7. [Google Scholar] [CrossRef]
- Vinogradova, I.L.; Gizatulin, A.R.; Meshkov, I.K.; Bagmanov, V.K. Nonlinear materials for preparation of entangled states of light. In Proceedings of the Optical Technologies for Telecommunications 2021, Samara, Russian, 23–26 November 2021; p. 122950I. [Google Scholar]
- Powers, P.E.; Haus, J.W. Chapter 5: Quasi-phase matching. In Fundamental of Nonlinear Optics; Liu, H., Ed.; CRC Press: Beijing, China, 2020; pp. 161–181. [Google Scholar]
- Armstrong, J.A.; Bloembergen, N.; Ducuing, J.; Pershan, P.S. Interactions between light waves in a nonlinear dielectric. Phys. Rev. 1962, 127, 1918. [Google Scholar] [CrossRef]
- Franken, P.A.; Hill, A.E.; Peters, C.W.; Weinreich, G. Generation of optical harmonics. Phys. Rev. Lett. 1961, 7, 118. [Google Scholar] [CrossRef]
- Franken, P.A.; Ward, J.F. Optical harmonic and nonlinear phenomena. Rev. Mod. Phys. 1963, 35, 23. [Google Scholar] [CrossRef]
- Fejer, M.M.; Magel, G.A.; Jundt, D.H.; Byer, R.L. Quasi-phase-matched second harmonic generation: Tuning and tolerances. IEEE J. Quantum Elect. 1992, 28, 2631–2654. [Google Scholar] [CrossRef]
- Xue, Y.H.; Ming, N.B.; Zhu, J.S.; Feng, D. The second harmonic generation in LiNbO3 crystals with period laminar ferroelectric domains. Chin. Phys. 1984, 4, 554–564. [Google Scholar]
- Feisst, A.; Koidl, P. Current induced periodic ferroelectric domain structures in LiNbO3 applied for efficient nonlinear optical frequency mixing. Appl. Phys. Lett. 1985, 47, 1125–1127. [Google Scholar] [CrossRef]
- Wang, W.S.; Zhou, Q.; Geng, Z.H.; Feng, D. Study of LiTaO3 crystals grown with a modulated structure I. second harmonic generation in LiTaO3 crystals grown with period laminar ferroelectric domains. J. Cryst. Growth 1986, 79, 706–709. [Google Scholar]
- Shur, V.Y.; Akhmatkhanov, A.R.; Baturin, I.S. Micro- and nano-domain engineering in lithium niobate. Appl. Phys. Rev. 2015, 2, 040604. [Google Scholar] [CrossRef]
- Wang, T.; Chen, P.; Xu, C.; Zhang, Y.; Wei, D.; Hu, X.; Zhao, G.; Xiao, M.; Zhu, S. Periodically poled LiNbO3 crystals from 1D and 2D to 3D. Sci. China Technol. Sci. 2020, 63, 1110–1126. [Google Scholar] [CrossRef]
- Zhang, B.; Li, L.; Lu, Q.; Wang, L.; Chen, F. Frequency doubling in PPLN depressed-cladding waveguides written by femtosecond laser. Opt. Mater. 2022, 125, 112074. [Google Scholar] [CrossRef]
- Lu, Y.L.; Lu, Y.Q.; Xue, C.C.; Ming, N.B. Growth of Nd3+-doped LiNbO3 optical superlattice crystals and its potential applications in self-frequency doubling. Appl. Phys. Lett. 1996, 68, 1467–1469. [Google Scholar] [CrossRef]
- Zheng, J.J.; Lu, Y.Q.; Luo, G.P.; Ma, J.; Lu, Y.L.; Ming, N.B.; He, J.L.; Xu, Z.Y. Visible dual-wavelength light generation in optical superlattice Er:LiNbO3 through upconversion and quasi-phase-matched frequency doubling. Appl. Phys. Lett. 1998, 72, 1808–1810. [Google Scholar] [CrossRef]
- Xu, T.X.; Lu, D.Z.; Yu, H.H.; Zhang, H.J.; Zhang, Y.; Wang, J.Y. A naturally grown three-dimensional nonlinear photonic crystal. Appl. Phys. Lett. 2016, 108, 051907. [Google Scholar] [CrossRef]
- Rosenman, G.; Urenski, P.; Agronin, A.; Rosenwaks, Y.; Molotskii, M. Submicron ferroelectric domain structures tailored by high-voltage scanning probe microscopy. Appl. Phys. Lett. 2003, 82, 103–105. [Google Scholar] [CrossRef]
- Yamada, M.; Kishima, K. Fabrication of periodically reversed domain structure for SHG in LiNbO3 by direct electron beam lithography beam lithography at room teperature. Electron. Lett. 1991, 27, 828–829. [Google Scholar] [CrossRef]
- Hsu, W.; Gupta, M.C. Domain inversion in LiTaO3 by electron beam. Appl. Phys. Lett. 1992, 60, 1–3. [Google Scholar] [CrossRef]
- Muir, A.C.; Sones, C.L.; Mailis, S.; Eason, R.W.; Jungk, T.; Hoffmann, Á.; Soergel, E. Direct-writing of inverted domains in lithium niobate using a continuous wave ultra violet laser. Opt. Express 2008, 16, 2336–2350. [Google Scholar] [CrossRef]
- Boes, A.; Steigerwald, H.; Crasto, T.; Wade, S.A.; Limboeck, T.; Soergel, E.; Mitchell, A. Tailor-made domain structures on the x- and y-face of lithium niobate crystals. Appl. Phys. B 2014, 115, 577–581. [Google Scholar] [CrossRef]
- Berger, V. Nonlinear photonic crystals. Phys. Rev. Lett. 1998, 81, 4136. [Google Scholar] [CrossRef]
- John, S. Strong localization of photons in certain disordered dielectric superlattices. Phys. Rev. Lett. 1987, 58, 2486–2489. [Google Scholar] [CrossRef]
- Yablonovitch, E. Inhibited spontaneous emission in solid-state physics and electronics. Phys. Rev. Lett. 1987, 58, 2059–2062. [Google Scholar] [CrossRef]
- Saltiel, S.M.; Neshev, D.N.; Fischer, R.; Krolikowski, W.; Arie, A.; Kivshar, Y.S. Generation of second-harmonic conical waves via nonlinear bragg diffraction. Phys. Rev. Lett. 2008, 100, 103902. [Google Scholar] [CrossRef]
- Saltiel, S.M.; Neshev, D.N.; Krolikowski, W.; Arie, A.; Bang, O.; Kivshar, Y.S. Multiorder nonlinear diffraction in frequency doubling processes. Opt. Lett. 2009, 34, 848–850. [Google Scholar] [CrossRef]
- Sheng, Y.; Kong, Q.; Roppo, V.; Kalinowski, K.; Wang, Q.; Cojocaru, C.; Krolikowski, W. Theoretical study of Čerenkov type second-harmonic generation in periodically poled ferroelectric crystals. J. Opt. Soc. Am. B 2012, 29, 312–318. [Google Scholar] [CrossRef]
- Vyunishev, A.M.; Arkhipkin, V.G.; Chirkin, A.S. Frequency doubling of femtosecond laser pulses in three dimensional nonlinear photonic crystals. Laser Phys. Lett. 2023, 20, 035402. [Google Scholar] [CrossRef]
- Trajtenberg-Mills, S.; Juwiler, I.; Arie, A. On-axis shaping of second-harmonic beams. Laser Photonics Rev. 2015, 9, L40–L44. [Google Scholar] [CrossRef]
- Wang, C.; Chen, P.; Wei, D.; Zhang, L.; Zhang, Z.; Xu, L.; Hu, Y.; Li, J.; Zhang, Y.; Xiao, M.; et al. Sequential Three-Dimensional Nonlinear Photonic Structures for Efficient and Switchable Nonlinear Beam Shaping. ACS Photonics 2023, 10, 456–463. [Google Scholar] [CrossRef]
- Wu, D.; Zhang, Z.; Wang, C.; Zhang, L.; Xu, L.; Wei, D.; Xiong, W.; Li, J.; Hu, Y.; Chu, J.; et al. Generation of nonlinear Airy beams withswitchable acceleration direction. J. Opt. 2023, 25, 07LT01. [Google Scholar] [CrossRef]
- Jin, H.; Xu, P.; Luo, X.W.; Leng, H.Y.; Gong, Y.X.; Yu, W.J.; Zhong, M.L.; Zhao, G.; Zhu, S.N. Compact engineering of path-entangled sources from a monolithic quadratic nonlinear photonic crystal. Phys. Rev. Lett. 2013, 111, 023603. [Google Scholar] [CrossRef]
- Trajtenberg-Mills, S.; Karnieli, A.; Voloch-Bloch, N.; Megidish, E.; Eisenberg, H.S.; Arie, A. Simulating correlations of structured spontaneously down-converted photon pairs. Laser Photonics Rev. 2020, 14, 1900321. [Google Scholar] [CrossRef]
- Wang, A.D.; Zhu, L.; Chen, S.; Du, C.; Mo, Q.; Wang, J. Characterization of LDPC-coded orbital angular momentum modes transmission and multiplexing over a 50-km fiber. Opt. Express 2016, 24, 11716–11726. [Google Scholar] [CrossRef]
- Yamada, M.; Nada, N.; Saitoh, M.; Watanabe, K. First order quasi-phase matched LiNbO3 waveguide periodically poled by applying an external field for efficient blue second harmonic generation. Appl. Phys. Lett. 1993, 62, 435–436. [Google Scholar] [CrossRef]
- Broderick, N.G.R.; Ross, G.W.; Offerhaus, H.L.; Richardson, D.J.; Hanna, D.C. Hexagonally poled lithium niobate: A two-dimensional nonlinear photonic crystal. Phys. Rev. Lett. 2000, 84, 4345–4348. [Google Scholar] [CrossRef]
- Mizuuchi, K.; Morikawa, A.; Sugita, T.; Yamamoto, K. Electric-field poling in Mg-doped LiNbO3. J. Appl. Phys. 2004, 96, 6585–6590. [Google Scholar] [CrossRef]
- Zhang, B.; Wang, L.; Chen, F. Recent advances in femtosecond laser processing of LiNbO3 crystals fir photonics applications. Laser Photonics Rev. 2000, 14, 1900407. [Google Scholar] [CrossRef]
- Lin, J.T.; Bo, F.; Cheng, Y.; Xu, J.J. Advances in on-chip photonic devices based on lithium niobate on insulator. Photonics Res. 2020, 8, 1910–1936. [Google Scholar] [CrossRef]
- Stoian, R.; Amico, C.D.; Bhuyan, M.K.; Cheng, G. [INVITED] Ultrafast laser photoinscription of large-mode-area waveguiding structures in bulk dielectrics. Opt. Laser Technol. 2016, 80, 98–103. [Google Scholar] [CrossRef]
- Stuart, B.C.; Feit, M.D.; Herman, S.; Rubenchik, A.M.; Shore, B.W.; Perry, M.D. Nanosecond-to-femtosecond laser-induced breakdown in dielectrics. Phys. Rev. B 1996, 53, 1749. [Google Scholar] [CrossRef]
- Qiu, J.; Miura, K.; Hirao, K. Three-dimensional optical memory using glasses as a recording medium through a multi-photon absorption process. Jpn. J. Appl. Phys. 1998, 37, 2263. [Google Scholar] [CrossRef]
- Chan, J.W.; Huser, T.; Risbud, S.; Krol, D.M. Structural changes in fused silica after exposure to focused femtosecond laser pulses. Opt. Lett. 2001, 26, 1726–1728. [Google Scholar] [CrossRef]
- Gorelik, T.; Will, M.; Nolte, S.; Tuennermann, A.; Glatzel, U. Transmission Electron Microscopy Studies of Femtosecond Laser Induced Modifications in Quartz. Appl. Phys. A 2003, 76, 309–311. [Google Scholar] [CrossRef]
- Couairon, A.; Sudrie, L.; Franco, M.; Prade, B.; Mysyrowicz, A. Filamentation and damage in fused silica induced by tightly focused femtosecond laser pulse. Phys. Rev. B 2005, 71, 125435. [Google Scholar] [CrossRef]
- Reichman, W.J.; Chan, J.W.; Smelser, S.W.; Mihailov, S.J.; Krol, D.M. Spectroscopic characterization of different femtosecond laser modification regimes in fused silica. J. Opt. Soc. Am. B 2007, 24, 1627–1632. [Google Scholar] [CrossRef]
- Little, D.J.; Ams, M.; Dekker, P.; Marshall, G.D.; Dawes, J.M.; Withford, M.J. Femtosecond laser modification of fused silica: The effect of writing polarization on Si-O ring structure. Opt. Express 2008, 16, 20029–20037. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.; Shimizu, M.; Zhu, B.; Dai, Y.; Qian, B.; Qiu, J.R.; Shimotsuma, Y.; Miura, K.; Hirao, K. Micromodification of element distribution in glass using femtosecond laser irradiation. Opt. Lett. 2009, 34, 136–138. [Google Scholar] [CrossRef] [PubMed]
- Mishchik, K.; Cheng, G.; Huo, G.; Burakov, I.M.; Mauclair, C.; Mermillod-Blondin, A.; Rosenfeld, A.; Ouerdane, Y.; Boukenter, A.; Parriaux, O.; et al. Nanosize structural modifications with polarization functions in ultrafast laser irradiated bulk fused silica. Opt. Express 2010, 18, 24809–24824. [Google Scholar] [CrossRef] [PubMed]
- Lancry, M.; Poumellec, B.; Chahid-Erraji, A.; Beresna, M.; Kazansky, P.G. Dependence of the femtosecond laser refractive index change thresholds on the chemical composition of doped-silica glasses. Opt. Mater. Express 2011, 1, 711–723. [Google Scholar] [CrossRef]
- Toney Fernandez, T.; Haro-González, P.; Sotillo, B.; Hernandez, M.; Jaque, D.; Fernandez, P.; Domingo, C.; Siegel, J.; Solis, J. Ion migration assisted inscription of high refractive index contrast waveguides by femtosecond laser pulses in phosphate glass. Opt. Lett. 2013, 38, 5248–5251. [Google Scholar] [CrossRef]
- Stuart, B.C.; Feit, M.D.; Rubenchik, A.M.; Shore, B.W.; Perry, M.D. Laser-Induced Damage in Dielectrics with Nanosecond to Subsecond Pulses. Phys. Rev. Lett. 1995, 74, 2248–2251. [Google Scholar] [CrossRef]
- Yamada, K.; Watanabe, W.; Toma, T.; Itoh, K. In situ observation of photoinduced refractive-index changes in filaments formed in glasses by femtosecond laser pulses. Opt. Lett. 2001, 26, 19–21. [Google Scholar] [CrossRef]
- Ponader, C.W.; Schroeder, J.F.; Streltsov, A.M. Origin of the refractive-index increase in laser-written waveguides in glasses. J. Appl. Phys. 2008, 103, 063516. [Google Scholar] [CrossRef]
- Glezer, E.N.; Milosavljevic, M.; Huang, L.; Finlay, R.J.; Her, T.-H.; Callan, J.P.; Mazur, E. Three-Dimensional Optical Storage Inside Transparent Materials. Opt. Lett. 1996, 21, 2023–2025. [Google Scholar] [CrossRef] [PubMed]
- Eberlea, G.; Schmidtb, M.; Pudec, F.; Wegenera, K. Laser surface subsurface of sapphireusing femtosecond pulses. Appl. Surface Sci. 2016, 378, 504–512. [Google Scholar] [CrossRef]
- Kroesen, S.; Horn, W.; Imbrock, J.; Denz, C. Electro-optical tunable waveguide embeded multiscan Bragg grattings in Lithium niobate by direct femtosecond laser writting. Opt. Express 2014, 22, 23339–23348. [Google Scholar] [CrossRef]
- Dai, Z.; Su, Q.; Wang, Y.; Qi, P.; Wang, X.; Liu, W. Fast fabrication of THz devices by femtosecond laser direct writing with a galvanometer scanner. Laser Phys. 2019, 29, 065301. [Google Scholar] [CrossRef]
- Shivakumar, V.B.; Jedrkiewicz, O.; Hadden, J.P.; Sotillo, B.; Vázquez, M.R.; Dentella, P.; Fernandez, T.T.; Chiappini, A.; Giakoumaki, A.N.; Phu, T.L.; et al. Femtosecond laser written photonic and microfluidic circuits in diamond. J. Phys. Photonics 2019, 1, 022001. [Google Scholar]
- Davis, K.M.; Miura, K.; Sugimoto, N.; Hirao, K. Writing waveguides in glass with a femtosecond laser. Opt. Lett. 1996, 21, 1729–1731. [Google Scholar] [CrossRef] [PubMed]
- Miura, K.; Qiu, J.R.; Inouye, H.; Mitsuyu, T. Photowritten optical waveguides in various glasses with ultrashort pulse laser. Appl. Phys. Lett. 1997, 71, 3329–3331. [Google Scholar] [CrossRef]
- Burghoff, J.; Grebing, C.; Nolte, S.; Tünnermann, A. Waveguides in lithium niobate fabricated by focused ultrashort laser pulses. Appl. Surface Sci. 2007, 253, 7899–7902. [Google Scholar] [CrossRef]
- Burghoff, J.; Nolte, S.; Tünnermann, A. Origins of waveguiding in femtosecond laser-structured LiNbO3. Appl. Phys. A 2007, 89, 127–132. [Google Scholar] [CrossRef]
- Campbell, S.; Thomson, R.R.; Hand, D.P.; Kar, A.K.; Reid, D.T.; Canalias, C.; Pasiskevicius, V.; Laurell, F. Frequency-doubling in femtosecond laser inscribed periodically-poled potassium titanyl phosphate waveguides. Opt. Express 2007, 15, 17146–17150. [Google Scholar] [CrossRef] [PubMed]
- Jia, Y.C.; Vázquez de Aldana, J.R.; Lu, Q.M.; Jaque, D.; Chen, F. Second harmonic generation of violet light in femtosecond-laser-inscribed BiB3O6 cladding waveguides. Opt. Mater. Express 2013, 3, 1279–1284. [Google Scholar] [CrossRef]
- Hasse, K.; Calmano, T.; Deppe, B.; Liebald, C.; Kränkel, C. Efficient Yb+3CaGdAlO4 bulk and Femtosecond Laser-written Waveguide Lasers. Opt. Lett. 2015, 40, 3552–3555. [Google Scholar] [CrossRef] [PubMed]
- Mishchik, K.; D’Amico, C.; Velpula, P.K.; Mauclair, C.; Boukenter, A.; Ouerdane, Y.; Stoiana, R. Ultrafast laser induced electronic and structural modifications in bulk fused silica. J. Appl. Phys. 2013, 114, 133502. [Google Scholar] [CrossRef]
- Glezer, E.N.; Mazur, E. Ultrafast-laser driven micro-explosions in transparent materials. Appl. Phys. Lett. 1997, 71, 882–884. [Google Scholar] [CrossRef]
- Thomas, J.; Hilbert, V.; Geiss, R.; Pertsch, T.; Tu¨nnermann, A.; Nolte, S. Quasi phase matching in femtosecond pulse volume structured x-cut lithium niobate. Laser Photonics Rev. 2013, 7, L17–L20. [Google Scholar] [CrossRef]
- Kroesen, S.; Tekce, K.; Imbrock, J.; Denz, C. Monolithic fabrication of quasi phase-matched waveguides by femtosecond laser structuring the χ(2) nonlinearity. Appl. Phys. Lett. 2015, 107, 101109. [Google Scholar] [CrossRef]
- Imbrock, J.; Wesemann, L.; Kroesen, S.; Ayoub, M.; Denz, C. Waveguide-integrated three-dimensional quasi-phase-matching structures. Optica 2020, 7, 28–34. [Google Scholar] [CrossRef]
- Wei, D.; Wang, C.; Wang, H.; Hu, X.; Wei, D.; Fang, X.; Zhang, Y.; Wu, D.; Hu, Y.; Li, J.; et al. Experimental demonstration of a three-dimensional lithium niobate nonlinear photonic crystal. Nat. Photonics 2018, 12, 596–600. [Google Scholar] [CrossRef]
- Wei, D.; Wang, C.; Xu, X.; Wang, H.; Hu, Y.; Chen, P.; Li, J.; Zhu, Y.; Xin, C.; Hu, X.; et al. Efficient nonlinear beam shaping in three-dimensional lithium niobate nonlinear photonic crystals. Nat. Commun. 2019, 10, 4193. [Google Scholar] [CrossRef]
- Zhu, B.; Liu, H.G.; Chen, Y.P.; Chen, X.F. High conversion efficiency second-harmonic beam shaping via amplitude-type nonlinear photoniccrystals. Opt. Lett. 2019, 45, 220–223. [Google Scholar] [CrossRef]
- Zhu, B.; Liu, H.G.; Liu, Y.A.; Yan, X.S.; Chen, Y.P.; Chen, X.F. Second-harmonic computer-generated holographic imaging through monolithic lithium niobate crystal by femtosecond laser micromachining. Opt. Lett. 2020, 45, 4132–4135. [Google Scholar] [CrossRef] [PubMed]
- Shao, M.; Liang, F.; Yu, H.; Zhang, H. Pushing periodic-disorder induced phase matching into the deep-ultraviolet spectral region: Theory and demonstration. Light Sci. Appl. 2020, 9, 45. [Google Scholar] [CrossRef] [PubMed]
- Shao, M.; Liang, F.; Yu, H.; Zhang, H. Angular engineering strategy of an additional periodic phase for widely tunable phase-matched deep-ultraviolet second harmonic generation. Light Sci. Appl. 2022, 11, 2047–7538. [Google Scholar] [CrossRef]
- Shao, M.; Liang, F.; Zhang, Z.; Yu, H.; Zhang, H. Spatial Frequency Manipulation of a Quartz Crystal for Phase-Matched Second-Harmonic Vacuum Ultraviolet Generation. Laser Photonics Rev. 2023, 2300244. [Google Scholar] [CrossRef]
- Yuan, T.; Zhu, B.; Tu, H.; Chen, Y.; Chen, X. Femtosecond laser direct writing quasi-phase matched type-II waveguide in lithium niobate. Opt. Mater. Express 2023, 13, 1–8. [Google Scholar] [CrossRef]
- Fujimura, M.; Sohmura, T.; Suhara, T. Fabrication of domain-inverted gratings in MgO:LiNbO3 by applying voltage under ultraviolet irradiation through photomask at room temperature. Electron. Lett. 2003, 39, 719–721. [Google Scholar] [CrossRef]
- M<i>u</i>¨ller, M.; Soergel, E.; Buse, K. Influence of ultraviolet illumination on the poling characteristics of lithium niobate crystals. Appl. Phys. Lett. 2003, 83, 1824–1826. [Google Scholar]
- Dierolf, V.; Sandmann, C. Direct-write method for domain inversion patterns in LiNbO3. Appl. Phys. Lett. 2004, 84, 3987–3989. [Google Scholar] [CrossRef]
- Wengler, M.C.; Fassbender, B.; Soergel, E.; Buse, K. Impact of ultraviolet light on coercive field, poling dynamics and poling quality of various lithium niobate crystals from different sources. J. Appl. Phys. 2004, 96, 2816–2820. [Google Scholar] [CrossRef]
- Sones, C.L.; Muir, A.C.; Ying, Y.J.; Mails, S.; Eason, R.W.; Jungk, T.; Hoffman, Á.; Soergel, E. Precision nanoscale domain engineering of lithium niobate via UV laser induced inhibition of poling. Appl. Phys. Lett. 2008, 92, 072905. [Google Scholar] [CrossRef]
- Fujimura, M.; Suhara, T. Formation of MgO:LiNbO domain-inverted gratings by voltage application under UV light irradiation at room temperature. Adv. Optoelectron. 2008, 2008, 421054. [Google Scholar] [CrossRef]
- Wang, W.J.; Kong, Y.F.; Liu, H.D.; Hu, Q.; Liu, S.G.; Chen, S.L.; Xu, J.J. Light-induced domain reversal in doped lithium niobate crystals. J. Appl. Phys. 2009, 105, 043105. [Google Scholar] [CrossRef]
- Fujimura, M.; Kitado, E.; Inoue, T.; Suhara, T. MgO:LiNbO3 waveguide quasi-phase-matched second-harmonic generation devices fabricated by two-step voltage application under UV light. IEEE Photonics Tech. L. 2011, 23, 1313–1315. [Google Scholar] [CrossRef]
- Kitado, E.; Fujimura, M.; Suhara, T. Ultraviolet Laser Writing of Ferroelectric-Domain-Inverted Gratings for MgO:LiNbO3 Waveguide Quasi-Phase-Matching Devices. Appl. Phys. Express 2013, 6, 102204. [Google Scholar] [CrossRef]
- Boes, A.; Steigerwald, H.; Yudistira, D.; Sivan, V.; Wade, S.; Mailis, S.; Soergel, E.; Mitchell, A. Ultraviolet laser-induced poling inhibition produces bulk domains in MgO-doped lithium niobate crystals. Appl. Phys. Lett. 2014, 105, 092904. [Google Scholar] [CrossRef]
- Fahy, S.; Merlin, R. Reversal of ferroelectric domains by ultrashort optical pulses. Phys. Rev. Lett. 1994, 73, 1122. [Google Scholar] [CrossRef] [PubMed]
- Valdivia, C.E.; Sones, C.L.; Scott, J.G.; Mailis, S.; Eason, R.W.; Scrymgeour, D.A.; Gopalan, V.; Jungk, T.; Soergel, E.; Clark, I. Nanoscale surface domain formation on the +z face of lithium niobate by pulsed ultraviolet laser illumination. Appl. Phys. Lett. 2005, 86, 022906. [Google Scholar] [CrossRef]
- Zhu, H.S.; Chen, X.F.; Chen, H.G.; Deng, X.W. Formation of domain reversal by direct irradiation with femtosecond laser in lithium niobate. Chin. Opt. Lett. 2009, 7, 169–172. [Google Scholar]
- Lao, H.Y.; Zhu, H.S.; Chen, X.F. Threshold fluence for domain reversal directly induced by femtosecond laser in lithium niobate. Appl. Phys. A 2010, 101, 313–317. [Google Scholar] [CrossRef]
- Chen, X.; Karpinski, P.; Shvedov, V.; Koynov, K.; Wang, B.X.; Trull, J.; Cojocaru, C.; Krolikowski, W.; Sheng, Y. Ferroelectric domain engineering by focused infrared femtosecond pulses. Appl. Phys. Lett. 2015, 107, 141102. [Google Scholar] [CrossRef]
- Chen, X.; Karpinski, P.; Shvedov, V.; Boes, A.; Mitchell, A.; Krolikowski, W.; Sheng, Y. Quasi-phase matching via femtosecond laser-induced domain inversion in lithium niobate waveguides. Opt. Lett. 2016, 41, 2410. [Google Scholar] [CrossRef]
- Imbrock, J.; Hanafi, H.; Ayoub, M.; Denz, C. Local domain inversion in MgO-doped lithium niobate by pyroelectric field-assisted femtosecond laser lithography. Appl. Phys. Lett. 2018, 113, 252901. [Google Scholar] [CrossRef]
- Xu, X.; Wang, T.; Chen, P.; Zhou, C.; Ma, J.; Wei, D.; Wang, H.; Niu, B.; Fang, X.; Wu, D.; et al. Femtosecond laser writing of lithium niobate ferroelectric nanodomains. Nature 2022, 609, 469–501. [Google Scholar] [CrossRef]
- Wang, X.; Cao, Q.; Wang, R.-N.; Cao, X.; Liu, S. Manipulation of ferroelectric domain inversion and growth by optically induced 3D thermoelectric field in lithium niobate. Appl. Phys. Lett. 2022, 121, 181111. [Google Scholar] [CrossRef]
- Maekawa, S.; Tohyama, T.; Barnes, S.E.; Ishihara, S.; Koshibae, W.; Khaliullin, G.; Appendices, D. Thermoelectric Effects. In Physics of Transition Metal Oxides; Cardona, M., Ed.; Springer: Berlin/Heidelberg, Germany, 2004; pp. 323–331. [Google Scholar]
- Kosorotov, V.F.; Kremenchugskij, L.S.; Levash, L.V.; Shchedrina, L.V. Tertiary pyroelectric effect in lithium niobate and lithium tantalate crystals. Ferroelectrics 1986, 70, 27–37. [Google Scholar] [CrossRef]
- Bhatt, R.; Kar, S.; Bartwal, K.S.; Shula, V.; Sen, P.; Sen, P.K.; Wadhawan, V.K. Studies on nonlinear optical properties of ferroelectric MgO-LiNbO3 single crystals. Ferroelectrics 2005, 323, 165–169. [Google Scholar] [CrossRef]
- Reddy, J.N.B.; Elizabeth, S.; Bhat, H.L.; Venkatram, N.; Rao, D.N. Influence of non-stoichiometric defects on nonlinear absorption and refraction in Nd:Zn co-doped lithium niobate. Opt. Mater. 2009, 31, 1022–1026. [Google Scholar] [CrossRef]
- Xu, T.; Switkowski, K.; Chen, X.; Liu, S.; Koynov, K.; Yu, H.; Zhang, H.; Wang, Y.; Sheng, Y.; Krolikowski, W. Three-dimensional nonlinear photonic crystal in ferroelectric barium calcium titanate. Nat. Photonics 2018, 12, 591–595. [Google Scholar] [CrossRef]
- Saltiel, S.M.; Neshev, D.N.; Krolikowski, W.; Voloch-Bloch, N.; Arie, A.; Bang, O.; Kivshar, Y.S. Nonlinear diffraction from a virtual beam. Phys. Rev. Lett. 2010, 104, 083902. [Google Scholar] [CrossRef]
- Zhou, G.; Jesacher, A.; Booth, M.; Wilson, T.; Ródenas, A.; Jaque, D.; Gu, M. Axial birefringence induced focus splitting in lithium niobate. Opt. Express 2009, 17, 17970–17975. [Google Scholar] [CrossRef]
- Karpinski, P.; Shvedov, V.; Krolikowski, W.; Hnatovsky, C. Laser-writing inside uniaxially birefringent crystals: Fine morphology of ultrashort pulseinduced changes in lithium niobate. Opt. Express 2016, 24, 7456–7476. [Google Scholar] [CrossRef]
- Liu, S.; Switkowski, K.; Xu, C.; Tian, J.; Wang, B.; Lu, P.; Krolikowski, W.; Sheng, Y. Nonlinear wavefront shaping with optically induced three-dimensional nonlinear photonic crystals. Nat. Commun. 2019, 10, 3208. [Google Scholar] [CrossRef] [PubMed]
- Liu, D.; Liu, S.; Mazur, L.M.; Wang, B.; Lu, P.; Krolikowski, W.; Sheng, Y. Smart optically induced nonlinear photonic crystals for frequency conversion and control. Appl. Phys. Lett. 2020, 116, 051104. [Google Scholar] [CrossRef]
- Ostrovsky, A.S.; Rickenstorff-Parrao, C.; Arrizŏn, V. Generation of the perfect optical vortex using a liquid-crystal spatial light modulator. Opt. Lett. 2013, 38, 534–536. [Google Scholar] [CrossRef] [PubMed]
- Topuzoski, S. Generation of optical vortices with curved fork-shaped holograms. Opt. Quantum Electron. 2016, 48, 138. [Google Scholar] [CrossRef]
- Liu, S.; Mazur, L.M.; Krolikowski, W.; Sheng, Y. Nonlinear volume holography in 3D nonlinear photonic crystals. Laser Photonics Rev. 2020, 14, 2000224. [Google Scholar] [CrossRef]
- Mazur, L.M.; Liu, S.; Chen, X.; Krolikowski, W.; Sheng, Y. Localized ferroelectric domains via laser poling in monodomain calcium barium niobate crystal. Laser Photonics Rev. 2021, 15, 2100088. [Google Scholar] [CrossRef]
- Imbrock, J.; Szalek, D.; Laubrock, S.; Hanafi, H.; Denz, C. Thermally assisted fabrication of nonlinear photonic structures in lithium niobate with femtosecond laser pulses. Opt. Express 2022, 30, 39340–39352. [Google Scholar] [CrossRef]
- Bhalla, A.S.; Newnham, R.E. Pyroelectric properties and phase transition in TRIS (dimethylammonium) nonabromodiantimonate uppercaseiii. Solid State Commun. 1988, 67, 1079–1083. [Google Scholar]
- Cao, H.; Fang, B.; Xu, H.; Luo, H. Crystal orientation dependence of dielectric and piezoelectric properties of tetragonal Pb(Mg1/3Nb2/3)O3–38%PbTiO3 single crystal. Mater. Res. Bull. 2002, 37, 2135–2143. [Google Scholar] [CrossRef]
- Gopalan, V.; Mitchell, T.E.; Furukawa, Y.; Kitamura, K. The role of nonstoichiometry in 180∘ domain switching of LiNbO3 crystals. Appl. Phys. Lett. 1998, 72, 1981–1983. [Google Scholar] [CrossRef]
- Kim, S.; Gopalana, V.; Gruverman, A. Coercive fields in ferroelectrics: A case study in lithium niobate and lithium tantalate. Appl. Phys. Lett. 2002, 80, 2740–2742. [Google Scholar] [CrossRef]
- Chen, X.; Liu, D.; Liu, S.; Mazur, L.M.; Liu, X.; Wei, X.; Xu, Z.; Wang, I.; Sheng, Y.; Wei, Z.; et al. Optical induction and erasure of ferroelectric domains in tetragonal PMN-38PT crystals. Adv. Opt. Mater 2021, 10, 2102115. [Google Scholar] [CrossRef]
- Chen, X.; Mazur, L.M.; Liu, D.; Liu, S.; Liu, X.; Xu, Z.; Wei, X.; Wang, J.; Sheng, Y.; Wei, Z.; et al. Quasi-phase matched second harmonic generation in a PMN-38PT crystal. Opt. Lett. 2022, 47, 2056–2059. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Wen, J.; Zhu, S.N.; Xiao, M. Nonlinear talbot effect. Phys. Rev. Lett. 2012, 104, 183901. [Google Scholar] [CrossRef] [PubMed]
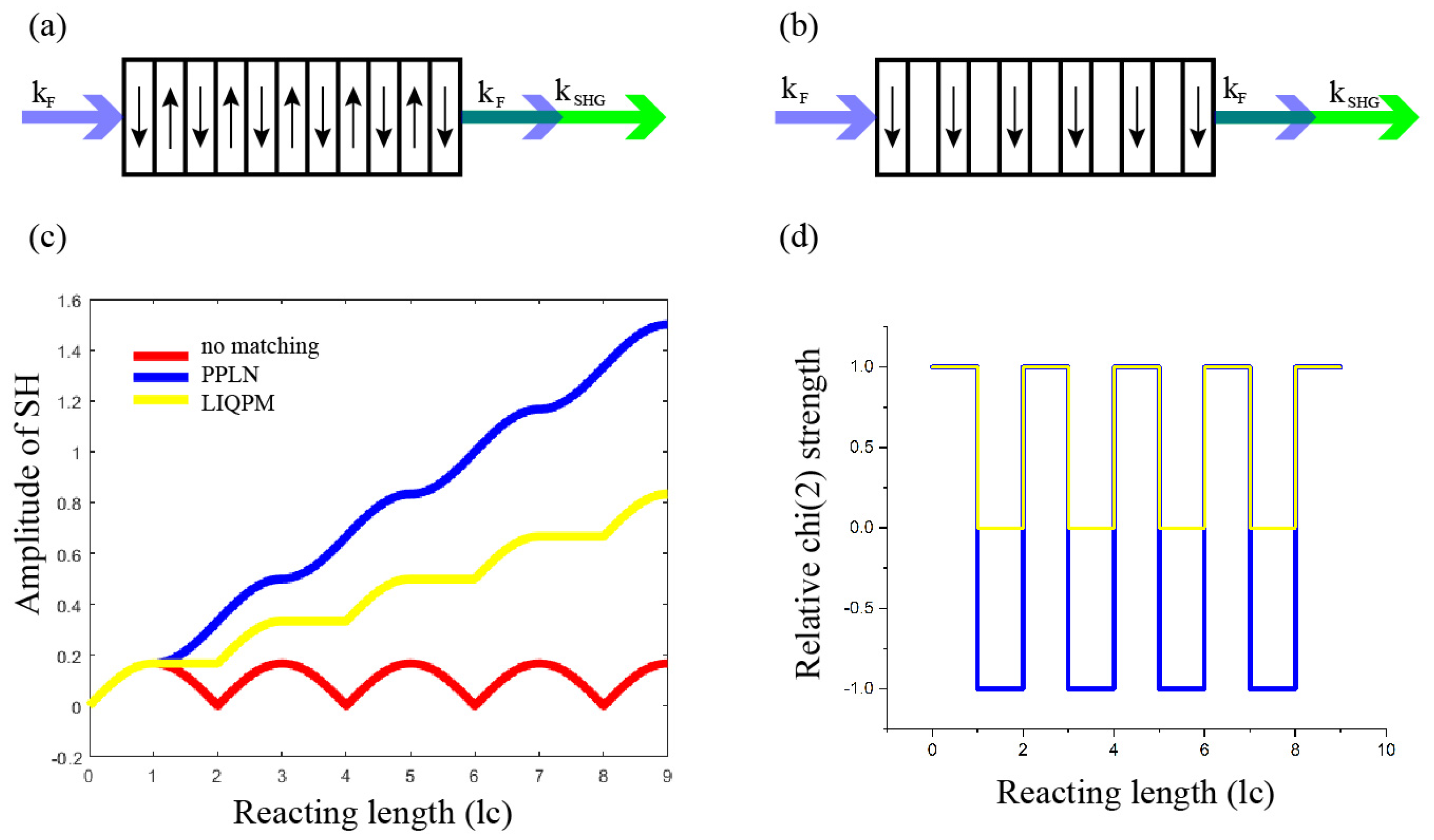
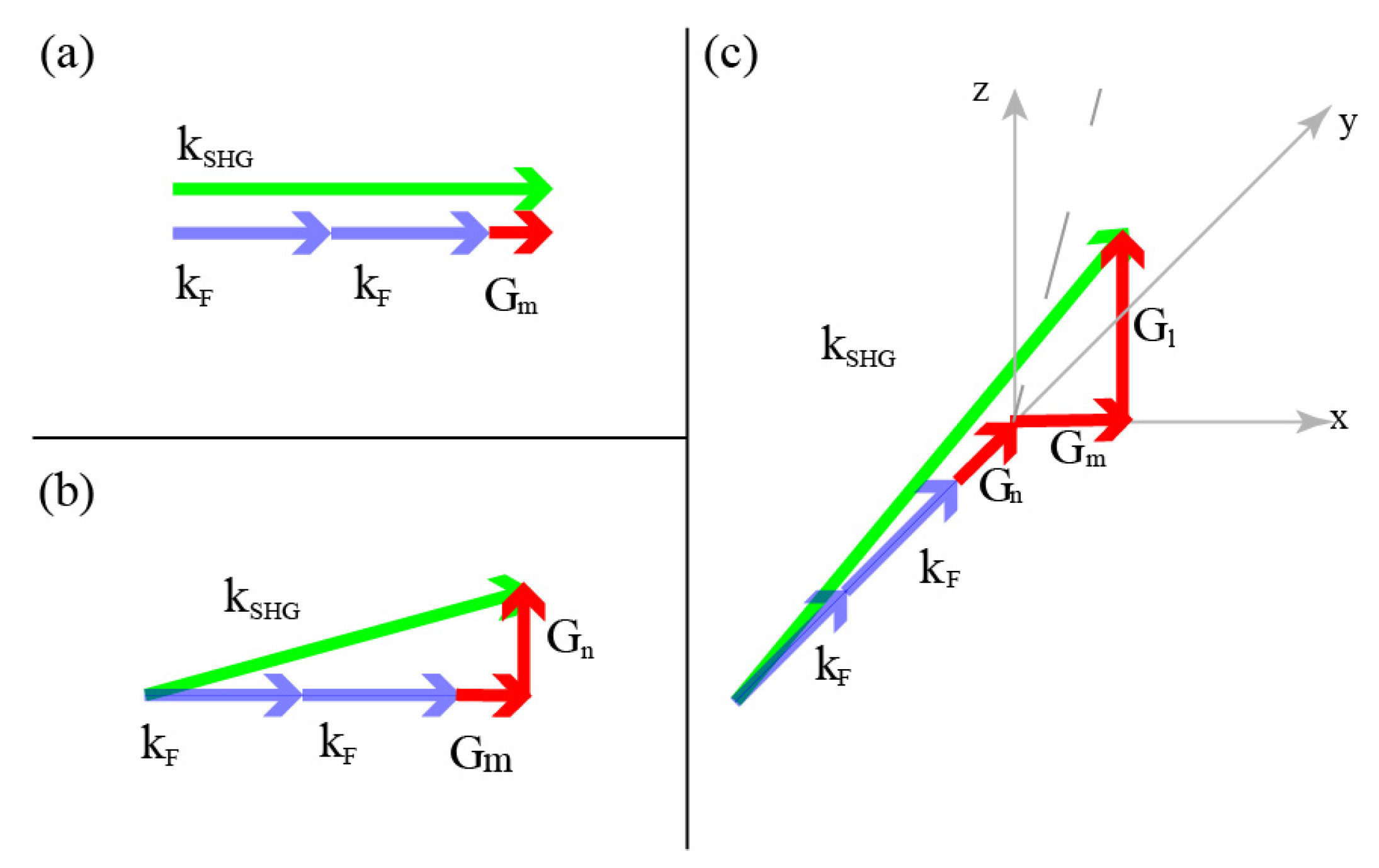

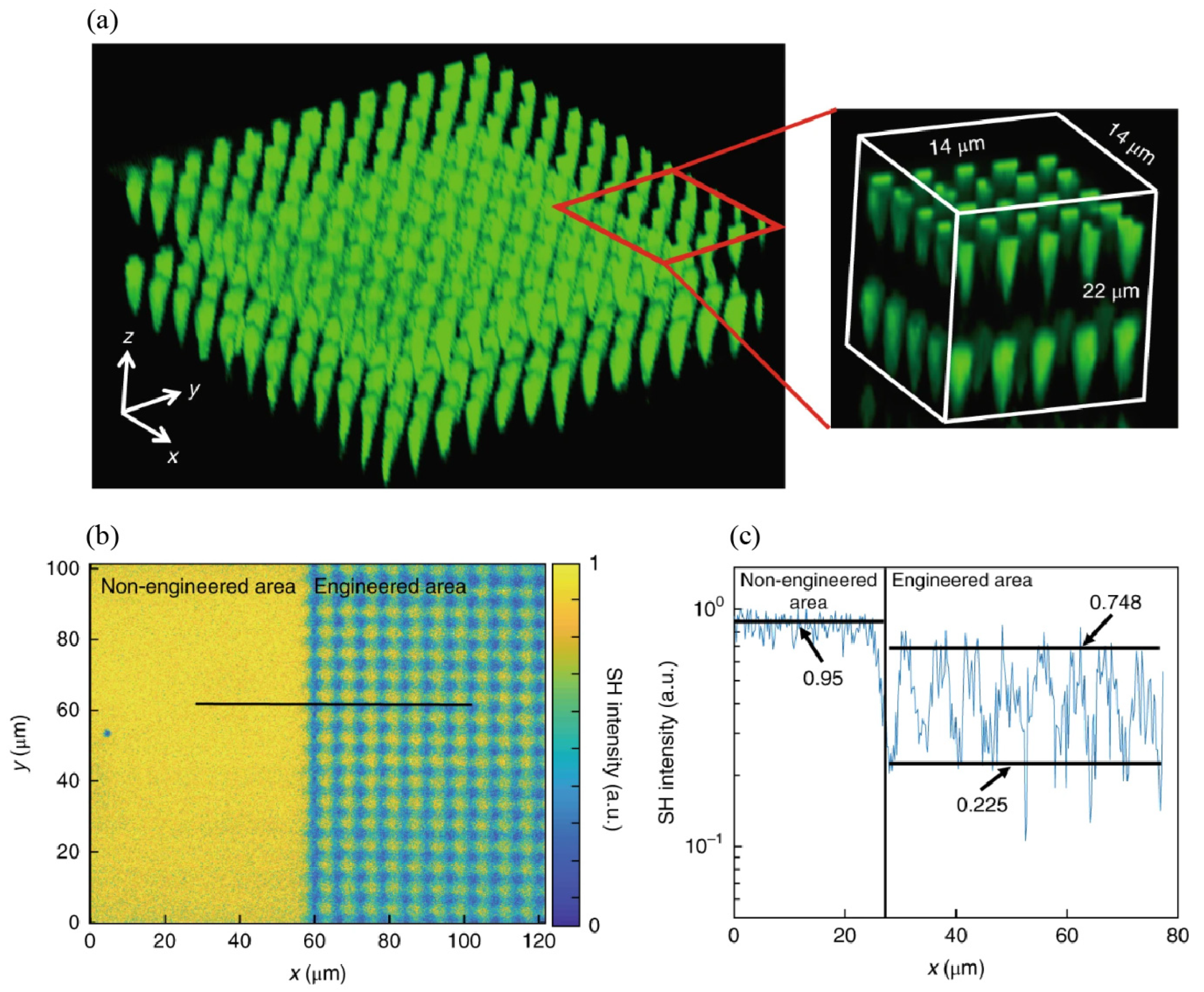

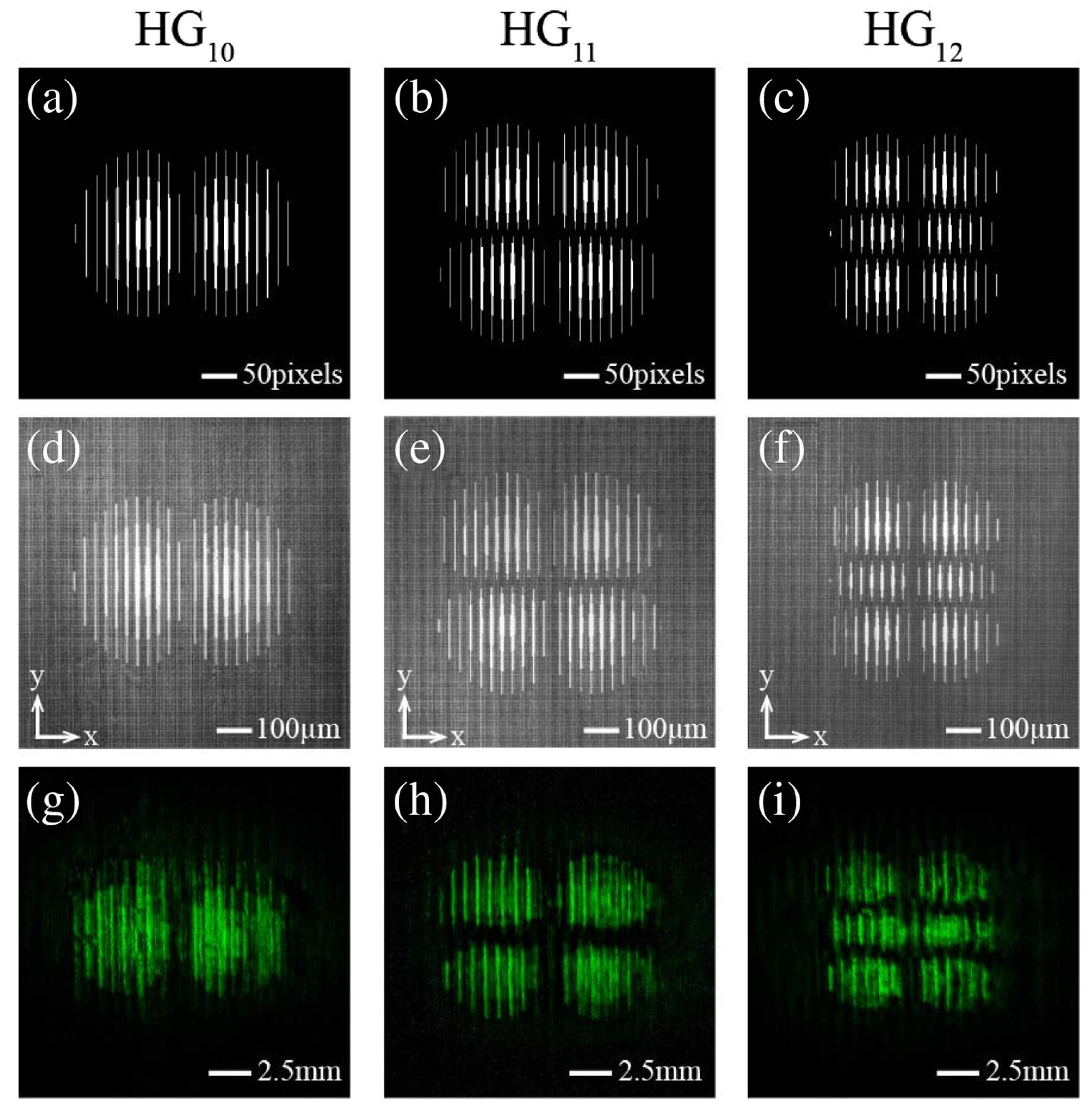

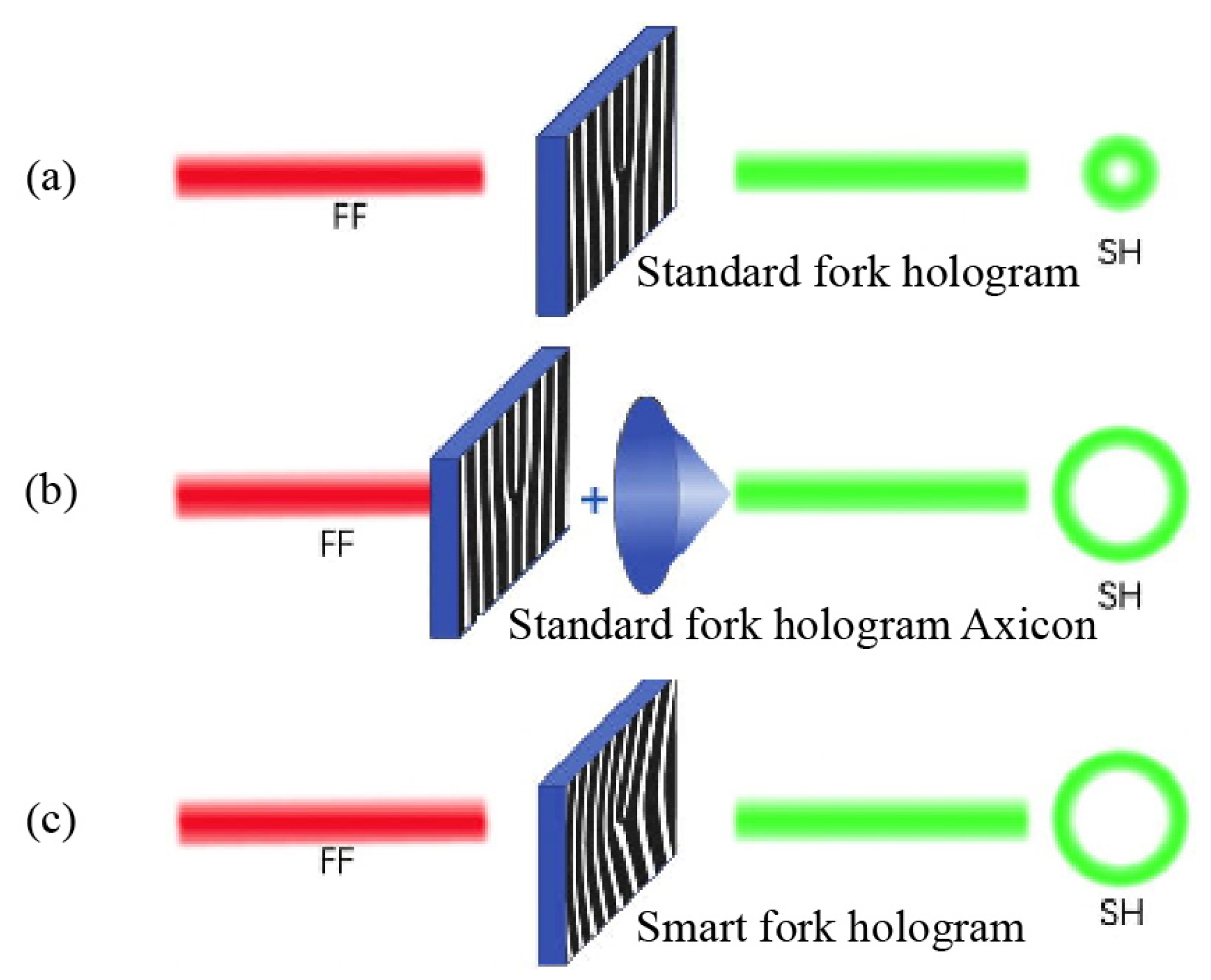



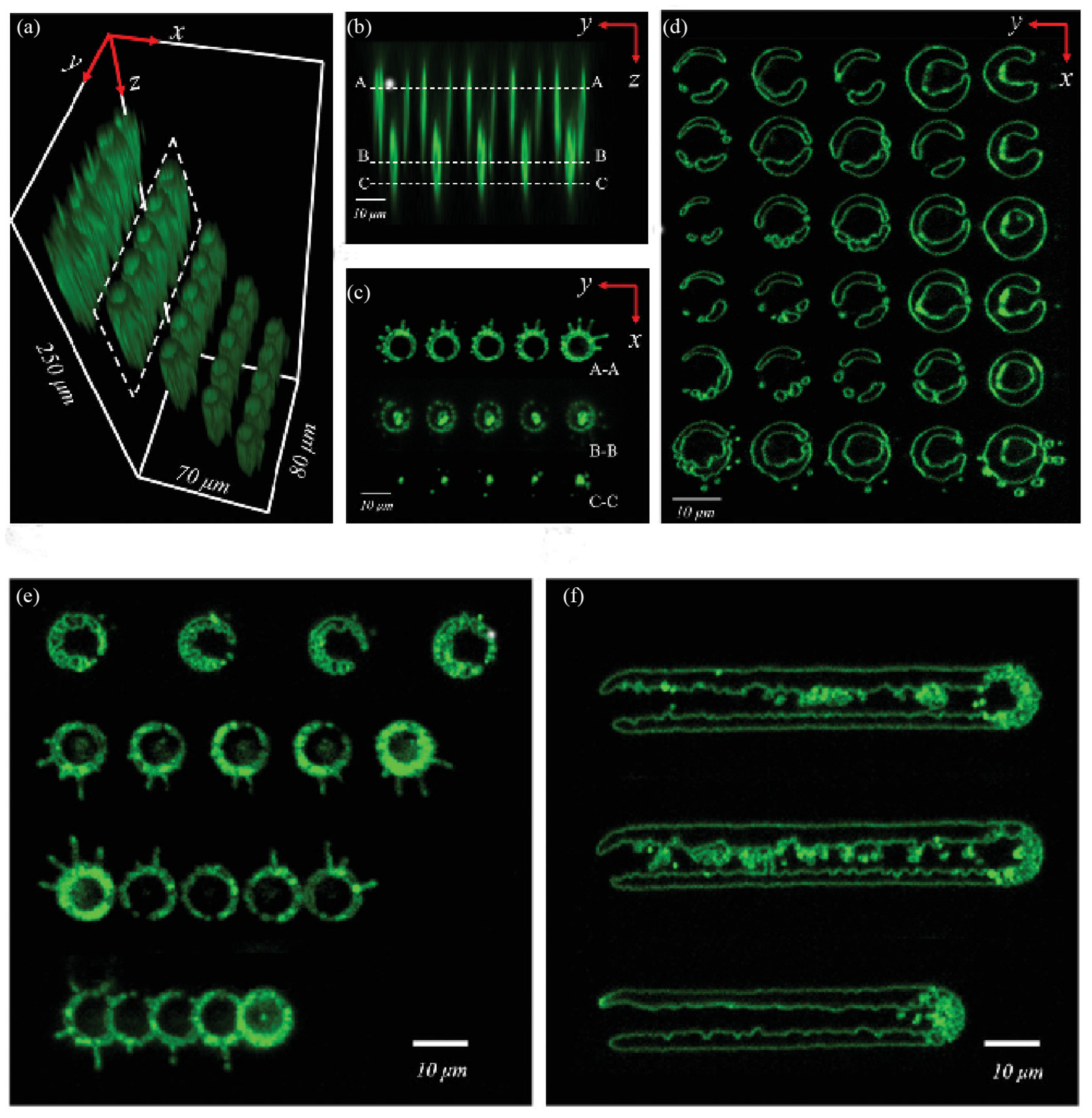
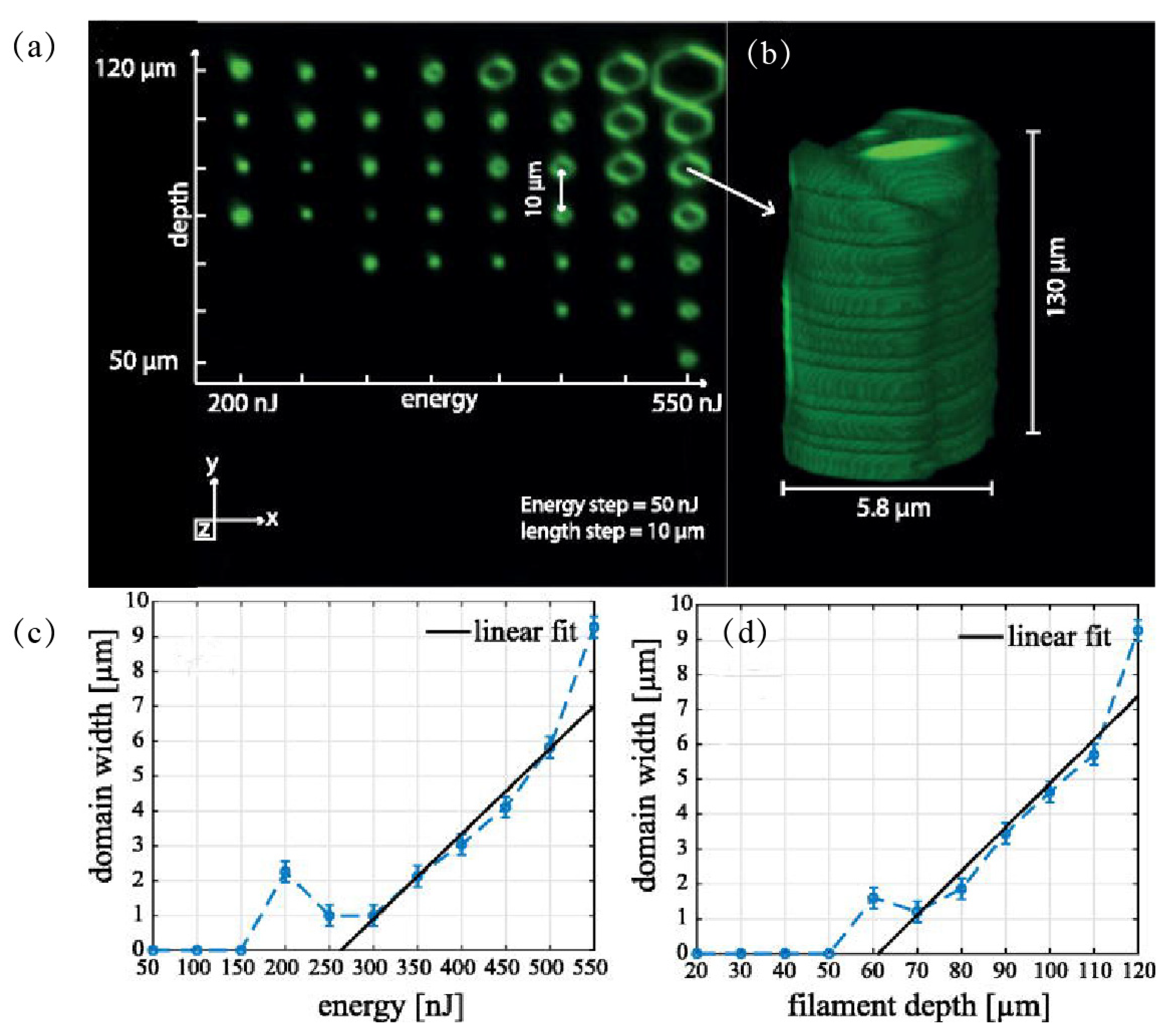
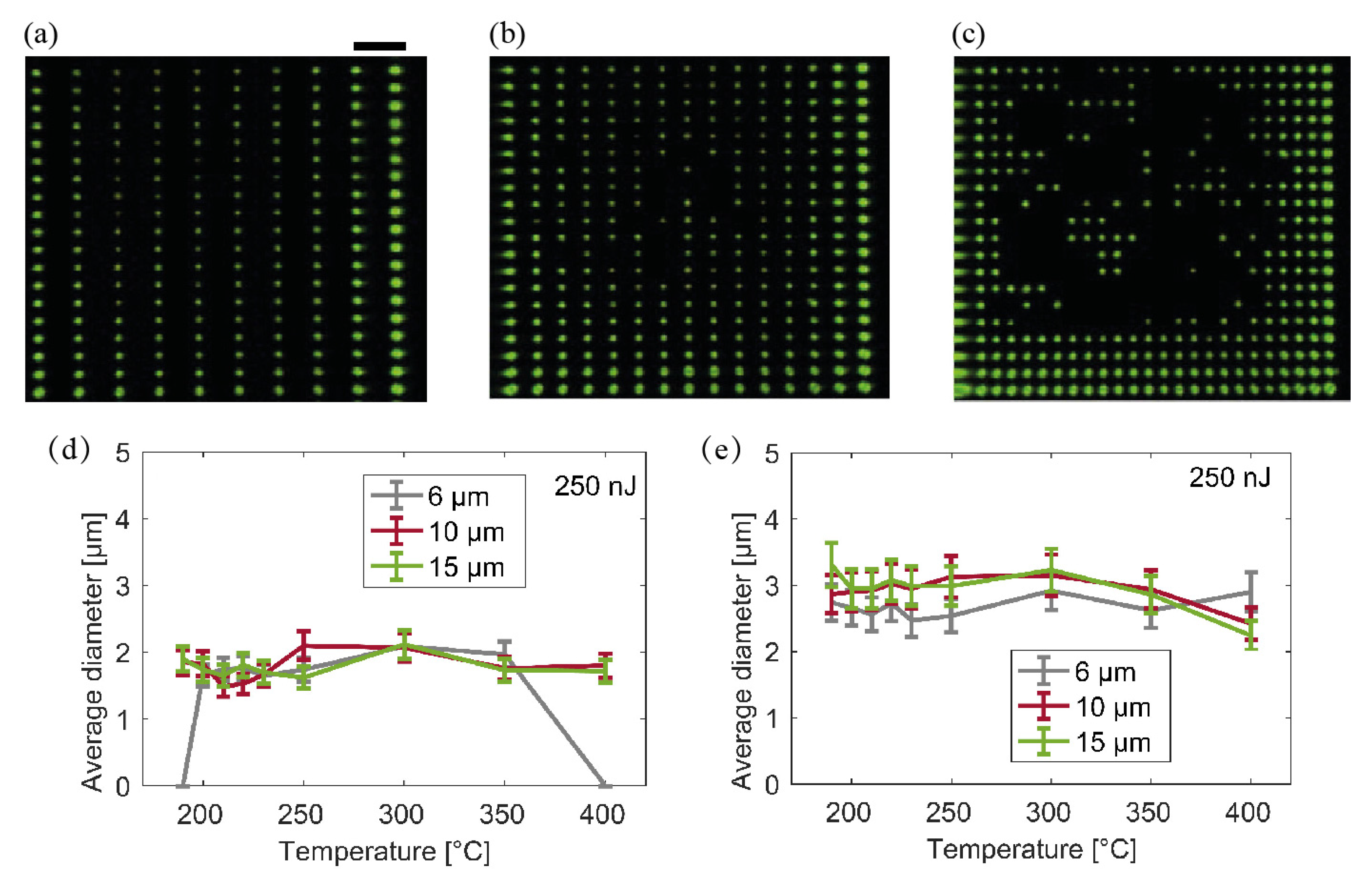
| -Erasing | Reference | Nonlinear Crystal | Repeat Frequency | Bandwidth | Wavelength | Pulse Energy | Scan Speed | N.A. |
|---|---|---|---|---|---|---|---|---|
| Ref. [79] | LiNbO | 100 kHz | 170 fs | 800 nm | 650 nJ | 1 mm/s | 0.5 | |
| Ref. [80] | LiNbO | 1 kHz | 120 fs | 800 nm | 60–72 nJ | 80 m/s | 0.8 | |
| Ref. [82] | LiNbO | 1 kHz | 104 fs | 800 nm | 100–200 nJ | 55–100 m/s | 0.8 | |
| Ref. [84] | MgO-doped LiNbO | 1 kHz | 500 fs | 1030 nm | 900 nJ | 280 m/s | 0.5 | |
| Ref. [86] | LiNbO | 200 kHz | 350 fs | 1040 nm | 16/20 nJ | 1 mm/s | 0.3 | |
| Quartz | 8/12 nJ | |||||||
| -Poling | References | Nonlinear Crystal | Repeat Frequency | Bandwidth | Wavelength | Pulse Energy | Scan Speed | N.A. |
| Ref. [104] | LiNbO | 76 MHz | 180 fs | 800 nm | 0–5 nJ | 10 m/s | 0.65 | |
| Ref. [113] | BCT | 76 MHz | 180 fs | 800 nm | ∽6 nJ | 10 m/s | 0.65 | |
| Ref. [117] | CBN | 76 MHz | 180 fs | 800 nm | 3.6–6.6 nJ | 10 m/s | 0.65 | |
| Ref. [128] | PMN-38PT | 80 MHz | 180 fs | 800 nm | 0–5 nJ | 0.4 | ||
| Seeds | Ref. [106] | MgO-doped LiNbO | 1 kHz | 100 fs | 800 nm | 50–500 nJ | 0.8 | |
| LM | Ref. [108] | MgO-doped LiNbO | 1000 kHz | 170 fs | 1026 nm | 150 nJ | 0.42 | |
| LI | 500 kHz | 300–900 nJ |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tian, Y.; Li, Q.; Yan, L.; Cao, X.; Zhao, X. A Brief Review on Nonlinear Photonic Crystals Induced by Direct Femtosecond Laser Writing. Photonics 2023, 10, 833. https://doi.org/10.3390/photonics10070833
Tian Y, Li Q, Yan L, Cao X, Zhao X. A Brief Review on Nonlinear Photonic Crystals Induced by Direct Femtosecond Laser Writing. Photonics. 2023; 10(7):833. https://doi.org/10.3390/photonics10070833
Chicago/Turabian StyleTian, Yaolan, Qingbo Li, Lili Yan, Xiangdong Cao, and Xian Zhao. 2023. "A Brief Review on Nonlinear Photonic Crystals Induced by Direct Femtosecond Laser Writing" Photonics 10, no. 7: 833. https://doi.org/10.3390/photonics10070833
APA StyleTian, Y., Li, Q., Yan, L., Cao, X., & Zhao, X. (2023). A Brief Review on Nonlinear Photonic Crystals Induced by Direct Femtosecond Laser Writing. Photonics, 10(7), 833. https://doi.org/10.3390/photonics10070833





