A High-Quality Optical Sensor with High Resolution Based on Coin-like Resonator
Abstract
1. Introduction
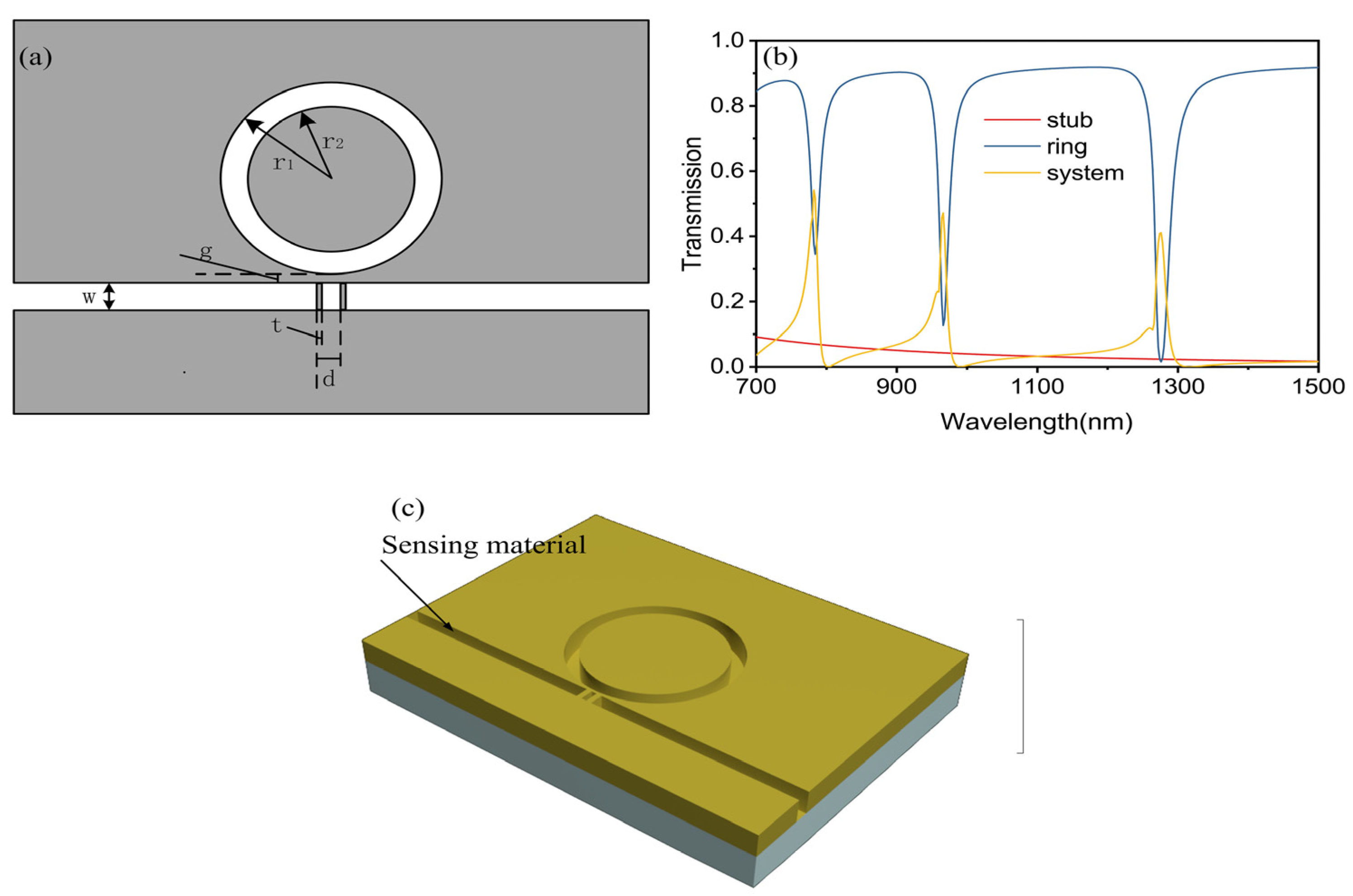
2. Schematic and Theoretical Analysis
3. Results and Discussion
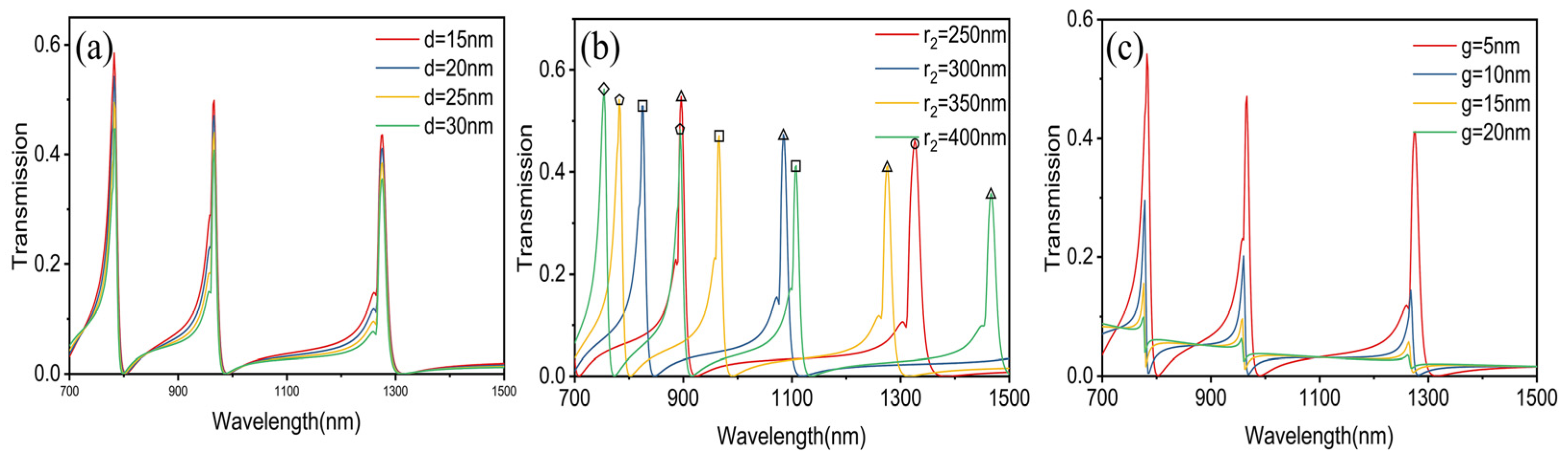
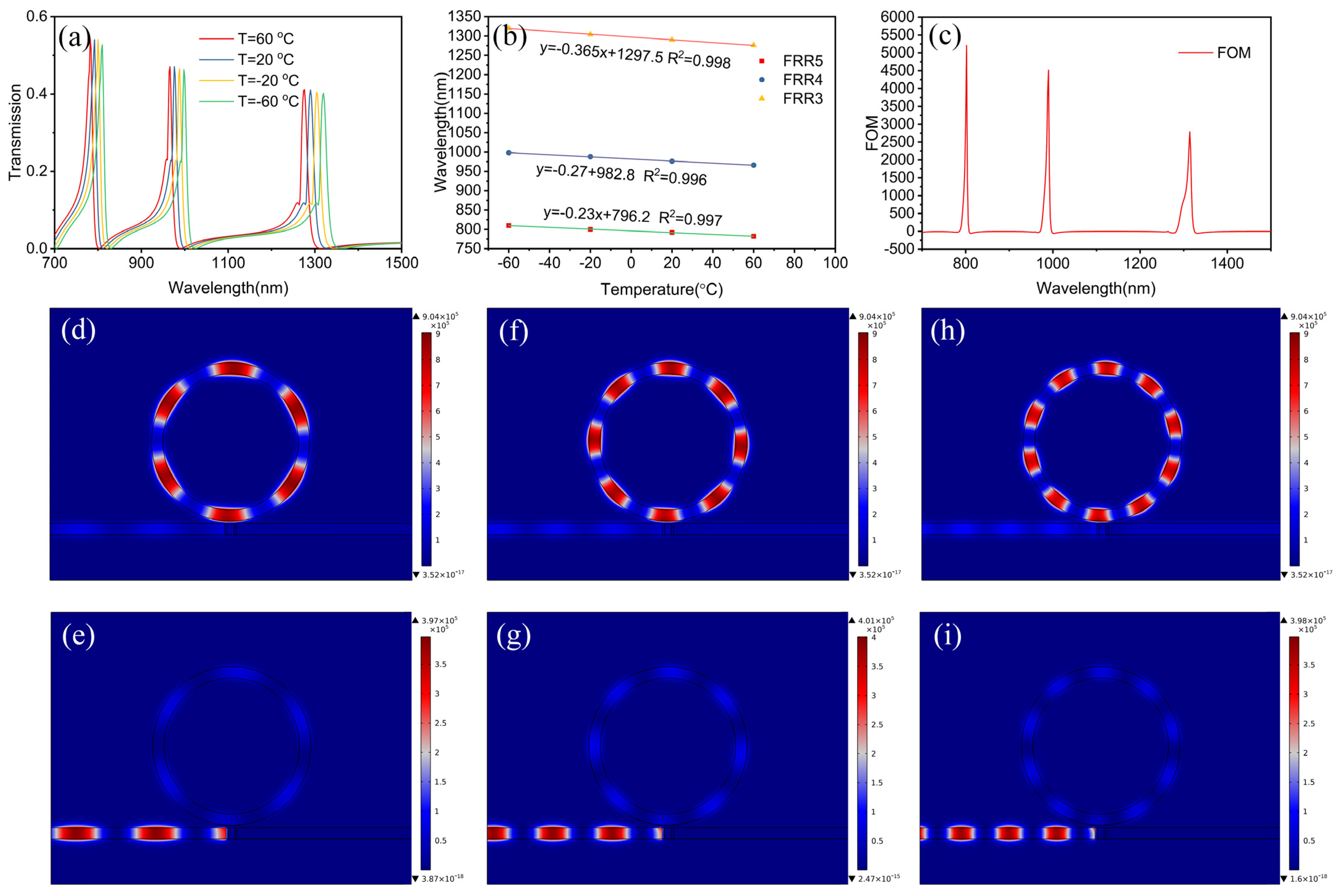
3.1. Ring Resonator with High Sensitivity
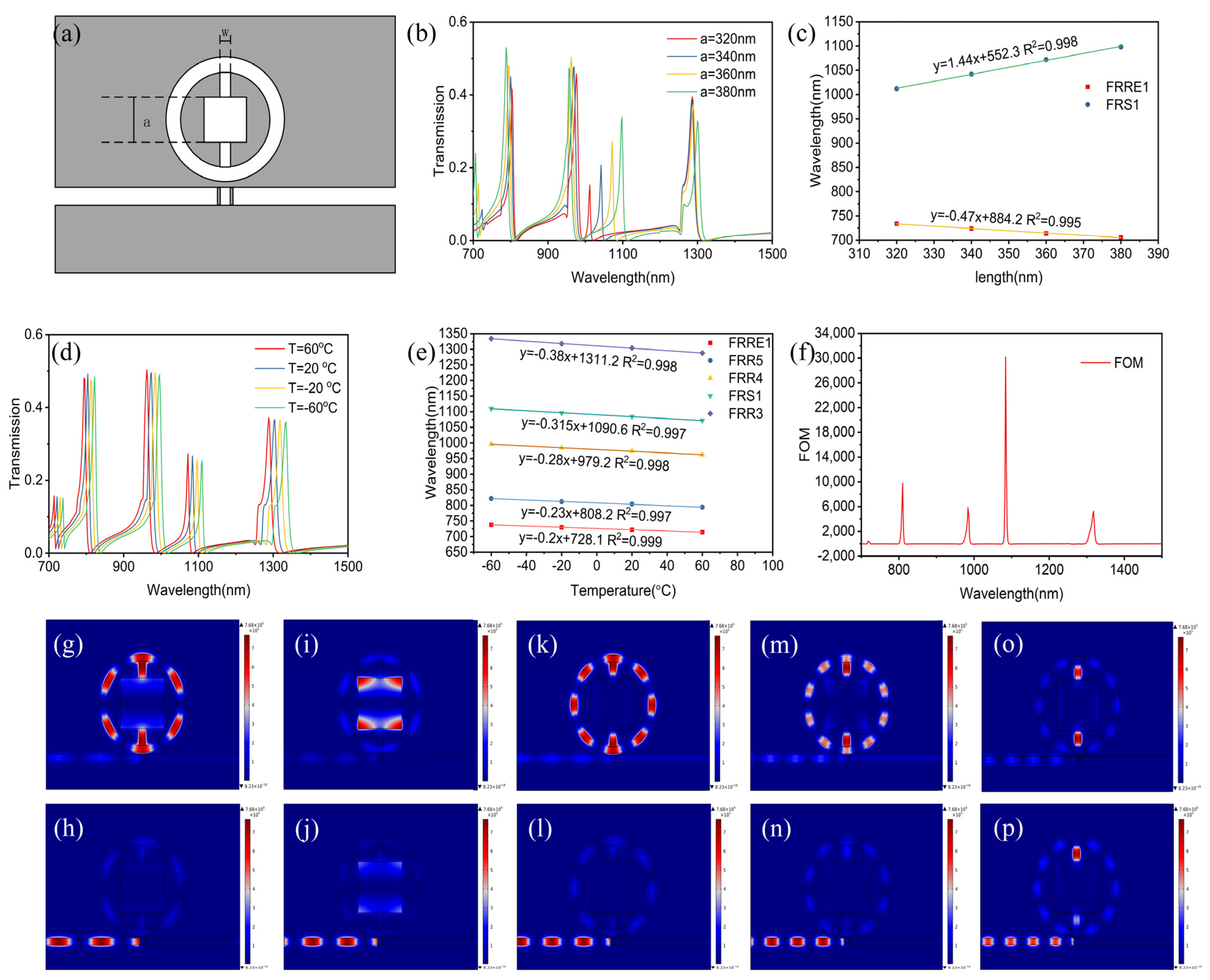
3.2. Coin-like Resonator
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Truong, C.D.; Van, T.N.; Trinh, M.T.; Manh, H.C.; Tan, H.N.; Hoai, B.D. Triple-wavelength filter based on the nanoplasmonic metal-insulator-metal waveguides. Opt. Quantum Electron. 2021, 53, 223. [Google Scholar] [CrossRef]
- Khonina, S.; Kazanskiy, N.; Butt, M.; Ka´zmierczak, A.; Piramidowicz, R. Plasmonic sensor based on metal-insulator-metal waveguide square ring cavity filled with functional material for the detection of CO2 gas. Opt. Express 2021, 29, 16584–16594. [Google Scholar] [CrossRef]
- Pooretemad, S.; Malekijavan, A.; Aslinezhad, M. Ultrawideband bandstop filter based on Fano resonance and rectangular resonators. Appl. Opt. 2021, 60, 4266–4272. [Google Scholar] [CrossRef]
- Karimi, Y.; Kaatuzian, H.; Tooghi, A.; Danaie, M. All-optical plasmonic switches based on Fano resonance in an X-shaped resonator coupled to parallel stubs for telecommunication applications. Optik 2021, 243, 167424. [Google Scholar] [CrossRef]
- Heenkenda, R.; Hirakawa, K.; Sarangan, A. Tunable optical filter using phase change materials for smart IR night vision applications. Opt. Express 2021, 29, 33795–33803. [Google Scholar] [CrossRef]
- Wahsheh, R.A.; Lu, Z.; Abushagur, M.A. Nanoplasmonic couplers and splitters. Opt. Express 2009, 17, 19033–19040. [Google Scholar] [CrossRef] [PubMed]
- Faghani, A.A.; Yaghoubi, E.; Yaghoubi, E. Triple-channel glasses-shape nanoplasmonic demultiplexer based on multi nanodisk resonators in MIM waveguide. Optik 2021, 237, 166697. [Google Scholar] [CrossRef]
- Haffner, C.; Heni, W.; Fedoryshyn, Y.; Niegemann, J.; Melikyan, A.; Elder, D.L.; Baeuerle, B.; Salamin, Y.; Josten, A.; Koch, U.; et al. All-plasmonic Mach–Zehnder modulator enabling optical high-speed communication at the microscale. Nat. Photonics 2015, 9, 525–528. [Google Scholar] [CrossRef]
- Karimi, Y.; Kaatuzian, H. Low-Power Fano Resonance-Based MIM Plasmonic Switch Using Kerr-Type Nonlinear Material. In Proceedings of the 2021 29th Iranian Conference on Electrical Engineering (ICEE), Tehran, Iran, 18–20 May 2021; pp. 32–35. [Google Scholar]
- Li, J.; Chen, J.; Liu, X.; Tian, H.; Wang, J.; Cui, J.; Rohimah, S. Optical sensing based on multimode Fano resonances in metal-insulator-metal waveguide systems with X-shaped resonant cavities. Appl. Opt. 2021, 60, 5312–5319. [Google Scholar] [CrossRef] [PubMed]
- Hassan, M.F.; Sagor, R.H.; Tathfif, I.; Rashid, K.S.; Radoan, M. An optimized dielectric-metal-dielectric refractive index nanosensor. IEEE Sens. J. 2020, 21, 1461–1469. [Google Scholar] [CrossRef]
- Liu, Z.; Gao, Y. Tunable ultra-narrow band band-stop filter based on metal–insulator–metal plasmonic waveguide with square resonator. Opt. Commun. 2021, 501, 127395. [Google Scholar] [CrossRef]
- Hessler, S.; Knopf, S.; Rommel, M.; Girschikofsky, M.; Schmauss, B.; Hellmann, R. Advancing the sensitivity of integrated epoxy-based Bragg grating refractometry by high-index nanolayers. Opt. Lett. 2020, 45, 5510–5513. [Google Scholar] [CrossRef] [PubMed]
- Saha, N.; Brunetti, G.; Kumar, A.; Armenise, M.N.; Ciminelli, C. Highly sensitive refractive index sensor based on polymer bragg grating: A case study on extracellular vesicles detection. Biosensors 2022, 12, 415. [Google Scholar] [CrossRef] [PubMed]
- Zhang, B.H.; Wang, L.L.; Li, H.J.; Zhai, X.; Xia, S.X. Two kinds of double Fano resonances induced by an asymmetric MIM waveguide structure. J. Opt. 2016, 18, 065001. [Google Scholar] [CrossRef]
- Li, X.; Wang, D.; Wang, S.; Yuan, L.; Lei, J.; Li, X. Enhanced plasmonic-induced absorption using a cascade scheme and its application as refractive-index sensor. Photonic Sens. 2020, 10, 162–170. [Google Scholar] [CrossRef]
- Rashid, K.S.; Tathfif, I.; Yaseer, A.A.; Hassan, M.F.; Sagor, R.H. Cog-shaped refractive index sensor embedded with gold nanorods for temperature sensing of multiple analytes. Opt. Express 2021, 29, 37541–37554. [Google Scholar] [CrossRef]
- Rakhshani, M.R. Refractive index sensor based on dual side-coupled rectangular resonators and nanorods array for medical applications. Opt. Quantum Electron. 2021, 53, 232. [Google Scholar] [CrossRef]
- Shen, S.; She, S.; Wang, Z.; Tan, Q.; Xiong, J.; Zhang, W. MIM waveguide structure consisting of two triangle stubs, side-coupled with an eight-like resonant cavity. Opt. Commun. 2021, 495, 127087. [Google Scholar] [CrossRef]
- Su, C.; Zhu, J. Novel SPR sensor based on MIM-based waveguide and an asymmetric cross-shaped resonator. Plasmonics 2021, 16, 769–775. [Google Scholar] [CrossRef]
- Rakhshani, M.R.; Mansouri-Birjandi, M.A. High-sensitivity plasmonic sensor based on metal–insulator–metal waveguide and hexagonal-ring cavity. IEEE Sens. J. 2016, 16, 3041–3046. [Google Scholar] [CrossRef]
- Fitrakis, E.P.; Kamalakis, T.; Sphicopoulos, T. Slow light in insulator–metal–insulator plasmonic waveguides. JOSA B 2011, 28, 2159–2164. [Google Scholar] [CrossRef]
- Dionne, J.A.; Verhagen, E.; Polman, A.; Atwater, H.A. Are negative index materials achievable with surface plasmon waveguides? A case study of three plasmonic geometries. Opt. Express 2008, 16, 19001–19017. [Google Scholar] [CrossRef]
- Ghasemi, M.R.; Bayati, M.S. Proposal for metal–insulator–metal plasmonic power splitter and demultiplexer suitable for implementation in optical switches. IET Optoelectron. 2021, 15, 200–206. [Google Scholar] [CrossRef]
- Butt, M.A.; Khonina, S.N.; Kazanskiy, N.L. A compact design of a modified Bragg grating filter based on a metal-insulator-metal waveguide for filtering and temperature sensing applications. Optik 2022, 251, 168466. [Google Scholar] [CrossRef]
- Tathfif, I.; Yaseer, A.A.; Rashid, K.S.; Sagor, R.H. Metal-insulator-metal waveguide-based optical pressure sensor embedded with arrays of silver nanorods. Opt. Express 2021, 29, 32365–32376. [Google Scholar] [CrossRef] [PubMed]
- Srivastava, A.; Verma, A.; Prajapati, Y.K. Theoretical study of hazardous carbon-di-oxide gas sensing using MIM structure-based SPR sensing scheme. IET Optoelectron. 2021, 15, 167–177. [Google Scholar] [CrossRef]
- Zhou, P.; Liang, K.; Wang, Y.; Sun, Q.A.; Guo, J.; Jin, L.; Yu, L. Research on A High-Sensitivity Temperature Sensor with Multi-Indicator Based on Nano-Cylinder-Loaded Ring Resonator. Photonics 2023, 10, 69. [Google Scholar] [CrossRef]
- Fan, H.; Fan, H.; Fan, H. Multiple Fano resonance refractive index sensor based on a plasmonic metal-insulator-metal based Taiji resonator. JOSA B 2022, 39, 32–39. [Google Scholar] [CrossRef]
- Chen, J.; Li, J.; Liu, X.; Rohimah, S.; Tian, H.; Qi, D. Fano resonance in a MIM waveguide with double symmetric rectangular stubs and its sensing characteristics. Opt. Commun. 2021, 482, 126563. [Google Scholar] [CrossRef]
- Wang, Y.; Li, S.; Zhang, Y.; Yu, L. Independently formed multiple Fano resonances for ultra-high sensitivity plasmonic nanosensor. Plasmonics 2018, 13, 107–113. [Google Scholar] [CrossRef]
- Khani, S.; Hayati, M. Optical sensing in single-mode filters base on surface plasmon H-shaped cavities. Opt. Commun. 2022, 505, 127534. [Google Scholar] [CrossRef]
- Guo, Z.; Wen, K.; Qin, Y.; Fang, Y.; Li, Z.; Chen, L. A plasmonic refractive-index sensor based multiple Fano resonance multiplexing in slot-cavity resonant system. Photonic Sens. 2022, 12, 175–184. [Google Scholar] [CrossRef]
- Hassan, M.F.; Hasan, M.M.; Radoan, M.; Sagor, R.H. Design and performance analysis of an ultra-compact nano-plasmonic refractive index sensor. In Proceedings of the 2020 8th International Electrical Engineering Congress (iEECON), Chiang Mai, Thailand, 4–6 March 2020; pp. 1–5. [Google Scholar]
- Shahamat, Y.; Ghaffarinejad, A.; Vahedi, M. Plasmon induced transparency and refractive index sensing in two nanocavities and double nanodisk resonators. Optik 2020, 202, 163618. [Google Scholar] [CrossRef]
- Brunetti, G.; Olio, F.D.; Conteduca, D.; Armenise, M.N.; Ciminelli, C. Comprehensive mathematical modelling of ultra-high Q grating-assisted ring resonators. J. Opt. 2020, 22, 035802. [Google Scholar] [CrossRef]
- Liu, K.; Jin, N.; Cheng, H.; Chauhan, N.; Puckett, M.W.; Nelson, K.D.; Behunin, R.O.; Rakich, P.T.; Blumenthal, D.J. Ultralow 0.034 dB/m loss wafer-scale integrated photonics realizing 720 million Q and 380 μW threshold Brillouin lasing. Opt. Lett. 2022, 47, 1855–1858. [Google Scholar] [CrossRef] [PubMed]
| Parameter | Symbol | Value |
|---|---|---|
| Waveguide width | w | 50 nm |
| Inner radius | r1 | 300 nm |
| Outer radius | r2 | 350 nm |
| Waveguide gap width | g | 10 nm |
| The thickness of the stub | t | 10 nm |
| Distance between the stub | d | 20 nm |
| Parameter | Value | Unit |
|---|---|---|
| Dielectric constant | 3.7 | 1 |
| Bulk plasma frequency | 9.1 | eV |
| Electron collision | 0.018 | eV |
| Incident frequency | 1.24 | eV |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, C.; Lv, N. A High-Quality Optical Sensor with High Resolution Based on Coin-like Resonator. Photonics 2023, 10, 641. https://doi.org/10.3390/photonics10060641
Yu C, Lv N. A High-Quality Optical Sensor with High Resolution Based on Coin-like Resonator. Photonics. 2023; 10(6):641. https://doi.org/10.3390/photonics10060641
Chicago/Turabian StyleYu, Congzhi, and Naijing Lv. 2023. "A High-Quality Optical Sensor with High Resolution Based on Coin-like Resonator" Photonics 10, no. 6: 641. https://doi.org/10.3390/photonics10060641
APA StyleYu, C., & Lv, N. (2023). A High-Quality Optical Sensor with High Resolution Based on Coin-like Resonator. Photonics, 10(6), 641. https://doi.org/10.3390/photonics10060641




