New Method for Measuring the Scattering Phase Function of Micron/Nano Particles
Abstract
1. Introduction
2. Experimental Measurement and Sample Characteristics
2.1. Experimental Method
2.2. Experimental Uncertainty
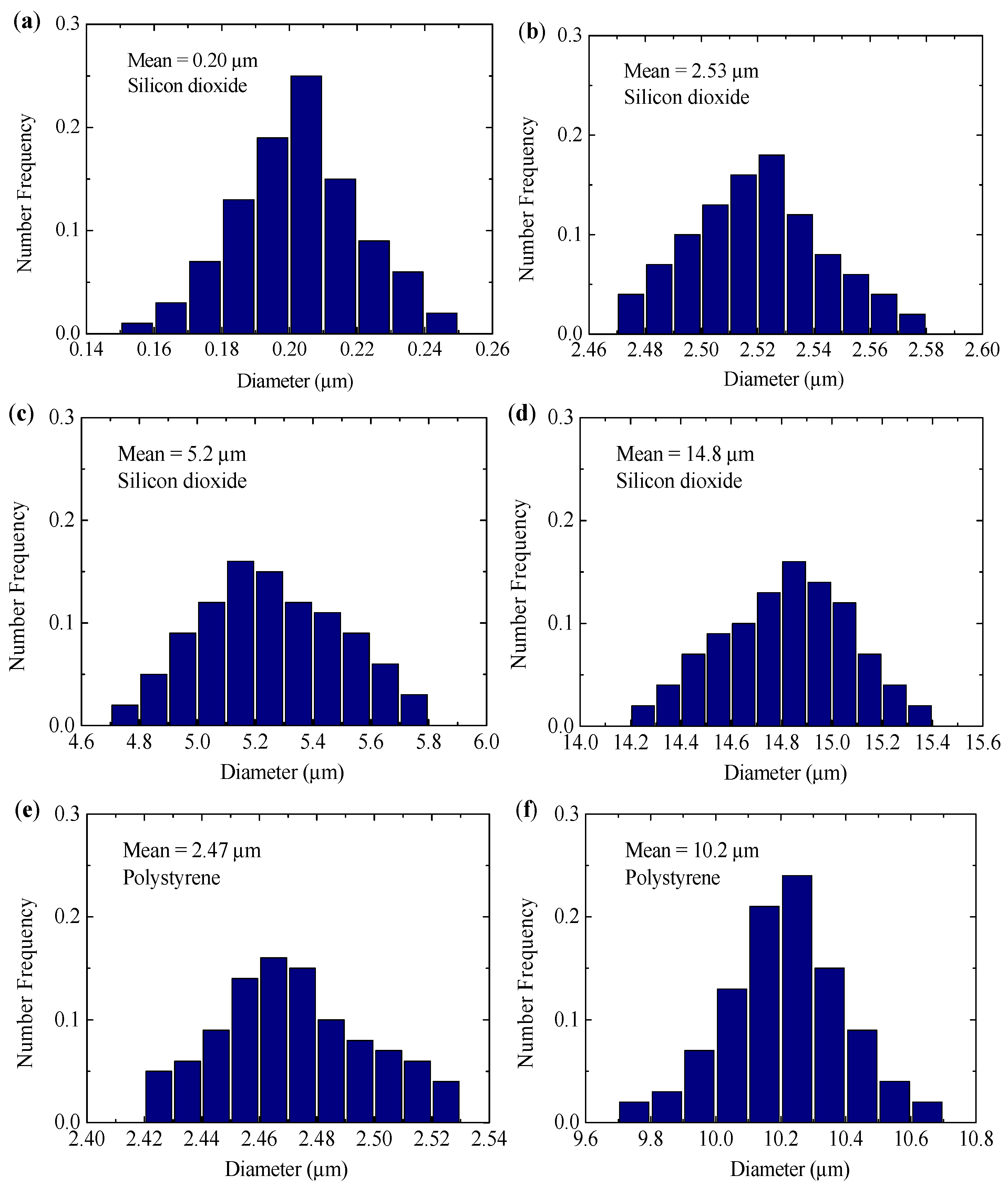
2.3. Sample Characteristics
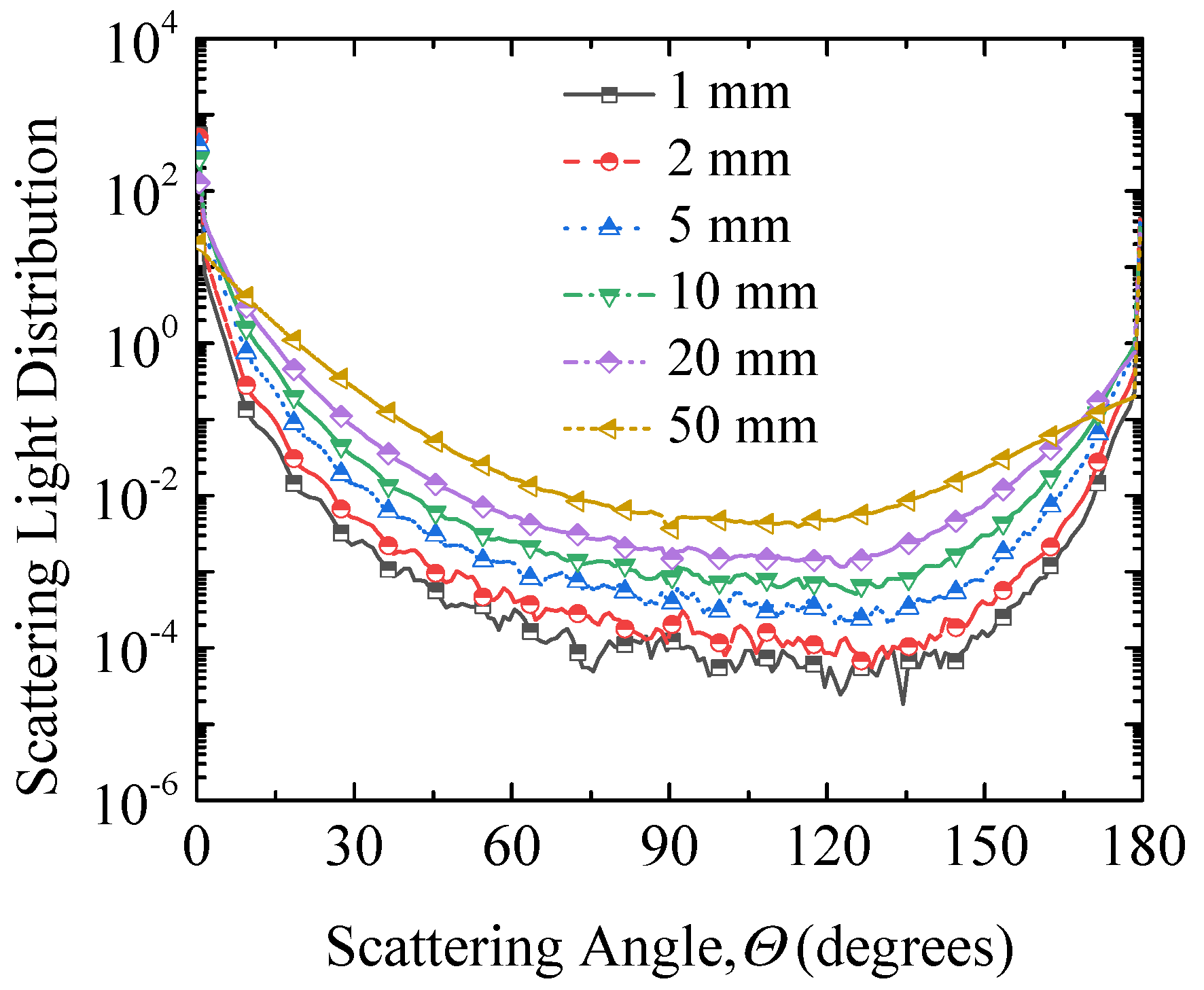
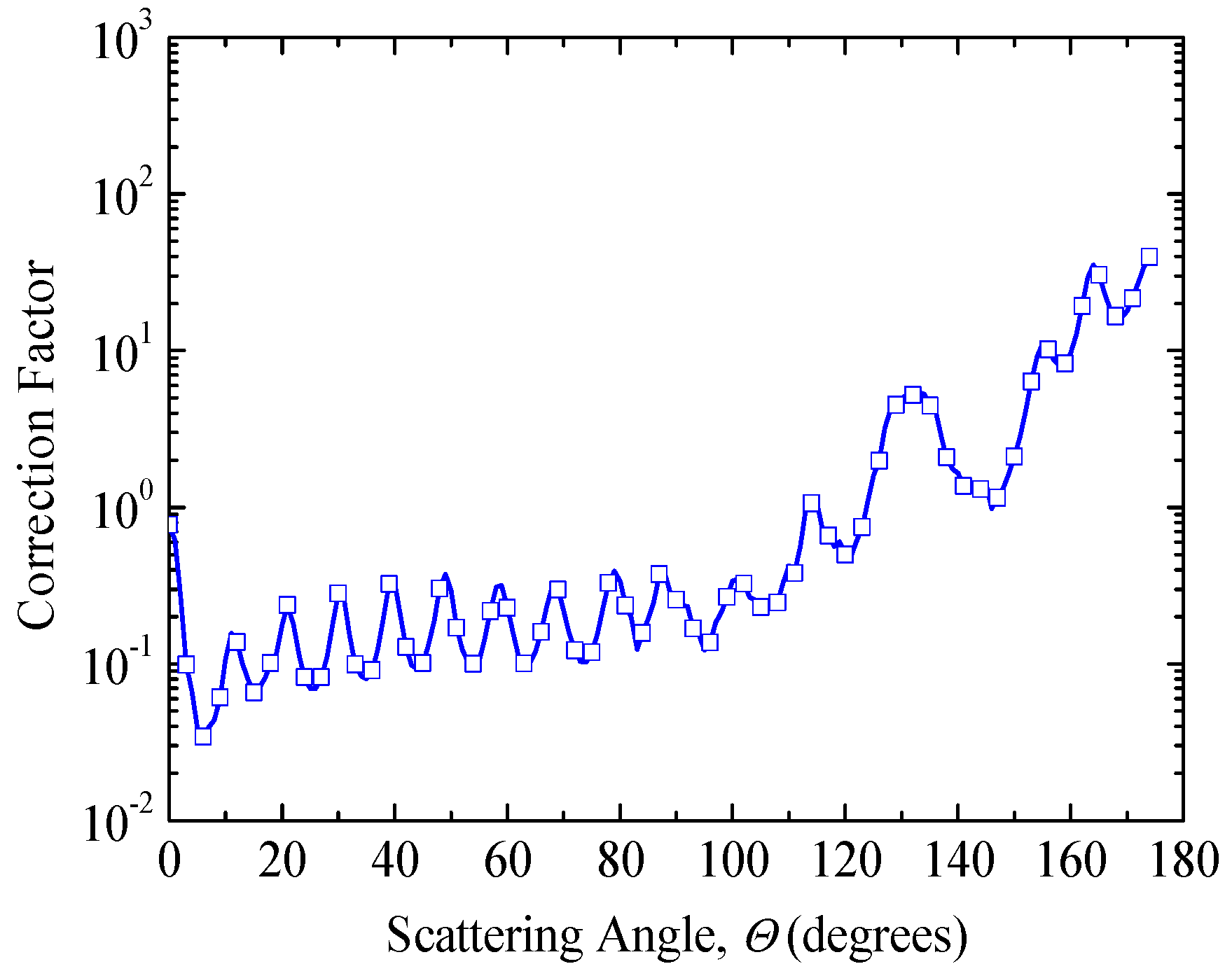
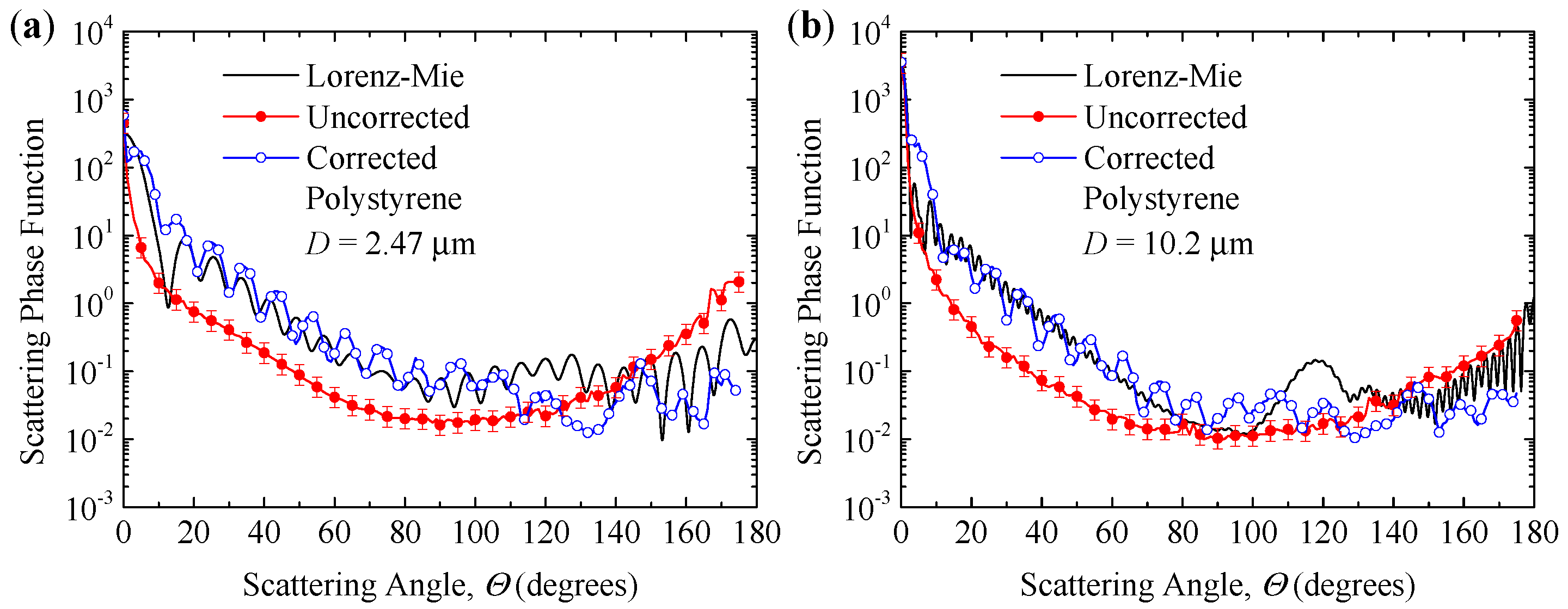
3. Experimental Validation
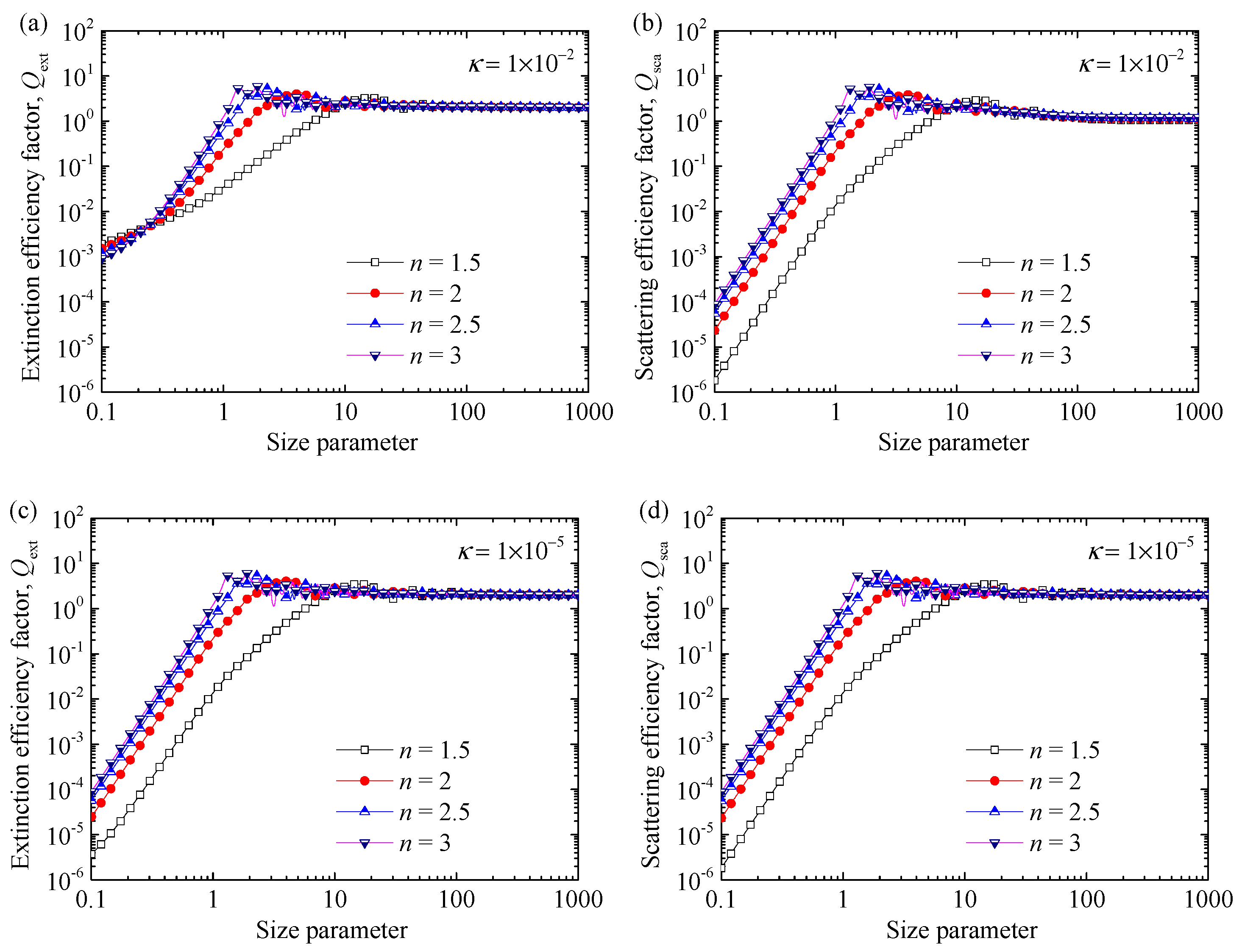
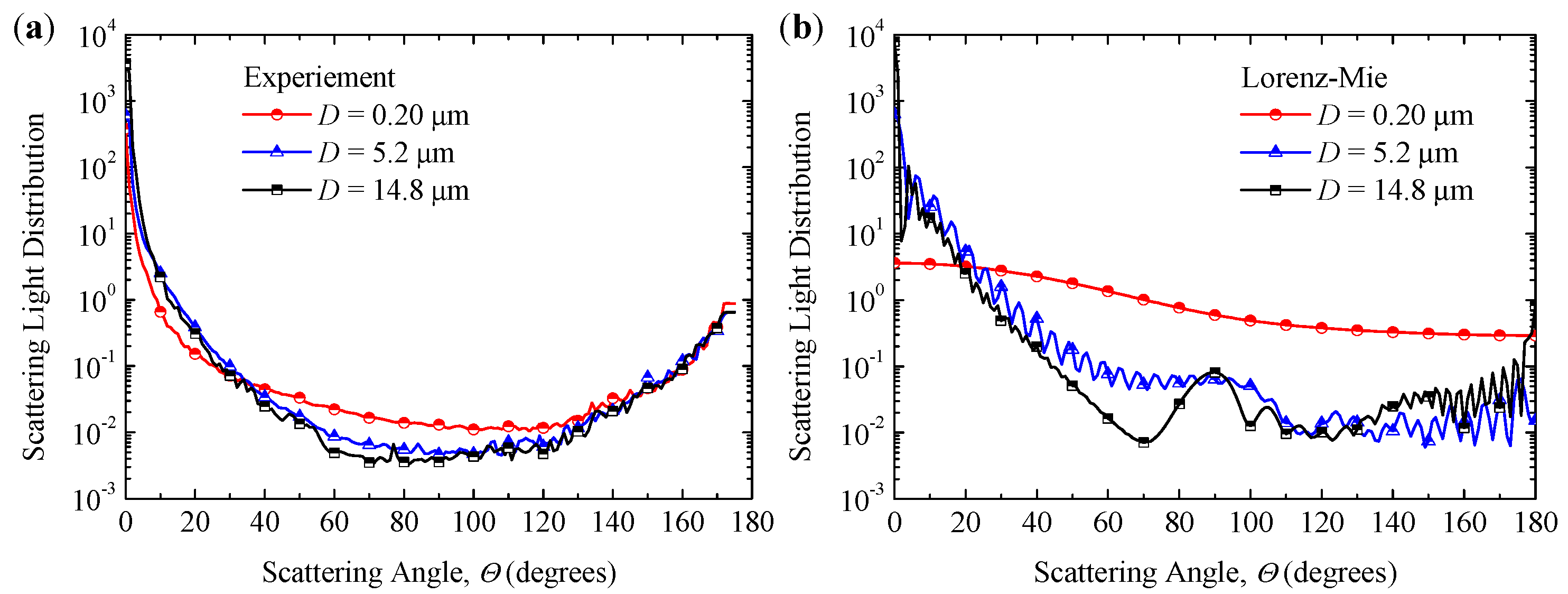
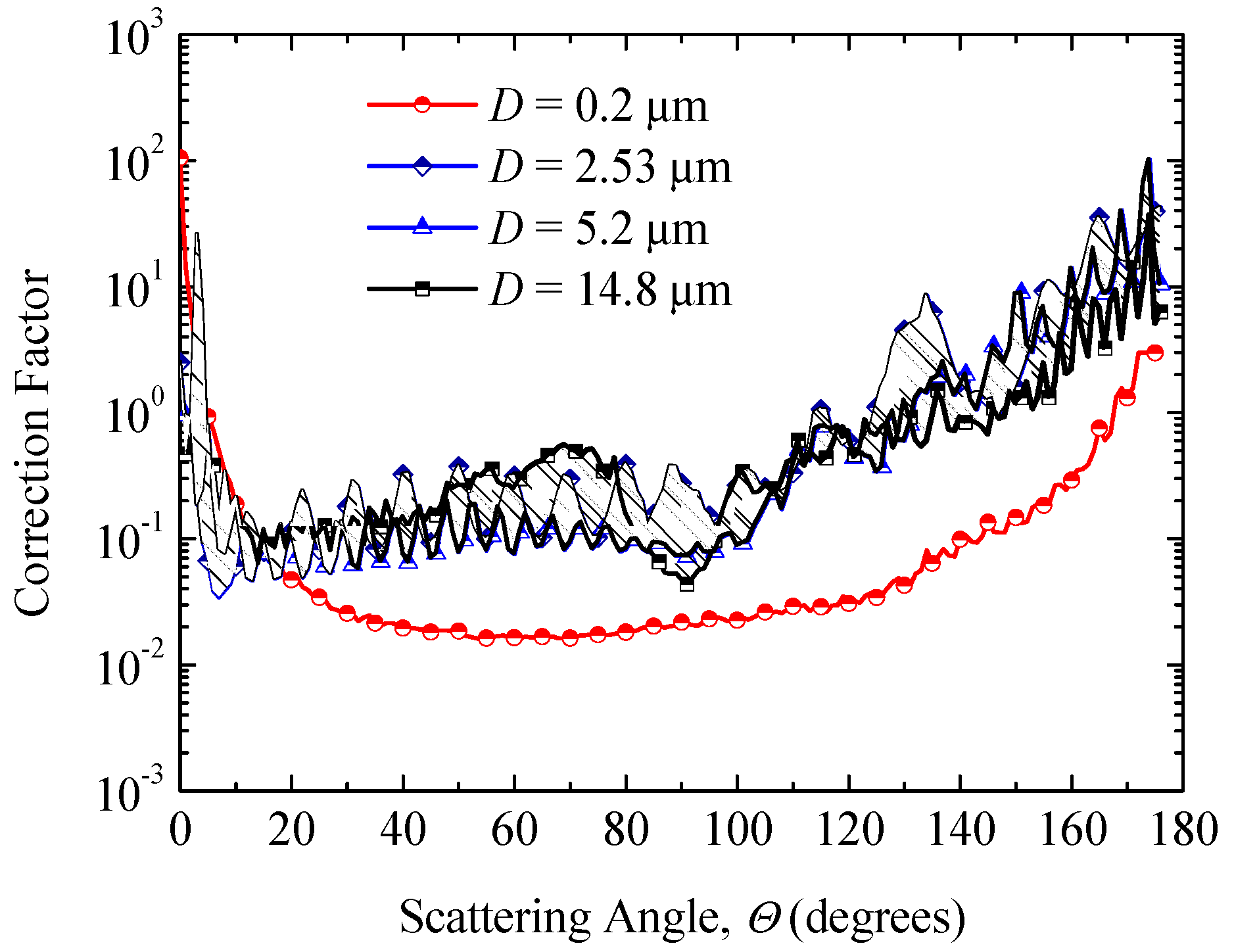
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Miroslaw, J.; Georges, R.F. Light Scattering by Particles in Water: Theoretical and Experimental Foundations; Academic Press: New York, NY, USA, 2007. [Google Scholar]
- Foschum, F.; Kienle, A. Optimized goniometer for determination of the scattering phase function of suspended particles: Simulations and measurements. J. Biomed. Opt. 2013, 18, 085002. [Google Scholar] [CrossRef]
- Riviere, N.; Ceolato, R.; Hespel, L. Polarimetric and angular light-scattering from dense media: Comparison of a vectorial radiative transfer model with analytical, stochastic and experimental approaches. J. Quant. Spectrosc. Radiat. Transf. 2013, 131, 88–94. [Google Scholar] [CrossRef]
- Berberoglu, H.; Pilon, L. Experimental measurements of the radiation characteristics of Anabaena variabilis ATCC 29413-U and Rhodobacter sphaeroides ATCC 49419. Int. J. Hydrogen Energy 2007, 32, 4772–4785. [Google Scholar] [CrossRef]
- Kong, B.; Vigil, R.D. Simulation of photosynthetically active radiation distribution in algal photobioreactors using a multidimensional spectral radiation model. Bioresour. Technol. 2014, 158, 141–148. [Google Scholar] [CrossRef]
- Kinnunen, M.; Karmenyan, A. Overview of single-cell elastic light scattering techniques. J. Biomed. Opt. 2015, 20, 051040. [Google Scholar] [CrossRef]
- Wu, T.T.; Qu, J.Y. Assessment of the relative contribution of cellular components to the acetowhitening effect in cell cultures and suspensions using elastic light-scattering spectroscopy. Appl. Opt. 2007, 46, 4834–4842. [Google Scholar] [CrossRef]
- Fang, H.; Ollero, M.; Vitkin, E.; Kimerer, L.; Cipolloni, P.; Zaman, M.; Freedman, S.; Bigio, I.; Itzkan, I.; Hanlon, E.; et al. Noninvasive sizing of subcellular organelles with light scattering spectroscopy. IEEE J. Sel. Top. Quantum Electron. 2003, 9, 267–276. [Google Scholar] [CrossRef]
- Kinnunen, M.; Karmenyan, A.; Särkelä, A.; Dimova, E.; Kietzmann, T. Low-Intensity Light Detection Methods for Selected Biophotonic Applications. In Proceedings of the Eighth International Conference on Advanced Optical Materials and Devices (AOMD-8), Riga, Latvia, 25–27 August 2014; SPIE: Bellingham, WA, USA, 2014; Volume 9421, p. 94210D. [Google Scholar]
- Hu, L.; Zhang, X.; Xiong, Y.; He, M.-X. Calibration of the LISST-VSF to derive the volume scattering functions in clear waters. Opt. Express 2019, 27, A1188–A1206. [Google Scholar] [CrossRef]
- Koestner, D.; Stramski, D.; Reynolds, R.A. Measurements of the Volume Scattering Function and the Degree of Linear Polarization of Light Scattered by Contrasting Natural Assemblages of Marine Particles. Appl. Sci. 2018, 8, 2690. [Google Scholar] [CrossRef]
- Zhang, X.; Gray, D.J.; Huot, Y.; You, Y.; Bi, L. Comparison of optically derived particle size distributions: Scattering over the full angular range versus diffraction at near forward angles. Appl. Opt. 2012, 51, 5085–5099. [Google Scholar] [CrossRef]
- Kaller, W. A new polar nephelometer for measurement of atmospheric aerosols. J. Quant. Spectrosc. Radiat. Transf. 2004, 87, 107–117. [Google Scholar] [CrossRef]
- Castagner, J.-L.; Bigio, I.J. Particle sizing with a fast polar nephelometer. Appl. Opt. 2007, 46, 527–532. [Google Scholar] [CrossRef]
- Manfred, K.M.; Washenfelder, R.A.; Wagner, N.L.; Adler, G.; Erdesz, F.; Womack, C.C.; Lamb, K.D.; Schwarz, J.P.; Franchin, A.; Selimovic, V.; et al. Supplementary material to “Investigating biomass burning aerosol morphology using a laser imaging nephelometer”. Atmos. Chem. Phys. 2018, 18, 1879–1894. [Google Scholar] [CrossRef]
- Volten, H.; Muñoz, O.; Rol, E.; De Haan, J.F.; Vassen, W.; Hovenier, J.W.; Muinonen, K.; Nousiainen, T. Scattering matrices of mineral aerosol particles at 441.6 nm and 632.8 nm. J. Geophys. Res. Atmos. 2001, 106, 17375–17401. [Google Scholar] [CrossRef]
- Ding, H.; Berl, E.; Wang, Z.; Millet, L.J.; Gillette, M.U.; Liu, J.; Boppart, M.; Popescu, G. Fourier Transform Light Scattering of Biological Structure and Dynamics. IEEE J. Sel. Top. Quantum Electron. 2010, 16, 909–918. [Google Scholar] [CrossRef]
- Jo, Y.; Jung, J.; Lee, J.W.; Shin, D.; Park, H.; Nam, K.T.; Park, J.-H.; Park, Y. Angle-resolved light scattering of individual rod-shaped bacteria based on Fourier transform light scattering. Sci. Rep. 2014, 4, 5090. [Google Scholar] [CrossRef]
- Brock, R.S.; Hu, X.-H.; Weidner, D.R.; Mourant, J.Q.; Lu, J. Effect of Detailed Cell Structure on Light Scattering Distribution: FDTD study of a B-cell with 3D Structure Constructed from Confocal Images. J. Quant. Spectrosc. Radiat. Transf. 2006, 102, 25–36. [Google Scholar] [CrossRef]
- Tan, H.; Doerffer, R.; Oishi, T.; Tanaka, A. A new approach to measure the volume scattering function. Opt. Express 2013, 21, 18697–18711. [Google Scholar] [CrossRef]
- McCrowey, C.J.; Tinilau, S.S.; Calderon, G.; Koo, J.-E.; Curtis, D.B. A Portable High-Resolution Polar Nephelometer for Measurement of the Angular Scattering Properties of Atmospheric Aerosol: Design and Validation. Aerosol Sci. Technol. 2013, 47, 592–605. [Google Scholar] [CrossRef]
- Mahariq, I.; Kurt, H. On- and off-optical-resonance dynamics of dielectric microcylinders under plane wave illumination. J. Opt. Soc. Am. B 2015, 32, 1022–1030. [Google Scholar] [CrossRef]
- Mahariq, I.; Astratov, V.N.; Kurt, H. Persistence of photonic nanojet formation under the deformation of circular boundary. J. Opt. Soc. Am. B 2016, 33, 535–542. [Google Scholar] [CrossRef]
- Shen, Y.J.; Zhu, Q.Z.; Zhang, Z.M. A scatterometer for measuring the bidirectional reflectance and transmittance of semiconductor wafers with rough surfaces. Rev. Sci. Instrum. 2003, 74, 4885–4892. [Google Scholar] [CrossRef]
- Modest, M.F. Radiative Heat Transfer, 3rd ed.; Academic Press: New York, NY, USA, 2013. [Google Scholar]
- Li, X.; Zhao, J.M.; Wang, C.C.; Liu, L.H. Improved transmission method for measuring the optical extinction coefficient of micro/nano particle suspensions. Appl. Opt. 2016, 55, 8171–8179. [Google Scholar] [CrossRef] [PubMed]
- Agrawal, B.M.; Mengüç, M.P. Forward and inverse analysis of single and multiple scattering of collimated radiation in an axisymmetric system. Int. J. Heat Mass Transf. 1991, 34, 633–647. [Google Scholar] [CrossRef]
- Kitamura, R.; Pilon, L.; Jonasz, M. Optical constants of silica glass from extreme ultraviolet to far infrared at near room temperature. Appl. Opt. 2007, 46, 8118–8133. [Google Scholar] [CrossRef]
- Ma, X.; Lu, J.Q.; Brock, R.S.; Jacobs, K.M.; Yang, P.; Hu, X.-H. Determination of complex refractive index of polystyrene microspheres from 370 to 1610 nm. Phys. Med. Biol. 2003, 48, 4165–4172. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, X.; Lin, L.; Wang, H.; Shang, Z.; Lv, J.; Hao, Y. New Method for Measuring the Scattering Phase Function of Micron/Nano Particles. Photonics 2023, 10, 511. https://doi.org/10.3390/photonics10050511
Li X, Lin L, Wang H, Shang Z, Lv J, Hao Y. New Method for Measuring the Scattering Phase Function of Micron/Nano Particles. Photonics. 2023; 10(5):511. https://doi.org/10.3390/photonics10050511
Chicago/Turabian StyleLi, Xingcan, Li Lin, Hongyang Wang, Zeguo Shang, Jinyuan Lv, and Yi Hao. 2023. "New Method for Measuring the Scattering Phase Function of Micron/Nano Particles" Photonics 10, no. 5: 511. https://doi.org/10.3390/photonics10050511
APA StyleLi, X., Lin, L., Wang, H., Shang, Z., Lv, J., & Hao, Y. (2023). New Method for Measuring the Scattering Phase Function of Micron/Nano Particles. Photonics, 10(5), 511. https://doi.org/10.3390/photonics10050511




