Frequency-Selective Surface Based on Negative-Group-Delay Bismuth–Mica Medium
Abstract
1. Introduction
2. Methods
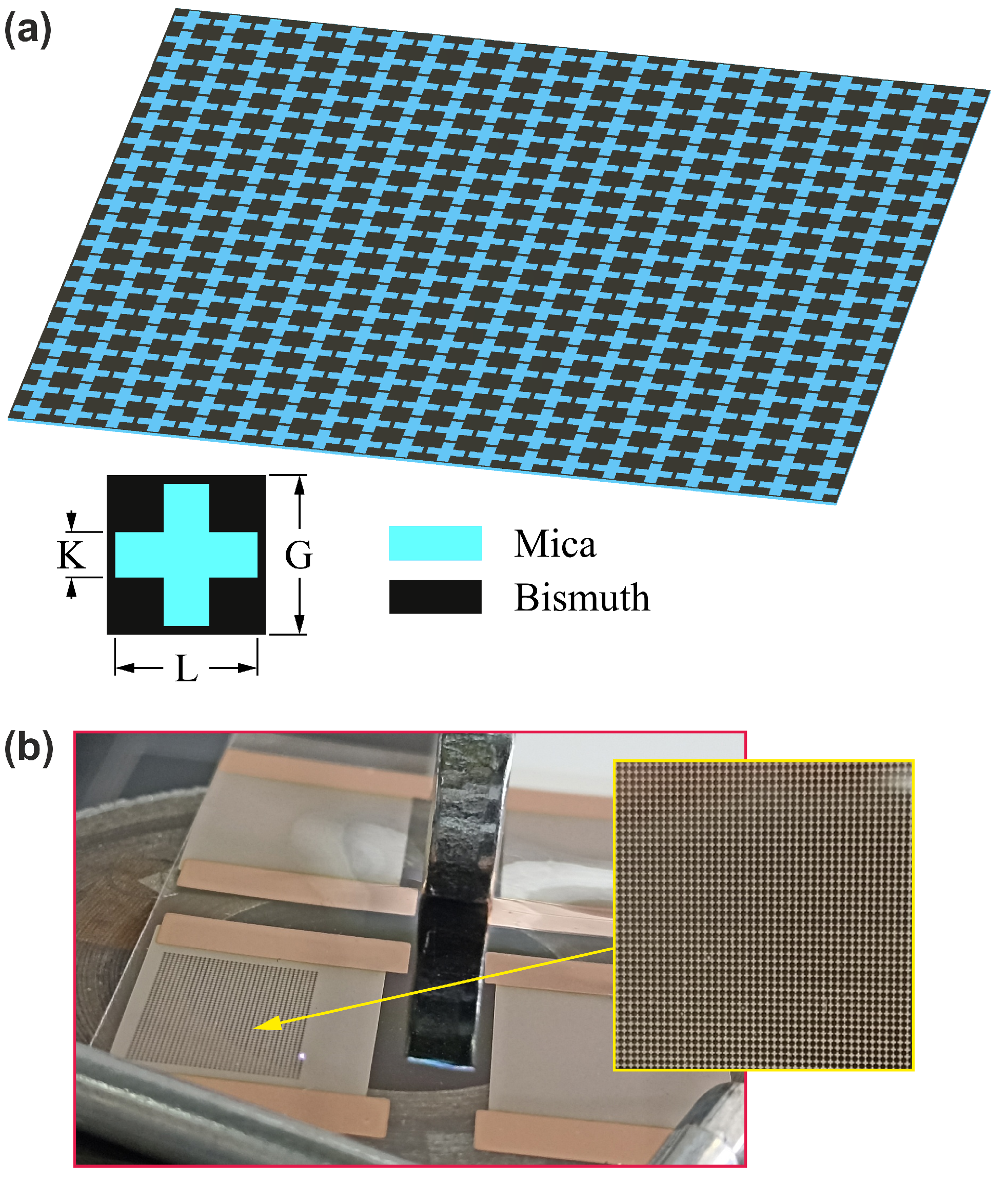
2.1. Sample Preparation
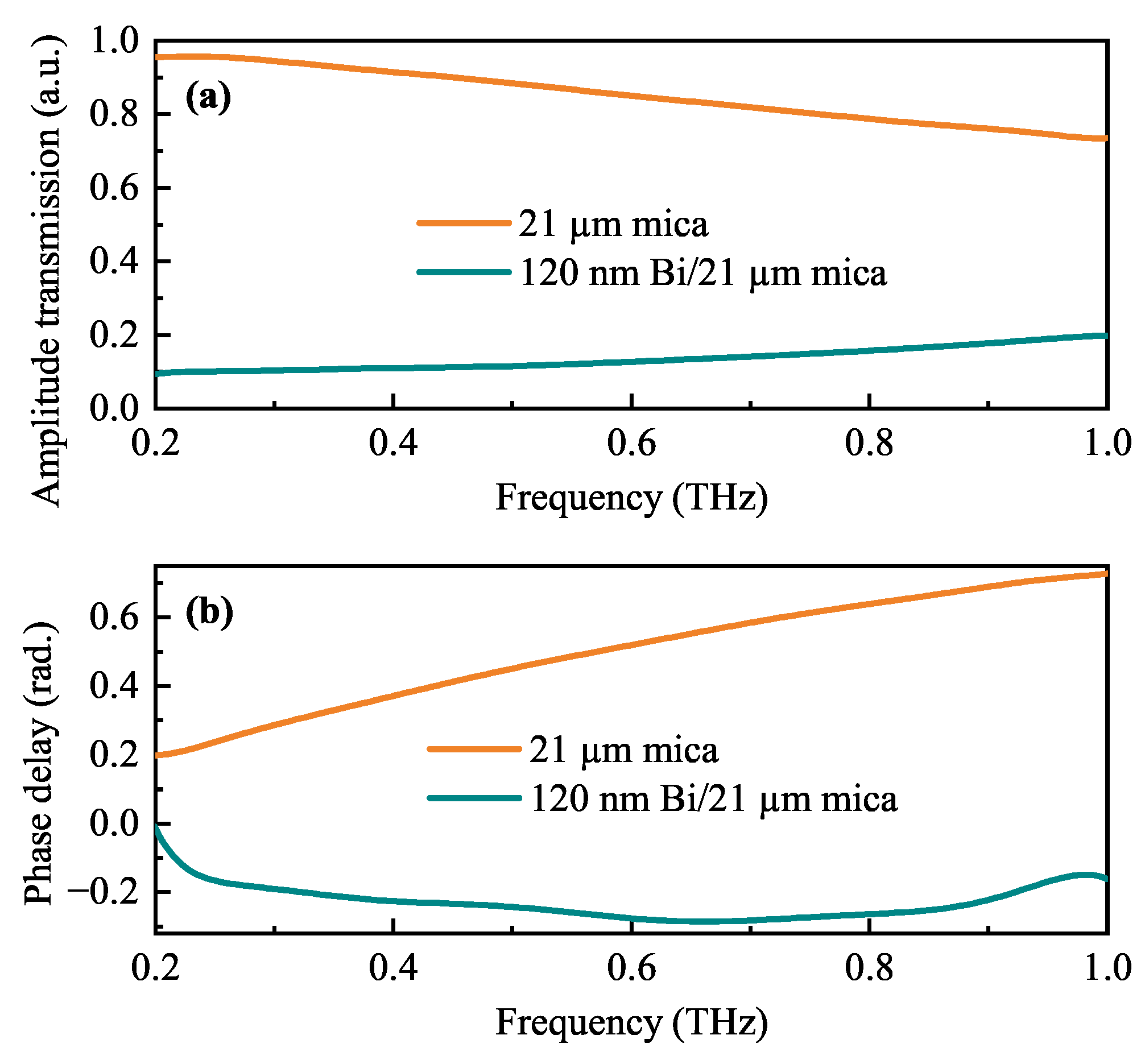
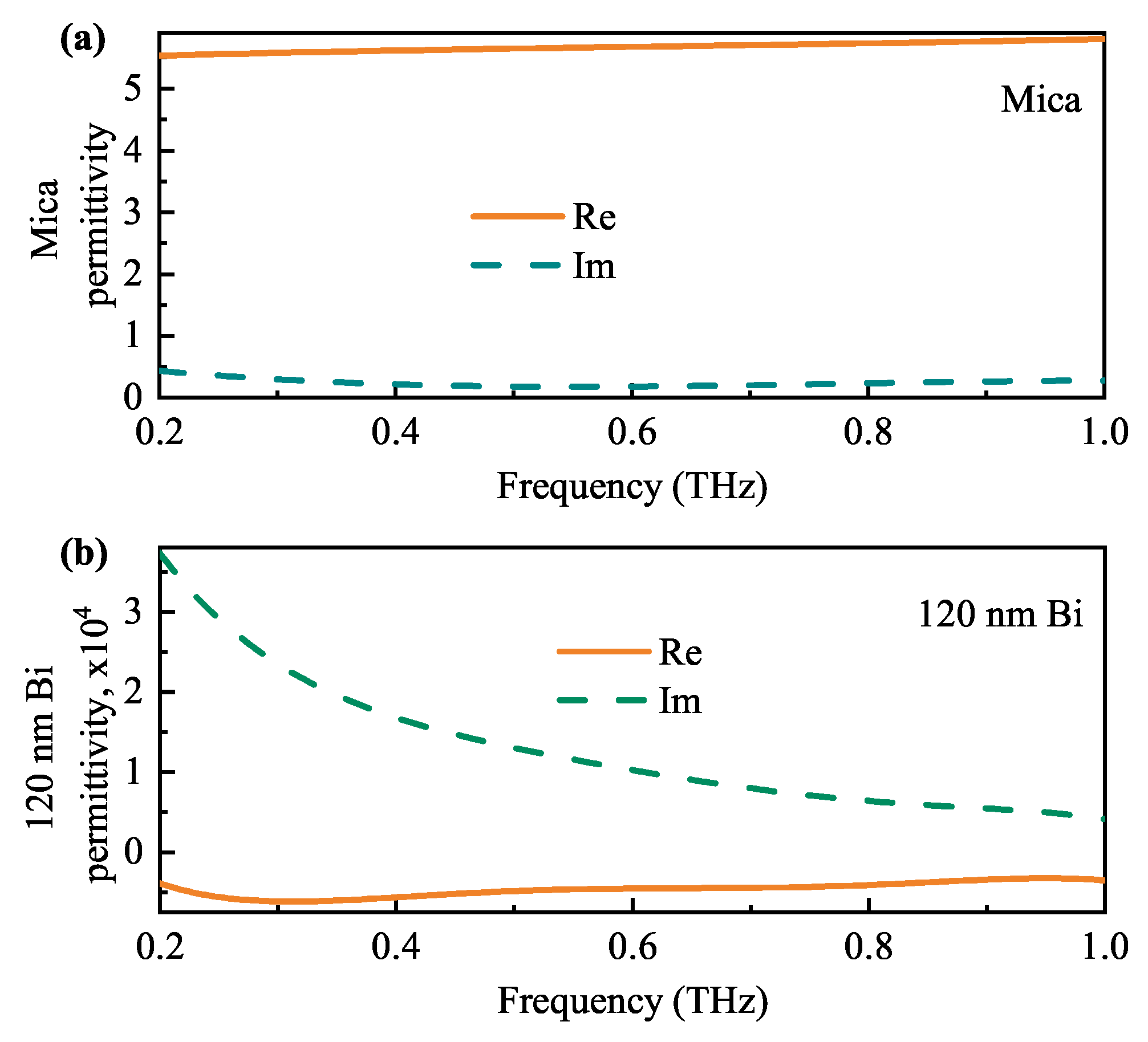
2.2. Measurements, Numerical Simulation, and Material Parameter Extraction
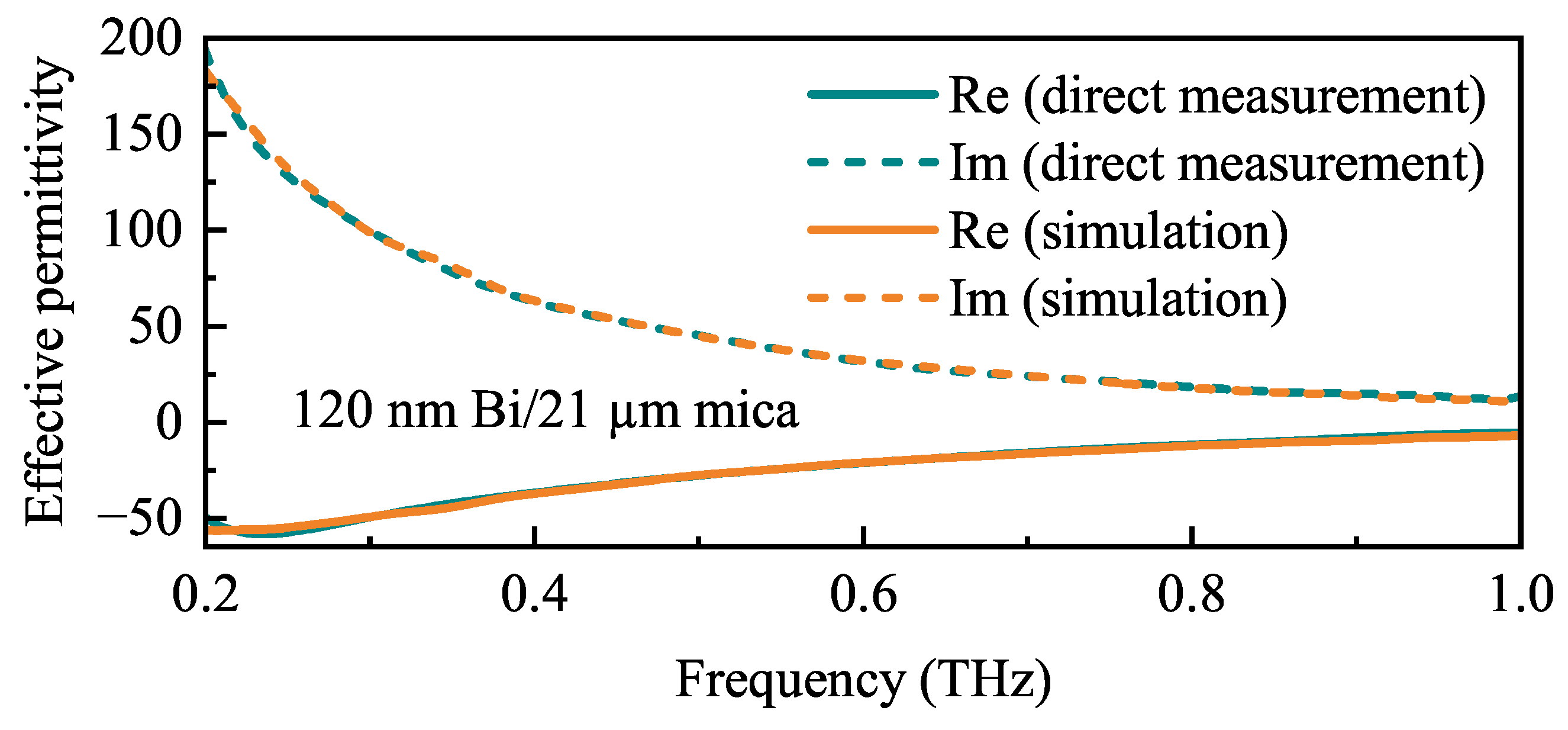
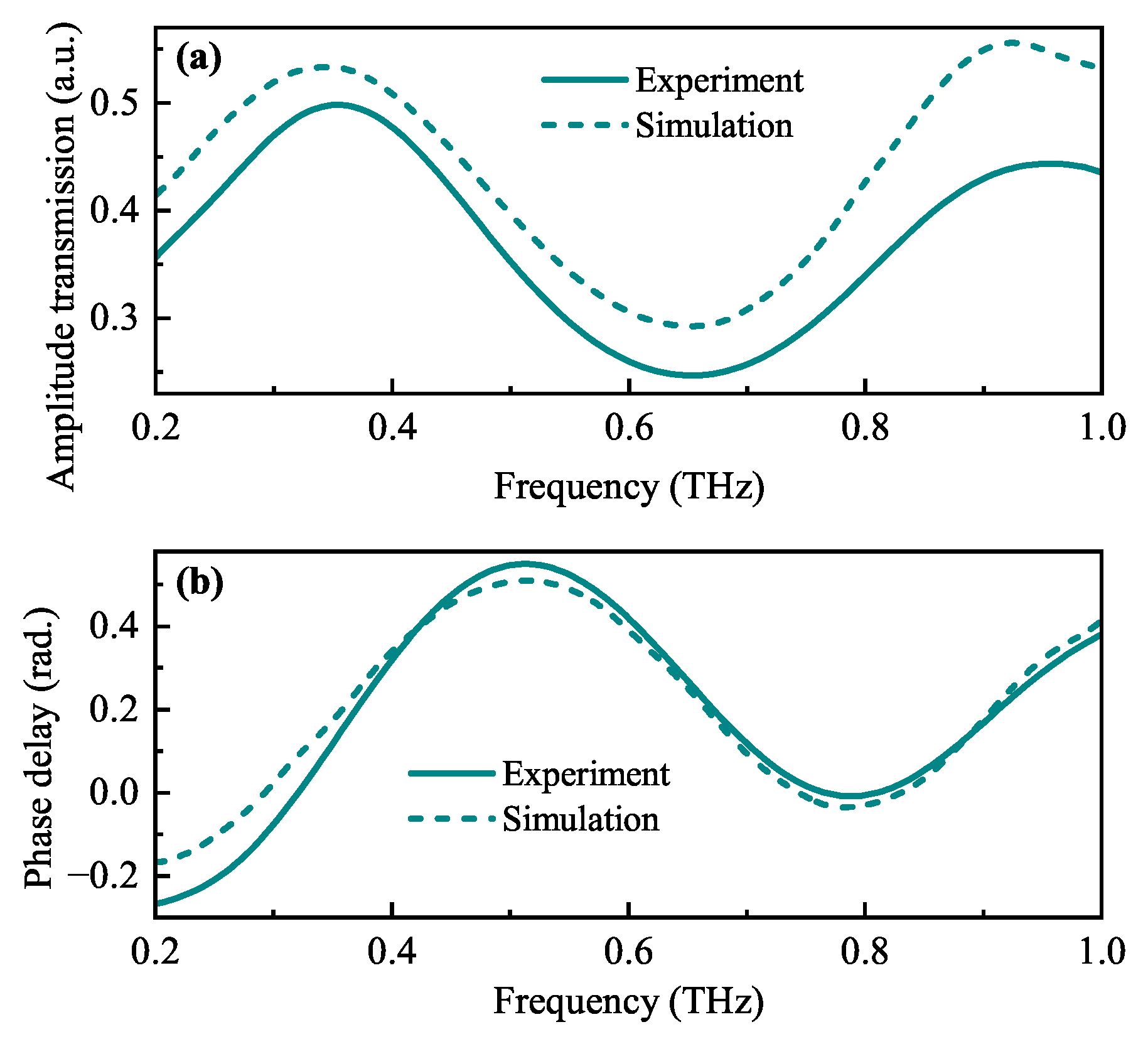
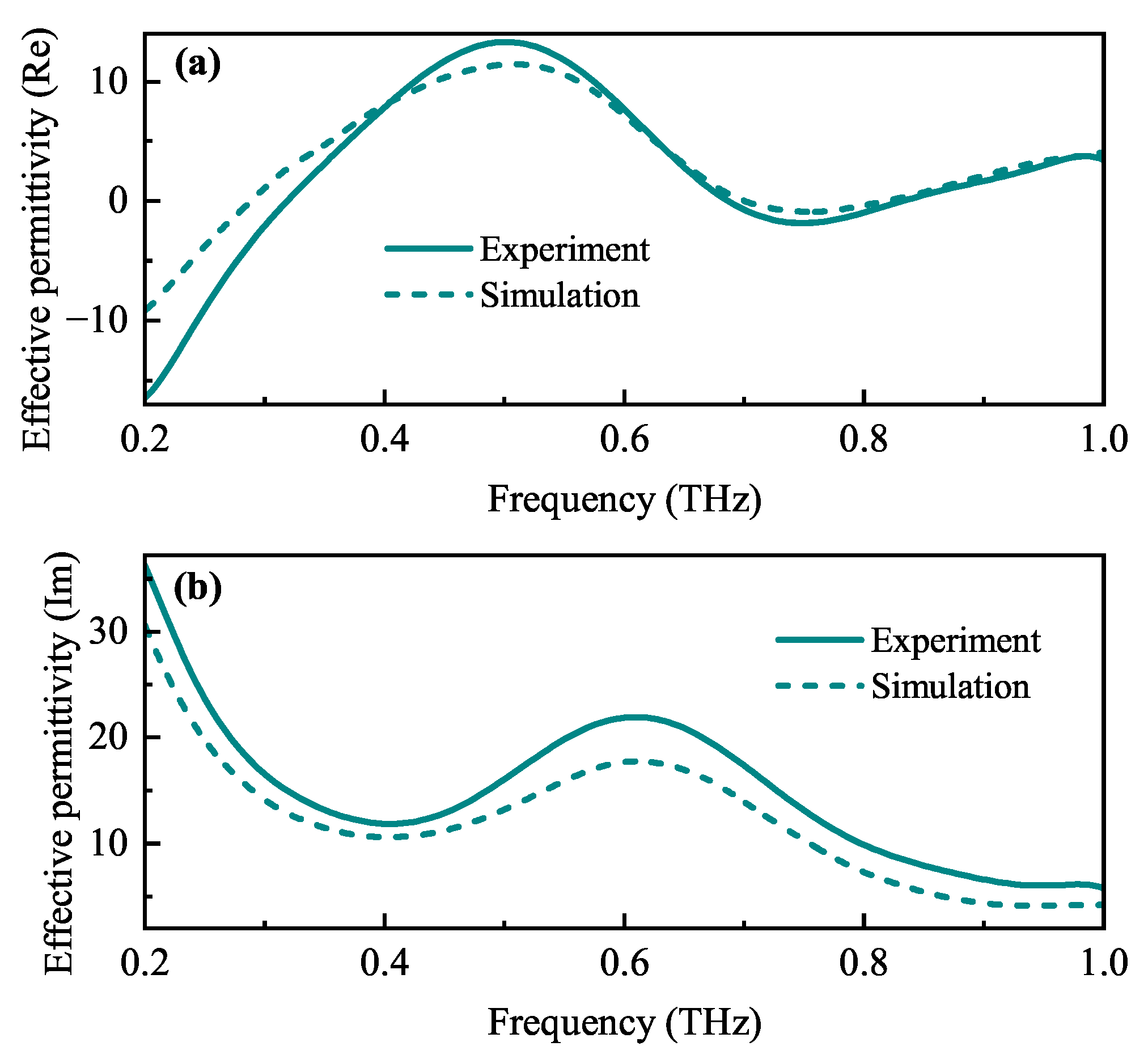
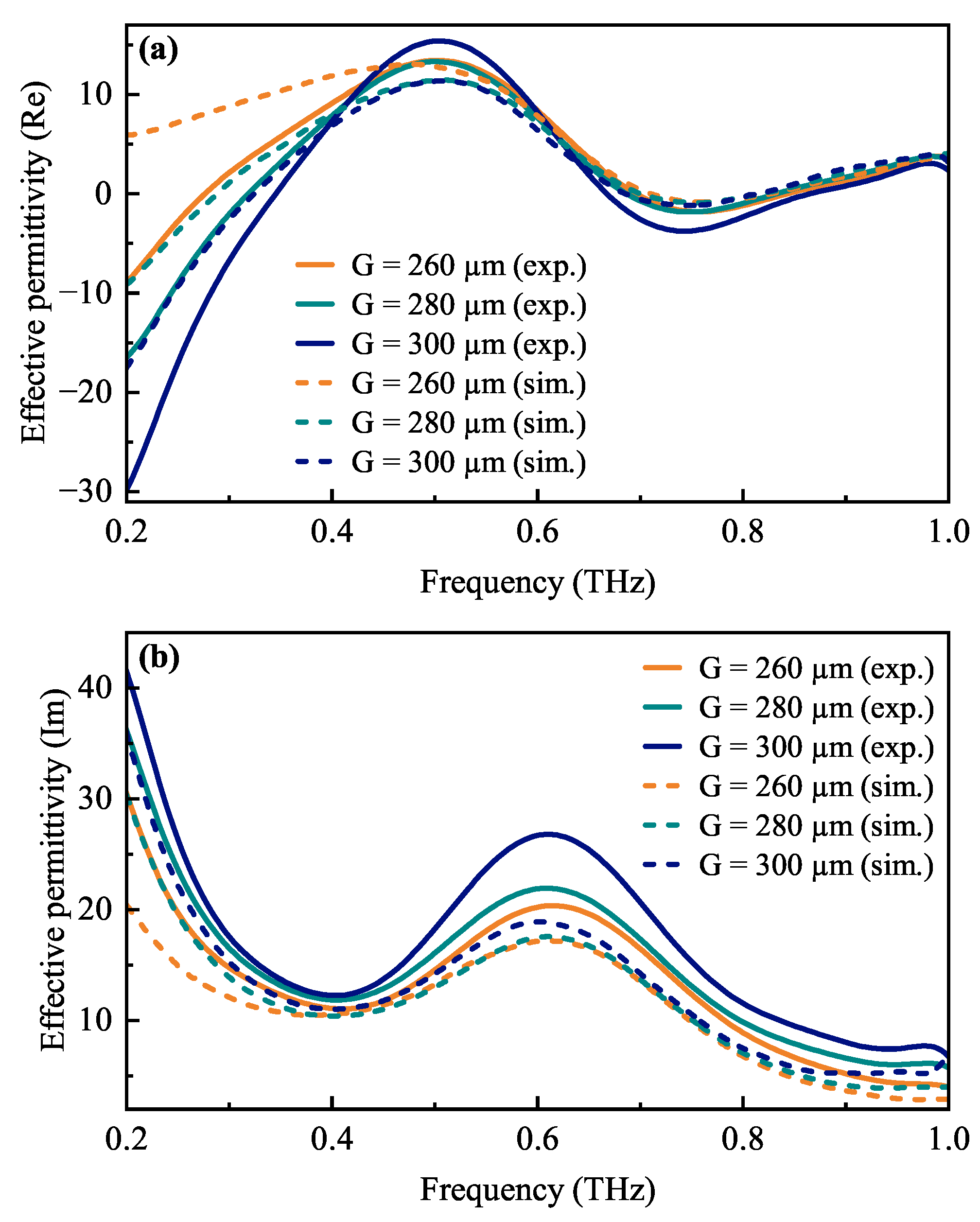
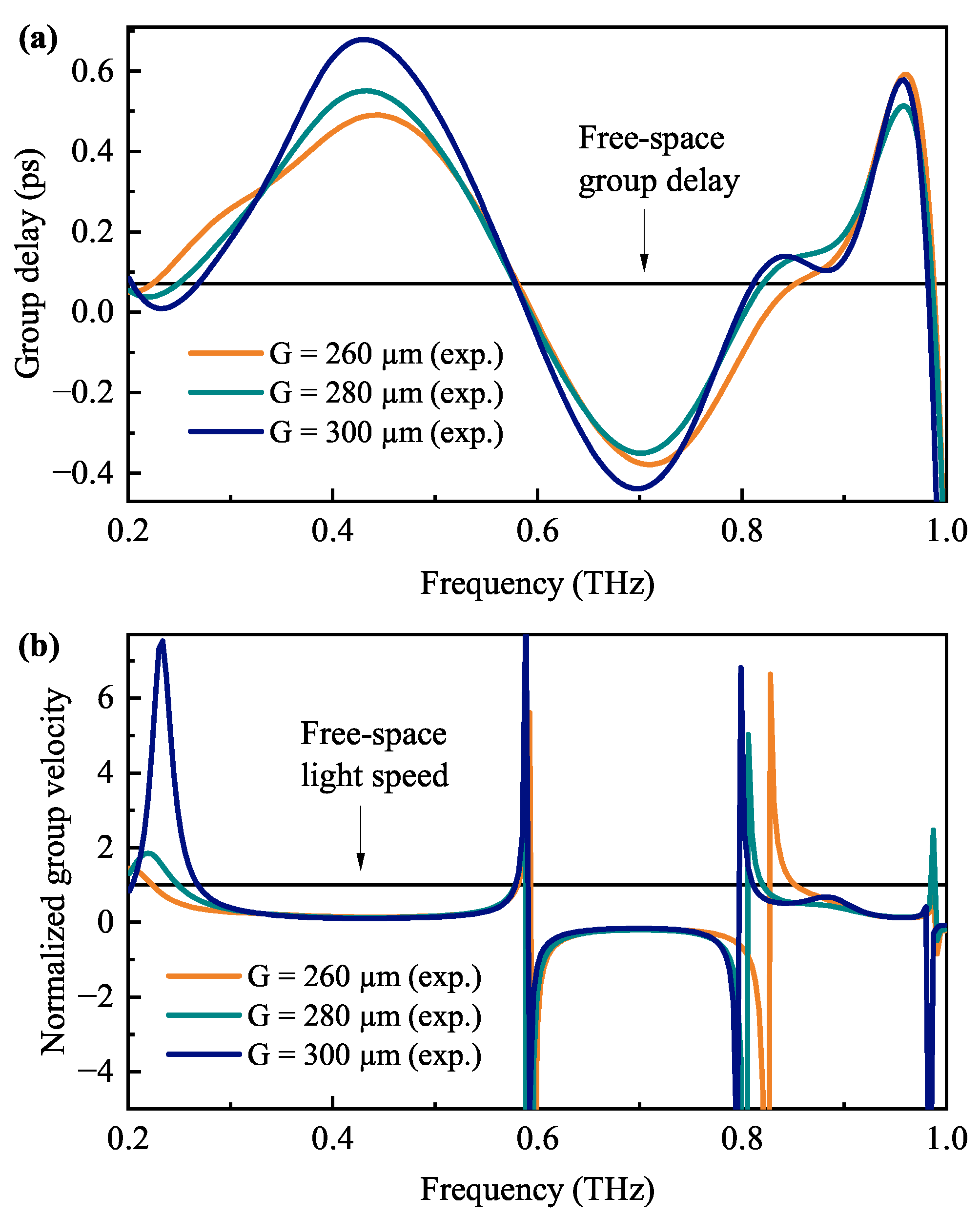
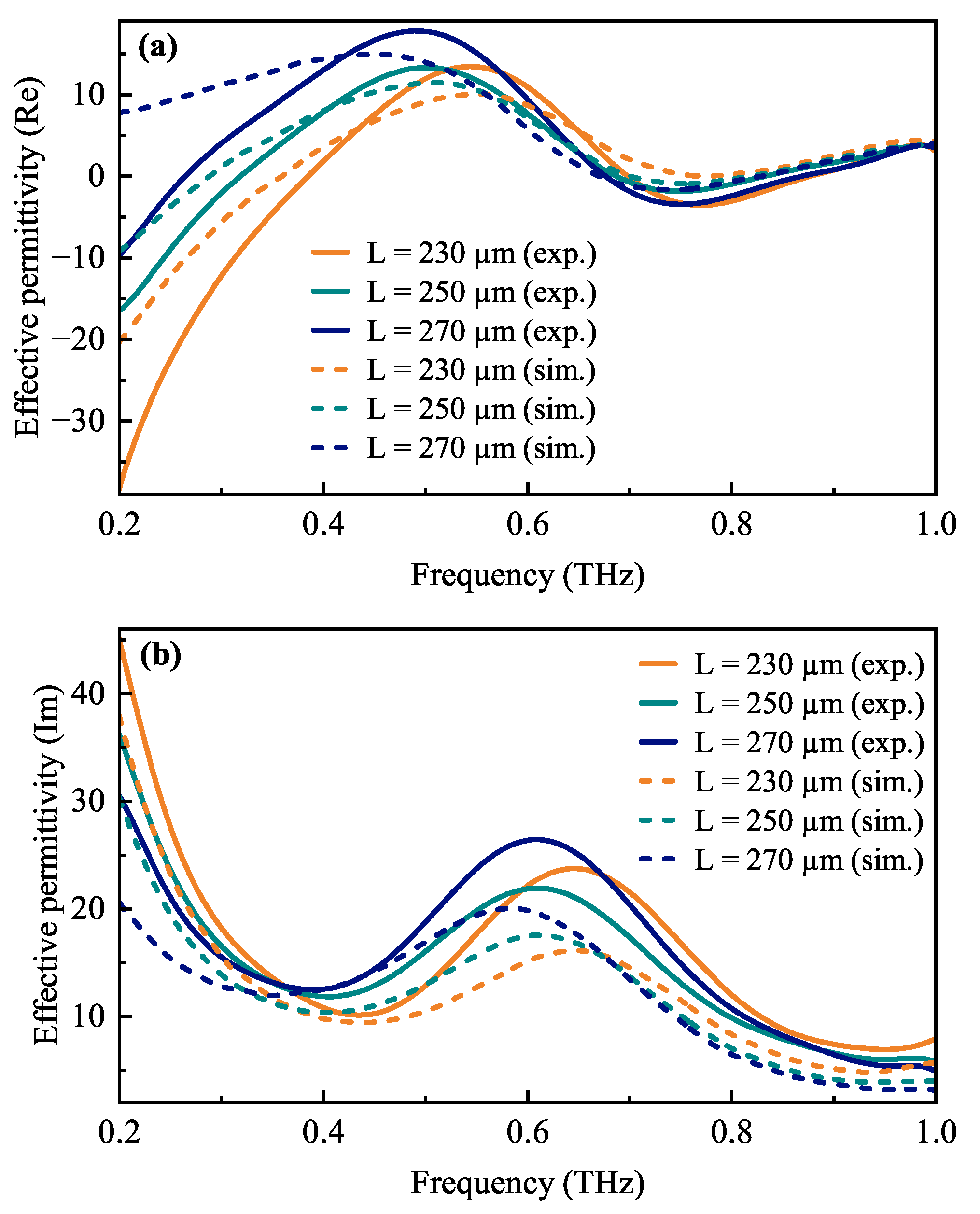
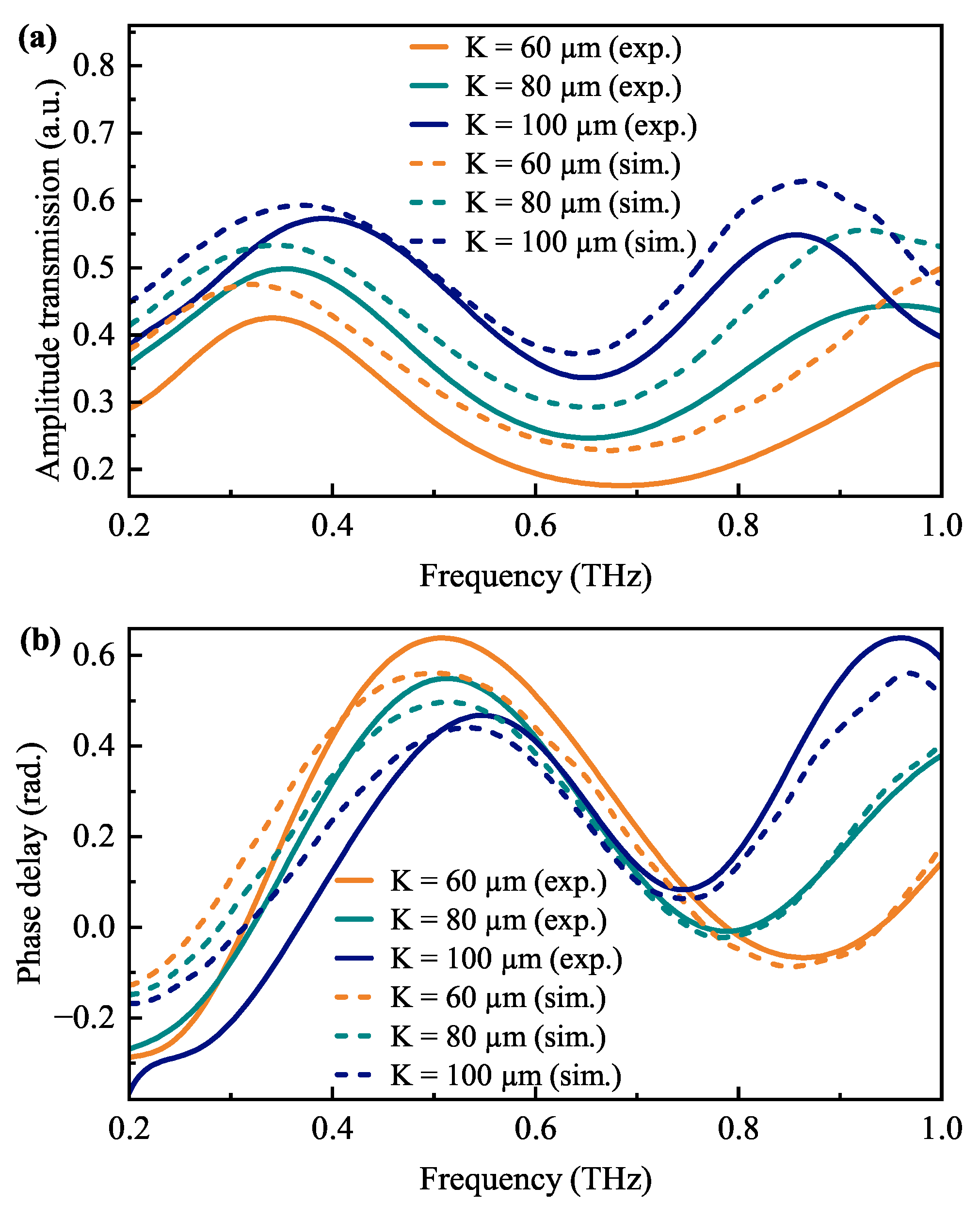
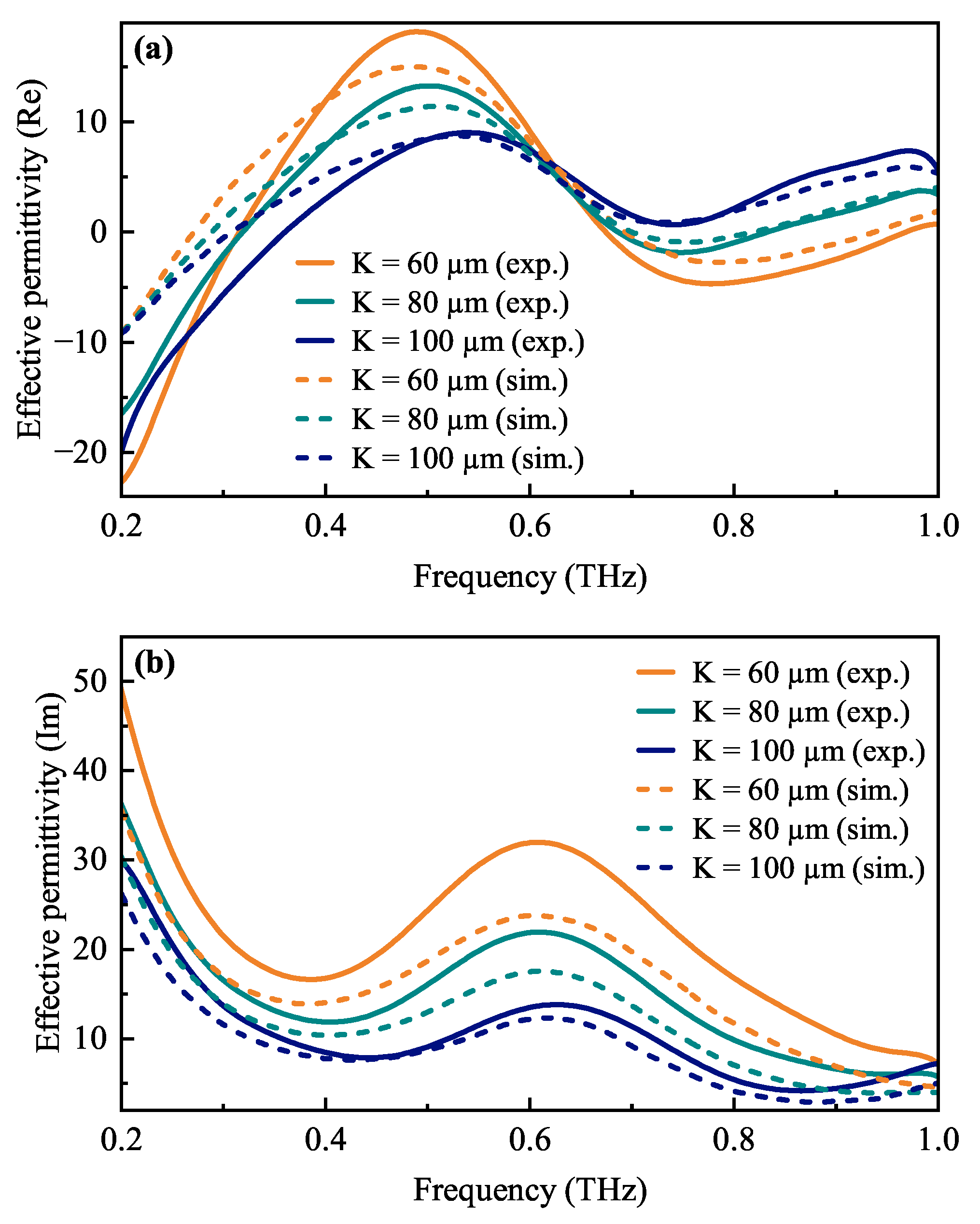
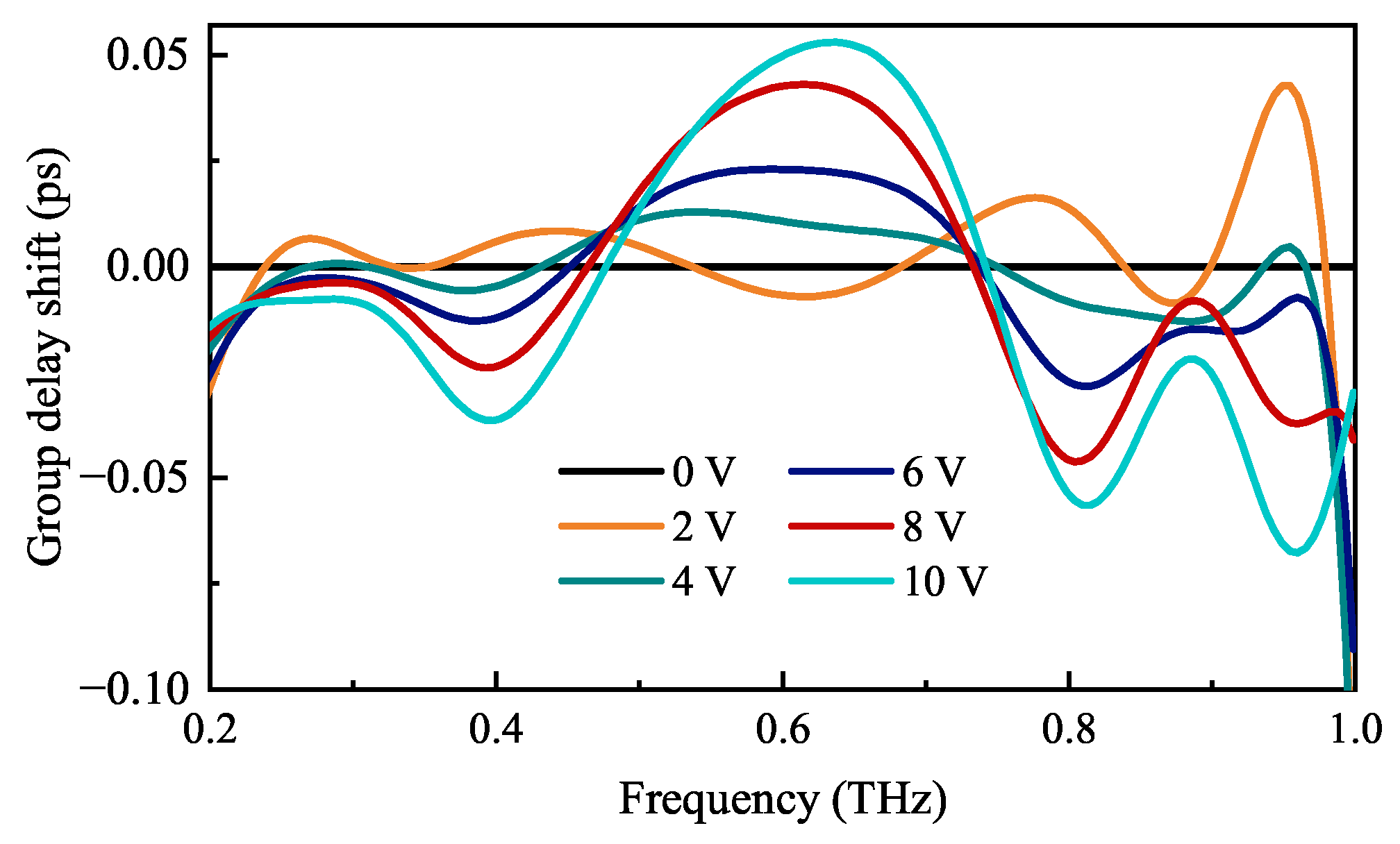
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Brillouin, L. Wave Propagation and Group Velocity; Academic Press: New York, NY, USA, 1960. [Google Scholar]
- Siddiqui, O.F.; Erickson, S.J.; Eleftheriades, G.V.; Mojahedi, M. Time-domain measurement of negative group delay in negative-refractive-index transmission-line metamaterials. IEEE Trans. Microw. Theory Tech. 2004, 52, 1449–1454. [Google Scholar] [CrossRef]
- Kandic, M.; Bridges, G.E. Limits of negative group delay phenomenon in linear causal media. Prog. Electromagn. Res. 2013, 134, 227–246. [Google Scholar] [CrossRef]
- Martin, T.; Landauer, R. Time delay of evanescent electromagnetic waves and the analogy to particle tunneling. Phys. Rev. A 1992, 45, 2611. [Google Scholar] [CrossRef] [PubMed]
- Grundmann, M. Kramers–kronig relations. In The Physics of Semiconductors; Springer: Berlin/Heidelberg, Germany, 2010; pp. 775–776. [Google Scholar]
- Stenner, M.D.; Gauthier, D.J.; Neifeld, M.A. The speed of information in a ‘fast-light’optical medium. Nature 2003, 425, 695–698. [Google Scholar] [CrossRef] [PubMed]
- Bigelow, M.S.; Lepeshkin, N.N.; Boyd, R.W. Superluminal and slow light propagation in a room-temperature solid. Science 2003, 301, 200–202. [Google Scholar] [CrossRef]
- Brillouin, L.; Parodi, M. Wave Propagation in Periodic Structures, International Series in Pure and Applied Physics; McGraw-Hill: New York, NY, USA, 1946. [Google Scholar]
- Woodley, J.; Mojahedi, M. Negative group velocity and group delay in left-handed media. Phys. Rev. E 2004, 70, 046603. [Google Scholar] [CrossRef]
- Galisteo-López, J.; Galli, M.; Balestreri, A.; Patrini, M.; Andreani, L.; López, C. Slow to superluminal light waves in thin 3D photonic crystals. Opt. Express 2007, 15, 15342–15350. [Google Scholar] [CrossRef]
- Novikov, V.; Leontiev, A.; Napolskii, K.; Murzina, T. Superluminal and slow femtosecond laser pulses in hyperbolic metamaterials in epsilon-near-zero regime. Opt. Lett. 2021, 46, 2276–2279. [Google Scholar] [CrossRef]
- Hrabar, S.; Krois, I.; Bonic, I.; Kiricenko, A. Ultra-broadband simultaneous superluminal phase and group velocities in non-Foster epsilon-near-zero metamaterial. Appl. Phys. Lett. 2013, 102, 054108. [Google Scholar] [CrossRef]
- Nimtz, G.; Haibel, A. Basics of superluminal signals. Ann. Der Phys. 2002, 514, 163–171. [Google Scholar] [CrossRef]
- Sabín, C.; Peropadre, B.; Lamata, L.; Solano, E. Simulating superluminal physics with superconducting circuit technology. Phys. Rev. A 2017, 96, 032121. [Google Scholar] [CrossRef]
- Song, K.Y.; Abedin, K.S.; Hotate, K. Gain-assisted superluminal propagation in tellurite glass fiber based on stimulated Brillouin scattering. Opt. Express 2008, 16, 225–230. [Google Scholar] [CrossRef] [PubMed]
- Mugnai, D.; Ranfagni, A.; Ronchi, L. The question of tunneling time duration: A new experimental test at microwave scale. Phys. Lett. A 1998, 247, 281–286. [Google Scholar] [CrossRef]
- Mitrofanov, O.; Lee, M.; Hsu, J.; Pfeiffer, L.; West, K.; Wynn, J.; Federici, J. Terahertz pulse propagation through small apertures. Appl. Phys. Lett. 2001, 79, 907–909. [Google Scholar] [CrossRef]
- Haché, A.; Poirier, L. Anomalous dispersion and superluminal group velocity in a coaxial photonic crystal: Theory and experiment. Phys. Rev. E 2002, 65, 036608. [Google Scholar] [CrossRef]
- Niang, A.; de Lustrac, A.; Burokur, S. Superluminal wave propagation in a non-Foster negative capacitor loaded transmission line. Electron. Lett. 2017, 53, 547–549. [Google Scholar] [CrossRef]
- Mojahedi, M.; Schamiloglu, E.; Hegeler, F.; Malloy, K.J. Time-domain detection of superluminal group velocity for single microwave pulses. Phys. Rev. E 2000, 62, 5758. [Google Scholar] [CrossRef]
- Schreier, F.; Schmitz, M.; Bryngdahl, O. Superluminal propagation of optical pulses inside diffractive structures. Opt. Commun. 1999, 163, 1–4. [Google Scholar] [CrossRef]
- Perenzoni, M.; Paul, D.J. Physics and Applications of Terahertz Radiation; Springer: Berlin/Heidelberg, Germany, 2014; Volume 173. [Google Scholar]
- Lewis, R.A. A review of terahertz sources. J. Phys. D Appl. Phys. 2014, 47, 374001. [Google Scholar] [CrossRef]
- Lewis, R. A review of terahertz detectors. J. Phys. D Appl. Phys. 2019, 52, 433001. [Google Scholar] [CrossRef]
- Mittleman, D.M. Twenty years of terahertz imaging. Opt. Express 2018, 26, 9417–9431. [Google Scholar] [CrossRef] [PubMed]
- Nagatsuma, T.; Ducournau, G.; Renaud, C.C. Advances in terahertz communications accelerated by photonics. Nat. Photonics 2016, 10, 371–379. [Google Scholar] [CrossRef]
- Gong, A.; Qiu, Y.; Chen, X.; Zhao, Z.; Xia, L.; Shao, Y. Biomedical applications of terahertz technology. Appl. Spectrosc. Rev. 2020, 55, 418–438. [Google Scholar] [CrossRef]
- Tzydynzhapov, G.; Gusikhin, P.; Muravev, V.; Dremin, A.; Nefyodov, Y.; Kukushkin, I. New real-time sub-terahertz security body scanner. J. Infrared Millim. Terahertz Waves 2020, 41, 632–641. [Google Scholar] [CrossRef]
- Grebenchukov, A.N.; Zaitsev, A.D.; Novoselov, M.G.; Demchenko, P.S.; Kovalska, E.O.; Alonso, E.T.; Walsh, K.; Russo, S.; Craciun, M.F.; Baldycheva, A.V.; et al. Photoexcited terahertz conductivity in multi-layered and intercalated graphene. Opt. Commun. 2020, 459, 124982. [Google Scholar] [CrossRef]
- Lee, S.H.; Choe, J.H.; Kim, C.; Bae, S.; Kim, J.S.; Park, Q.H.; Seo, M. Graphene assisted terahertz metamaterials for sensitive bio-sensing. Sens. Actuators B Chem. 2020, 310, 127841. [Google Scholar] [CrossRef]
- Withayachumnankul, W.; Fischer, B.M.; Ferguson, B.; Davis, B.R.; Abbott, D. A systemized view of superluminal wave propagation. Proc. IEEE 2010, 98, 1775–1786. [Google Scholar] [CrossRef]
- Rivas, J.G.; Benet, A.F.; Niehusmann, J.; Bolivar, P.H.; Kurz, H. Time-resolved broadband analysis of slow-light propagation and superluminal transmission of electromagnetic waves in three-dimensional photonic crystals. Phys. Rev. B 2005, 71, 155110. [Google Scholar] [CrossRef]
- Wynne, K.; Carey, J.J.; Zawadzka, J.; Jaroszynski, D.A. Tunneling of single-cycle terahertz pulses through waveguides. Opt. Commun. 2000, 176, 429–435. [Google Scholar] [CrossRef]
- Zhang, Z.; Deng, F.; Wu, F.; Zhu, C. Dynamical characteristics of ultrashort terahertz pulse in metamaterials. Laser Phys. Lett. 2022, 19, 056001. [Google Scholar] [CrossRef]
- He, X.; Yang, X.; Lu, G.; Yang, W.; Wu, F.; Yu, Z.; Jiang, J. Implementation of selective controlling electromagnetically induced transparency in terahertz graphene metamaterial. Carbon 2017, 123, 668–675. [Google Scholar] [CrossRef]
- Bossard, J.A.; Liang, X.; Li, L.; Yun, S.; Werner, D.H.; Weiner, B.; Mayer, T.S.; Cristman, P.F.; Diaz, A.; Khoo, I. Tunable frequency selective surfaces and negative-zero-positive index metamaterials based on liquid crystals. IEEE Trans. Antennas Propag. 2008, 56, 1308–1320. [Google Scholar] [CrossRef]
- Zaitsev, A.; Demchenko, P.; Makarova, E.; Tukmakova, A.; Kablukova, N.; Asach, A.; Novotelnova, A.; Khodzitsky, M. Hyperbolic Bismuth–Dielectric Structure for Terahertz Photonics. Phys. Status Solidi (RRL)–Rapid Res. Lett. 2020, 14, 2000093. [Google Scholar] [CrossRef]
- Zaitsev, A.; Zykov, D.; Demchenko, P.; Novoselov, M.; Nazarov, R.; Masyukov, M.; Makarova, E.; Tukmakova, A.; Asach, A.; Novotelnova, A.; et al. Experimental investigation of optically controlled topological transition in bismuth-mica structure. Sci. Rep. 2021, 11, 1–13. [Google Scholar] [CrossRef] [PubMed]
- Grabov, V.; Demidov, E.; Komarov, V. Optimization of the conditions for vacuum thermal deposition of bismuth films with control of their imperfection by atomic force microscopy. Phys. Solid State 2010, 52, 1298–1302. [Google Scholar] [CrossRef]
- Garcia, N.; Kao, Y.; Strongin, M. Galvanomagnetic studies of bismuth films in the quantum-size-effect region. Phys. Rev. B 1972, 5, 2029. [Google Scholar] [CrossRef]
- Available online: http://tydex.ru (accessed on 25 April 2023).
- Naftaly, M.; Miles, R.E. Terahertz time-domain spectroscopy for material characterization. Proc. IEEE 2007, 95, 1658–1665. [Google Scholar] [CrossRef]
- Naftaly, M. Terahertz Metrology; Artech House: Norfolk, MA, USA, 2015. [Google Scholar]
- Taschin, A.; Bartolini, P.; Tasseva, J.; Torre, R. THz time-domain spectroscopic investigations of thin films. Measurement 2018, 118, 282–288. [Google Scholar] [CrossRef]
- Tinkham, M. Energy gap interpretation of experiments on infrared transmission through superconducting films. Phys. Rev. 1956, 104, 845. [Google Scholar] [CrossRef]
- Novotny, L.; Hecht, B. Principles of Nano-Optics; Cambridge University Press: Cambridge, UK, 2012. [Google Scholar]
- Qi, L.; Liu, C. Complex band structures of 1D anisotropic graphene photonic crystal. Photonics Res. 2017, 5, 543–551. [Google Scholar] [CrossRef]
- Available online: https://www.3ds.com/products-services/simulia/products/cst-studio-suite (accessed on 25 April 2023).
- Soboleva, V.Y.; Gomon, D.A.; Sedykh, E.A.; Balya, V.K.; Khodzitskii, M.K. Development of narrow bandpass filters based on cross cavities for the terahertz frequency range. J. Opt. Technol. 2017, 84, 521–524. [Google Scholar] [CrossRef]
- Boyd, I.W.; Wilson, J.I. Laser processing of silicon. Nature 1983, 303, 481–486. [Google Scholar] [CrossRef]
- Alekseyev, L.V.; Podolskiy, V.A.; Narimanov, E.E. Homogeneous hyperbolic systems for terahertz and far-infrared frequencies. Adv. Optoelectron. 2012, 2012, 1–6. [Google Scholar] [CrossRef]
- Koziol, M. It’s never too early to think about 6G. IEEE Spectr. 2018, 22, 1. [Google Scholar]
- Sizov, F. Infrared and terahertz in biomedicine. Semicond. Phys. Quantum Electron. Optoelectron. 2017, 20, 273–283. [Google Scholar] [CrossRef]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zaitsev, A.D.; Demchenko, P.S.; Kablukova, N.S.; Vozianova, A.V.; Khodzitsky, M.K. Frequency-Selective Surface Based on Negative-Group-Delay Bismuth–Mica Medium. Photonics 2023, 10, 501. https://doi.org/10.3390/photonics10050501
Zaitsev AD, Demchenko PS, Kablukova NS, Vozianova AV, Khodzitsky MK. Frequency-Selective Surface Based on Negative-Group-Delay Bismuth–Mica Medium. Photonics. 2023; 10(5):501. https://doi.org/10.3390/photonics10050501
Chicago/Turabian StyleZaitsev, Anton D., Petr S. Demchenko, Natallya S. Kablukova, Anna V. Vozianova, and Mikhail K. Khodzitsky. 2023. "Frequency-Selective Surface Based on Negative-Group-Delay Bismuth–Mica Medium" Photonics 10, no. 5: 501. https://doi.org/10.3390/photonics10050501
APA StyleZaitsev, A. D., Demchenko, P. S., Kablukova, N. S., Vozianova, A. V., & Khodzitsky, M. K. (2023). Frequency-Selective Surface Based on Negative-Group-Delay Bismuth–Mica Medium. Photonics, 10(5), 501. https://doi.org/10.3390/photonics10050501





