Effect of Wavefront Distortion on the Performance of Coherent Detection Systems: Theoretical Analysis and Experimental Research
Abstract
1. Introduction
2. Theoretical Research
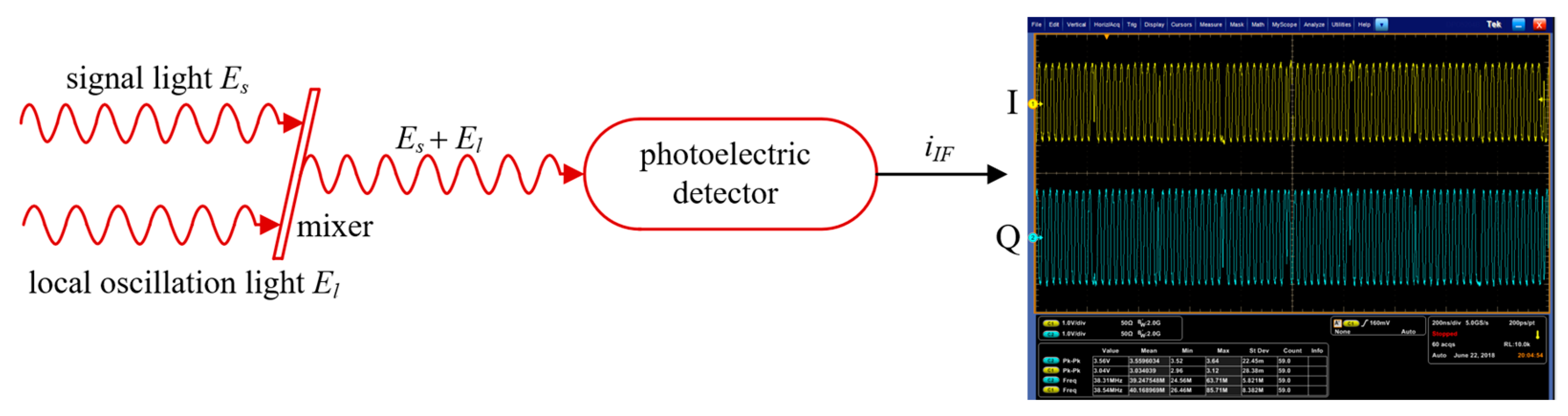
2.1. Coherent Detection Principle
2.2. Phase Wavefront Distortion
3. Numerical Simulation
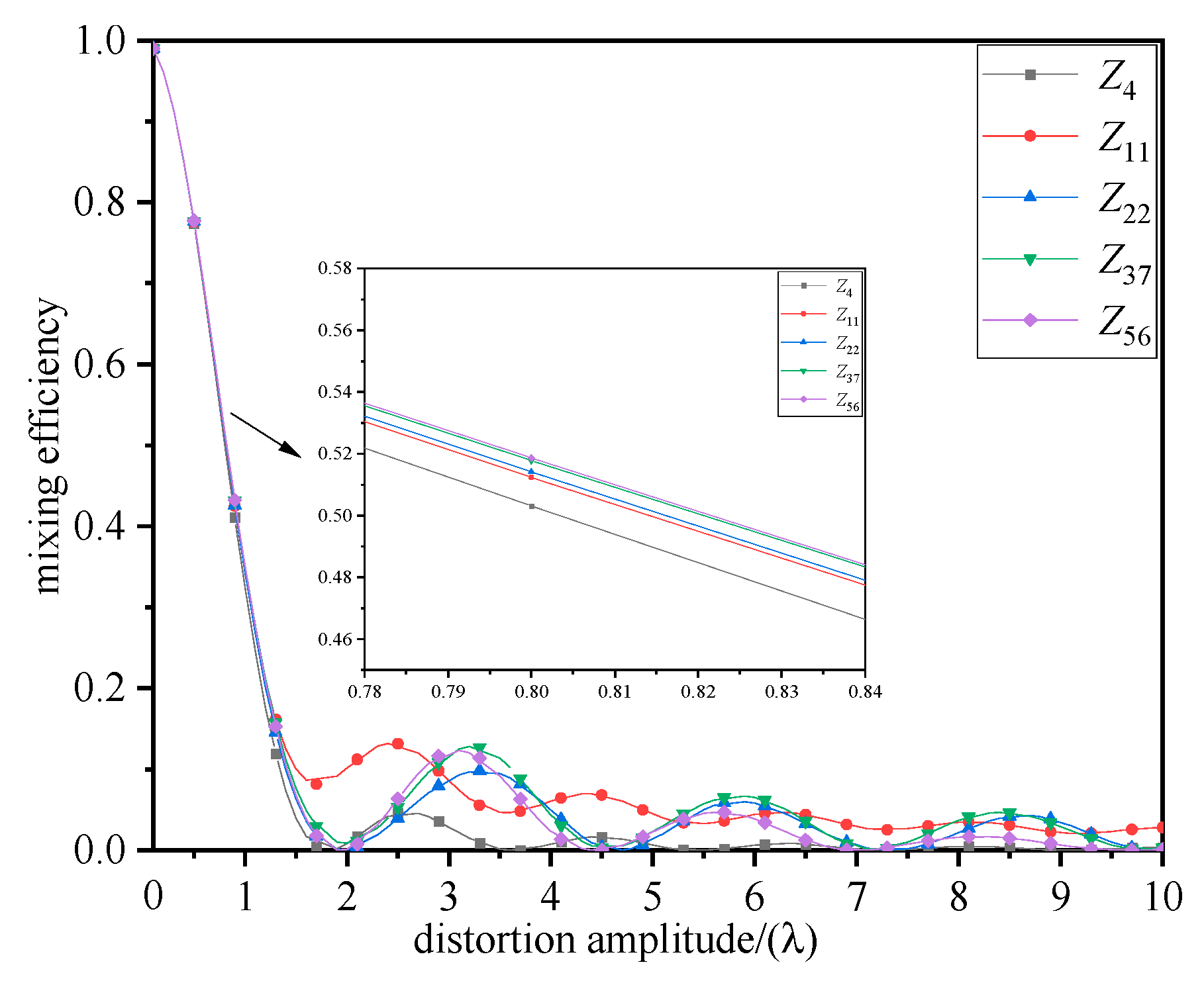
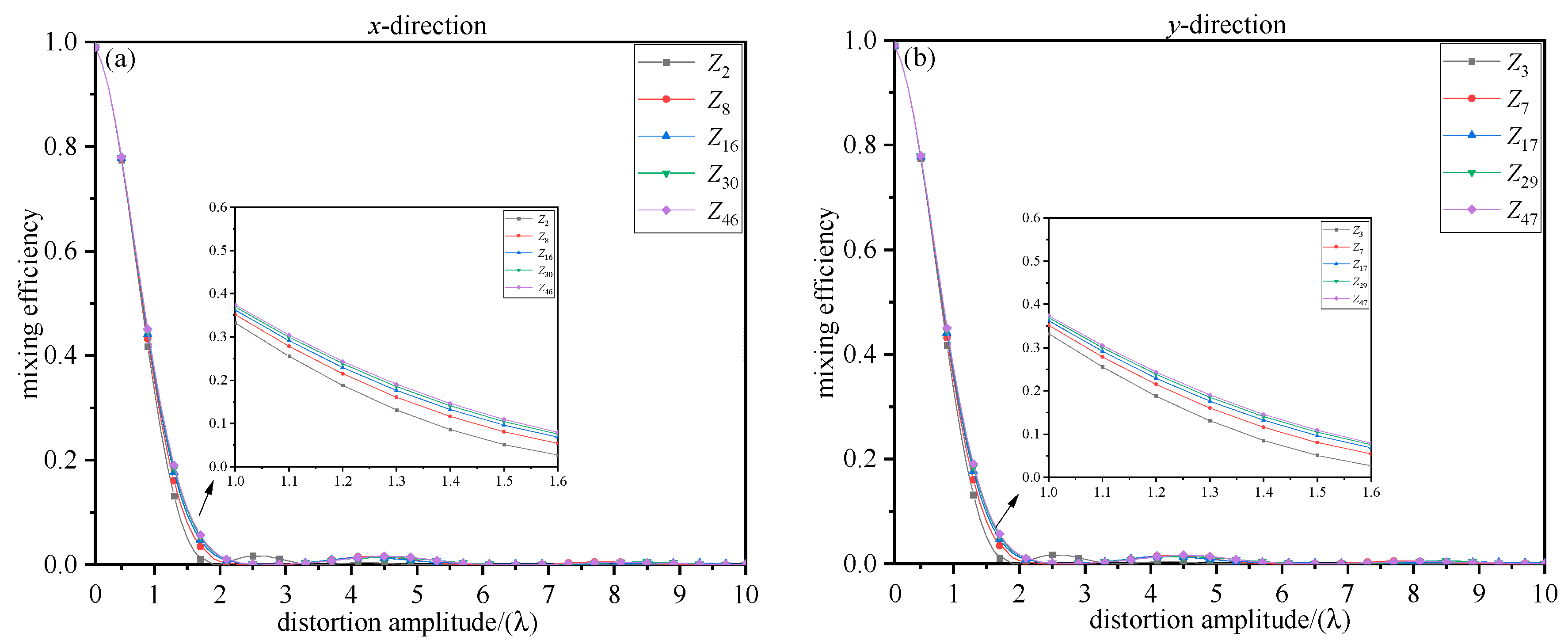
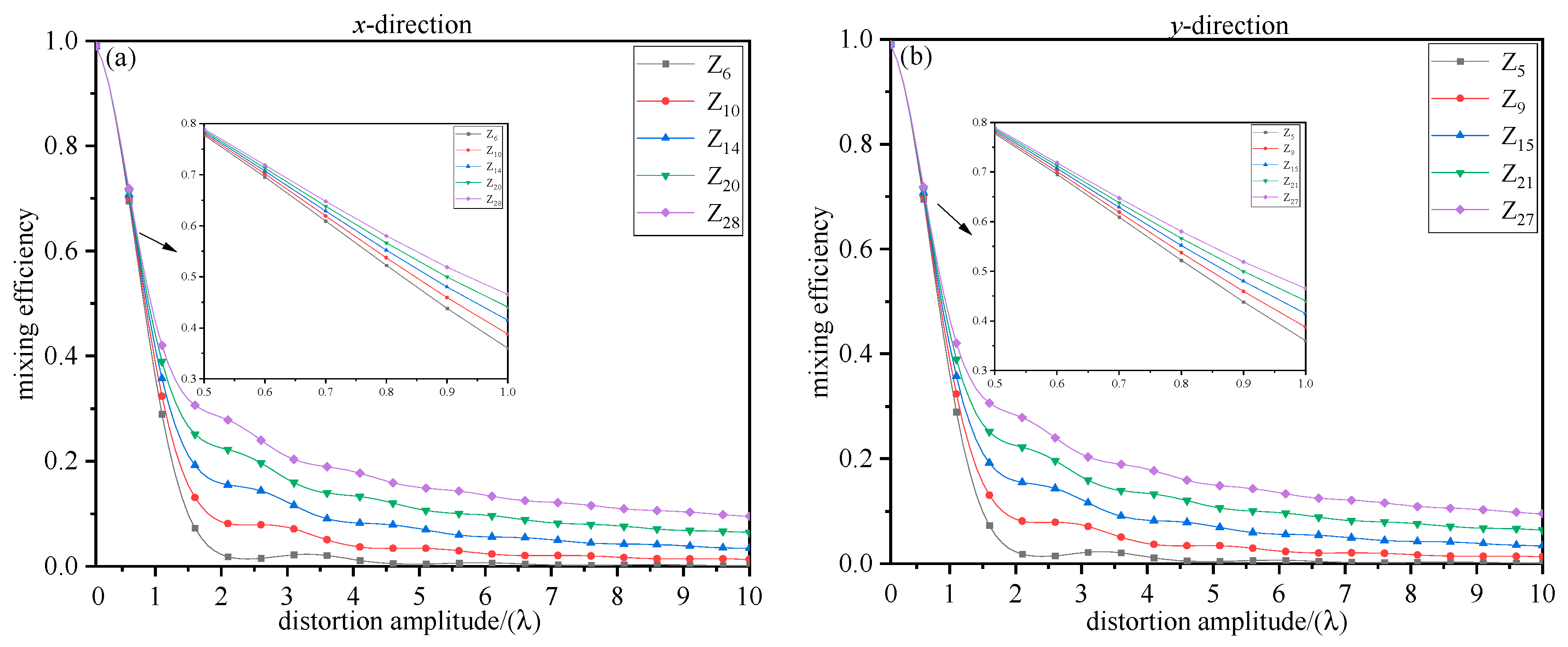
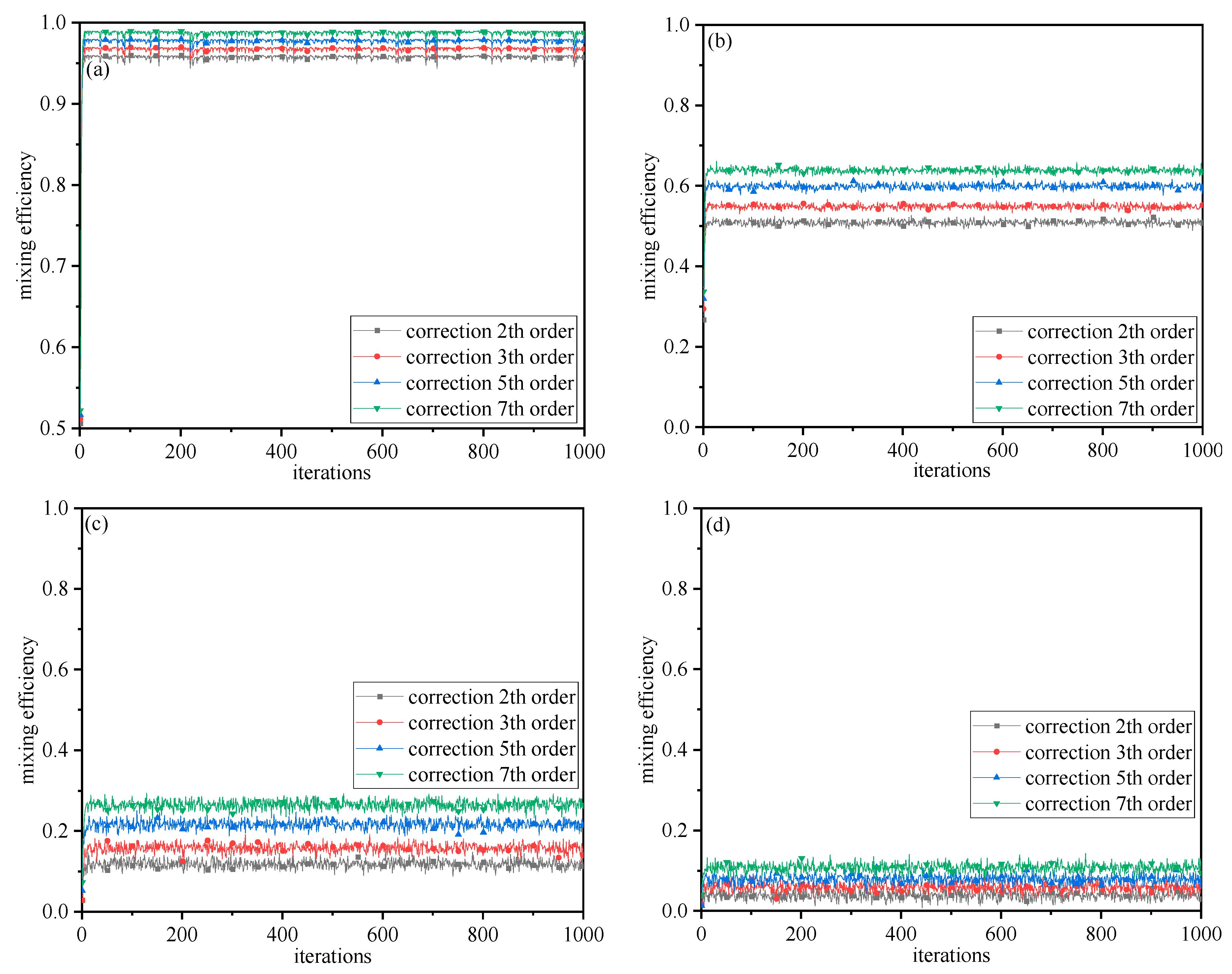
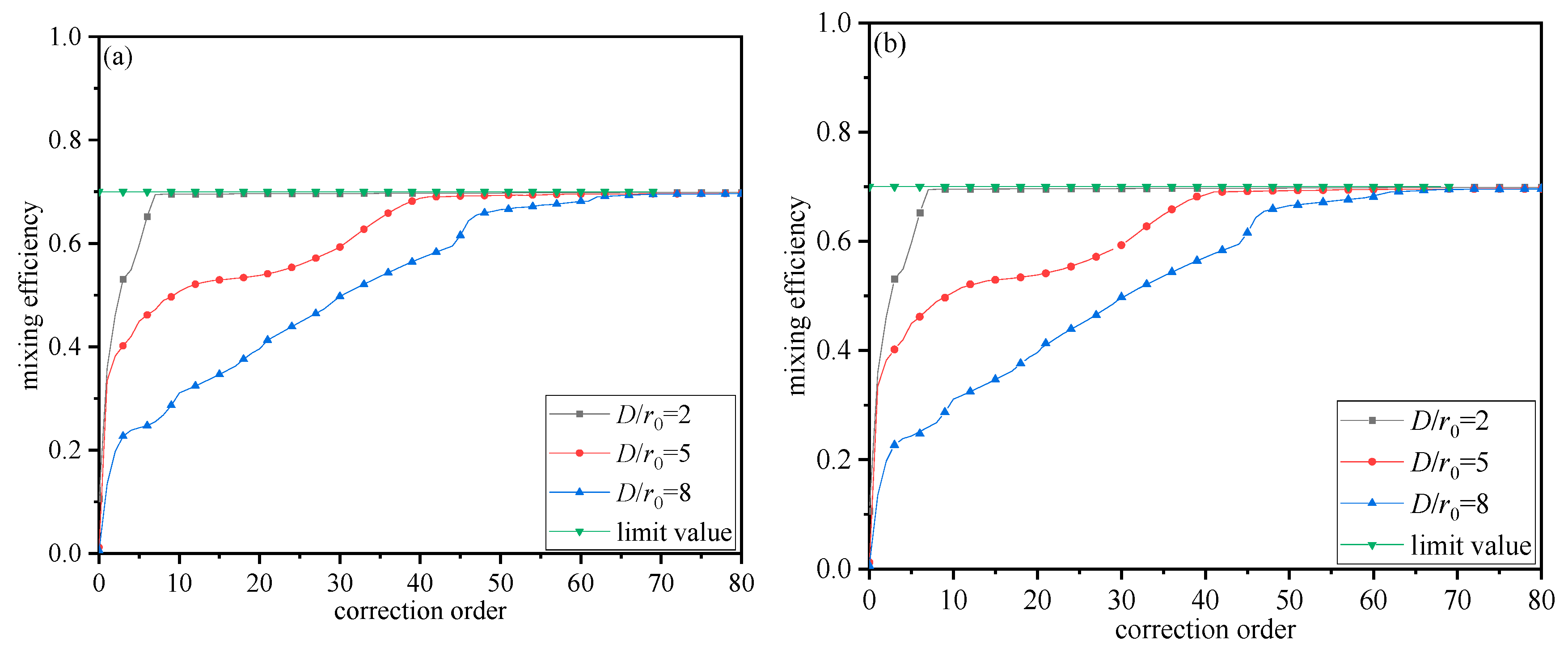
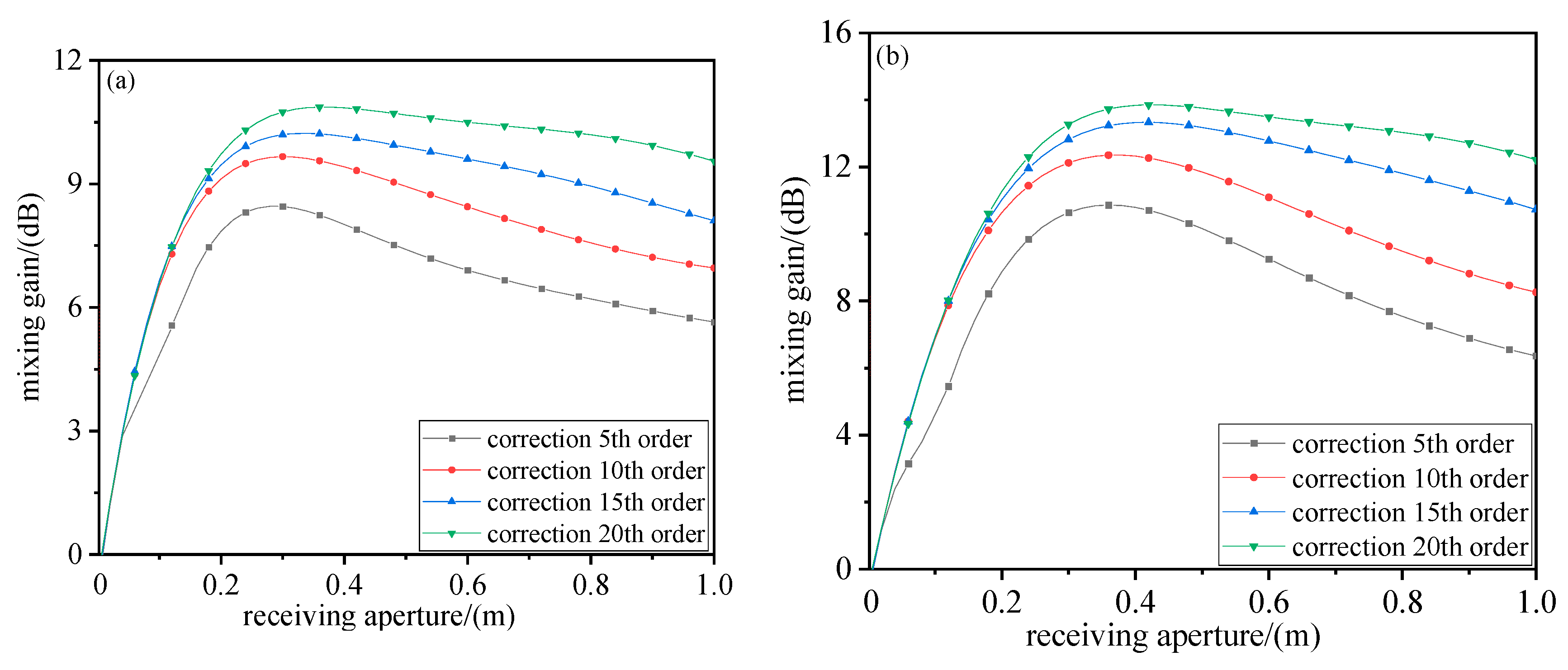
3.1. Effect of Wavefront Distortion on Mixing Efficiency
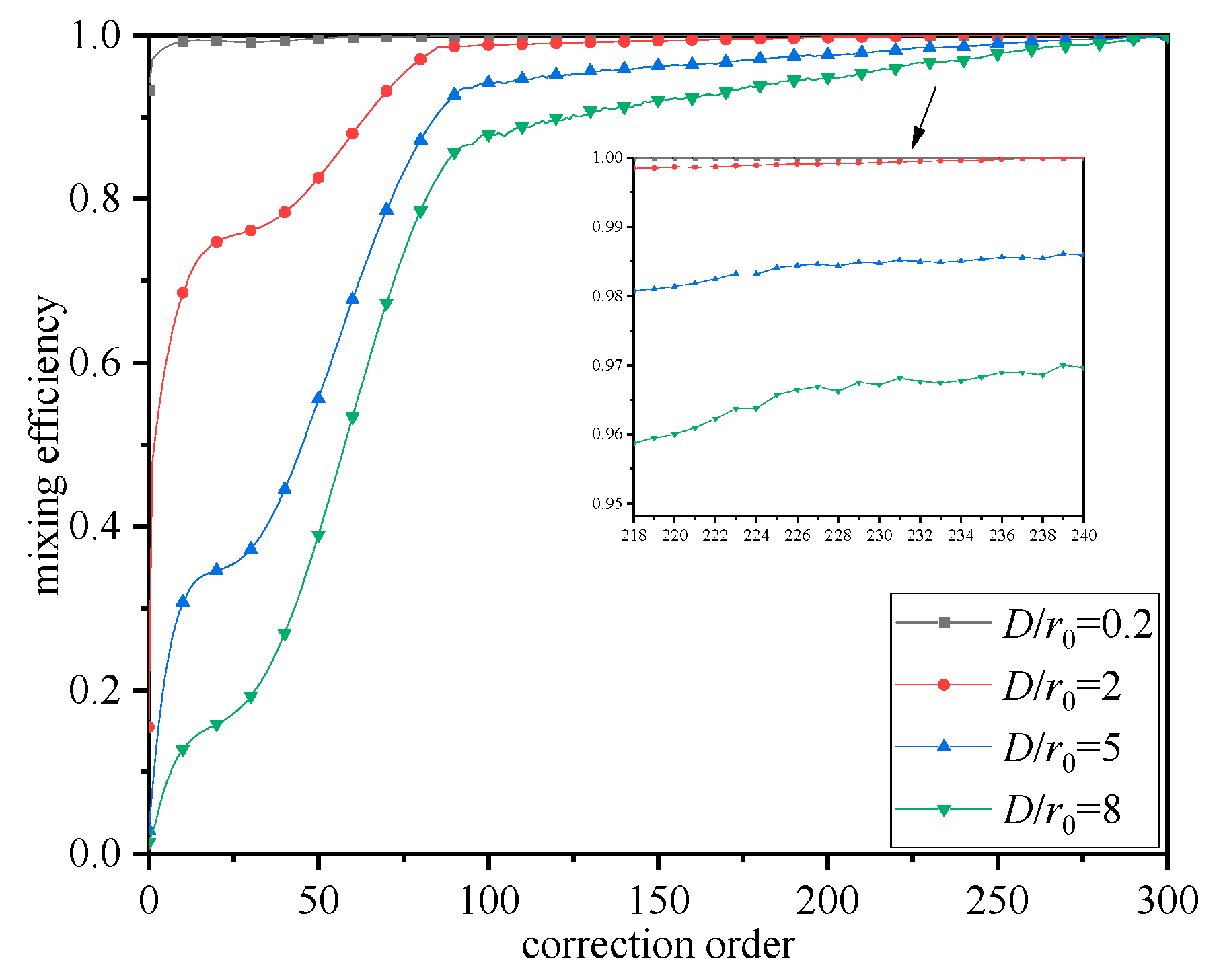
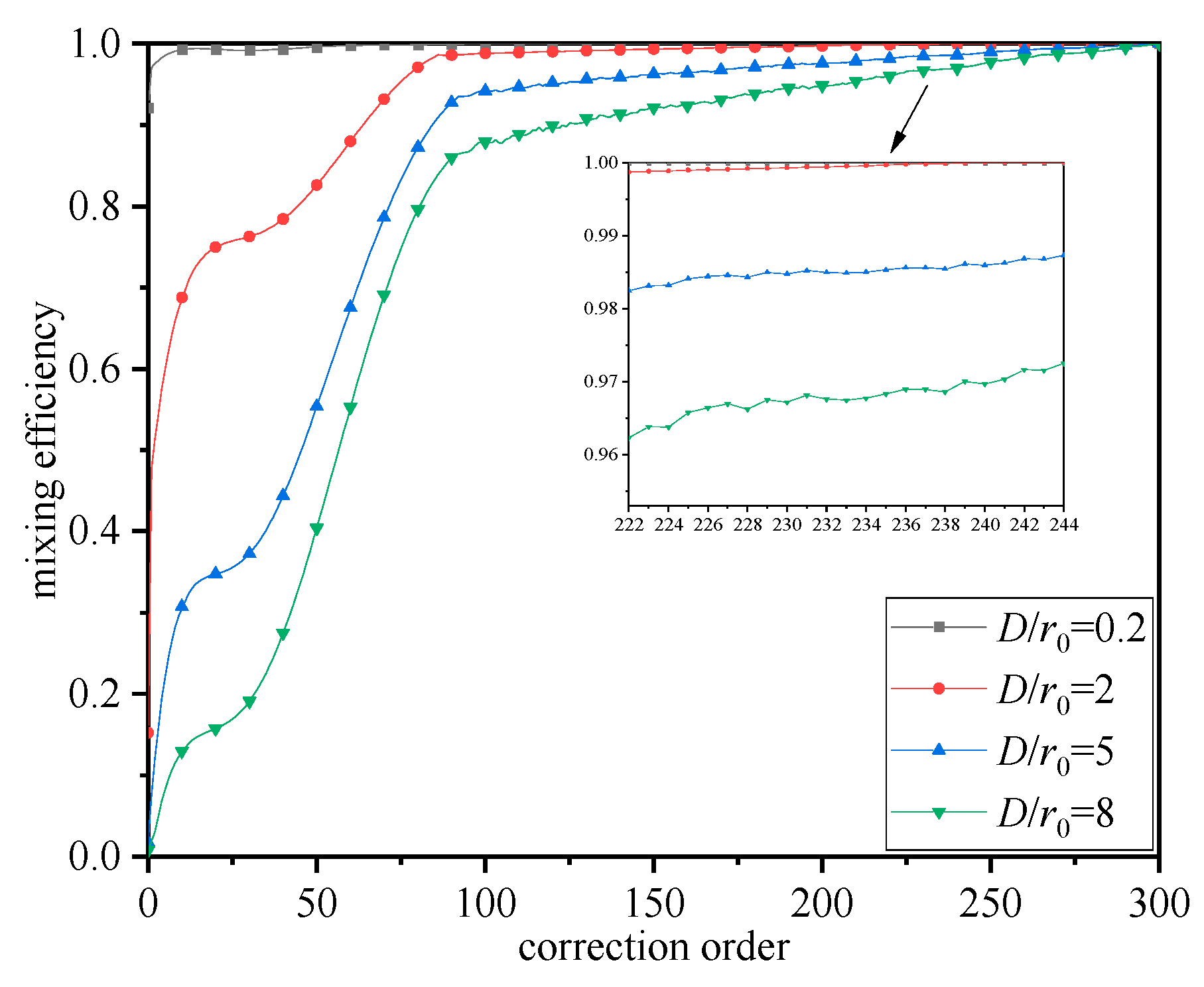
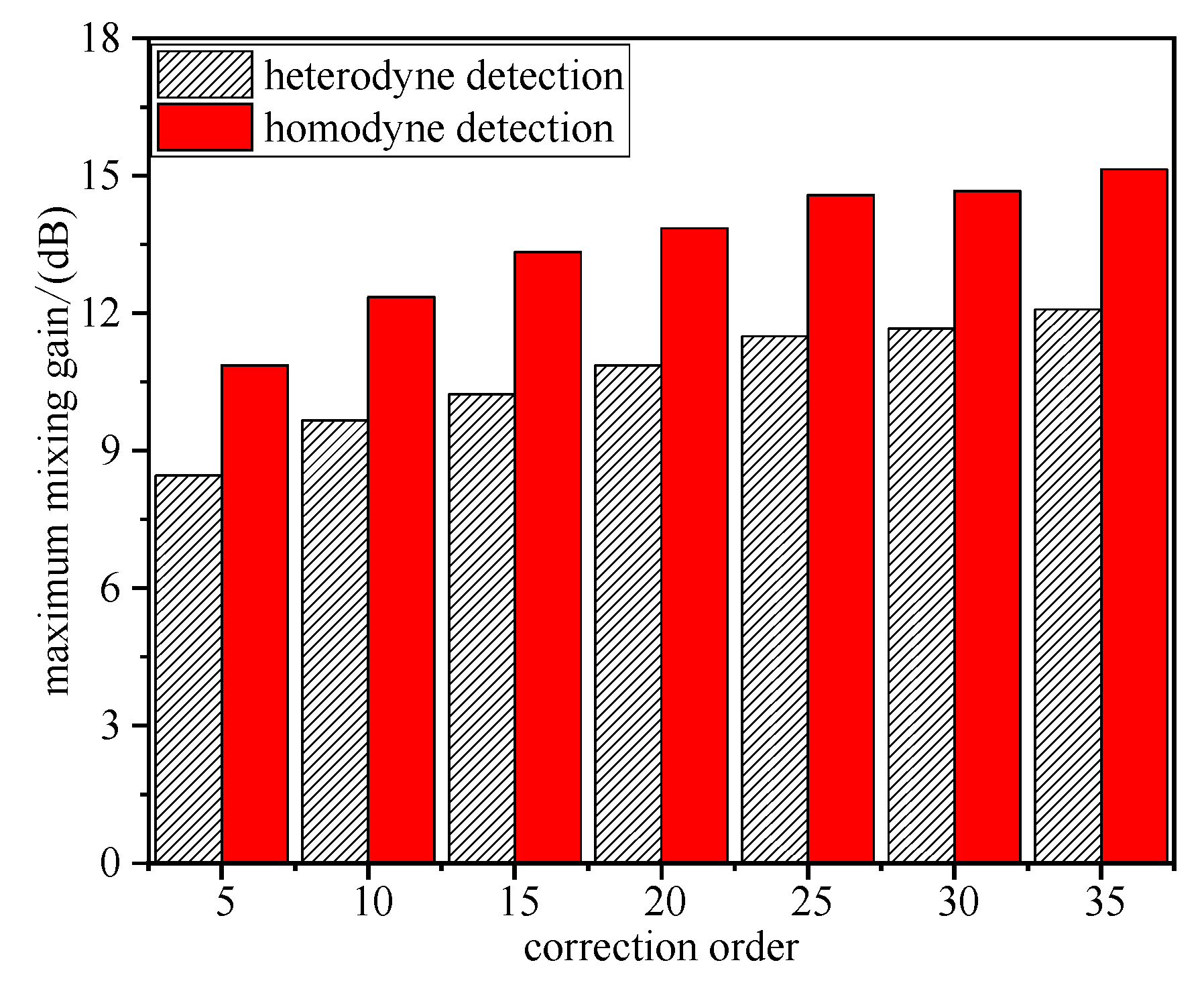
3.2. Evaluation of Mixing Efficiency and Mixing Gain
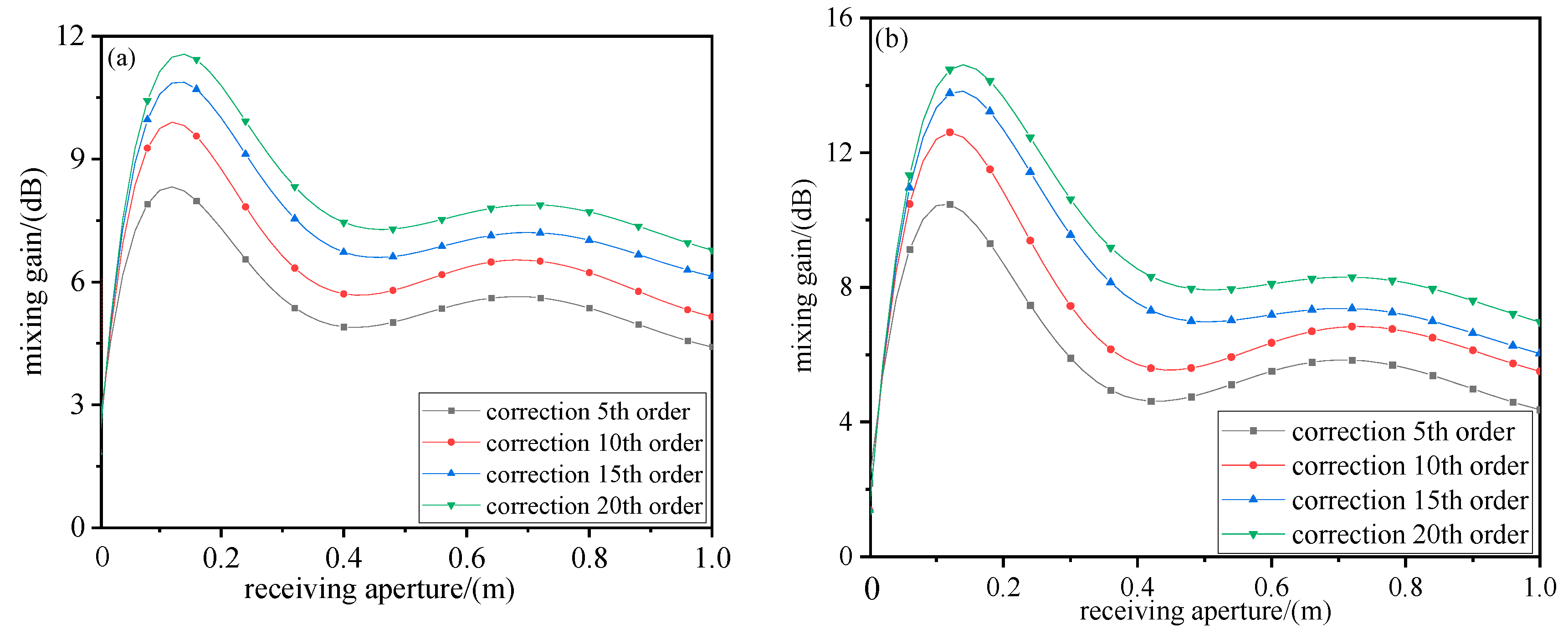
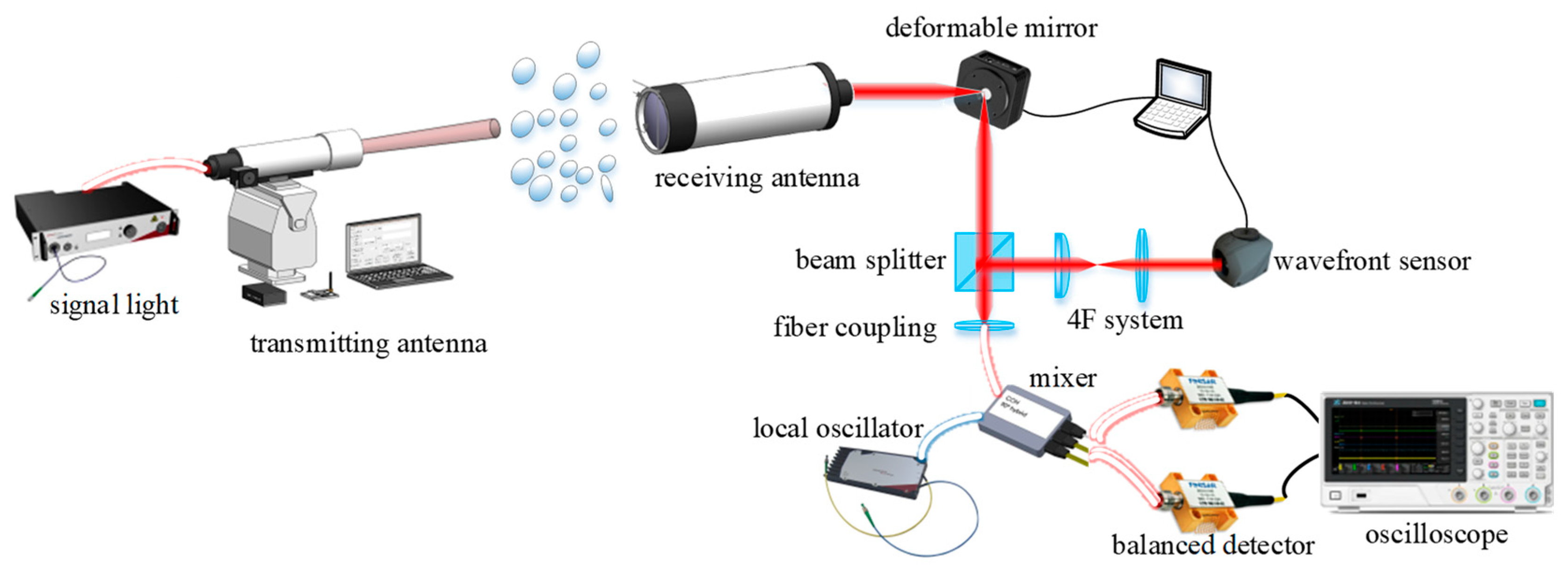
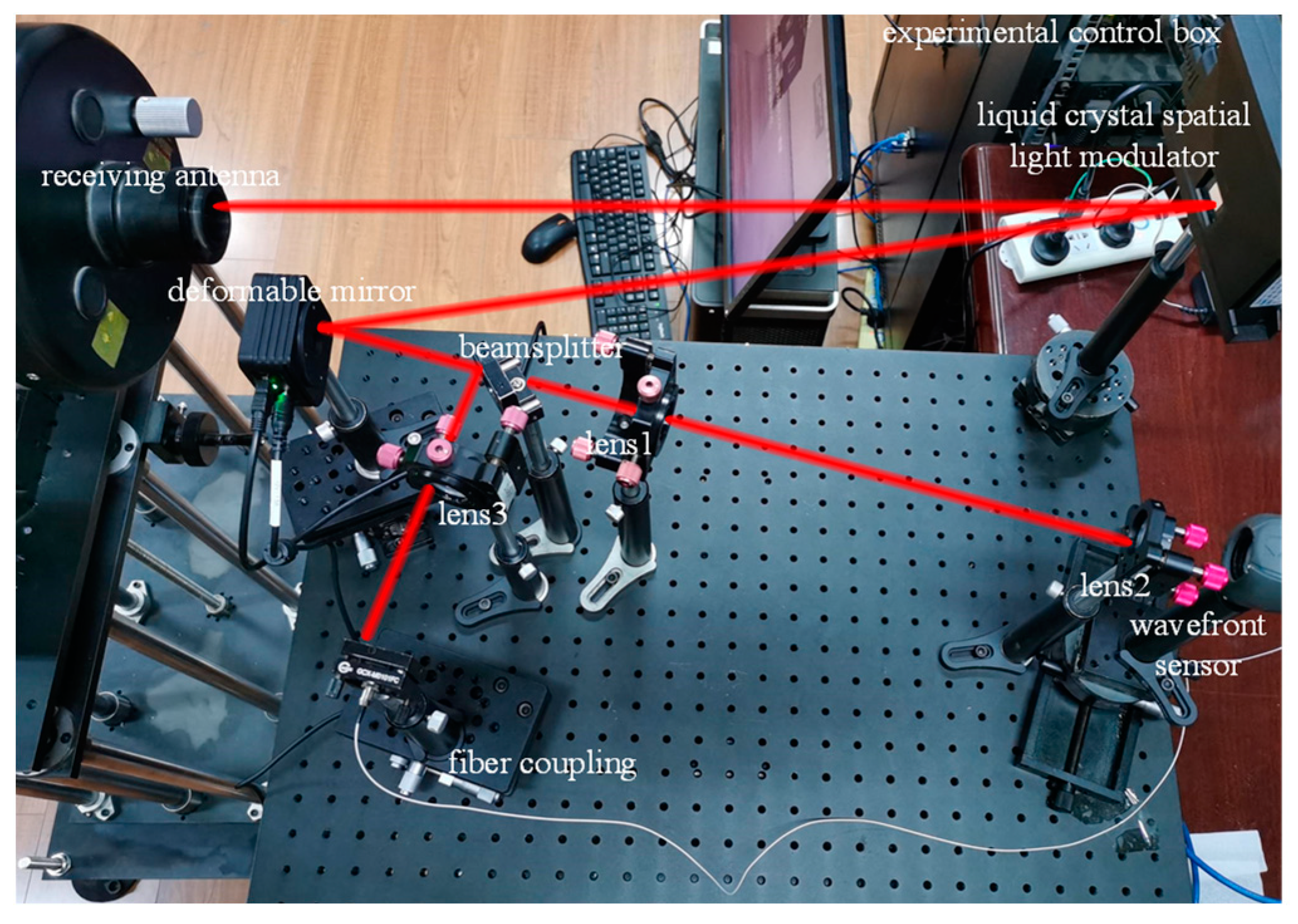
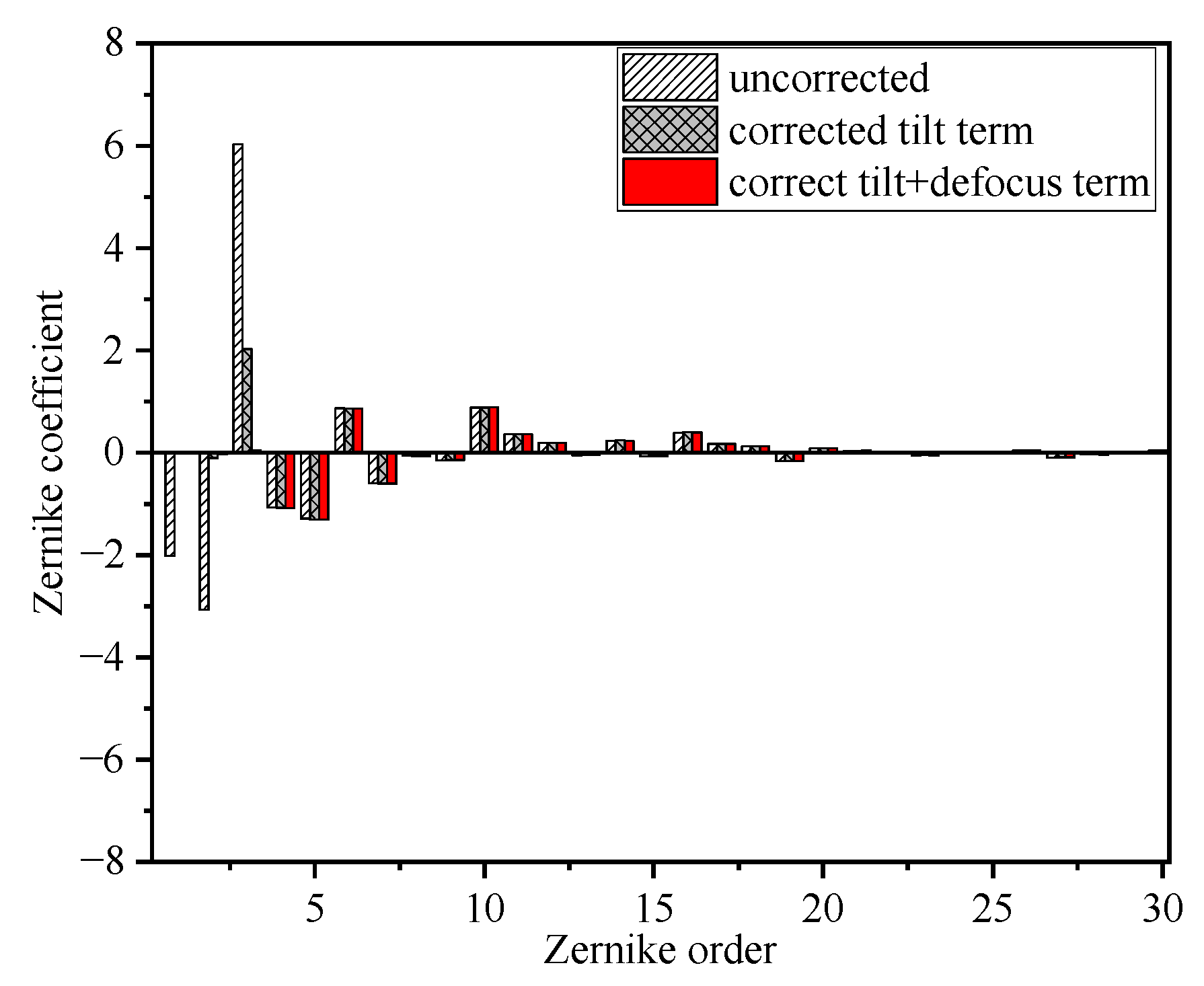
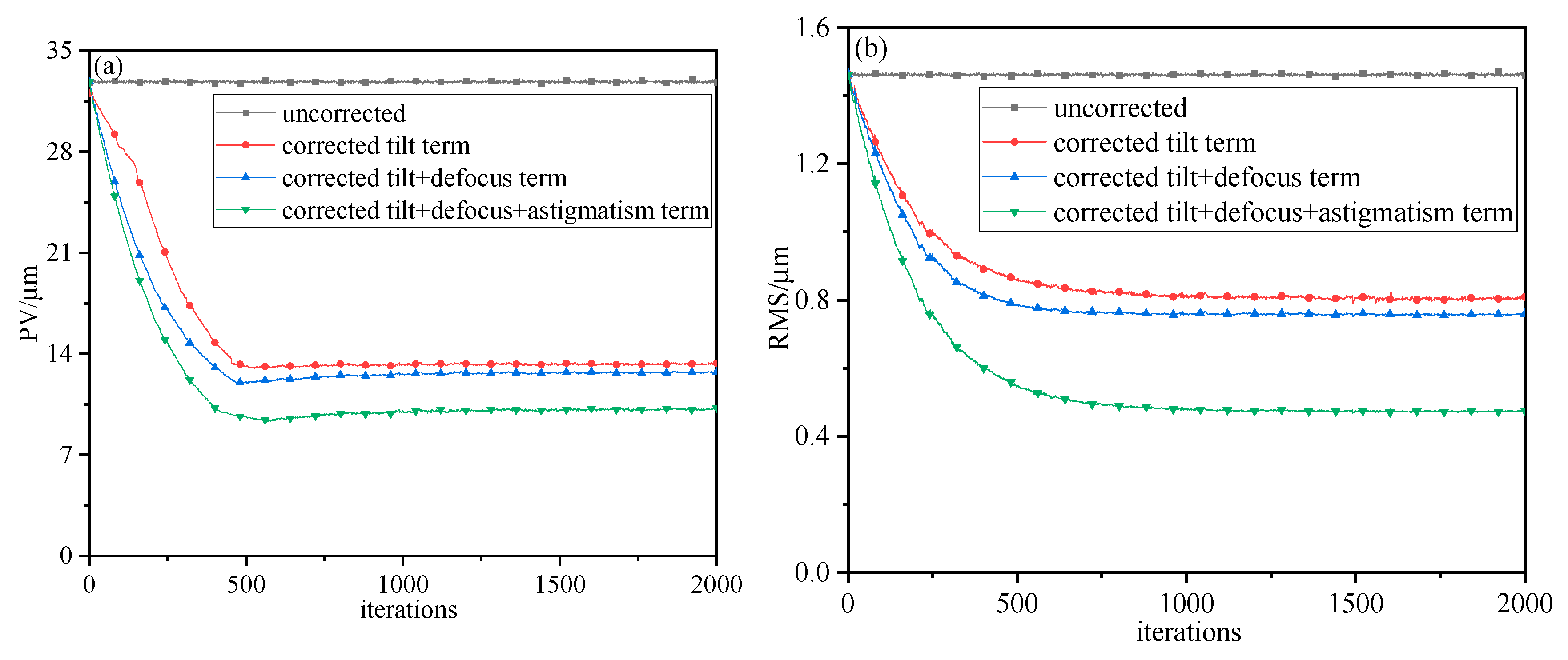
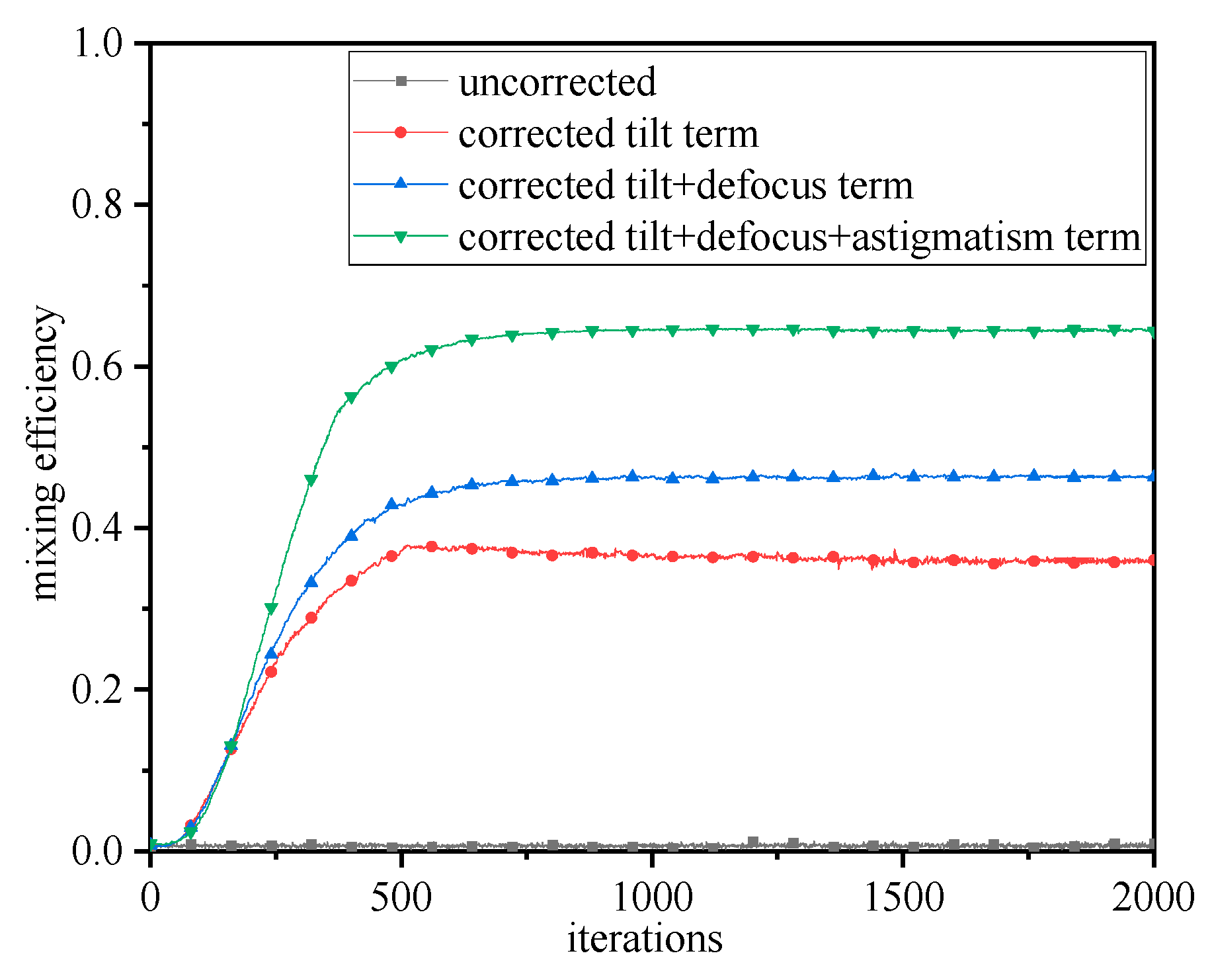
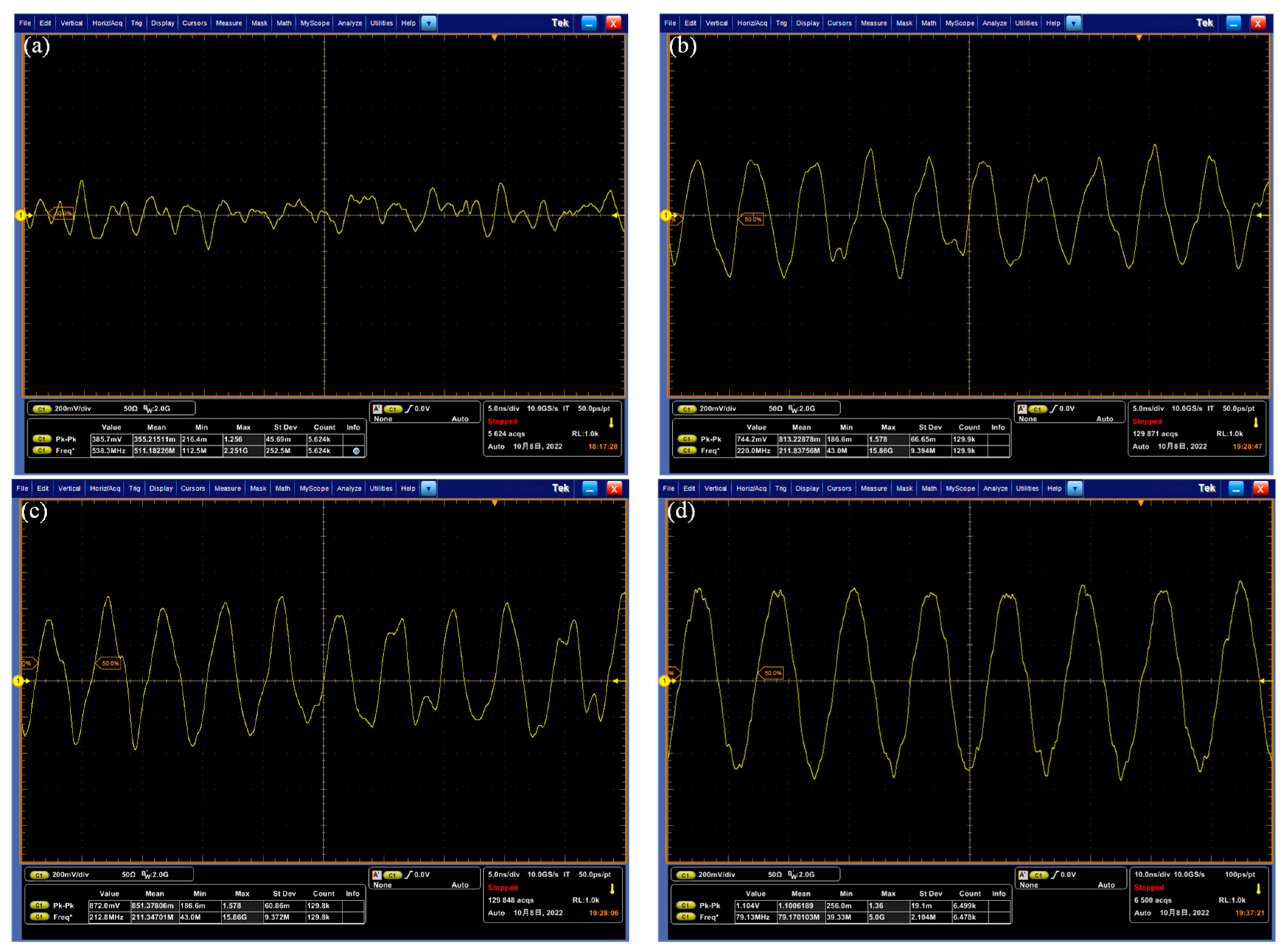
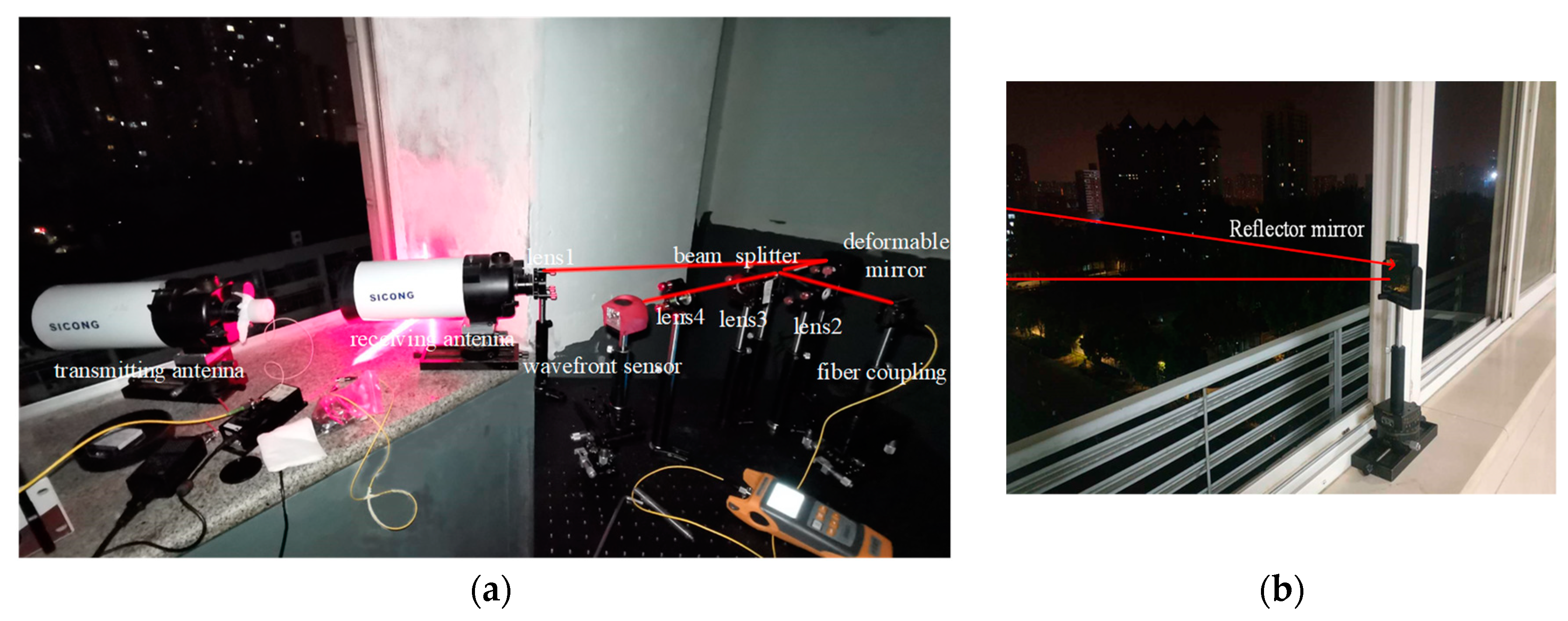
4. Experiment Research
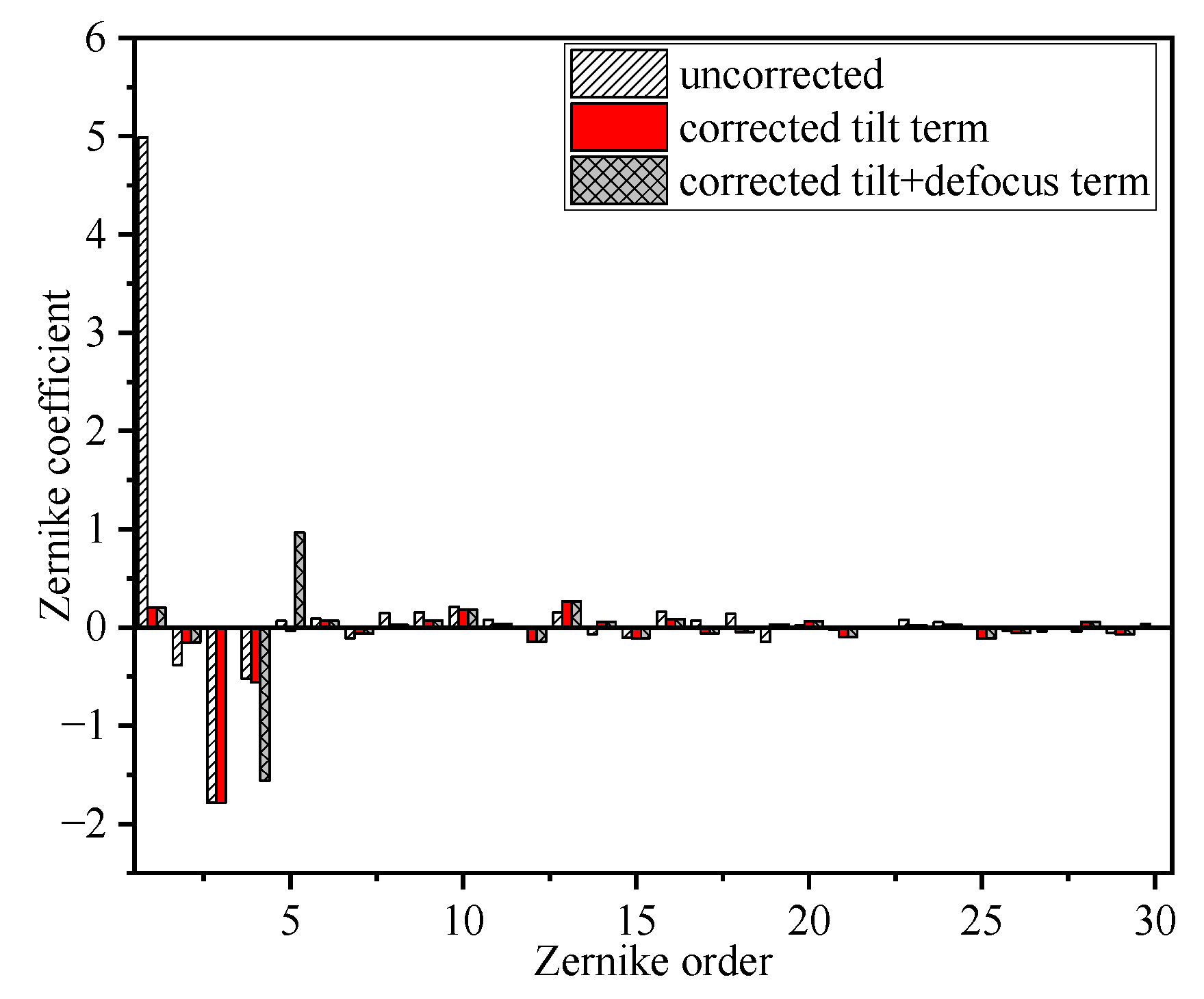
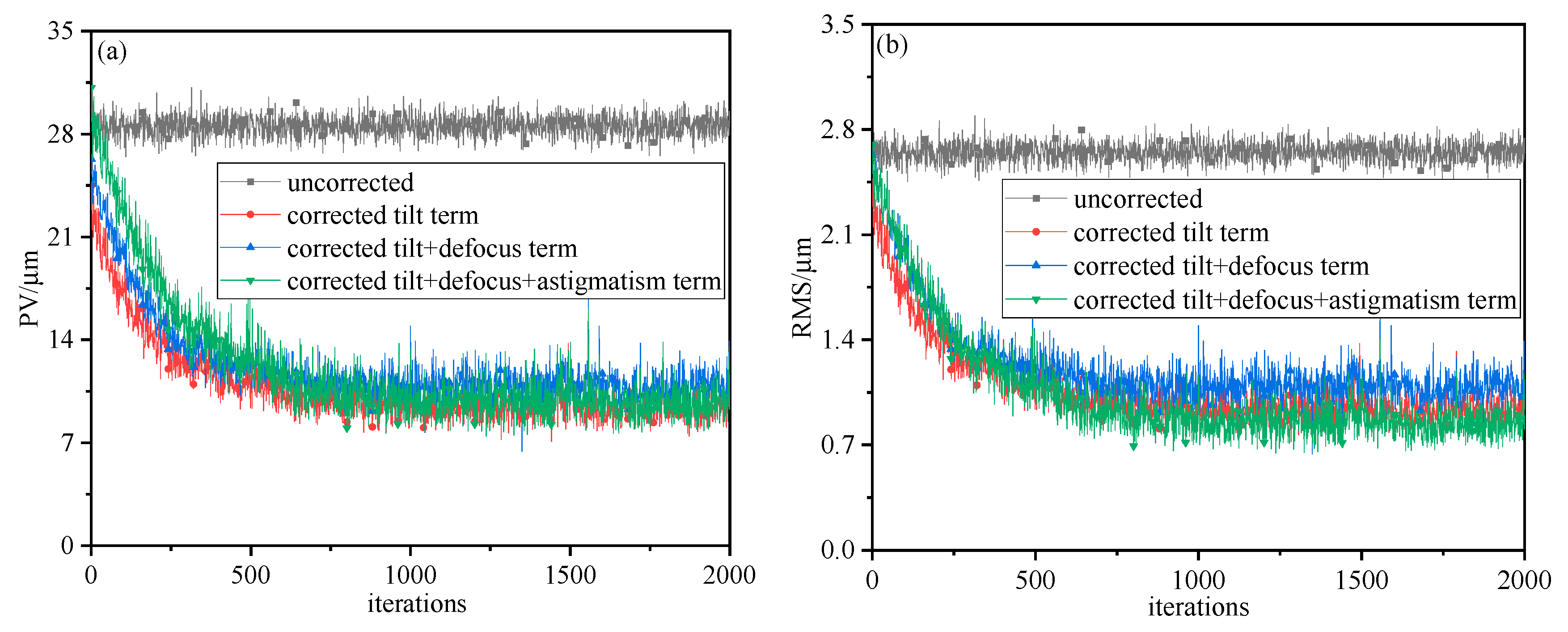
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Liu, Y.J.; Chen, K.; Song, S.; Pan, Y.; Liu, Y.C.; Guo, L. Reinforcement learning aided geometric shaping and self-canceling coherent detection for a PAM4 FSO communication system. J. Opt. Commun. Netw. 2022, 15, 16–28. [Google Scholar] [CrossRef]
- Wang, Y.Y.; Liu, W.C.; Zhou, X.Y. Splitting Receiver With Joint Envelope and Coherent Detection. IEEE Commun. Lett. 2022, 26, 1328–1332. [Google Scholar] [CrossRef]
- Liu, Y.T.; Gao, M.D.; Zeng, X.D.; Liu, F.; Bi, W.H. Factors influencing the applications of active heterodyne detection. Opt. Lasers Eng. 2021, 146, 106694. [Google Scholar] [CrossRef]
- Suleiman, I.; Nielsen, J.A.H.; Guo, X.; Jain, N.; Neergaard-Nielsen, J.; Gehring, T.; Andersen, U.L. 40 km Fiber Transmission of Squeezed Light Measured with a Real Local Oscillator. Quantum Phys. 2022, 7, 1328–1332. [Google Scholar] [CrossRef]
- Ke, X.; Wu, J. Wavefront correction system. In Coherent Optical Wireless Communication Principle and Application, 1st ed.; Li, P., Ed.; China Science Publishing & Media Ltd.: Beijing, China, 2019; Volume 6, pp. 225–252. [Google Scholar]
- Banakh, V.A.; Zhmylevskii, V.V.; Ignatiev, A.B.; Morozov, V.V.; Razenkov, I.A.; Rostov, A.P.; Tsvyk, R.S. Optical-beam wavefront control based on the atmospheric backscatter signal. Quantum Electron. 2015, 45, 153–160. [Google Scholar] [CrossRef]
- Hu, L.J.; Hu, S.W.; Gong, W.; Si, K. Learning-based Shack-Hartmann wavefront sensor for high-order aberration detection. Opt. Express 2019, 27, 33504–33517. [Google Scholar] [CrossRef]
- Zhang, Z.T.; Bharmal, N.; Morris, T.; Liang, Y.H. Laboratory quantification of a plenoptic wavefront sensor with extended objects. Mon. Not. R. Astron. Soc. 2020, 497, 4580–4586. [Google Scholar] [CrossRef]
- Rani, N.; Singh, P.; Kaur, P. Mitigation of Scintillation Effects in WDM-FSO System Using Homodyne Detection. Optik 2021, 248, 168165. [Google Scholar] [CrossRef]
- Yan, X.; Cao, C.Q.; Zhang, W.R.; Zeng, X.D.; Feng, Z.J.; Wu, Z.Y.; Wang, T. Wavefront Detection and Compensation Technology Based on Signal Light Nutation under Atmospheric Turbulence. IEEE Commun. Lett. 2021, 25, 3340–3344. [Google Scholar] [CrossRef]
- Zhong, Z.Q.; Zhang, X.; Zhang, B.; Yuan, X. Mitigation of atmospheric turbulence effect by light beams carrying self-rotating wavefront. Opt. Express 2022, 30, 24421–24430. [Google Scholar] [CrossRef]
- Betanzos-Torres, M.A.; Castillo-Mixcóatl, J.; Muoz-Aguirre, S.; Beltrán-Pérez, G. Adaptive optics system simulator—ScienceDirect. Opt. Laser Technol. 2018, 105, 118–128. [Google Scholar] [CrossRef]
- Lechner, D.; Zepp, A.; Eichhorn, M.; Gladysz, S. Adaptable Shack-Hartmann wavefront sensor with diffractive lenslet arrays to mitigate the effects of scintillation. Opt. Express 2020, 28, 36188–36205. [Google Scholar] [CrossRef] [PubMed]
- Zhang, M.; Jiang, L.; Huang, Z. Adaptive optics technology and urban horizontal link laser communication system. Opt. Eng. 2018, 60, 116107. [Google Scholar] [CrossRef]
- Norris, B.R.M.; Wei, J.; Betters, C.H.; Wong, A.; Leon-Saval, S.G. An all-photonic focal-plane wavefront sensor. Nat. Commun. 2020, 11, 5335. [Google Scholar] [CrossRef] [PubMed]
- Quintavalla, M.; Bergomi, M.; Magrin, D.; Bonora, S.; Ragazzoni, R. Correction of non-common path aberrations in pyramid wavefront sensors to recover the optimal magnitude gain using a deformable lens. Appl. Opt. 2020, 59, 5151–5157. [Google Scholar] [CrossRef]
- Yang, Y.F.; Yan, C.X.; Hu, C.H.; Wu, C.J. Modified heterodyne efficiency for coherent laser communication in the presence of polarization aberrations. Opt. Express 2017, 25, 7567–7591. [Google Scholar] [CrossRef]
- Stotts, L.B.; Andrews, L.C. Adaptive optics model characterizing turbulence mitigation for free space optical communications link budgets. Opt. Express 2021, 29, 20307–20321. [Google Scholar] [CrossRef]
- Hu, L.J.; Hu, S.W.; Li, Y.N.; Gong, W.; Si, K. Reliability of wavefront shaping based on coherent optical adaptive technique in deep tissue focusing. J. Biophotonics 2021, 13, e201900245. [Google Scholar] [CrossRef]
- Wu, J.L.; Ke, X.Z.; Yang, Y.Q.; Liang, J.Y.; Liu, M.Y. Correction of Distorted Wavefront Using Dual Liquid Crystal Spatial Light Modulators. Photonics 2022, 9, 426. [Google Scholar] [CrossRef]
- Xu, D.L.; Yue, P.; Yi, X. A Theoretical Analysis Method of OAM-Based FSO Error Performance. Acta Electron. Sin. 2021, 49, 1934–1944. [Google Scholar]
- Liu, Q.; Chew, K.-H.; Huang, Y.; Liu, C.; Hu, X.; Li, Y.; Chen, R.-P. Effect of Twisting Phases on the Polarization Dynamics of a Vector Optical Field. Photonics 2022, 9, 722. [Google Scholar] [CrossRef]
- Ren, J.Y.; Sun, H.Y.; Zhang, L.X.; Zhao, Y.Z. Receiving characteristics analysis of large dynamic range based on four-optic coherent mixing. Optik 2020, 221, 165348. [Google Scholar] [CrossRef]
- Karar, A.S.; Falou, A.R.E.; Barakat, J.M.H.; Gürkan, Z.N.; Zhong, K. Recent Advances in Coherent Optical Communications for Short-Reach: Phase Retrieval Methods. Photonics 2023, 10, 308. [Google Scholar] [CrossRef]
- Magidi, S.; Jabeena, A. Free Space Optics, Channel Models and Hybrid Modulation Schemes: A Review. Wirel. Pers. Commun. 2021, 119, 2951–2974. [Google Scholar] [CrossRef]
- Fice, M.J.; Chiuchiarelli, A.; Ciaramella, E.; Seeds, A.J. Homodyne coherent optical receiver using an optical injection phase-lock loop. J. Light. Technol. 2011, 29, 1152–1164. [Google Scholar] [CrossRef]
- Liu, C.; Chen, S.; Li, X.Y.; Xian, H. Performance evaluation of adaptive optics for atmospheric coherent laser communications. Opt. Express 2014, 22, 15554–15563. [Google Scholar] [CrossRef]
- Geng, C.; Li, F.; Zuo, J.; Liu, J.Y.; Yang, X.; Yu, T.; Jiang, J.L.; Li, X.Y. Fiber laser transceiving and wavefront aberration mitigation with adaptive distributed aperture array for free-space optical communications. Opt. Lett. 2020, 45, 1906–1909. [Google Scholar] [CrossRef]
- Konwar, S.; Boruah, B.R. Leveraging the orthogonality of Zernike modes for robust free-space optical communication. Commun. Phys. 2020, 3, 203. [Google Scholar] [CrossRef]
- Erol, B.; Altiner, B.; Adali, E.; Delibasi, A. H-infinity Suboptimal controller design for adaptive optic systems. Trans. Inst. Meas. Control. 2019, 41, 2100–2113. [Google Scholar] [CrossRef]
- Paneva-Konovska, J. A family of hyper-Bessel functions and convergent series in them. Fract. Calc. Appl. Anal. 2014, 17, 1001–1015. [Google Scholar] [CrossRef][Green Version]
- Soto-Quiros, P.; Torokhti, A. Optimal transforms of random vectors: The case of successive optimizations. Signal Process. 2017, 132, 183–196. [Google Scholar] [CrossRef]
- Im, C.S.; Lee, W.B.; Gwon, J.Y.; Lee, S.S. Float optical phased array receiver incorporating an on-chip metalens concentrator. Opt. Lett. 2022, 47, 2060–2063. [Google Scholar] [CrossRef] [PubMed]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, S.; Xing, T.; Ke, C.; Liang, J.; Ke, X. Effect of Wavefront Distortion on the Performance of Coherent Detection Systems: Theoretical Analysis and Experimental Research. Photonics 2023, 10, 493. https://doi.org/10.3390/photonics10050493
Yang S, Xing T, Ke C, Liang J, Ke X. Effect of Wavefront Distortion on the Performance of Coherent Detection Systems: Theoretical Analysis and Experimental Research. Photonics. 2023; 10(5):493. https://doi.org/10.3390/photonics10050493
Chicago/Turabian StyleYang, Shangjun, Tian Xing, Chenghu Ke, Jingyuan Liang, and Xizheng Ke. 2023. "Effect of Wavefront Distortion on the Performance of Coherent Detection Systems: Theoretical Analysis and Experimental Research" Photonics 10, no. 5: 493. https://doi.org/10.3390/photonics10050493
APA StyleYang, S., Xing, T., Ke, C., Liang, J., & Ke, X. (2023). Effect of Wavefront Distortion on the Performance of Coherent Detection Systems: Theoretical Analysis and Experimental Research. Photonics, 10(5), 493. https://doi.org/10.3390/photonics10050493




