Optical and Mechanical Performance and Feasibility Analysis of Meter-Level Corrector Lenses for Survey Telescope
Abstract
1. Introduction
1.1. Optical System
1.2. Common Optical Systems
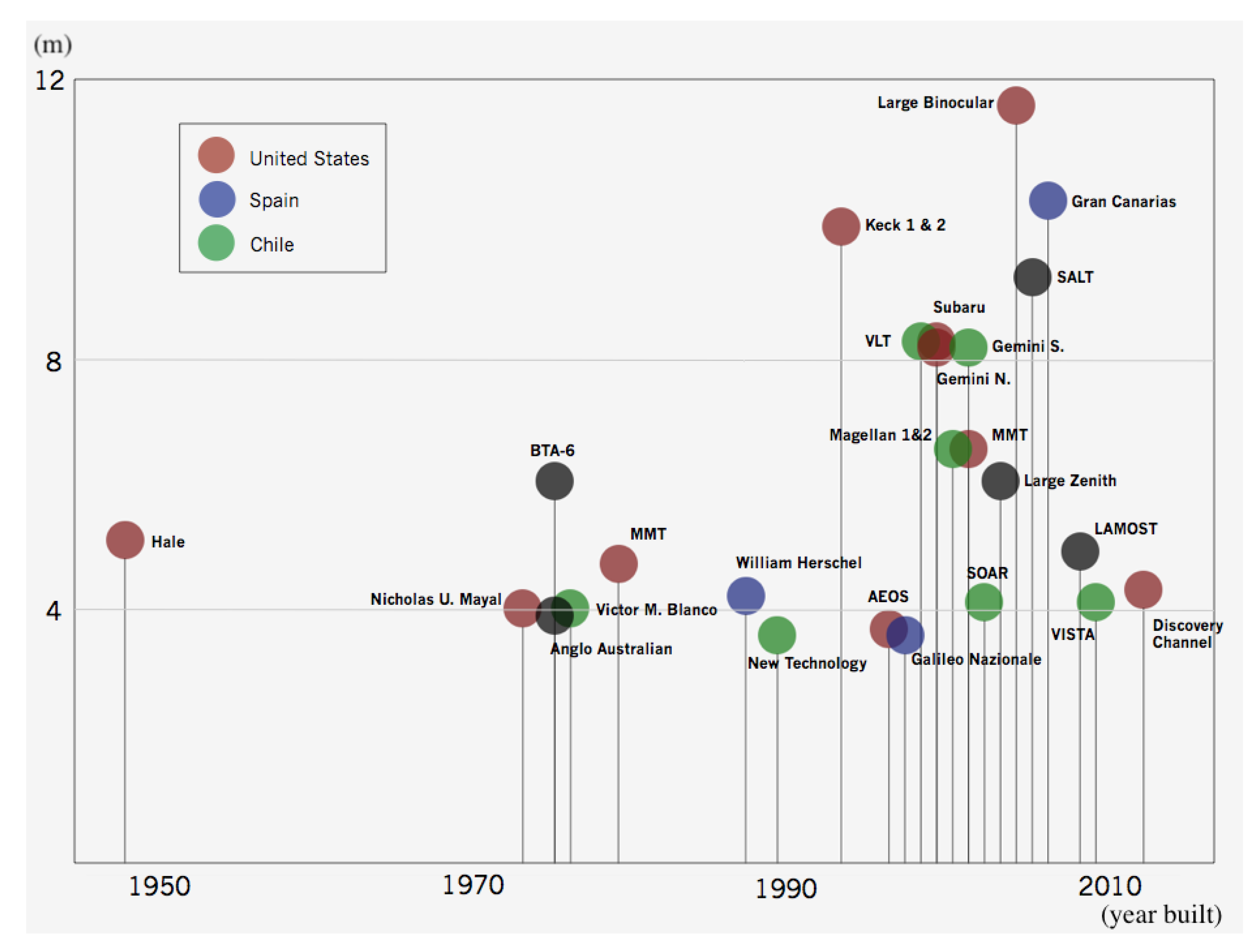
1.3. Typical Telescopes in the Form of Prime Focus
- 2.
- 8.2 m Subaru Telescope
2. Materials and Methods
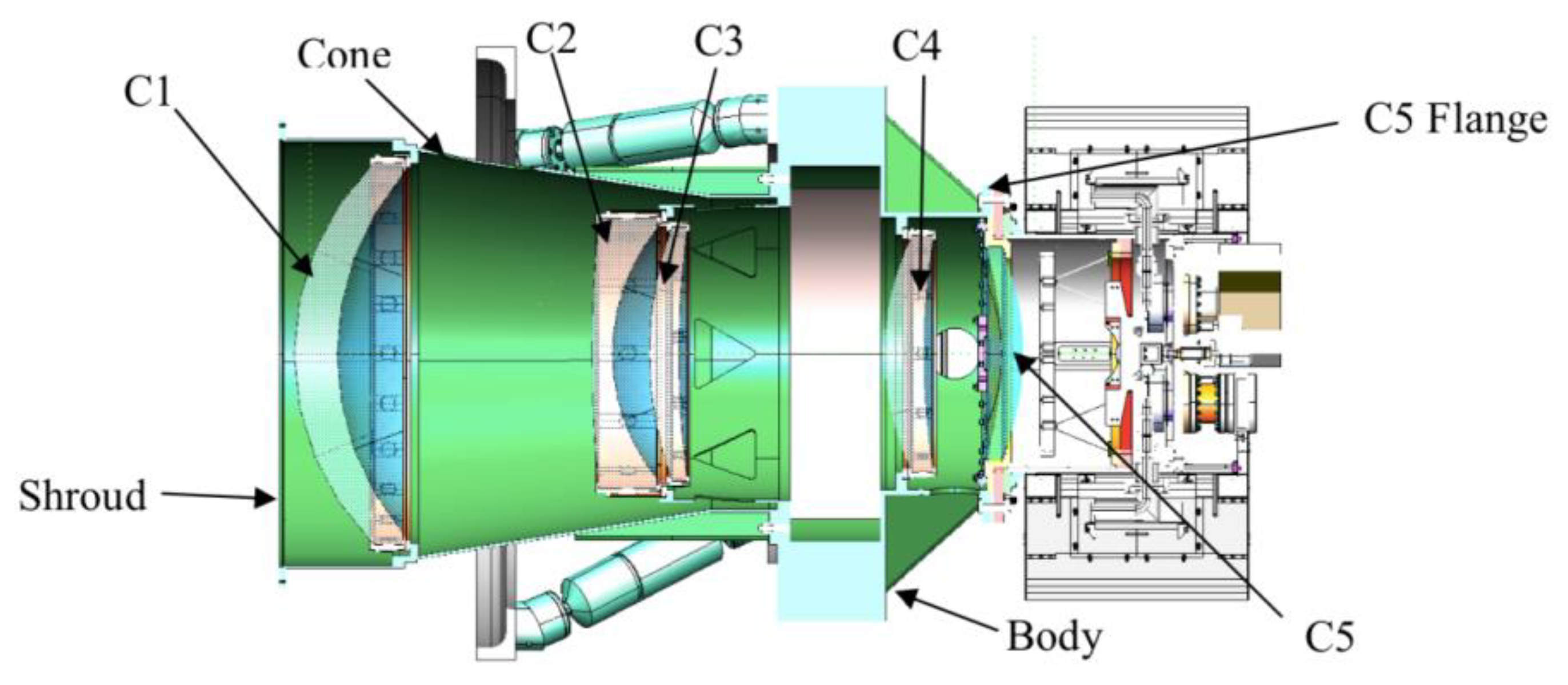
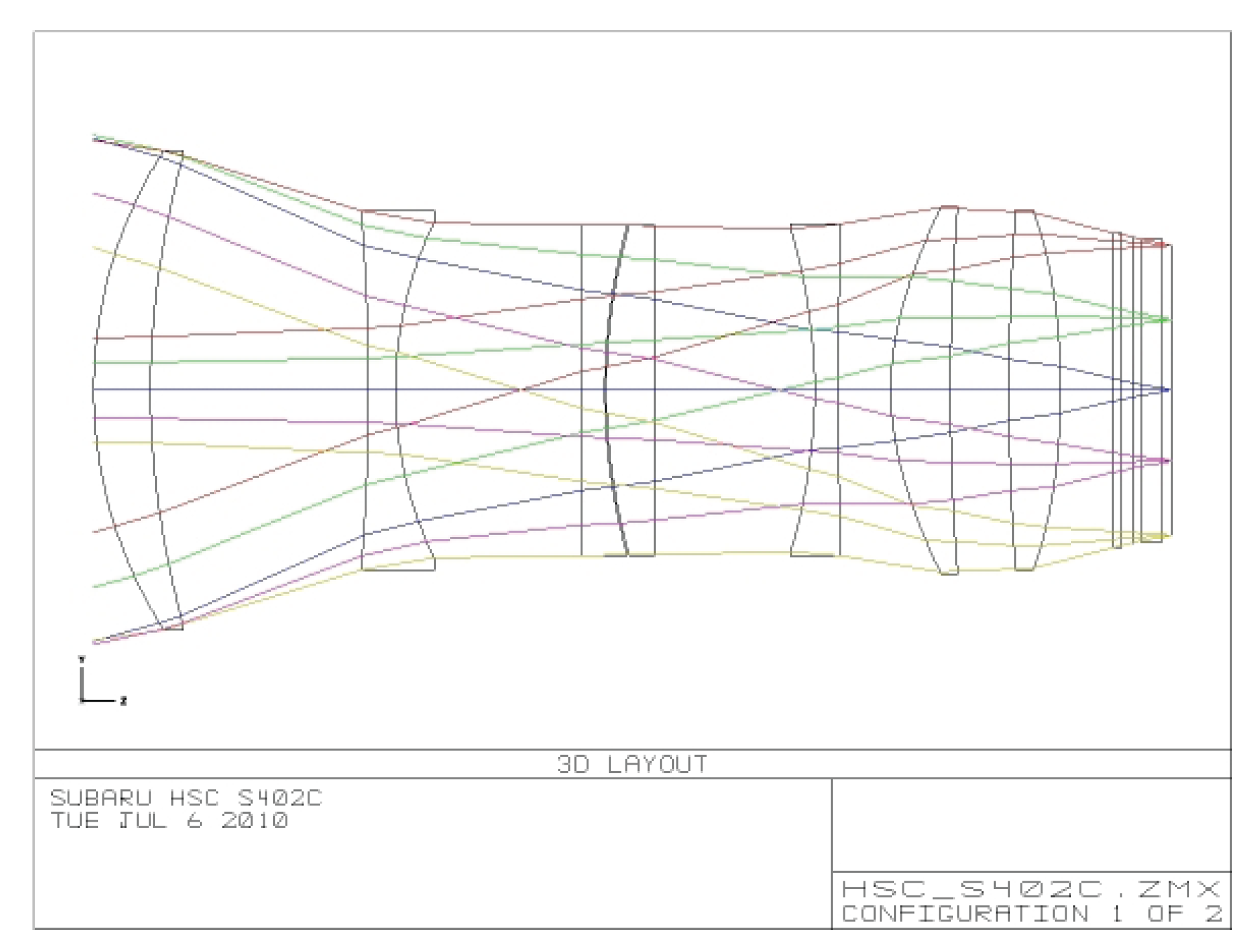
2.1. Our Optical System Design
2.2. Integrated Optomechanical Analysis
2.2.1. Analytical Method
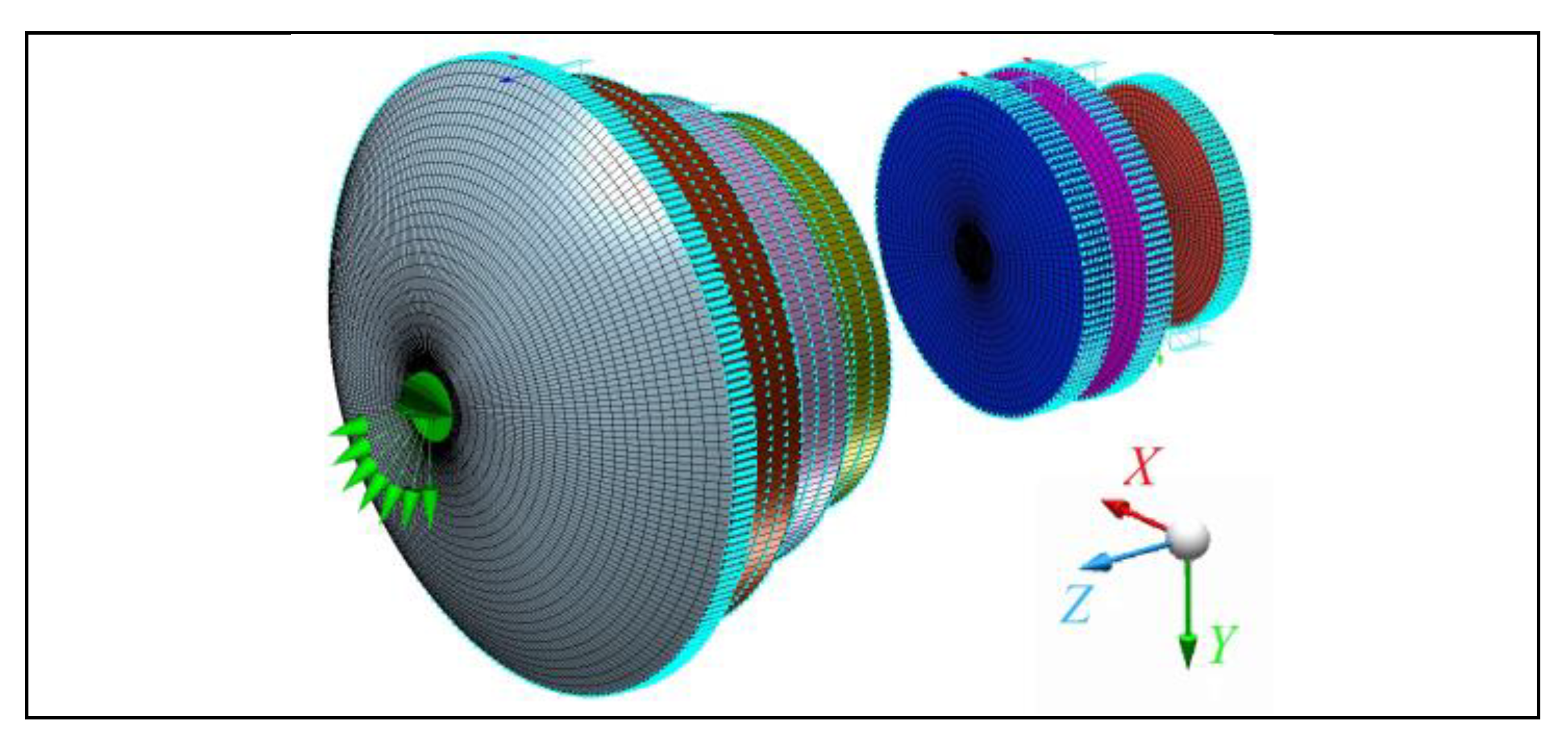
2.2.2. Finite Element Model
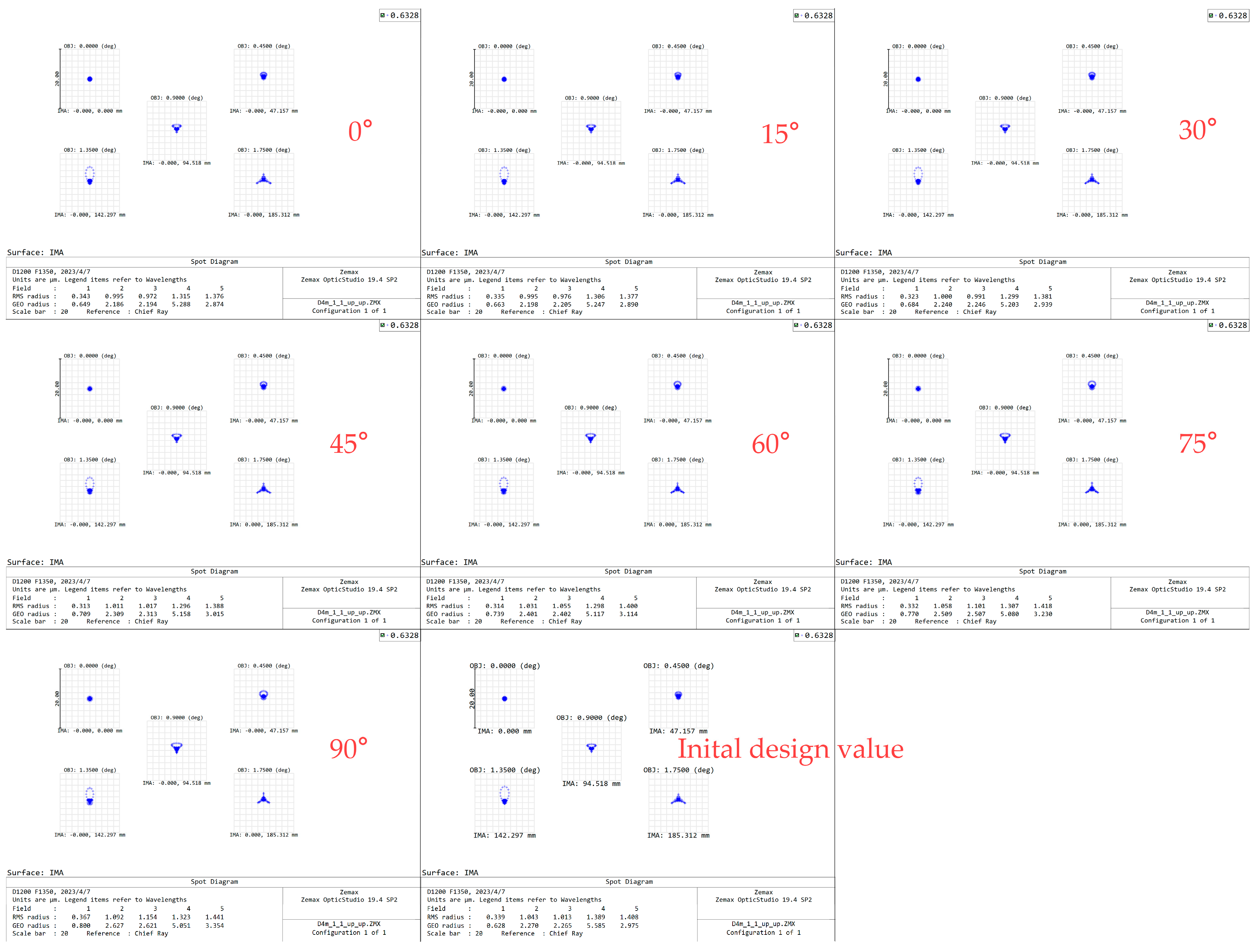
3. Results
3.1. Global Deformation of Corrector Lenses
3.2. Surface Shape Fitting Results of Optical Surfaces L1S1
3.2.1. Curvature Radius Error
3.2.2. Rigid Body Displacement Error
3.2.3. Surface Shape Error
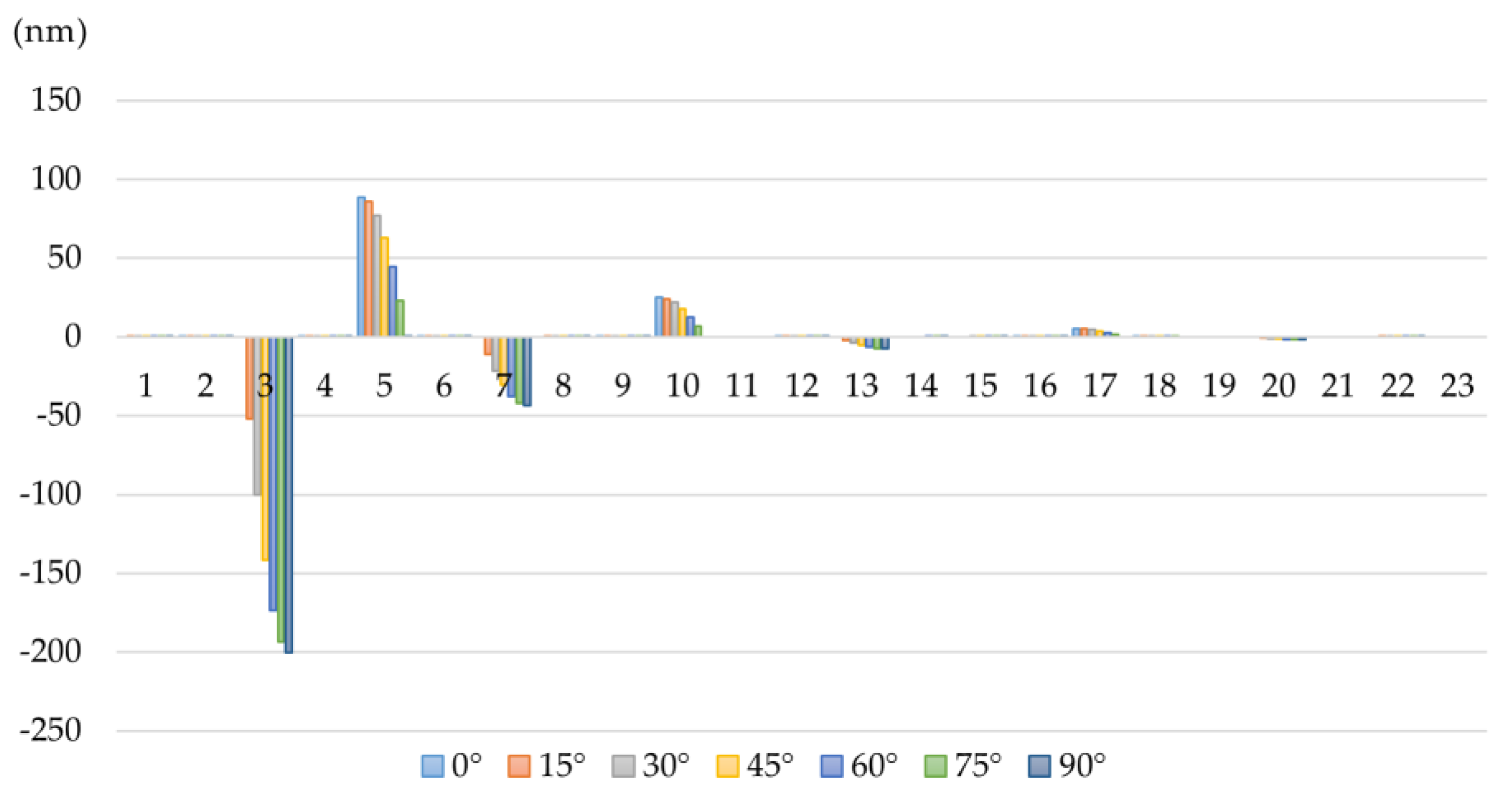
3.2.4. Zernike Polynomial Fitting
3.3. Analysis Results of the Corrector Lenses
3.3.1. Curvature Radius Variation
3.3.2. Rigid Body Displacement Variation
3.3.3. Surface Shape Variation
3.3.4. Zernike Polynomial Fitting
3.4. Feasibility Analysis Results of the Optical Design Scheme
4. Discussion
- The optical design scheme for a meter-level correction lens is feasible. When the correction lens varies with the pitch angle of the telescope, the shape of the optical surface changes, and the wavefront aberration caused by the corrector lens mainly shows defocus, coma, and spherical aberrations. The two main types of aberration alternate as the altitude angle of the telescope changes, with little effect on the imaging quality and energy concentration. The feasibility of the prime-focus optical design scheme for a ground-based, large-aperture, wide-field-of-view telescope is thus demonstrated.
- An auxiliary wavefront correction method is needed. Ideally, the wavefront aberration caused by the corrector lenses is small, but in practical engineering applications where discrete multi-point support structures are required, the shape deformation of the optical surface is inevitable, especially when multiple optical surfaces have the same variation trends. In subsequent engineering applications, a high-precision, heavy-load, six-degree-of-freedom platform should be used to correct the aberration in real time.
- Optical, mechanical, and thermal integration analyses should be carried out. In this paper, an integrated optomechanical analysis of the corrector lens was realized and a feasibility analysis of the meter-level corrector lens structure was verified. For a more detailed analysis, mechanically and thermally induced wavefront aberration variations will be fully taken into account in a subsequent study, thus allowing a near-realistic integrated optomechanical analysis.
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Yan, P.; Yang, Y.; She, W.; Liu, K.; Jiang, K.; Duan, J.; Shan, Q. Wide-field and large aperture optical system design for ground-based photoelectric detection. In Proceedings of the 9th International Symposium on Advanced Optical Manufacturing and Testing Technologies: Large Mirrors and Telescopes, Chengdu, China, 26–29 June 2019; Volume 10837, p. 108370U. [Google Scholar] [CrossRef]
- Morgan, J.S.; Kaiser, N. Alignment of the Pan-STARRS PS1 prototype telescope optics. In Proceedings of the Ground-based and Airborne Telescopes II, Marseille, France, 23–28 June 2008; Volume 7012, p. 70121K. [Google Scholar] [CrossRef]
- Ming, M.; Yang, F.; Zhao, J.Y.; Zhang, L.M.; Wu, X.X. Catadioptric optical system with large aperture, wide field of view and broad waveband. Infrared Laser Eng. 2012, 41, 149–154. [Google Scholar] [CrossRef]
- Cheng, J.Q. Principle and Design of Astronomical Telescopes; Nanjing University Press: Nanjing, China, 2020. [Google Scholar]
- Terebizh, V.Y. A wide-field corrector at the prime focus of a Ritchey-Chrétien telescope. Astron. Lett. 2004, 30, 200–208. [Google Scholar] [CrossRef]
- Wang, F.G.; Wang, Z.; Ming, M.; Li, H.; Lu, T. Opto-mechanical Structure Design, Alignment and Test of Prime Focus Optical System. Acta Photon. Sin. 2012, 41, 21–25. [Google Scholar] [CrossRef]
- Wang, F.G.; Yang, F.; Chen, B.G.; Li, W.Y. Lightweight Structure Design, Analysis and Test of Lager Aperture and Prime Focus Optical System. Infrared Technol. 2011, 33, 4–8. [Google Scholar] [CrossRef]
- Doel, P.; Sholl, M.J.; Liang, M.; Brooks, D.; Flaugher, B.; Gutierrez, G.; Kent, S.; Lampton, M.; Miller, T.; Sprayberry, D. The DESI wide field corrector optics. In Proceedings of the Ground-based and Airborne Instrumentation for Astronomy V, Montréal, QC, Canada, 22–27 June 2014; Volume 9147, p. 91476R. [Google Scholar] [CrossRef]
- Kent, S.; Bernstein, R.; Abbott, T.; Bigelow, B.C.; Brooks, D.C.; Doel, P.; Flaugher, B.; Gladders, M.D.; Walker, A.R.H.; Worswick, S. Preliminary optical design for a 2.2 degree diameter prime focus corrector for the Blanco 4 meter telescope. In Proceedings of the Ground-based and Airborne Instrumentation for Astronomy, Orlando, FL, USA, 24–31 May 2006; Volume 6269, p. 626937. [Google Scholar] [CrossRef]
- Doel, P.; Abbott, T.; Antonik, M.; Bernstein, R.; Bigelow, B.; Brooks, D.; Cease, H.; DePoy, D.L.; Flaugher, B.; Gladders, M.; et al. Design and status of the optical corrector for the DES survey instrument. In Proceedings of the Ground-based and Airborne Instrumentation for Astronomy II, Marseille, France, 23–28 June 2008; Volume 7014, p. 70141V. [Google Scholar] [CrossRef]
- Antonik, M.; Doel, P.; Brooks, D.; Bridle, S.; Abbot, T.; Bernstein, R.; Bigelow, B.; Cease, H.; Depoy, D.L.; Flaugher, B.; et al. The design and alignment of the DECam lenses and modelling of the static shear pattern and its impact on weak lensing measurements. In Proceedings of the Optical System Alignment, Tolerancing, and Verification III, San Diego, CA, USA, 2–6 August 2009; Volume 7433, p. 74330M. [Google Scholar] [CrossRef]
- Flaugher, B.L.; Abbott, T.M.C.; Angstadt, R.; Annis, J.; Antonik, M.L.; Bailey, J.; Ballester, O.; Bernstein, J.P.; Bernstein, R.A.; Bonati, M.; et al. Status of the Dark Energy Survey Camera (DECam) project. In Proceedings of the Ground-based and Airborne Instrumentation for Astronomy IV, Amsterdam, The Netherlands, 1–6 July 2012; Volume 8446, p. 844611. [Google Scholar] [CrossRef]
- Diehl, T. The Dark Energy Survey Camera (DECam). Phys. Procedia 2012, 37, 1332–1340. [Google Scholar] [CrossRef]
- DePoy, D.L.; Abbott, T.; Annis, J.; Antonik, M.; Barceló, M.; Bernstein, R.; Bigelow, B.; Brooks, D.; Buckley-Geer, E.; Campa, J.; et al. The Dark Energy Camera (DECam). In Proceedings of the Ground-based and Airborne Instrumentation for Astronomy II, Marseille, France, 23–28 June 2008; Volume 7014, p. 70140E. [Google Scholar] [CrossRef]
- Doel, P.; Brooks, D.; Antonik, M.L.; Flaugher, B.L.; Stefanik, A.; Kent, S.M.; Gutierrez, G.; Cease, H.P.; Abbott, T.M.; Walker, A.R.; et al. Assembly, alignment, and testing of the DECam wide field corrector optics. In Proceedings of the Ground-based and Airborne Instrumentation for Astronomy IV, Amsterdam, The Netherlands, 1–6 July 2012; Volume 8446, p. 84466F. [Google Scholar] [CrossRef]
- Overview of Subaru Telescope. Available online: https://subarutelescope.org/en/about/ (accessed on 25 February 2023).
- Komiyama, Y.; Miyazaki, S.; Nakaya, H.; Furusawa, H.; Takeshi, K. Subaru next-generation wide-field camera: HyperSuprime. In Proceedings of the Ground-based Instrumentation for Astronomy, Glasgow, UK, 21–25 June 2004; Volume 5492, pp. 525–533. [Google Scholar] [CrossRef]
- Komiyama, Y.; Aihara, H.; Fujimori, H.; Furusawa, H.; Kamata, Y.; Karoji, H.; Kawanomoto, S.; Mineo, S.; Miyatake, H.; Miyazaki, S.; et al. Hyper Suprime-Cam: Camera design. In Proceedings of the Ground-based and Airborne Instrumentation for Astronomy III, San Diego, CA, USA, 27 June–2 July 2010; Volume 7735, p. 77353F. [Google Scholar] [CrossRef]
- Nakaya, H.; Miyatake, H.; Uchida, T.; Fujimori, H.; Mineo, S.; Aihara, H.; Furusawa, H.; Kamata, Y.; Karoji, H.; Kawanomoto, S.; et al. Hyper Suprime-Cam: Performance of the CCD readout electronics. In Proceedings of the High Energy, Optical, and Infrared Detectors for Astronomy V, Amsterdam, The Netherlands, 1–6 July 2012; Volume 8453, p. 84532R. [Google Scholar] [CrossRef]
- Nakaya, H.; Uchida, T.; Miyatake, H.; Fujimori, H.; Mineo, S.; Aihara, H.; Furusawa, H.; Kamata, Y.; Karoji, H.; Kawanomoto, S.; et al. Hyper Suprime-Cam: Development of the CCD readout electronics. In Proceedings of the Ground-based and Airborne Instrumentation for Astronomy III, San Diego, CA, USA, 27 June–2 July 2010; Volume 7735, p. 77352P. [Google Scholar] [CrossRef]
- Miyazaki, S.; Komiyama, Y.; Nakaya, H.; Doi, Y.; Furusawa, H.; Gillingham, P.; Kamata, Y.; Takeshi, K.; Nariai, K. Hyper Suprime: Project overview. In Proceedings of the Ground-based and Airborne Instrumentation for Astronomy, Orlando, FL, USA, 24–31 May 2006; Volume 6269, p. 62690B. [Google Scholar] [CrossRef]
- Komiyama, Y.; Miyazaki, S.; Doi, Y.; Nakaya, H.; Furusawa, H.; Takeshi, K.; Nariai, K. Hyper Suprime: Imaging optics. In Proceedings of the Ground-based and Airborne Instrumentation for Astronomy, Orlando, FL, USA, 24–31 May 2006; Volume 6269, p. 62693E. [Google Scholar] [CrossRef]
- Kamata, Y.; Miyazaki, S.; Nakaya, H.; Komiyama, Y.; Obuchi, Y.; Kawanomoto, S.; Uraguchi, F.; Utsumi, Y.; Suzuki, H.; Miyazaki, Y.; et al. Hyper Suprime-Cam: Characteristics of 116 fully depleted back-illuminated CCDs. In Proceedings of the High Energy, Optical, and Infrared Detectors for Astronomy V, Amsterdam, The Netherlands, 1–6 July 2012; Volume 8453, p. 84531X. [Google Scholar] [CrossRef]
- Hyper Suprime-Cam Ushers in a New Era of Observational Astronomy. Available online: https://subarutelescope.org/en/news/topics/2012/09/12/2531.html (accessed on 25 February 2023).
- Hu, M.; Pan, Y.; Zhang, N.; Xu, X. A Review on Zernike Coefficient-Solving Algorithms (CSAs) Used for Integrated Optomechanical Analysis (IOA). Photonics 2023, 10, 177. [Google Scholar] [CrossRef]
- Born, M.; Wolf, E. Principles of Optics; Pergamon Press: New York, NY, USA, 1964. [Google Scholar]



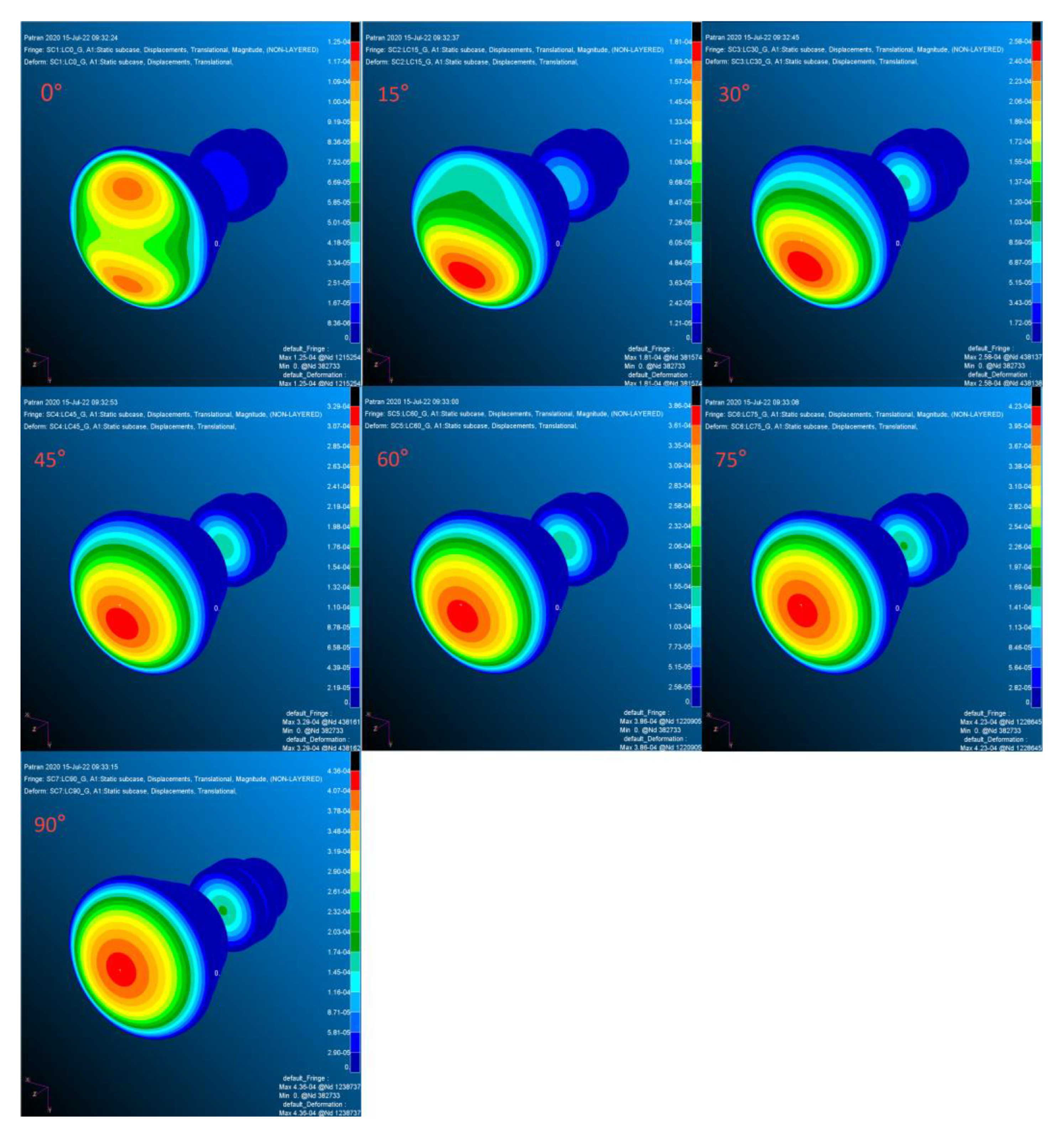

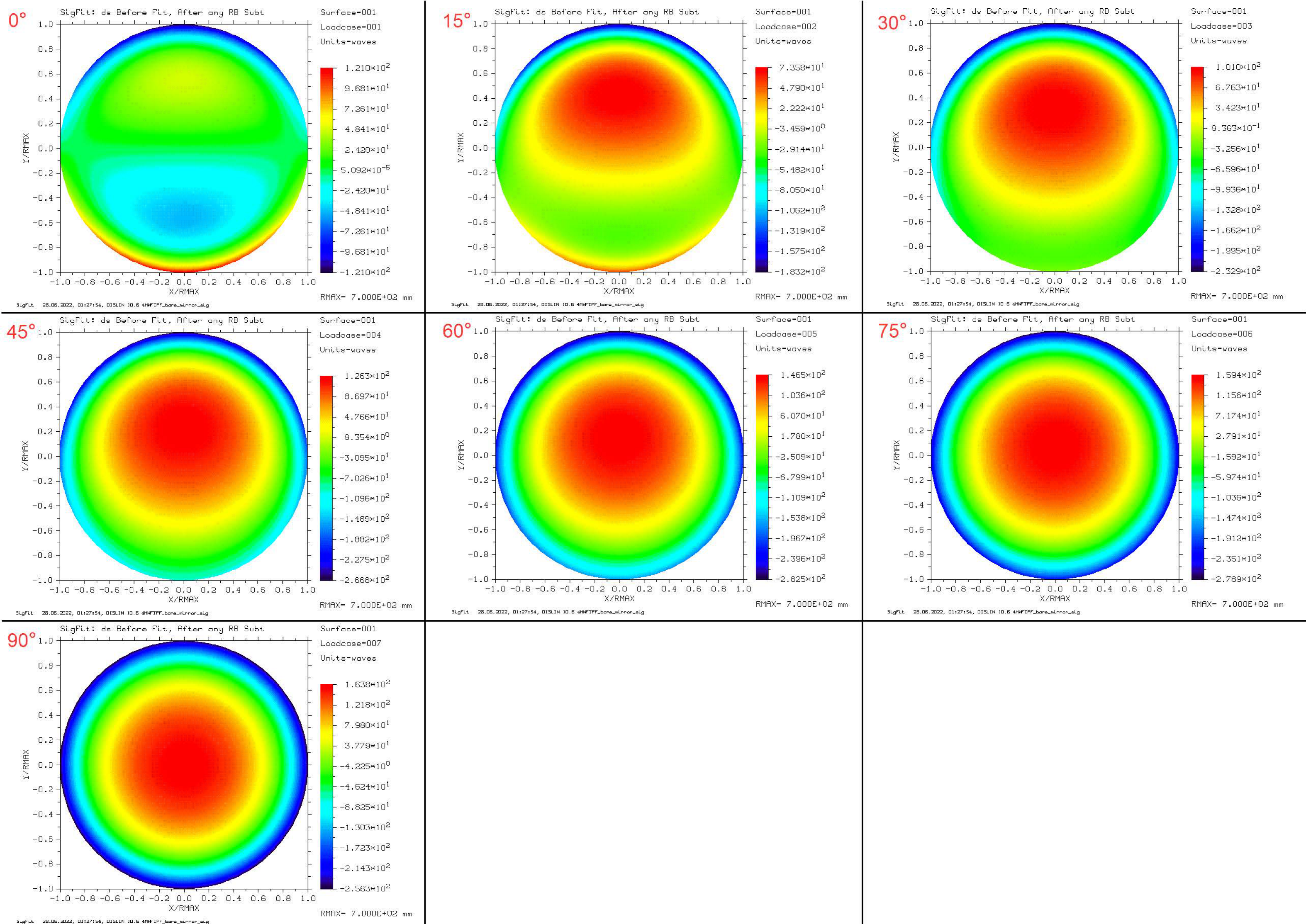
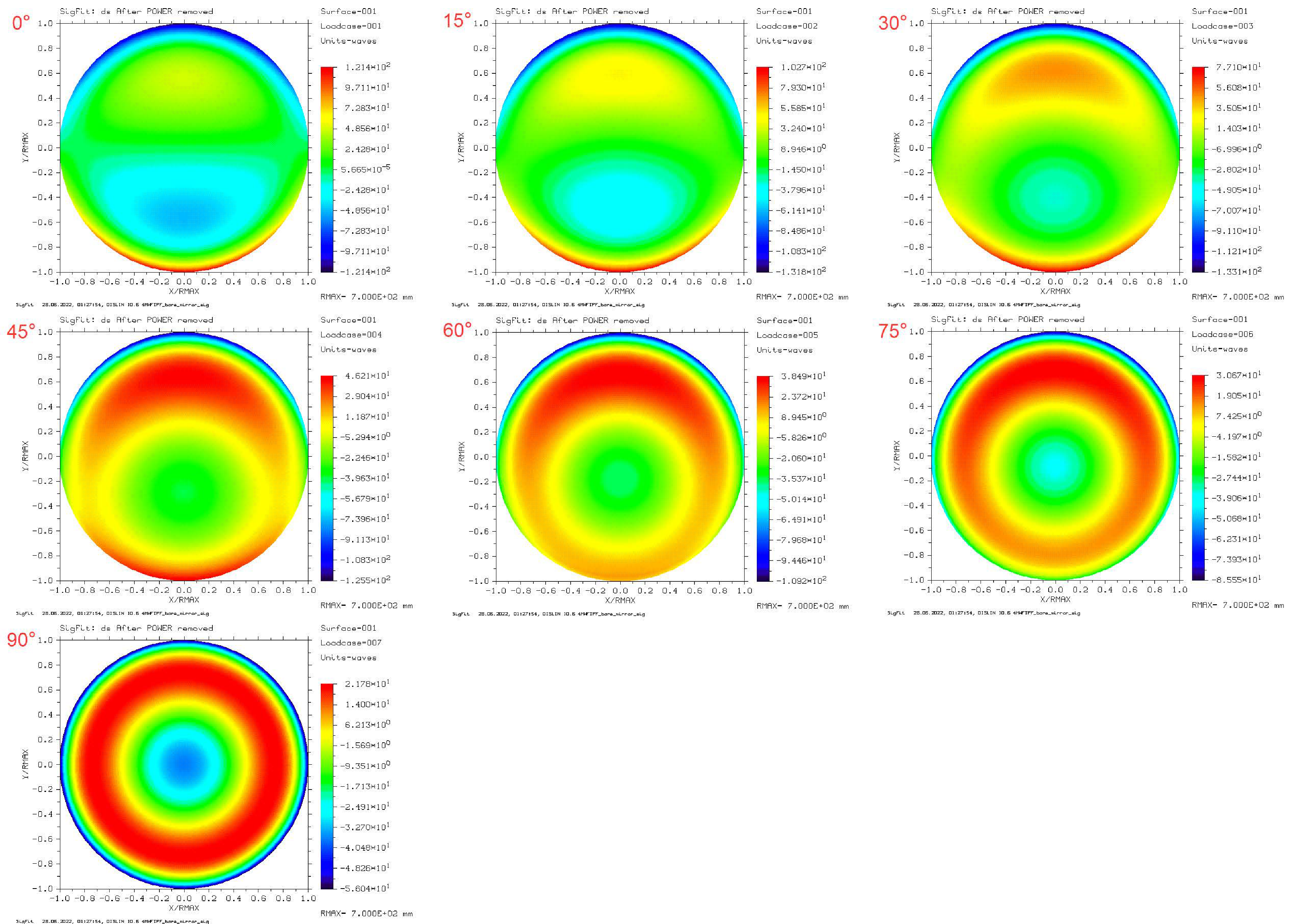



| Optical System Form | Typical Telescope | Effective Aperture or Primary Mirror Aperture |
|---|---|---|
| Prime focus | Desi Telescope [8] | 4.2 m |
| Blanco Telescope [9] | 4 m | |
| LBT Telescope | 11.8 m | |
| Subaru Telescope | 8.2 m | |
| DCT Telescope | 4.3 m | |
| VISTA Telescope | 4.1 m | |
| AEOS Telescope (recoat) | 3.6 m | |
| Mersenne–Schmidt | Space Surveillance Telescope | 3.5 m |
| Large Synoptic Survey Telescope | 8.4 m |
| Name of Corrector Lens | Lens Diameter (mm) | Central Thickness (mm) | Edge Diameter (mm) | Weight (kg) |
|---|---|---|---|---|
| C1 | 980 | 110 | 72.6 | 172.68 |
| C2 | 690 | 52 | 148.1 | 87.16 |
| C3 | 652 | 75.1 | 37.739 | 42.11 |
| C4 | 604 | 101.7 | 52.7 | 49.58 |
| C5 | 512 | 55 | 36.3 | 24.33 |
| No. | Name | Primary Mirror Aperture | Field of View | Maximum Corrector Aperture |
|---|---|---|---|---|
| 1 | The Blanco Telescope | 4 m | 2.2° | 980 mm |
| 2 | The Subaru Telescope | 8.2 m | 1.5° | 850 mm |
| 3 | The KMTNet Telescope | 1.6 m | 2° | 552 mm |
| 4 | The MMT Telescope | 6.5 m | 1° | 750 mm |
| 5 | Our System | 4 m | 3.5° | 1400 mm |
| Name of Optical Surface | Curvature Radius (mm) | Distance (mm) | Lens Diameter (mm) | Weight (kg) |
|---|---|---|---|---|
| L1S1 | −1007.630 | −260.000 | 1400 | 574 |
| L1S2 | −2811.320 | −22.587 | ||
| L2S1 | −1546.940 | −75.000 | 1220 | 312 |
| L2S2 | −878.310 | −164.118 | ||
| L3S1 | −3465.840 | −65.000 | 1040 | 259 |
| L3S2 | −727.470 | −151.030 | ||
| L4S1 | −3712.420 | −65.000 | 880 | 156.9 |
| L4S2 | −756.220 | −688.534 | ||
| L5S1 | −2857.135 | −100.000 | 730 | 78 |
| L5S2 | 9475.188 | −29.938 | ||
| L6S1 | −1062.845 | −120.000 | 730 | 83.4 |
| L6S2 | −12,580.000 | −204.865 | ||
| L7S1 | −1322.140 | −108.000 | 540 | 42 |
| L7S2 | 1795.050 | −187.774 | ||
| Focal plane diameter | Φ 370 | |||
| Name of Optical Surface | L1S1(mm) | L1S2(mm) | |
|---|---|---|---|
| Original curvature radius | −1007.63 | −2811.32 | |
| Curvature radius error | 0° | 4.28435 × 10−10 | 2.74851 × 10−9 |
| 15° | 2.74884 × 10−4 | 3.60830 × 10−3 | |
| 30° | 5.31034 × 10−4 | 6.97068 × 10−3 | |
| 45° | 7.50996 × 10−4 | 9.85804 × 10−3 | |
| 60° | 9.19778 × 10−4 | 1.20736 × 10−2 | |
| 75° | 1.02588 × 10−3 | 1.34663 × 10−2 | |
| 90° | 1.06207 × 10−3 | 1.39414 × 10−2 |
| Displacement Decomposition Terms | Tx (mm) | Ty (mm) | Tz (mm) | Rx (rad) | Ry (rad) | Rz (rad) | |
|---|---|---|---|---|---|---|---|
| Rigid body displacement error | 0° | −1.358 × 10−11 | 5.608 × 10−5 | −4.035 × 10−11 | 6.869 × 10−8 | 2.513 × 10−13 | 2.485 × 10−14 |
| 15° | −3.101 × 10−11 | 5.417 × 10−5 | 6.633 × 10−5 | 6.635 × 10−8 | 6.433 × 10−14 | 5.155 × 10−16 | |
| 30° | −4.621 × 10−11 | 4.857 × 10−5 | 1.281 × 10−4 | 5.949 × 10−8 | −1.264 × 10−13 | −2.376 × 10−14 | |
| 45° | −5.873 × 10−11 | 3.965 × 10−5 | 1.812 × 10−4 | 4.857 × 10−8 | −3.115 × 10−13 | −4.615 × 10−14 | |
| 60° | −6.673 × 10−11 | 2.804 × 10−5 | 2.220 × 10−4 | 3.435 × 10−8 | −4.713 × 10−13 | −6.562 × 10−14 | |
| 75° | −7.029 × 10−11 | 1.451 × 10−5 | 2.476 × 10−4 | 1.778 × 10−8 | −6.008 × 10−13 | −8.066 × 10−14 | |
| 90° | −6.923 × 10−11 | 3.785 × 10−11 | 2.563 × 10−4 | −3.180 × 10−13 | −6.890 × 10−13 | −8.995 × 10−14 | |
| L1S1 | 0° | 15° | 30° | 45° | 60° | 75° | 90° | |||
|---|---|---|---|---|---|---|---|---|---|---|
| K | N | M | Aberration | Amplitude | Amplitude | Amplitude | Amplitude | Amplitude | Amplitude | Amplitude |
| 1 | 0 | 0 | Piston | 0.00001 | 0.05992 | 0.11576 | 0.1637 | 0.20049 | 0.22361 | 0.2315 |
| 2 | 1 | 1 | Tilt | 0.37529 | 0.36251 | 0.32502 | 0.26539 | 0.18766 | 0.09715 | 0.00016 |
| 3 | 2 | 0 | Defocus | −0.00001 | −51.88784 | −100.2394 | −141.7601 | −173.6199 | −193.6479 | −200.4790 |
| 4 | 2 | 2 | Pri Astigmatism | 0.00023 | 0.00033 | 0.00079 | 0.0012 | 0.00154 | 0.00178 | 0.00189 |
| 5 | 3 | 1 | PriComa | 88.71321 | 85.69039 | 76.82786 | 62.72966 | 44.35648 | 22.9606 | 0.00016 |
| 6 | 3 | 3 | Pri Trefoil | 0.00027 | 0.00031 | 0.00034 | 0.00035 | 0.00035 | 0.00032 | 0.00027 |
| 7 | 4 | 0 | PriSpherical | 0.00005 | −11.24575 | −21.72513 | −30.72404 | −37.62913 | −41.96986 | −43.4504 |
| 8 | 4 | 2 | Sec Astigmatism | 0.00006 | 0.00032 | 0.00064 | 0.00091 | 0.00112 | 0.00126 | 0.00131 |
| 9 | 4 | 4 | Pri Tetrafoil | 0.00015 | 0.00036 | 0.00055 | 0.00069 | 0.0008 | 0.00084 | 0.00083 |
| 10 | 5 | 1 | SecComa | 25.09534 | 24.24024 | 21.73319 | 17.74506 | 12.54763 | 6.49512 | 0.00011 |
| 11 | 5 | 3 | Sec Trefoil | 0.00002 | 0.00003 | 0.00003 | 0.00003 | 0.00003 | 0.00003 | 0.00003 |
| 12 | 5 | 5 | Pri Pentafoil | 0.00039 | 0.00041 | 0.00041 | 0.00038 | 0.00032 | 0.00024 | 0.00015 |
| 13 | 6 | 0 | SecSpherical | 0.00003 | −1.9746 | −3.81466 | −5.39477 | −6.60722 | −7.36941 | −7.62939 |
| 14 | 6 | 2 | Ter Astigmatism | 0.00004 | 0.00006 | 0.00009 | 0.00011 | 0.00012 | 0.00013 | 0.00013 |
| 15 | 6 | 4 | Sec Tetrafoil | 0.00003 | 0.00007 | 0.00012 | 0.00017 | 0.00021 | 0.00023 | 0.00024 |
| 16 | 6 | 6 | Pri Hexafoil | 0.00013 | 0.00014 | 0.00014 | 0.00014 | 0.00014 | 0.00013 | 0.00012 |
| 17 | 7 | 1 | TerComa | 5.08125 | 4.90811 | 4.4005 | 3.59299 | 2.54063 | 1.31513 | 0.00002 |
| 18 | 7 | 3 | Ter Trefoil | 0.00031 | 0.00031 | 0.00029 | 0.00025 | 0.00019 | 0.00012 | 0.00004 |
| 19 | 7 | 5 | Sec Pentafoil | 0.00001 | 0.00002 | 0.00002 | 0.00001 | 0.00001 | 0.00001 | 0.00001 |
| 20 | 8 | 0 | TerSpherical | 0 | −0.47184 | −0.91152 | −1.28908 | −1.5788 | −1.76092 | −1.82303 |
| 21 | 8 | 2 | Qua Astigmatism | 0.00001 | 0.00002 | 0.00005 | 0.00007 | 0.00009 | 0.0001 | 0.00011 |
| 22 | 8 | 4 | Ter Tetrafoil | 0.00002 | 0.00038 | 0.00072 | 0.001 | 0.00123 | 0.00136 | 0.00141 |
| 23 | 8 | 6 | Sec Hexafoil | 0.00002 | 0.00002 | 0.00001 | 0.00001 | 0.00002 | 0.00002 | 0.00003 |
| Unfitted term | 1.6877% | 1.3746% | 1.1129% | 1.6877% | 1.3746% | 1.1129% | 1.0050% | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cheng, X.; Liu, C. Optical and Mechanical Performance and Feasibility Analysis of Meter-Level Corrector Lenses for Survey Telescope. Photonics 2023, 10, 422. https://doi.org/10.3390/photonics10040422
Cheng X, Liu C. Optical and Mechanical Performance and Feasibility Analysis of Meter-Level Corrector Lenses for Survey Telescope. Photonics. 2023; 10(4):422. https://doi.org/10.3390/photonics10040422
Chicago/Turabian StyleCheng, Xue, and Changhua Liu. 2023. "Optical and Mechanical Performance and Feasibility Analysis of Meter-Level Corrector Lenses for Survey Telescope" Photonics 10, no. 4: 422. https://doi.org/10.3390/photonics10040422
APA StyleCheng, X., & Liu, C. (2023). Optical and Mechanical Performance and Feasibility Analysis of Meter-Level Corrector Lenses for Survey Telescope. Photonics, 10(4), 422. https://doi.org/10.3390/photonics10040422





