Depolarization Measurement through a Single-Mode Fiber-Based Endoscope for Full Mueller Endoscopic Polarimetric Imaging
Abstract
1. Introduction
2. Method and Numerical Simulations
- -
- The Mueller matrix of each pixel of an image of the sample (“elementary matrix”) is first measured and registered following the procedure previously reported in [25]. As already stated, since the optical fiber is single-mode, each elementary matrix is a non-depolarizing matrix;
- -
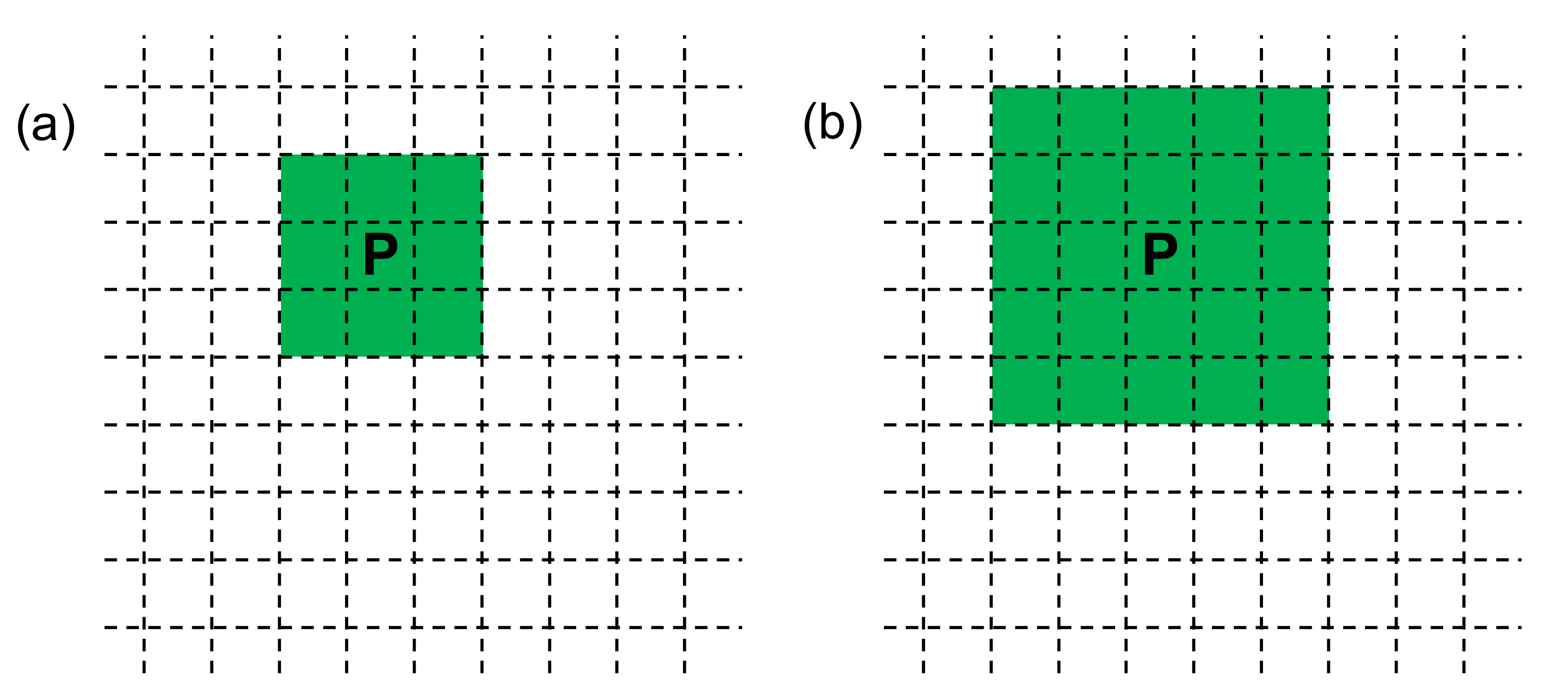
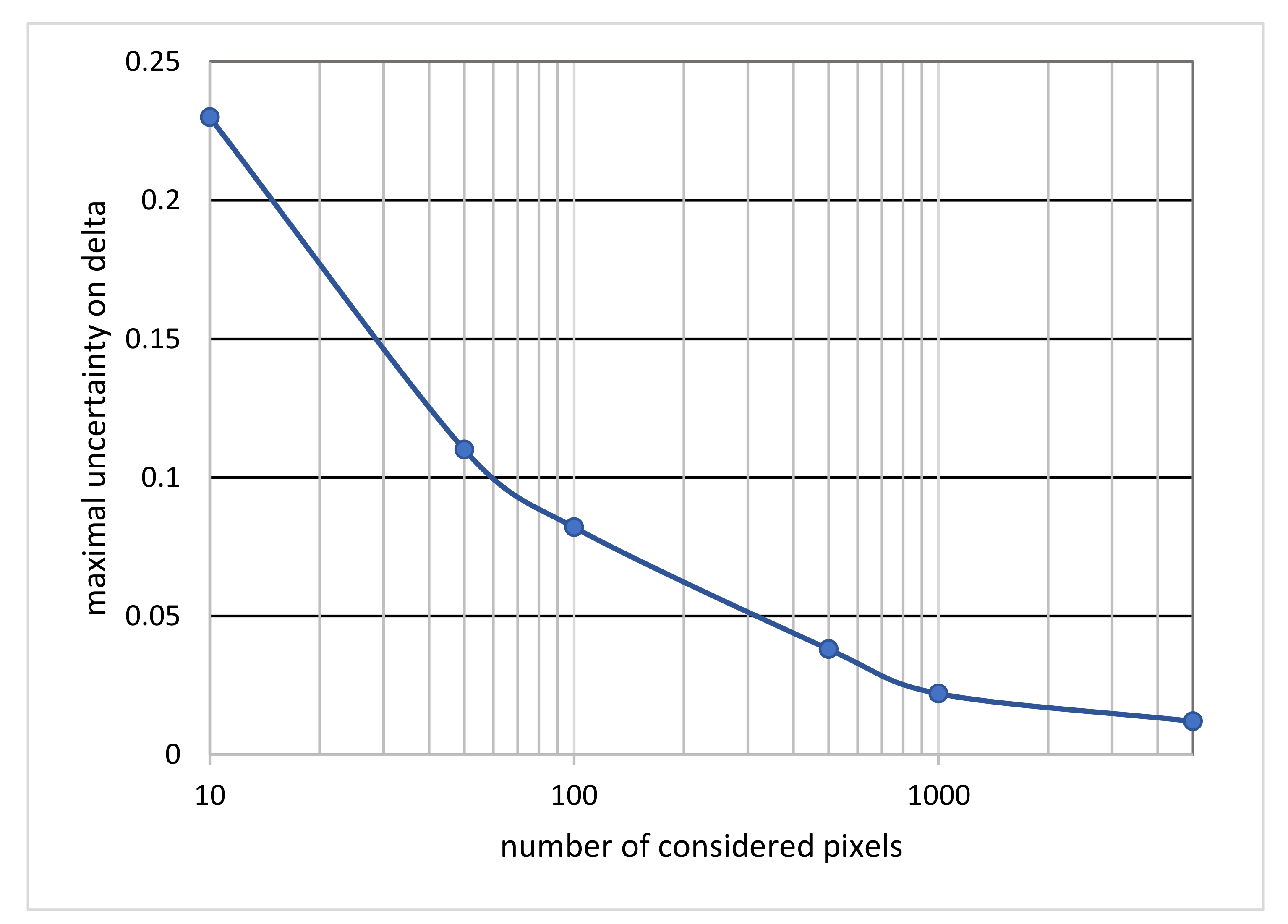
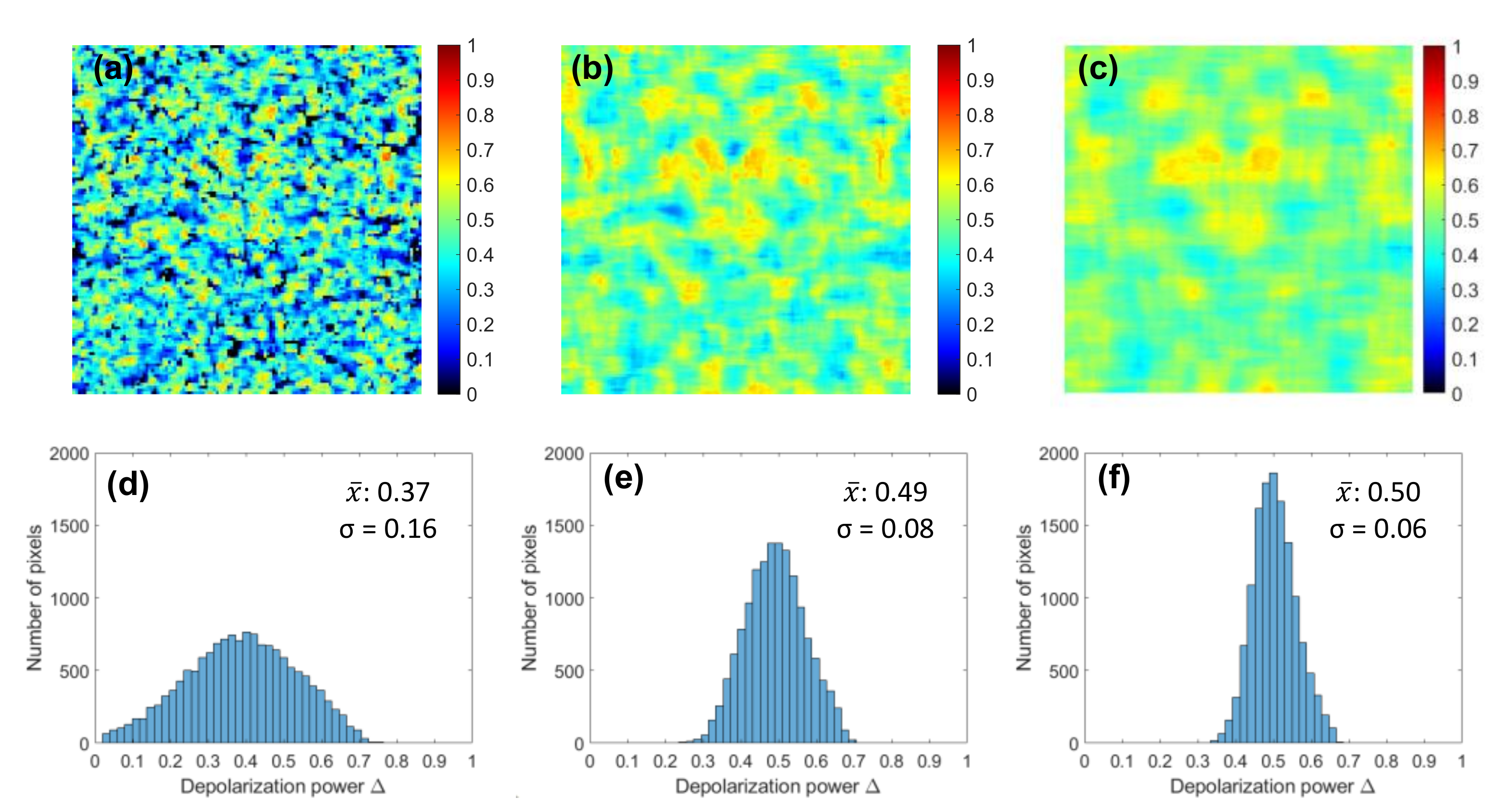
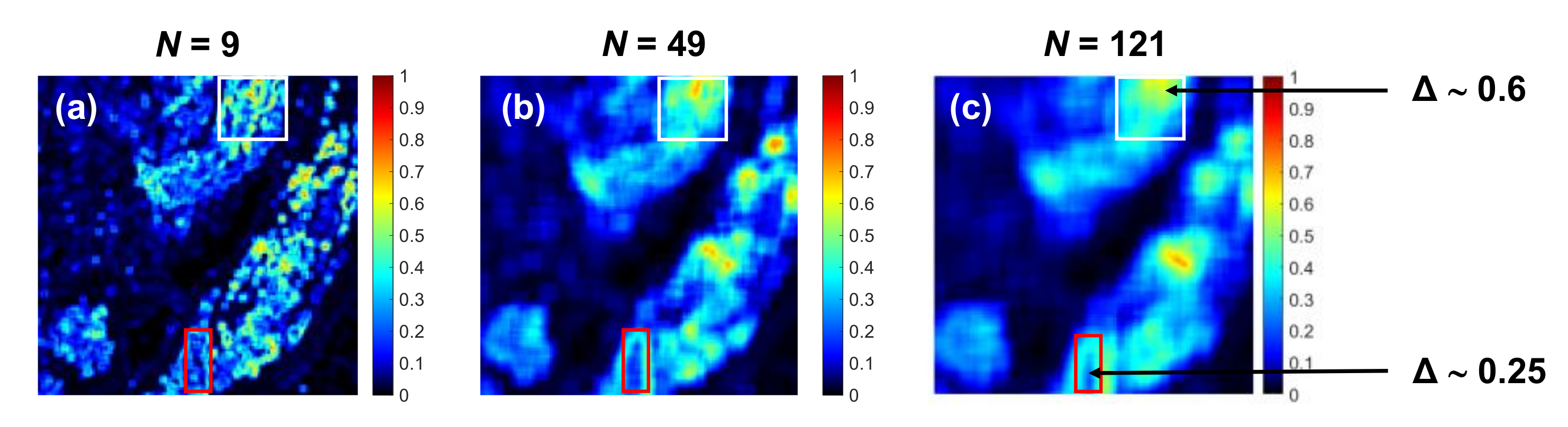
- Then, for each pixel P, the average Mueller matrix over adjacent pixels is calculated, these pixels being those within a floating square window around P, as depicted in Figure 2. The size of this floating window, chosen beforehand, is N = (2n + 1)2 pixels, n being the number of considered rings of pixels around P;
- -
- Each average Mueller matrix is decomposed by means of the Lu–Chipman method, in order to extract the associated depolarization matrix MΔ whose form is given in Equation (5);
- -
- The depolarization power Δ of each pixel is finally calculated by means of Equation (8), and a pixelated image of Δ(x,y) is plotted.
- -
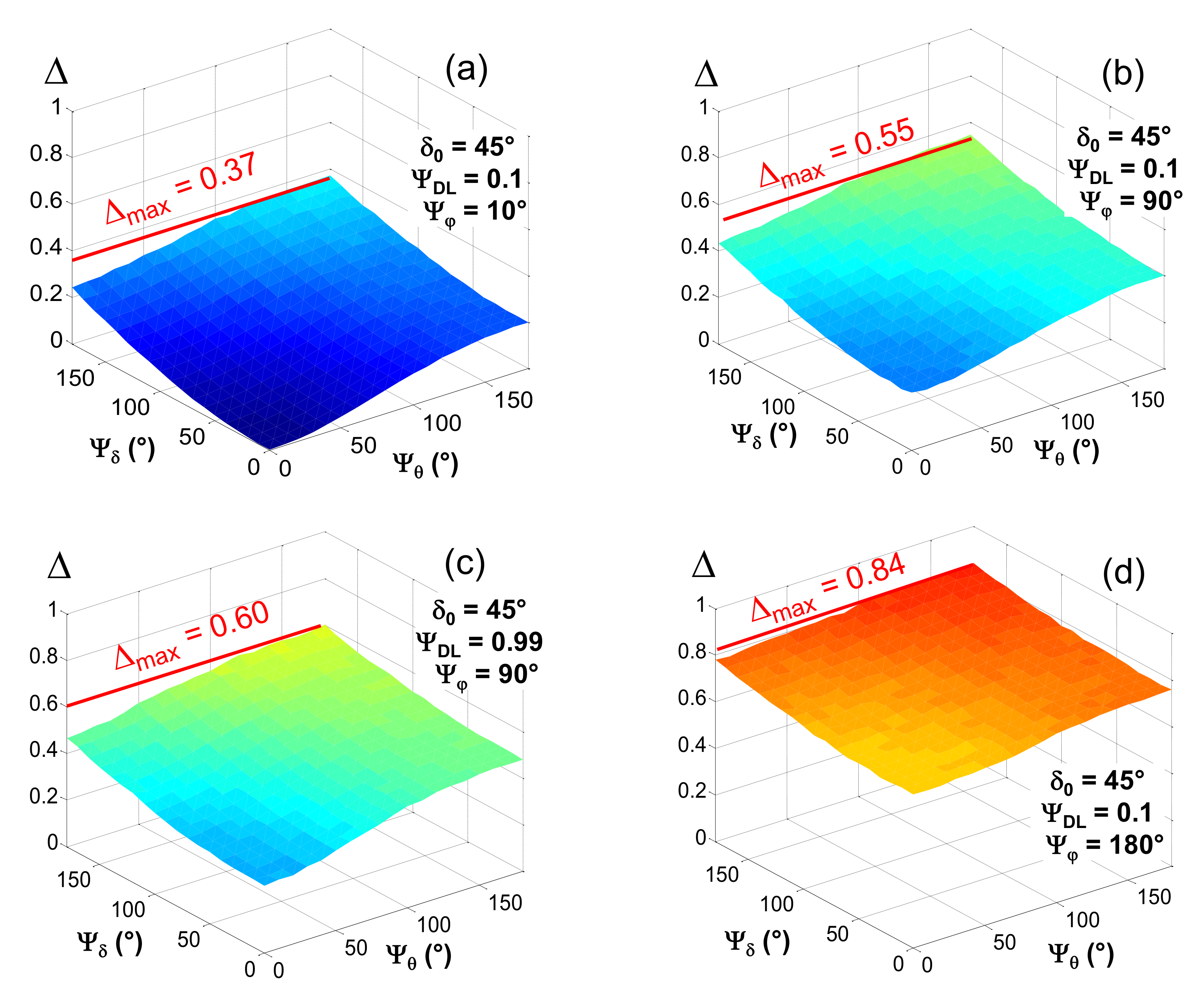
- the depolarization power Δ is zero when all the elementary matrices remain the same (Ψδ = 0, Ψθ = 0) whatever the retardance δ0;
- -
- Δ increases with both Ψδ and Ψθ. In other words, Δ is higher as the diversity of the elementary matrices is large;
- -
- if the orientation of the eigenaxes remains the same for all the retarders (i.e., Ψθ = 0), the increase in Δ as a function of Ψδ remains the same whatever the central retardance δ0;
- -
- for given ranges of variations Ψδ and Ψθ, the depolarization power Δ increases with δ0.
3. Material and Experimental Results
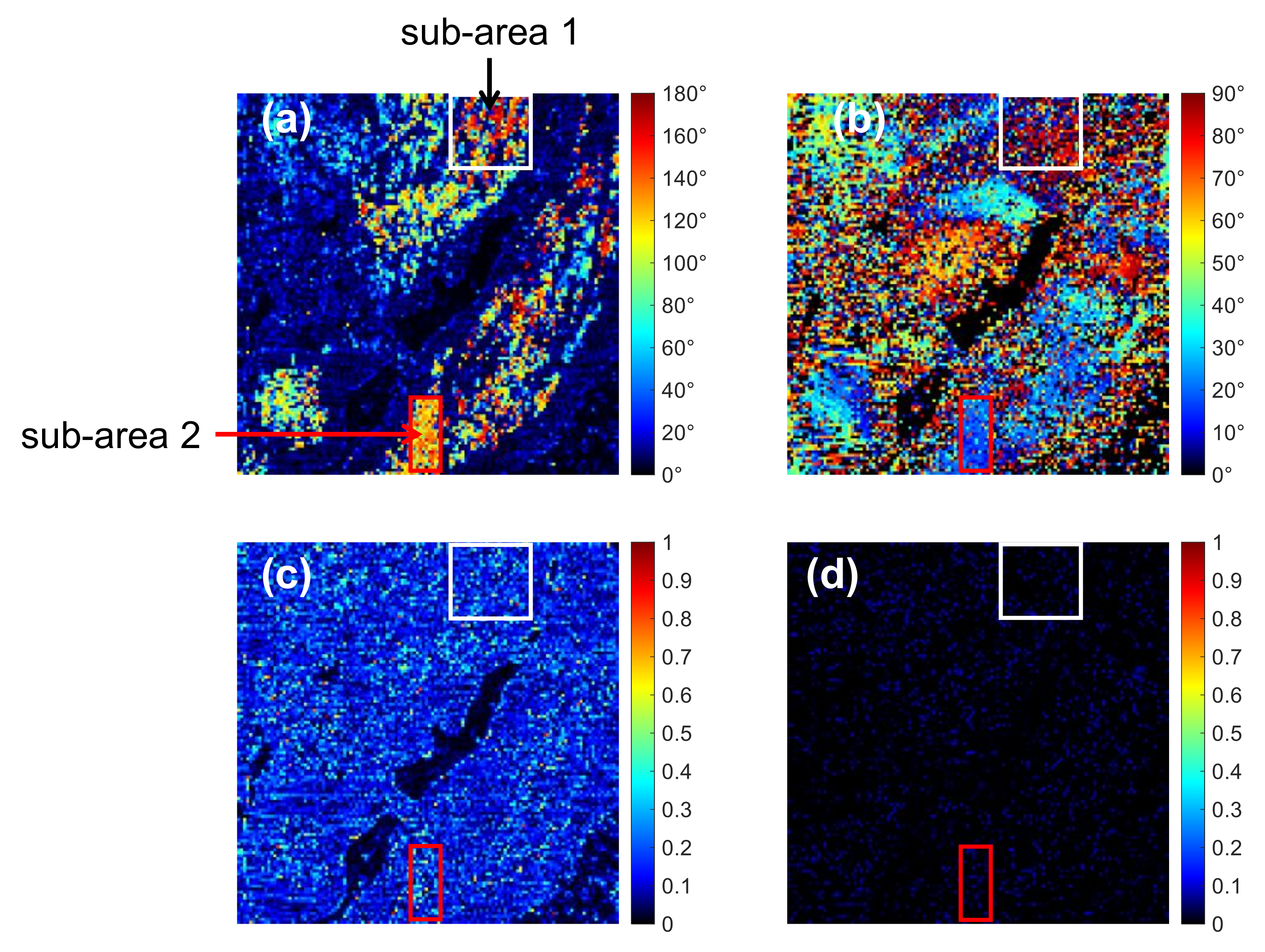
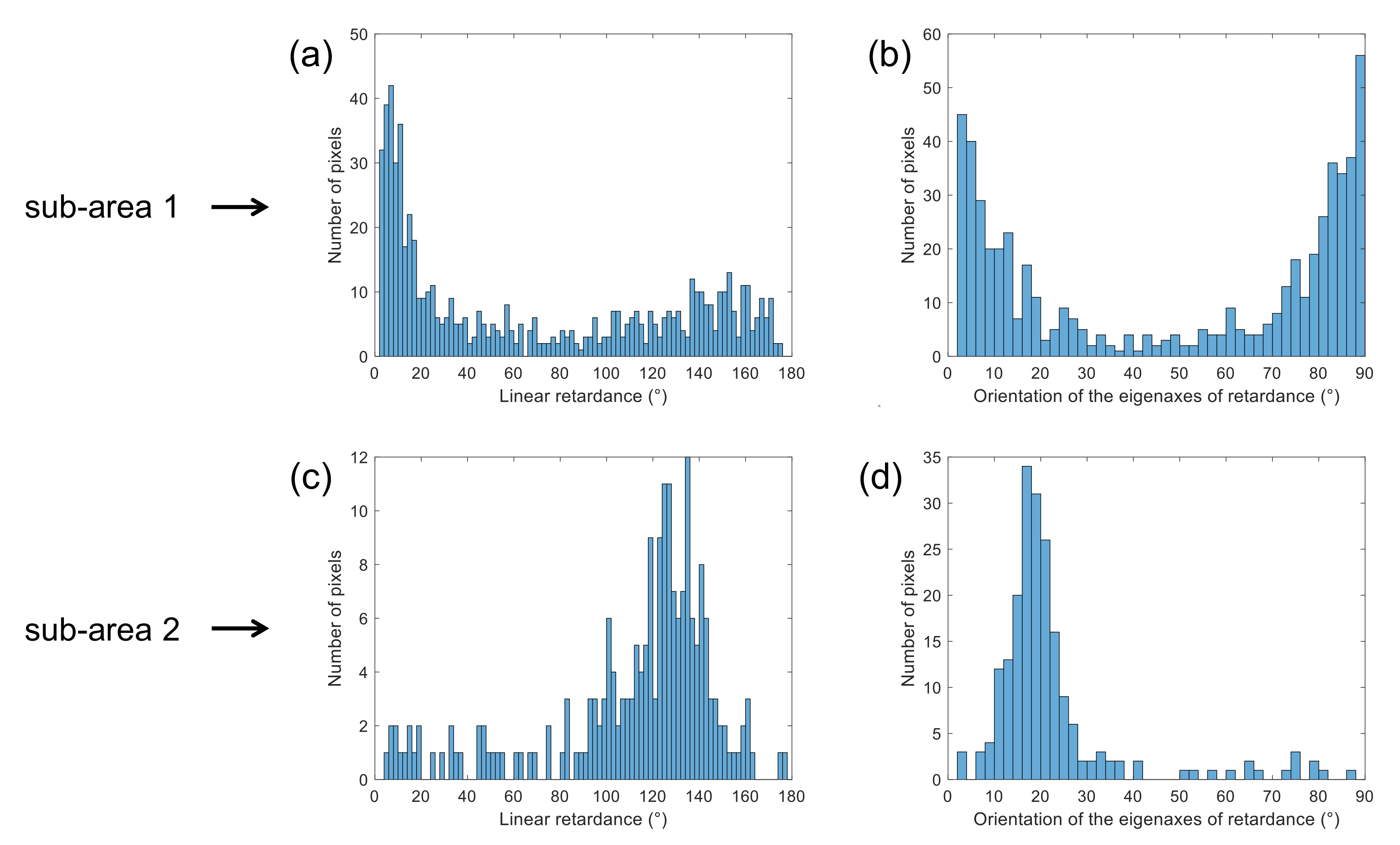
3.1. Depolarization Measurement on a Manufactured Sample
3.2. Depolarization Measurement on a Biological Sample
4. Conclusions
- -
- calculating a new matrix as the normalized sum of the Mueller matrices of all pixels included in a floating window centered on P;
- -
- performing the polar decomposition of by the Lu–Chipman method;
- -
- calculating Δ from the depolarization matrix resulting from this decomposition.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Jacques, S.; Ramella-Roman, J.; Lee, K. Imaging skin pathology with polarized light. J. Biomed. Opt. 2002, 7, 329–340. [Google Scholar] [CrossRef] [PubMed]
- Ghosh, N.; Vitkin, I.A. Tissue polarimetry: Concepts, challenges, applications, and outlook. J. Biomed. Opt. 2011, 16, 110801. [Google Scholar] [CrossRef] [PubMed]
- Vitkin, A.; Ghosh, N.; De Martino, A. Tissue Polarimetry. In Photonics: Biomedical Photonics, Spectroscopy and Microscopy; John Wiley and Sons: Hoboken, NJ, USA, 2015. [Google Scholar]
- Tuchin, V.V. Polarized light interaction with tissues. J. Biomed Opt. 2016, 21, 071114. [Google Scholar] [CrossRef] [PubMed]
- Yasui, T.; Tohno, Y.; Araki, T. Determination of collagen fiber orientation in human tissue by use of polarization measurement of molecular second-harmonic-generation light. Appl. Opt. 2004, 43, 2861–2867. [Google Scholar] [CrossRef]
- Bancelin, S.; Nazac, A.; Ibrahim, B.H.; Dokládal, P.; Decencière, E.; Teig, B.; Haddad, H.; Fernandez, H.; Schanne-Klein, M.-C.; De Martino, A. Determination of collagen fiber orientation in histological slides using Mueller microscopy and validation by second harmonic generation imaging. Opt. Express 2014, 22, 22561–22574. [Google Scholar] [CrossRef]
- Chipman, R.A. Polarimetry. In Handbook of Optics Volume II; McGraw-Hill: New York, NY, USA, 1995. [Google Scholar]
- Wood, M.F.G.; Ghosh, N.; Wallenburg, M.A.; Li, S.-H.; Weizel, R.D.; Wilson, B.C.; Li, R.-K.; Vitkin, I.A. Polarization birefringence measurements for characterizing the myocardium, including healthy, infarcted, and stem-cell-regenerated tissues. J. Biomed. Opt. 2010, 15, 047009. [Google Scholar]
- Pierangelo, A.; Benali, A.; Antonelli, M.R.; Novikova, T.; Validire, P.; Gayet, B.; De Martino, A. Ex-vivo characterization of human colon cancer by Mueller polarimetric imaging. Opt. Express 2011, 19, 1582–1593. [Google Scholar] [CrossRef]
- Dubreuil, M.; Babilotte, P.; Martin, L.; Sevrain, D.; Rivet, S.; Le Grand, Y.; Le Brun, G.; Turlin, B.; Le Jeune, B. Mueller matrix polarimetry for improved liver fibrosis diagnosis. Opt. Lett. 2012, 37, 1061–1063. [Google Scholar] [CrossRef]
- Jagtap, J.; Chandel, S.; Das, N.; Soni, J.; Chatterjee, S.; Pradhan, A.; Ghosh, N. Quantitative Mueller matrix fluorescence spectroscopy for precancer detection. Opt. Lett. 2014, 39, 243–246. [Google Scholar] [CrossRef]
- Qi, J.; Alson, D.S. Mueller polarimetric imaging for surgical and diagnostic applications: A review. J. Biophotonics 2017, 10, 950–982. [Google Scholar] [CrossRef]
- Kupinski, M.; Boffety, M.; Goudail, F.; Ossikovski, R.; Pierangelo, A.; Rehbinder, J.; Vizet, J.; Novikova, T. Polarimetric measurement utility for pre-cancer detection from uterine cervix specimens. Biomed. Opt. Express 2018, 9, 5691–5702. [Google Scholar] [CrossRef] [PubMed]
- Goldstein, D.H. Mueller Matrix Polarimetry. In Polarized Light, 3rd ed.; CRC Press: Boca Raton, FL, USA, 2011. [Google Scholar]
- Morio, J.; Goudail, F. Influence of the order of diattenuator, retarder, and polarizer in polar decomposition of Mueller matrices. Opt. Lett. 2004, 29, 2234–2236. [Google Scholar] [CrossRef] [PubMed]
- Ossikovski, R. Analysis of depolarizing Mueller matrices through a symmetric decomposition. J. Opt. Soc. Am. A 2009, 26, 1109–1118. [Google Scholar] [CrossRef] [PubMed]
- Vizet, J.; Ossikovski, R. Symmetric decomposition of experimental depolarizing Mueller matrices in the degenerate case. Appl. Opt. 2018, 57, 1159–1167. [Google Scholar] [CrossRef] [PubMed]
- Gil, J.J.; San José, I. Arbitrary decomposition of a Mueller matrix. Opt. Lett. 2019, 44, 5715–5718. [Google Scholar] [CrossRef]
- Lu, S.Y.; Chipman, R.A. Interpretation of Mueller matrices based on polar decomposition. JOSA A 1996, 13, 1106–1113. [Google Scholar] [CrossRef]
- Desroches, J.; Pagnoux, D.; Louradour, F.; Barthélémy, A. Fiber-optic device for endoscopic polarization imaging. Opt. Lett. 2009, 34, 3409–3411. [Google Scholar] [CrossRef]
- Fade, J.; Alouini, M. Depolarization remote sensing by orthogonality breaking. Phys. Rev. Lett. 2012, 109, 043901. [Google Scholar] [CrossRef]
- Vizet, J.; Brevier, J.; Desroches, J.; Barthélémy, A.; Louradour, F.; Pagnoux, D. One shot endoscopic polarization measurement device based on a spectrally encoded polarization states generator. Opt. Express 2015, 23, 16439–16448. [Google Scholar] [CrossRef]
- Forward, S.; Gribble, A.; Alali, S.; Lindenmaier, A.A.; Vitkin, I.A. Flexible polarimetric probe for 3 × 3 Mueller matrix measurements of biological tissue. Sci. Rep. 2017, 7, 11958. [Google Scholar] [CrossRef]
- Fu, Y.; Huang, Z.; He, H.; Ma, H.; Wu, J. Flexible 3 × 3 Mueller matrix endoscope prototype for cancer detection. IEEE Trans. Instrum. Meas. 2018, 67, 1700–1712. [Google Scholar] [CrossRef]
- Vizet, J.; Manhas, S.; Tran, J.; Validire, P.; Benali, A.; Garcia-Caurel, E.; Pierangelo, A.; De Martino, A.; Pagnoux, D. Optical fiber-based full Mueller polarimeter for endoscopic imaging using a two-wavelength simultaneous measurement method. J. Biomed. Opt. 2016, 21, 071106. [Google Scholar] [CrossRef] [PubMed]
- Buckley, C.; Fabert, M.; Kinet, D.; Kucikas, V.; Pagnoux, D. Design of an endomicroscope including a resonant fiber-based microprobe dedicated to endoscopic polarimetric imaging for medical diagnosis. Biomed. Opt. Express 2020, 11, 7032–7052. [Google Scholar] [CrossRef]
- Van Eeckhout, A.; Garcia-Caurel, E.; Ossikovski, R.; Lizana, A.; Rodriguez, C.; González-Arnay, E.; Campos, J. Depolarization metric spaces for biological tissues classification. J. Biophotonics 2020, 13, e202000083. [Google Scholar] [CrossRef] [PubMed]
- Rehbinder, J.; Vizet, J.; Park, J.; Ossikovsk, R.; Vanel, J.C.; Nazac, A.; Pierangelo, A. Depolarization imaging for fast and non-invasive monitoring of cervical microstructure remodeling in vivo during pregnancy. Sci. Rep. 2022, 12, 1232. [Google Scholar] [CrossRef] [PubMed]
- Du, E.; He, H.; Zeng, N.; Sun, M.; Guo, Y.; Wu, S.; Liu, S.; Ma, H. Mueller matrix polarimetry for differentiating characteristic features of cancerous tissues. J. Biomed. Opt. 2014, 19, 076013. [Google Scholar] [CrossRef] [PubMed]
- Zhou, X.; Maloufi, S.; Louie, D.C.; Zhang, N.; Liu, Q.; Lee, T.K.; Tang, S. Investigating the depolarization property of skin tissue by degree of polarization uniformity contrast using polarization-sensitive optical coherence tomography. Biomed. Opt. Express 2021, 12, 5073–5088. [Google Scholar] [CrossRef]
- Ushenko, V.A.; Hogan, B.T.; Dubolazov, A.; Piavchenko, G.; Kuznetsov, S.; Ushenko, A.G.; Ushenko, Y.O.; Gorsky, M.; Bykov, A.; Meglinski, I. 3D Mueller matrix mapping of layered distributions of depolarisation degree for analysis of prostate adenoma and carcinoma diffuse tissues. Sci. Rep. 2021, 11, 5162. [Google Scholar] [CrossRef]
- Novikova, T.; Pierangelo, A.; de Martino, A.; Benali, A.; Validire, P. Polarimetric imaging for cancer diagnosis and staging. Opt. Photonics News 2012, 13, 26–33. [Google Scholar] [CrossRef]
- Ivanov, D.; Dremin, V.; Borisova, E.; Bykov, A.; Novikova, T.; Meglinski, I.; Ossikovski, R. Polarization and depolarization metrics as optical markers in support to histopathology of ex vivo colon tissue. Biomed Opt. Express 2021, 12, 4560–4572. [Google Scholar] [CrossRef]
- Gil, J.J.; Bernabeu, E. A Depolarization Criterion in Mueller Matrices. Opt. Acta Int. J. Opt. 1985, 32, 259–261. [Google Scholar]
- Goldstein, D.H. Stokes Polarization Parameters. In Polarized Light, 3rd ed.; CRC Press: Boca Raton, FL, USA, 2011. [Google Scholar]
- Chipman, R.A. Depolarization. In Polarization: Measurement, Analysis, and Remote Sensing II; Proceedings of SPIE 3754; SPIE: Bellingham, WA, USA, 1999; pp. 14–20. [Google Scholar]
- Cloud, S.R. Group theory and polarization algebra. Optik 1986, 75, 26–36. [Google Scholar]
- Ortega-Quijano, N.; Fanjul-Vélez, F.; Arce-Diego, J.L. Physically meaningful depolarization metric based on the differential Mueller matrix. Opt. Lett. 2015, 40, 3280–3283. [Google Scholar] [CrossRef] [PubMed]
- Van de Hulst, H.C. Light Scattering by Small Particles; John Wiley and Sons: New York, NY, USA, 1957. [Google Scholar]
- Götzinger, E.; Pircher, M.; Geitzenauer, W.; Ahlers, C.; Baumann, B.; Michels, S.; Schmidt-Erfurth, U.; Hitzenberger, C.K. Retinal pigment epithelium segmentation by polarization sensitive optical coherence tomography. Opt. Express 2008, 16, 16410–16422. [Google Scholar] [CrossRef]
- Götzinger, E.; Pircher, M.; Baumann, B.; Ahlers, C.; Geitzenauer, W.; Schmidt-Erfurth, U.; Hitzenberger, C.K. Three-dimensional polarization sensitive OCT imaging and interactive display of the human retina. Opt. Express 2009, 17, 4151–4165. [Google Scholar] [CrossRef]
- Ghosh, N.; Wood, M.F.; Vitkin, I.A. Mueller matrix decomposition for extraction of individual polarization parameters from complex turbid media exhibiting multiple scattering, optical activity, and linear birefringence. J. Biomed. Opt. 2008, 13, 044036. [Google Scholar] [CrossRef]
- Voss, K.J.; Zhang, H. Bidirectional reflectance of dry and submerged Labsphere Spectralon plaque. Appl. Opt. 2006, 45, 7924–7927. [Google Scholar] [CrossRef]
- Bhandari, A.A.; Hamre, B.; Frette, Ø.; Zhao, L.; Stamnes, J.J.; Kildemo, M. Bidirectional reflectance distribution function of Spectralon white reflectance standard illuminated by incoherent unpolarized and plane-polarized light. Appl. Opt. 2011, 50, 2431–2442. [Google Scholar] [CrossRef]
- Svensen, Ø.; Kildemo, M.; Maria, J.; Stamnes, J.J.; Frette, Ø. Mueller matrix measurements and modeling pertaining to Spectralon white reflectance standards. Opt. Express 2012, 20, 15045–15053. [Google Scholar] [CrossRef]
- Kildemo, M.; Maria, J.; Ellingsen, P.G.; Aas, L.M.S. Parametric model of the Mueller matrix of a Spectralon white reflectance standard deduced by polar decomposition techniques. Opt. Express 2013, 21, 18509–18524. [Google Scholar] [CrossRef]
- Dupont, J.; Orlik, X.; Ceolato, R.; Dartigalongue, T. Spectralon spatial depolarization: Towards an intrinsic characterization using a novel phase shift distribution analysis. Opt. Express 2017, 25, 9544–9555. [Google Scholar] [CrossRef] [PubMed]
- Sanz, J.M.; Extremiana, C.; Saiz, J.M. Comprehensive polarimetric analysis of Spectralon white reflectance standard in a wide visible range. Appl. Opt. 2013, 52, 6051–6062. [Google Scholar] [CrossRef] [PubMed]


| Ψθ (°) | Ψδ (°) | δ0 = 45° | δ0 = 90° | δ0 = 135° | ||
|---|---|---|---|---|---|---|
| 0 | 0 | 0 | 0 | 0 | ||
| 0 | 90 | 0.07 | 0.07 | 0.07 | ||
| 0 | 180 | Δ→ | 0.24 | 0.24 | 0.24 | |
| 90 | 90 | 0.17 | 0.42 | 0.63 | ||
| 180 | 0 | 0.20 | 0.67 | 0.67 | ||
| 180 | 90 | 0.24 | 0.67 | 0.67 |
| δ | Retardance of a given pixel |
| δ0 | Central retardance of the N considered pixels |
| Ψδ | Extend of the range of the retardances around δ0 for the N considered pixels |
| θ | Orientation of the eigen axis of a given pixel |
| θ0 | Central orientation of the eigen axes of the N considered pixels (θ0 = 0) |
| Ψθ | Extend of the range of the orientations of the eigen axes around θ0 for the N considered pixels |
| DL | Linear diattenuation of a given pixel |
| ΨDL | Extend of the range of the linear diattenuations, from 0 to the maximum value DLmax, for the N considered pixels (i.e., ΨDL = DLmax) |
| φ | Circular retardance of a given pixel |
| Ψφ | Extend of the range of the circular retardances, from 0 to the maximum value φmax, for the N considered pixels (i.e., Ψφ = φmax) |
| Δ | Depolarization power calculated for the N considered pixels, for given δ0, Ψδ, Ψθ, ΨDL and Ψφ |
| Δmax | Maximum attainable depolarization power calculated for the N considered pixels, for given δ0, ΨDL and Ψφ (Ψδ = 180°, Ψθ = 180°) |
| ΨDL → | 0 | 0.1 | 0.5 | 0.8 | 0.99 | |||||
| Ψφ (°) ↓ | ||||||||||
| 0 | 0.37 | 0.37 | 0.38 | 0.40 | 0.43 | |||||
| 60 | 0.45 | 0.46 | 0.47 | 0.50 | 0.51 | |||||
| 120 | 0.65 | 0.65 | 0.66 | 0.69 | 0.71 | |||||
| 180 | 0.84 | 0.85 | 0.85 | 0.86 | 0.88 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Buckley, C.; Fabert, M.; Pagnoux, D. Depolarization Measurement through a Single-Mode Fiber-Based Endoscope for Full Mueller Endoscopic Polarimetric Imaging. Photonics 2023, 10, 387. https://doi.org/10.3390/photonics10040387
Buckley C, Fabert M, Pagnoux D. Depolarization Measurement through a Single-Mode Fiber-Based Endoscope for Full Mueller Endoscopic Polarimetric Imaging. Photonics. 2023; 10(4):387. https://doi.org/10.3390/photonics10040387
Chicago/Turabian StyleBuckley, Colman, Marc Fabert, and Dominique Pagnoux. 2023. "Depolarization Measurement through a Single-Mode Fiber-Based Endoscope for Full Mueller Endoscopic Polarimetric Imaging" Photonics 10, no. 4: 387. https://doi.org/10.3390/photonics10040387
APA StyleBuckley, C., Fabert, M., & Pagnoux, D. (2023). Depolarization Measurement through a Single-Mode Fiber-Based Endoscope for Full Mueller Endoscopic Polarimetric Imaging. Photonics, 10(4), 387. https://doi.org/10.3390/photonics10040387




