Lithography Alignment Techniques Based on Moiré Fringe
Abstract
1. Introduction
2. Generation Mode of Moiré Fringe and Its Development in Lithography Alignment
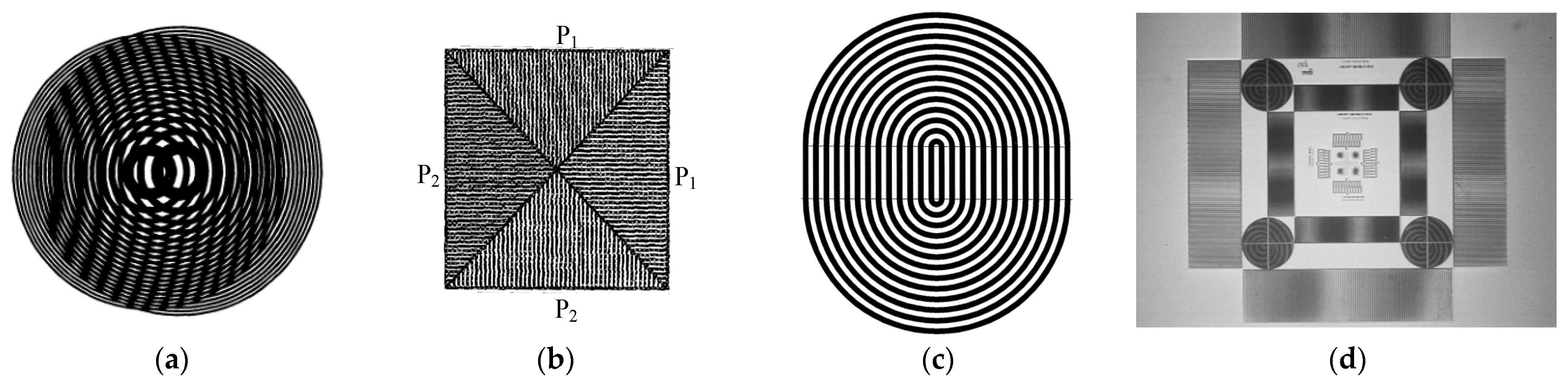
2.1. Generation and Classification of Moiré Fringe
2.2. Application Status of Moiré Fringes in Lithography Alignment
2.2.1. Development of Physical Grating
- (1)
- Circular/linear single grating:
- (2)
- Composite grating:
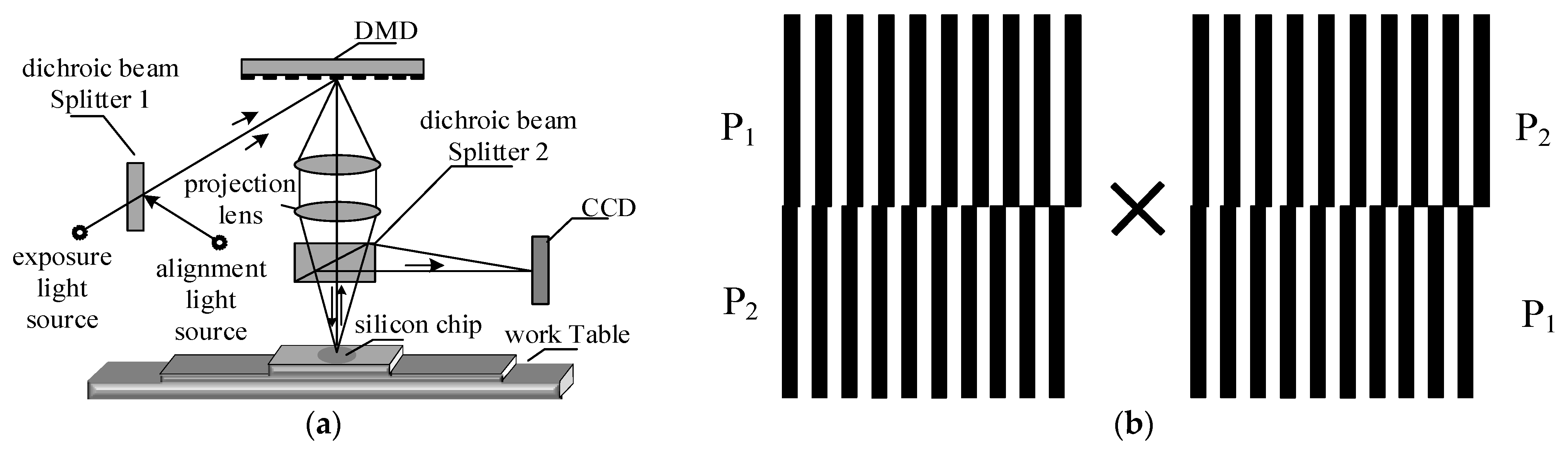
2.2.2. Development of Digital Grating
2.3. Summary of Moiré Fringe Lithography Alignment Technology
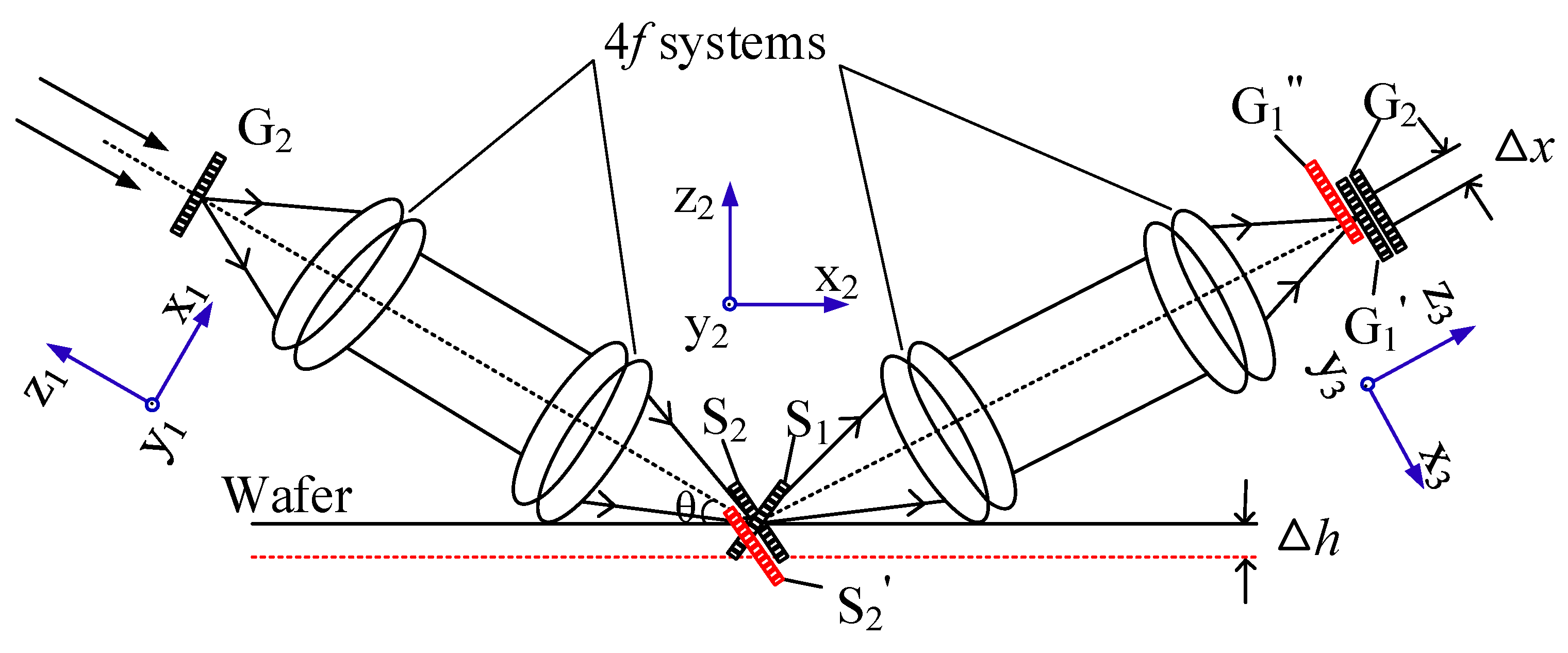
3. Moiré Fringe Alignment Principle and Alignment Process
3.1. Alignment Principle
3.1.1. Calibration Principle of Out-of-2D Plane Inclination Angle
3.1.2. Principle of In-Plane Rotation Angle Calibration
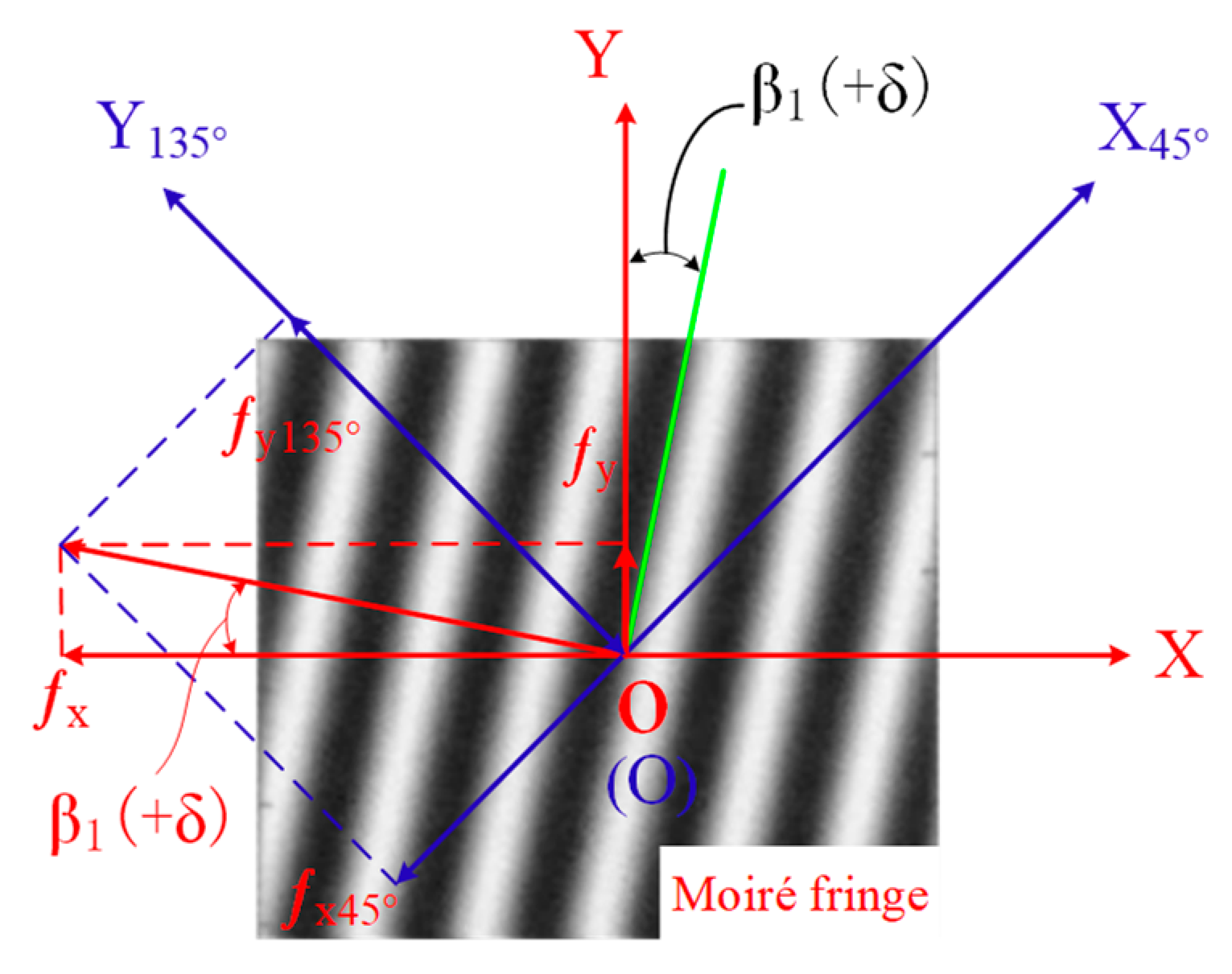
3.1.3. Principle of Fine Calibration in the Vertical Grid Line Direction
3.2. Alignment Process
3.2.1. Calibration of Out-of-2D Plane Inclination Angle
3.2.2. In-Plane Angular Displacement Calibration
3.2.3. Fine Displacement Correction after Grating Parallelization
4. Accuracy Analysis of Moiré Fringe Lithography Alignment Technology
4.1. Generation Stage of Moiré Fringes
- (1)
- Structural parameters and fabrication process of the grating:
- (2)
- Type, wavelength, and accuracy of the alignment light source:
- (3)
- Alignment marking design:
4.2. Alignment of Mask to Wafer Stage
- (1)
- Periodic repeatability of the Moiré fringe:
- (2)
- The Talbot effect:
- (3)
- Parallelism between the mask and the wafer
4.3. Moiré Image Generation and Post-Processing Stages
- (1)
- Performance parameters of CCD:
- (2)
- Spectrum filtering algorithm:
- (3)
- Phase extraction algorithm
- (4)
- Image processing algorithms
4.4. Other Factors
5. Optimization of the Moiré Fringe Alignment Scheme
5.1. Extending Moiré Fringe Alignment Range
5.2. Enhancing Moiré Image Contrast
5.3. Improving the Flexibility of Moiré Fringe Alignment
5.4. Improving the Alignment Accuracy of Moiré Fringe
5.4.1. Lithographic Alignment Based on Self-Coherent Moiré Grating Fringes
5.4.2. Moiré Fringe Alignment Based on Deep Learning
6. Development Trends and Prospects
- (1)
- Marking structure:
- (2)
- Alignment scheme:
- (3)
- Phase algorithm:
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yang, X.F.; Zeng, B.B.; Wang, C.T.; Luo, X.G. Breaking the feature sizes down to sub-22 nm by plasmonic interference lithography using dielectric-metal multilayer. Opt. Express 2009, 17, 21560–21565. [Google Scholar] [CrossRef] [PubMed]
- Guo, L.J. Nanoimprint lithography: Methods and material requirements. Adv. Mater. 2007, 19, 495–513. [Google Scholar] [CrossRef]
- Gwyn, C.W.; Stulen, R.; Sweeney, D.; Attwood, D. Extreme ultraviolet lithography. J. Vac. Sci. Technol. B Microelectron. Nanometer Struct. Process. Meas. Phenom. 1998, 16, 3142–3149. [Google Scholar] [CrossRef]
- Whang AJ, W.; Gallagher, N.C. Synthetic approach to designing optical alignment systems. Appl. Opt. 1988, 27, 3534–3541. [Google Scholar] [CrossRef] [PubMed]
- Tan, C.B.; Yeo, S.H.; Koh, H.P.; Koo, C.K.; Foong, Y.M.; Siew, Y.K. Evaluation of alignment marks using ASML ATHENA alignment system in 90-nm BEOL process. In Proceedings of the Metrology, Inspection, and Process Control for Microlithography XVII, Santa Clara, CA, USA, 23–28 February 2003; Volume 5038, pp. 1211–1218. [Google Scholar]
- Itoh, J.; Kanayama, T.; Atoda, N.; Hoh, K. An alignment system for synchrotron radiation x-ray lithography. J. Vac. Sci. Technol. B Microelectron. Process. Phenom. 1988, 6, 409–412. [Google Scholar] [CrossRef]
- Uzun, Y.; Kuran, O.; Avci, I. Fabrication of Superconducting YBa2Cu3O7−x Thin Films on Si Wafer via YSZ/CeO2 buffer layers. J. Supercond. Nov. Magn. 2017, 30, 2335–2340. [Google Scholar] [CrossRef]
- Amidror, I. The Theory of the Moiré Phenomenon. In Theoretical and Mathematical Physicsl; Springer: Berlin/Heidelberg, Germany, 2000. [Google Scholar]
- Dai, X.; Cao, Q.; Xie, H. Characterization for Young’s modulus of TBCs using soft lithography gratings and moiré interferometry. Measurement 2018, 122, 201–211. [Google Scholar] [CrossRef]
- Xing, H.D.; Gao, Z.X.; Wang, H.T.; Lei, Z.K.; Ma, L.L.; Qiu, W. Digital rotation moiré method for strain measurement based on high-resolution transmission electron microscope lattice image. Opt. Lasers Eng. 2019, 122, 347–353. [Google Scholar] [CrossRef]
- Yen, K.S.; Ratnam, M.M. Simultaneous measurement of 3-D displacement components from circular grating moiré fringes: An experimental approach. Opt. Lasers Eng. 2012, 50, 887–899. [Google Scholar] [CrossRef]
- Arai, Y.; Yokozeki, S.; Yamada, T. Fringe-scanning method using a general function for shadow moiré. Appl. Opt. 1995, 34, 4877–4882. [Google Scholar] [CrossRef]
- Bryngdahl, O. Characteristics of superposed patterns in optics. J. Opt. Soc. Am. A 1976, 66, 87–94. [Google Scholar] [CrossRef]
- King, M.C.; Berry, D.H. Photolithographic mask alignment using moiré techniques. Appl. Opt. 1972, 11, 2455–2459. [Google Scholar] [CrossRef] [PubMed]
- Kawai, S.; Torii, Y. Highly precise alignments using moiré diffraction methods. Jpn. J. Appl. Phys. 1998, 37, 3691–3694. [Google Scholar] [CrossRef]
- Flanders, D.C.; Smith, H.I.; Austin, S. A new interferometric alignment technique. Appl. Phys. Lett. 1977, 31, 426–428. [Google Scholar] [CrossRef]
- Lyszczarz, T.M.; Flanders, D.C.; Economou, N.P.; DeGraff, P.D. Experimental evaluation of interferometric alignment techniques for multiple mask registration. J. Vac. Sci. Technol. 1981, 19, 1214–1218. [Google Scholar] [CrossRef]
- Moel, A.; Moon, E.E.; Frankel, R.D.; Smith, H.I. Novel on-axis interferometric alignment method with sub-10 nm precision. J. Vac. Sci. Technol. B Microelectron. Nanometer Struct. Process. Meas. Phenom. 1993, 11, 2191–2194. [Google Scholar] [CrossRef]
- Song, J.S.; Lee, Y.H.; Jo, J.H.; Chang, S.; Yuk, K.C. Moiré patterns of two different elongated circular gratings for the fine visual measurement of linear displacements. Opt. Commun. 1998, 154, 100–108. [Google Scholar] [CrossRef]
- Li, N.; Wu, W.; Chou, S.Y. Sub-20-nm alignment in nanoimprint lithography using Moire fringe. Nano Lett. 2006, 6, 2626–2629. [Google Scholar] [CrossRef]
- Mühlberger, M.; Bergmair, I.; Schwinger, W.; Gmainer, M.; Schoeftner, R.; Glinsner, T.; Hasenfuss, C.; Hingerl, K.; Vogler, M.; Schmidt, H.; et al. A Moiré method for high accuracy alignment in nanoimprint lithography. Microelectron. Eng. 2007, 84, 925–927. [Google Scholar] [CrossRef]
- Zhu, J.P.; Hu, S.; Yu, J.S.; Zhou, S.L.; Tang, Y.; Zhong, M.; Zhao, L.X.; Chen, M.Y.; Li, L.L.; He, Y.; et al. Four-quadrant gratings moiré fringe alignment measurement in proximity lithography. Opt. Express 2013, 21, 3463–3473. [Google Scholar] [CrossRef]
- Zhu, J.P.; Hu, S.; Yu, J.S.; Tang, Y.; Xu, F.; He, Y.; Zhou, S.L.; Li, L.L. Influence of tilt moire fringe on alignment accuracy in proximity lithography. Opt. Lasers Eng. 2013, 51, 371–381. [Google Scholar] [CrossRef]
- Asundi, A.; Yung, K.H. Phase-shifting and logical moiré. J. Opt. Soc. Am. A 1991, 8, 1591–1600. [Google Scholar] [CrossRef]
- Tang, L.; Hu, S.; Xu, F.; Tang, Y.; Chen, M.; Zhu, J. A digital-grating-based alignment technique in maskless lithography. Chin. J. Lasers 2012, 39, 244–249. [Google Scholar]
- Zhang, T.; Ma, Y.R.; Li, J.A.; Sun, T.; Zhao, X.Y.; Cui, J.W. Focus calibration method based on the illumination beam scanning angle modulation in a grating alignment system. Opt. Express 2021, 29, 9429–9445. [Google Scholar] [CrossRef] [PubMed]
- Di, C.L.; Yan, W.; Hu, S.; Li, Y.L.; Yin, D.D.; Tang, Y.; Tong, J.M. A moiré-based four-channel focusing and leveling scheme for projection lithography. IEEE Photonics J. 2014, 6, 1–12. [Google Scholar] [CrossRef]
- Zhou, S.L.; Yang, Y.; Zhao, L.; Zhang, L.X.; Hu, S. Tilt-modulated spatial phase imaging method for wafer-mask leveling in proximity lithography. Opt. Lett. 2010, 35, 3132–3134. [Google Scholar] [CrossRef]
- Jiang, W.; Liu, A. Image motion deblurring based on deep residual shrinkage and generative adversarial networks. Comput. Intell. Neurosci. 2022, 2022, 15–29. [Google Scholar] [CrossRef]
- Zhou, S.L.; Xie, C.Q.; Yang, Y.; Hu, S.; Xu, X.M.; Yang, J. Moiré-based phase imaging for sensing and adjustment of in-plane twist angle. IEEE Photonics Technol. Lett. 2013, 25, 1847–1850. [Google Scholar] [CrossRef]
- Zhu, J.P.; Hu, S.; Su, X.Y.; You, Z.S. Adjustment strategy for inclination Moiré fringes in lithography by spatial frequency decomposition. IEEE Photonics Technol. Lett. 2014, 27, 395–398. [Google Scholar]
- Chan JY, E.; Ruan, Q.; Ng RJ, H.; Qiu, C.W.; Yang, J.K. Rotation-selective moiré magnification of structural color pattern arrays. ACS Nano 2019, 13, 14138–14144. [Google Scholar] [CrossRef]
- Zhu, J.P.; Hu, S.; Yu, J.S.; Tang, Y. Alignment method based on matched dual-grating moiré fringe for proximity lithography. Opt. Eng. 2012, 51, 113603. [Google Scholar] [CrossRef]
- Wang, N.; Jiang, W.; Zhu, J.P.; Tang, Y.; Yan, W.; Tong, J.M.; Hu, S. Influence of collimation on alignment accuracy in proximity lithography. IEEE Photonics J. 2014, 6, 1–10. [Google Scholar] [CrossRef]
- Zhu, J.P.; Hu, S.; Zhou, P.; Yu, J.S. Experimental study of Talbot imaging moiré-based lithography alignment method. Opt. Lasers Eng. 2014, 58, 54–59. [Google Scholar] [CrossRef]
- Cheng, J.L.; Zuo, F.; Yang, Z.M.; Ye, J.F.; Gao, Z.S.; Yuan, Q. Additive-to-multiplicative moiré fringe transition in simultaneous dual-wavelength interferometry. Opt. Lasers Eng. 2021, 141, 106561. [Google Scholar] [CrossRef]
- Di, C.L.; Yan, W.; Hu, S.; Yin, D.D.; Ma, C.F. Moiré-based absolute interferometry with large measurement range in wafer-mask alignment. IEEE Photonics Technol. Lett. 2014, 27, 435–438. [Google Scholar] [CrossRef]
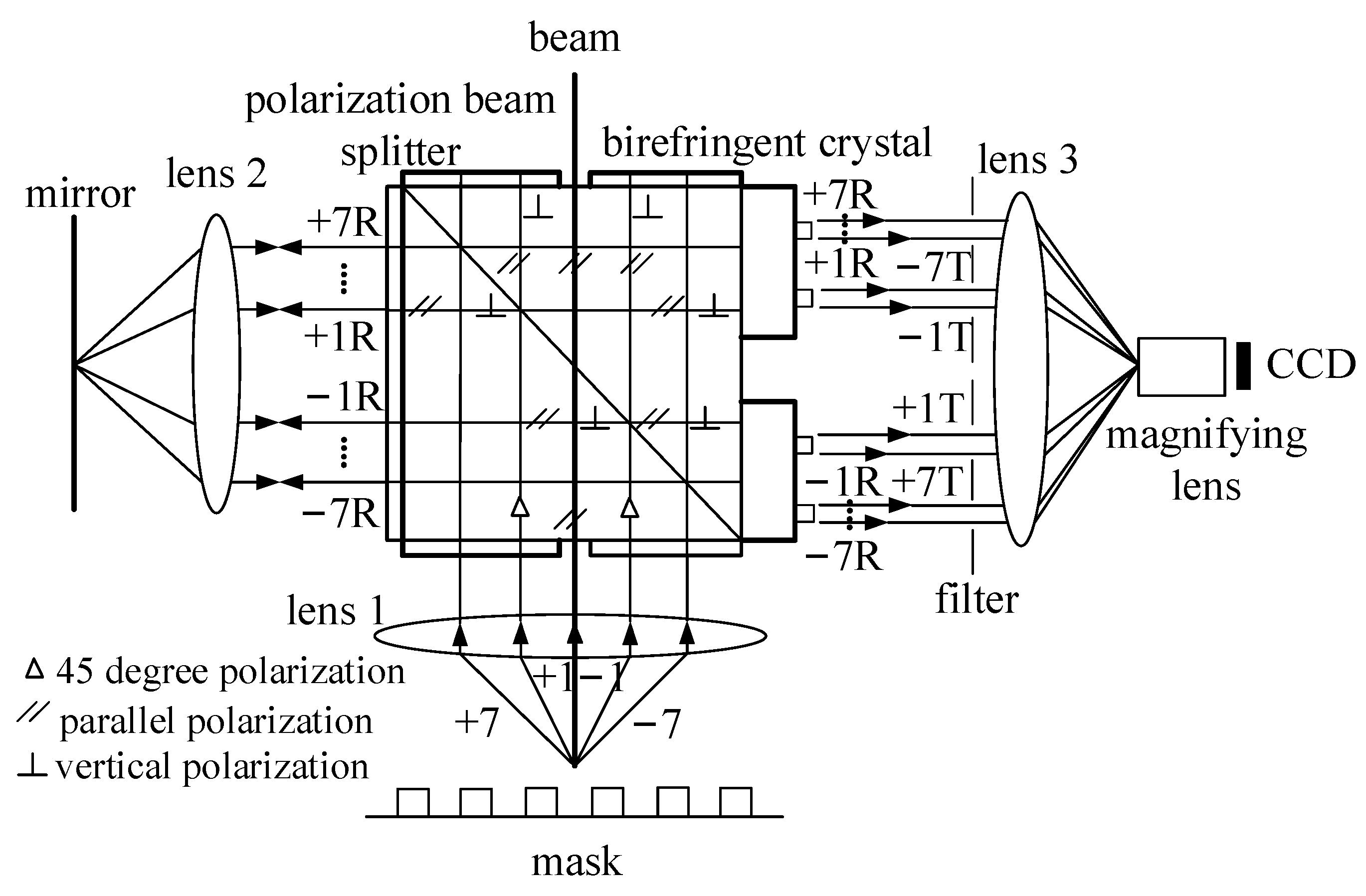
- Tang, Y.; Liu, J.B.; Yang, Y.; Hu, S.; Zhao, L.X.; Si, X.C. Large range nano alignment for proximity lithography using complex grating. Opt. Laser Technol. 2019, 112, 101–106. [Google Scholar] [CrossRef]
- Huang, B.Y.; Wang, C.X.; Fang, H.; Zhou, S.C.; Suga, T. Moiré-based alignment using centrosymmetric grating marks for high-precision wafer bonding. Micromachines 2019, 10, 339. [Google Scholar] [CrossRef]
- Wang, N.; Jiang, W.; Zhang, Y. Misalignment measurement with dual-frequency moiré fringe in nanoimprint lithography. Opt. Express 2020, 28, 6755–6765. [Google Scholar] [CrossRef]
- Qin, J.; Ding, L.; Wang, L. In situ UV nano-imprint lithography alignment using high contrast mark. Opt. Express 2015, 23, 18518–18524. [Google Scholar] [CrossRef]
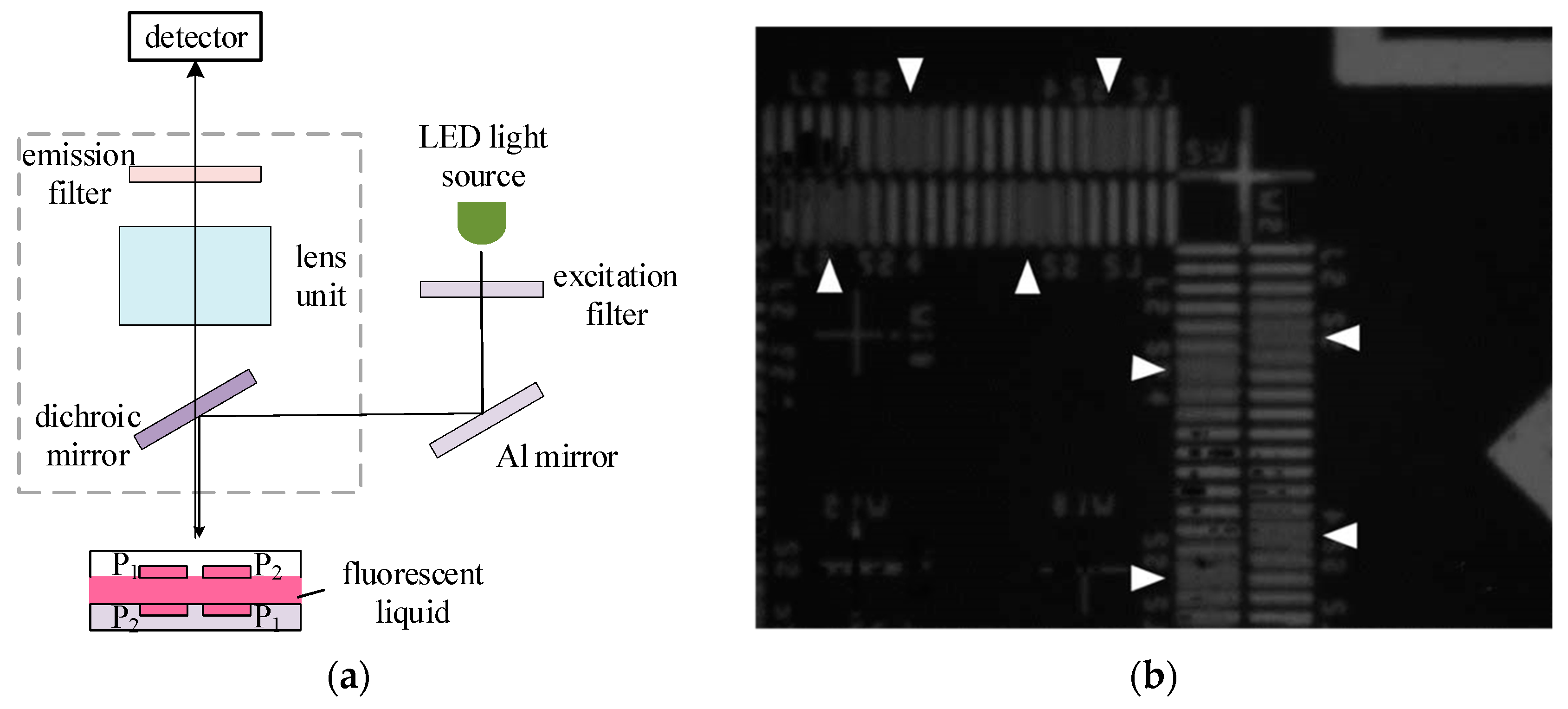
- Yoshida, T.; Ito, S.; Ochiai, K.; Nakamura, T.; Nakagawa, M. Fluorescence imprint alignment using additive-type inclination moiré fringes. Jpn. J. Appl. Phys. 2020, 59, SIIJ04. [Google Scholar] [CrossRef]
- Shao, J.Y.; Ding, Y.C.; Tian, H.M.; Li, X.; Li, X.M.; Liu, H.Z. Digital moiré fringe measurement method for alignment in imprint lithography. Opt. Laser Technol. 2012, 44, 446–451. [Google Scholar] [CrossRef]
- Du, J.Y.; Dai, F.Z.; Yang, B.; Wang, X.Z. Alignment technique using Moiré fringes based on self-coherence in lithographic tools. Chin. J. Lasers 2017, 44, 183–190. [Google Scholar]
- Wang, N.; Jiang, W.; Zhang, Y. Moiré-based sub-nano misalignment sensing via deep learning for lithography. Opt. Lasers Eng. 2021, 143, 106620. [Google Scholar] [CrossRef]
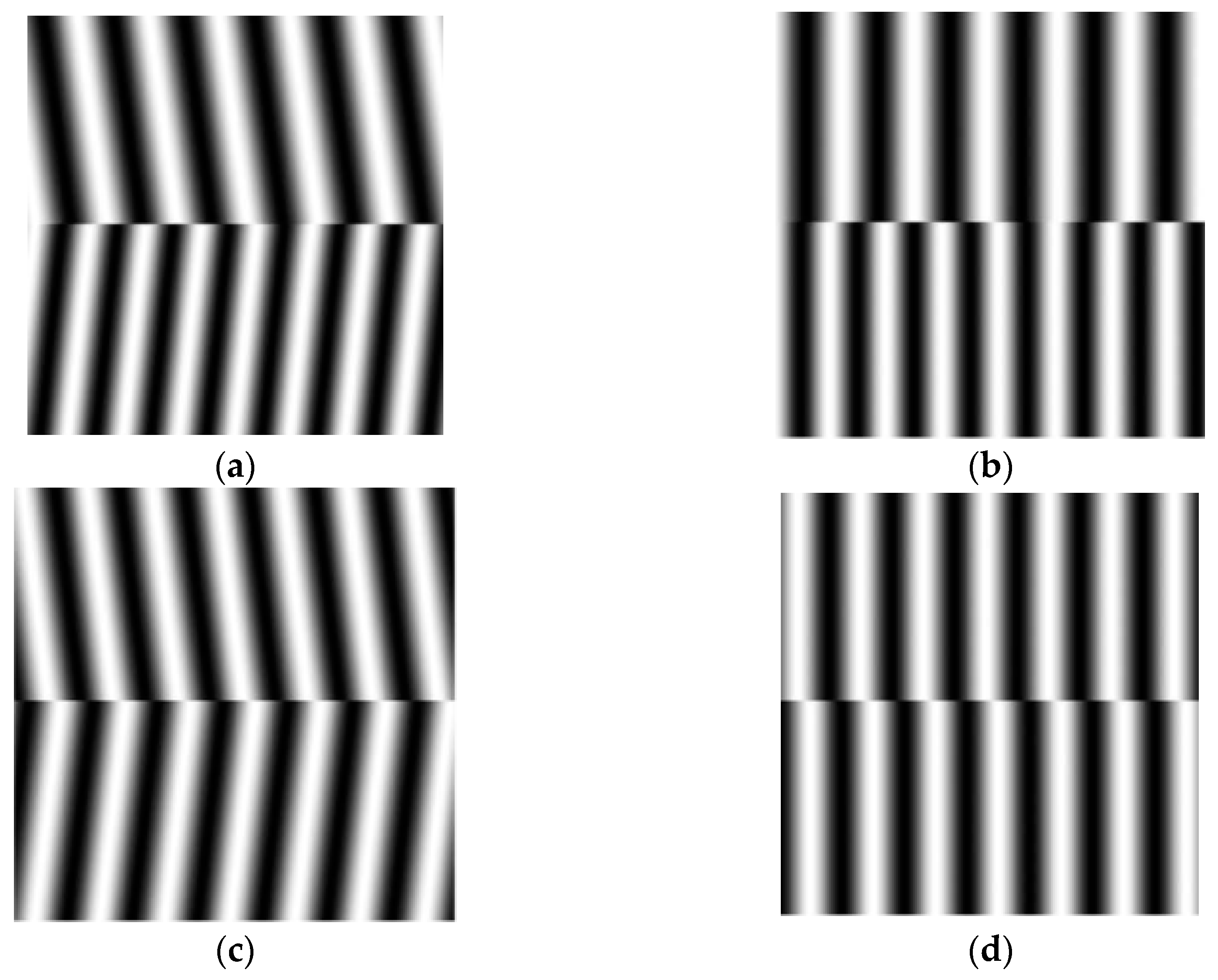




| Method | P1 = 4 μm and P2 = 4.4 μm | P1 = 8 μm and P2 = 10 μm | ||||
|---|---|---|---|---|---|---|
| MaAE | MeAE | SD | MaAE | MeAE | SD | |
| FFT | 13.05 | 5.67 | 7.96 | 13.13 | 5.85 | 7.03 |
| WT | 12.65 | 5.58 | 6.86 | 12.76 | 5.76 | 7.85 |
| DLMRM | 0.60 | 0.22 | 0.42 | 0.58 | 0.23 | 0.31 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, W.; Wang, H.; Xie, W.; Qu, Z. Lithography Alignment Techniques Based on Moiré Fringe. Photonics 2023, 10, 351. https://doi.org/10.3390/photonics10040351
Jiang W, Wang H, Xie W, Qu Z. Lithography Alignment Techniques Based on Moiré Fringe. Photonics. 2023; 10(4):351. https://doi.org/10.3390/photonics10040351
Chicago/Turabian StyleJiang, Wenbo, Huaran Wang, Wenda Xie, and Zhefei Qu. 2023. "Lithography Alignment Techniques Based on Moiré Fringe" Photonics 10, no. 4: 351. https://doi.org/10.3390/photonics10040351
APA StyleJiang, W., Wang, H., Xie, W., & Qu, Z. (2023). Lithography Alignment Techniques Based on Moiré Fringe. Photonics, 10(4), 351. https://doi.org/10.3390/photonics10040351





