Photonic Generation of Background-Free Phase-Coded Microwave Pulses with Elimination of Power Fading
Abstract
1. Introduction
2. Principle and Methods
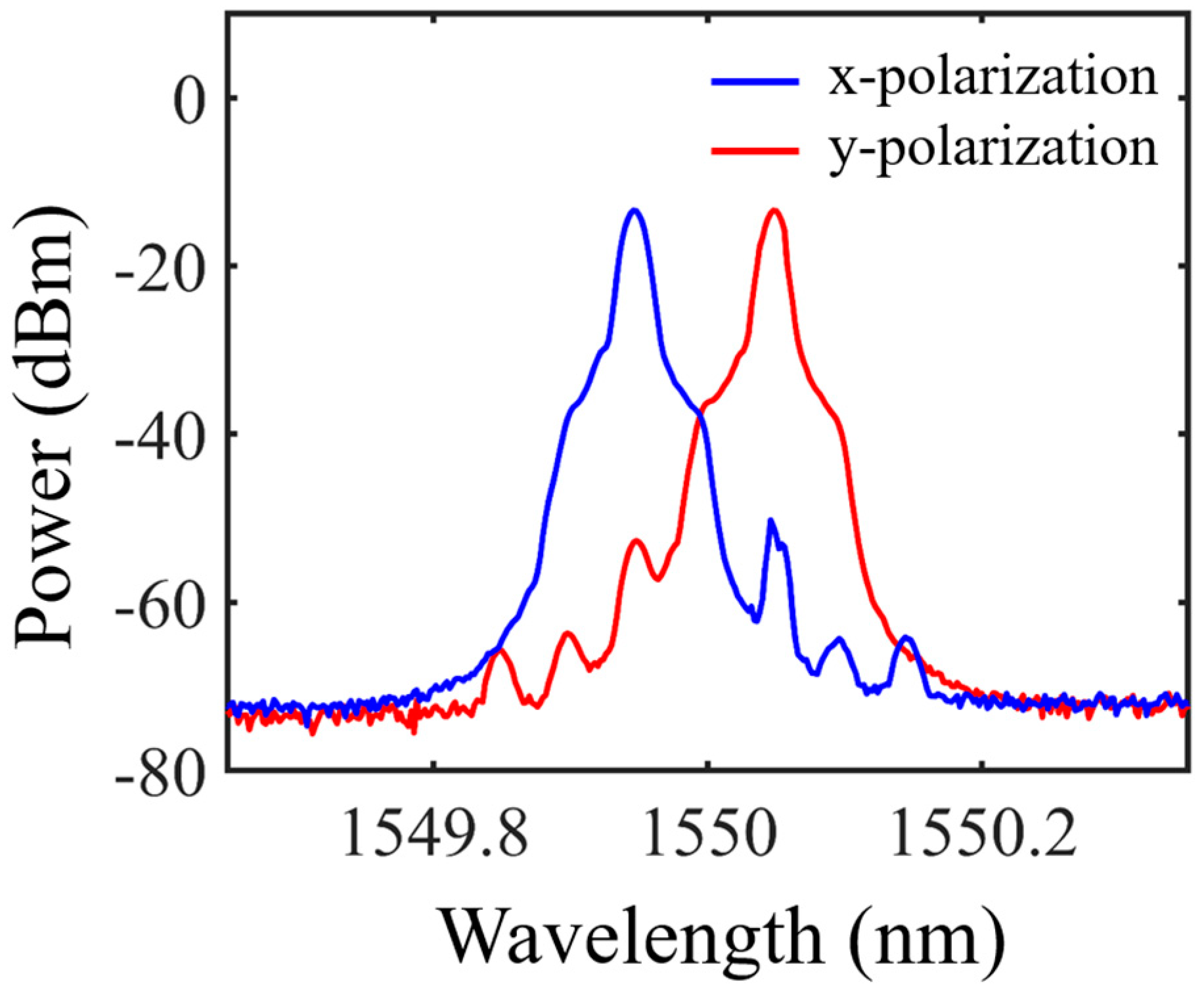
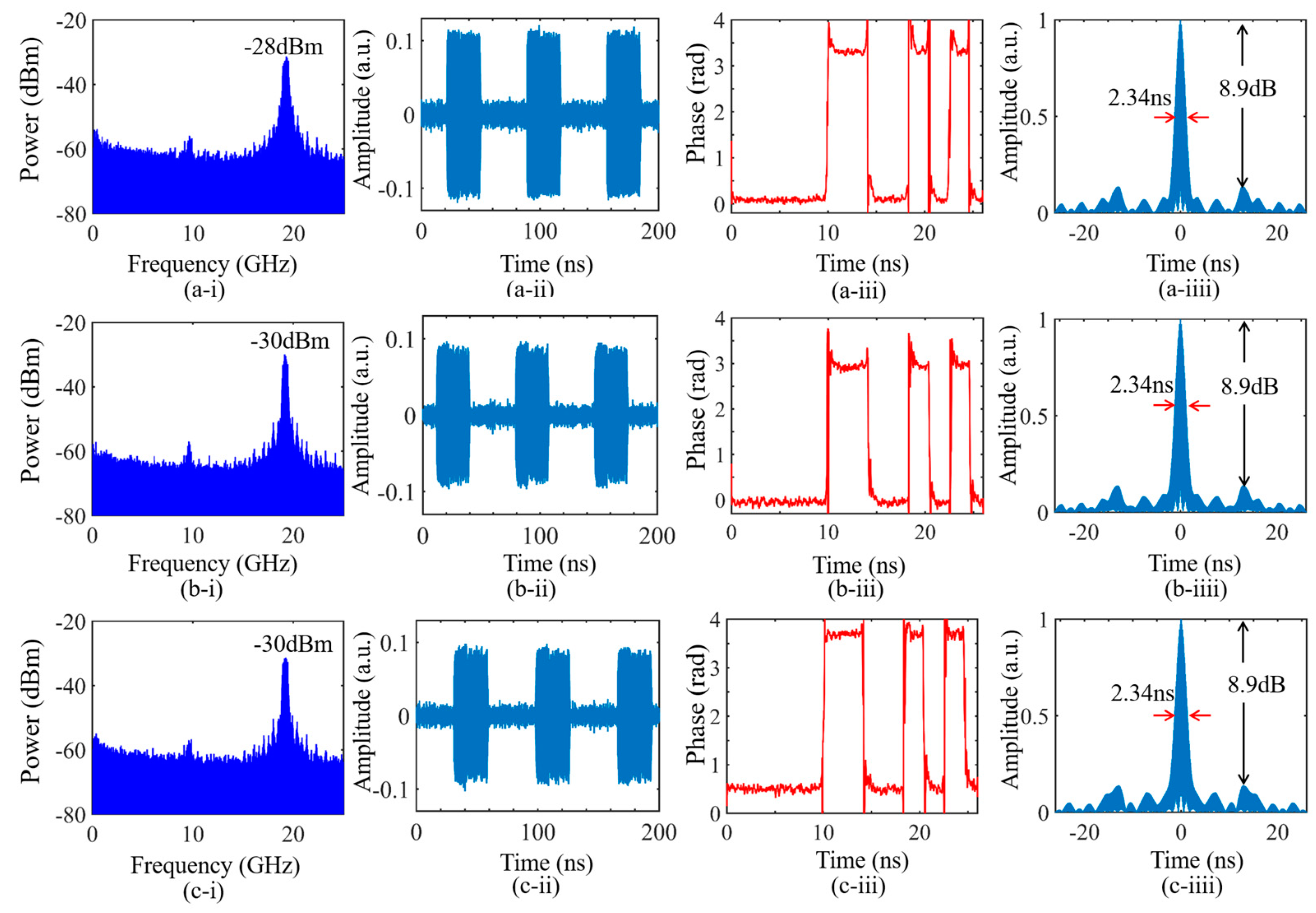
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Skolnik, M. Role of radar in microwaves. IEEE Trans. Microw. Theory Tech. 2002, 50, 625–632. [Google Scholar] [CrossRef]
- Ghelfi, P.; Laghezza, F.; Scotti, F. A fully photonics-based coherent radar system. Nature 2014, 507, 341–345. [Google Scholar] [CrossRef]
- McKinney, J.D.; Leaird, D.E.; Weiner, A.M. Millimeter-wave arbitrary waveform generation with a direct space-to-time pulse shaper. Opt. Lett. 2002, 27, 1345–1347. [Google Scholar] [CrossRef] [PubMed]
- Ye, J.; Yan, L.; Chen, Z.; Pan, W.; Luo, B.; Zou, X.; Yi, A.; Yao, S. Photonic generation of microwave phase-coded signals based on frequency-to-time conversion. IEEE Photon. Technol. Lett. 2012, 24, 1527–1529. [Google Scholar] [CrossRef]
- Tang, Z.; Zhang, T.; Zhang, F.; Pan, S. Photonic generation of a phase-coded microwave signal based on a single dual-drive Mach–Zehnder modulator. Opt. Lett. 2013, 38, 5365–5368. [Google Scholar] [CrossRef] [PubMed]
- Li, W.; Wang, L.; Li, M.; Wang, H.; Zhu, N. Photonic generation of binary phase-Coded microwave signals with large frequency tunability using a Dual-Parallel Mach–Zehnder Modulator. IEEE Photon. J. 2013, 38, 5365–5368. [Google Scholar]
- Chen, Y.; Pan, S. A frequency-tunable binary phase-coded microwave signal generator with a tunable frequency multiplication factor. IEEE Photon. J. 2017, 9, 1–15. [Google Scholar] [CrossRef]
- Zhu, S.; Shi, Z.; Li, M.; Zhu, N.; Li, W. Simultaneous frequency up-conversion and phase coding of a radio-frequency signal for photonic radars. Opt. Lett. 2018, 43, 583–586. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Zhang, F.; Pan, S. Generation of frequency-multiplied and phase-coded signal using an optical polarization division multiplexing modulator. IEEE Trans. Theory Tech. 2017, 65, 651–660. [Google Scholar] [CrossRef]
- Zhai, W.; Wen, A. Microwave photonic multifunctional phase coded signal generator. IEEE Photon. Technol. Lett. 2019, 31, 1377–1380. [Google Scholar] [CrossRef]
- Zhai, W.; Wen, A. Photonic generation of a dual-band polyphase-coded microwave signal with a tunable frequency multiplication factor. J. Light. Technol. 2019, 37, 4911–4920. [Google Scholar] [CrossRef]
- Chi, H.; Yao, J. An approach to photonic generation of high-frequency phase-coded RF pulses. IEEE Photon. Technol. Lett. 2007, 19, 768–770. [Google Scholar] [CrossRef]
- Chi, H.; Yao, J. Photonic Generation of Phase-Coded Millimeter-Wave Signal Using a Polarization Modulator. IEEE Microw. Wirel. Compon. Lett. 2008, 18, 371–373. [Google Scholar] [CrossRef]
- Fan, X.; Cao, X.; Li, M.; Zhu, N.; Li, W. Photonic Generation of Multi-Band Phase-Coded Microwave Pulses by Polarization Manipulation of Optical Signals. J. Light. Technol. 2021, 40, 672–680. [Google Scholar] [CrossRef]
- Li, W.; Wang, L.; Wang, H.; Zhu, N. Photonic generation of widely tunable and background-free binary phase-coded radio-frequency pulses. Opt. Lett. 2013, 38, 3441–3444. [Google Scholar] [CrossRef] [PubMed]
- Zhu, S.; Li, M.; Zhu, N.; Li, W. Transmission of dual-chirp microwave waveform over fiber with compensation of dispersion-induced power fading. Opt. Lett. 2018, 43, 2466–2469. [Google Scholar] [CrossRef] [PubMed]
- Wang, L.; Li, W.; Wang, H.; Zheng, J.; Liu, J.; Zhu, N. Photonic generation of phase coded microwave pulses using cascaded polarization modulators. IEEE Photon. Technol. Lett. 2013, 25, 678–681. [Google Scholar] [CrossRef]
- Yao, J.; Zeng, F.; Wang, Q. Photonic generation of ultrawideband signals. J. Light. Technol. 2007, 25, 3219–3235. [Google Scholar]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guan, M.; Wang, L.; Li, F.; Chen, X.; Li, M.; Zhu, N.; Li, W. Photonic Generation of Background-Free Phase-Coded Microwave Pulses with Elimination of Power Fading. Photonics 2023, 10, 66. https://doi.org/10.3390/photonics10010066
Guan M, Wang L, Li F, Chen X, Li M, Zhu N, Li W. Photonic Generation of Background-Free Phase-Coded Microwave Pulses with Elimination of Power Fading. Photonics. 2023; 10(1):66. https://doi.org/10.3390/photonics10010066
Chicago/Turabian StyleGuan, Mengyuan, Lu Wang, Fangping Li, Xiaoyu Chen, Ming Li, Ninghua Zhu, and Wei Li. 2023. "Photonic Generation of Background-Free Phase-Coded Microwave Pulses with Elimination of Power Fading" Photonics 10, no. 1: 66. https://doi.org/10.3390/photonics10010066
APA StyleGuan, M., Wang, L., Li, F., Chen, X., Li, M., Zhu, N., & Li, W. (2023). Photonic Generation of Background-Free Phase-Coded Microwave Pulses with Elimination of Power Fading. Photonics, 10(1), 66. https://doi.org/10.3390/photonics10010066





