Near-Perfect Narrow-Band Tunable Graphene Absorber with a Dual-Layer Asymmetric Meta-Grating
Abstract
1. Introduction
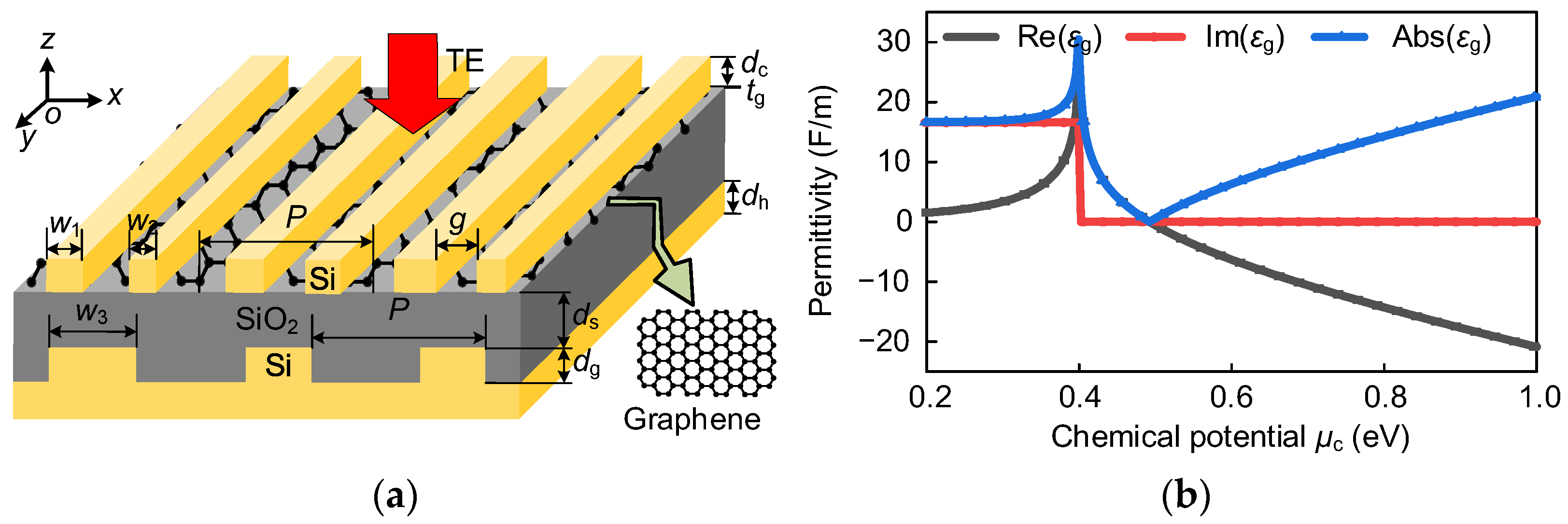
2. Structure and Theory
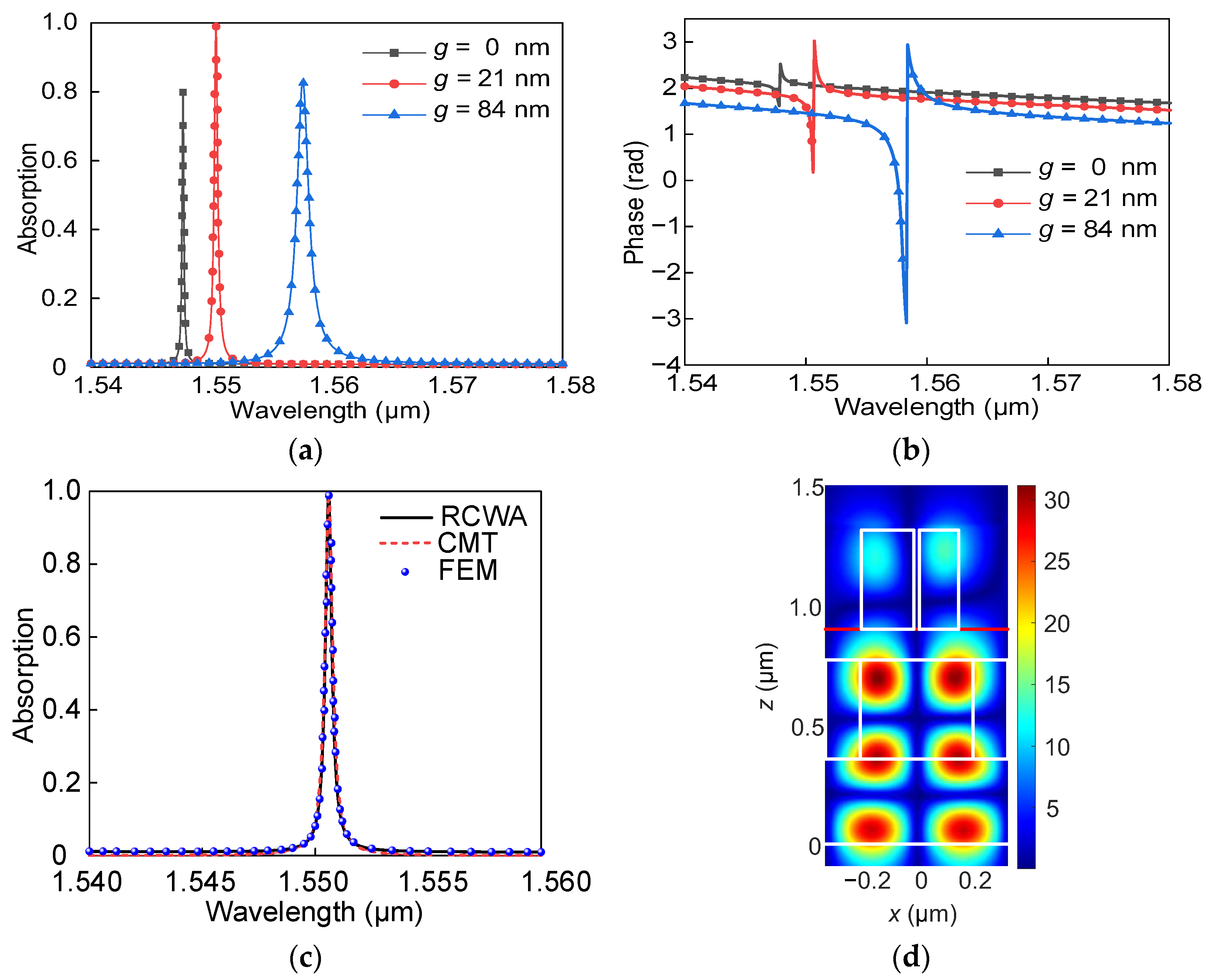
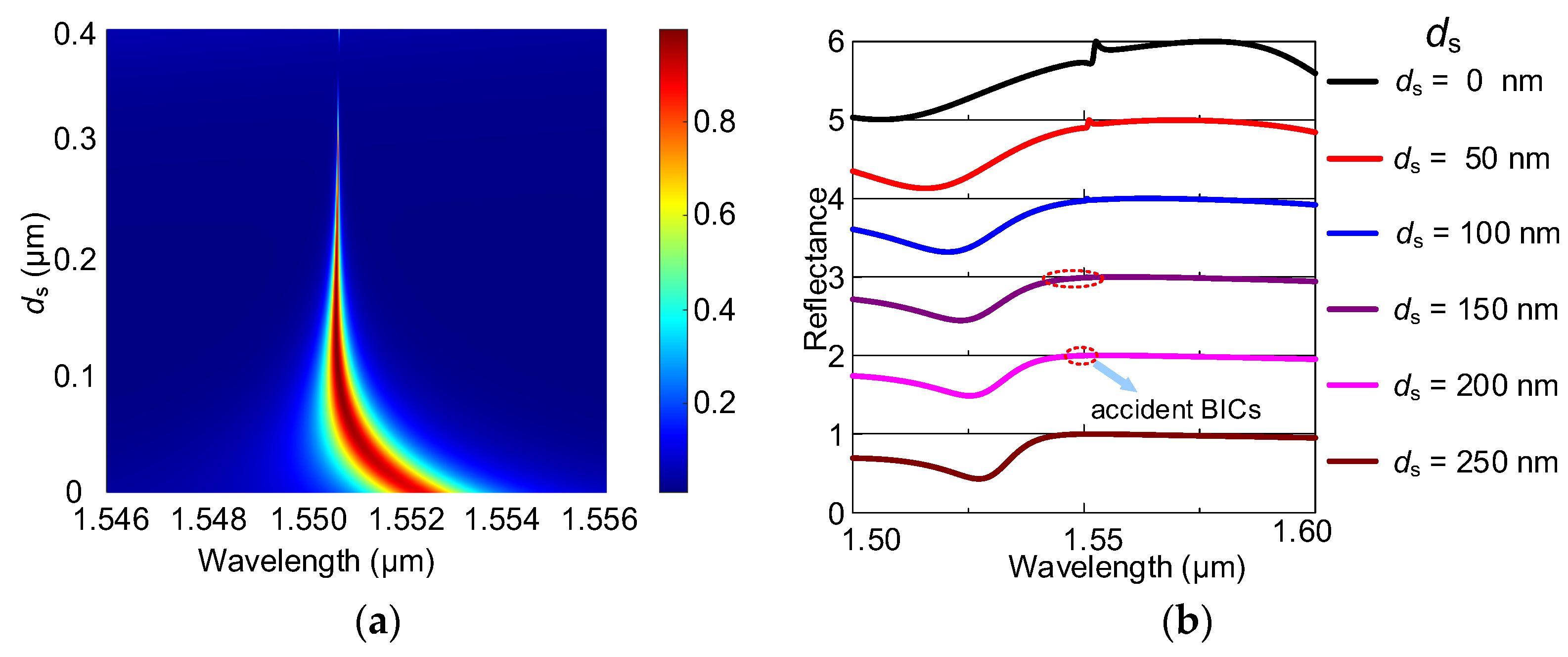
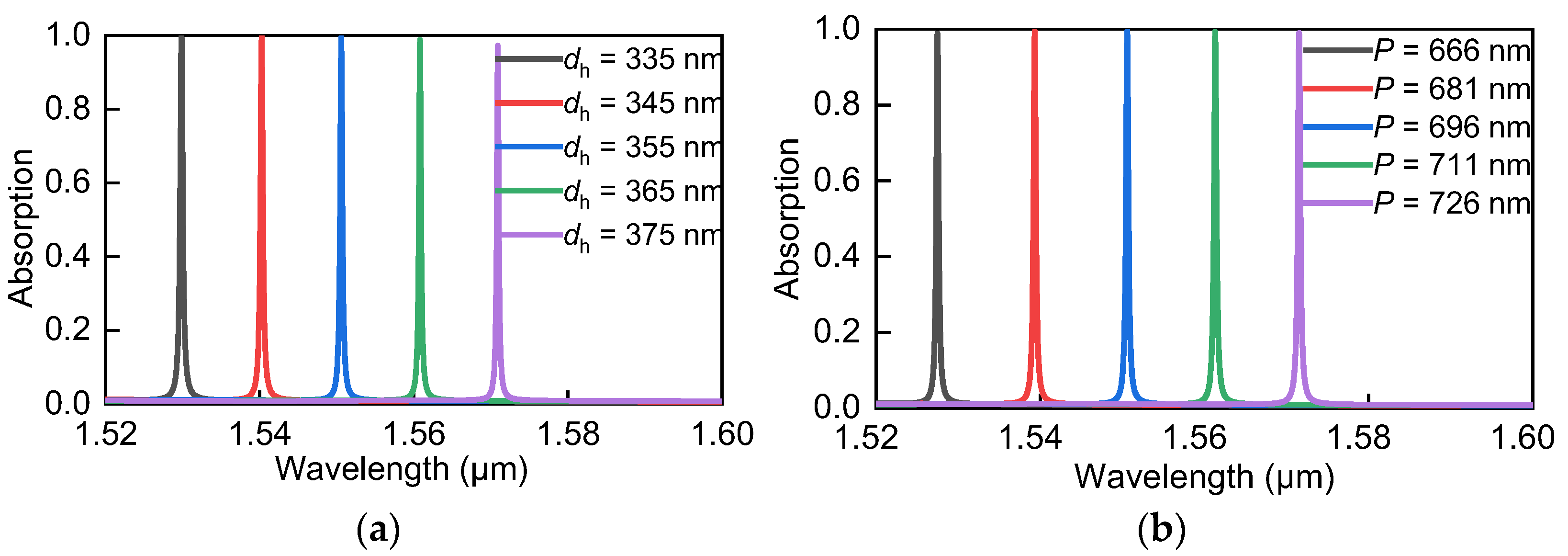
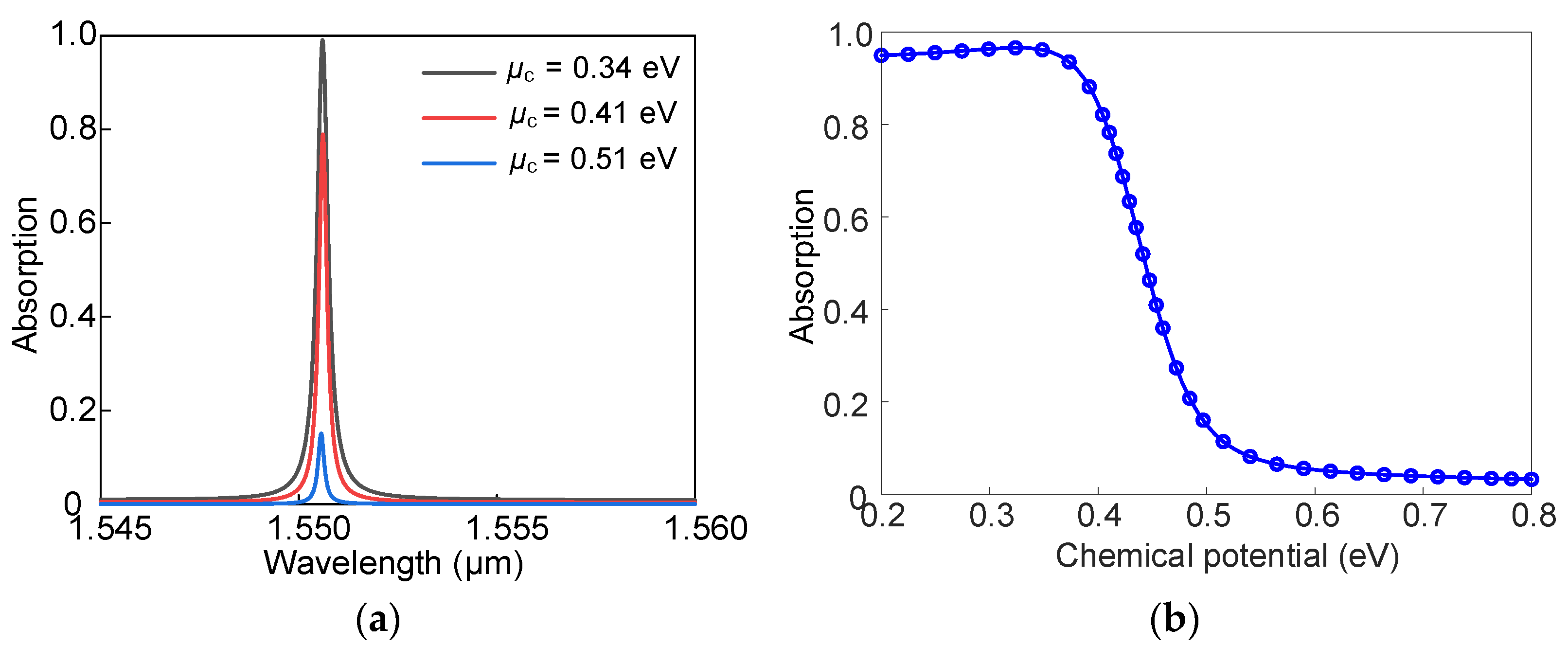
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Duplock, E.J.; Scheffler, M.; Lindan, P.J.D. Hallmark of perfect graphene. Phys. Rev. Lett. 2004, 92, 225502. [Google Scholar] [CrossRef] [PubMed]
- Sarma, S.D.; Adam, S.; Hwang, E.H.; Rossi, E. Electronic transport in two-dimensional graphene. Rev. Mod. Phys. 2011, 83, 407. [Google Scholar] [CrossRef]
- Qing, Y.M.; Ren, Y.Z.; Lei, D.Y.; Ma, H.F.; Cui, T.J. Strong coupling in two-dimensional materials-based nanostructures: A review. J. Opt. 2022, 24, 024009. [Google Scholar] [CrossRef]
- Luo, X.; Zhou, Y.G.; Cai, Y.J.; Cheng, Z.Q.; Liu, Z.M.; Wang, W.Q. A review of perfect absorbers based on the two dimensional materials in the visible and near-infrared regimes. J. Phys. D Appl. Phys. 2021, 55, 093002. [Google Scholar] [CrossRef]
- Furchi, M.; Urich, A.; Pospischil, A.; Lilley, G.; Unterrainer, K.; Detz, H.; Klang, P.; Andrews, A.M.; Schrenk, W.; Strasser, G.; et al. Microcavity-integrated graphene photodetector. Nano Lett. 2012, 12, 2773–2777. [Google Scholar] [CrossRef] [PubMed]
- Liu, C.H.; Chang, Y.C.; Norris, T.B.; Zhong, Z.H. Graphene photodetectors with ultra-broadband and high responsivity at room temperature. Nat. Nanotechnol. 2014, 9, 273–278. [Google Scholar] [CrossRef]
- Zhang, J.F.; Zhu, Z.H.; Liu, W.; Yuan, X.D.; Qin, S.Q. Towards photodetection with high efficiency and tunable spectral selectivity: Graphene plasmonics for light trapping and absorption engineering. Nanoscale 2015, 32, 13530–13536. [Google Scholar] [CrossRef]
- Guan, H.Y.; Hong, J.Y.; Wang, X.L.; Ming, J.Y.; Zhang, Z.L.; Liang, A.J.; Han, X.Y.; Dong, J.L.; Qiu, W.T.; Chen, Z.; et al. Broadband, high-sensitivity graphene photodetector based on ferroelectric polarization of lithium niobate. Adv. Opt. Mater. 2021, 9, 2100245. [Google Scholar] [CrossRef]
- Ojaghi, S.; Golmohammadi, S.; Soofi, H. All-optical hybrid plasmonic waveguide modulator based on Kerr nonlinearity of graphene. Opt. Eng. 2022, 61, 117102. [Google Scholar] [CrossRef]
- Zhong, C.Y.; Li, J.Y.; Lin, H.T. Graphene-based all-optical modulators. Front. Optoelectron. 2020, 13, 114–128. [Google Scholar] [CrossRef]
- Rahim, A.; Hermans, A.; Wohlfeil, B.; Petousi, D.; Kuyken, B.; Van Thourhout, D.; Baets, R.G. Taking silicon photonics modulators to a higher performance level: State-of-the-art and a review of new technologies. Adv. Photonics 2021, 3, 024003. [Google Scholar] [CrossRef]
- Liu, S.Y.; Wang, M.Z.; Liu, T.Y.; Xu, Y.; Yue, J.B.; Sun, X.Q.; Zhang, D.M. Polarization-insensitive graphene modulator based on hybrid plasmonic waveguide. Photonics 2022, 9, 609. [Google Scholar] [CrossRef]
- Jin, M.; Wei, Z.Y.; Meng, Y.F.; Shu, H.W.; Bai, B.W.; Wang, X.J. Silicon-based graphene electro-optical modulators. Photonics 2022, 9, 82. [Google Scholar] [CrossRef]
- Shi, B.; Cai, W.; Zhang, X.Z.; Xiang, Y.X.; Zhan, Y.; Geng, J.; Ren, M.X.; Xu, J.J. Tunable band-stop filters for graphene plasmons based on periodically modulated graphene. Sci. Rep. 2016, 6, 26796. [Google Scholar] [CrossRef]
- Zhu, Z.H.; Guo, C.C.; Liu, K.; Zhang, J.F.; Ye, W.M.; Yuan, X.D.; Qin, S.Q. Electrically controlling the polarizing direction of a graphene polarizer. J. Appl. Phys. 2014, 116, 104304. [Google Scholar] [CrossRef]
- Maleki, M.; Mehran, M. Analytical investigation and systematic design approach for high-sensitivity guided mode resonance sensors with graphene-enabled tunability. IEEE Sens. J. 2022, 22, 14177–14184. [Google Scholar] [CrossRef]
- Mousavi-Kiasari, S.M.G.; Rashidi, K.; Fathi, D.; Taleb, H.; Mirjalili, S.M.; Faramarzi, V. Computational design of highly-sensitive graphene-based multilayer SPR biosensor. Photonics 2022, 9, 688. [Google Scholar] [CrossRef]
- Nair, R.R.; Blake, P.; Grigorenko, A.N.; Novoselov, K.S.; Booth, T.J.; Stauber, T.; Peres, N.M.R.; Geim, A.K. Fine structure constant defines visual transparency of graphene. Science 2008, 320, 1308. [Google Scholar] [CrossRef]
- Mak, K.F.; Sfeir, M.Y.; Wu, Y.; Lui, C.H.; Misewich, J.A.; Heinz, T.F. Measurement of the optical conductivity of graphene. Phys. Rev. Lett. 2008, 101, 196405. [Google Scholar] [CrossRef]
- Zhou, K.; Cheng, Q.; Song, J.; Lu, L.; Luo, Z.X. Highly efficient narrow-band absorption of a graphene-based Fabry–Perot structure at telecommunication wavelengths. Opt. Lett. 2019, 44, 3430–3433. [Google Scholar] [CrossRef]
- Zhao, B.; Zhao, J.M.; Zhang, Z.M. Enhancement of near-infrared absorption in graphene with metal gratings. Appl. Phys. Lett. 2014, 105, 031905. [Google Scholar] [CrossRef]
- Wu, J. Enhancement of absorption in graphene strips with cascaded grating structures. IEEE Photonics Technol. Lett. 2016, 28, 1041–1135. [Google Scholar] [CrossRef]
- Zhang, H.J.; Zheng, G.G.; Xian, F.L.; Zou, X.J.; Wang, J.C. Near-unity absorption of graphene monolayer with a triple-layer waveguide coupled grating. Opt. Mater. 2017, 72, 476–481. [Google Scholar] [CrossRef]
- Hu, J.H.; Fu, J.; Liu, X.H.; Ren, D.P.; Zhao, J.J.; Huang, Y.H. Perfect absorption in a monolayer graphene at the near-infrared using a compound waveguide grating by robust critical coupling. Chin. Opt. Lett. 2019, 17, 010501. [Google Scholar]
- Hu, J.H.; Huang, Y.Q.; Duan, X.F.; Wang, Q.; Zhang, X.; Wang, J.; Ren, X.M. Enhanced absorption of graphene strips with a multilayer subwavelength grating structure. Appl. Phys. Lett. 2014, 105, 221113. [Google Scholar] [CrossRef]
- Zhang, X.W.; John, S. Broadband light-trapping enhancement of graphene absorptivity. Phys. Rev. B Condens. Matter 2019, 99, 035417. [Google Scholar] [CrossRef]
- Piper, J.R.; Fan, S.H. Total absorption in a graphene monolayer in the optical regime by critical coupling with a photonic crystal guided resonance. ACS Photonics 2014, 1, 345–353. [Google Scholar] [CrossRef]
- Wang, X.; Duan, J.Y.; Chen, W.Y.; Zhou, C.B.; Liu, T.T.; Xiao, S.Y. Controlling light absorption of graphene at critical coupling through magnetic dipole quasi-bound states in the continuum resonance. Phys. Rev. B 2020, 102, 155432. [Google Scholar] [CrossRef]
- Hu, J.H.; Liang, J.F.; Zou, J.; Shi, C.Y.; Zhao, J.J. Dual-band perfect graphene absorber with an all-dielectric zero-contrast grating-based resonant cavity. Opt. Commun. 2023, 527, 128908. [Google Scholar] [CrossRef]
- Jiang, L.Y.; Yuan, C.; Li, Z.Y.; Su, J.; Yi, Z.; Yao, W.T.; Wu, P.H.; Liu, Z.M.; Cheng, S.B.; Pan, M. Multi-band and high-sensitivity perfect absorber based on monolayer graphene metamaterial. Diam. Relat. Mater. 2021, 111, 108227. [Google Scholar] [CrossRef]
- Qing, Y.M.; Ma, H.F.; Cui, T.J. Flexible control of light trapping and localization in a hybrid Tamm plasmonic system. Opt. Lett. 2019, 44, 3302–3305. [Google Scholar] [CrossRef] [PubMed]
- Heo, H.; Lee, S.; Kim, S. Broadband absorption enhancement of monolayer graphene by prism coupling in the visible range. Carbon 2019, 154, 42–47. [Google Scholar] [CrossRef]
- Chen, J.; Zhang, M.X.; Gu, P.; Yan, Z.D.; Tang, C.J.; Lv, B.; Wang, X.X.; Yi, Z.; Zhu, M.W. Broadband, wide-incident-angle, and polarization-insensitive high-efficiency absorption of monolayer graphene with nearly 100% modulation depth at communication wavelength. Results Phys. 2022, 40, 105833. [Google Scholar] [CrossRef]
- Wu, F.; Liu, D.J.; Xiao, S.Y. Bandwidth-tunable near-infrared perfect absorption of graphene in a compound grating waveguide structure supporting quasi-bound states in the continuum. Opt. Express 2021, 29, 41975–41989. [Google Scholar] [CrossRef]
- Xiao, S.Y.; Liu, T.T.; Wang, X.; Liu, X.J.; Zhou, C.B. Tailoring the absorption bandwidth of graphene at critical coupling. Phys. Rev. B 2020, 102, 085410. [Google Scholar] [CrossRef]
- Zhang, S.S.; Liu, H.X.; Zhang, J.; Li, W.J.; Wang, H.; Tian, C.X.; Luo, L.; Zhao, L.J. Dynamically switchable triple-band absorption enhancement of graphene by a subwavelength grating coupled hybrid structure. Results Phys. 2022, 43, 106057. [Google Scholar] [CrossRef]
- Qing, Y.M.; Ma, H.F.; Ren, Y.Z.; Cui, T.J. Near-infrared absorption-induced switching effect via guided mode resonances in a graphene-based metamaterial. Opt. Express 2019, 27, 5253–5263. [Google Scholar] [CrossRef]
- Hanson, G.W. Dyadic Green’s functions and guided surface waves for a surface conductivity model of graphene. J. Appl. Phys. 2008, 103, 064302. [Google Scholar] [CrossRef]
- Lu, H.; Gan, X.T.; Mao, D.; Zhao, J.L. Graphene-supported manipulation of surface plasmon polaritons in metallic nanowaveguides. Photonics Res. 2017, 5, 162–167. [Google Scholar] [CrossRef]
- Thongrattanasiri, S.; Koppens, F.H.L.; De Abajo, F.J.G. Complete optical absorption in periodically patterned graphene. Phys. Rev. Lett. 2012, 108, 047401. [Google Scholar] [CrossRef]
- Bian, L.A.; Yang, L.; Liu, P.G.; Chen, Y.W.; Liu, H.Q.; Zhou, Q.H. Controllable perfect absorption in a double-cavity photonic crystal with one graphene monolayer. J. Phys. D Appl. Phys. 2017, 51, 025106. [Google Scholar] [CrossRef]
- Fan, S.H.; Suh, W.; Joannopoulos, J.D. Temporal coupled-mode theory for the Fano resonance in optical resonators. J. Opt. Soc. Am. A 2003, 20, 569–572. [Google Scholar] [CrossRef] [PubMed]
- Yoon, J.; Seol, K.H.; Song, S.H.; Magnusson, R. Critical coupling in dissipative surface-plasmon resonators with multiple ports. Opt. Express 2010, 18, 25702–25711. [Google Scholar] [CrossRef] [PubMed]
- Qing, Y.M.; Ma, H.F.; Cui, T.J. Tailoring anisotropic perfect absorption in monolayer black phosphorus by critical coupling at terahertz frequencies. Opt. Express 2018, 26, 32442–32450. [Google Scholar] [CrossRef] [PubMed]
- Vyas, H.; Hegde, R.S. Improved refractive-index sensing performance in medium contrast gratings by asymmetry engineering. Opt. Mater. Express 2020, 10, 1616–1629. [Google Scholar] [CrossRef]
- Huang, L.J.; Xu, L.; Rahmani, M.; Neshev, D.; Miroshnichenko, A.E. Pushing the limit of high-Q mode of a single dielectric nanocavity. Adv. Photonics 2021, 3, 016004. [Google Scholar] [CrossRef]
- Wang, S.S.; Magnusson, R. Theory and applications of guided-mode resonance filters. Appl. Opt. 1993, 32, 2606–2613. [Google Scholar] [CrossRef]
- Xiao, S.Y.; Qin, M.B.; Duan, J.Y.; Wu, F.; Liu, T.T. Polarization-controlled dynamically switchable high-harmonic generation from all-dielectric metasurfaces governed by dual bound states in the continuum. Phys. Rev. B 2022, 105, 195440. [Google Scholar] [CrossRef]
- Vakil, A.; Engheta, N. Transformation optics using graphene. Science 2011, 332, 1291–1294. [Google Scholar] [CrossRef]
- Yan, Z.D.; Kong, L.C.; Tang, C.J.; Deng, J.; Gu, P.; Chen, J.; Wang, X.X.; Yi, Z.; Zhu, M.W. Ultra-broadband and completely modulated absorption enhancement of monolayer graphene in a near-infrared region. Opt. Express 2022, 30, 34787–34796. [Google Scholar] [CrossRef]
- Zhang, X.G.; Sun, Y.L.; Zhu, B.C.; Jiang, W.X.; Zhang, Z.C.; Cui, T.J. Light-controllable time-domain digital coding metasurfaces. Adv. Photonics 2022, 4, 025001. [Google Scholar] [CrossRef]
| ds (nm) | N | Nreal/(2 × Nimag) |
|---|---|---|
| 50 | 1.21441 × 1015 − 2.3160 × 1011i | 2621.8 |
| 100 | 1.21473 × 1015 − 1.1039 × 1011i | 5502.2 |
| 150 | 1.21479 × 1015 − 4.9016 × 1010i | 12,393 |
| 200 | 1.21479 × 1015 − 2.0509 × 1010i | 29,616 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liang, J.; Hu, J.; Liu, X.; Zhao, J. Near-Perfect Narrow-Band Tunable Graphene Absorber with a Dual-Layer Asymmetric Meta-Grating. Photonics 2023, 10, 14. https://doi.org/10.3390/photonics10010014
Liang J, Hu J, Liu X, Zhao J. Near-Perfect Narrow-Band Tunable Graphene Absorber with a Dual-Layer Asymmetric Meta-Grating. Photonics. 2023; 10(1):14. https://doi.org/10.3390/photonics10010014
Chicago/Turabian StyleLiang, Junfang, Jinhua Hu, Xiuhong Liu, and Jijun Zhao. 2023. "Near-Perfect Narrow-Band Tunable Graphene Absorber with a Dual-Layer Asymmetric Meta-Grating" Photonics 10, no. 1: 14. https://doi.org/10.3390/photonics10010014
APA StyleLiang, J., Hu, J., Liu, X., & Zhao, J. (2023). Near-Perfect Narrow-Band Tunable Graphene Absorber with a Dual-Layer Asymmetric Meta-Grating. Photonics, 10(1), 14. https://doi.org/10.3390/photonics10010014





