Grating Configurations for the Spectral Selection of Coherent Ultrashort Pulses in the Extreme-Ultraviolet

Abstract

The design and realization of grating instruments to handle and condition coherent ultrafast pulses in the extreme ultraviolet spectral region are discussed. The main application of such instruments is the spectral selection of high-order laser harmonics and free-electron-laser pulses in the femtosecond time scale. Broad-band monochromators require the use of diffraction gratings at grazing incidence. Here, we discuss two configurations useful for the realization of grating monochromator with ultrafast response: the single-grating design, applied to high-order laser harmonics, and the time-delay-compensated configuration with two gratings, applied to free-electron lasers.

1. Introduction

The developments in laser technology over the last thirty years lead to the generation of pulses as short as few femtoseconds, providing a unique tool for high-resolution time-domain spectroscopy that has revolutionized many areas of science [1]. While femtosecond optical lasers have offered unique insights into ultra-fast dynamics, they are limited by the fact that the structural arrangement and motion of nuclei are not directly accessible from measured optical properties. This scientific gap has been filled by the availability of femtosecond sources in the extreme-ultraviolet (XUV) spectral region, such as high-order laser harmonics (HHs) and free-electron-lasers (FELs). HHs are generated when a very intense ultrashort pulsed laser is focused on a gas jet/cell [2]. They have high brightness and degree of coher­ence. The radiation generated with the scheme of the HHs using few-optical-cycles laser pulses is currently the main tool for the investigation of matter with attosecond resolution [3,4]. FEL sources generate spatially coherent UV/X-ray radiation with characteristics similar to the light from optical lasers, ultrashort time duration and an increase of six to eight orders of magnitude on the peak brilliance with respect to third-generation synchrotrons [5,6,7,8].

The HH spectrum is generally described as a sequence of peaks corresponding to the odd harmonics of the fundamental laser frequency with an intensity distribution characterized by a plateau of which extension is related to the wavelength of the driving laser field and the intensity. As many multiple orders of high harmonics are generated coaxially along with the intense fundamental laser pulses, the application to time-resolved and nonlinear spectroscopy may give some difficulties since the target signals probed by an appropriate harmonic order are often covered with the noises caused by the other harmonic orders [9], therefore, a suitable monochromatizing system may be required to select a single harmonics. Furthermore, monochromatization may be important for imaging applications as a high spectral purity is necessary to achieve a high resolution in imaging [10]. Similarly, some of the existing FEL beamlines have monochromators either to increase the spectral purity of the source or to select the harmonics of the FEL emission (typically the third or fifth) and filter out the fundamental [11,12,13]. The monochromator demanded for the spectral selection of ultrashort pulses has to ideally preserve the temporal duration as short as in the generation process.

The simplest way to obtain the spectral selection of ultrashort pulses is the use of a multilayer mirror which does not alter the pulse time duration up to fractions of femtosecond, is very efficient and can be used at normal incidence with very low aberrations and tight focusing [14,15]. However, the main drawback of the use of multilayer optics is the lack of tunability, indeed, different multilayer-coated mirrors have to be adopted to tune the monochromator in a broad spectral band [16].

Tuneable XUV monochromators are usually realized by reflection gratings at grazing incidence. In this case, the spectral band of operation is intrinsically large because of the grazing-incidence operation. However, a grating introduces a stretch of the pulse duration by the pulse-front tilt, compromising the advantages of ultrashort pulses. Two are the options in designing an ultrafast monochromator, namely the single-grating design [17] or the double-grating time-delay compensated one [18,19]. In the first case, being adopted a single grating, a residual pulse-front tilt has to be accepted at the output of the monochromator. Aim of the design is to find a suitable grating geometry that minimizes the temporal broadening. In the second case, the design consists of a pair of gratings to compensate for the pulse-front tilt. The first grating is demanded to perform the spectral selection on an intermediate slit, the second grating compensates for the pulse-front tilt of the diffracted beam, giving a temporal resolution much higher than the single-grating design. The choice between the two options has to be performed as a trade-off between efficiency, that are maximized in the single-grating design, and temporal resolution, that is maximized in the two-grating design.

In this paper, we discuss the use of both configurations for ultrashort HHs or FEL pulses. In particular, the application of the single-grating configuration adopting the off-plane mount to HH selection will be discussed, with particular attention to design parameters and temporal performance. The double-grating configuration will be also discussed, with a possible application to FEL radiation.

2. Single-Grating Monochromators for Ultrashort Pulses

Single-grating monochromators for ultrafast pulses aim to perform the spectral selection in the simplest configuration, using a grating as the dispersive element and tolerating a residual pulse-front tilt at the output given by the diffraction. In fact, each ray that is diffracted by two adjacent grooves is delayed by mλ/c, where m is the diffraction order, λ is the wavelength and c is the speed of light in vacuum. The pulse-front tilt is given by the total difference in the optical paths of the diffracted beam, that is Δτ_G = mλN/c, where N is the number of the illuminated grooves.

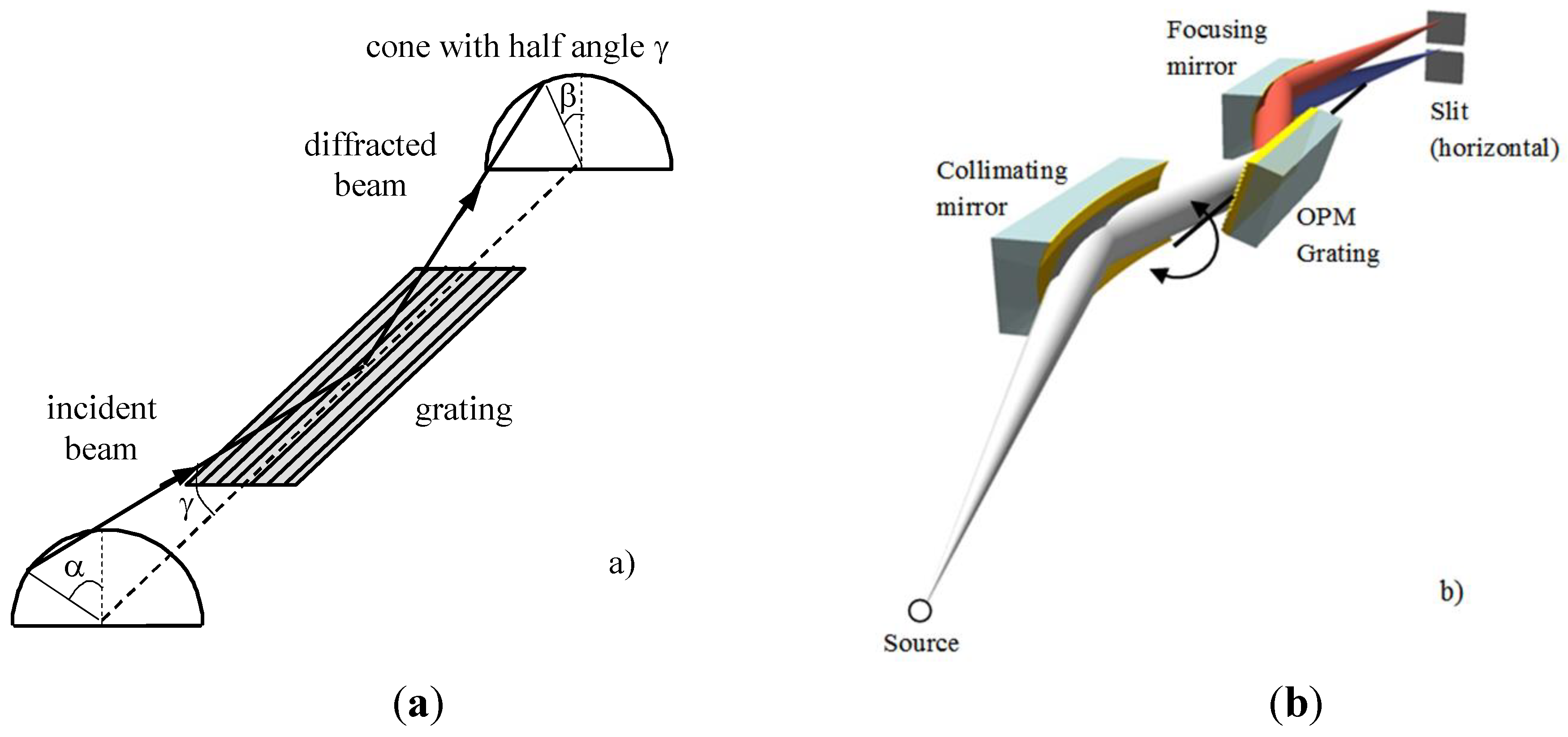
Grazing-incidence diffraction gratings may be used in two different geometries: the classical-diffraction mount (CDM) and the off-plane mount (OPM). The latter differs from the classical one in that the incident and diffracted wave vectors are almost parallel to the grating grooves. The geometry is shown in Figure 1a. The direction of the incoming rays is described by two parameters; the altitude and the azimuth. The altitude γ is the angle between the direction of the incoming rays and the direction of the grooves. It defines the half-angle of the cone into which the light is diffracted: all the rays leave the grating at the same altitude angle at which they approach. The azimuth α of the incoming rays is defined to be zero if they lie in the plane perpendicular to the grating surface and parallel to the rulings; thus; −α is the azimuth of the zero order light. Let β define the azimuth of the diffracted light at wavelength λ and order m. In the case of a monochromator; the grating is operated in the condition α = β and the grating equation is written as:

$$\alpha =\text{arcsin}\left[\frac{m\lambda \sigma }{2\text{sin}\gamma }\right]$$

(1)

where σ is the groove density.

Figure 1. (a) The OPM grating geometry. (b) The OPM monochromator: the polychromatic light at the input is dispersed in the vertical direction and the spectral selection is performed through the horizontal slit. The blue beam is the radiation at the wavelength λ that has been selected to be transmitted through the slit by choosing the proper grating rotation, the red beam is the radiation at another generic wavelength that is stopped by the slit.

Figure 1. (a) The OPM grating geometry. (b) The OPM monochromator: the polychromatic light at the input is dispersed in the vertical direction and the spectral selection is performed through the horizontal slit. The blue beam is the radiation at the wavelength λ that has been selected to be transmitted through the slit by choosing the proper grating rotation, the red beam is the radiation at another generic wavelength that is stopped by the slit.

Gratings in the OPM are operated in parallel light, therefore, a monochromator has three optical elements, two concave mirrors and a plane grating, as shown in Figure 1b. The first mirror acts as the collimator, the second mirror as the condenser. The magnification is unity to minimize the aberrations, i.e., p = q, where p is the input arm of the collimator and q the output arm of the condenser. The wavelength scanning is performed by rotating the grating around an axis tangent to its vertex and parallel to the grooves. In such a way, the altitude angle γ is kept constant while the azimuth α is varied with the wavelength following Equation (1).

The blaze condition of maximum efficiency is when the light leaves the grating in such a way that it performs a specular reflection on the groove surface, that is α + β = 2γ, where γ is the grating blaze angle. In addition, shadowing effects from adjacent grooves must be avoided, that is α = β. It follows that the highest efficiency of a blaze grating in the OPM is achieved when α = β = γ. It has been theoretically shown and experimentally measured that the efficiency in the off-plane mount is close to the reflectivity of the coating, thus, much higher efficiencies than in the classical diffraction mount can be obtained [20]. In particular, it has been shown that the XUV efficiency may be as high as 40% and 15%, respectively, for the single- and double-grating setup.

In case of low-resolution monochromators, having α ≤ 15°, the number of illuminated grooves can be approximated as N = S σ, where S is the grating illuminated area in the direction perpendicular to the grooves. The corresponding pulse-front tilt is ΔτG = S σ mλ/c.

By comparing the OPM to the CDM applied to ultrafast pulses in the XUV, it has been demonstrated in [21] that for pulse-front tilts above few hundreds of femtoseconds, the CDM requires groove densities of few-to-several hundreds of grooves per millimeter and blaze angles that are feasible with present technologies, while the OPM requires higher groove densities and azimuth angles, therefore introducing a large distortion and rotation of the image after the diffraction [20]. For pulse-front tilts below 100 fs, the groove densities required for CDM are extremely low and the corresponding blaze angles are practically unfeasible. On the contrary, the blazed gratings used in the OPM have parameters within the manufacturing capabilities even for few-tens of femtoseconds time responses. Single-grating monochromators using gratings in the OPM have been recently realized and are being used in HH beamlines, where monochromatic pulses as short as 30 fs have already been measured [22,23,24,25].

As a test case, the design of a monochromator to be used for the spectral selection of HHs that has been developed in the framework of a collaboration between CNR-IFN Padova (Italy) and the Laboratory of Ultrafast Spectroscopy of the École Politechnique Fédérale de Lausanne (LSU-EPFL, Switzerland) to be used for time-resolved photoelectron spectroscopy from liquid samples [26] is reported here. The instrumental parameters are summarized in Table 1. The monochromator is operated without the entrance slit, the source being directly the HH generation point. The central section has five gratings which are mounted on a motorized linear stage and can be selected by the user for different resolutions and blazed wavelengths. All the optical elements are gold coated. A schematic is shown in Figure 2. The output bandwidth in the OPM is almost constant: Δλ_FWHM = ΔS (σ q)⁻¹, where ΔS is the width of the slit. The values for a 50-μm-wide slit are reported in Table 1.

Table 1. Parameters of the OPM monochromator developed for LSU-EPFL HH beamline.

Spectral region	30-100 eV (41–12 nm)
Gratings	Altitude	3.5°
G1	Energy region	80-100 eV (15.5–12 nm)
	Groove density	600 gr/mm
	Bandwidth (100-um slit)	Δλ = 0.26 nm, ΔE = 1.7 eV@90 eV
G2	Energy region	80-100 eV (15.5–12 nm)
	Groove density	1200 gr/mm
	Bandwidth (100-um slit)	Δλ = 0.13 nm, ΔE = 0.85 eV @90 eV
G3	Energy region	80-100 eV (15.5–12 nm)
	Groove density	2400 gr/mm
	Bandwidth (50-um slit)	Δλ = 0.06 nm, ΔE = 0.42 eV @90 eV
G4	Energy region	30-40 eV (41–31 nm)
	Groove density	200 gr/mm
	Bandwidth (50-um slit)	Δλ = 0.77 nm, ΔE = 0.8 eV @35 eV
G5	Energy region	30-40 eV (41–31 nm)
	Groove density	900 gr/mm
	Bandwidth (50-um slit)	Δλ = 0.17 nm, ΔE = 0.17 eV @35 eV

Table 1. Parameters of the OPM monochromator developed for LSU-EPFL HH beamline.

Spectral region	30-100 eV (41–12 nm)
Gratings	Altitude	3.5°
G1	Energy region	80-100 eV (15.5–12 nm)
	Groove density	600 gr/mm
	Bandwidth (100-um slit)	Δλ = 0.26 nm, ΔE = 1.7 eV@90 eV
G2	Energy region	80-100 eV (15.5–12 nm)
	Groove density	1200 gr/mm
	Bandwidth (100-um slit)	Δλ = 0.13 nm, ΔE = 0.85 eV @90 eV
G3	Energy region	80-100 eV (15.5–12 nm)
	Groove density	2400 gr/mm
	Bandwidth (50-um slit)	Δλ = 0.06 nm, ΔE = 0.42 eV @90 eV
G4	Energy region	30-40 eV (41–31 nm)
	Groove density	200 gr/mm
	Bandwidth (50-um slit)	Δλ = 0.77 nm, ΔE = 0.8 eV @35 eV
G5	Energy region	30-40 eV (41–31 nm)
	Groove density	900 gr/mm
	Bandwidth (50-um slit)	Δλ = 0.17 nm, ΔE = 0.17 eV @35 eV

Figure 2. Schematic of the OPM monochromator.

Figure 2. Schematic of the OPM monochromator.

The calculated pulse front-tilt at the output of the monochromator is reported in Table 2 in case of a 4 mrad × 4 mrad XUV beam full aperture. It has been calculated as the half-width spread of the optical paths at the output slit, being 30−35 fs for the two low-resolution gratings and 140 fs for the medium-resolution ones. Clearly, the time response depends on the actual divergence of the beam, since it defines the area illuminated on the grating in the direction perpendicular to the grooves and correspondingly the number of grooves that are involved in the diffraction process. In particular, the response increases linearly with the beam divergence in the vertical direction, that is the direction perpendicular to the grooves. A diaphragm can be placed just in front of the first mirror to limit the vertical aperture: this reduces the front-tilt but obviously decreases also the photon flux for HH beams with large angular apertures. The size of such a diaphragm is few-to-several millimeters, therefore, diffraction effects are almost negligible. If the aperture is reduced to 2 mrad in the vertical direction, the corresponding front-tilt is reduced by a factor two with respect to the previous case, assuming values shorter than 20 fs and 70 fs, respectively, for the low-resolution and medium-resolution gratings.

Table 2. Time response of the OPM monochromator for a beam aperture of 4 mrad × 4 mrad and 4 mrad × 2 mrad, calculated as the half-width spread of the optical paths at the output slit.

	4 mrad × 4 mrad Full Aperture	4 mrad × 2 mrad Full Aperture
G1, 600 gr/mm	35 fs at 90 eV	18 fs at 90 eV
G2, 1200 gr/mm	70 fs at 90 eV	35 fs at 90 eV
G3, 2400 gr/mm	140 fs at 90 eV	70 fs at 90 eV
G4, 200 gr/mm	30 fs at 35 eV	15 fs at 35 eV
G5, 900 gr/mm	140 fs at 35 eV	70 fs at 35 eV

Table 2. Time response of the OPM monochromator for a beam aperture of 4 mrad × 4 mrad and 4 mrad × 2 mrad, calculated as the half-width spread of the optical paths at the output slit.

	4 mrad × 4 mrad Full Aperture	4 mrad × 2 mrad Full Aperture
G1, 600 gr/mm	35 fs at 90 eV	18 fs at 90 eV
G2, 1200 gr/mm	70 fs at 90 eV	35 fs at 90 eV
G3, 2400 gr/mm	140 fs at 90 eV	70 fs at 90 eV
G4, 200 gr/mm	30 fs at 35 eV	15 fs at 35 eV
G5, 900 gr/mm	140 fs at 35 eV	70 fs at 35 eV

XUV radiation in the wavelength range 12–73 nm (100–17 eV) is produced by HH generation using 45-fs Ti:Sa laser pulses focused on the 5-mm gas cell by a 40-cm lens. The IR co-propagates with the HHs up to the grating. When the grating is rotated to perform the wavelength scanning, the IR beam is totally diffracted on the zero-order and is blocked by a suitable beam stop placed just before the output toroidal mirror, where the minimum separation in the vertical direction between the zero order and the harmonics is 10 mm. Being that the beam angular aperture is limited to 4 mrad, the size of the beams is about 2.5 mm, making it very effective to stop the IR. The monochromatized XUV flux is measured after the exit slit with an absolutely calibrated metallic photodiode. 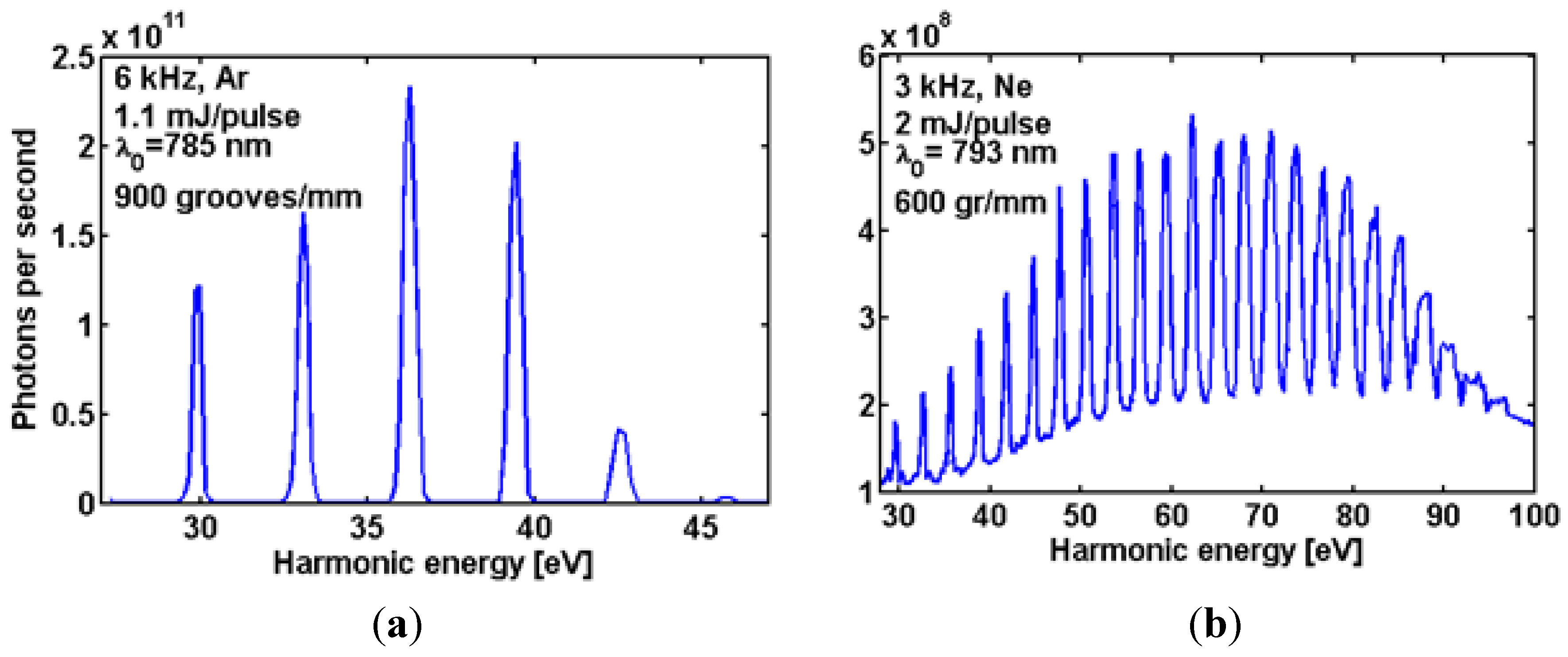Figure 3 shows two typical scanned HH spectra with the exit slit closed to 100 µm. A flux as high as 2.5 × 10¹¹ photons/s for H23 (35.6 eV) in argon and 5 × 10⁸ photons/s in the H33-H49 region (35.6−80 eV) in neon has been measured at the output. Only odd harmonics are generated, therefore, the radiation diffracted by the grating at second order is not overlapped to any of the harmonics. No evidence of second orders contribution is visible in the spectra. Note that the monochromator, although designed to operate in two relatively narrow spectral regions that are tailored to the experimental requirements, may be tuned-in a much broader region.

The experimental data here presented are indicative of the flexibility of the design in terms of choice of spectral region, energy resolution and time resolution and confirm the advantage of the OPM geometry for ultrafast responses in a simple optical set-up.

Figure 3. HH spectra scanned with the monochromator in argon (a) and neon (b).

Figure 3. HH spectra scanned with the monochromator in argon (a) and neon (b).

3. Double-Grating Monochromators for Ultrashort Pulses

The single-grating design, although simple and attractive, may not be used in case of high resolution, as the grating will give an unacceptable pulse front-tilt due to the high number of grooves involved in the diffraction process. To overcome this limit, double-grating designs have been proposed and realized by using two gratings in a time-delay compensated configuration, where the second grating compensates for the time and spectral spread introduced by the first one [27,28,29,30,31]. Pulses as short as 8 fs have been measured at the output of a double-grating monochromator at 35 nm, i.e., H23 of Ti:Sa laser (see Reference [28]). The main drawback of these configurations is the use of two gratings that increase the complexity and reduce the efficiency, although this may be the only solution to be adopted when high resolution and simultaneously negligible pulse front-tilt are requested in the XUV.

As a design example, we discuss here the application of the double-grating design to FEL radiation. At present, there are two FEL beamlines operated with a monochromator, one at FLASH (see References [11,12]) and one at LCLS (see Reference [13]). They adopt the single-grating design, since it is the simplest one and is already used in many synchrotrons. In case of the monochromatic beamline at LCLS, that is, being operated in the 500−2000 eV range, the estimated pulse stretch is 30 fs, which is well below the LCLS pulse duration. In the case of the monochromatic beamline at FLASH, that is being operated at lower energies in the 20–200 eV range, the stretch introduced by the grating is definitely longer than the FLASH pulse duration, being in the picosecond time scale.

The upcoming FLASH II is the major extension of the FLASH facility in Hamburg [32]. This substantial upgrade will lead to a new experimental hall and many new beamlines operated in parallel with FLASH. Since FLASH II is operated in the same spectral region as FLASH, a single-grating monochromator will give at the output a duration that is similar to that obtained now in the monochromatic beamline at FLASH. A monochromator, with simultaneously high resolution and negligible pulse front tilt, would be highly desirable for the users of FLASH II.

Here we present the design of a double-grating monochromator tunable in the whole FLASH II range (6–60 nm) with spectral resolution E/ΔE in the 1000–2000 interval and pulse front-tilt lower than 10 fs. The design originates from the variable-line-spaced (VLS) grating monochromator, whic has been proposed by Hettrick [33] and already adopted for synchrotron radiation beamlines [34], high-order laser harmonics [35,36] and FEL radiation (see Reference [13]). A concave mirror produces a converging beam toward a VLS plane grating that diffracts the radiation onto the exit slit. The variable groove spacing of the grating provides the free parameters to give an almost aberration-free image at a constant focal distance as a function of the photon energy. The VLS monochromator is also simple mechanically in that only two optical elements are required and the photon energy is scanned by a single rotation of the grating around an axis passing through its center.

The design has been adapted for XUV ultrafast response by adding a second section with an identical VLS plane grating illuminated by the diverging light coming out from the slit and mounted in a compensated geometry, i.e., the incidence angle of the second grating is equal to the diffraction angle of the first one. Furthermore, the design has been tailored to the requirements of FLASH II: (a) due to the high stability of the FEL beam, the monochromator works without an entrance slit, i.e., the FEL itself acts as the source point (as the case of LCLS); (b) a plane mirror has been inserted between the two gratings, in order to fold the beam and reduce the lateral displacement of the beam; (c) due to the high photon flux, horizontal and vertical foci have to be kept separated to reduce the radiation density on the slit blades.

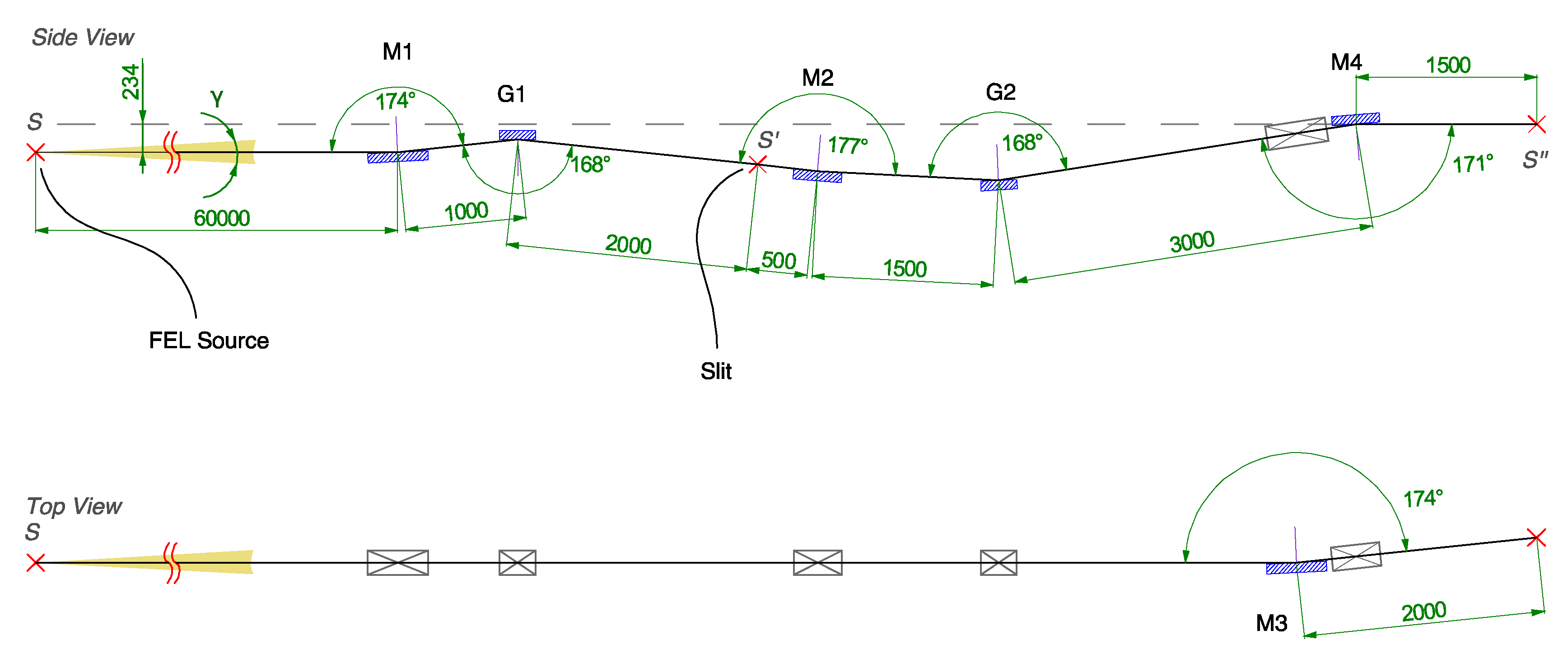
The optical layout is shown in Figure 4. The FEL beam is focused by the plane-elliptical mirror M1 toward the plane VLS grating G1. The beam is monochromatized on the intermediate slit, being focused only in the spectral dispersion plane. The plane mirror M2 is used to fold the beam. The grating G2 has the same groove-space-variation parameters as G1 and compensates for the pulse-front tilt given by G1. The diverging radiation coming out from G2 is finally focused to the sample area by two plane-elliptical mirrors in Kirkpatrick-Baez configuration.

The optical parameters are resumed in Table 3. Two sets of gratings, with, respectively, 600 mm⁻¹ and 200 mm⁻¹ central groove density, are used to cover the 6−60 nm spectral region. G1 is operated in the internal spectrum, i.e., β_G1 < α_G1, resulting in α_G1 > 85°, that is safe under the intense FEL beam. G2 is operated in the external spectrum to compensate for the pulse-front tilt, i.e., β_G2 = α_G1> α_G2 = β_G1.

The FLASH II source has been assumed to have 200-μm FWHM size and 75-μrad FWHM divergence at 40 nm, scaling as λ^3/4 (see Reference [32]). The resolution, as calculated from ray-tracing simulations, is shown in Figure 5a for a 50-um slit. The pulse-front tilt is shown in Figure 5b both at the slit plane and at the output. The double-grating configuration is really effective, being able to compensate for the front-tilt from the picosecond time scale down to few femtoseconds. Indeed, the temporal resolution of the beamline is increased by almost three orders of magnitudes by using the double-grating design. Finally, the spot size at the output has been simulated to be in the 10–15 um FWHM range in the whole interval of operation.

Figure 4. Optical layout of a double-grating monochromator for FEL radiation.

Figure 4. Optical layout of a double-grating monochromator for FEL radiation.

Since the expected efficiency of a single grating is in the 0.2−0.4 range, as already measured in other FEL beamlines (e.g., see Reference [13]), the expected transmission of such a monochromator is lower than a factor 2.5-to-5 with respect to the single-grating design. Let us suppose that the spot size is the same in both designs. Since the pulse duration at the output is reduced by at least two orders of magnitudes with respect to the single-grating monochromator, the peak intensity at the sample is increased by more than a factor 10.

The main characteristics of the proposed configuration are summarized as: (1) it minimizes the number of optical elements, just one grating and one plane mirror are added with respect to a standard VLS monochromator beamline; (2) it gives very low displacement of the output beam with respect to the input; (3) it guarantees high focusing properties in the whole spectral range of operation; (4) it requires simple mechanical movements. This confirms the advantage of the double-grating design when applied to FEL radiation.

Table 3. Parameters of the beamline for FLASH II.

Distances
S–M1	60 m
M1–G1	1 m
G1–Slit	2 m
Slit-M2	0.5 m
M2–G2	1.5 m
G2–M3	2.5 m
M3–M4	0.5 m
M4–focus	1.5 m
Mirror M1	Plane / Elliptical
Entrance arm	60 m
Exit arm	3 m
Incidence angle	87°
Mirror M2	Plane
Incidence angle	88.5°
Mirror M3	Plane / Elliptical
Entrance arm	67.5 m
Exit arm	2 m
Incidence angle	87°
Mirror M4	Plane / Elliptical
Entrance arm	5 m
Exit arm	1.5 m
Incidence angle	85.5°
Gratings
Type	VLS plane
Deviation Angle	168°
Grating set 1	6–20 nm, 600 mm−1
Grating set 2	18–60 nm, 200 mm−1

Table 3. Parameters of the beamline for FLASH II.

Distances
S–M1	60 m
M1–G1	1 m
G1–Slit	2 m
Slit-M2	0.5 m
M2–G2	1.5 m
G2–M3	2.5 m
M3–M4	0.5 m
M4–focus	1.5 m
Mirror M1	Plane / Elliptical
Entrance arm	60 m
Exit arm	3 m
Incidence angle	87°
Mirror M2	Plane
Incidence angle	88.5°
Mirror M3	Plane / Elliptical
Entrance arm	67.5 m
Exit arm	2 m
Incidence angle	87°
Mirror M4	Plane / Elliptical
Entrance arm	5 m
Exit arm	1.5 m
Incidence angle	85.5°
Gratings
Type	VLS plane
Deviation Angle	168°
Grating set 1	6–20 nm, 600 mm−1
Grating set 2	18–60 nm, 200 mm−1

Figure 5. Resolution on a 50-μm slit (a) and pulse front-tilt (b) of the double-grating monochromator for FLASH II. The two scales on the x-axis refer to the two grating sets. The values in the y-axis are the same for both sets of gratings.

Figure 5. Resolution on a 50-μm slit (a) and pulse front-tilt (b) of the double-grating monochromator for FLASH II. The two scales on the x-axis refer to the two grating sets. The values in the y-axis are the same for both sets of gratings.

4. Conclusions

We have discussed the applications of grating monochromators to ultrafast pulses in the XUV for applications to HHs and FELs. Both single- and double-grating configurations have been discussed. When compared to other schemes that are used to obtain a monochromatized XUV beam, such as the combination of multilayer mirrors and thin metal foils, the use of grating monochromators gives higher spectral purity of the radiation and tunability in a broad region, furthermore thin metal foils are not needed to stop the fundamental IR laser beam in case of HHs.