Nonlinear Finite Element Model for FGM Porous Circular and Annular Micro-Plates Under Thermal and Mechanical Loads Using Modified Couple Stress-Based Third-Order Plate Theory
Abstract
1. Introduction
2. Model Development
2.1. Displacement Field and Strain Definition
2.2. Hamilton’s Principle and Equations of Motion
2.3. Constitutive Relations
2.4. Finite Element Model
2.5. Computational Considerations
2.6. Steady State Heat Conduction
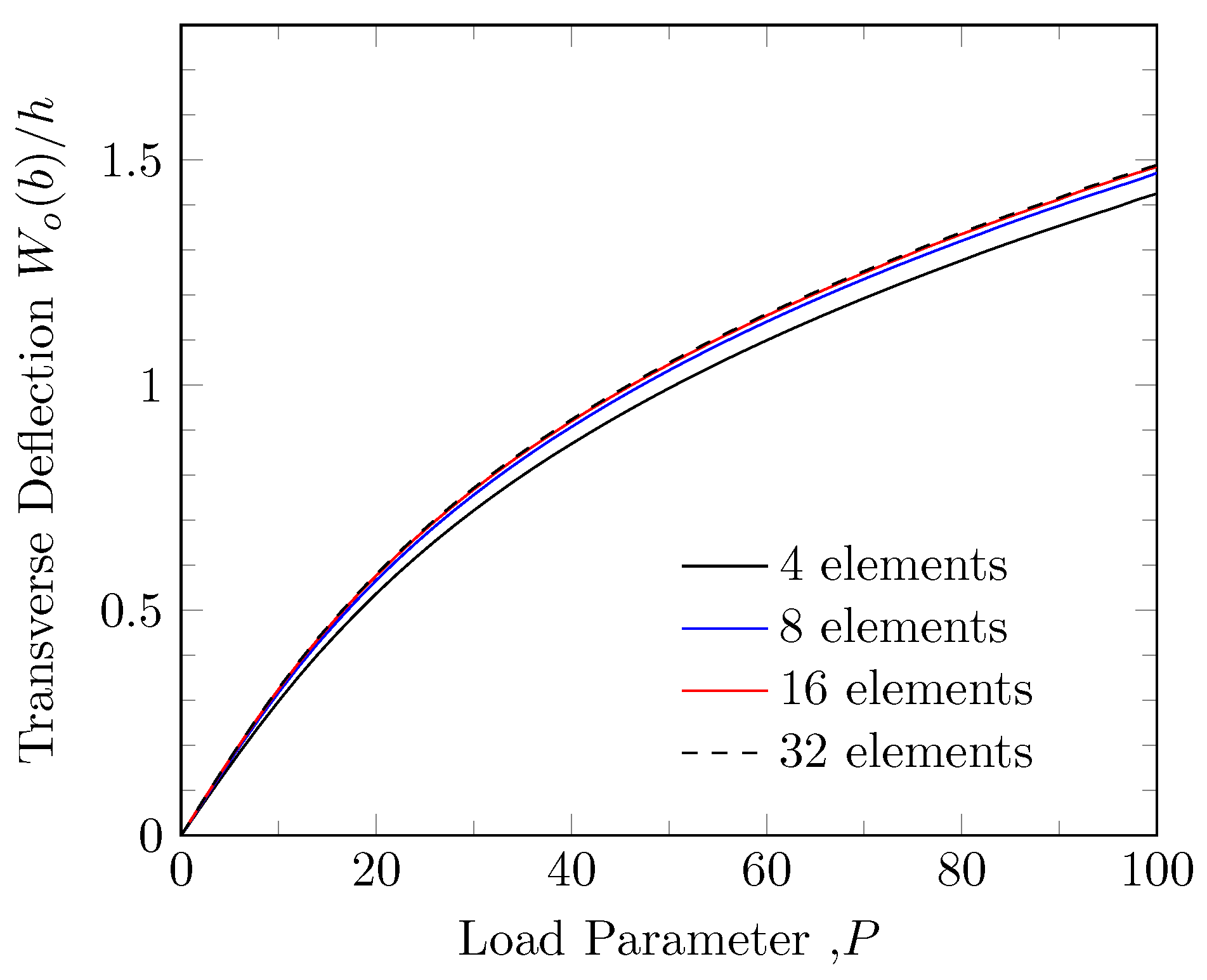
3. Model Validation
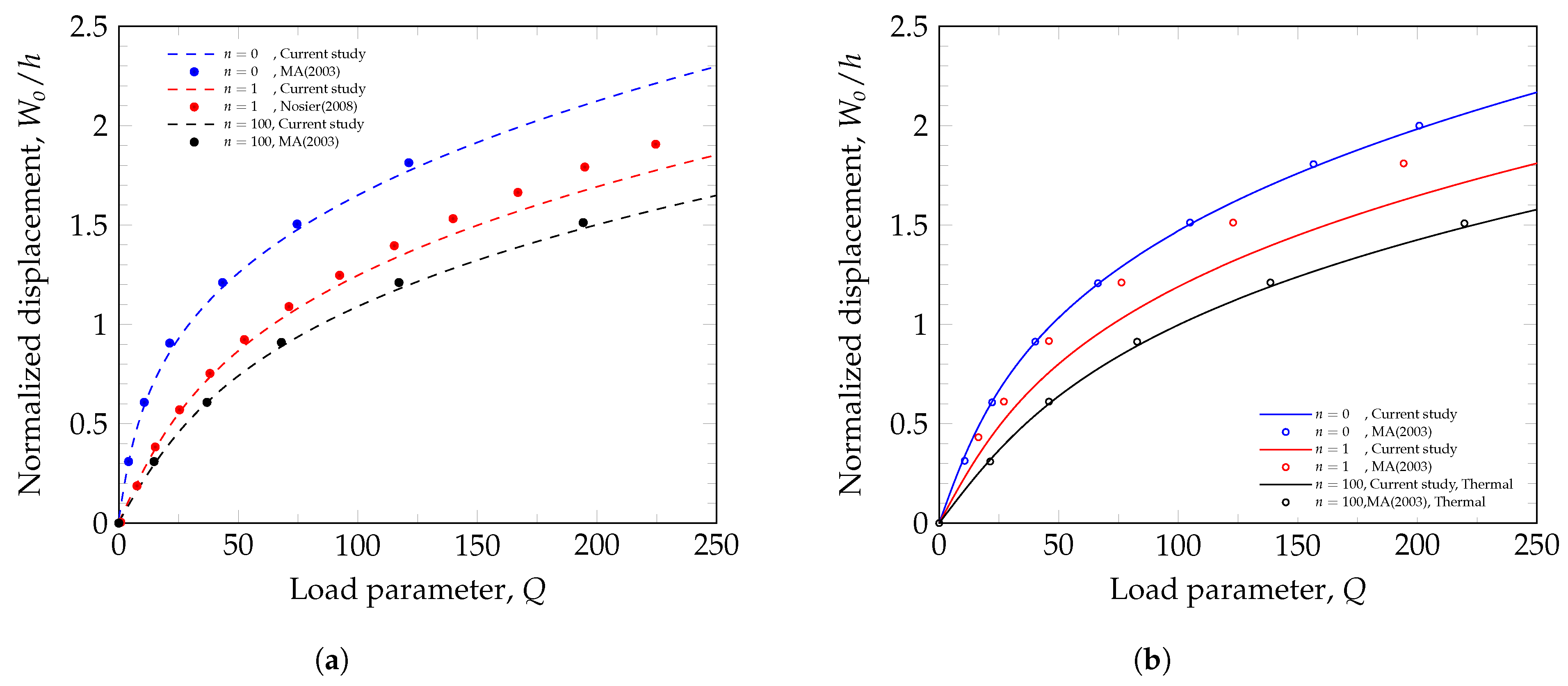
3.1. Static Load
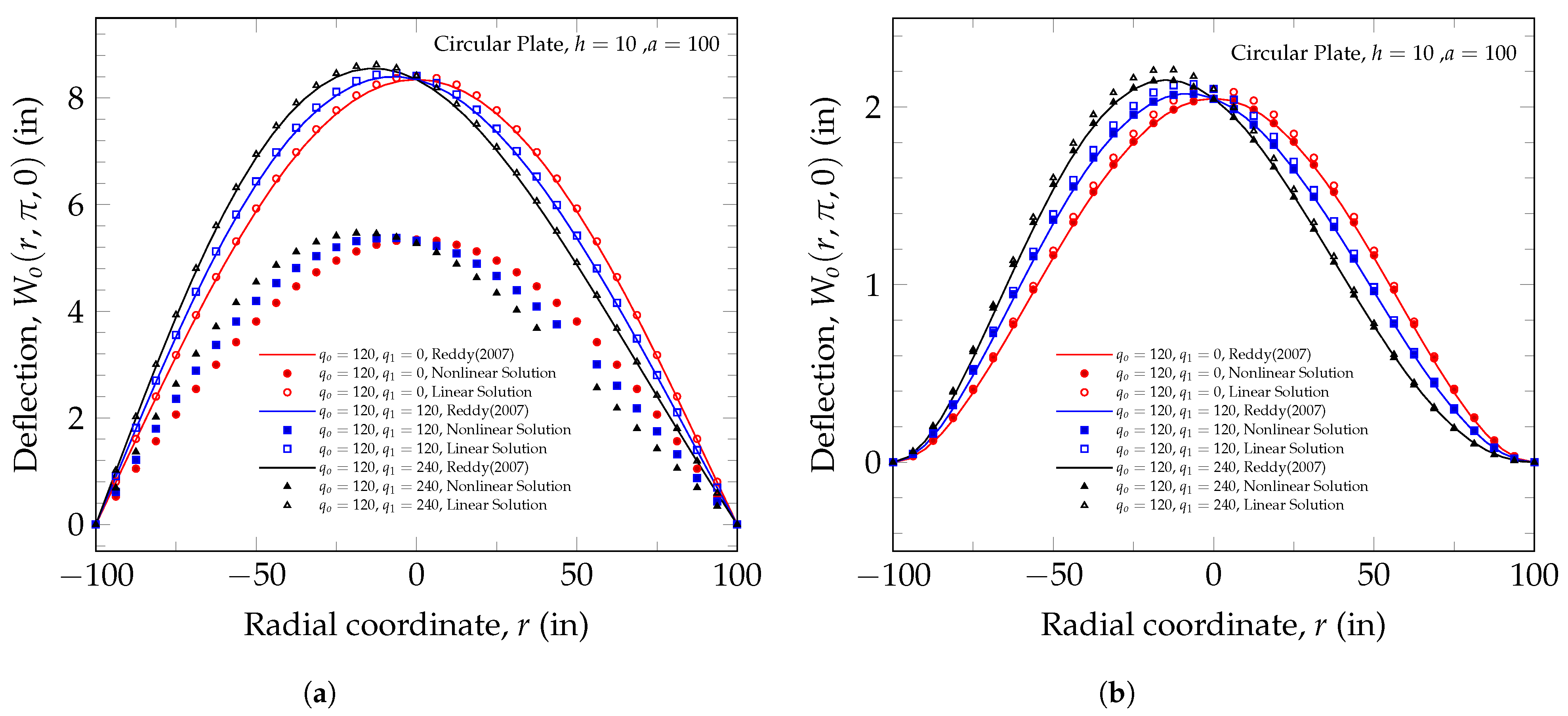
3.2. Asymmetric Load
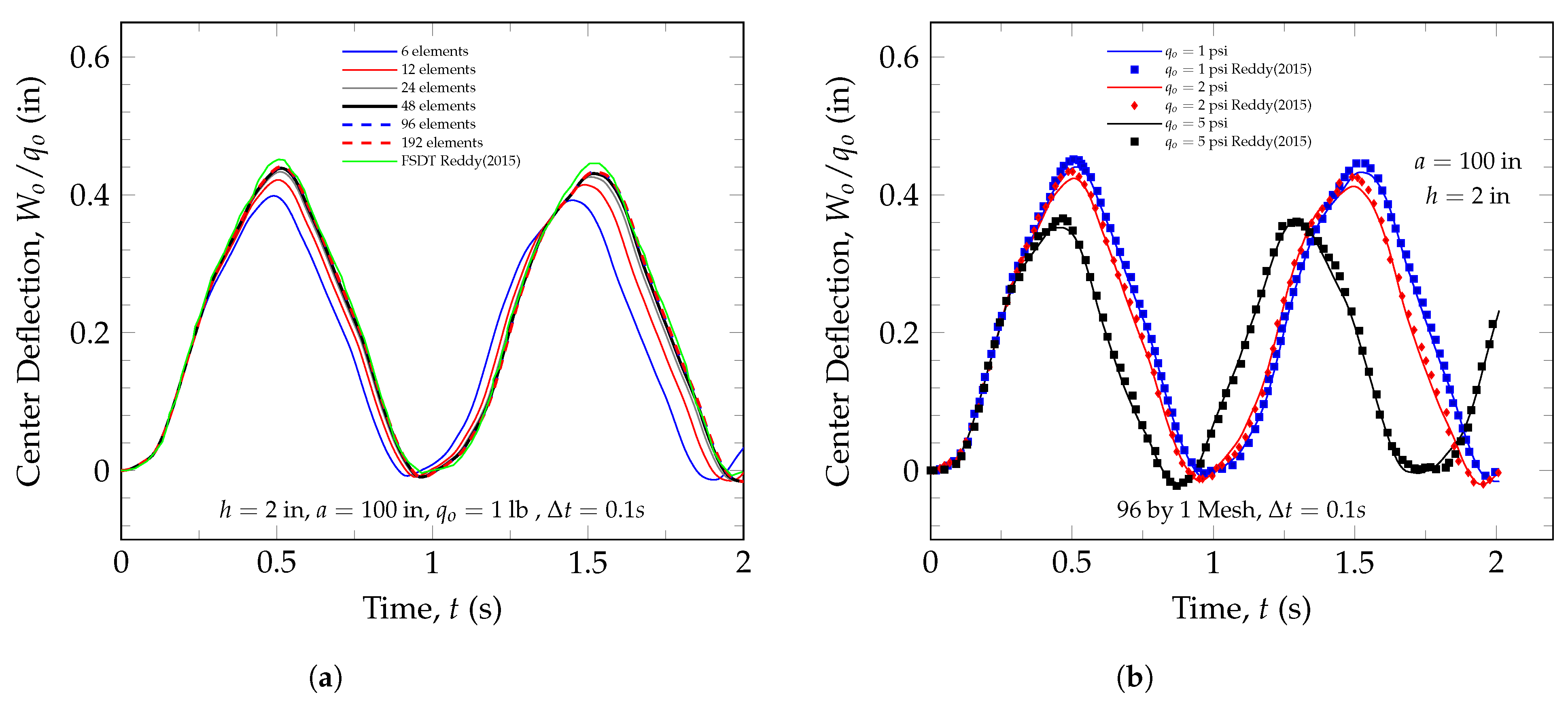
3.3. Transient Response
3.4. Temperature Distribution
3.5. Thermo-Mechanical LOAD
4. Numerical Examples
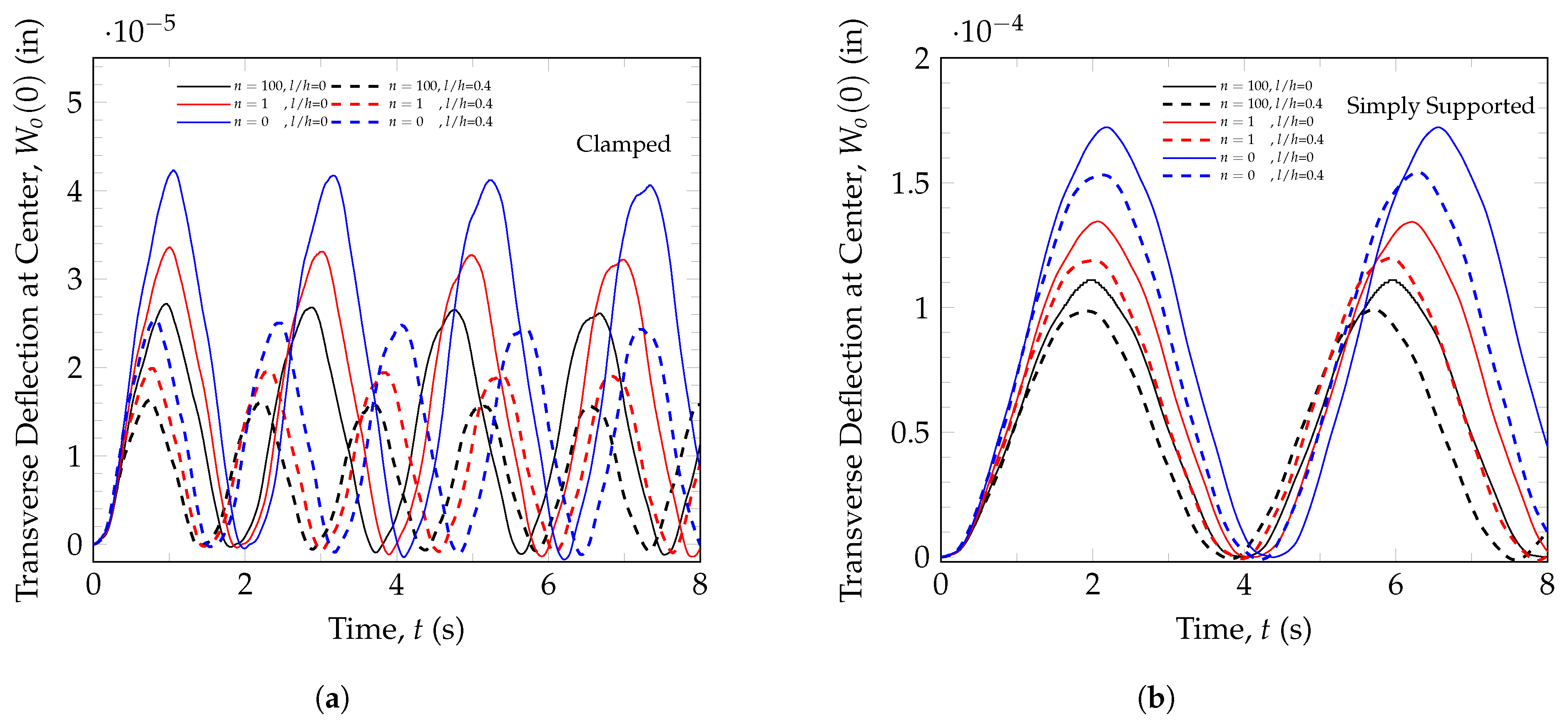
4.1. Dynamic Response
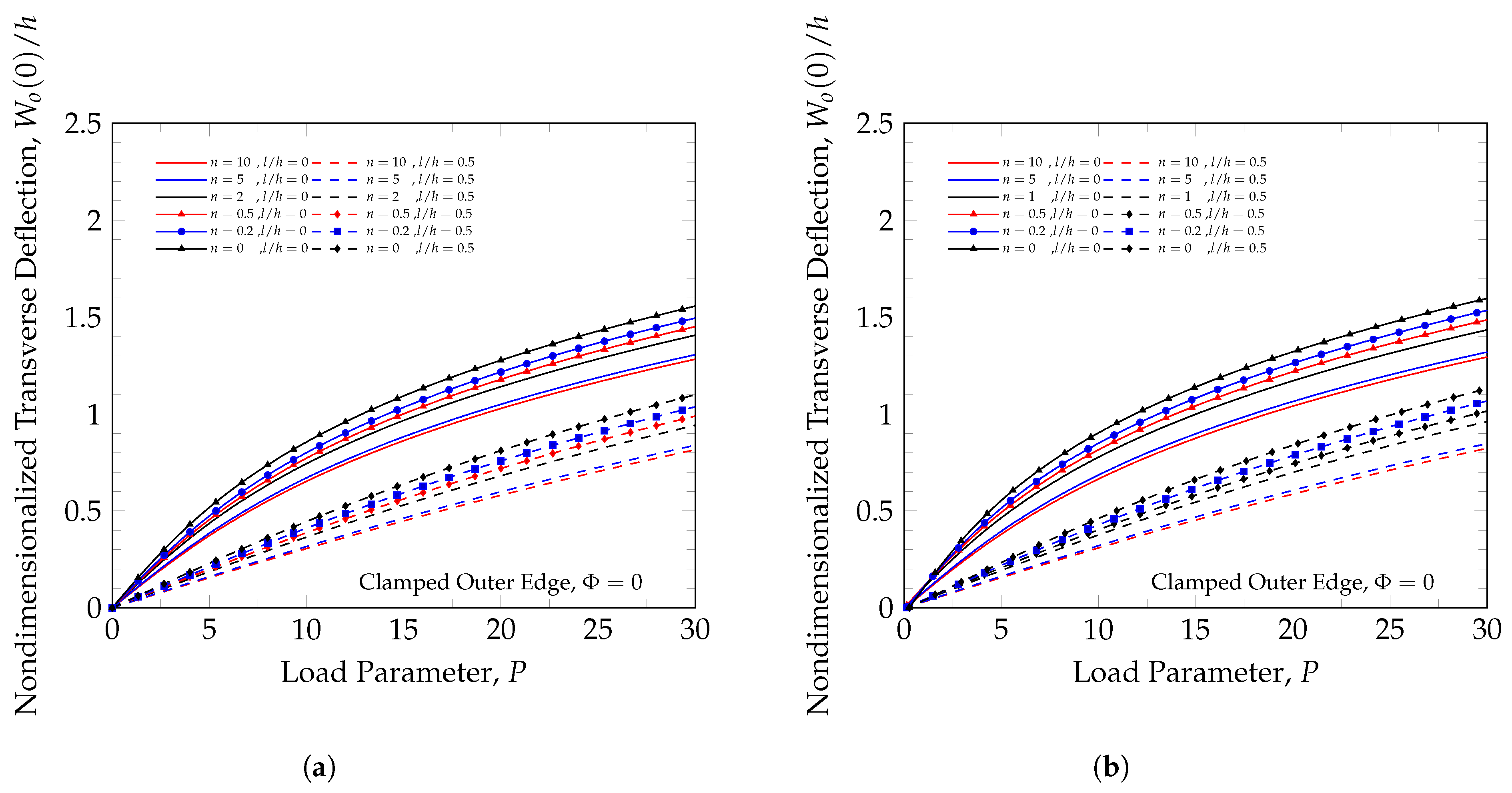
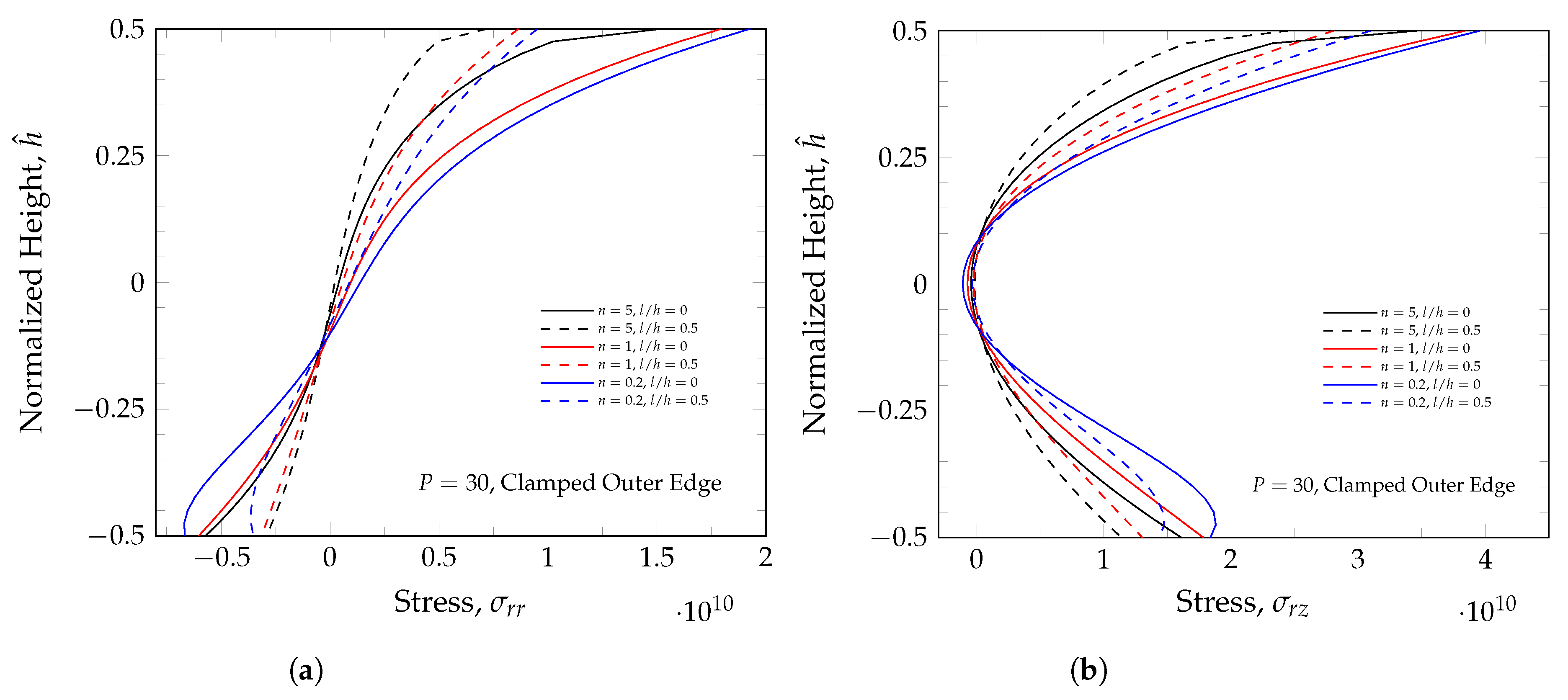
4.2. Static Response Under Symmetric Thermo-Mechanical Loads
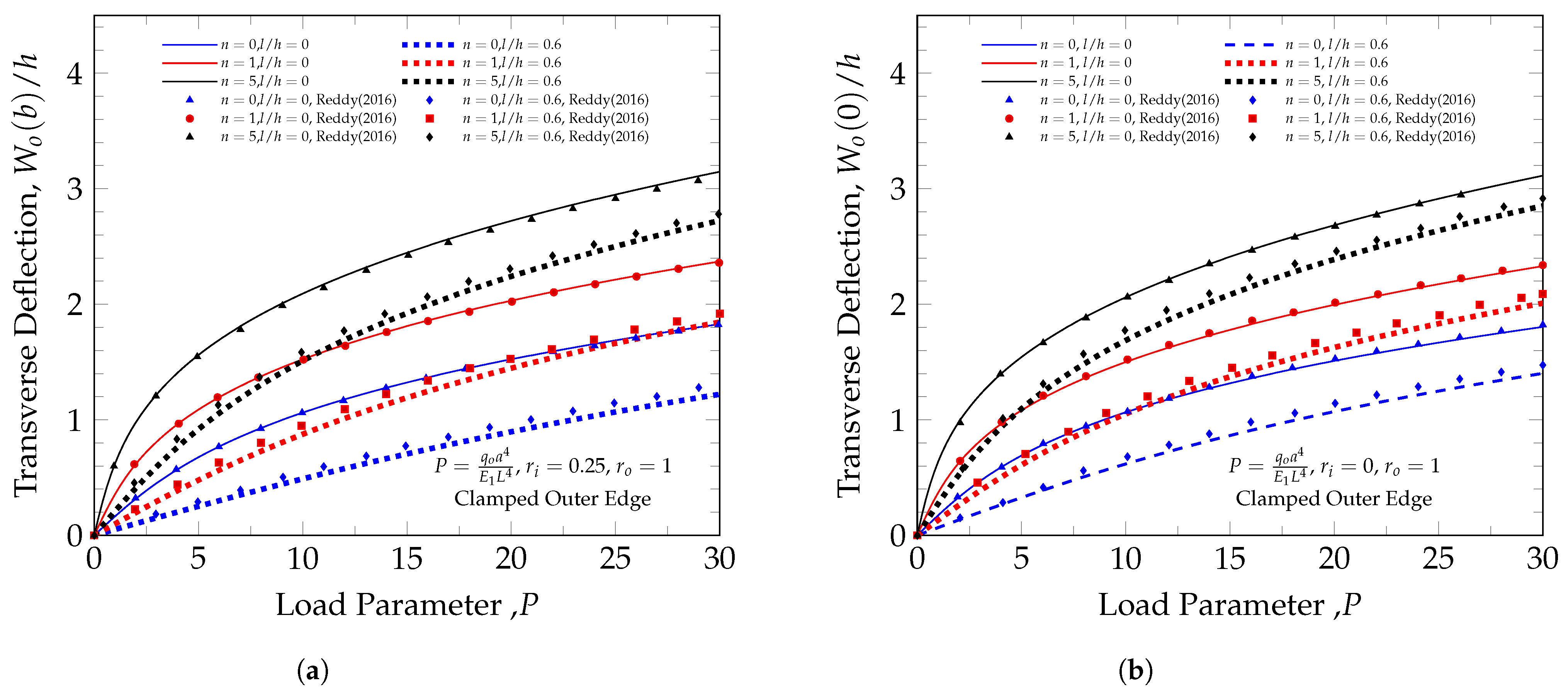
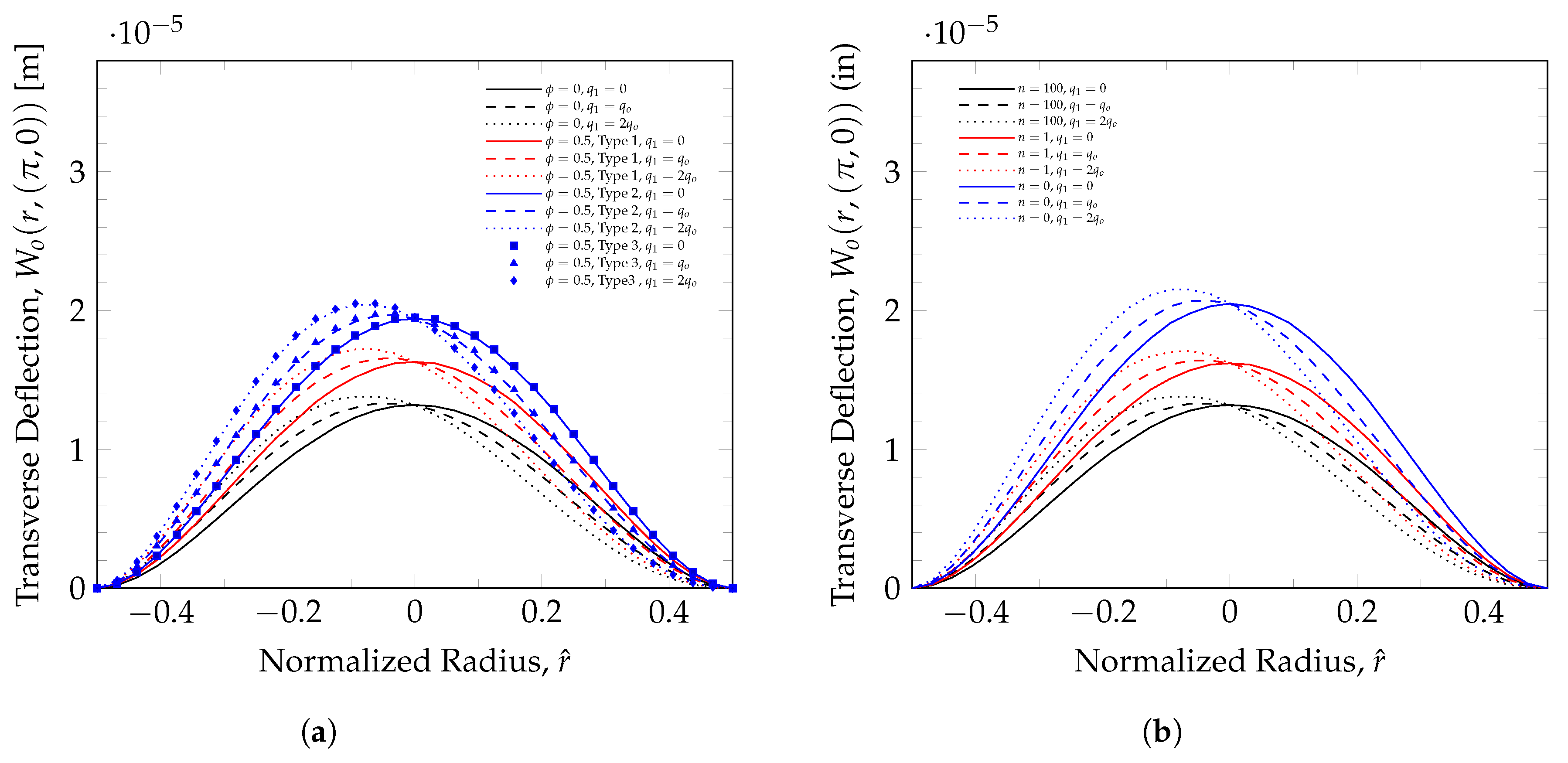
4.3. Static Response Under Asymmetric Loads
5. Conclusions
- A stiffening effect of the length scale parameter in the modified couple stress theory was observed for all boundary conditions and loads;
- Boundary conditions play an important role in the nonlinear behavior of the plate;
- The variation in the power-law index makes the plate softer or stiffer as it transitions from metallic- to ceramic-dominated properties;
- The model is able to capture nonlinear behavior and material variations in plate thickness. The proposed model was validated against other models in the literature, and it could be useful to study cases where these effects are expected to be significant;
- The proposed model has been found to be computationally expensive. This was expected due to the higher-order terms, and it is in line with the observations made by Reddy et al. [20] for their model, which also operates in cylindrical coordinates.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix A.1. Stiffness Matrix Elements
Appendix A.2. Mass Matrix Elements
Appendix A.3. Tangent Matrix Elements
References
- Koizumi, M. FGM activities in Japan. Compos. Part B Eng. 1997, 28, 1–4. [Google Scholar] [CrossRef]
- Birman, V.; Byrd, L.W. Modeling and Analysis of Functionally Graded Materials and Structures. Appl. Mech. Rev. 2007, 60, 195–216. [Google Scholar] [CrossRef]
- Mahamood, R.; Akinlabi, E.; Shukla, D.M.; Pityana, S. Functionally graded material: An overview. In Proceedings of the World Congress on Engineering, London, UK, 4–6 July 2012; Volume III. [Google Scholar]
- Jha, D.; Kant, T.; Singh, R. A critical review of recent research on functionally graded plates. Compos. Struct. 2013, 96, 833–849. [Google Scholar] [CrossRef]
- Swaminathan, K.; Sangeetha, D. Thermal analysis of FGM plates—A critical review of various modeling techniques and solution methods. Compos. Struct. 2017, 160, 43–60. [Google Scholar] [CrossRef]
- Judy, J.W. Microelectromechanical systems (MEMS): Fabrication, design and applications. Smart Mater. Struct. 2001, 10, 1115. [Google Scholar] [CrossRef]
- Tahani, M.; Askari, A.R.; Mohandes, Y.; Hassani, B. Size-dependent free vibration analysis of electrostatically pre-deformed rectangular micro-plates based on the modified couple stress theory. Int. J. Mech. Sci. 2015, 94–95, 185–198. [Google Scholar] [CrossRef]
- Yang, F.; Chong, A.; Lam, D.; Tong, P. Couple stress based strain gradient theory for elasticity. Int. J. Solids Struct. 2002, 39, 2731–2743. [Google Scholar] [CrossRef]
- Ma, Q.; Clarke, D. Size dependent hardness of silver single crystals. J. Mater. Res. 1995, 10, 853–863. [Google Scholar] [CrossRef]
- Toupin, R.A. Elastic materials with couple-stresses. Arch. Ration. Mech. Anal. 1962, 11, 385–414. [Google Scholar] [CrossRef]
- Mindlin, R.D.; Tiersten, H.F. Effects of couple-stresses in linear elasticity. Arch. Ration. Mech. Anal. 1962, 11, 415–448. [Google Scholar] [CrossRef]
- Eringen, A. Nonlocal polar elastic continua. Int. J. Eng. Sci. 1972, 10, 1–16. [Google Scholar] [CrossRef]
- Fleck, N.; Muller, G.; Ashby, M.; Hutchinson, J. Strain gradient plasticity: Theory and experiment. Acta Metall. Mater. 1994, 42, 475–487. [Google Scholar] [CrossRef]
- Lam, D.; Yang, F.; Chong, A.; Wang, J.; Tong, P. Experiments and theory in strain gradient elasticity. J. Mech. Phys. Solids 2003, 51, 1477–1508. [Google Scholar] [CrossRef]
- Fleck, N.; Hutchinson, J. A Reformulation of Strain Gradient Plasticity. J. Mech. Phys. Solids 2001, 49, 2245–2271. [Google Scholar] [CrossRef]
- Shu, J.Y.; King, W.E.; Fleck, N.A. Finite elements for materials with strain gradient effects. Int. J. Numer. Methods Eng. 1999, 44, 373–391. [Google Scholar] [CrossRef]
- Yapor Genao, F.; Kim, J.; Żur, K.K. Nonlinear finite element analysis of temperature-dependent functionally graded porous micro-plates under thermal and mechanical loads. Compos. Struct. 2021, 256, 112931. [Google Scholar] [CrossRef]
- Aghababaei, R.; Reddy, J. Nonlocal third-order shear deformation plate theory with application to bending and vibration of plates. J. Sound Vib. 2009, 326, 277–289. [Google Scholar] [CrossRef]
- Ke, L.L.; Yang, J.; Kitipornchai, S.; Bradford, M.A. Bending, buckling and vibration of size-dependent functionally graded annular microplates. Compos. Struct. 2012, 94, 3250–3257. [Google Scholar] [CrossRef]
- Reddy, J.; Romanoff, J.; Loya, J.A. Nonlinear finite element analysis of functionally graded circular plates with modified couple stress theory. Eur. J. Mech.—A/Solids 2016, 56, 92–104. [Google Scholar] [CrossRef]
- Reddy, J.; Berry, J. Nonlinear theories of axisymmetric bending of functionally graded circular plates with modified couple stress. Compos. Struct. 2012, 94, 3664–3668. [Google Scholar] [CrossRef]
- Tadi Beni, Y.; Mehralian, F.; Razavi, H. Free vibration analysis of size-dependent shear deformable functionally graded cylindrical shell on the basis of modified couple stress theory. Compos. Struct. 2015, 120, 65–78. [Google Scholar] [CrossRef]
- Kim, J.; Żur, K.K.; Reddy, J. Bending, free vibration, and buckling of modified couples stress-based functionally graded porous micro-plates. Compos. Struct. 2019, 209, 879–888. [Google Scholar] [CrossRef]
- Eshraghi, I.; Dag, S.; Soltani, N. Bending and free vibrations of functionally graded annular and circular micro-plates under thermal loading. Compos. Struct. 2016, 137, 196–207. [Google Scholar] [CrossRef]
- Hosseini-Hashemi, S.; Bedroud, M.; Nazemnezhad, R. An exact analytical solution for free vibration of functionally graded circular/annular Mindlin nanoplates via nonlocal elasticity. Compos. Struct. 2013, 103, 108–118. [Google Scholar] [CrossRef]
- Chi, S.H.; Chung, Y.L. Mechanical behavior of functionally graded material plates under transverse load—Part I: Analysis. Int. J. Solids Struct. 2006, 43, 3657–3674. [Google Scholar] [CrossRef]
- Chi, S.H.; Chung, Y.L. Mechanical behavior of functionally graded material plates under transverse load—Part II: Numerical results. Int. J. Solids Struct. 2006, 43, 3675–3691. [Google Scholar] [CrossRef]
- Nguyen, T.K.; Sab, K.; Bonnet, G. First-order shear deformation plate models for functionally graded materials. Compos. Struct. 2008, 83, 25–36. [Google Scholar] [CrossRef]
- Reddy, J. Analysis of functionally graded plates. Int. J. Numer. Methods Eng. 2000, 47, 663–684. [Google Scholar]
- Reddy, J.; Chin, C. Thermomechanical analysis of functionally graded cylinders and plates. J. Therm. Stress. 1998, 21, 593–626. [Google Scholar]
- Praveen, G.; Reddy, J. Nonlinear transient thermoelastic analysis of functionally graded ceramic-metal plates. Int. J. Solids Struct. 1998, 35, 4457–4476. [Google Scholar] [CrossRef]
- Reddy, J.; Wang, C.; Kitipornchai, S. Axisymmetric bending of functionally graded circular and annular plates. Eur. J. Mech.–A/Solids 1999, 18, 185–199. [Google Scholar] [CrossRef]
- Ma, L.; Wang, T. Nonlinear bending and post-buckling of a functionally graded circular plate under mechanical and thermal loadings. Int. J. Solids Struct. 2003, 40, 3311–3330. [Google Scholar] [CrossRef]
- Reddy, J. Theory and analysis of Elastic Plates and Shells; CRC Press: Boca Raton, FL, USA, 2007. [Google Scholar]
- Najafizadeh, M.; Heydari, H. Thermal buckling of functionally graded circular plates based on higher order shear deformation plate theory. Eur. J. Mech.—A/Solids 2004, 23, 1085–1100. [Google Scholar] [CrossRef]
- Reddy, J.; Kim, J. A nonlinear modified couple stress-based third-order theory of functionally graded plates. Compos. Struct. 2012, 94, 1128–1143. [Google Scholar] [CrossRef]
- Kim, J.; Reddy, J. Analytical solutions for bending, vibration, and buckling of FGM plates using a couple stress-based third-order theory. Compos. Struct. 2013, 103, 86–98. [Google Scholar] [CrossRef]
- Prakash, T.; Ganapathi, M. Asymmetric flexural vibration and thermoelastic stability of FGM circular plates using finite element method. Compos. Part B Eng. 2006, 37, 642–649. [Google Scholar] [CrossRef]
- Efraim, E.; Eisenberger, M. Exact vibration analysis of variable thickness thick annular isotropic and FGM plates. J. Sound Vib. 2007, 299, 720–738. [Google Scholar] [CrossRef]
- Żur, K. Free vibration analysis of discrete-continuous functionally graded circular plates via Neumann series method. Appl. Math. Model. 2019, 73, 166–189. [Google Scholar] [CrossRef]
- Rahmat Talabi, M.; Saidi, A. An explicit exact analytical approach for free vibration of circular/annular functionally graded plates bonded to piezoelectric actuator/sensor layers based on Reddy’s plate theory. Appl. Math. Model. 2013, 37, 7664–7684. [Google Scholar] [CrossRef]
- Zhao, J.; Xie, F.; Wang, A.; Shuai, C.; Tang, J.; Wang, Q. Dynamics analysis of functionally graded porous (FGP) circular, annular and sector plates with general elastic restraints. Compos. Part B Eng. 2019, 159, 20–43. [Google Scholar] [CrossRef]
- Talha, M.; Singh, B. Static response and free vibration analysis of FGM plates using higher order shear deformation theory. Appl. Math. Model. 2010, 34, 3991–4011. [Google Scholar] [CrossRef]
- Vinh, P.V. A novel modified nonlocal strain gradient theory for comprehensive analysis of functionally graded nanoplates. Acta Mech. 2025, 236, 173–204. [Google Scholar]
- Wang, S.; Ding, W.; Li, Z.; Xu, B.; Zhai, C.; Kang, W.; Yang, W.; Li, Y. A size-dependent quasi-3D model for bending and buckling of porous functionally graded curved nanobeam. Int. J. Eng. Sci. 2023, 193, 103962. [Google Scholar]
- Nosier, A.; Fallah, F. Non-linear analysis of functionally graded circular plates under asymmetric transverse loading. Int. J.-Non-Linear Mech. 2009, 44, 928–942. [Google Scholar] [CrossRef]
- Nosier, A.; Fallah, F. Reformulation of Mindlin–Reissner governing equations of functionally graded circular plates. Acta Mech. 2008, 198, 209–233. [Google Scholar]
- Fallah, F.; Nosier, A. Nonlinear behavior of functionally graded circular plates with various boundary supports under asymmetric thermo-mechanical loading. Compos. Struct. 2012, 94, 2834–2850. [Google Scholar] [CrossRef]
- Kim, J.; Nava, E.; Rakici, S. Nonlinear Finite Element Model for Bending Analysis of Functionally-Graded Porous Circular/Annular Micro-Plates under Thermomechanical Loads Using Quasi-3D Reddy Third-Order Plate Theory. Materials 2023, 16, 3505. [Google Scholar] [CrossRef] [PubMed]
- Piotr, D. Finite element method formulation in polar coordinates for transient heat conduction problems. J. Therm. Sci. 2016, 25, 188–194. [Google Scholar] [CrossRef]
- Touloukian, Y.S. Thermophysical Properties of High Temperature Solid Materials. Volume 4. Oxides and Their Solutions and Mixtures; Defense Technical Information Center: Fort Belvoir, VA, USA, 1966. [Google Scholar]
- Nguyen, L.B.; Thai, C.H.; Zenkour, A.; Nguyen-Xuan, H. An isogeometric Bézier finite element method for vibration analysis of functionally graded piezoelectric material porous plates. Int. J. Mech. Sci. 2019, 157–158, 165–183. [Google Scholar] [CrossRef]
- Carranza, J.C.; Pérez, L.; Ganesan, R.; Casas, B.Y.; Drew, R.A.L.; Ruiz-Aguilar, C.; Figueroa, I.A.; Alfonso, I. Effect of fractal distribution of the porosity on mechanical properties of Al foams manufactured by infiltration. J. Braz. Soc. Mech. Sci. Eng. 2019, 41, 379. [Google Scholar] [CrossRef]
- Gao, K.; Li, R.; Yang, J. Dynamic characteristics of functionally graded porous beams with interval material properties. Eng. Struct. 2019, 197, 109441. [Google Scholar]
- Coskun, S.; Kim, J.; Toutanji, H. Bending, Free Vibration, and Buckling Analysis of Functionally Graded Porous Micro-Plates Using a General Third-Order Plate Theory. J. Compos. Sci. 2019, 3, 15. [Google Scholar] [CrossRef]
- Mota, A.F.; Loja, M.A.R.; Barbosa, J.I.; Rodrigues, J.A. Porous Functionally Graded Plates: An Assessment of the Influence of Shear Correction Factor on Static Behavior. Math. Comput. Appl. 2020, 25, 25. [Google Scholar] [CrossRef]
- Kim, J.; Reddy, J. A general third-order theory of functionally graded plates with modified couple stress effect and the von Kármán nonlinearity: Theory and finite element analysis. Acta Mech. 2015, 226, 1973–2998. [Google Scholar] [CrossRef]
- Golmakani, M.; Kadkhodayan, M. Nonlinear bending analysis of annular FGM plates using higher-order shear deformation plate theories. Composite Structures 2011, 93, 973–982. [Google Scholar] [CrossRef]
- Mognhod Bezzie, Y.; Woldemichael, D. Effects of graded-index and Poisson’s ratio on elastic-solutions of a pressurized functionally graded material thick-walled cylinder. Forces Mech. 2021, 4, 100032. [Google Scholar] [CrossRef]
- Bogner, F. The generation of interelement compatible stiffness and mass matrices by the use of interpolation formulae. In Proceedings of the Conference on Matrix Methods in Structural Mechanics, Wright-Patterson Air Force Base, Fairborn, OH, USA, 26–28 October 1965. [Google Scholar]
- Deng, Z.; Huang, Y.; Zhang, C.; Chen, Y. Heat Conduction in Porous Media Characterized by Fractal Geometry. Energies 2017, 10, 1230. [Google Scholar] [CrossRef]
- Reddy, J. An Introduction to Nonlinear Finite Element Analysis, 2nd ed.; Oxford University Press: Oxford, UK, 2015. [Google Scholar]

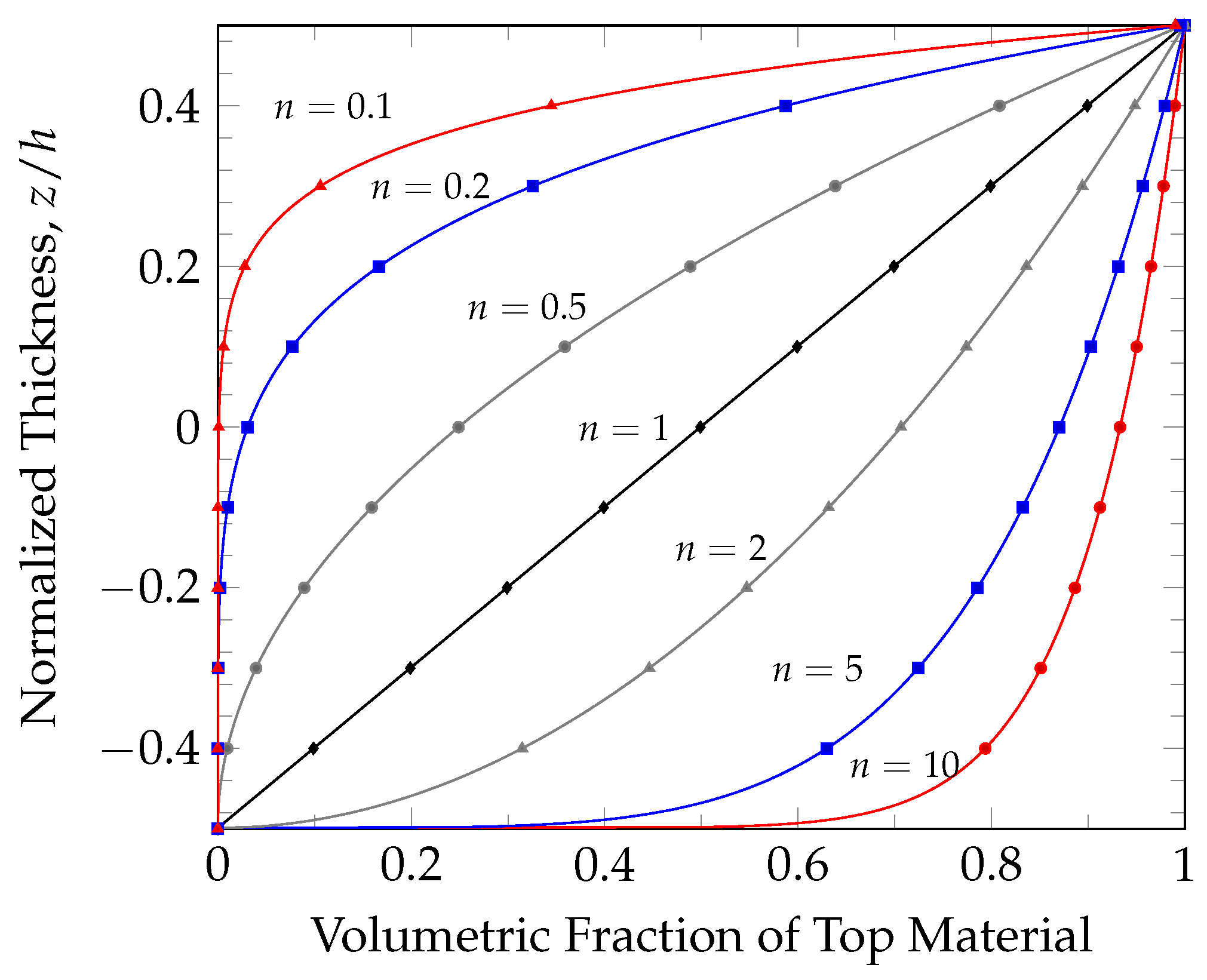
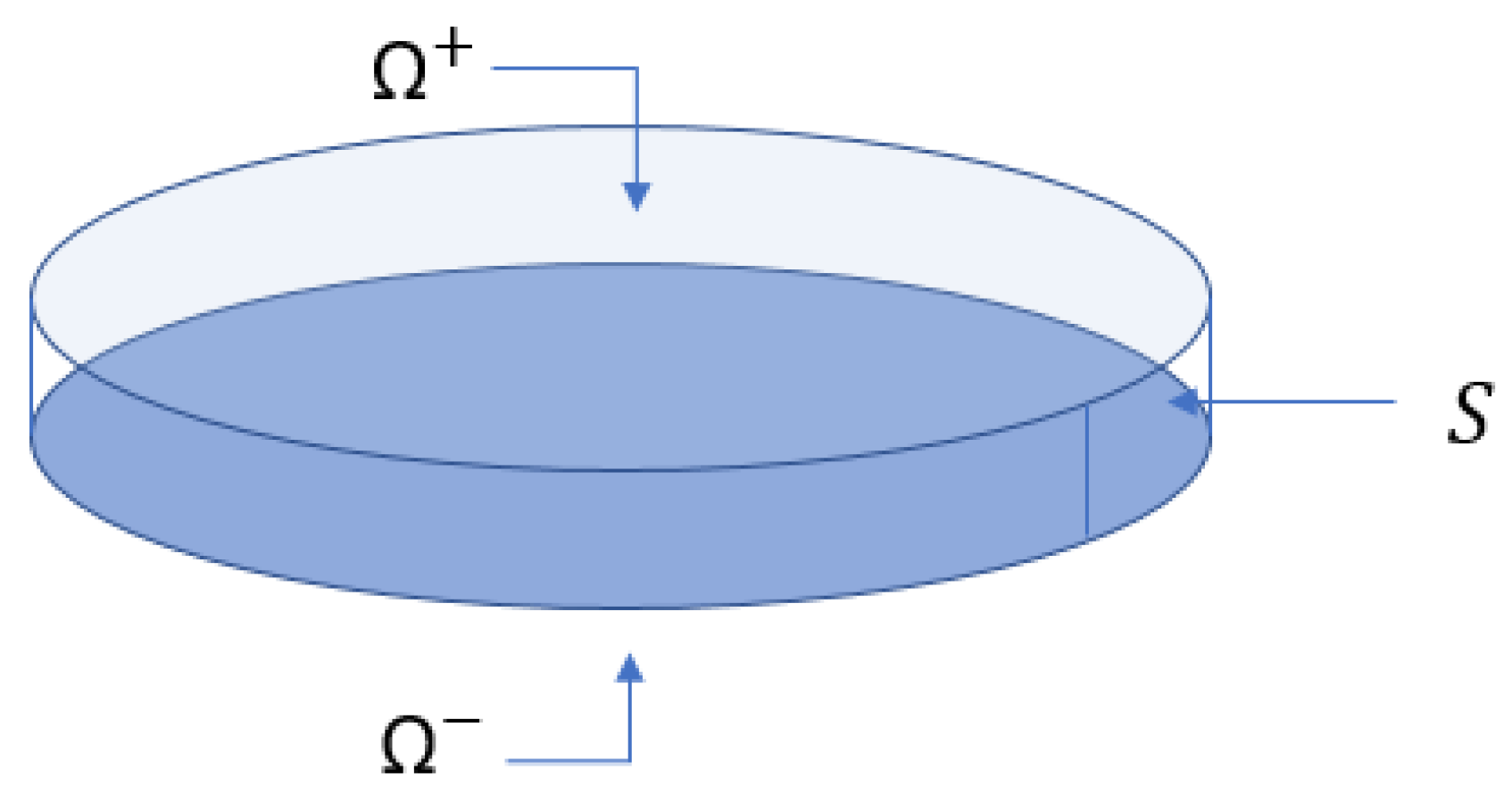
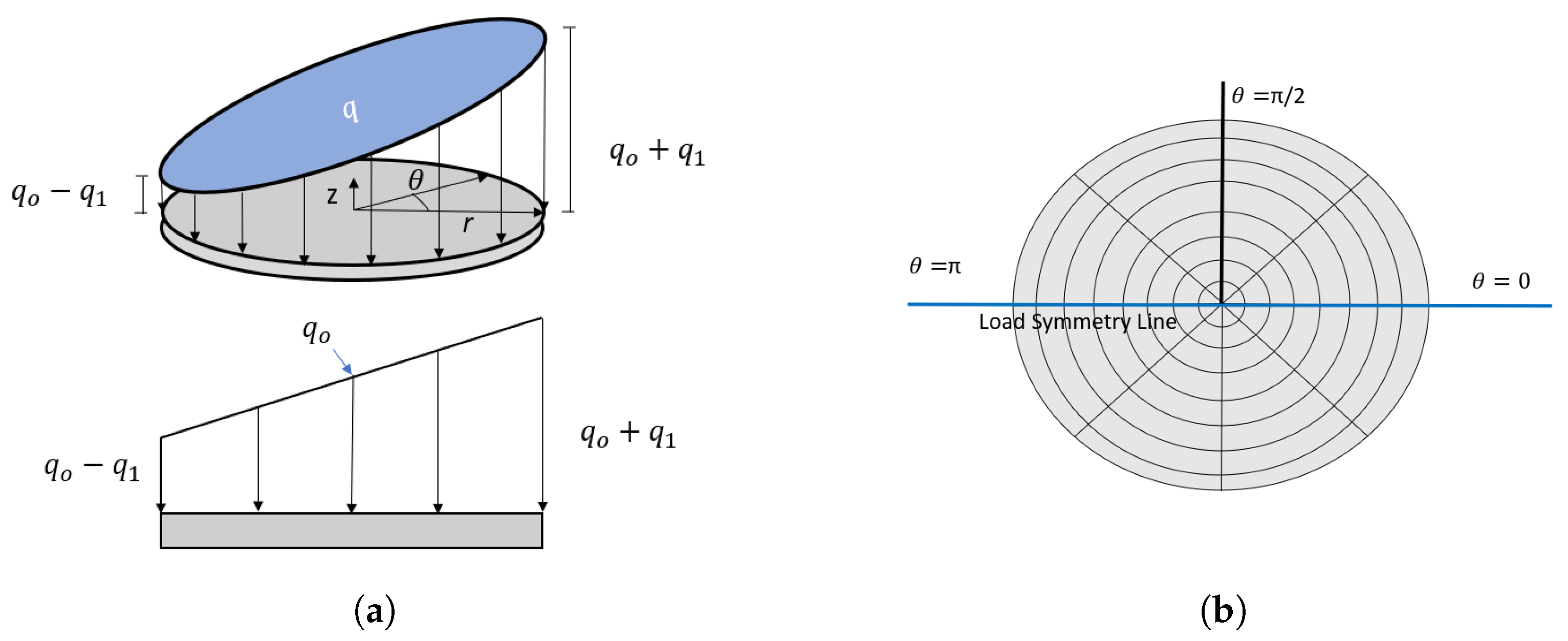


| Boundary Condition | Edge Definition | Constrained Generalized Displacements | ||||||
|---|---|---|---|---|---|---|---|---|
| Clamped outer edge | ||||||||
| Clamped inner edge (Only annular plates) | ||||||||
| Simply supported | ||||||||
| Zirconia | ||||
|---|---|---|---|---|
| Density, | 5700 | 0 | 0 | 0 |
| Thermal conductivity, k (W/m K) | 1.276 | 0.6485 | 0 | |
| Coefficient of thermal expansion, (K) | −14.4 | 0.0001 | −0.0678 | |
| Poison’s ratio, | 1.1335 | 0 | 0 | |
| Specific heat, (J/kg K) | 3.0491 | −6.0372 | 0 | |
| Young’s Modulus, E (Pa) | −13.707 | 121.393 | −3.6814 |
| Ti-6Al-4V | ||||
|---|---|---|---|---|
| Density, | 4429 | 0 | 0 | 0 |
| Thermal conductivity, k (W/m K) | 139.375 | 0 | 0 | |
| Coefficient of thermal expansion, (K) | 6.5 | 31.467 | 0 | |
| Poison’s ratio, | 1.1214 | 0 | 0 | |
| Specific heat, (J/kg K) | −4.2239 | 71.7865 | 0 | |
| Young’s Modulus, E (Pa) | −4.5864 | 0 | −3.6814 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nava, E.; Kim, J. Nonlinear Finite Element Model for FGM Porous Circular and Annular Micro-Plates Under Thermal and Mechanical Loads Using Modified Couple Stress-Based Third-Order Plate Theory. Math. Comput. Appl. 2025, 30, 35. https://doi.org/10.3390/mca30020035
Nava E, Kim J. Nonlinear Finite Element Model for FGM Porous Circular and Annular Micro-Plates Under Thermal and Mechanical Loads Using Modified Couple Stress-Based Third-Order Plate Theory. Mathematical and Computational Applications. 2025; 30(2):35. https://doi.org/10.3390/mca30020035
Chicago/Turabian StyleNava, Enrique, and Jinseok Kim. 2025. "Nonlinear Finite Element Model for FGM Porous Circular and Annular Micro-Plates Under Thermal and Mechanical Loads Using Modified Couple Stress-Based Third-Order Plate Theory" Mathematical and Computational Applications 30, no. 2: 35. https://doi.org/10.3390/mca30020035
APA StyleNava, E., & Kim, J. (2025). Nonlinear Finite Element Model for FGM Porous Circular and Annular Micro-Plates Under Thermal and Mechanical Loads Using Modified Couple Stress-Based Third-Order Plate Theory. Mathematical and Computational Applications, 30(2), 35. https://doi.org/10.3390/mca30020035






