A New Fractional Boundary Element Model for the 3D Thermal Stress Wave Propagation Problems in Anisotropic Materials
Abstract
1. Introduction
2. Formulation of the Problem
3. Boundary Element Implementation
3.1. Case 1:
3.2. Case 2:
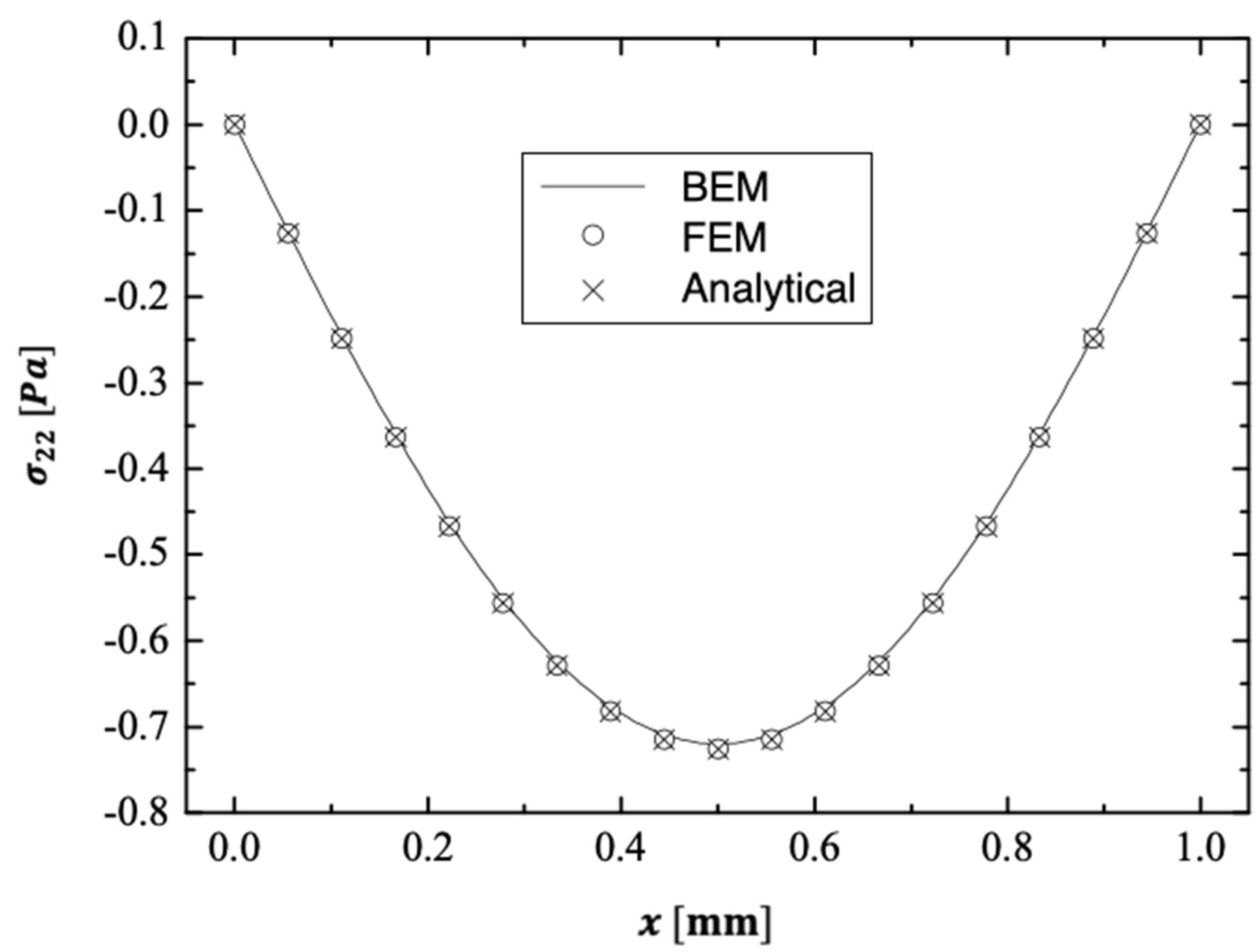
4. Numerical Results and Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Coefficients of thermal expansion | |
| thermal moduli | |
| Kronecker delta | |
| Strains | |
| Stress | |
| Time | |
| Fractional-order parameter | |
| Elastic constants | |
| Specific heat at constant strain | |
| boundary | |
| dilatation | |
| Body force vector | |
| Non-Gaussian temporal profile | |
| Total energy intensity | |
| Thermal conductivity | |
| Outward normal components | |
| Heat source intensity | |
| Irradiated surface absorptivity | |
| radial distance | |
| Temperature | |
| Temperature fundamental solutions | |
| Reference temperature | |
| Laser pulse time characteristic | |
| Displacement vector | |
| Displacement fundamental solutions | |
Appendix A. Laplace Transform Implementation
Appendix B. Helmholtz Theorem Implementation
References
- Ezzat, M.; Zakaria, M.; Shaker, O.; Barakat, F. State space formulation to viscoelastic fluid flow of magnetohydrodynamic free convection through a porous medium. Acta Mech. 1996, 119, 147–164. [Google Scholar] [CrossRef]
- Ezzat, M.A. Free convection effects on perfectly conducting fluid. Int. J. Eng. Sci. 2001, 39, 799–819. [Google Scholar] [CrossRef]
- Fahmy, M.A. Thermoelastic stresses in a rotating non-homogeneous aniso-tropic body. Numer. Heat Transf. Part A 2008, 53, 1001–1011. [Google Scholar] [CrossRef]
- Abouelregal, A.E.; Mohammed, W.W. Effects of nonlocal thermoelasticity on nanoscale beams based on couple stress theory. Math. Methods Appl. Sci. 2020. [Google Scholar] [CrossRef]
- Othman, M.I.A.; Abd-Elaziz, E.M. Dual-phase-lag model on micropolar thermoelastic rotating medium under the effect of thermal load due to laser pulse. Indian J. Phys. 2020, 94, 999–1008. [Google Scholar] [CrossRef]
- Othman, M.I.A.; Zidan, M.E.M.; Mohamed, I.E.A. Dual-phase-lag model on thermo-microstretch elastic solid under the effect of initial stress and temperature-dependent. Steel Compos. Struct. 2021, 38, 355–363. [Google Scholar]
- Bagley, R.L.; Torvik, P.J. On the fractional calculus model of viscoelastic behavior. J. Rheol. 1986, 30, 133–155. [Google Scholar] [CrossRef]
- Machado, J.A.T. Analysis and design of fractional-order digital control systems. SAMS J. Syst. Anal. Model. Simul. 1997, 27, 107–122. [Google Scholar]
- Oldham, K.B.; Spanier, J. The Fractional Calculus: Theory and Applications of Differentiation and Integration to Arbitrary Order, 1st ed.; Dover Publication: Mineola, NY, USA, 2006; Volume 111, pp. 1–64. [Google Scholar]
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations, 1st ed.; Elsevier Science: Amsterdam, The Netherlands, 2006; Volume 204, pp. 449–463. [Google Scholar]
- Sabatier, J.; Agrawal, O.P.; Machado, J.A.T. Advances in Fractional Calculus: Theoretical Developments and Applications in Physics and Engineering, 1st ed.; Springer: Dordrecht, The Netherlands, 2007; Volume 1, pp. 169–302. [Google Scholar]
- Wang, J.L.; Li, H.F. Surpassing the fractional derivative: Concept of the memory-dependent derivative. Comput. Math. Appl. 2011, 62, 1562–1567. [Google Scholar] [CrossRef]
- Yu, Y.J.; Zhao, L.J. Fractional thermoelasticity revisited with new definitions of fractional derivative. Eur. J. Mech.—A/Solids 2020, 84, 104043. [Google Scholar] [CrossRef]
- Shiah, Y.C.; Tuan, N.A.; Hematiyan, M.R. Direct transformation of the volume integral in the boundary integral equation for treating three-dimensional steady-state anisotropic thermoelasticity involving volume heat source. Int. J. Solids Struct. 2018, 143, 287–297. [Google Scholar] [CrossRef]
- Fahmy, M.A. Boundary element algorithm for nonlinear modeling and simulation of three temperature anisotropic generalized micropolar piezothermoelasticity with memory-dependent derivative. Int. J. Appl. Mech. 2020, 12, 2050027. [Google Scholar] [CrossRef]
- Fahmy, M.A. Boundary element modeling of fractional nonlinear generalized photothermal stress wave propagation problems in FG anisotropic smart semiconductors. Eng. Anal. Bound. Elem. 2022, 134, 665–679. [Google Scholar] [CrossRef]
- Fahmy, M.A.; Shaw, S.; Mondal, S.; Abouelregal, A.E.; Lotfy, K.; Kudinov, I.A.; Soliman, A.H. Boundary Element Modeling for Simulation and Optimization of Three-Temperature Anisotropic Micropolar Magneto-thermoviscoelastic Problems in Porous Smart Structures Using NURBS and Genetic Algorithm. Int. J. Thermophys. 2021, 42, 29. [Google Scholar] [CrossRef]
- Othman, M.I.A.; Atwa, S.Y.; Farouk, R.M. Generalized magneto-thermoviscoelastic plane waves under the effect of rotation without energy dissipation. Int. J. Eng. Sci. 2008, 46, 639–653. [Google Scholar] [CrossRef]
- Othman, M.I.A.; Song, Y. Effect of rotation on plane waves of generalized electro magneto-thermoviscoelasticity with two relaxation times. Appl. Math. Model. 2008, 32, 811–825. [Google Scholar] [CrossRef]
- Ezzat, M.A.; Awad, E.S. Micropolar generalized magneto-thermoelasticity with modified Ohm’s and Fourier’s laws. J. Math. Anal. Appl. 2009, 353, 99–113. [Google Scholar] [CrossRef]
- Ezzat, M.A.; Youssef, H.M. Generalized magneto-thermoelasticity in a perfectly conducting medium. Int. J. Solids Struct. 2005, 42, 6319–6334. [Google Scholar] [CrossRef]
- Othman, M.I.A.; Eraki, E.E.M. Effect of gravity on generalized thermoelastic diffusion due to laser pulse using dual-phase-lag model. Multidiscip. Model. Mater. Struct. 2018, 14, 457–481. [Google Scholar] [CrossRef]
- Fahmy, M.A. A Nonlinear Fractional BEM Model for Magneto-Thermo-Visco-Elastic Ultrasound Waves in Temperature-Dependent FGA Rotating Granular Plates. Fractal Fract. 2023, 7, 214. [Google Scholar] [CrossRef]
- Fahmy, M.A. A New Boundary Element Strategy for Modeling and Simulation of Three Temperatures Nonlinear Generalized Micropolar-Magneto-Thermoelastic Wave Propagation Problems in FGA Structures. Eng. Anal. Bound. Elem. 2019, 108, 192–200. [Google Scholar] [CrossRef]
- Duhamel, J. Some memoire sur les phenomenes thermo-mechanique. J. L’école Polytech. 1837, 15, 1–57. [Google Scholar]
- Neumann, F. Vorlesungen Uber die Theorie der Elasticitat; Meyer: Brestau, Germany, 1885. [Google Scholar]
- Biot, M. Thermoelasticity and irreversible thermo-dynamics. J. Appl. Phys. 1956, 27, 249–253. [Google Scholar] [CrossRef]
- Lord, H.W.; Shulman, Y. A generalized dynamical theory of thermoelasticity. J. Mech. Phys. Solids 1967, 15, 299–309. [Google Scholar] [CrossRef]
- Green, A.E.; Lindsay, K.A. Thermoelasticity. J. Elast. 1972, 2, 1–7. [Google Scholar] [CrossRef]
- Green, A.E.; Naghdi, P.M. On undamped heat waves in an elastic solid. J. Therm. Stress. 1992, 15, 253–264. [Google Scholar] [CrossRef]
- Green, A.E.; Naghdi, P.M. Thermoelasticity without energy dissipation. J. Elast. 1993, 31, 189–208. [Google Scholar] [CrossRef]
- Chen, P.J.; Gurtin, M.E. On a theory of heat conduction involving two temperatures. Z. Angew. Math. Phys. ZAMP 1968, 19, 614–627. [Google Scholar] [CrossRef]
- Chen, P.J.; Gurtin, M.E.; Williams, W.O. A note on non-simple heat conduction. Z. Angew. Math. Phys. ZAMP 1968, 19, 969–970. [Google Scholar] [CrossRef]
- Chen, P.J.; Gurtin, M.E.; Williams, W.O. On the thermodynamics of non-simple elastic materials with two temperatures. Z. Angew. Math. Phys. ZAMP 1969, 20, 107–112. [Google Scholar] [CrossRef]
- Quintanilla, R. On existence, structural stability, convergence and spatial behavior in thermoelasticity with two-temperatures. Acta Mech. 2004, 168, 61–73. [Google Scholar] [CrossRef]
- Youssef, H.M. Theory of two-temperature generalized thermoelasticity. IMA J. Appl. Math. 2006, 71, 383–390. [Google Scholar] [CrossRef]
- Youssef, H.M.; Al-Lehaibi, E.A. State-space approach of two-temperature generalized thermoelasticity of one dimensional problem. Int. J. Solids Struct. 2007, 44, 1550–1562. [Google Scholar] [CrossRef]
- Bassiouny, E.; Youssef, H.M. Two-temperature generalized thermopiezoelasticity of finite rod subjected to different types of thermal loading. J. Therm. Stress. 2008, 31, 233–245. [Google Scholar] [CrossRef]
- Youssef, H.M. Two-dimensional problem of two-temperature generalized thermoelastic half-space subjected to ramp-type heating. Comput. Math. Model. 2008, 19, 201–216. [Google Scholar] [CrossRef]
- Fahmy, M.A. Shape design sensitivity and optimization for two-temperature generalized magneto-thermoelastic problems using time-domain DRBEM. J. Therm. Stress. 2018, 41, 119–138. [Google Scholar] [CrossRef]
- Rizzo, F.J.; Shippy, D.J. An advanced boundary integral equation method for three-dimensional thermoelasticity. Int. J. Numer. Methods Eng. 1977, 11, 1753–1768. [Google Scholar] [CrossRef]
- Brebbia, C.A. The Boundary Element Method for Engineers; Pentech Press: London, UK, 1978. [Google Scholar]
- Brebbia, C.A.; Walker, S. Boundary Element Method Techniques in Engineering; Newness-Butterworths: London, UK, 1980. [Google Scholar]
- Banerjee, P.K.; Butterfield, R. Boundary Element Methods in Engineering Science; McGraw-Hill: London, UK, 1981. [Google Scholar]
- Fenner, R.T. Boundary element for stress problems. In Seminar on Finite Elements or Boundary Elements; Institution of Mechanical Engineers: London, UK, 1982. [Google Scholar]
- Brebbia, C.A.; Tells, J.C.F.; Wrobel, L.C. Boundary Element Techniques: Theory and Applications in Engineering; Springer: Heidelberg, Germany, 1984. [Google Scholar]
- Sladek, V.; Sladek, J. Boundary integral equation method in two-dimensional thermoelasticity. Eng. Anal. 1984, 1, 135–148. [Google Scholar] [CrossRef]
- Sladek, V.; Sladek, J. Boundary integral equation method in thermoelasticity Part III: Uncoupled thermoelasticity. Appl. Math. Model. 1984, 8, 413–418. [Google Scholar] [CrossRef]
- Cruse, T.A.; Rizzo, F.J. Boundary integral equation methods-computational applications. In Applied Mechanics, Proceedings of the ASME Conference on Boundary Integral Equation Methods; ASME: New York, NY, USA, 1985; pp. 118–123. [Google Scholar]
- Cao, G.; Yu, B.; Chen, L.; Yao, W. Isogeometric dual reciprocity BEM for solving non-Fourier transient heat transfer problems in FGMs with uncertainty analysis. Int. J. Heat Mass Transf. 2022, 203, 123783. [Google Scholar] [CrossRef]
- Sladek, J.; Sladek, V.; Markechova, I. Boundary element method analysis of stationary thermoelasticity problems in non-homogeneous media. Int. J. Numer. Methods Eng. 1990, 30, 505–516. [Google Scholar] [CrossRef]
- Zhang, S.; Yu, B.; Chen, L. Non-iterative reconstruction of time-domain sound pressure and rapid prediction of large-scale sound field based on IG-DRBEM and POD-RBF. J. Sound Vib. 2024, 573, 118226. [Google Scholar] [CrossRef]
- Tanaka, M.; Matsumoto, T.; Moradi, M. Application of boundary element method to 3-D problems of coupled thermoelasticity. Eng. Anal. Boundary. Elem. 1995, 16, 297–303. [Google Scholar] [CrossRef]
- Ang, W.T.; Clements, D.L.; Cooke, T. A Complex Variable Boundary Element Method for a Class of Boundary Value Problems in Anisotropic Thermoelasticity. Int. J. Comput. Math. 1999, 70, 571–586. [Google Scholar] [CrossRef]
- Shiah, Y.C.; Tan, C.L. Exact boundary integral transformation of the thermoelastic domain integral in BEM for general 2D anisotropic elasticity. Comput. Mech. 1999, 23, 87–96. [Google Scholar] [CrossRef]
- Kogl, M.; Gaul, L. A boundary element method for anisotropic coupled thermoelasticity. Arch. Appl. Math. 2003, 73, 377–398. [Google Scholar]
- El-Naggar, A.M.; Abd-Alla, A.M.; Fahmy, M.A. The propagation of thermal stresses in an infinite elastic slab. Appl. Math. Comput. 2004, 157, 307–312. [Google Scholar] [CrossRef]
- Fahmy, M.A.; Jeli, R.A.A. A New Fractional Boundary Element Model for Anomalous Thermal Stress Effects on Cement-Based Materials. Fractal Fract. 2024, 8, 753. [Google Scholar] [CrossRef]
- Abd-Alla, A.M.; Fahmy, M.A.; El-Shahat, T.M. Magneto-thermo-elastic problem of a rotating non-homogeneous anisotropic solid cylinder. Arch. Appl. Mech. 2008, 78, 135–148. [Google Scholar] [CrossRef]
- Fahmy, M.A. A time-stepping DRBEM for nonlinear fractional sub-diffusion bio-heat ultrasonic wave propagation problems during electromagnetic radiation. J. Umm Al-Qura Univ. Appl. Sci. 2024. [Google Scholar] [CrossRef]
- Fahmy, M.A.; Almehmadi, M.M. Fractional Dual-Phase-Lag Model for Nonlinear Viscoelastic Soft Tissues. Fractal Fract. 2023, 7, 66. [Google Scholar] [CrossRef]
- Zhang, S.; Yu, B.; Chen, L.; Lian, H.; Bordas, S.P.A. Isogeometric dual reciprocity BEM for solving time-domain acoustic wave problems; Non-iterative reconstruction of time-domain sound pressure and rapid prediction of large-scale sound field based on IG-DRBEM and POD-RBF. Comput. Math. Appl. 2024, 160, 125–141. [Google Scholar] [CrossRef]
- Fahmy, M.A.; Alzubaidi, M.H.M. A boundary element analysis of quasi-potential inviscid incompressible flow in multiply connected airfoil wing. J. Umm Al-Qura Univ. Eng. Archit. 2024, 15, 398–402. [Google Scholar] [CrossRef]
- Fahmy, M.A.; Toujani, M. Fractional Boundary Element Solution for Nonlinear Nonlocal Thermoelastic Problems of Anisotropic Fibrous Polymer Nanomaterials. Computation 2024, 12, 117. [Google Scholar] [CrossRef]
- Fahmy, M.A.; Alsulami, M.O.; Abouelregalm, A.E. Three-Temperature Boundary Element Modeling of Ultrasound Wave Propagation in Anisotropic Viscoelastic Porous Media. Axioms 2023, 12, 473. [Google Scholar] [CrossRef]
- Fahmy, M.A. Fractional Temperature-Dependent BEM for Laser Ultrasonic Thermoelastic Propagation Problems of Smart Nanomaterials. Fractal Fract. 2023, 7, 536. [Google Scholar] [CrossRef]
- Fahmy, M.A. BEM Modeling for Stress Sensitivity of Nonlocal Thermo-Elasto-Plastic Damage Problems. Computation 2024, 12, 87. [Google Scholar] [CrossRef]
- Yu, B.; Jing, R. SCTBEM: A scaled coordinate transformation boundary element method with 99-line MATLAB code for solving Poisson’s equation. Comput. Phys. Commun. 2024, 300, 109185. [Google Scholar] [CrossRef]
- Jing, R.; Yu, B.; Ren, S.; Yao, W. A novel SCTBEM with inversion-free Padé series expansion for 3D transient heat transfer analysis in FGMs. Comput. Methods Appl. Mech. Eng. 2025, 433, 117546. [Google Scholar] [CrossRef]
- Takahashi, T.; Tanigawa, M.; Miyazawa, N. An enhancement of the fast time-domain boundary element method for the three-dimensional wave equation. Comput. Phys. Commun. 2022, 271, 108229. [Google Scholar] [CrossRef]
- Cattaneo, C. A form of heat conduction equation which eliminates the paradox of instantaneous propagation. Comptes Rendus 1958, 247, 431–433. [Google Scholar]
- Vernotte, P. Les paradoxes de la theorie continue de l‘equation de la chaleur. Comptes Rendus 1958, 246, 3154–3155. [Google Scholar]
- Vernotte, P. Some possible complications in the phenomena of thermal conduction. Comptes Rendus 1961, 252, 2190–2191. [Google Scholar]
- Sherief, H.H.; El Sayed, A.; El-Latief, A. Fractional Order Theory of Thermoelasticity. Int. J. Solids Struct. 2010, 47, 269–275. [Google Scholar] [CrossRef]
- Tiwari, R.; Mukhopadhyay, S. Boundary integral equations formulation for fractional order thermoelasticity. Comput. Methods Sci. Eng. CMST 2014, 20, 49–58. [Google Scholar] [CrossRef]
- Podlubny, I. Fractional Differential Equations. An Introduction to Fractional Order Derivatives, Fractional Differential Equations, to Methods of Their Solutions and Some of Their Applications; Academic Press: Cambridge, MA, USA, 1999; Volume 198. [Google Scholar]
- Li, Y.R.; Shao, X.F.; Li, S.Y. New Preconditioned Iteration Method Solving the Special Linear System from the PDE-Constrained Optimal Control Problem. Mathematics 2021, 9, 510. [Google Scholar] [CrossRef]
- Marin, M.; Hobiny, A.; Abbas, I. The Effects of Fractional Time Derivatives in Porothermoelastic Materials Using Finite Element Method. Mathematics 2021, 9, 1606. [Google Scholar] [CrossRef]
- Sharifi, H. Analytical Solution for Thermoelastic Stress Wave Propagation in an Orthotropic Hollow Cylinder. Eur. J. Comput. Mech. 2022, 31, 239–274. [Google Scholar] [CrossRef]
- Jing, Y.F.; Huang, T.Z. On a new iterative method for solving linear systems and comparison results. J. Comput. Appl. Math. 2008, 220, 74–84. [Google Scholar] [CrossRef]
- Darvishi, M.T.; Hessari, P. A modified symmetric successive overrelaxation method for augmented systems. Comput. Math. Appl. 2011, 61, 3128–3135. [Google Scholar] [CrossRef]

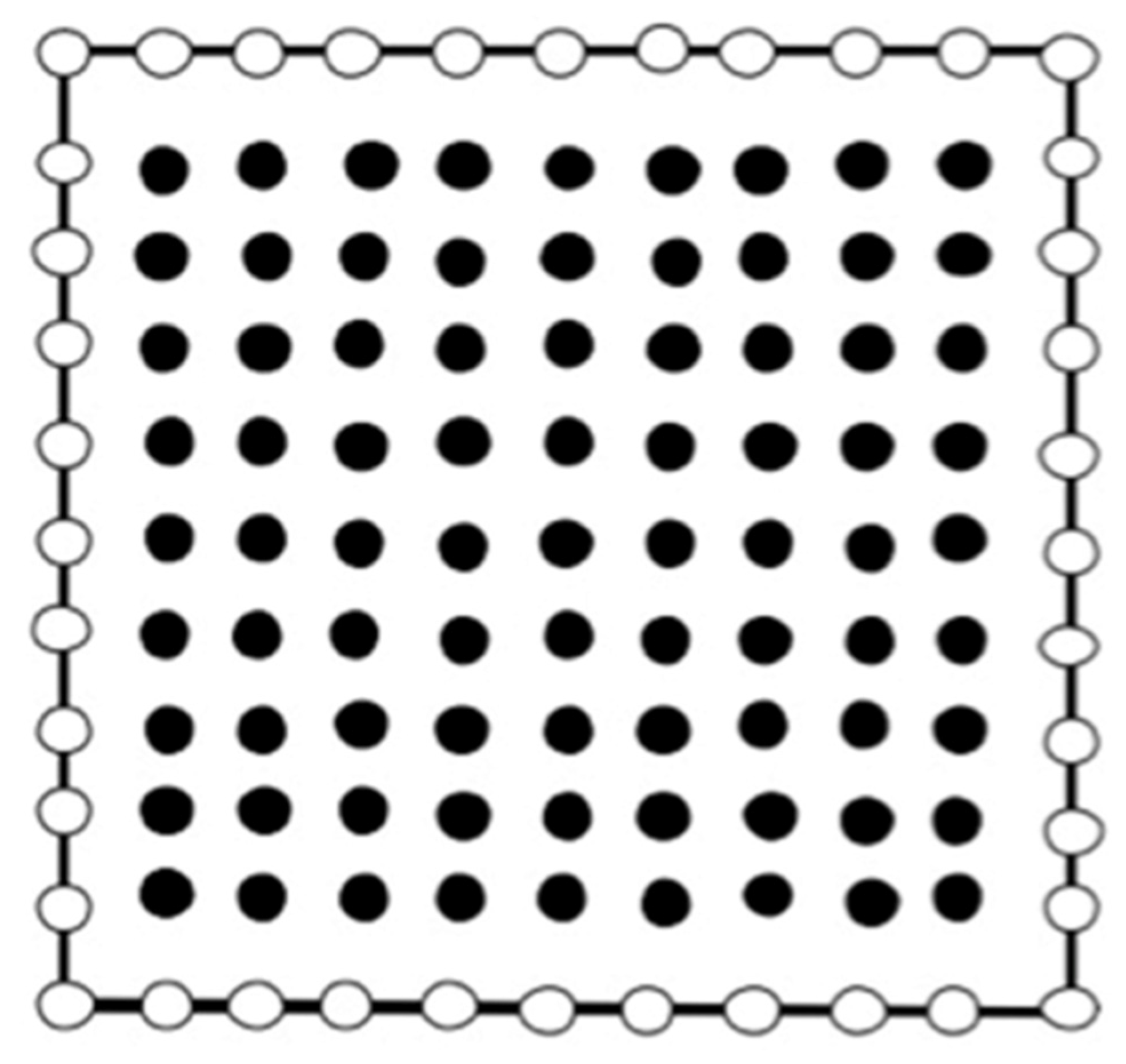
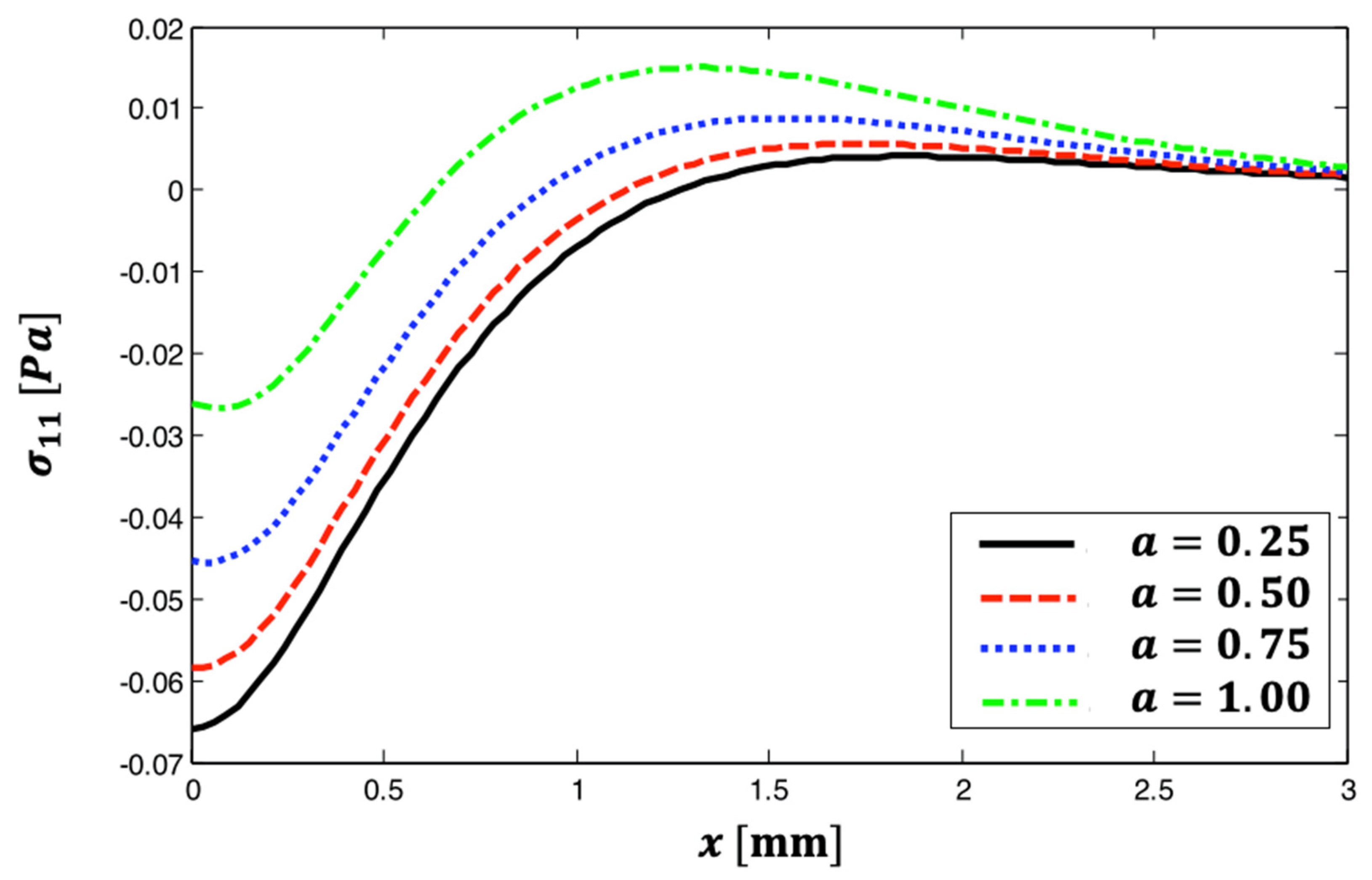

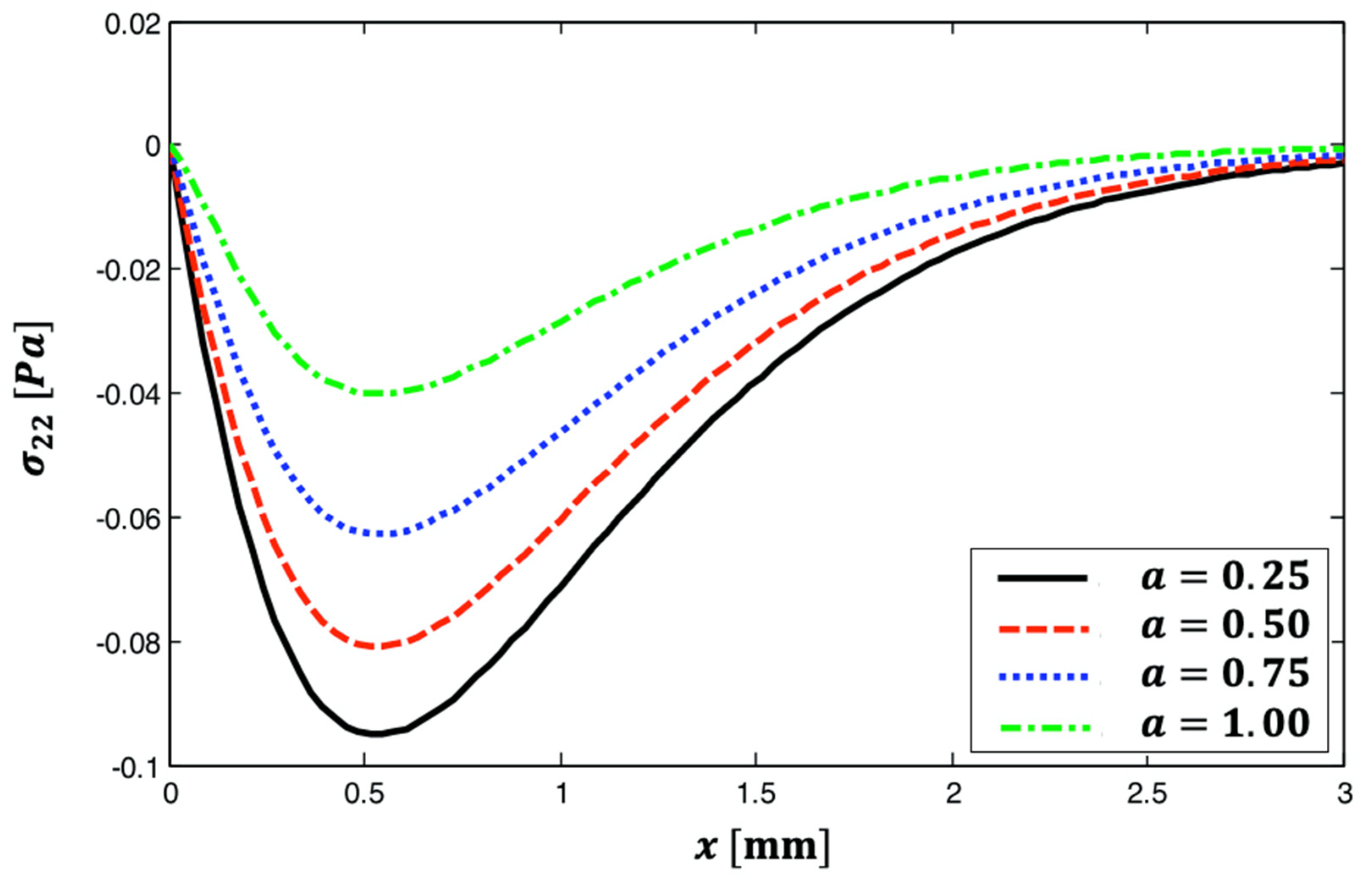
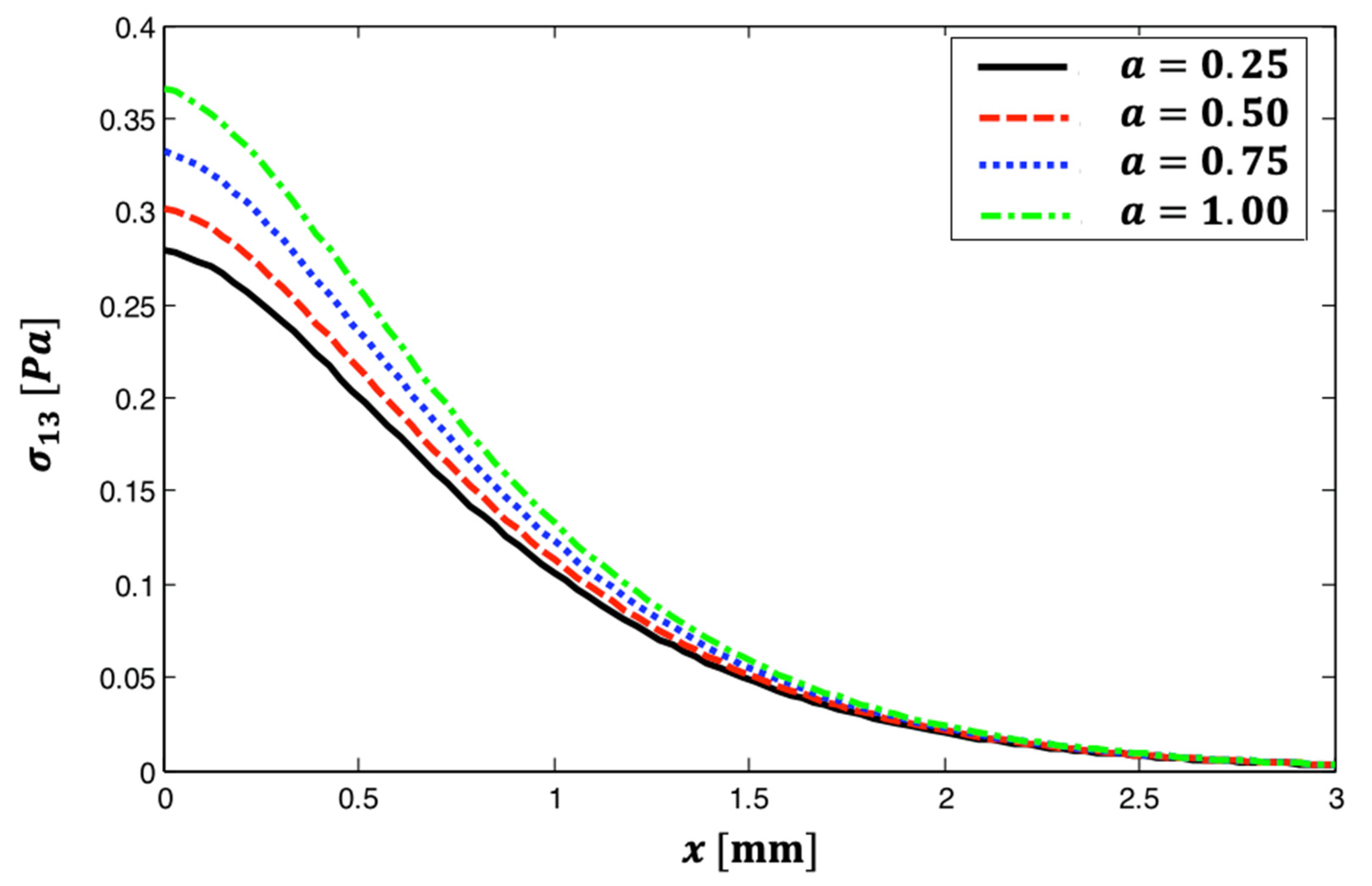
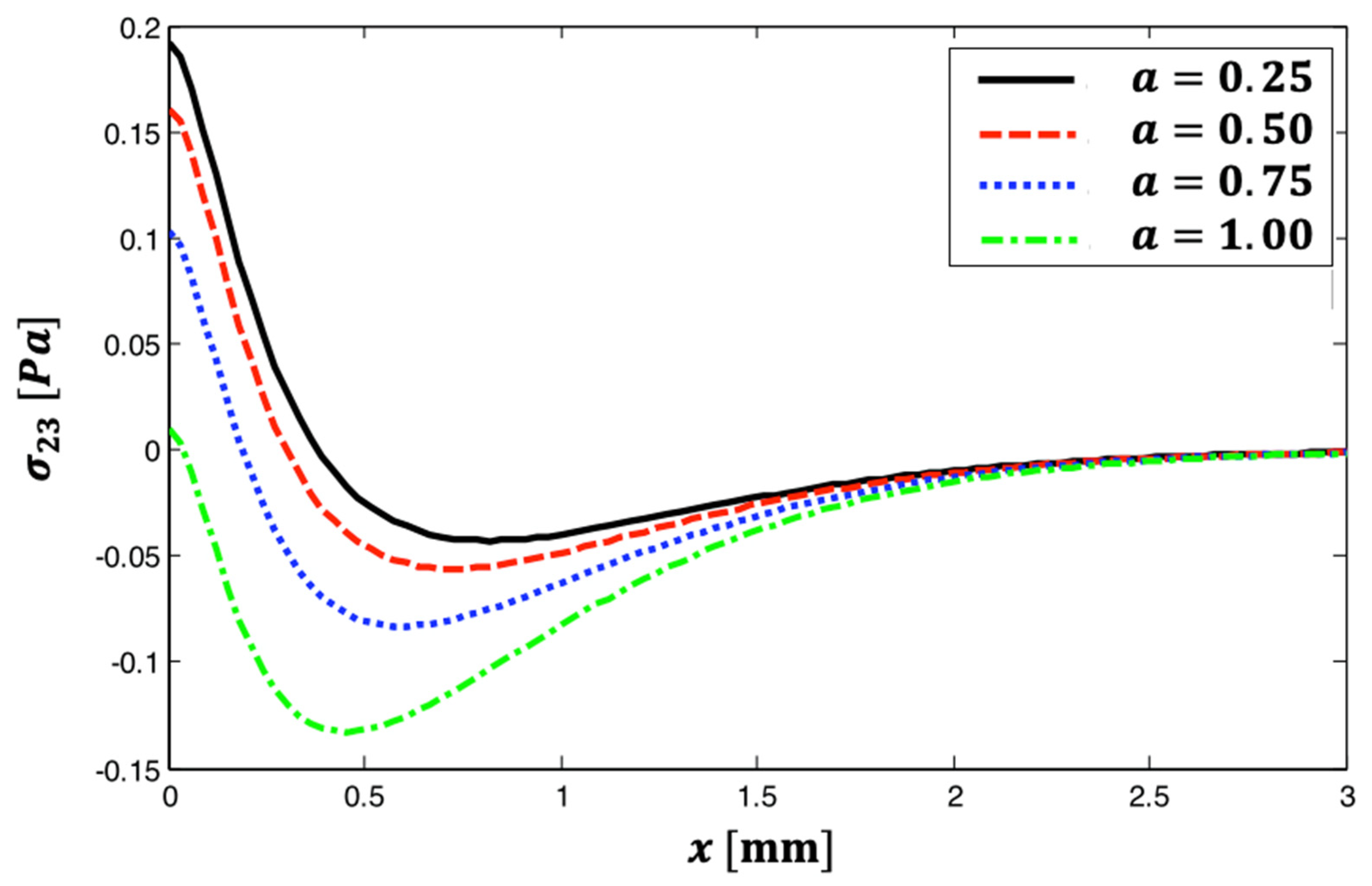
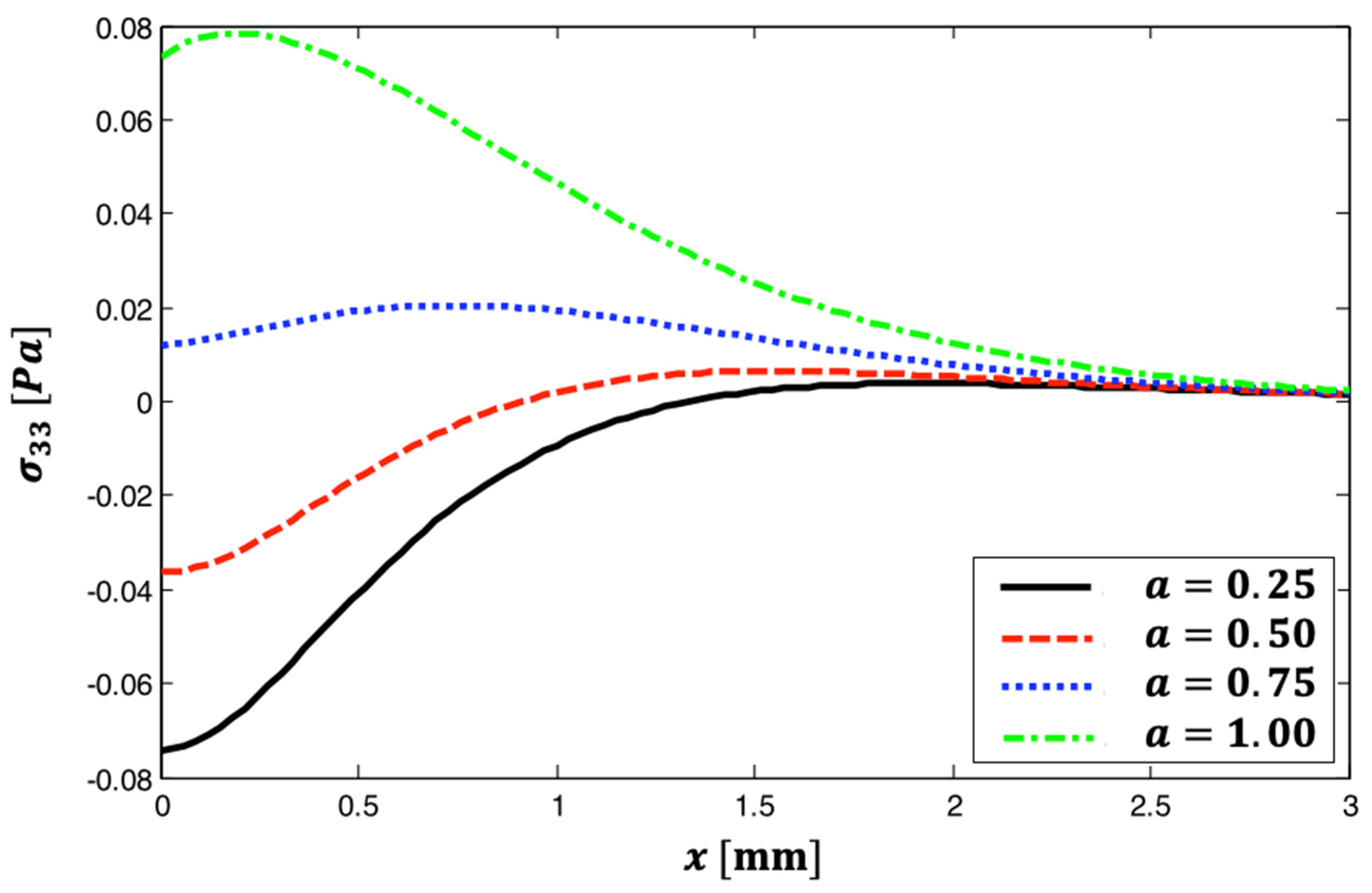
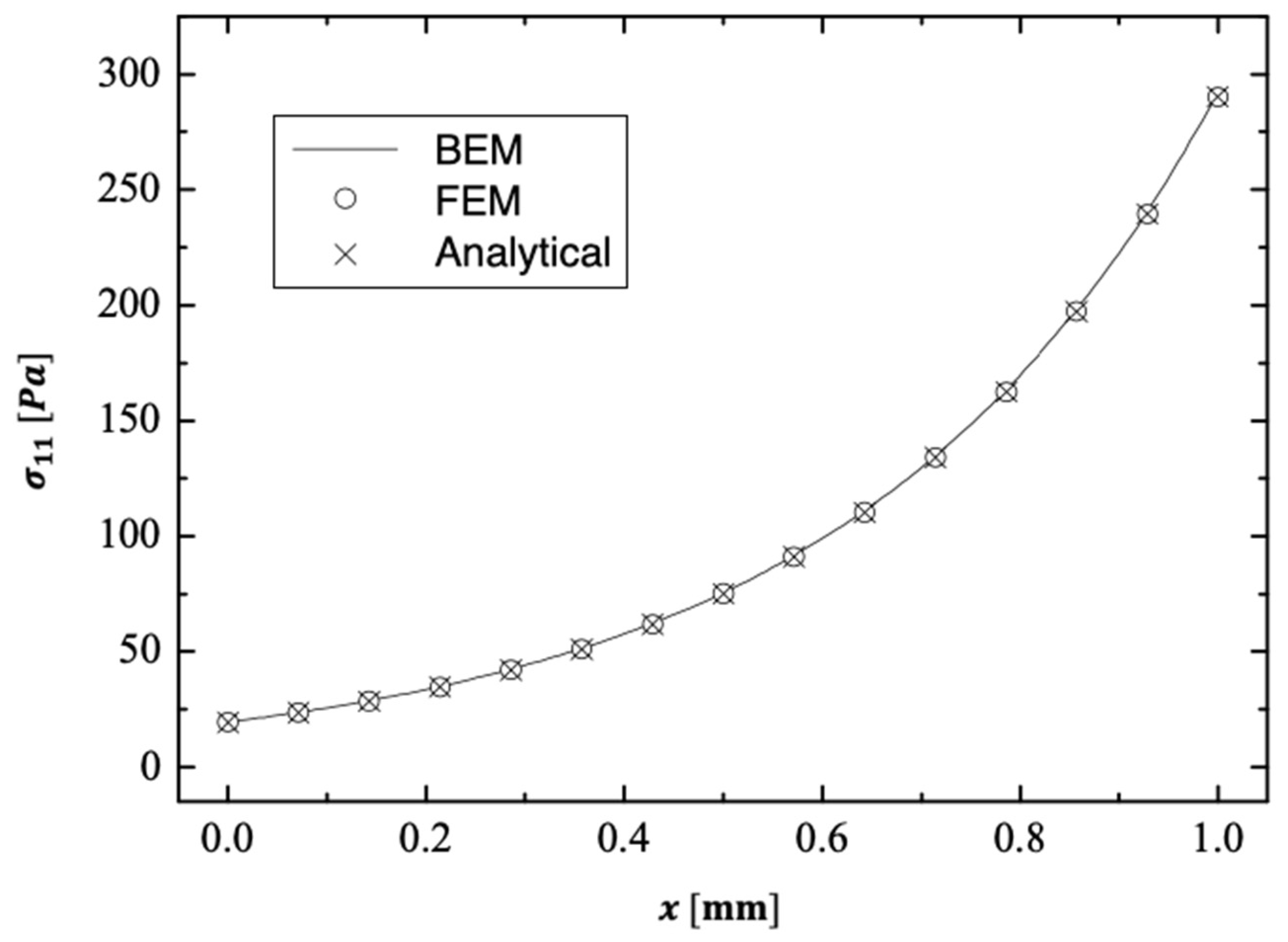
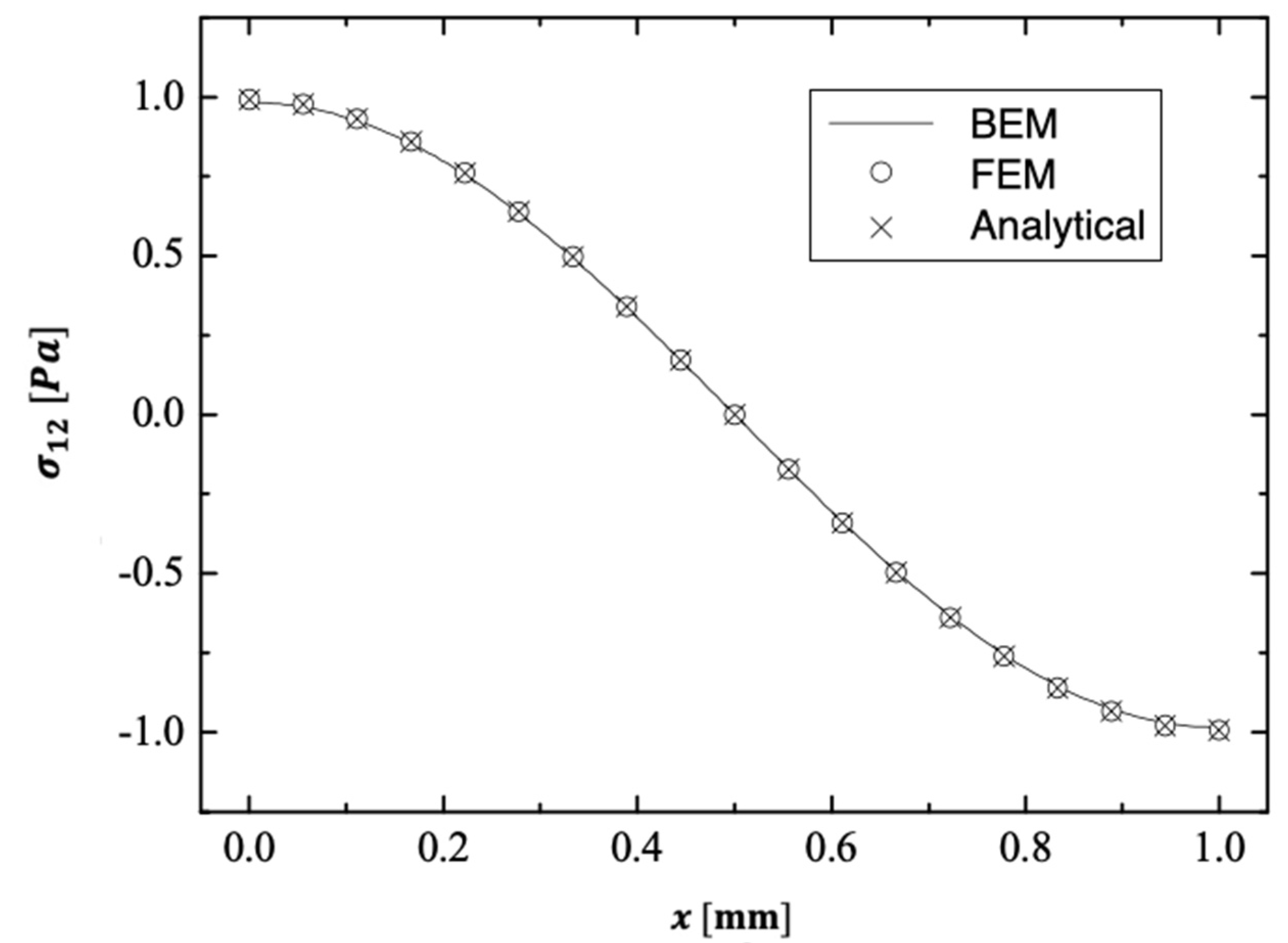
| Discretization Level | Preconditioning Level | 2D-DSPM | MSSOR | TBS | ||||||
|---|---|---|---|---|---|---|---|---|---|---|
| CPU Time | Iteration Number | Error | CPU Time | Iteration Number | Error | CPU Time | Iteration Number | Error | ||
| 1 (36) | 0 | 0.07 | 8 | 0.0099 | 0.08 | 8 | 0.0098 | 0.05 | 8 | 0.0098 |
| 2 (72) | 0 | 0.19 | 9 | 0.0098 | 0.23 | 9 | 0.0097 | 0.16 | 8 | 0.0094 |
| 1 | 0.16 | 7 | 0.0097 | 0.19 | 7 | 0.0096 | 0.11 | 7 | 0.0090 | |
| 3 (144) | 0 | 0.52 | 10 | 0.0095 | 0.62 | 11 | 0.0091 | 0.42 | 10 | 0.0085 |
| 1 | 0.47 | 8 | 0.0092 | 0.52 | 9 | 0.0087 | 0.34 | 6 | 0.0080 | |
| 2 | 0.44 | 6 | 0.0088 | 0.48 | 7 | 0.0084 | 0.30 | 4 | 0.0078 | |
| 4 (288) | 0 | 2.42 | 13 | 0.0090 | 2.52 | 17 | 0.0086 | 1.88 | 11 | 0.0084 |
| 1 | 1.90 | 11 | 0.0084 | 2.10 | 15 | 0.0080 | 1.58 | 7 | 0.0078 | |
| 2 | 1.62 | 7 | 0.0080 | 1.82 | 11 | 0.0078 | 1.42 | 5 | 0.0076 | |
| 3 | 1.42 | 5 | 0.0078 | 1.52 | 7 | 0.0076 | 1.38 | 3 | 0.0074 | |
| 5 (576) | 0 | 9.98 | 15 | 0.0088 | 12.04 | 19 | 0.0084 | 7.84 | 13 | 0.0082 |
| 1 | 9.12 | 11 | 0.0084 | 10.12 | 17 | 0.0082 | 6.82 | 9 | 0.0080 | |
| 2 | 8.20 | 9 | 0.0082 | 9.32 | 15 | 0.0076 | 6.12 | 7 | 0.0075 | |
| 3 | 7.26 | 7 | 0.0080 | 8.48 | 11 | 0.0074 | 5.84 | 5 | 0.0073 | |
| 4 | 6.46 | 5 | 0.0078 | 7.01 | 7 | 0.0072 | 5.20 | 3 | 0.0070 | |
| 6 (1152) | 0 | 40.4 | 19 | 0.0086 | 46.6 | 21 | 0.0082 | 34.4 | 15 | 0.0080 |
| 1 | 36.3 | 17 | 0.0084 | 43.0 | 19 | 0.0080 | 32.6 | 11 | 0.0078 | |
| 2 | 33.9 | 15 | 0.0082 | 41.0 | 17 | 0.0078 | 30.8 | 9 | 0.0076 | |
| 3 | 29.0 | 11 | 0.0080 | 34.9 | 13 | 0.0076 | 29.0 | 7 | 0.0074 | |
| 4 | 26.8 | 9 | 0.0076 | 32.8 | 11 | 0.0074 | 22.9 | 5 | 0.0072 | |
| 5 | 24.9 | 7 | 0.0074 | 29.0 | 9 | 0.0072 | 21.1 | 3 | 0.0070 | |
| BEM | FEM | |
|---|---|---|
| Number of nodes | 54 | 56,000 |
| Number of elements | 18 | 9000 |
| CPU time [min] | 2 | 200 |
| Memory [Mbyte] | 1 | 140 |
| Disk space [Mbyte] | 0 | 260 |
| Accuracy of results [%] | 1.0 | 2.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fahmy, M.A.; Toujani, M. A New Fractional Boundary Element Model for the 3D Thermal Stress Wave Propagation Problems in Anisotropic Materials. Math. Comput. Appl. 2025, 30, 6. https://doi.org/10.3390/mca30010006
Fahmy MA, Toujani M. A New Fractional Boundary Element Model for the 3D Thermal Stress Wave Propagation Problems in Anisotropic Materials. Mathematical and Computational Applications. 2025; 30(1):6. https://doi.org/10.3390/mca30010006
Chicago/Turabian StyleFahmy, Mohamed Abdelsabour, and Moncef Toujani. 2025. "A New Fractional Boundary Element Model for the 3D Thermal Stress Wave Propagation Problems in Anisotropic Materials" Mathematical and Computational Applications 30, no. 1: 6. https://doi.org/10.3390/mca30010006
APA StyleFahmy, M. A., & Toujani, M. (2025). A New Fractional Boundary Element Model for the 3D Thermal Stress Wave Propagation Problems in Anisotropic Materials. Mathematical and Computational Applications, 30(1), 6. https://doi.org/10.3390/mca30010006






