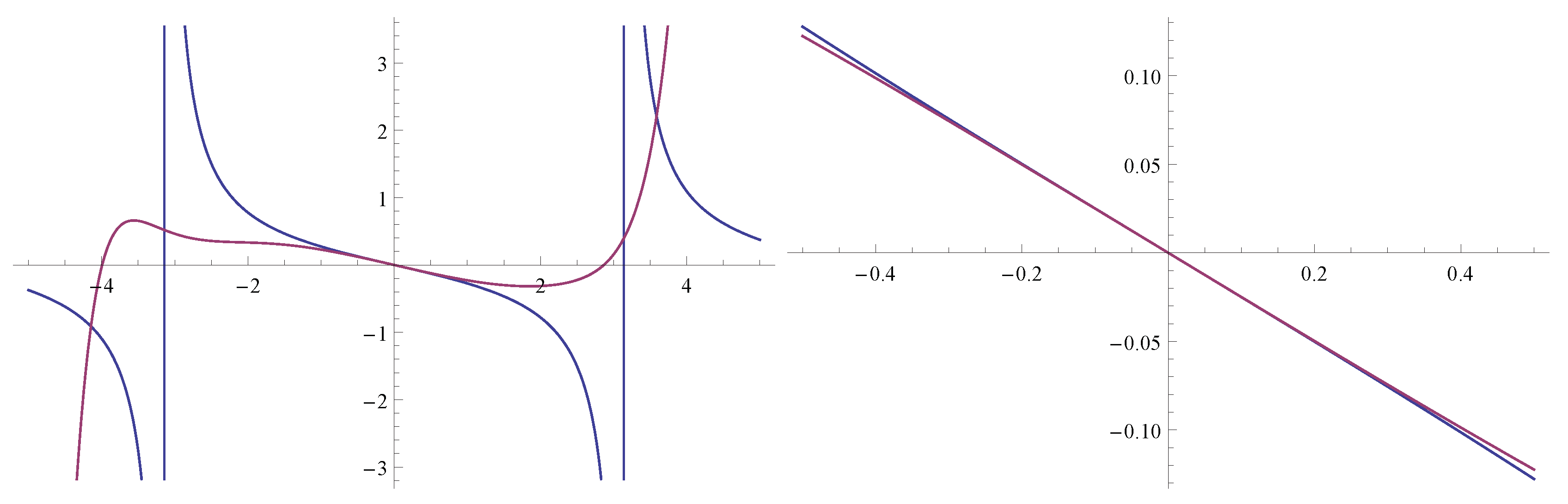Chaos and Relativistic Energy-Momentum of the Nonlinear Time Fractional Duffing Equation
Abstract
:1. Introduction
2. Application
2.1. Exp -Expansion Method
2.2. Improved F-Expansion Method
2.3. Extended -Expansion Method
2.4. Extended Tanh-Function Method
2.5. Simplest Equation Method
2.6. Extended Simplest Equation Method
2.7. Extended Fan-Expansion Method
2.8. Generalized Kudryashov Method
2.9. Generalized Riccati Expansion Method
2.10. Generalized Sinh-Gordon Expansion Method
2.11. Modified Khater Method
2.12. Adomian Decomposition Method
3. Stability Analysis
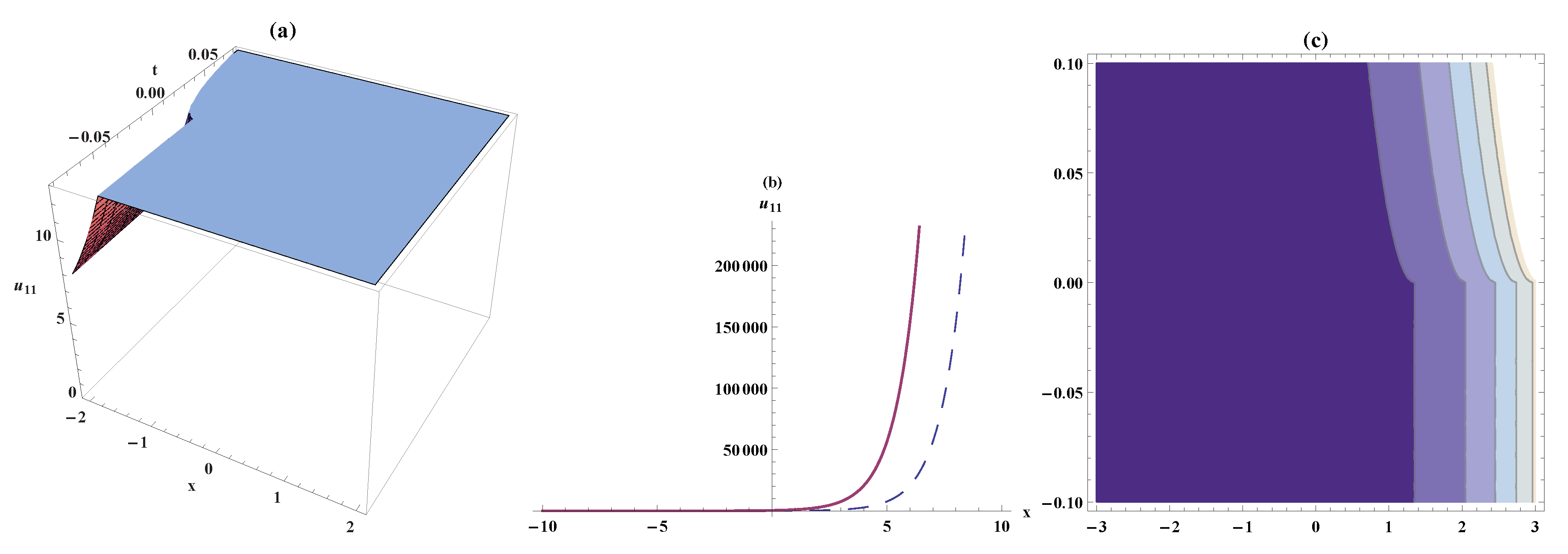
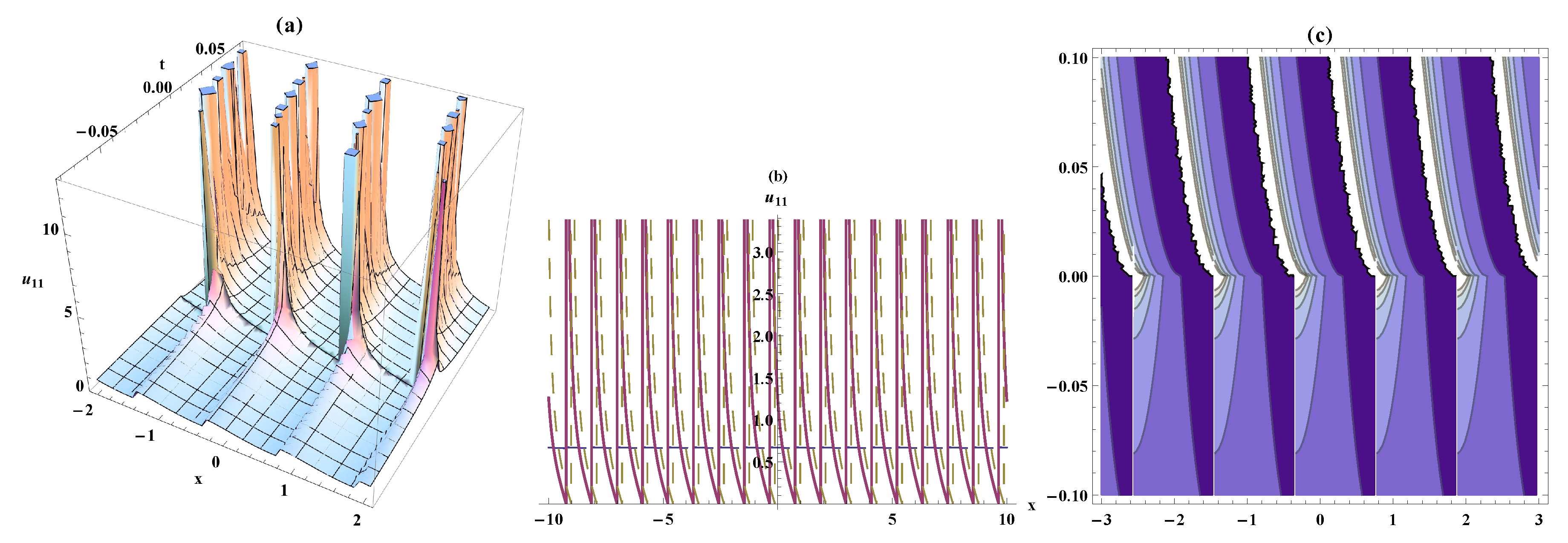
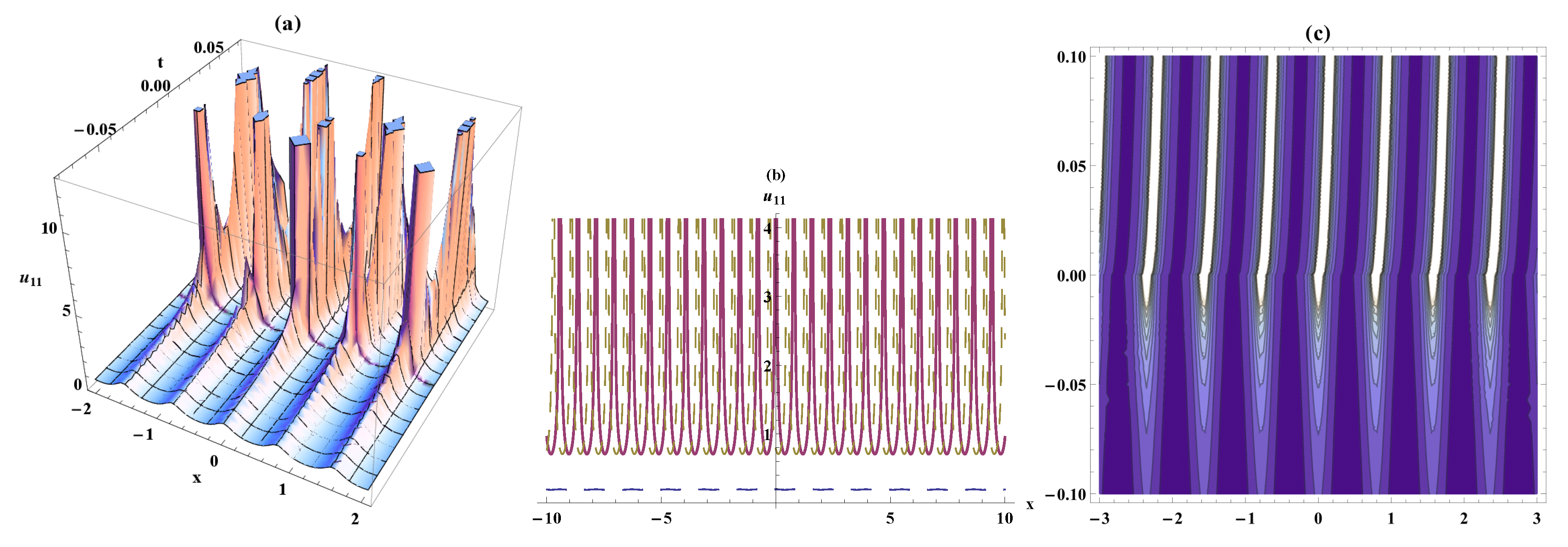
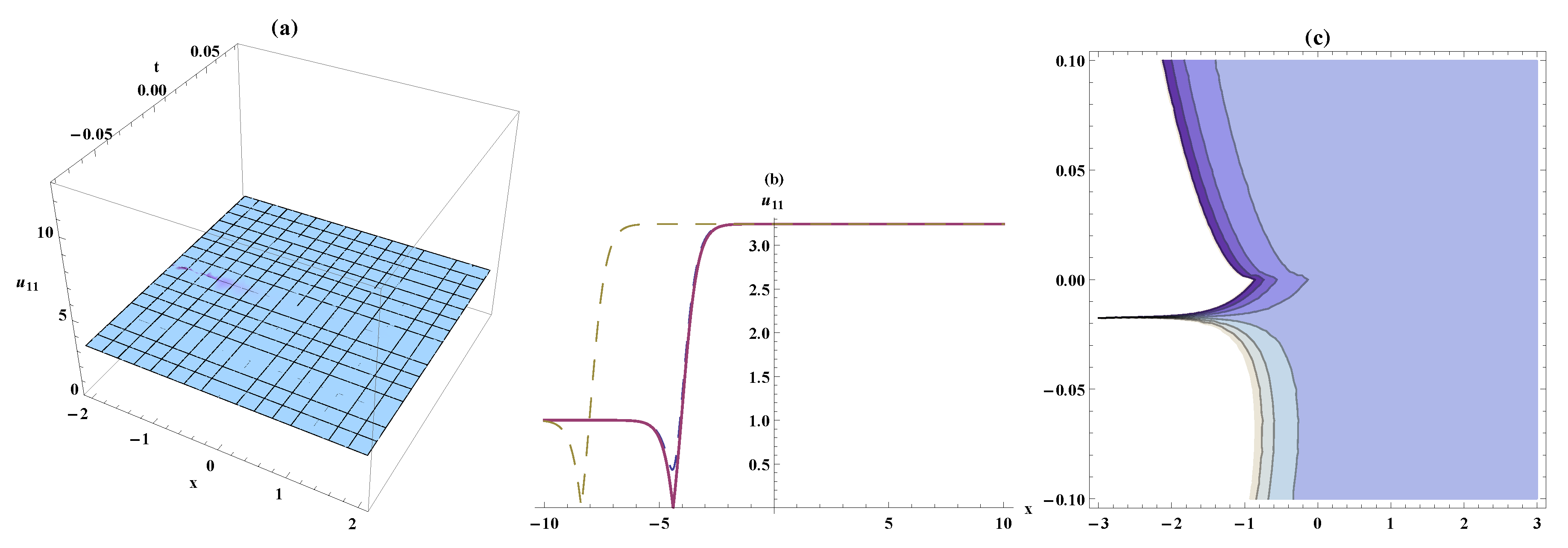
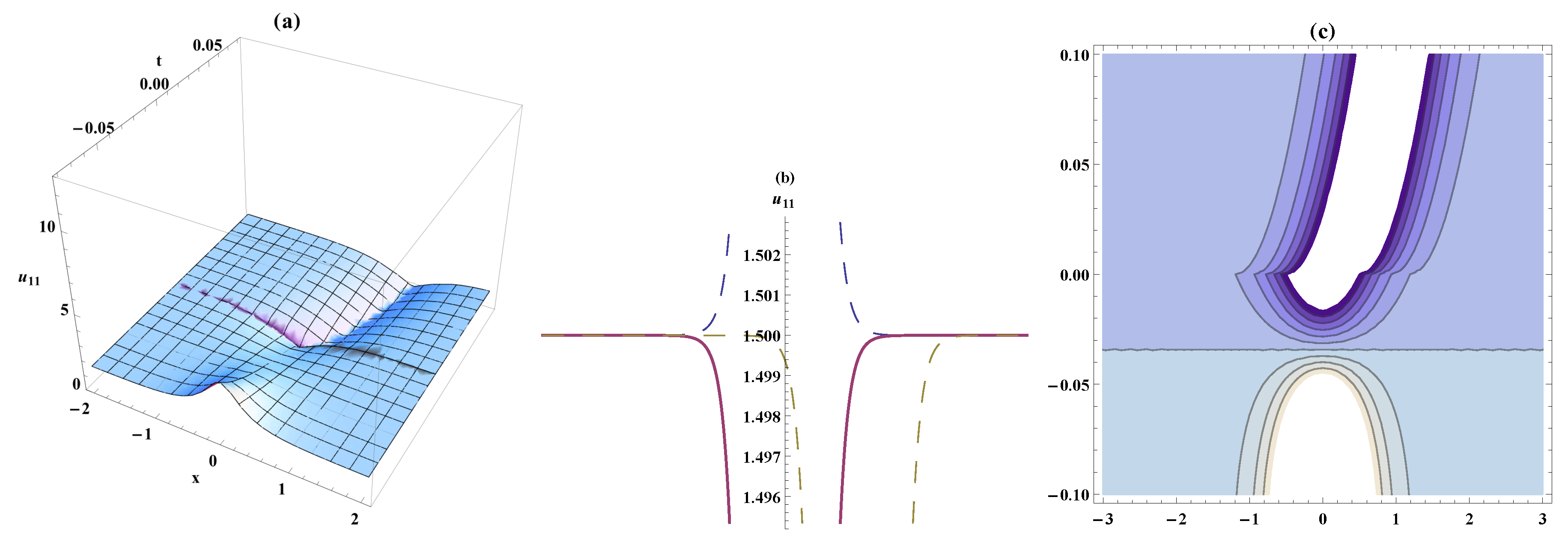
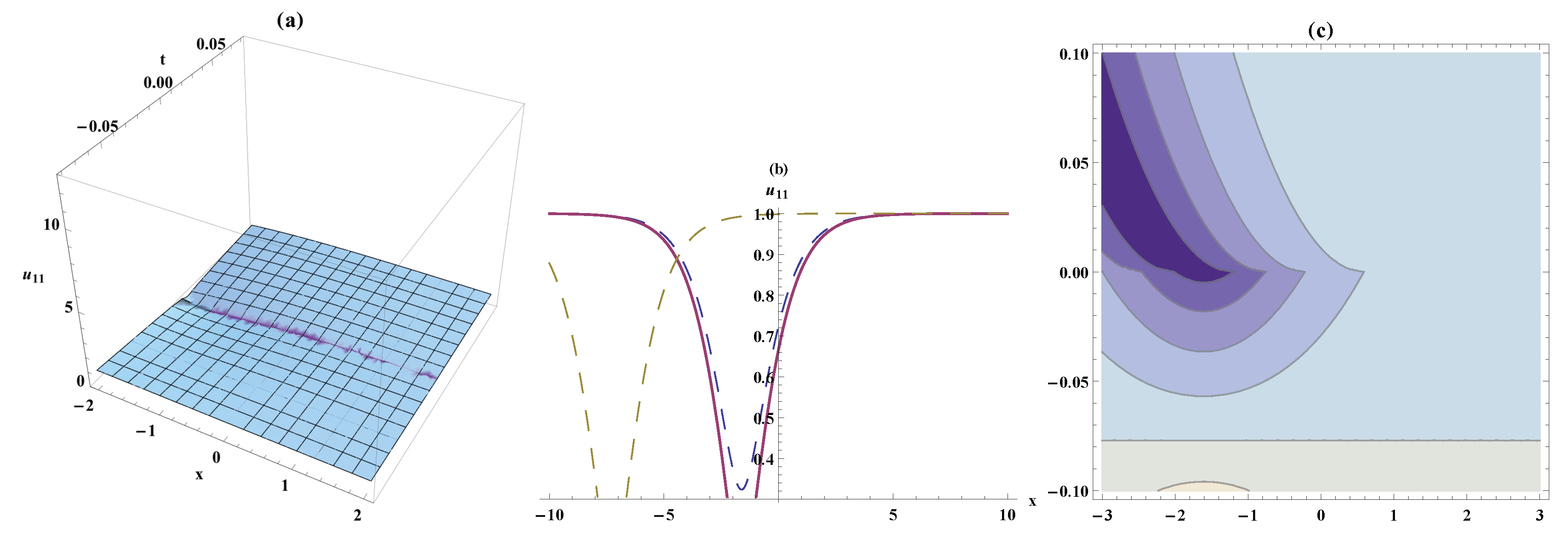
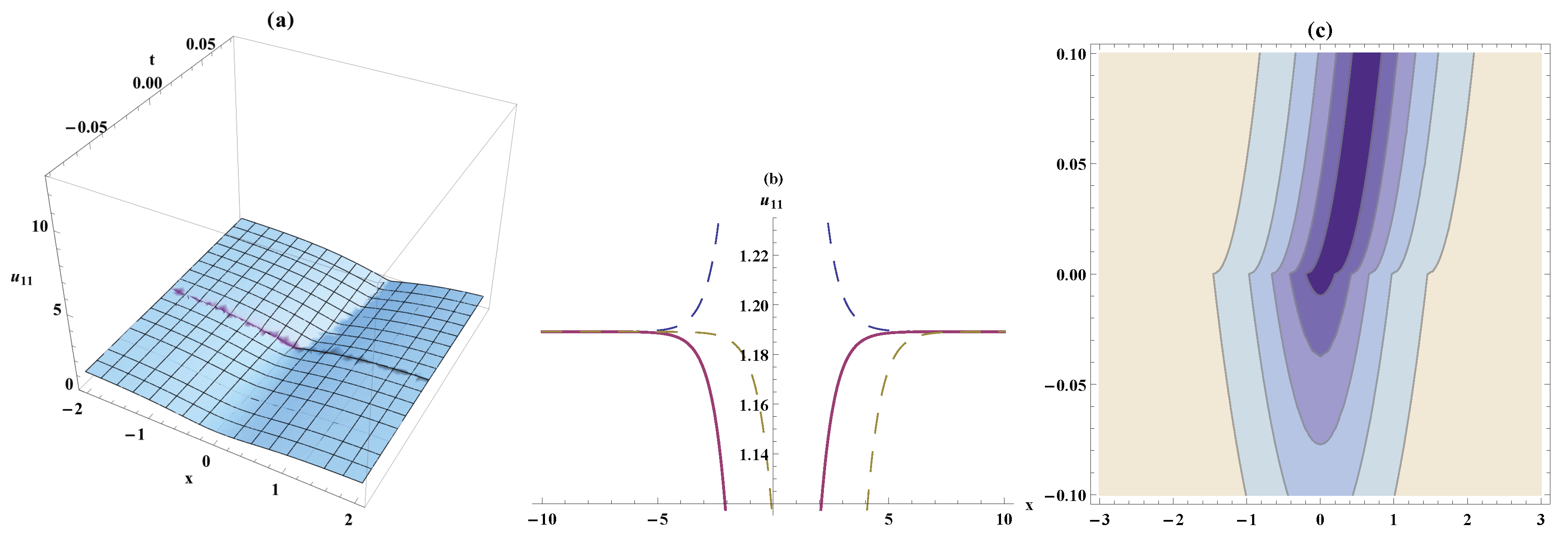
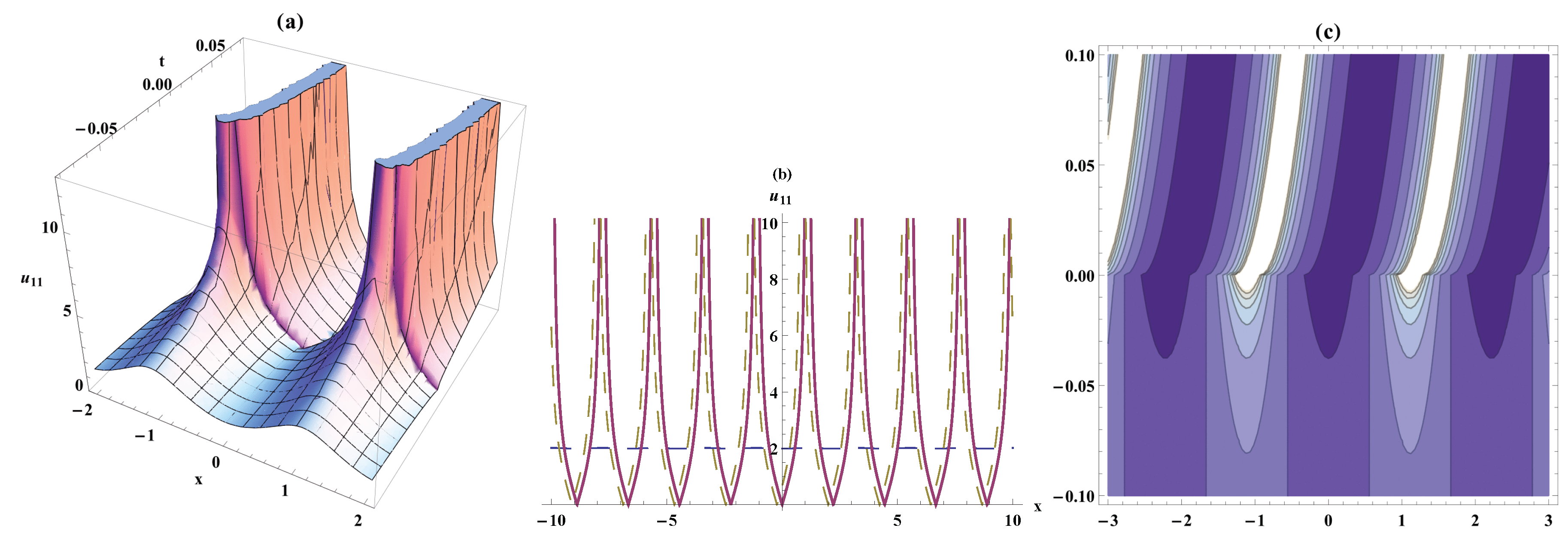
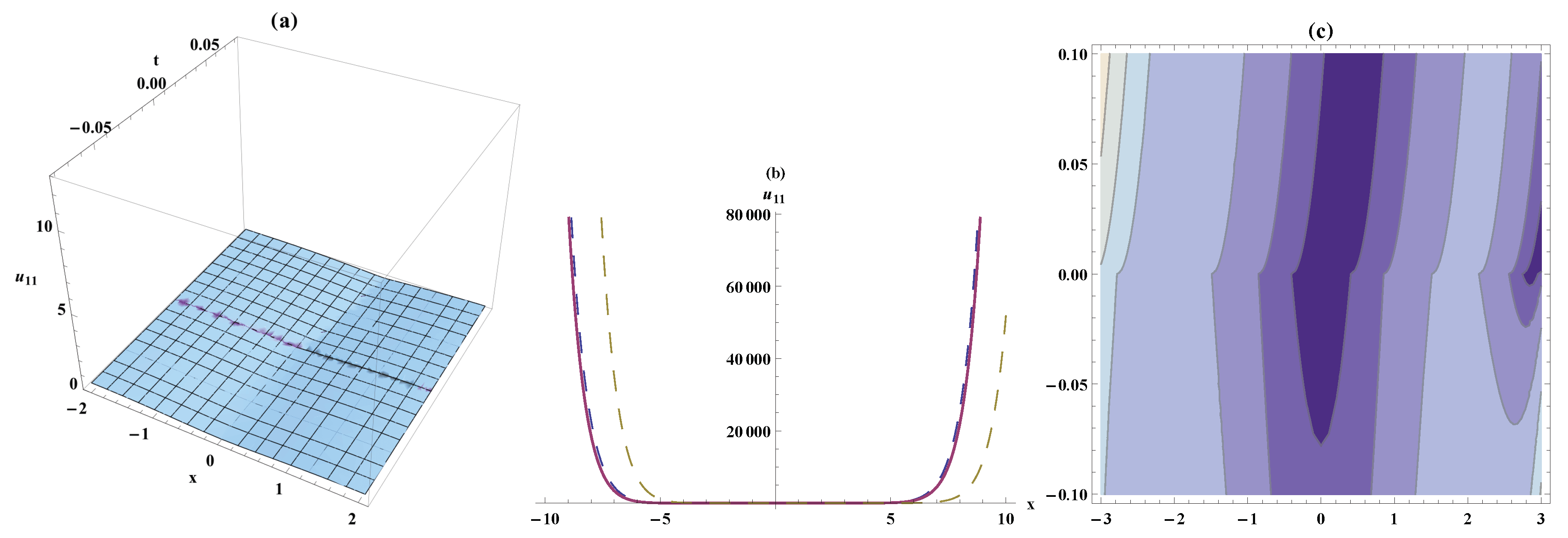
4. Results and Discussion
- In Reference [5], Akbar et al. applied the generalized -expansion method; here, we also applied an extended model of the -expansion method. Applying Akbar’s technique permits us to get five various formulae of solutions; our extended method allows us to get three different solutions. Our obtained solutions differ completely from those in Reference [5]. That means we succeeded in obtaining a new formula of solutions for our model.
- This convergence of solutions for the fractional nonlinear Duffing equation shows the accuracy of our obtained solutions.
- Table 1 shows the convergence between exact and approximate solutions. The absolute value of error illustrates this convergence.
- Table 1 shows the accuracy of the Adomian decomposition method in the period that is near to zero, like .
- According to the solutions that were obtained by the modified Khater method (modified auxiliary equation method) [30], this is considered one of the most general methods in this field, since it covers many of solutions that were obtained by other methods and is also able to obtain more novel and different solutions than other methods.
5. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Kovacic, I.; Brennan, M.J. The Duffing Equation: Nonlinear Oscillators and their Behaviour; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Wang, Y.; An, J.Y. Amplitude-frequency relationship to a fractional Duffing oscillator arising in microphysics and tsunami motion. J. Low Freq. Noise Vib. Act. Control 2018. [Google Scholar] [CrossRef]
- Yusufoǧlu, E. Numerical solution of Duffing equation by the Laplace decomposition algorithm. Appl. Math. Comput. 2006, 177, 572–580. [Google Scholar]
- Daino, B.; Gregori, G.; Wabnitz, S. Stability analysis of nonlinear coherent coupling. J. Appl. Phys. 1985, 58, 4512–4514. [Google Scholar] [CrossRef]
- Akbar, M.A.; Ali, N.H.M.; Roy, R. Closed form solutions of two time fractional nonlinear wave equations. Results Phys. 2018, 9, 1031–1039. [Google Scholar] [CrossRef]
- Zahran, E.H.; Khater, M.M. Modified extended tanh-function method and its applications to the Bogoyavlenskii equation. Appl. Math. Model. 2016, 40, 1769–1775. [Google Scholar] [CrossRef]
- Abdou, M.A. A generalized auxiliary equation method and its applications. Nonlinear Dyn. 2008, 52, 95–102. [Google Scholar] [CrossRef]
- Abdou, M.A. An analytical method for space-time fractional nonlinear differential equations arising in plasma physics. J. Ocean Eng. Sci. 2017, 2, 288–292. [Google Scholar] [CrossRef]
- Abdou, M.A. New periodic solitary wave solutions for a variable-coefficient gardner equation from fluid dynamics and plasma physics. Appl. Math. 2010, 1, 307. [Google Scholar] [CrossRef]
- Abdou, M.A.; Abulwafa, E.M. The Three-wave Method and its Applications. Nonlinear Sci. Lett. A 2010, 1, 373–378. [Google Scholar]
- Abdou, M.A. On the fractional order space-time nonlinear equations arising in plasma physics. Indian J. Phys. 2018, 1–5. [Google Scholar] [CrossRef]
- Abdou, M.A. An analytical approach for space-time fractal order nonlinear dynamics of micro tubules. Waves Random Complex Media 2018, 1–8. [Google Scholar] [CrossRef]
- Biswas, A.; Fessak, M.; Johnson, S.; Beatrice, S.; Milovic, D.; Jovanoski, Z.; Kohl, R.; Majid, F. Optical soliton perturbation in non-Kerr law media: Traveling wave solution. Opt. Laser Technol. 2012, 44, 263–268. [Google Scholar] [CrossRef]
- Khater, M.M.A. Exact traveling wave solutions for the generalized Hirota–Satsuma couple KdV system using the exp (−ϕ(ξ))-expansion method. Cogent Math. 2016, 3, 1172397. [Google Scholar] [CrossRef]
- Rezazadeh, H.; Korkmaz, A.; Eslami, M.; Vahidi, J.; Asghari, R. Traveling wave solution of conformable fractional generalized reaction Duffing model by generalized projective Riccati equation method. Opt. Quantum Electron. 2018, 50, 150. [Google Scholar] [CrossRef]
- Eslami, M.; Rezazadeh, H. The first integral method for Wu-Zhang system with conformable time-fractional derivative. Calcolo 2016, 53, 475–485. [Google Scholar] [CrossRef]
- Eslami, M. Exact traveling wave solutions to the fractional coupled nonlinear Schrödinger equations. Appl. Math. Comput. 2016, 285, 141–148. [Google Scholar] [CrossRef]
- Eslami, M. Trial solution technique to chiral nonlinear Schrödinger’s equation in (1+2)-dimensions. Nonlinear Dyn. 2016, 85, 813–816. [Google Scholar] [CrossRef]
- Attia, R.A.; Lu, D.; Khater, M.M. Structure of New Solitary Solutions for The Schwarzian Korteweg De Varies Equation And (2+1)-Ablowitz–Kaup–Newell–Segur Equation. Phys. J. 2018, 1, 3. [Google Scholar]
- Liu, J.G.; Eslami, M.; Rezazadeh, H.; Mirzazadeh, M. Rational solutions and lump solutions to a non-isospectral and generalized variable-coefficient Kadomtsev–Petviashvili equation. Nonlinear Dyn. 2018, 1–7. [Google Scholar] [CrossRef]
- Wazwaz, A.-M. The tanh method for traveling wave solutions of nonlinear equations. Appl. Math. Comput. 2004, 154, 713–723. [Google Scholar] [CrossRef]
- Liu, J.; Xu, D.; Du, Z. Traveling Wave Solution of a Reaction–Diffusion Predator–Prey System. Qual. Theory Dyn. Syst. 2018, 1–11. [Google Scholar] [CrossRef]
- Taha, W.M.; Aziz, S.I.; Hameed, R.A.; Taha, I.M. New traveling wave solutions of a nonlinear diffusion-convection equation by using standard tanh method. Tikrit J. Pure Sci. 2018, 23, 143–147. [Google Scholar] [CrossRef]
- Khater, M.M.A. The modified simple equation method and its applications in mathematical physics and biology. Glob. J. Sci. Front. Res. F Math. Decis. Sci. 2015, 15, 69–85. [Google Scholar]
- Hosseini, K.; Mayeli, P.; Ansari, R. Modified Kudryashov method for solving the conformable time-fractional Klein-Gordon equations with quadratic and cubic nonlinearities. Opt. Int. J. Light Electron Opt. 2017, 130, 737–742. [Google Scholar] [CrossRef]
- Eslami, M.; Khodadad, F.S.; Nazari, F.; Rezazadeh, H. The first integral method applied to the Bogoyavlenskii equations by means of conformable fractional derivative. Opt. Quantum Electron. 2017, 49, 391. [Google Scholar] [CrossRef]
- Zhao, D.; Luo, M. General conformable fractional derivative and its physical interpretation. Calcolo 2017, 54, 903–917. [Google Scholar] [CrossRef]
- Ilie, M.; Biazar, J.; Ayati, Z. General solution of Bernoulli and Riccati fractional differential equations based on conformable fractional derivative. Int. J. Appl. Math. Res. 2017, 6, 49–51. [Google Scholar]
- Mozaffari, F.S.; Hassanabadi, H.; Sobhani, H.; Chung, W.S. Investigation of the Dirac Equation by Using the Conformable Fractional Derivative. J. Korean Phys. Soc. 2018, 72, 987–990. [Google Scholar] [CrossRef]
- Khater, M.; Attia, R.; Lu, D. Modified Auxiliary Equation Method versus Three Nonlinear Fractional Biological Models in Present Explicit Wave Solutions. Math. Comput. Appl. 2019, 24, 1. [Google Scholar] [CrossRef]
- Bibi, S.; Mohyud-Din, S.T.; Khan, U.; Ahmed, N. Khater method for nonlinear Sharma Tasso-Olever (STO) equation of fractional order. Results Phys. 2017, 7, 4440–4450. [Google Scholar] [CrossRef]



| Value of | Exact Solution | Approximate Solution | Error |
|---|---|---|---|
| 0.00250002 | 0.00249998 | 4.1667 × 10 | |
| 0.00500017 | 0.00499983 | 3.33343 × 10 | |
| 0.00750056 | 0.00749944 | 1.12508 × 10 | |
| 0.0100013 | 0.00999867 | 2.66699 × 10 | |
| 0.0125026 | 0.0124974 | 5.20931 × 10 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Attia, R.A.M.; Lu, D.; M. A. Khater, M. Chaos and Relativistic Energy-Momentum of the Nonlinear Time Fractional Duffing Equation. Math. Comput. Appl. 2019, 24, 10. https://doi.org/10.3390/mca24010010
Attia RAM, Lu D, M. A. Khater M. Chaos and Relativistic Energy-Momentum of the Nonlinear Time Fractional Duffing Equation. Mathematical and Computational Applications. 2019; 24(1):10. https://doi.org/10.3390/mca24010010
Chicago/Turabian StyleAttia, Raghda A. M., Dianchen Lu, and Mostafa M. A. Khater. 2019. "Chaos and Relativistic Energy-Momentum of the Nonlinear Time Fractional Duffing Equation" Mathematical and Computational Applications 24, no. 1: 10. https://doi.org/10.3390/mca24010010
APA StyleAttia, R. A. M., Lu, D., & M. A. Khater, M. (2019). Chaos and Relativistic Energy-Momentum of the Nonlinear Time Fractional Duffing Equation. Mathematical and Computational Applications, 24(1), 10. https://doi.org/10.3390/mca24010010