The Effect of Initial Water Content and Density on the Swelling-Shrinkage and Cracking Characteristics of Compacted Clay
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Specimen Preparation and Test Methods
2.2.1. Expansion Test
2.2.2. Drying-Shrinkage Test
2.2.3. Cracking Test after Wetting-Drying Cycles
2.2.4. Micropore Test
3. Results and Discussion
3.1. The Expansion Characteristics
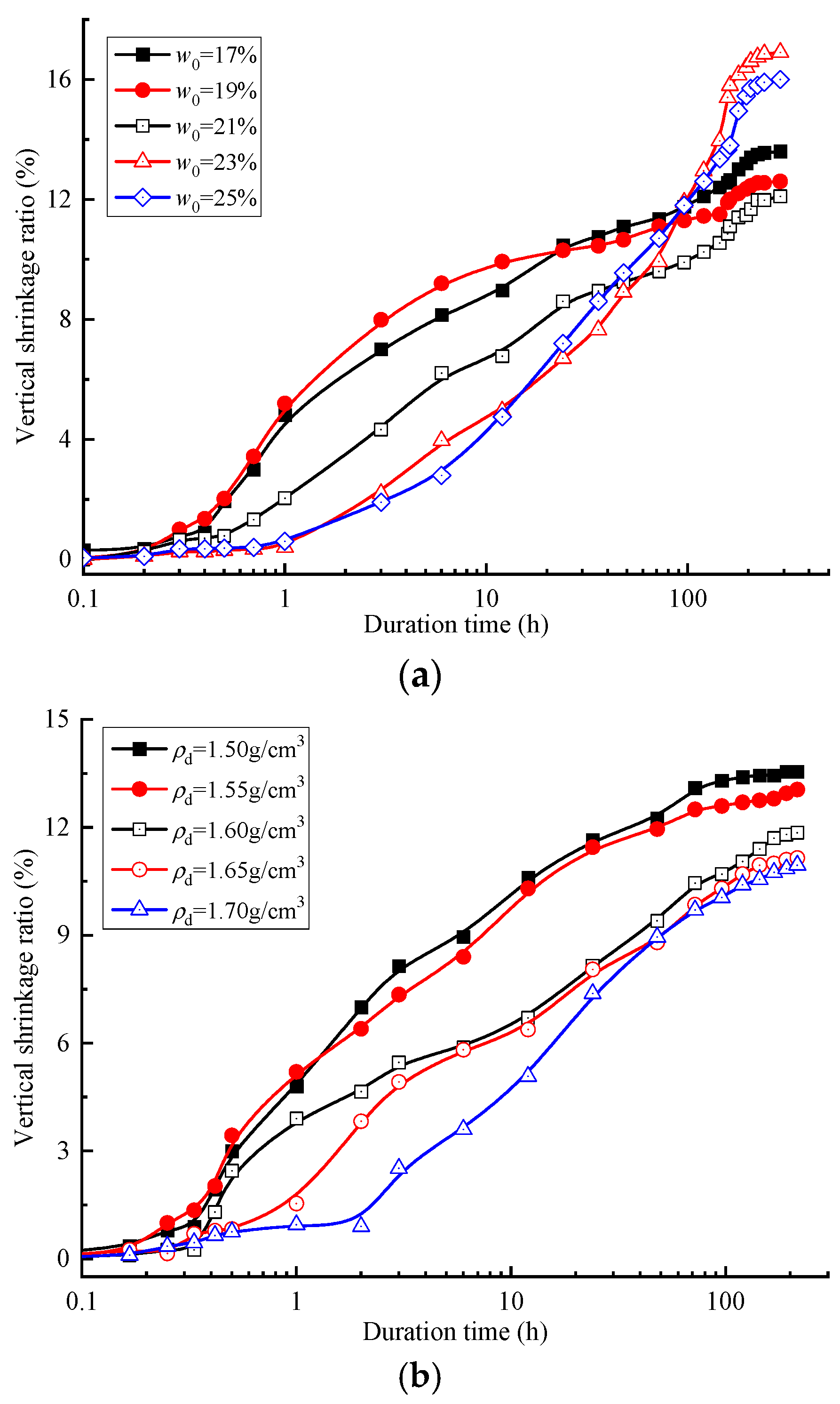
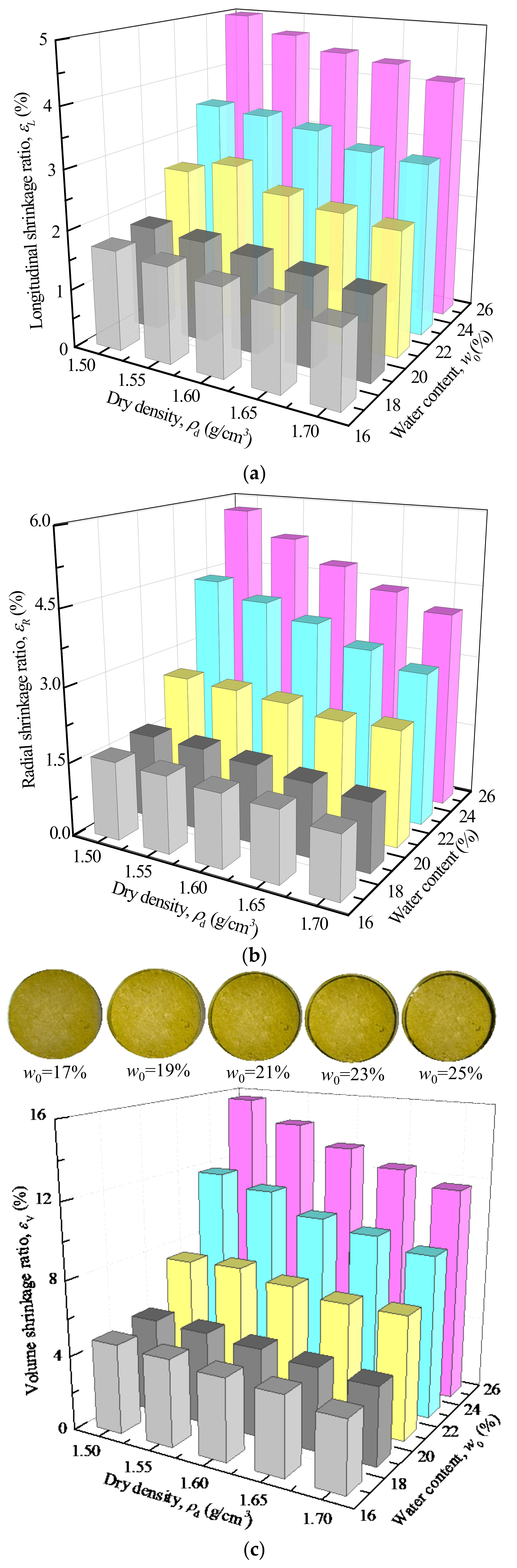
3.2. Dehydration-Shrinkage Characteristics
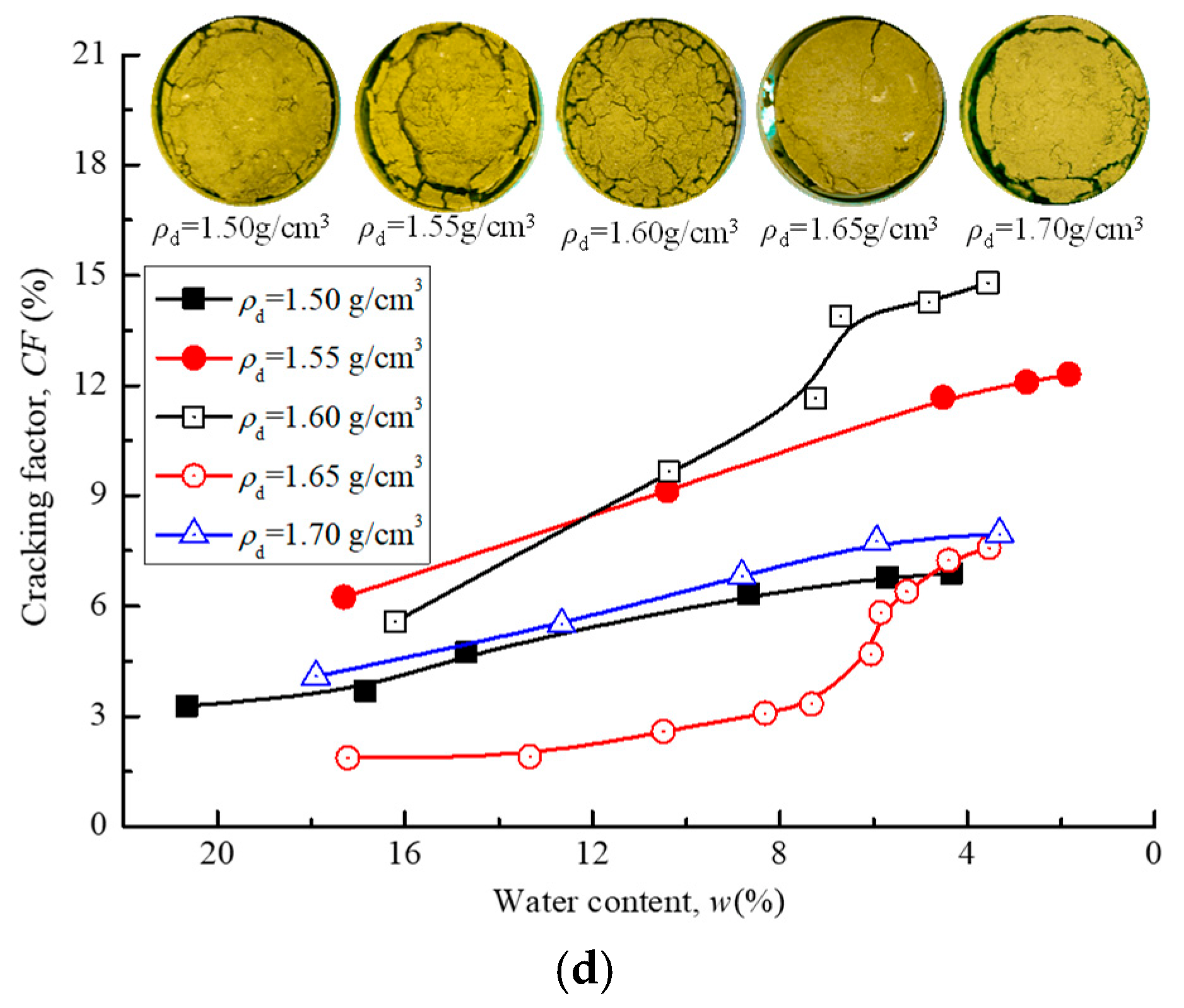
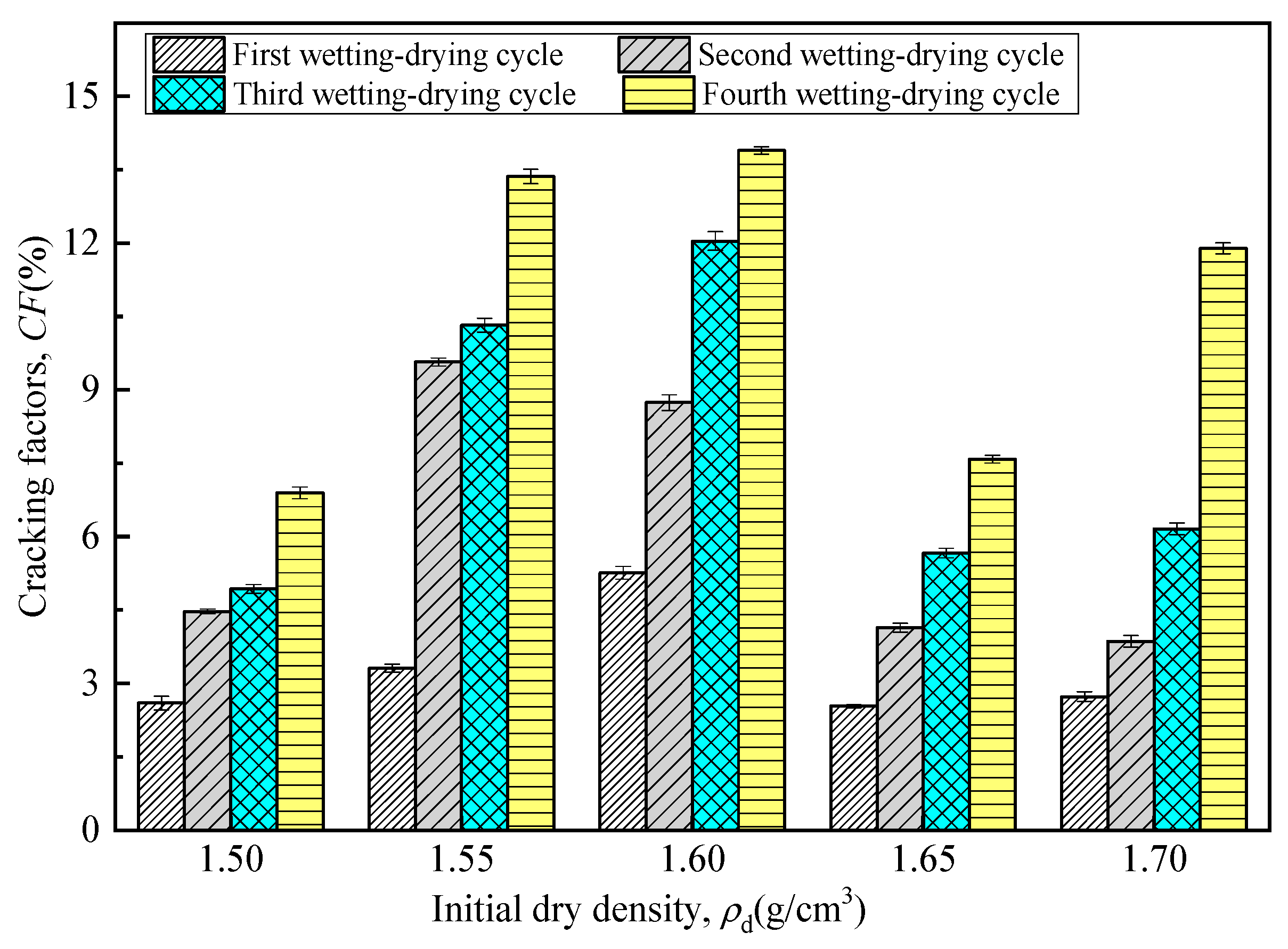
3.3. Cracking Characteristics
3.3.1. Influence of Initial Water Content
3.3.2. Influence of Initial Dry Density
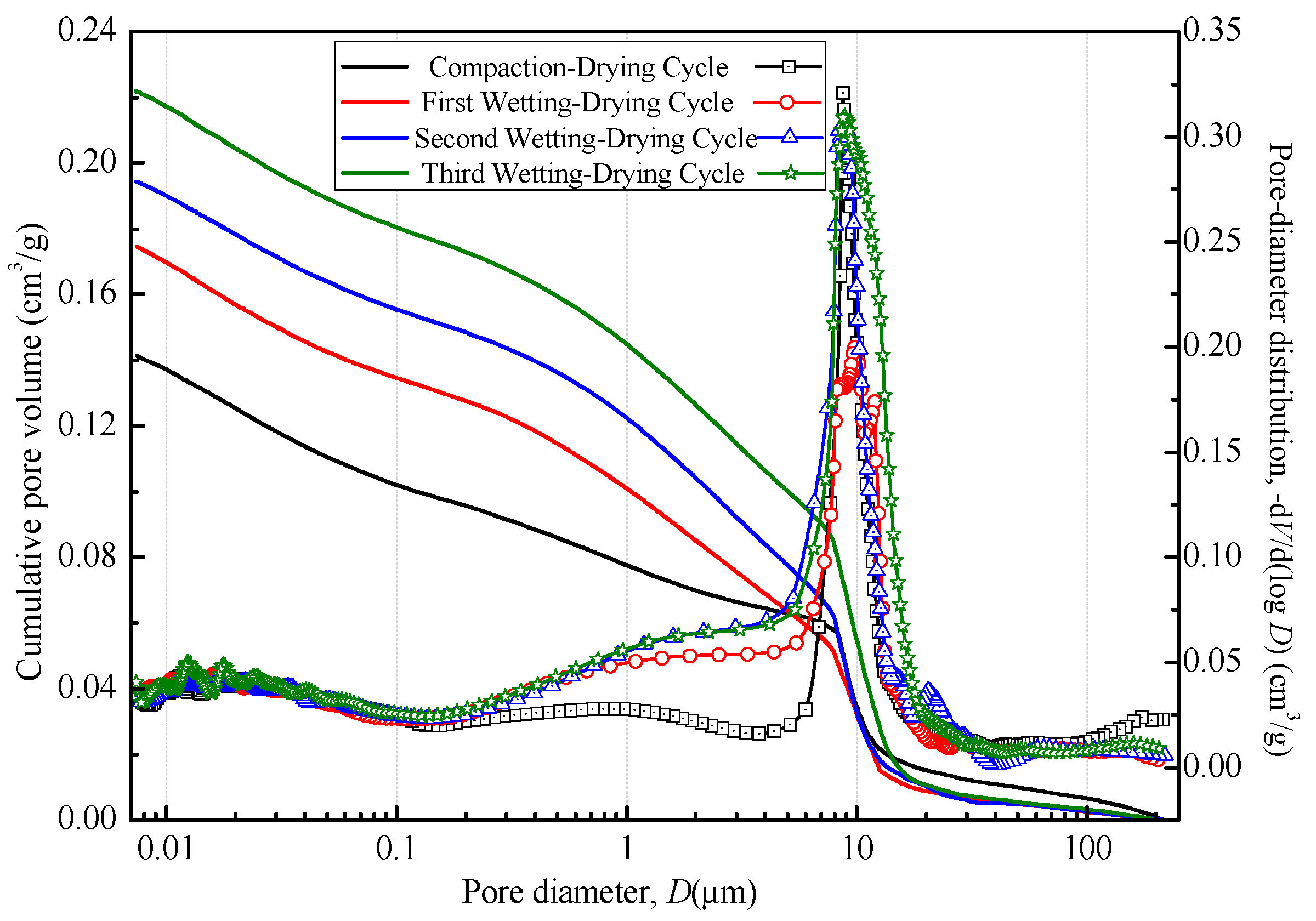
3.4. Microscopic Characteristics
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Morris, P.H.; Graham, J.; Williams, D.J. Cracking in drying soils. Can. Geotech. J. 1992, 29, 263–277. [Google Scholar] [CrossRef]
- Sadek, S.; Ghanimeh, S.; El-Fadel, M. Predicted performance of clay-barrier landfill covers in arid and semi-arid environments. Waste Manag. 2007, 27, 572–583. [Google Scholar] [CrossRef] [PubMed]
- Bouazza, A.; Vangpaisal, T.; Abuel-Naga, H.; Kodikara, J. Analytical modeling of gas leakage rate through a geosynthetic clay liner–geomembrane composite liner due to a circular defect in the geomembrane. Geotext. Geomembr. 2008, 26, 122–129. [Google Scholar] [CrossRef]
- El-Zein, A.; Rowe, R.K. Impact on groundwater of concurrent leakage and diffusion of dichloromethane through geomembranes in landfill liners. Geosynth. Int. 2008, 15, 55–71. [Google Scholar] [CrossRef]
- Saidi, F.; Touze-Foltz, N.; Goblet, P. Numerical modeling of advective flow through composite liners in case of two interacting adjacent square defects in the geomembrane. Geotext. Geomembr. 2008, 26, 196–204. [Google Scholar] [CrossRef]
- Tay, Y.Y.; Stewart, D.I.; Cousens, T.W. Shrinkage and desiccation cracking in bentonite–sand landfill liners. Eng. Geol. 2001, 60, 263–274. [Google Scholar] [CrossRef]
- Osinubi, K.J.; Nwaiwu, C.M.O. Desiccation-induced Shrinkage in Compacted Lateritic Soils. Geotech. Geol. Eng. 2008, 26, 603–611. [Google Scholar] [CrossRef]
- Groenevelt, P.H.; Grant, C.D. Analysis of soil shrinkage data. Soil Till. Res. 2004, 79, 71–77. [Google Scholar] [CrossRef]
- Miller, C.J.; Mishra, M. Modeling of leakage through cracked clay liners-I: State of the art. Water Resour. Bull. AWRA. 1989, 25, 551–555. [Google Scholar] [CrossRef]
- Albrecht, B.A.; Benson, C.H. Effect of Desiccation on Compacted Natural Clays. J. Geotech. Geoenviron. Eng. 2001, 127, 67–75. [Google Scholar] [CrossRef]
- Liu, J.P.; Yang, P.; Yang, Z.H. Water and salt migration mechanisms of saturated chloride clay during freeze-thaw in an open system. Cold Reg. Sci. Technol. 2021, 186, 103277. [Google Scholar] [CrossRef]
- Wang, J.H.; Zhang, F.; Yang, Z.H.; Yang, P. Experimental investigation on the mechanical properties of thawed deep permafrost from the Kuparuk River Delta of the North Slope of Alaska. Cold Reg. Sci. Technol. 2022, 195, 103482. [Google Scholar] [CrossRef]
- Xue, Q.; Zhao, Y.; Liu, L.; Lu, H.J. Study of Thermo-Hydro-Mechanical-Chemical Coupling Effect of Catastrophe Process of Laddfill. Chin. J. Rock Mech. Eng. 2011, 30, 1970–1988. [Google Scholar]
- Li, J.H.; Zhang, L.M. Study of desiccation crack initiation and development at ground surface. Eng. Geol. 2011, 123, 347–358. [Google Scholar] [CrossRef]
- Peng, M.; Zhang, L.M. Breaching parameters of landslide dams. Landslides 2011, 9, 13–31. [Google Scholar] [CrossRef]
- Xu, Y.; Zhang, L.M. Breaching parameters of earth and rockfill dams. J. Geotech. Geoenviron. Eng. 2009, 135, 1957–1970. [Google Scholar] [CrossRef]
- Liu, P.; Shao, G.H.; Huang, R.P. Treatment of bayer-process eed mud through microbially induced carbonate precipitation. J. Mater. Civ. Eng. 2021, 33, 4021067. [Google Scholar]
- Nowamooz, H.; Mrad, M.; Abdallah, A.; Masrouri, F. Experimental and numerical studies of the hydromechanical behaviour of a natural unsaturated swelling soil. Can. Geotech. J. 2009, 46, 393–410. [Google Scholar]
- Rodríguez, R.; Sánchez, M.; Ledesma, A.; Lloret, A. Experimental and numerical analysis of desiccation of a mining waste. Can. Geotech. J. 2007, 44, 644–658. [Google Scholar]
- Tang, C.S.; Cui, Y.J.; Shi, B.; Tang, A.M.; Liu, C. Desiccation and cracking behaviour of clay layer from slurry state under wetting–drying cycles. Geoderma 2011, 166, 111–118. [Google Scholar] [CrossRef]
- Tang, C.S.; Shi, B.; Liu, C.; Zhao, L.Z.; Wang, B.J. Influencing factors of geometrical structure of surface shrinkage cracks in clayey soils. Eng. Geol. 2008, 101, 204–217. [Google Scholar] [CrossRef]
- Liu, S.Y.; Ji, P.; Fang, L. Approach to cyclic swelling behavior of compacted expansive clays. Chin. J. Geotech. Eng. 1999, 21, 9–13. [Google Scholar]
- Nahlawi, H.; Kodikara, J.K. Laboratory experiments on desiccation cracking of thin soil layers. Geotech. Geol. Eng. 2006, 24, 1641–1664. [Google Scholar] [CrossRef]
- Tang, C.S.; Cui, Y.J.; Tang, A.M.; Shi, B. Experiment evidence on the temperature dependence of desiccation cracking behavior of clayey soils. Eng. Geol. 2010, 114, 261–266. [Google Scholar] [CrossRef]
- Vogel, H.J.; Hoffmann, H.; Roth, K. Studies of crack dynamics in clay soil. Geoderma 2005, 125, 203–211. [Google Scholar] [CrossRef]
- Kodikara, J.K.; Choi, X. A simplified analytical model for desiccation cracking of clay layers in laboratory tests. Unsaturated Soils 2006, 12, 2558–2569. [Google Scholar]
- Konrad, J.M.; Ayad, R. Desiccation of a sensitive clay-field experimental observations. Can. Geotech. J. 1997, 34, 929–942. [Google Scholar] [CrossRef]
- Peron, H.; Laloui, L.; Hueckel, T.; Hu, L.B. Desiccation cracking of soils. Eur. J. Environ. Civ. Eng. 2009, 13, 869–888. [Google Scholar] [CrossRef]
- Camp, S.; Gourc, J.P.; Ple, O. Landfill clay barrier subjected to cracking: Multi-scale analysis of bending tests. Appl. Clay Sci. 2010, 48, 384–392. [Google Scholar] [CrossRef]
- The Ministry of Construction of the People’s Republic of China. Technical Code for Sanitary Landefill of Municipal Domestic Refuse (CJJ17-2004); China Building Industry Press: Beijing, China, 2004.
- Zhong, Y.Q.; Cai, G.H.; Wang, S.Q.; Qin, H.J.; Zhang, C.H.; Li, J.S. Influence of Organic Content on the Mechanical Properties of Organic-Rich Soils Stabilized with CaO-GGBS Binder and PC. Water 2022, 14, 3053. [Google Scholar] [CrossRef]
- Cai, G.H.; Zhou, Y.F.; Poon, C.S.; Li, J.S. Engineering performance and microstructure characteristics of natural marine sediment stabilized with quicklime-activated GGBS under different lime proportions. Mar. Georesour. Geotechnol. 2022, 1–15. [Google Scholar] [CrossRef]
- Chertkov, V.Y.; Ravina, I. Shrinking–swelling phenomenon of clay soils attributed to capillary-crack network. Theor. Appl. Fract. Mech. 2000, 34, 61–71. [Google Scholar] [CrossRef]
- Konrad, J.M.; Ayad, R. A idealized framework for the analysis of cohesive soils undergoing desiccation. Can. Geotech. J. 1997, 34, 477–488. [Google Scholar] [CrossRef]
- Cai, G.H.; Zhou, Y.F.; Li, J.S.; Han, L.J.; Poon, C.S. Deep insight into mechanical behavior and microstructure mechanism of quicklime-activated ground granulated blast-furnace slag pastes. Cem. Concr. Compos. 2022, 134, 104767. [Google Scholar] [CrossRef]
- Kong, L.R.; Huang, H.W.; Zhang, D.M.; Hicher, P.Y. Experiment study on eelationship between pore distribution and different stress levels due to consolidation of soft clays. Chin. J. Undergr. Space Eng. 2007, 3, 1036–1040. [Google Scholar]
- Xue, Q.; Zhang, Q. Effects of leachate concentration on the integrity of solidified clay liners. Waste Manag. Res. 2014, 32, 198–206. [Google Scholar] [CrossRef]
- Tang, A.M.; Cui, Y.J.; Vu, M.N. Effects of the maximum soil aggregates size and cyclic wetting–drying on the stiffness of a lime-treated clayey soil. Géotechnique 2011, 61, 421–429. [Google Scholar] [CrossRef]
- Tang, C.S.; Shi, B.; Liu, C.; Suo, W.B.; Gao, L. Experimental characterization of shrinkage and desiccation cracking in thin clay layer. Appl. Clay Sci. 2011, 52, 69–77. [Google Scholar] [CrossRef]








| Property | Value | |
|---|---|---|
| Natural moisture content, wn/% | 27 | |
| Natural unit weight, γ (kN/m3) | 41.4 | |
| Optimum water content, wopt (%) | 20.7 | |
| Maximum dry density, ρdmax (g/cm3) | 1.66 | |
| Liquid limit, wL/% | 38.9 | |
| Plastic limit, wP/% | 22.5 | |
| Plasticity index, IP | 16.4 | |
| Specific gravity, GS | 2.72 | |
| Grain-size distribution/% | <0.005 mm | 57 |
| 0.005–0.075 mm | 33 | |
| >0.075 mm | 10.0 | |
| Soil pH (water: soil = 2:1) | 8.27 | |
| MgO | Al2O3 | CaO | SiO2 | Fe2O3 | Na2O | K2O | TiO2 | Loss on Ignition |
|---|---|---|---|---|---|---|---|---|
| 0.28 | 23.80 | 0.43 | 60.69 | 0.30 | 2.03 | 4.03 | 0.03 | 8.21 |
| Samples | Total Mercury Amount/[cm3/g] | Large Porosity/% | Middle-Sized Porosity/% | Small Porosity/% | Tiny Porosity/% | Ultra-Micro Porosity/% |
|---|---|---|---|---|---|---|
| >10 μm | 2.5~10 μm | 0.4~2.5 μm | 0.04~0.4 μm | <0.04 μm | ||
| Compaction-Drying Cycle | 0.1414 | 24.045 | 24.045 | 14.36 | 17.96 | 19.59 |
| First Wetting-Drying Cycle | 0.1746 | 18.27 | 27.38 | 18.38 | 15.86 | 16.55 |
| Second Wetting-Drying Cycle | 0.1944 | 16.51 | 33.54 | 21.91 | 13.89 | 14.15 |
| Third Wetting-Drying Cycle | 0.2220 | 24.59 | 29.41 | 19.50 | 13.24 | 13.24 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhong, Y.; Cai, G.; Zeng, G. The Effect of Initial Water Content and Density on the Swelling-Shrinkage and Cracking Characteristics of Compacted Clay. Separations 2022, 9, 424. https://doi.org/10.3390/separations9120424
Zhong Y, Cai G, Zeng G. The Effect of Initial Water Content and Density on the Swelling-Shrinkage and Cracking Characteristics of Compacted Clay. Separations. 2022; 9(12):424. https://doi.org/10.3390/separations9120424
Chicago/Turabian StyleZhong, Yuqing, Guanghua Cai, and Gang Zeng. 2022. "The Effect of Initial Water Content and Density on the Swelling-Shrinkage and Cracking Characteristics of Compacted Clay" Separations 9, no. 12: 424. https://doi.org/10.3390/separations9120424
APA StyleZhong, Y., Cai, G., & Zeng, G. (2022). The Effect of Initial Water Content and Density on the Swelling-Shrinkage and Cracking Characteristics of Compacted Clay. Separations, 9(12), 424. https://doi.org/10.3390/separations9120424






