From Formulas to Functions through Geometry: A Path to Understanding Algebraic Computations
Abstract
:1. Introduction and Theoretical Framework
1.1. Towards a Functional Approach to Teaching Algebra
1.2. Geometry as a Context to Work with Algebra
1.3. Algebra, Problem Solving, and Creativity
1.4. The Role of Technologies in Algebraic Modelling Problems
1.5. Algebra as a Language for Modelling
- generalization and formalization of patterns and constraints;
- syntactically guided manipulation of (opaque) formalisms;
- study of structures, abstracted from computations and relations;
- study of functions, relations, and joint variation;
- cluster of modeling and phenomena-controlling languages.
1.6. Goal of the Paper and Research Questions
- (RQ1)
- Could an activity of algebraic thinking in a geometric context help students to develop mathematical creativity?
- (RQ2)
- In what ways can the use of multiple representations support the understanding of algebraic formulas?
- (RQ3)
- What are the main problems faced by students in using algebraic thinking in geometry?
2. Materials and Methods
The Interactive Activities
- In the classroom, displayed through the interactive whiteboard (IWB) and asking students to solve them in small groups, with paper and pen or with tablets, if available.
- At home, as homework, to be carried out individually.
- observation and notes, taken during the classroom activities by the teachers and the researcher;
- videotapes of the classroom activities;
- students’ answers to specific items of the final test.
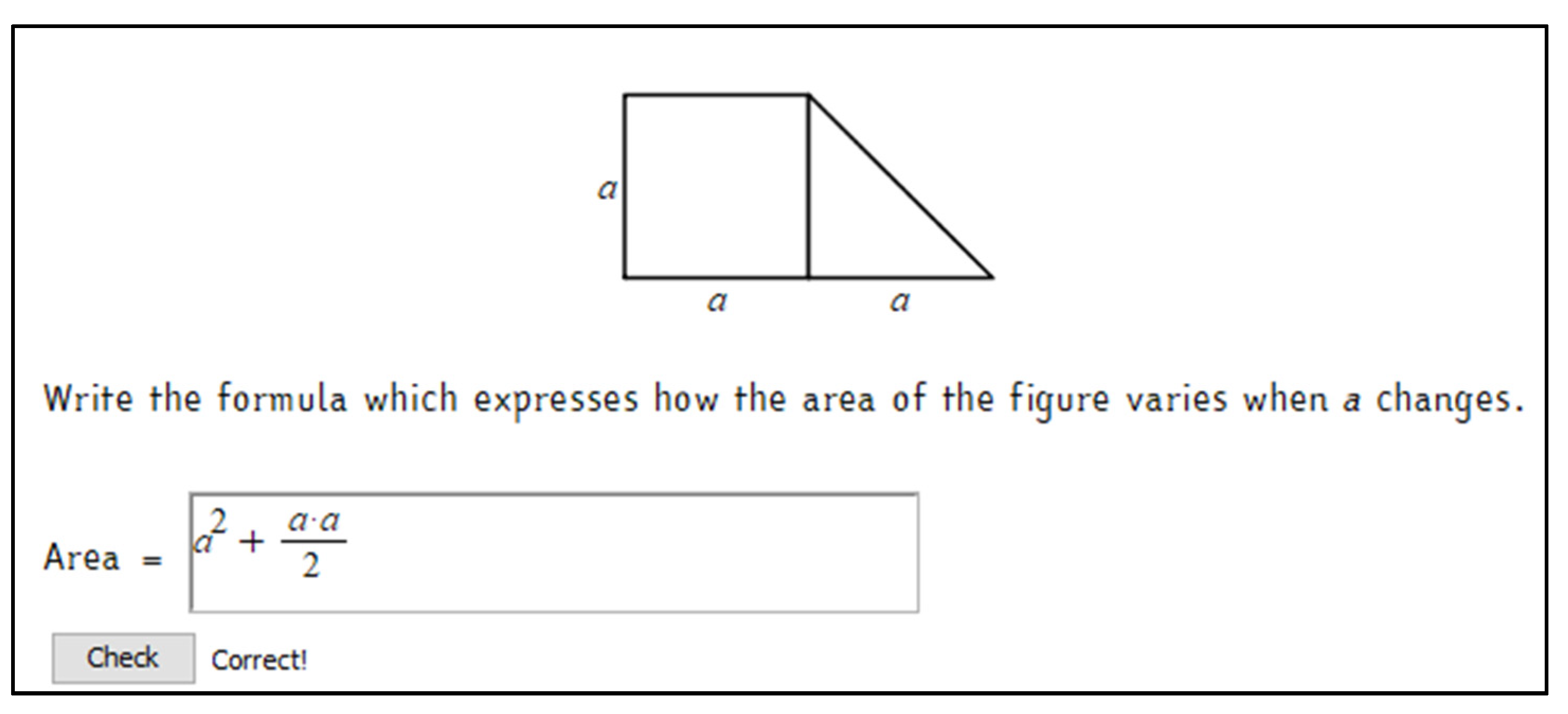
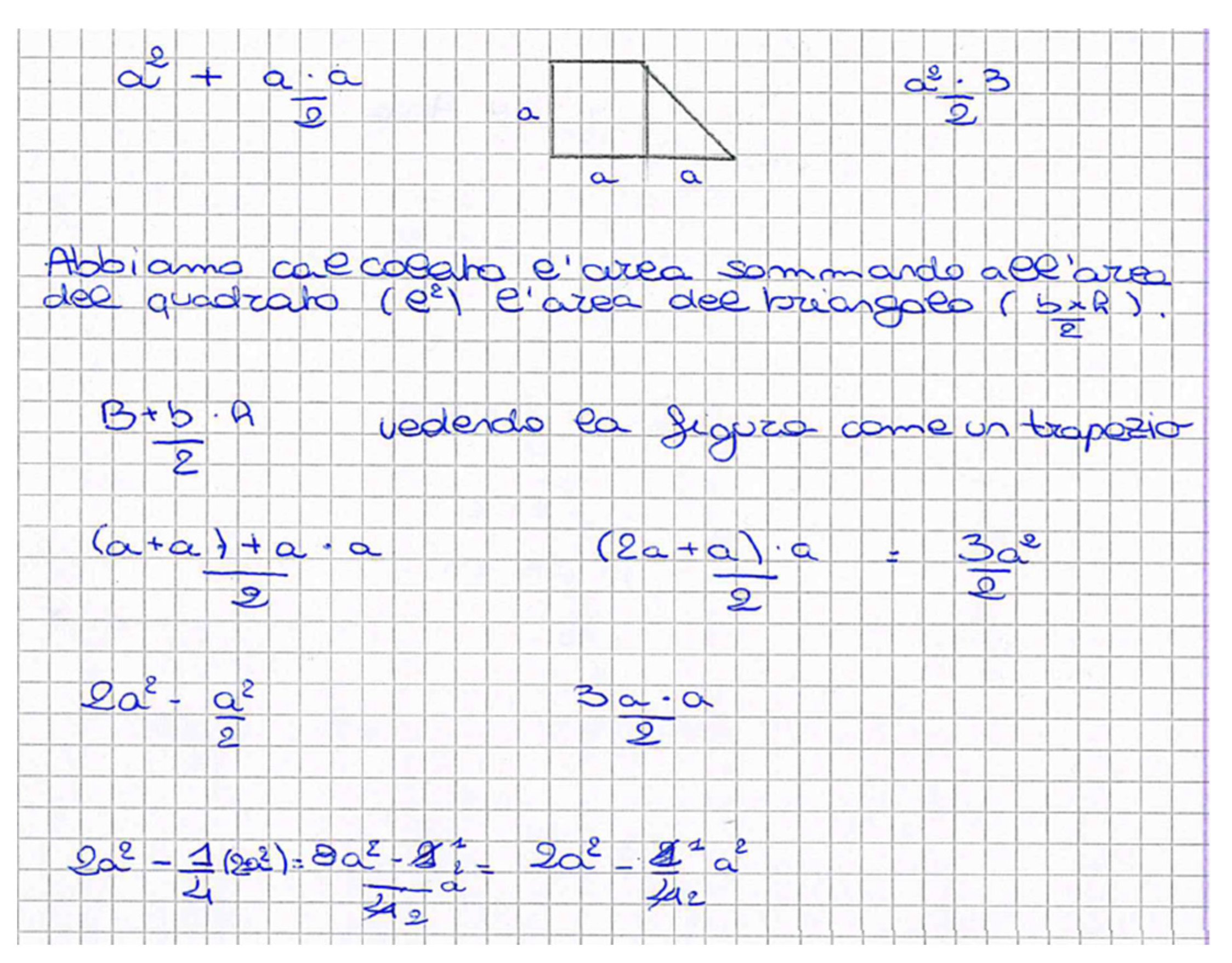
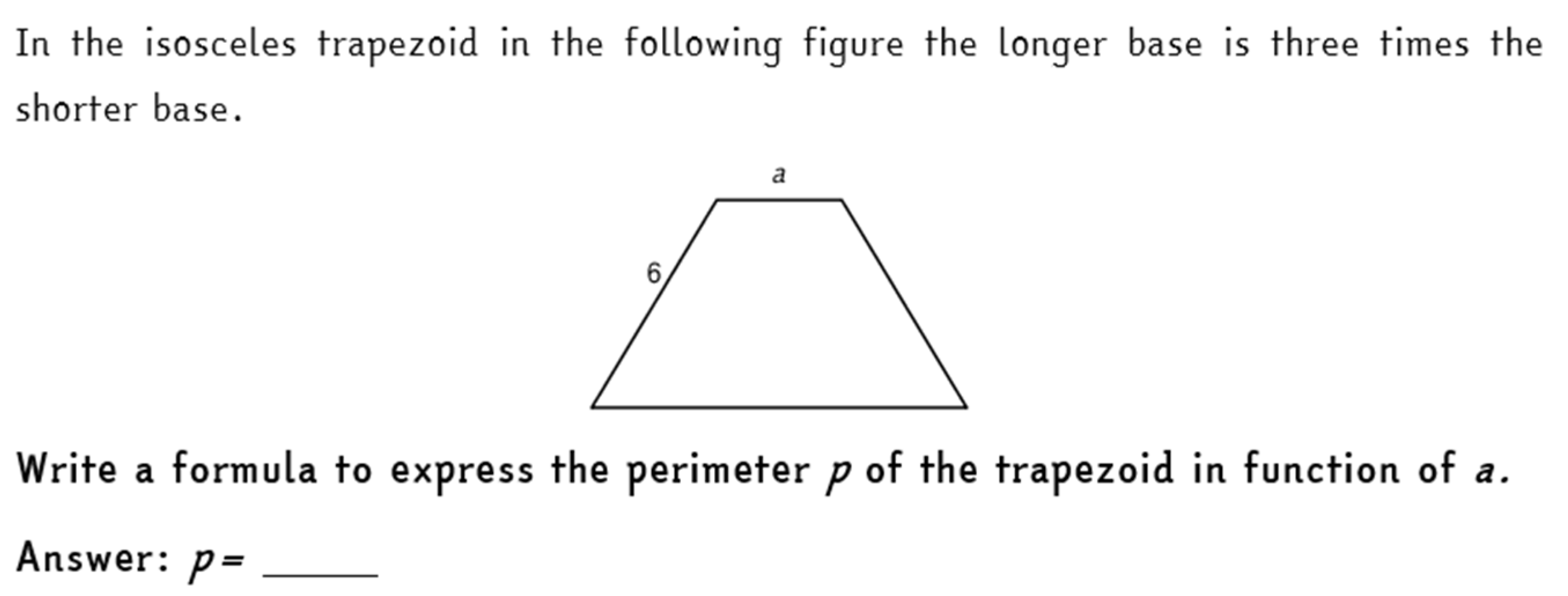
3. Results
- —seeing the figure as a trapezoid;
- —interpreting it as the composition of a square and a triangle;
- —splitting the square into two triangles through the diagonal, and interpreting the trapezoid as composed by three identical triangles;
- —seeing a rectangle with base 2a, minus the upper right triangle;
- —seeing a rectangle to which a quarter of the rectangle itself was removed;
- —dividing the rectangle into 4 parts to find a triangle and multiplying it by 3.
4. Discussion
- Fundamental misconceptions from arithmetic concepts, such as Mariana and Federico’s problems with fractions; and algebraic errors, such as a + a = a2
- Errors rooted in the comprehension of geometry, such as Marco’s recalling of the Pythagorean theorem to compute the triangle’s area.
- Problems coming from the well-established practice of studying the various mathematics branches separately, which lead students to stop reasoning algebraically when dealing with geometry.
- We began early, since the activities involved students of grade eight, while algebraic manipulations will be formally developed in the following years. However, we need to point out that, although the study was aimed at grade eight students, activities of algebraic modelling can be introduced even earlier, from the primary level [31].
- The activities tried to apply algebra and integrate it in a geometry context, so to give a deeper meaning to the formulas and computations.
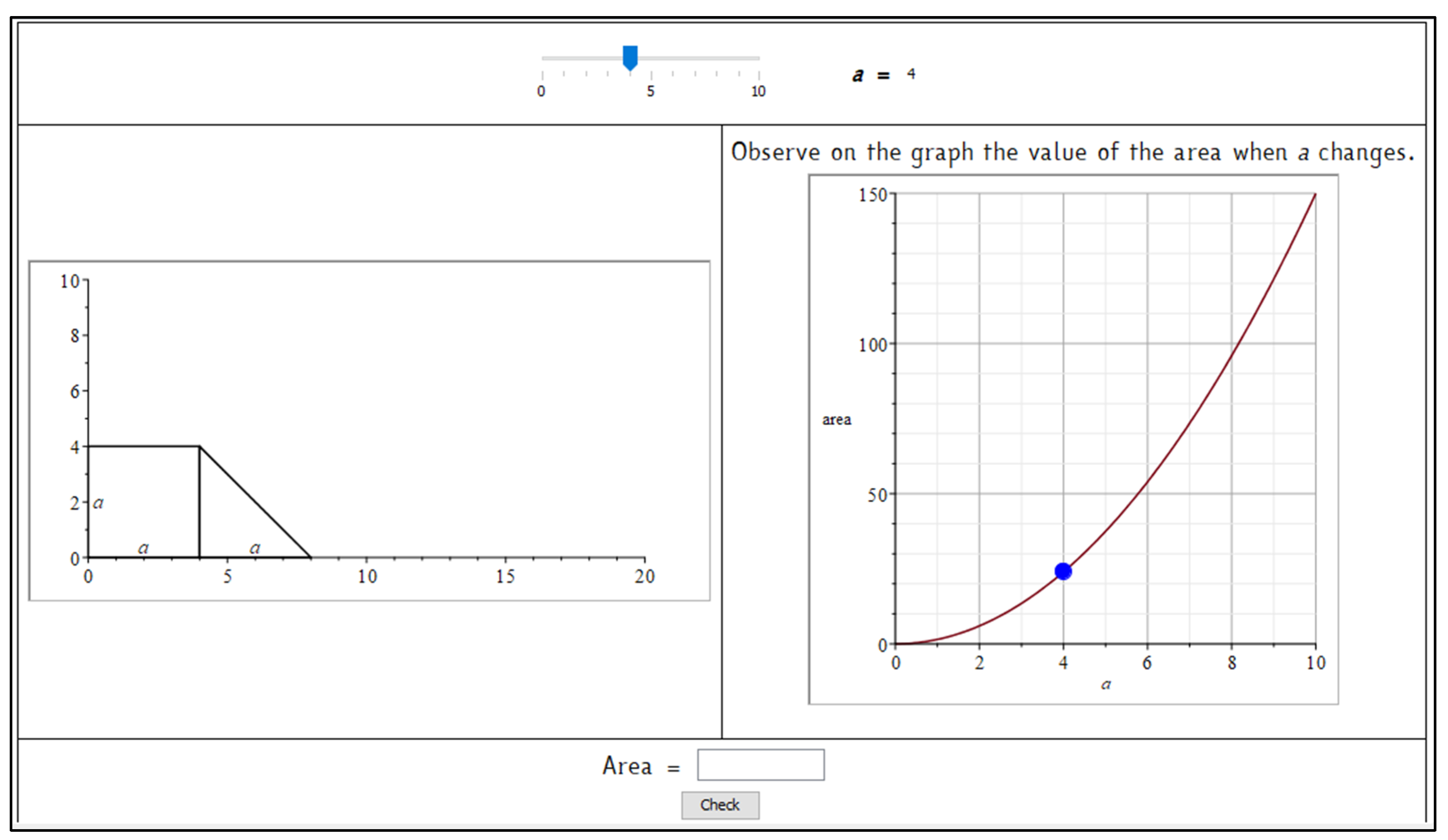
- They included different forms of algebraic thinking, in particular, considering Kaput’s framework [30], the syntactically guided manipulation of formalisms (when students were finding the formulas expressing the area of some figures), and the study of functions, relations, and joint variation (when the requests shifted to observe the growth of the area and display the function representing it).
- They are grounded in the students’ cognitive and, especially, linguistic abilities, since through the activities, attention was paid in translating from natural language to algebra as well as explaining and justifying students’ solutions.
- Active learning is encouraged through group work and digital interactive activities. In all the activities, students had the chance to explore and solve the problems themselves, and each group was asked to explain their solutions.
5. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Huntley, M.A.; Rasmussen, C.L.; Villarubi, R.S.; Sangtong, J.; Fey, J.T. Effects of Standards-Based Mathematics Education: A Study of the Core-plus Mathematics Project Algebra and Functions Strand. J. Res. Math. Educ. 2000, 31, 328. [Google Scholar] [CrossRef]
- Greer, B. Algebra for All? Math. Enthus. 2008, 5, 423–428. [Google Scholar]
- Arcavi, A. Symbol Sense: Informal Sense-Making in Formal Mathematics. Learn. Math. 1994, 14, 24–35. [Google Scholar]
- MIUR. Indicazioni Nazionali Per Il Curricolo Della Scuola Dell’infanzia e Del Primo Ciclo D’istruzione; MIUR: Rome, Italy, 2012.
- Ministero della Pubblica Istruzione. Decreto Ministeriale 9 Febbraio 1979: Programmi, Orari Di Insegnamento e Prove Di Esame per La Scuola Media Statale. Suppl. Ordin. Alla Gazz. Uff. Della Repubb. Ital. 1979, 50, 1–50. [Google Scholar]
- Cusi, A.; Malara, N.A.; Navarra, G. Early Algebra: Theoretical Issues and Educational Strategies for Bringing the Teachers to Promote a Linguistic and Metacognitive approach to it. In Early Algebraization: Cognitive, Curricular, and Instructional Perspectives; Springer: Berlin/Heidelberg, Germany, 2011; pp. 483–510. [Google Scholar]
- Kieran, C. Algebra Teaching and Learning. In Encyclopedia of Mathematics Education; Lerman, S., Ed.; Springer: Dordrecht, The Netherlands, 2014; pp. 27–31. ISBN 978-94-007-4977-1. [Google Scholar]
- Kieran, C. Algebraic Thinking in the Early Grades: What Is It? Math. Educ. 2004, 8, 139–151. [Google Scholar]
- Yerushalmy, M. Problem Solving Strategies and Mathematical Resources: A Longitudinal View on Problem Solving in a Function Based Approach to Algebra. Educ. Stud. Math. 2000, 43, 125–147. [Google Scholar] [CrossRef]
- Malara, N.A. Il pensiero algebrico: Come promuoverlo sin dalla scuola dell’obbligo limitandone le difficoltà? L’educazione Mat. 1996, 17, 80–99. [Google Scholar]
- Goldin, G.A. Mathematical Representations. In Encyclopedia of Mathematics Education; Lerman, S., Ed.; Springer: Dordrecht, The Netherlands, 2014; pp. 409–413. ISBN 978-94-007-4977-1. [Google Scholar]
- Duval, R. Representation, Vision and Visualization: Cognitive Functions in Mathematical Thinking. In Proceedings of the Annual Meeting of the North American Chapter of the International Group for the Psychology of Mathematics Education, Cuernavaca, Morelos, Mexico, 23–26 October 1999; pp. 3–26. [Google Scholar]
- Bolondi, G.; Ferretti, F.; Santi, G. National Standardized Tests Database Implemented as a Research Methodology in Mathematics Education. The Case of Algebraic Powers. In Proceedings of the Eleventh Congress of the European Society for Research in Mathematics Education, Utrecht, The Netherlands, 6–10 February 2019. [Google Scholar]
- Duval, R. Registres de Représentations Sémiotique et Fonctionnement Cognitif de La Pensée. Ann. De Didact. Et De Sci. Cogn. 1993, 5, 37–65. [Google Scholar]
- Pozio, S.; Bolondi, G. Difficulties in Formulating a Geometric Situation Algebraically: Hints from a Large-Scale Assessment. In Proceedings of the 43rd Conference of the International Group for the Psychology of Mathematics Education, Pretoria, South Africa, 7–12 July 2019. [Google Scholar]
- Bolondi, G.; Ferretti, F. Quantifying Solid Findings in Mathematics Education: Loss of Meaning for Algebraic Symbols. IJISME 2021, 29, 1–15. [Google Scholar] [CrossRef]
- Gambini, A.; Banchelli, S.; Nolli, N. Analisi verticale del concetto di pendenza: Dalla scuola secondaria di primo grado all’università. In Il Dato Nella Didattica Delle Discipline. II Seminario “I dati INVALSI: Uno Strumento Per La Ricerca”; Falzetti, P., Ed.; INVALSI per la ricerca; FrancoAngeli: Milano, Italy, 2020; pp. 184–200. ISBN 978-88-351-0158-1. [Google Scholar]
- Best, M.; Bikner-Ahsbahs, A. The Function Concept at the Transition to Upper Secondary School Level: Tasks for a Situation of Change. ZDM 2017, 49, 865–880. [Google Scholar] [CrossRef]
- Dindyal, J. Algebraic Thinking in Geometry at High School Level: Students’ Use of Variables and Unknowns. In Proceedings of the Mathematics Education for the Third Millennium: Towards 2010: Proceedings of the 27th Annual Conference of the Mathematics Education Research Group of Australasia; Putt, I., Faragher, R., McLean, M., Eds.; MERGA Inc.: Townsville, Australia, 2004; pp. 183–190. [Google Scholar]
- Wilkie, K.J. Seeing Quadratics in a New Light: Secondary Mathematics Pre-Service Teachers’ Creation of Figural Growing Patterns. Educ. Stud. Math. 2021, 106, 91–116. [Google Scholar] [CrossRef]
- Garzón, J.; Bautista, J. Virtual Algebra Tiles: A Pedagogical Tool to Teach and Learn Algebra through Geometry. J. Comput. Assist. Learn. 2018, 34, 876–883. [Google Scholar] [CrossRef]
- Florio, E. A Synergy between History of Mathematics and Mathematics Education: A Possible Path from Geometry to Symbolic Algebra. Educ. Sci. 2020, 10, 243. [Google Scholar] [CrossRef]
- D’Amore, B.; Pinilla, M.I.F. Che problema i problemi! L’insegnamento Della Mat. e Delle Sci. Integr. 2006, 6, 645–664. [Google Scholar]
- Pehkonen, E. The State-of-Art in Mathematical Creativity. ZDM Math. Educ. 1997, 29, 63–67. [Google Scholar] [CrossRef]
- Sriraman, B.; Haavold, P.; Lee, K. Creativity in Mathematics Education. In Encyclopedia of Mathematics Education; Lerman, S., Ed.; Springer: Dordrecht, The Netherlands, 2014; pp. 109–115. ISBN 978-94-007-4977-1. [Google Scholar]
- Leikin, R.; Lev, M. Mathematical Creativity in Generally Gifted and Mathematically Excelling Adolescents: What Makes the Difference? ZDM Math. Educ. 2013, 45, 183–197. [Google Scholar] [CrossRef]
- Torrance, E.P. The nature of creativity as manifest in its testing. In The Nature of Creativity: Contemporary Psychological Perspectives; Sternberg, R.J., Ed.; Cambridge University Press: Cambridge, UK, 1988; pp. 43–75. [Google Scholar]
- Silver, E.A. Fostering Creativity through Instruction Rich in Mathematical Problem Solving and Problem Posing. ZDM Math. Educ. 1997, 29, 75–80. [Google Scholar] [CrossRef]
- Acevedo Nistal, A.; Dooren, W.; Clarebout, G.; Elen, J.; Verschaffel, L. Conceptualising, Investigating and Stimulating Representational Flexibility in Mathematical Problem Solving and Learning: A Critical Review. ZDM Math. Educ. 2009, 41, 627–636. [Google Scholar] [CrossRef]
- Kaput, J.J. Teaching and Learning a New Algebra. In Mathematics Classrooms That Promote Understanding; Fennema, E., Ronberg, T.A., Eds.; Routledge: New York, NY, USA, 1999. [Google Scholar]
- Kieran, C.; Pang, J.; Schifter, D.; Swee Fong, N. Early Algebra. In ICME-13 Topical Surveys; Springer: New York, NY, USA, 2016; ISBN 978-3-319-32257-5. [Google Scholar]
- Barana, A.; Marchisio, M.; Sacchet, M. Advantages of Using Automatic Formative Assessment for Learning Mathematics. In Technology Enhanced Assessment; Draaijer, S., Joosten-ten Brinke, D., Ras, E., Eds.; Springer: Cham, Switzerland, 2019; Volume 1014, pp. 180–198. ISBN 978-3-030-25263-2. [Google Scholar]
- Barana, A.; Marchisio, M. An Interactive Learning Environment to Empower Engagement in Mathematics. Interact. Des. Archit. 2020, 45, 302–321. [Google Scholar]
- Barana, A.; Marchisio, M.; Sacchet, M. Interactive Feedback for Learning Mathematics in a Digital Learning Environment. Educ. Sci. 2021, 11, 279. [Google Scholar] [CrossRef]
- Barana, A.; Marchisio, M. Analyzing Interactions in Automatic Formative Assessment Activities for Mathematics in Digital Learning Environments. In Proceedings of the 13th International Conference on Computer Supported Education-Volume 1: CSEDU, 23–25 April 2021; pp. 497–504. [Google Scholar]
- Liljedahl, P.; Santos-Trigo, M.; Malaspina, U.; Bruder, R. Problem Solving in Mathematics Education. In ICME-13 Topical Surveys; Springer: New York, NY, USA, 2016; ISBN 978-3-319-40729-6. [Google Scholar]
- Samo, D.D.; Darhim, D.; Kartasasmita, B. Culture-Based Contextual Learning to Increase Problem-Solving Ability of First Year University Student. J. Math. Educ. 2017, 9, 81–94. [Google Scholar] [CrossRef]
- Fissore, C.; Marchisio, M.; Floris, F.; Sacchet, M. Maple for Distance Education in Secondary Schools during the COVID-19 Emergency. In Maple in Mathematics Education and Research. MC 2020; Communications in Computer and Information Science; Springer: Cham, Switzerland, 2021; Volume 1414, pp. 204–218. [Google Scholar]
- Fissore, C.; Marchisio, M.; Roman, F.; Sacchet, M. Development of Problem Solving Skills with Maple in Higher Education. In Maple in Mathematics Education and Research. MC 2020; Communications in Computer and Information Science; Springer: Cham, Switzerland, 2021; Volume 1414, pp. 219–233. [Google Scholar]
- Marchisio, M.; Margaria, T.; Sacchet, M. Automatic Formative Assessment in Computer Science: Guidance to Model-Driven Design. In Proceedings of the 2020 IEEE 44th Annual Computers, Software, and Applications Conference (COMPSAC), Madrid, Spain, 13–17 July 2020; IEEE: Madrid, Spain, 2020; pp. 201–206. [Google Scholar]
- Di Caro, L.; Fioravera, M.; Marchisio, M.; Rabellino, S. Towards Adaptive Systems for Automatic Formative Assessment in Virtual Learning Communities. In Proceedings of the 2018 IEEE 42nd Annual Computer Software and Applications Conference (COMPSAC), Tokyo, Japan, 23–27 July 2018; IEEE: Tokyo, Japan, 2018; pp. 1000–1005. [Google Scholar]
- Barana, A.; Conte, A.; Fioravera, M.; Marchisio, M.; Rabellino, S. A Model of Formative Automatic Assessment and Interactive Feedback for STEM. In Proceedings of the 2018 IEEE 42nd Annual Computer Software and Applications Conference (COMPSAC), Tokyo, Japan, 23–27 July 2018; IEEE: Tokyo, Japan, 2018; pp. 1016–1025. [Google Scholar]
- Fissore, C.; Marchisio, M.; Rabellino, S. Online Tutoring through an Integrated Platform to Support Learning Mathematics at Lower Secondary School. In Proceedings of the 14th International Conference on e-Learning, Zagreb, Croazia, 21–23 July 2020; IADIS Press: Budapest, Hungary, 2020; pp. 71–78. [Google Scholar]
- Gestinv 3.0. Available online: https://gestinv.it/Index.aspx (accessed on 18 November 2021).
- Barana, A.; Fioravera, M.; Marchisio, M.; Rabellino, S. Adaptive Teaching Supported by ICTs to Reduce the School Failure in the Project “Scuola Dei Compiti”. In Proceedings of the 2017 IEEE 41st Annual Computer Software and Applications Conference (COMPSAC), Turin, Italy, 4–8 July 2017; IEEE: Torino, Italy, 2017; pp. 432–437. [Google Scholar]
- Tomlinson, C.A. How to Differentiate Instruction in Mixed-Ability Classrooms; Association for Supervision & Curriculum Development: Alexandri, Egypt, 2005; ISBN 978-0-87120-917-7. [Google Scholar]
- Brancaccio, A.; Marchisio, M.; Palumbo, C.; Pardini, C.; Patrucco, A.; Zich, R. Problem Posing and Solving: Strategic Italian Key Action to Enhance Teaching and Learning Mathematics and Informatics in the High School. In Proceedings of the 2015 IEEE 39th Annual Computer Software and Applications Conference, Taichung, Taiwan, 1–5 July 2015; IEEE: Taichung, Taiwan, 2015; pp. 845–850. [Google Scholar]
- Barana, A.; Fissore, C.; Marchisio, M.; Pulvirenti, M. Teacher Training for the Development of Computational Thinking and Problem Posing & Solving Skills with Technologies. In Proceedings of the 16th International Scientific Conference ELearning and Software for Education, Bucharest, Romania, 23–24 April 2020; Volume 2, pp. 136–144. [Google Scholar]
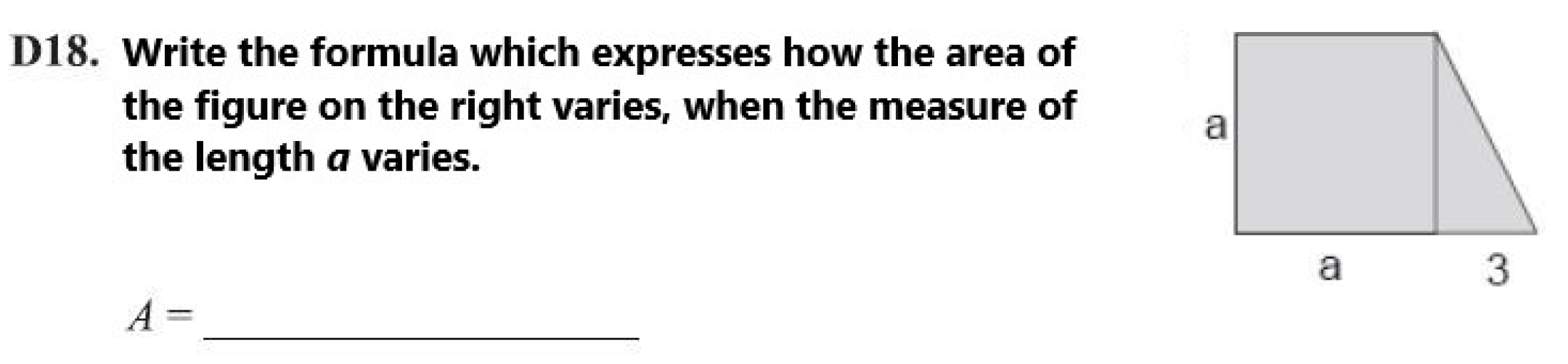

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Barana, A. From Formulas to Functions through Geometry: A Path to Understanding Algebraic Computations. Eur. J. Investig. Health Psychol. Educ. 2021, 11, 1485-1502. https://doi.org/10.3390/ejihpe11040106
Barana A. From Formulas to Functions through Geometry: A Path to Understanding Algebraic Computations. European Journal of Investigation in Health, Psychology and Education. 2021; 11(4):1485-1502. https://doi.org/10.3390/ejihpe11040106
Chicago/Turabian StyleBarana, Alice. 2021. "From Formulas to Functions through Geometry: A Path to Understanding Algebraic Computations" European Journal of Investigation in Health, Psychology and Education 11, no. 4: 1485-1502. https://doi.org/10.3390/ejihpe11040106
APA StyleBarana, A. (2021). From Formulas to Functions through Geometry: A Path to Understanding Algebraic Computations. European Journal of Investigation in Health, Psychology and Education, 11(4), 1485-1502. https://doi.org/10.3390/ejihpe11040106





