Computational Investigation of the Combined Impact of Nonlinear Radiation and Magnetic Field on Three-Dimensional Rotational Nanofluid Flow across a Stretchy Surface
Abstract
:1. Introduction
2. Materials and Methods
3. Results and Discussion
4. Conclusions
- The local Nusselt number increases as magnetic field increases;
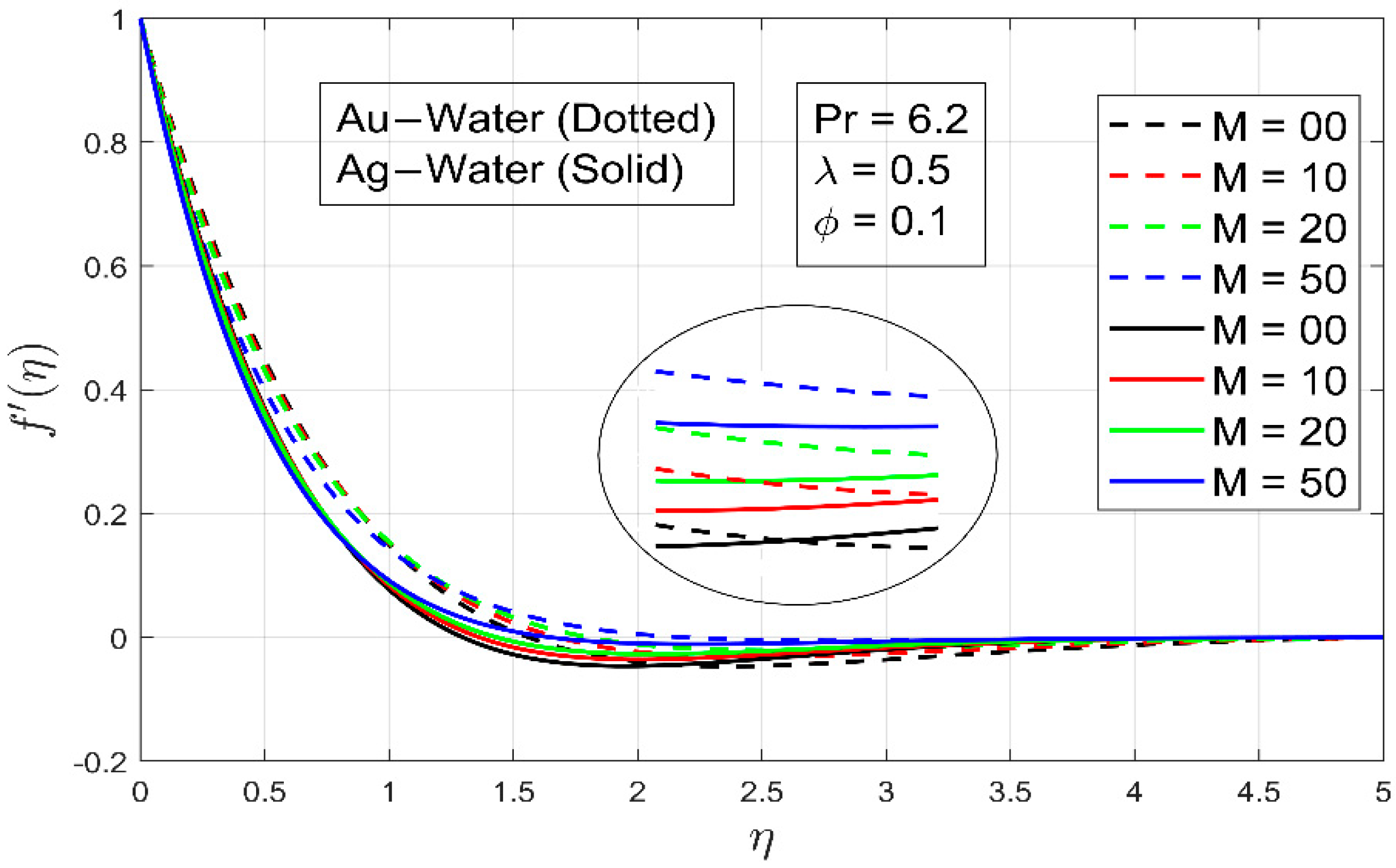
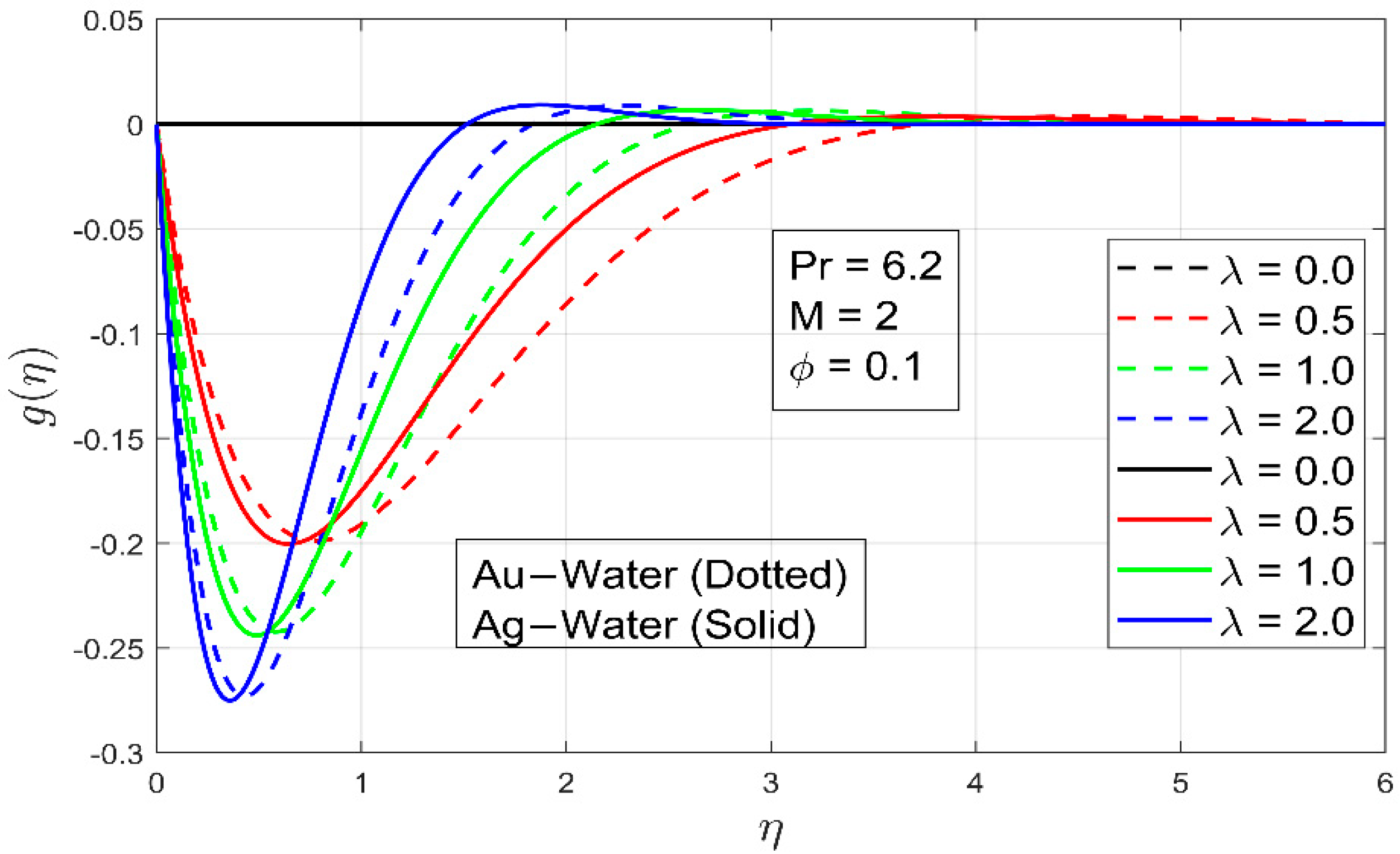
- The y component of velocity is negative for both types of nanofluids, and the velocity distribution is parabolic;
- The parameter has fundamentally different impacts on the and components of velocity;
- The velocity distributions and both decay when a large magnetic field is applied;
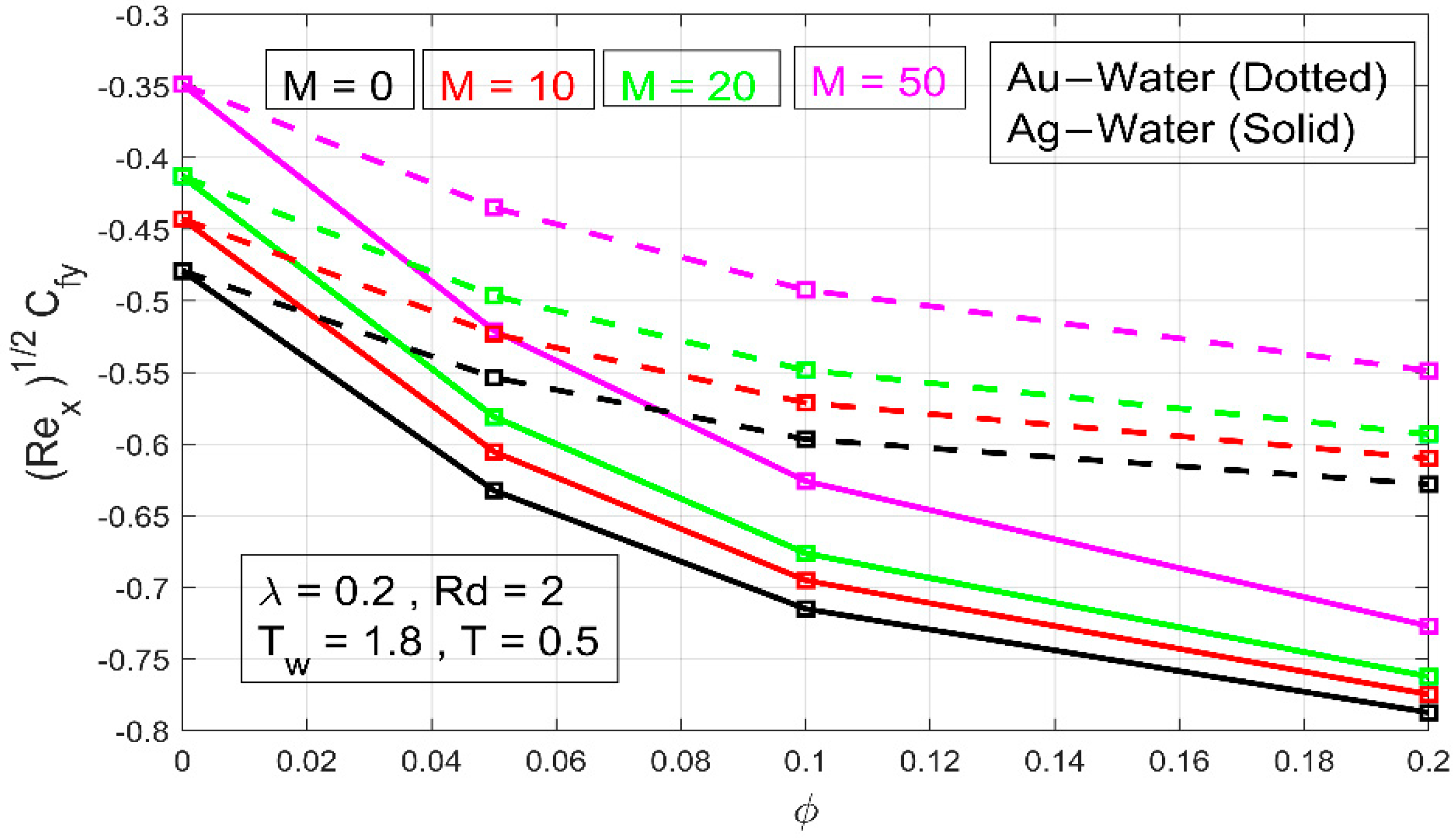
- The magnetic field and the volume fraction have inverse relationships with the skin friction coefficient and local Nusselt numbers, respectively;
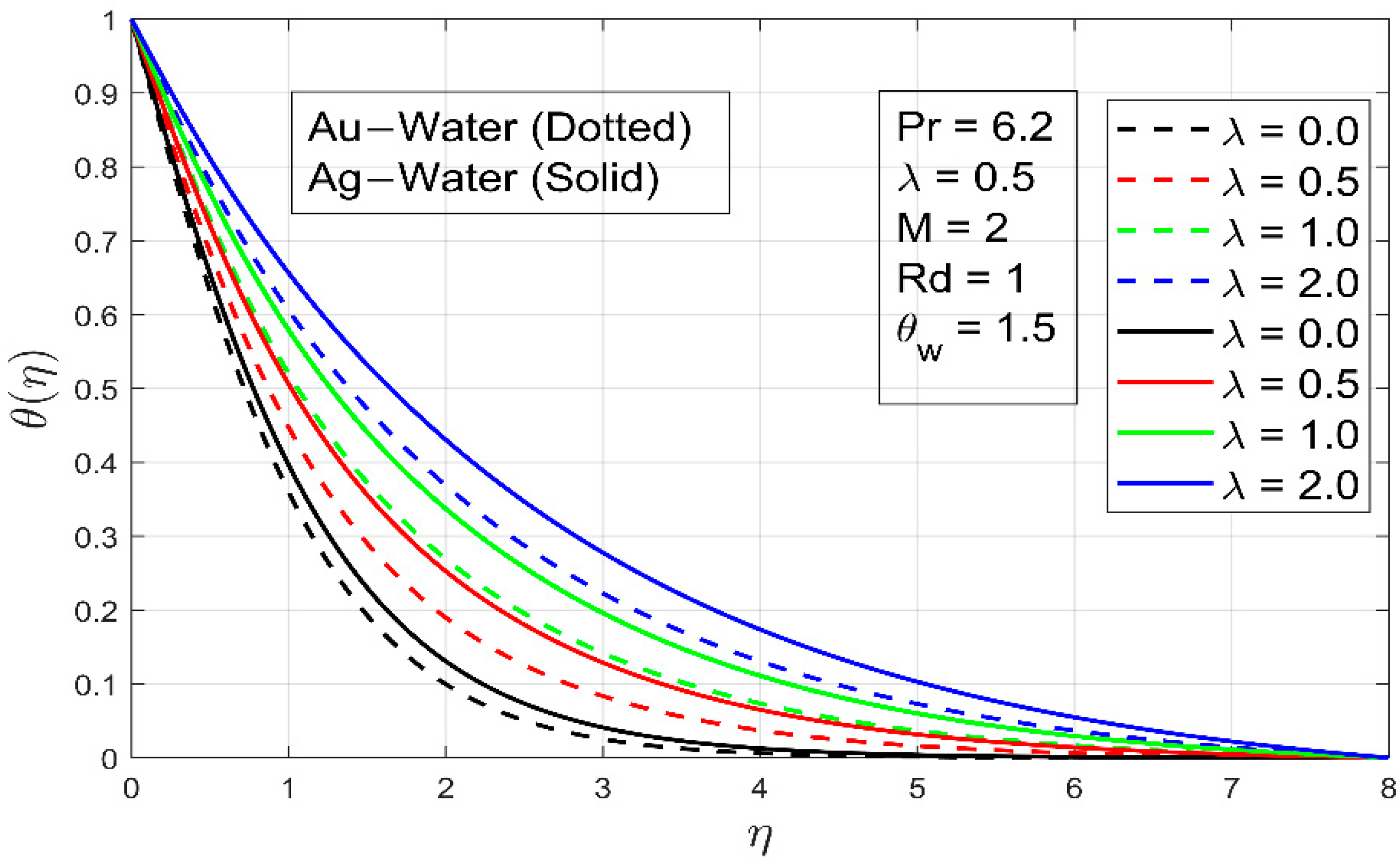
- The parameter enhances the local Nusselt number as it increases;
- -water nanofluid has a high-temperature transfer constant compared to -water nanofluid.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wang, C.Y. Stretching a surface in a rotating fluid. Z. Angew. Math. Und Phys. 1988, 39, 177–185. [Google Scholar] [CrossRef]
- Rajeswari, V.; Nath, G. Unsteady flow over a stretching surface in a rotating fluid. Int. J. Eng. Sci. 1992, 30, 747–756. [Google Scholar] [CrossRef]
- Nazar, R.; Amin, N.; Pop, I. Unsteady boundary layer flow due to a stretching surface in a rotating fluid. Mech. Res. Commun. 2004, 31, 121–128. [Google Scholar] [CrossRef]
- Vajravelu, K.; Kumar, B. Analytical and numerical solutions of a coupled non-linear system arising in a three-dimensional rotating flow. Int. J. Non-Linear Mech. 2004, 39, 13–24. [Google Scholar] [CrossRef]
- Hayat, T.; Javed, T.; Sajid, M. Analytic solution for MHD rotating flow of a second grade fluid over a shrinking surface. Phys. Lett. A 2008, 372, 3264–3273. [Google Scholar] [CrossRef]
- Zaimi, K.; Ishak, A.; Pop, I. Stretching surface in rotating viscoelastic fluid. Appl. Math. Mech. 2013, 34, 945–952. [Google Scholar] [CrossRef]
- Hayat, T.; Farooq, M.; Alsaedi, A.; Al-Solamy, F. Impact of Cattaneo-Christov heat flux in the flow over a stretching sheet with variable thickness. AIP Adv. 2015, 5, 087159. [Google Scholar] [CrossRef]
- Rashidi, M.M.; Abelman, S.; Mehr, N.F. Entropy generation in steady MHD flow due to a rotating porous disk in a nanofluid. Int. J. Heat Mass Transf. 2013, 62, 515–525. [Google Scholar] [CrossRef]
- Choi, S.U.; Eastman, J.A. Enhancing Thermal Conductivity of Fluids with Nanoparticles; No. ANL/MSD/CP-84938; CONF-951135-29; Argonne National Lab.: Lemont, IL, USA, 1995. [Google Scholar]
- Kuznetsov, A.; Nield, D. Natural convective boundary-layer flow of a nanofluid past a vertical plate. Int. J. Therm. Sci. 2010, 49, 243–247. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Sheykholeslami, F.B.; Khoshhal, S.; Mola-Abasia, H.; Ganji, D.D.; Rokni, H.B. Effect of magnetic field on Cu–water nanofluid heat transfer using GMDH-type neural network. Neural Comput. Appl. 2014, 25, 171–178. [Google Scholar] [CrossRef]
- Nield, D.; Kuznetsov, A. Forced convection in a parallel-plate channel occupied by a nanofluid or a porous medium saturated by a nanofluid. Int. J. Heat Mass Transf. 2014, 70, 430–433. [Google Scholar] [CrossRef]
- Khan, J.A.; Mustafa, M.; Hayat, T.; Sheikholeslami, M.; Alsaedi, A. Three-Dimensional Flow of Nanofluid Induced by an Exponentially Stretching Sheet: An Application to Solar Energy. PLoS ONE 2015, 10, e0116603. [Google Scholar] [CrossRef]
- Mustafa, M.; Khan, J.A. Model for flow of Casson nanofluid past a non-linearly stretching sheet considering magnetic field effects. AIP Adv. 2015, 5, 077148. [Google Scholar] [CrossRef] [Green Version]
- Rashidi, M.M.; Freidoonimehr, N.; Hosseini, A.; Bég, O.A.; Hung, T.K. Homotopy simulation of nanofluid dynamics from a non-linearly stretching isothermal permeable sheet with transpiration. Meccanica 2014, 49, 469–482. [Google Scholar] [CrossRef]
- Turkyilmazoglu, M. Nanofluid flow and heat transfer due to a rotating disk. Comput. Fluids 2014, 94, 139–146. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Ganji, D.D. Ferrohydrodynamic and magnetohydrodynamic effects on ferrofluid flow and convective heat transfer. Energy 2014, 75, 400–410. [Google Scholar] [CrossRef]
- Moraveji, M.K.; Hejazian, M. Natural convection in a rectangular enclosure containing an oval-shaped heat source and filled with Fe3O4/water nanofluid. Int. Commun. Heat Mass Transf. 2013, 44, 135–146. [Google Scholar] [CrossRef]
- Aydın, O.; Kaya, A. MHD mixed convective heat transfer flow about an inclined plate. Heat Mass Transf. 2009, 46, 129–136. [Google Scholar] [CrossRef]
- Ellahi, R. The effects of MHD and temperature dependent viscosity on the flow of non-Newtonian nanofluid in a pipe: Analytical solutions. Appl. Math. Model. 2013, 37, 1451–1467. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Hatami, M.; Ganji, D.D. Nanofluid flow and heat transfer in a rotating system in the presence of a magnetic field. J. Mol. Liq. 2014, 190, 112–120. [Google Scholar] [CrossRef]
- Takhar, H.S.; Nath, G. Unsteady flow over a stretching surface with a magnetic field in a rotating fluid. Z. Für Angew. Math. Und Phys. 1998, 49, 989–1001. [Google Scholar] [CrossRef]
- Hayat, T.; Shafique, M.; Tanveer, A.; Alsaedi, A. Magnetohydrodynamic effects on peristaltic flow of hyperbolic tangent nanofluid with slip conditions and Joule heating in an inclined channel. Int. J. Heat Mass Transf. 2016, 102, 54–63. [Google Scholar] [CrossRef]
- Rizwana, R.; Hussain, A.; Nadeem, S. Mix convection non-boundary layer flow of unsteady MHD oblique stagnation point flow of nanofluid. Int. Commun. Heat Mass Transf. 2021, 124, 105285. [Google Scholar] [CrossRef]
- Ahmed, Z.; Nadeem, S.; Saleem, S.; Ellahi, R. Numerical study of unsteady flow and heat transfer CNT-based MHD nanofluid with variable viscosity over a permeable shrinking surface. Int. J. Numer. Methods Heat Fluid Flow 2019, 29, 4607–4623. [Google Scholar] [CrossRef]
- Ahmad, S.; Nadeem, S.; Ullah, N. Entropy generation and temperature-dependent viscosity in the study of SWCNT–MWCNT hybrid nanofluid. Appl. Nanosci. 2020, 10, 5107–5119. [Google Scholar] [CrossRef]
- Shahid, A.; Huang, H.L.; Khalique, C.M.; Bhatti, M.M. Numerical analysis of activation energy on MHD nanofluid flow with exponential temperature-dependent viscosity past a porous plate. J. Ther. Anal. Calorim. 2021, 143, 2585–2596. [Google Scholar] [CrossRef]
- Bahiraei, M.; Hosseinalipour, S.M.; Hangi, M. Numerical study and optimization of hydrothermal characteristics of Mn–Zn ferrite nanofluid within annulus in the presence of magnetic field. J. Supercond. Novel Magn. 2014, 27, 527–534. [Google Scholar] [CrossRef]
- Zhang, X.; Huang, H. Effect of magnetic obstacle on fluid flow and heat transfer in a rectangular duct. Int. Commun. Heat Mass Transf. 2014, 51, 31–38. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Bandpy, M.G.; Ellahi, R.; Zeeshan, A. Simulation of MHD CuO–water nanofluid flow and convective heat transfer considering Lorentz forces. J. Magn. Magn. Mater. 2014, 369, 69–80. [Google Scholar] [CrossRef]
- Bahiraei, M.; Hangi, M. Flow and heat transfer characteristics of magnetic nanofluids: A review. J. Magn. Magn. Mater. 2015, 374, 125–138. [Google Scholar] [CrossRef]
- Mahian, O.; Kolsi, L.; Amani, M.; Estellé, P.; Ahmadi, G.; Kleinstreuer, C.; Pop, I. Recent advances in modeling and simulation of nanofluid flows-Part I: Fundamentals and theory. Phys. Rep. 2019, 790, 1–48. [Google Scholar] [CrossRef]
- Waqas, H.; Farooq, U.; Naseem, R.; Hussain, S.; Alghamdi, M. Impact of MHD radiative flow of hybrid nanofluid over a rotating disk. Case Stud. Ther. Eng. 2021, 26, 101015. [Google Scholar] [CrossRef]
- Yasin, M.H.M.; Ishak, A.; Pop, I. MHD heat and mass transfer flow over a permeable stretching/shrinking sheet with radiation effect. J. Magn. Magn. Mater. 2016, 407, 235–240. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Rashidi, M.M.; Ganji, D.D. Effect of non-uniform magnetic field on forced convection heat transfer of Fe3O4–water nanofluid. Comput. Methods Appl. Mech. Eng. 2015, 294, 299–312. [Google Scholar] [CrossRef]
- Pantokratoras, A.; Fang, T. Blasius flow with non-linear Rosseland thermal radiation. Meccanica 2014, 49, 1539–1545. [Google Scholar] [CrossRef]
- Mushtaq, A.; Mustafa, M.; Hayat, T.; Alsaedi, A. Effects of thermal radiation on the stagnation-point flow of upper-convected Maxwell fluid over a stretching sheet. J. Aerosp. Eng. 2014, 27, 04014015. [Google Scholar] [CrossRef]
- Imtiaz, M.; Hayat, T.; Alsaedi, A.; Ahmad, B. Convective flow of carbon nanotubes between rotating stretchable disks with thermal radiation effects. Int. J. Heat Mass Transf. 2016, 101, 948–957. [Google Scholar] [CrossRef]
- Cortell, R. Effects of viscous dissipation and radiation on the thermal boundary layer over a nonlinearly stretching sheet. Phys. Lett. A 2008, 372, 631–636. [Google Scholar] [CrossRef]
- Hady, F.M.; Ibrahim, F.S.; Abdel-Gaied, S.M.; Eid, M.R. Radiation effect on viscous flow of a nanofluid and heat transfer over a nonlinearly stretching sheet. Nanoscale Res. Lett. 2012, 7, 229. [Google Scholar] [CrossRef] [Green Version]
- Hussain, A.; Rehman, A.; Nadeem, S.; Malik, M.Y.; Issakhov, A.; Sarwar, L.; Hussain, S. A combined convection Carreau–Yasuda nanofluid model over a convective heated surface near a stagnation point: A numerical study. Math. Prob. Eng. 2021, 2021, 6665743. [Google Scholar] [CrossRef]
- Benos, L.T.; Mahabaleshwar, U.S.; Sakanaka, P.H.; Sarris, I.E. Thermal analysis of the unsteady sheet stretching subject to slip and magnetohydrodynamic effects. Ther. Sci. Eng. Prog. 2019, 13, 100367. [Google Scholar] [CrossRef]
- Aslani, K.E.; Mahabaleshwar, U.S.; Singh, J.; Sarris, I.E. Combined Effect of Radiation and Inclined MHD Flow of a Micropolar Fluid Over a Porous Stretching/Shrinking Sheet with Mass Transpiration. Int. J. Appl. Comput. Math. 2021, 7, 60. [Google Scholar] [CrossRef]
- Hussain, A.; Sarwar, L.; Akbar, S.; Malik, M.Y.; Ghafoor, S. Model for MHD viscoelastic nanofluid flow with prominence effects of radiation. Heat Transf. Asian Res. 2019, 48, 463–482. [Google Scholar] [CrossRef]
- Mousavi, S.M.; Rostami, M.N.; Yousefi, M.; Dinarvand, S.; Pop, I.; Sheremet, M.A. Dual solutions for Casson hybrid nanofluid flow due to a stretching/shrinking sheet: A new combination of theoretical and experimental models. Chin. J. Phys. 2021, 71, 574–588. [Google Scholar] [CrossRef]
- Mikhailenko, S.A.; Sheremet, M.A.; Oztop, H.F.; Abu-Hamdeh, N. Thermal convection in Al2O3–water nanoliquid rotating chamber with a local isothermal heater. Int. J. Mech. Sci. 2019, 156, 137–145. [Google Scholar] [CrossRef]
- Mikhailenko, S.A.; Sheremet, M.A.; Pop, I. Convective heat transfer in a rotating nanofluid cavity with sinusoidal temperature boundary condition. J. Ther. Anal. Calorim. 2019, 137, 799–809. [Google Scholar] [CrossRef]
- Mustafa, M.; Mushtaq, A.; Hayat, T.; Ahmad, B. Nonlinear radiation heat transfer effects in the natural convective boundary layer flow of nanofluid past a vertical plate: A numerical study. PLoS ONE 2014, 9, e103946. [Google Scholar] [CrossRef]
- Tiwari, R.K.; Das, M.K. Heat transfer augmentation in a two-sided lid-driven differentially heated square cavity utilizing nanofluids. Int. J. Heat Mass Transf. 2007, 50, 2002–2018. [Google Scholar] [CrossRef]
- Nadeem, S.; Ur Rehman, A.; Mehmood, R.; Adil Sadiq, M. Partial Slip effects on a rotating flow of two phase nanofluid over a stretching surface. Curr. Nanosci. 2014, 10, 846–854. [Google Scholar] [CrossRef]
- Maxwell, J.C. A Treatise on Electricity and Magnetism; Clarendon Press: Oxford, UK, 1873; Volume 1. [Google Scholar]
- Mushtaq, A.; Mustafa, M.; Hayat, T.; Alsaedi, A. On the numerical solution of the nonlinear radiation heat transfer problem in a three-dimensional flow. Z. Nat. A 2014, 69, 705–713. [Google Scholar] [CrossRef]






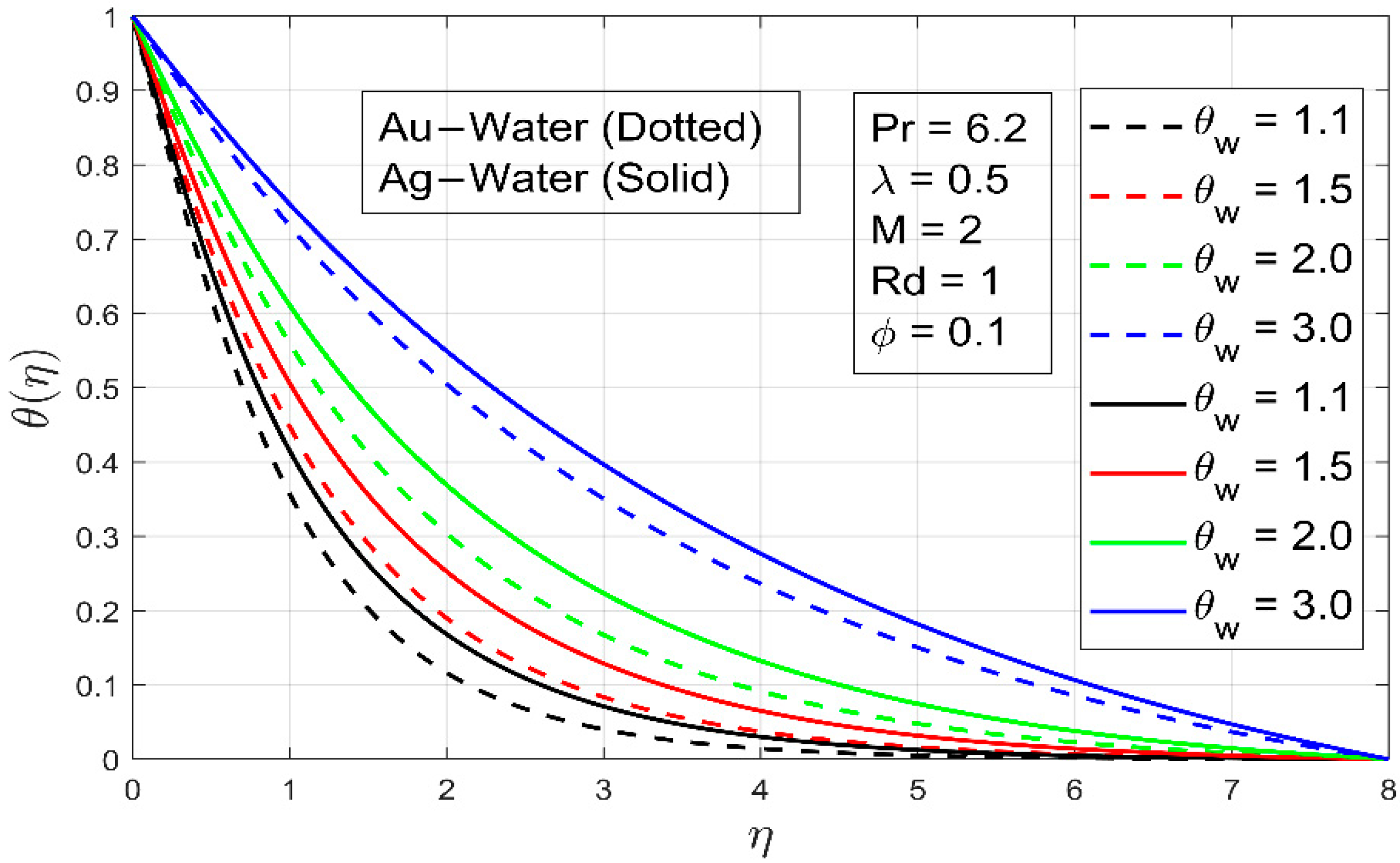


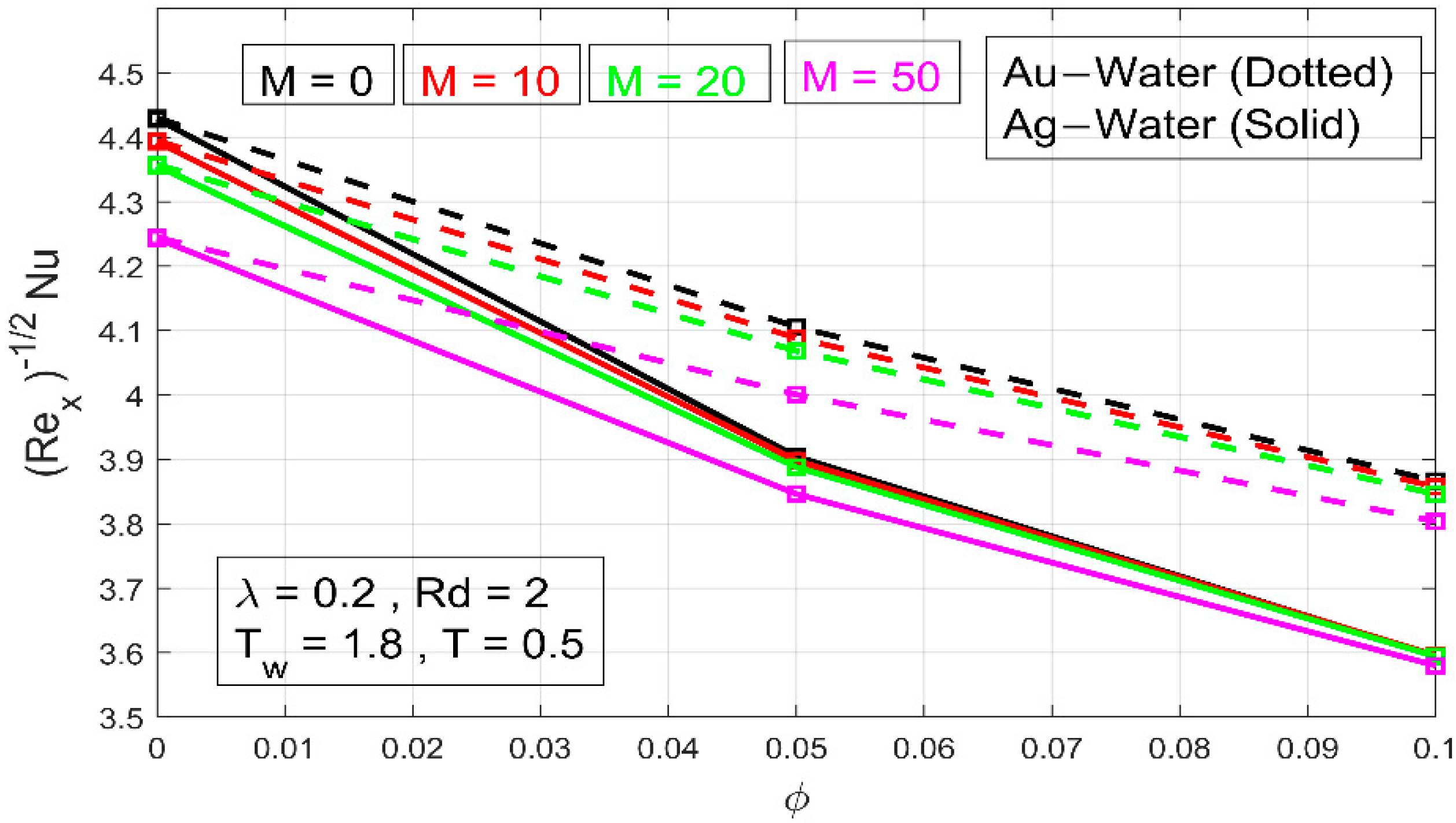
| Wang [1] | Nazar et al. [3] | Present Results | ||||
|---|---|---|---|---|---|---|
| Linear Radiation | Nonlinear Radiation | |||||
|---|---|---|---|---|---|---|
| Linear Radiation | Nonlinear Radiation | |||||
|---|---|---|---|---|---|---|
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hussain, A.; Elkotb, M.A.; Arshad, M.; Rehman, A.; Sooppy Nisar, K.; Hassan, A.; Saleel, C.A. Computational Investigation of the Combined Impact of Nonlinear Radiation and Magnetic Field on Three-Dimensional Rotational Nanofluid Flow across a Stretchy Surface. Processes 2021, 9, 1453. https://doi.org/10.3390/pr9081453
Hussain A, Elkotb MA, Arshad M, Rehman A, Sooppy Nisar K, Hassan A, Saleel CA. Computational Investigation of the Combined Impact of Nonlinear Radiation and Magnetic Field on Three-Dimensional Rotational Nanofluid Flow across a Stretchy Surface. Processes. 2021; 9(8):1453. https://doi.org/10.3390/pr9081453
Chicago/Turabian StyleHussain, Azad, Mohamed Abdelghany Elkotb, Mubashar Arshad, Aysha Rehman, Kottakkaran Sooppy Nisar, Ali Hassan, and C. Ahamed Saleel. 2021. "Computational Investigation of the Combined Impact of Nonlinear Radiation and Magnetic Field on Three-Dimensional Rotational Nanofluid Flow across a Stretchy Surface" Processes 9, no. 8: 1453. https://doi.org/10.3390/pr9081453
APA StyleHussain, A., Elkotb, M. A., Arshad, M., Rehman, A., Sooppy Nisar, K., Hassan, A., & Saleel, C. A. (2021). Computational Investigation of the Combined Impact of Nonlinear Radiation and Magnetic Field on Three-Dimensional Rotational Nanofluid Flow across a Stretchy Surface. Processes, 9(8), 1453. https://doi.org/10.3390/pr9081453









