A Discrete Multi-Physics Model to Simulate Fluid Structure Interaction and Breakage of Capsules Filled with Liquid under Coaxial Load
Abstract
1. Introduction
2. Methodology
2.1. Modelling Approach
2.1.1. Smoothed Particle Hydrodynamics (SPH)
2.1.2. Lattice Spring Model (LSM)
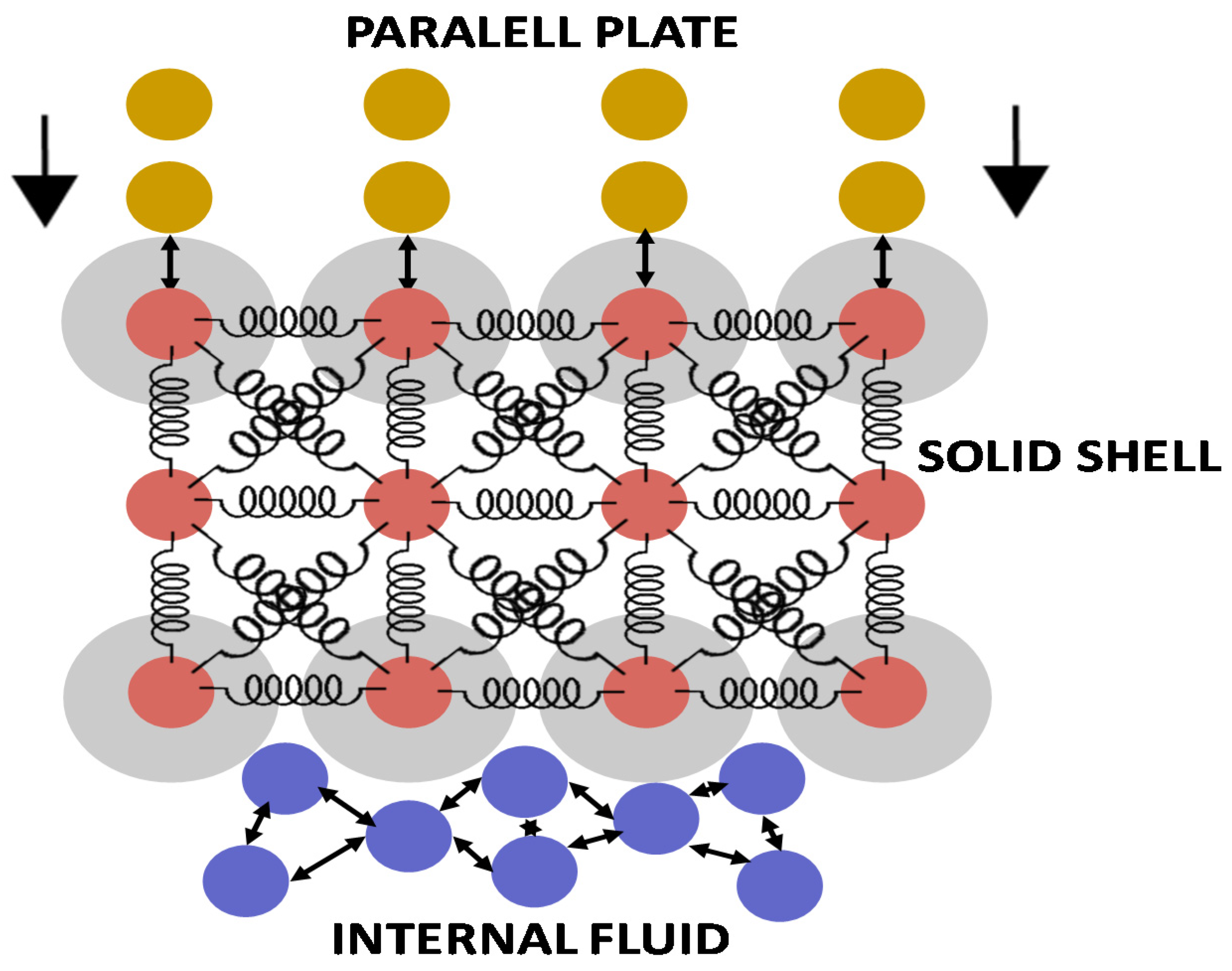
2.1.3. Coupling the Two Models
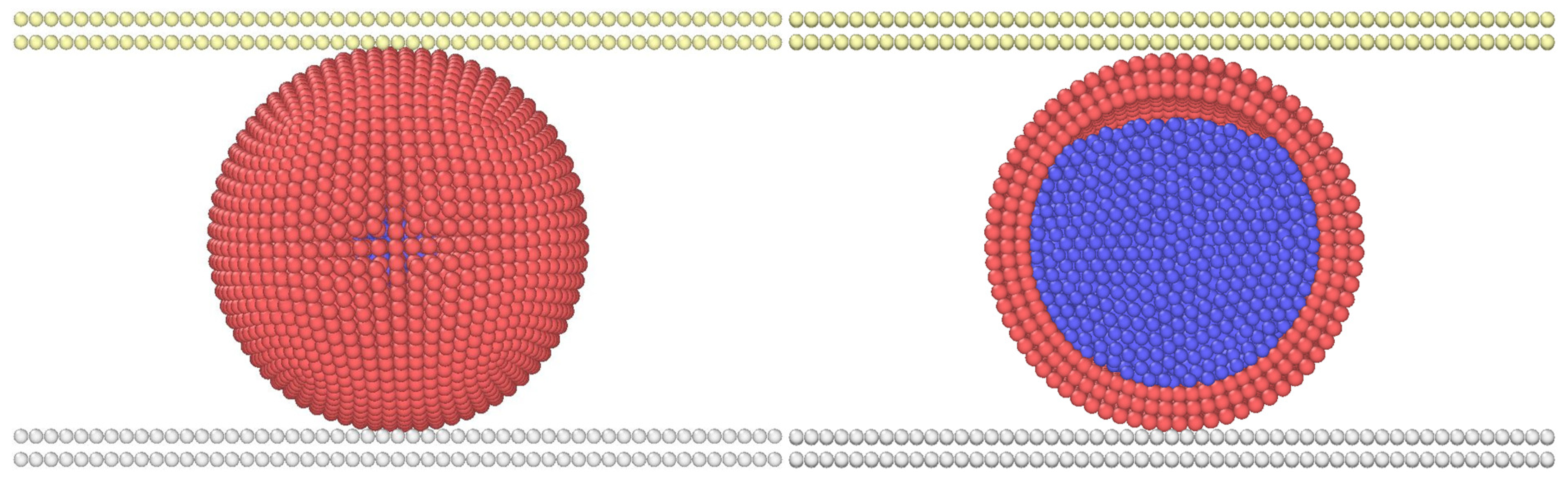
2.2. Geometry
2.3. Main Simulation Parameters
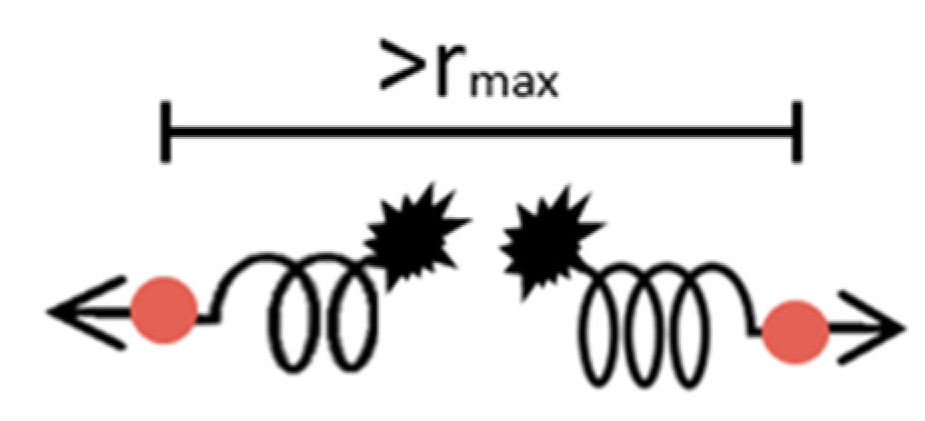
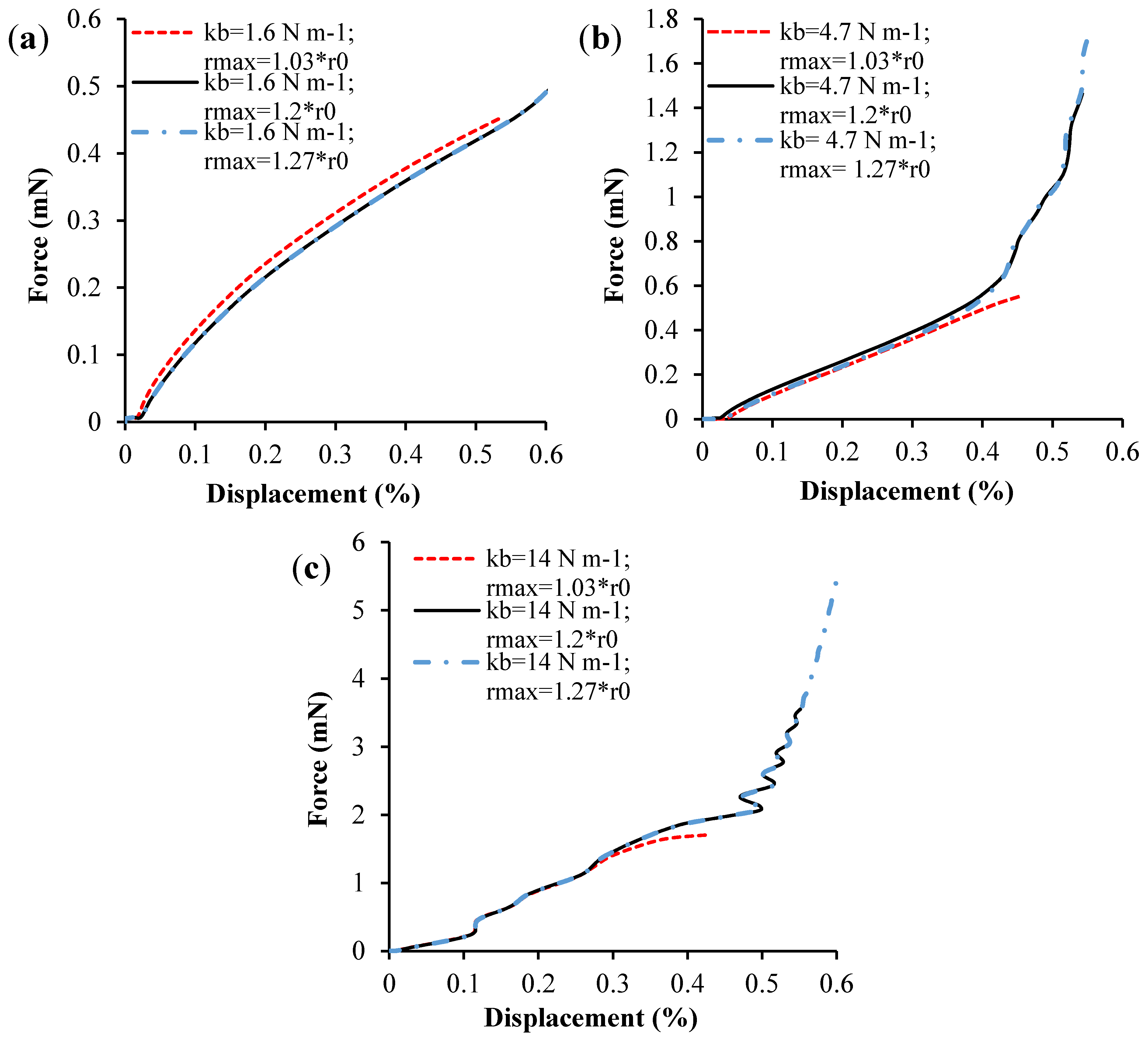
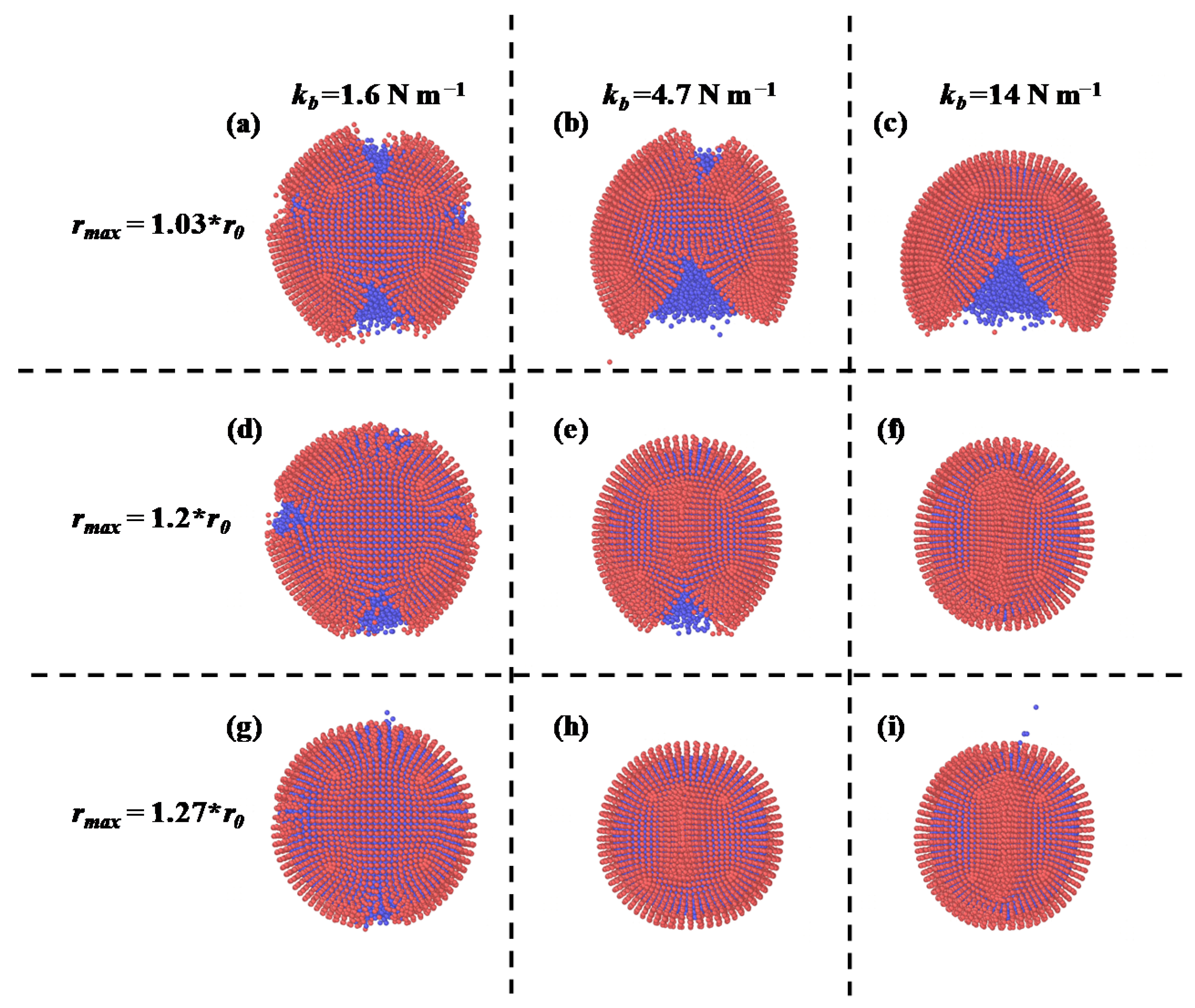
2.4. Parametric Study and Influence of kb and rmax
3. Results and Discussion
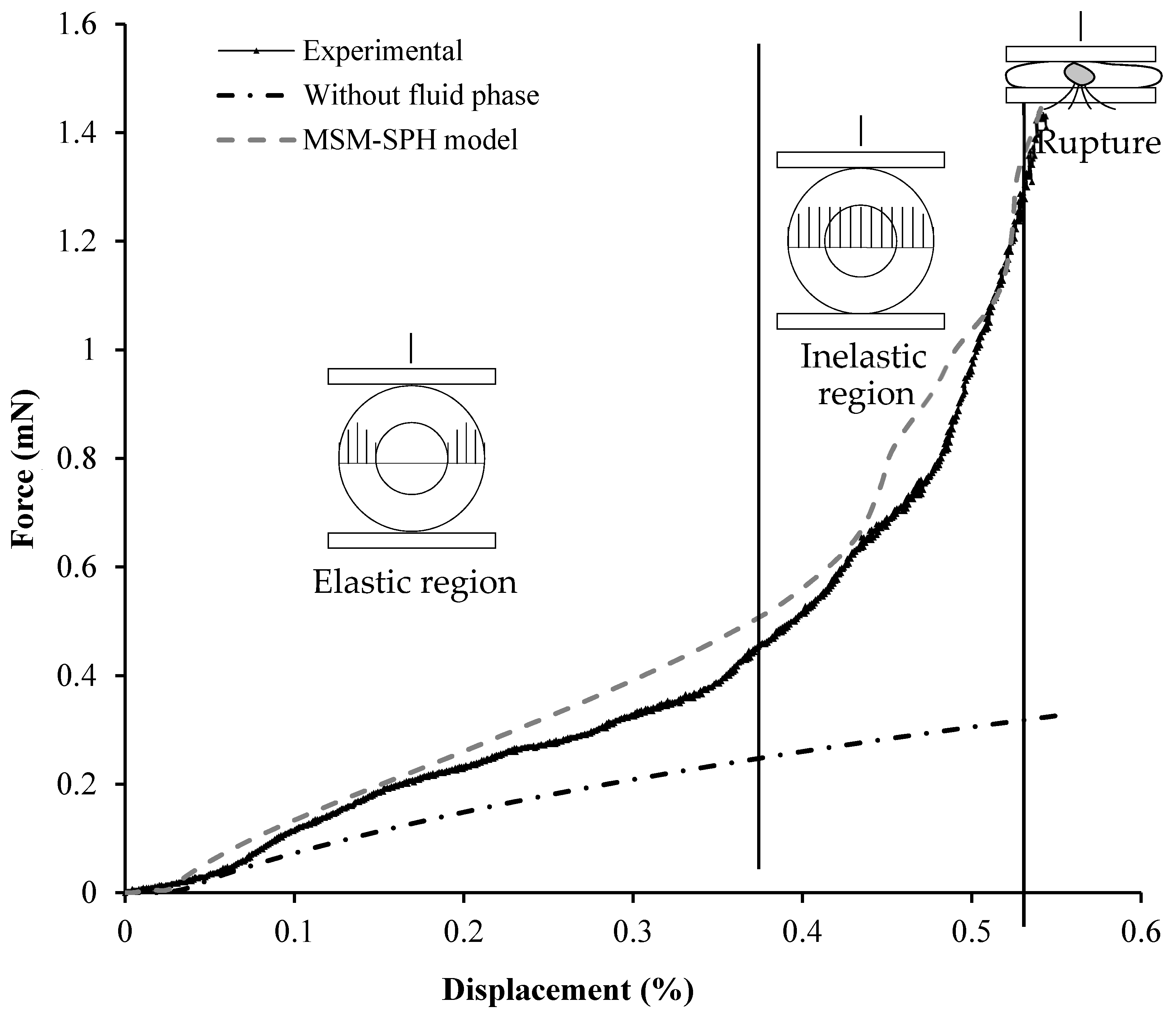
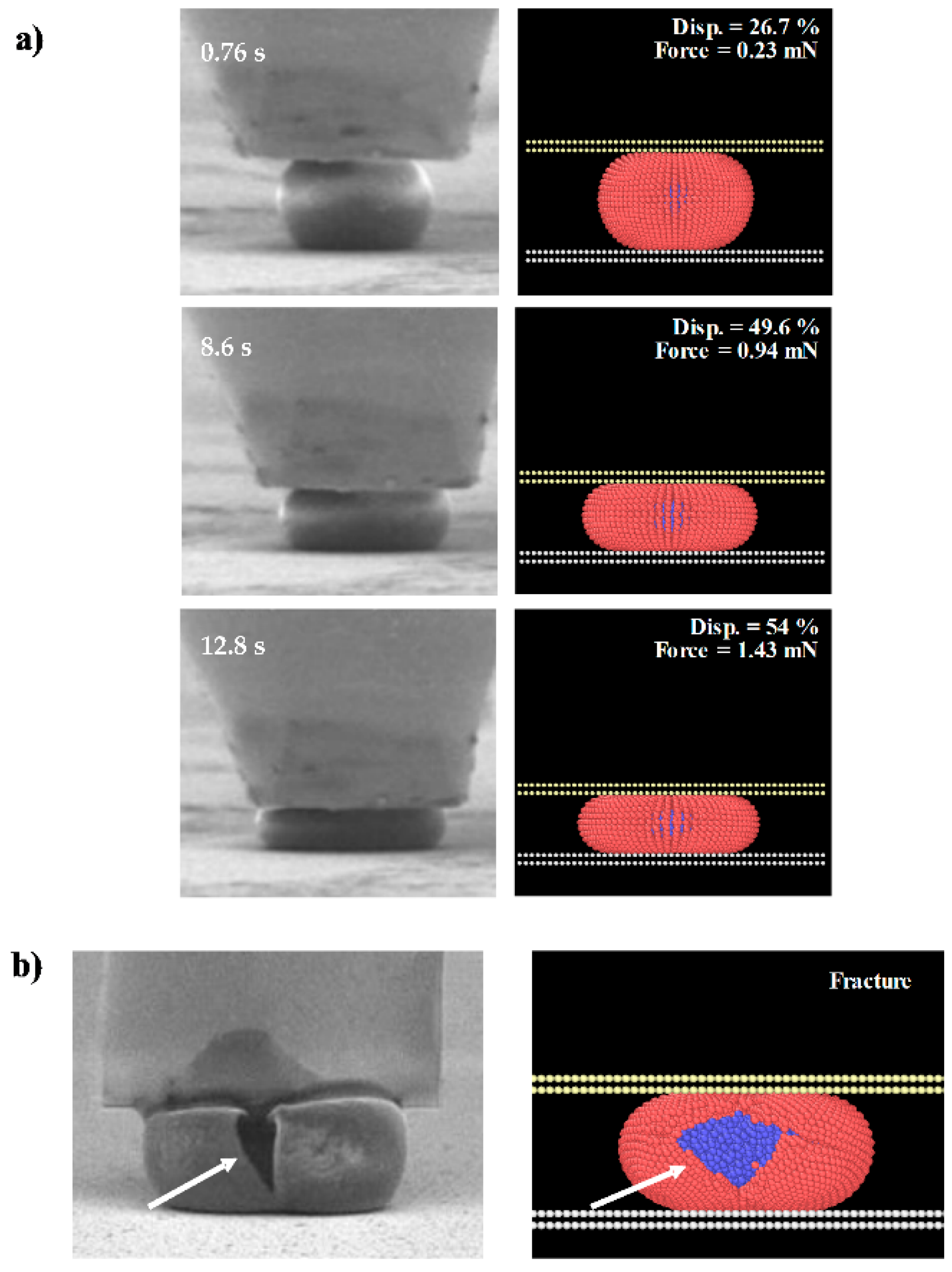
3.1. Validation of the Simulation of Capsule Compression between Two Parallel Plates
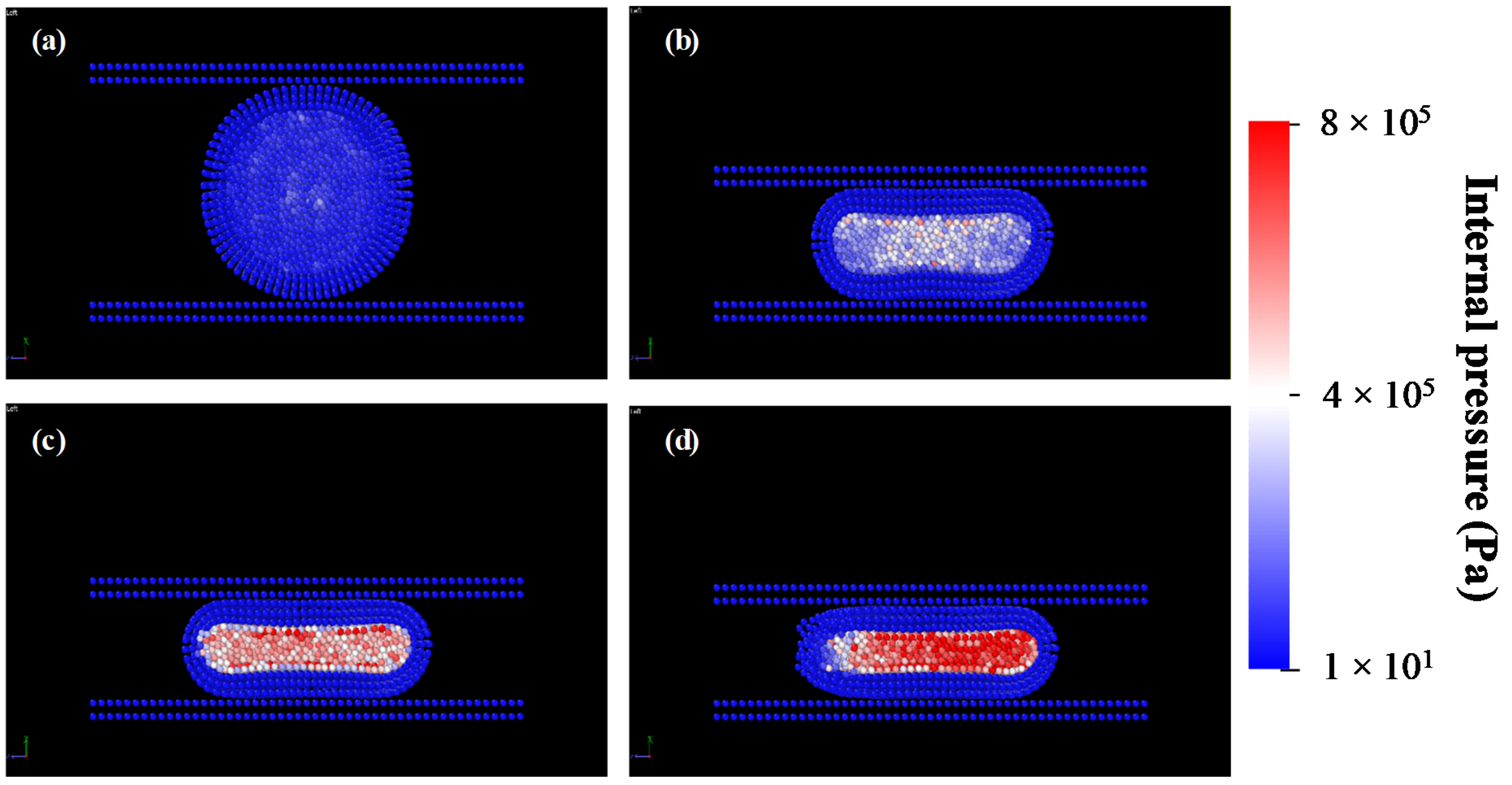
3.2. Influence of the Fluid on the Capsule’s Strength
3.3. Deformability and Strength of the Capsule (a Parametric Study)
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Trojanowska, A.; Nogalska, A.; Garcia, R.; Giamberini, M.; Tylkowski, B. Technological Solutions for Encapsulation. J. Magn. Magn. Mater. 2017, 2, 1–20. [Google Scholar]
- Martins, E.; Poncelet, D.; Rodrigues, R.C.; Renard, D. Oil Encapsulation Techniques Using Alginate as Encapsulating Agent: Applications and Drawbacks. J. Microencapsul. 2017, 34, 754–771. [Google Scholar] [CrossRef]
- Thuong, N.P.; Thanh, V.T.; Cang, M.H.; Lam, T.D.; Huong, N.C.; Hong Nhan, L.T.; Truc, T.T.; Tran, Q.T.; Bach, L.G. Microencapsulation of Lemongrass (Cymbopogon Citratus) Essential Oil via Spray Drying: Effects of Feed Emulsion Parameters. Processes 2020, 8, 40. [Google Scholar] [CrossRef]
- O’Sullivan, M.; Zhang, Z.; Vincent, B. Silica-Shell/Oil-Core Microcapsules with Controlled Shell Thickness and Their Breakage Stress. Langmuir 2009, 25, 7962–7966. [Google Scholar] [CrossRef]
- Norambuena-contreras, J.; Yalcin, E.; Garcia, A.; Al-mansoori, T.; Yilmaz, M.; Hudson-griffiths, R. Effect of Mixing and Ageing on the Mechanical and Self-Healing Properties of Asphalt Mixtures Containing Polymeric Capsules. Constr. Build. Mater. 2018, 175, 254–266. [Google Scholar] [CrossRef]
- Zhu, D.Y.; Rong, M.Z.; Zhang, M.Q. Self-Healing Polymeric Materials Based on Microencapsulated Healing Agents: From Design to Preparation. Prog. Polym. Sci. 2015, 49–50, 175–220. [Google Scholar] [CrossRef]
- Casanova, F.; Santos, L. Encapsulation of Cosmetic Active Ingredients for Topical Application-a Review. J. Microencapsul. 2016, 33, 1–17. [Google Scholar] [CrossRef]
- Tsai, F.H.; Chiang, P.Y.; Kitamura, Y.; Kokawa, M.; Islam, M.Z. Producing Liquid-Core Hydrogel Beads by Reverse Spherification: Effect of Secondary Gelation on Physical Properties and Release Characteristics. Food Hydrocoll. 2017, 62, 140–148. [Google Scholar] [CrossRef]
- Stan, M.S.; Chirila, L.; Popescu, A.; Radulescu, D.M.; Radulescu, D.E.; Dinischiotu, A. Essential Oil Microcapsules Immobilized on Textiles and Certain Induced Effects. Materials 2019, 12, 2029. [Google Scholar] [CrossRef]
- Rodríguez-Dorado, R.; Landín, M.; Altai, A.; Russo, P.; Aquino, R.P.; Del Gaudio, P. A Novel Method for the Production of Core-Shell Microparticles by Inverse Gelation Optimized with Artificial Intelligent Tools. Int. J. Pharm. 2018, 538, 97–104. [Google Scholar] [CrossRef]
- Sheng, W.; Li, W.; Li, B.; Li, C.; Xu, Y.; Guo, X.; Zhou, F.; Jia, X. Mussel-Inspired Photografting on Colloidal Spheres: A Generalized Self-Template Route to Stimuli-Responsive Hollow Spheres for Controlled Pesticide Release. Macromol. Rapid Commun. 2015, 36, 1640–1645. [Google Scholar] [CrossRef]
- Huang, H.; Ye, G.; Qian, C.; Schlangen, E. Self-Healing in Cementitious Materials: Materials, Methods and Service Conditions. Mater. Des. 2016, 92, 499–511. [Google Scholar] [CrossRef]
- García, Á.; Schlangen, E.; Van De Ven, M. Properties of Capsules Containing Rejuvenators for Their Use in Asphalt Concrete. Fuel 2011, 90, 583–591. [Google Scholar] [CrossRef]
- Al-Mansoori, T.; Norambuena-Contreras, J.; Micaelo, R.; Garcia, A. Self-Healing of Asphalt Mastic by the Action of Polymeric Capsules Containing Rejuvenators. Constr. Build. Mater. 2018, 161, 330–339. [Google Scholar] [CrossRef]
- Mercadé-Prieto, R.; Zhang, Z. Mechanical Characterization of Microspheres Capsules, Cells and Beads: A Review. J. Microencapsul. 2012, 29, 277–285. [Google Scholar] [CrossRef] [PubMed]
- Walter, J.; Salsac, A.V.; Barthes-Biesel, D.; Le Tallec, P. Coupling of Finite Element and Boundary Integral Methods for a Capsule in a Stokes Flow. Int. J. Numer. Methods Eng. 2010, 83, 829–850. [Google Scholar] [CrossRef]
- Müller, M.; Schirm, S.; Teschner, M.; Heidelberger, B.; Gross, M. Interaction of Fluids with Deformable Solids. Comput. Animat. Virtual Worlds 2004, 15, 159–171. [Google Scholar] [CrossRef]
- Alexiadis, A. The Discrete Multi-Hybrid System for the Simulation of Solid-Liquid Flows. PLoS ONE 2015, 10, e0124678. [Google Scholar] [CrossRef]
- Ariane, M.; Wen, W.; Vigolo, D.; Brill, A.; Nash, F.G.B.; Barigou, M.; Alexiadis, A. Modelling and Simulation of Flow and Agglomeration in Deep Veins Valves Using Discrete Multi Physics. Comput. Biol. Med. 2017, 89, 96–103. [Google Scholar] [CrossRef]
- Alexiadis, A.; Stamatopoulos, K.; Wen, W.; Batchelor, H.K.; Bakalis, S.; Barigou, M.; Simmons, M.J.H. Using Discrete Multi-Physics for Detailed Exploration of Hydrodynamics in an in Vitro Colon System. Comput. Biol. Med. 2017, 81, 188–198. [Google Scholar] [CrossRef]
- Ariane, M.; Kassinos, S.; Velaga, S.; Alexiadis, A. Discrete Multi-Physics Simulations of Diffusive and Convective Mass Transfer in Boundary Layers Containing Motile Cilia in Lungs. Comput. Biol. Med. 2018, 95, 34–42. [Google Scholar] [CrossRef]
- Ariane, M.; Vigolo, D.; Brill, A.; Nash, F.G.B.; Barigou, M.; Alexiadis, A. Using Discrete Multi-Physics for Studying the Dynamics of Emboli in Flexible Venous Valves. Comput. Fluids 2018, 166, 57–63. [Google Scholar] [CrossRef]
- Alexiadis, A.; Ghraybeh, S.; Qiao, G. Natural Convection and Solidification of Phase-Change Materials in Circular Pipes: A SPH Approach. Comput. Mater. Sci. 2018, 150, 475–483. [Google Scholar] [CrossRef]
- Rahmat, A.; Barigou, M.; Alexiadis, A. Deformation and Rupture of Compound Cells under Shear: A Discrete Multiphysics Study. Phys. Fluids 2019, 31. [Google Scholar] [CrossRef]
- Mercadé-Prieto, R.; Nguyen, B.; Allen, R.; York, D.; Preece, J.A.; Goodwin, T.E.; Zhang, Z. Determination of the Elastic Properties of Single Microcapsules Using Micromanipulation and Finite Element Modeling. Chem. Eng. Sci. 2011, 66, 2042–2049. [Google Scholar] [CrossRef]
- Gray, A.; Egan, S.; Bakalis, S.; Zhang, Z. Determination of Microcapsule Physicochemical, Structural, and Mechanical Properties. Particuology 2016, 24, 32–43. [Google Scholar] [CrossRef]
- Monaghan, J.J. Smoothed Particle Hydrodynamics. Rep. Prog. Phys. 2005, 68, 1703–1759. [Google Scholar] [CrossRef]
- Huang, Y.J.; Nydal, O.J. Coupling of Discrete-Element Method and Smoothed Particle Hydrodynamics for Liquid-Solid Flows. Theor. Appl. Mech. Lett. 2012, 2, 012002. [Google Scholar] [CrossRef][Green Version]
- Lucy, L.B. A Numerical Approach to the Testing of the Fission Hypothesis. Astron. J. 1977, 82, 1013–1024. [Google Scholar] [CrossRef]
- Morris, J.P.; Fox, P.J.; Zhu, Y. Modeling Low Reynolds Number Incompressible Flows Using SPH. Int. J. Numer. Methods Fluids 1997, 136, 214–226. [Google Scholar] [CrossRef]
- Cole, R.H. Underwater Explosions; Pinceton University Press: Pinceton, NJ, USA, 1948. [Google Scholar]
- Kot, M.; Nagahashi, H.; Szymczak, P. Elastic Moduli of Simple Mass Spring Models. Vis. Comput. 2015, 31, 1339–1350. [Google Scholar] [CrossRef]
- Alexiadis, A. A New Framework for Modelling the Dynamics and the Breakage of Capsules, Vesicles and Cells in Fluid Flow. Procedia IUTAM 2015, 16, 80–88. [Google Scholar] [CrossRef]
- Plimpton, S. Fast Parallel Algorithms for Short-Range Molecular Dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef]
- Ganzenm, G.C.; Steinhauser, M.O.; Van Liedekerke, P. The Implementation of Smooth Particle Hydrodynamics in LAMMPS. 2011; pp. 1–23. Available online: https://lammps.sandia.gov/doc/PDF/SPH_LAMMPS_userguide.pdf (accessed on 10 December 2018).
- Sahputra, I.H.; Alexiadis, A.; Adams, M.J. A Coarse Grained Model for Viscoelastic Solids in Discrete Multiphysics Simulations. ChemEngineering 2020, 4, 30. [Google Scholar] [CrossRef]
- Yap, S.F.; Adams, M.J.; Seville, J.P.K.; Zhang, Z. Single and Bulk Compression of Pharmaceutical Excipients: Evaluation of Mechanical Properties. Powder Technol. 2008, 185, 1–10. [Google Scholar] [CrossRef]
- Van Liedekerke, P.; Tijskens, E.; Ramon, H.; Ghysels, P.; Samaey, G.; Roose, D. Particle-Based Model to Simulate the Micromechanics of Biological Cells. Phys. Rev. E Stat. Nonlinear Soft Matter Phys. 2010, 81, 1–15. [Google Scholar] [CrossRef]
- Ghasemi, H.; Darjani, S.; Mazloomi, H.; Mozaffari, S. Preparation of Stable Multiple Emulsions Using Food-Grade Emulsifiers: Evaluating the Effects of Emulsifier Concentration, W/O Phase Ratio, and Emulsification Process. SN Appl. Sci. 2020, 2. [Google Scholar] [CrossRef]
- Ghasemi, H.; Aghabarari, B.; Alizadeh, M.; Khanlarkhani, A.; Abu-Zahra, N. High Efficiency Decolorization of Wastewater by Fenton Catalyst: Magnetic Iron-Copper Hybrid Oxides. J. Water Process Eng. 2020, 37, 101540. [Google Scholar] [CrossRef]
- Sokolov, A.; Mozaffari, A.; Zhang, R.; De Pablo, J.J.; Snezhko, A. Emergence of Radial Tree of Bend Stripes in Active Nematics. Phys. Rev. X 2019, 9, 31014. [Google Scholar] [CrossRef]
- Li, J.; Dong, S.; Hua, W.; Li, X.; Pan, X. Numerical Investigation of Hydraulic Fracture Propagation Based on Cohesive Zone Model in Naturally Fractured Formations. Processes 2019, 7, 28. [Google Scholar] [CrossRef]
- Ren, Y.L.; Donald, A.M.; Zhang, Z.B. Investigation of Radiation Damage to Microcapsules in Environmental SEM. Mater. Sci. Technol. 2007, 23, 857–864. [Google Scholar] [CrossRef]
| SPH | |
| Number of SPH fluid particles | 5731 |
| Mass of each particle, m | 2.7 × 10−15 kg |
| Initial distance among particles, dL | 1.24 × 10−6 m |
| Smoothing length, h | 1.1∙dL |
| Artificial sound speed, | 1.1 m s−1 |
| Density, ρ0 | 900 kg m−3 |
| Viscosity, µ0 | 0.001 Pa∙s |
| LSM | |
| Number of particles (shell) | 5604 |
| Mass of each particle | 2.7 × 10−15 kg |
| Hookean coefficient, kb | 4.6 N m−1 |
| Maximum distance for fracture, rmax | 1.2 |
| Shell thickness, t0 | 2.5 × 10−6 m |
| Capsule diameter | 31 × 10−6 m |
| BOUNDARIES | |
| Repulsive potential constant, | 5 × 10−15 J |
| Repulsive radius, σ | 1.1∙dL |
| Repulsive coefficient, a | 1 |
| Repulsive coefficient, b | 0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ruiz-Riancho, I.N.; Alexiadis, A.; Zhang, Z.; Garcia Hernandez, A. A Discrete Multi-Physics Model to Simulate Fluid Structure Interaction and Breakage of Capsules Filled with Liquid under Coaxial Load. Processes 2021, 9, 354. https://doi.org/10.3390/pr9020354
Ruiz-Riancho IN, Alexiadis A, Zhang Z, Garcia Hernandez A. A Discrete Multi-Physics Model to Simulate Fluid Structure Interaction and Breakage of Capsules Filled with Liquid under Coaxial Load. Processes. 2021; 9(2):354. https://doi.org/10.3390/pr9020354
Chicago/Turabian StyleRuiz-Riancho, Ignacio Nilo, Alessio Alexiadis, Zhibing Zhang, and Alvaro Garcia Hernandez. 2021. "A Discrete Multi-Physics Model to Simulate Fluid Structure Interaction and Breakage of Capsules Filled with Liquid under Coaxial Load" Processes 9, no. 2: 354. https://doi.org/10.3390/pr9020354
APA StyleRuiz-Riancho, I. N., Alexiadis, A., Zhang, Z., & Garcia Hernandez, A. (2021). A Discrete Multi-Physics Model to Simulate Fluid Structure Interaction and Breakage of Capsules Filled with Liquid under Coaxial Load. Processes, 9(2), 354. https://doi.org/10.3390/pr9020354







