Estimating Relaxation Time and Fractionality Order Parameters in Fractional Non-Fourier Heat Conduction Using Conjugate Gradient Inverse Approach in Single and Three-Layer Skin Tissues
Abstract
:1. Introduction
2. Conjugate Gradient Inverse Method
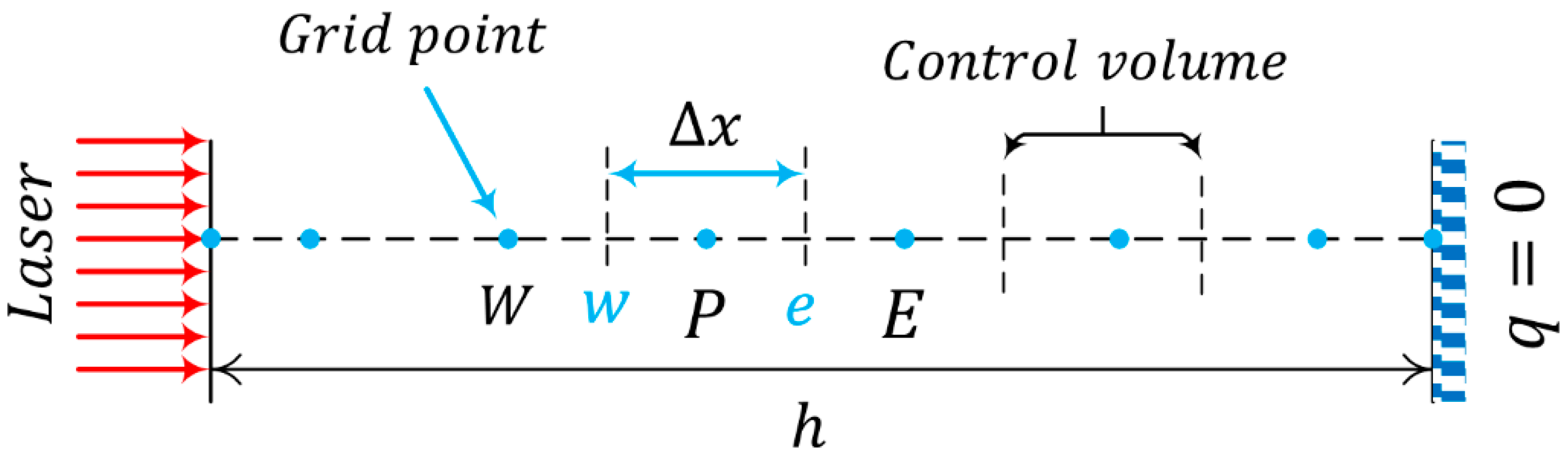
3. Governing Equations and Discretization
3.1. Test Case 1
3.1.1. Direct Problem
3.1.2. Inverse Problem
3.2. Test Case 2
3.2.1. Direct Problem
3.2.2. Inverse Problem
4. Results and Discussion
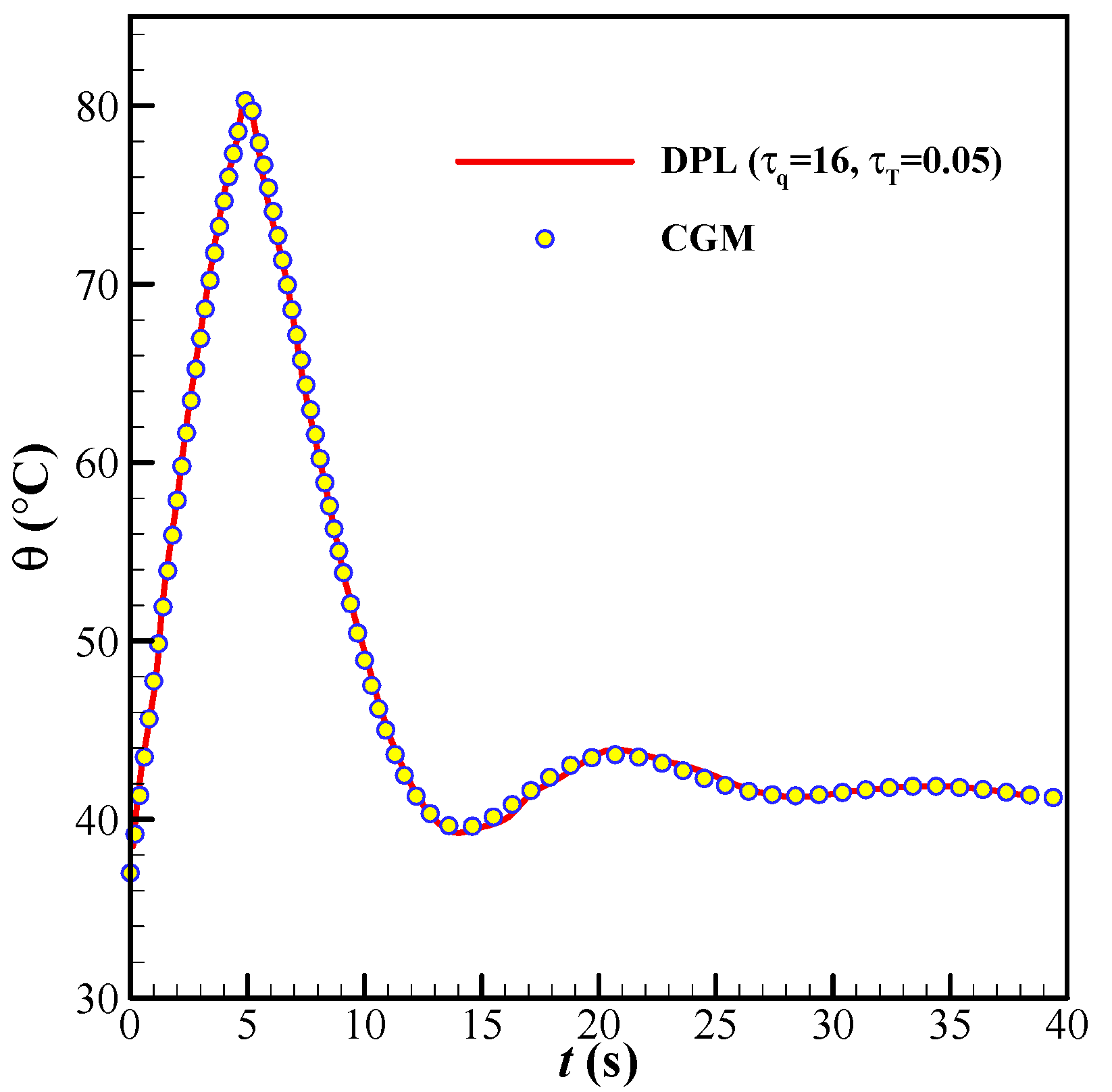
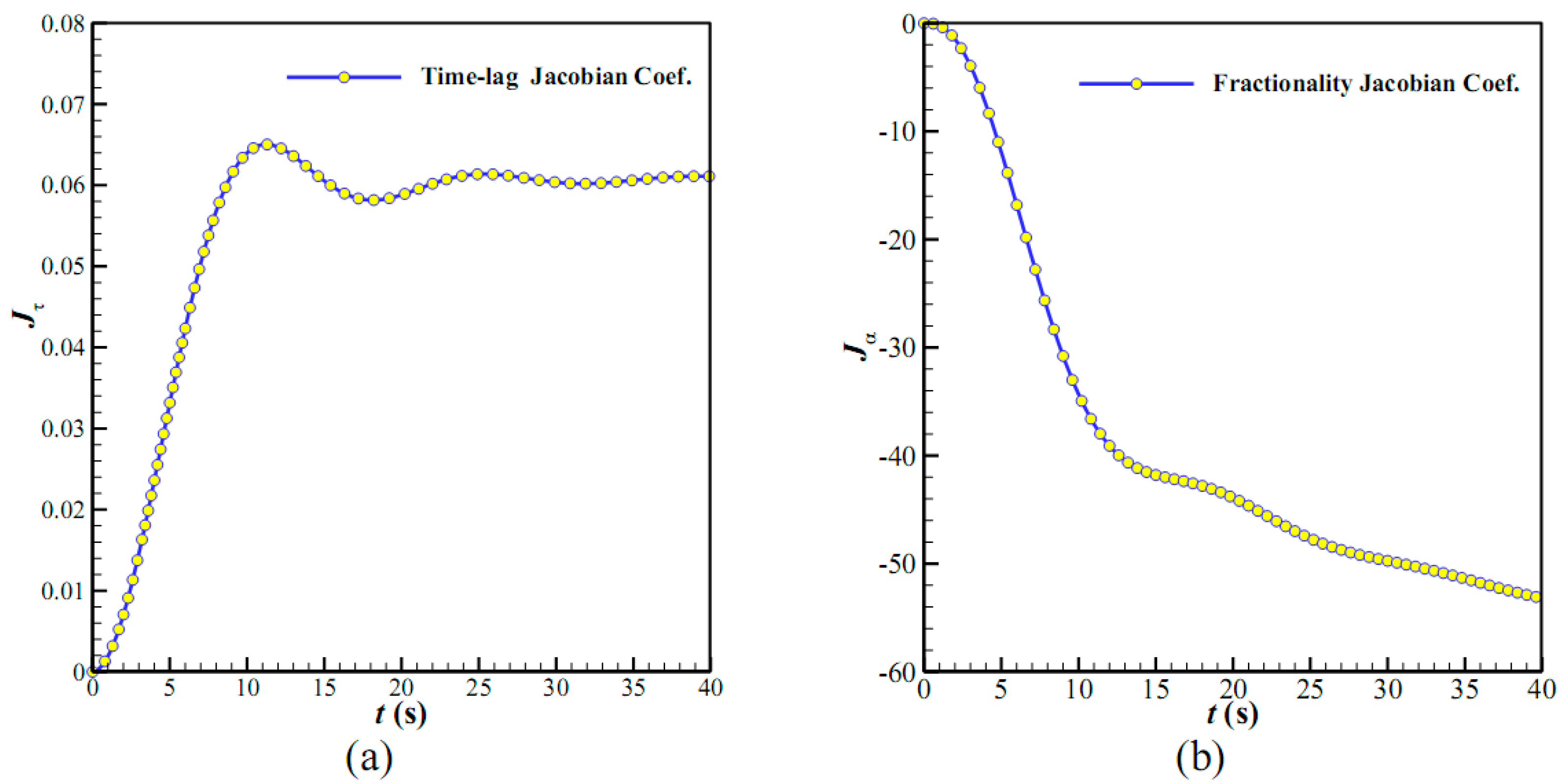
4.1. Test Case 1
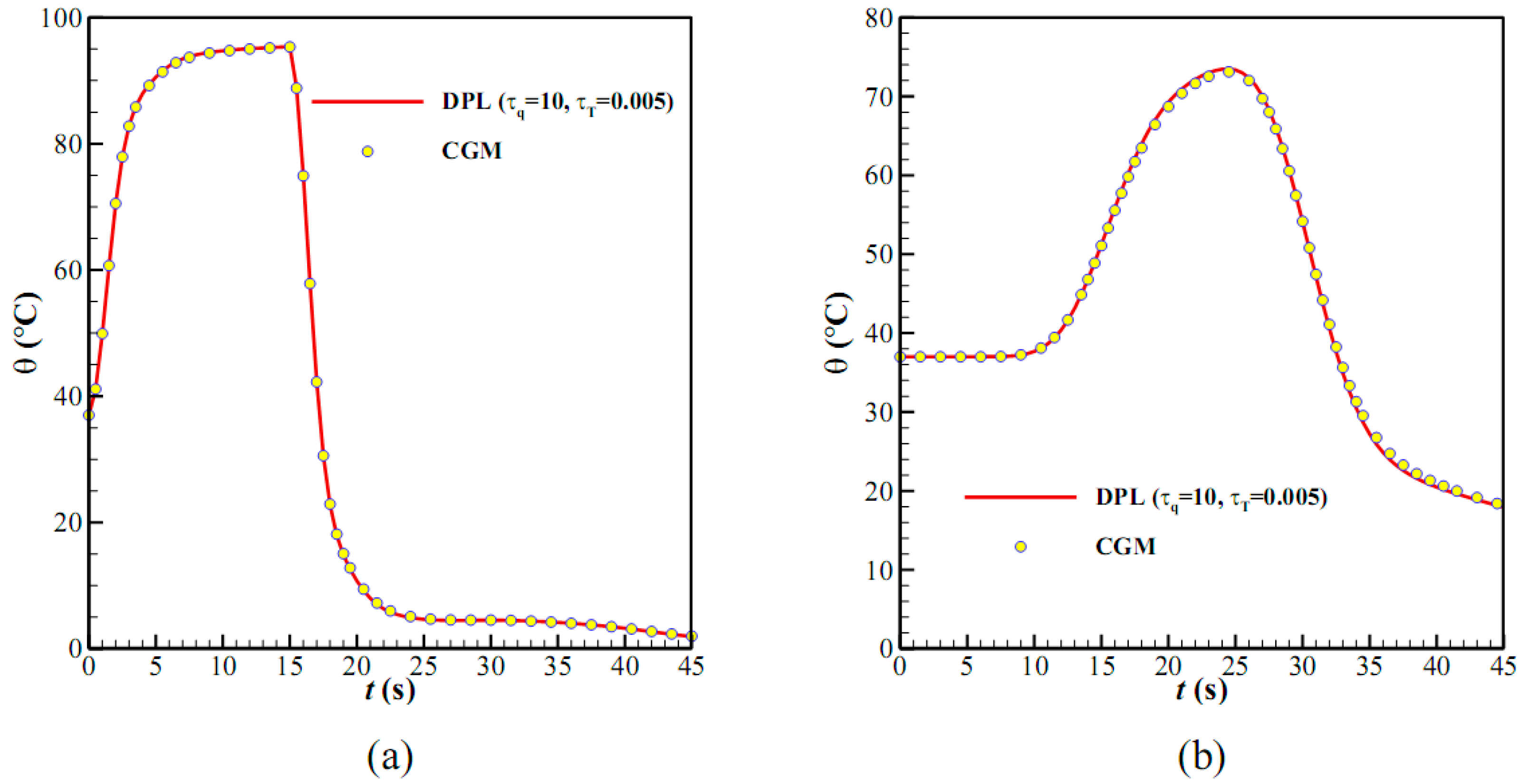
4.2. Test Case 2
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Latin symbols | |
| c | tissue heat capacity, Jkg−1 K−1 |
| cb | blood heat capacity Jkg−1 K−1 |
| D | coefficient of thermal diffusion WJ−1 m−3 |
| time derivative order | |
| f(t) | continuous function |
| h | skin thickness (mm) |
| sensitivity coefficient of heat respect to order of fractionality | |
| sensitivity coefficient of heat respect to time lag | |
| Jα | sensitivity coefficient respect to order of fractionality |
| Jτ | sensitivity coefficient respect to time lag |
| k | tissue thermal conductivity, Wm−1 K−1 |
| m | number of iterations |
| P | unknown parameters in inverse problem |
| qgen | generated heat in tissue, Wm−3 |
| qmet | metabolic heating source, Wm−3 |
| Qin | laser intensity, Wcm−2 |
| Rd | diffusion reflection |
| t | time, s |
| tf | time duration from the onset to the end, s |
| tτ | time period of laser radiation on the skin, s |
| u(t) | unit step function |
| wb | blood perfusion rate, m3m−3 tissue |
| wj | average of weighted arithmetic |
| wr | weight function |
| Greek symbols | |
| α | order of fractional derivative |
| α0 | initial guess for order of fractional derivative |
| ε | error tolerance |
| θ | temperature of tissue, °C |
| θ0 | initial temperature, °C |
| θb | blood temperature, °C |
| θc | calculated temperature, °C |
| θDPL | measured temperature, °C |
| ρ | density of tissue, kgm−3 |
| ρb | blood density, kgm−3 |
| Γ | gamma function |
| τ | time lag |
| τT | temperature gradient time lag |
| τq | heat flux time lag |
| τ0 | initial guess for time lag |
| Abbreviations | |
| ACGM | Adjoint Conjugate Gradient Method |
| CGIM | Conjugate Gradient inverse Method |
| DF | Dermic-Fat interface |
| DPL | Dual Phase Lag |
| ED | Epidermis-Dermic interface |
| FSPL | Fractional Single-Phase Lag |
| SPL | Single Phase Lag |
Appendix A
Appendix B
References
- Tang, D.W.; Araki, N. Non-fourier heat conduction behavior in finite mediums under pulse surface heating. Mater. Sci. Eng. A 2000, 292, 173–178. [Google Scholar] [CrossRef]
- Hsu, P.T.; Chu, Y.H. An inverse non-fourier heat conduction problem approach for estimating the boundary condition in electronic device. Appl. Math. Model. 2004, 28, 639–652. [Google Scholar] [CrossRef]
- Yang, C.Y. Direct and inverse solutions of the hyperbolic heat conduction problems. J. Thermophys. Heat Transf. 2005, 19, 217–225. [Google Scholar] [CrossRef]
- Hsu, P.T. Estimating the boundary condition in a 3D inverse hyperbolic heat conduction problem. Appl. Math. Comput. 2006, 177, 453–464. [Google Scholar] [CrossRef]
- Krishna, M.V.; Chamkha, A.J. Hall and ion slip effects on magnetohydrodynamic convective rotating flow of Jeffreys fluid over an impulsively moving vertical plate embedded in a saturated porous medium with ramped wall temperature. Numer. Methods Partial. Differ. Equ. 2021, 37, 2150–2177. [Google Scholar] [CrossRef]
- Hussain, S.; Ismael, M.A.; Chamkha, A.J. Impinging jet into an open trapezoidal cavity partially filled with a porous layer. Int. Commun. Heat Mass Transf. 2020, 118, 104870. [Google Scholar] [CrossRef]
- Kumar, B.; Seth, G.; Singh, M.; Chamkha, A. Carbon nanotubes (CNTs)-based flow between two spinning discs with porous medium, Cattaneo–Christov (non-fourier) model and convective thermal condition. J. Therm. Anal. Calorim. 2021, 146, 241–252. [Google Scholar] [CrossRef]
- Kumar, K.G.; Reddy, M.G.; Sudharani, M.; Shehzad, S.; Chamkha, A.J. Cattaneo–Christov heat diffusion phenomenon in Reiner–Philippoff fluid through a transverse magnetic field. Phys. A 2020, 541, 123330. [Google Scholar] [CrossRef]
- Kotha, G.; Kolipaula, V.R.; Rao, M.V.S.; Penki, S.; Chamkha, A.J. Internal heat generation on bioconvection of an MHD nanofluid flow due to gyrotactic microorganisms. Eur. Phys. J. Plus 2020, 135, 1–19. [Google Scholar] [CrossRef]
- Chamkha, A.J.; Nabwey, H.A.; Abdelrahman, Z.; Rashad, A. Mixed bioconvective flow over a wedge in porous media drenched with a nanofluid. J. Nanofluids 2019, 8, 1692–1703. [Google Scholar] [CrossRef]
- Sheremet, M.A.; Pop, I. Thermo-bioconvection in a square porous cavity filled by oxytactic microorganisms. Transp. Porous Media 2014, 103, 191–205. [Google Scholar] [CrossRef]
- Liu, K.C.; Lin, C.T. Solution of an inverse heat conduction problem in a bi-layered spherical tissue. Numer. Heat Transf. A 2010, 58, 802–818. [Google Scholar] [CrossRef]
- Azimi, A.; Ahmadikia, A.; Masouleh, K.B. Base temperature estimation of non-fourier fin with different profiles by the use of inverse analysis. J. Appl. Math. Modeling 2009, 33, 2907–2918. [Google Scholar]
- Das, R.; Mishra, S.C.; Kumar, T.P.; Uppaluri, R. An inverse analysis for parameter estimation applied to a non-fourier conduction–radiation problem. Heat Transf. Eng. 2011, 32, 455–466. [Google Scholar] [CrossRef]
- Ghazizadeh, H.R.; Azimi, A.; Maerefat, M. An inverse problem to estimate relaxation parameter and order of fractionality in fractional single-phase-lag heat equation. Int. J. Heat Mass Transf. 2012, 55, 2095–2101. [Google Scholar] [CrossRef]
- Azimi, A.; Bamdad, K.; Ahmadikia, H. Inverse hyperbolic heat conduction in fins with arbitrary profiles. Numer. Heat Transf. A 2012, 61, 220–240. [Google Scholar] [CrossRef]
- Wu, T.S.; Lee, H.L.; Chang, W.J.; Yang, Y.C. An inverse hyperbolic heat conduction problem in estimating pulse heat flux with a dual-phase-lag model. Int. Commun. Heat Mass Transf. 2015, 60, 1–8. [Google Scholar] [CrossRef]
- Mozafarifard, M.; Azimi, A.; Mehrzad, S. Numerical simulation of dual-phase-lag model and inverse fractional single-phase-lag problem for the non-fourier heat conduction in a straight fin. J. Therm. Sci. 2020, 29, 632–646. [Google Scholar] [CrossRef]
- Ali, M.; Aziz, S.; Malik, S.A. Inverse source problems for a space–time fractional differential equation. Inverse Probl. Sci. Eng. 2020, 28, 47–68. [Google Scholar] [CrossRef]
- Cheng, X.; Yuan, L.; Liang, K. Inverse source problem for a distributed-order time fractional diffusion equation. J. Inverse Ill-Posed Probl. 2020, 28, 17–32. [Google Scholar] [CrossRef] [Green Version]
- Sun, C.; Liu, J. An inverse source problem for distributed order time-fractional diffusion equation. Inverse Probl. 2020, 36, 055008. [Google Scholar] [CrossRef]
- Tuan, N.H.; Zhou, Y.; Can, N.H. Identifying inverse source for fractional diffusion equation with Riemann–Liouville derivative. Comput. Appl. Math. 2020, 39, 75. [Google Scholar] [CrossRef]
- Goudarzi, P.; Azimi, A. Numerical simulation of fractional non-fourier heat conduction in skin tissue. J. Therm. Biol. 2019, 84, 274–284. [Google Scholar] [CrossRef]
- Ozisik, M.N.; Orlande, H.; Kassab, A. Inverse heat transfer: Fundamentals and Applications. Appl. Mech. Rev. 2002, 55, B18–B19. [Google Scholar] [CrossRef]
- Liu, K.C.; Cheng, P.J.; Wang, Y.N. Analysis of non-fourier thermal behavior for multi-layer skin model. Therm. Sci. 2011, 15, 61–67. [Google Scholar] [CrossRef]
- Zhou, J.; Chen, J.; Zhang, Y. Dual-phase lag effects on thermal damage to biological tissues caused by laser irradiation. Comput. Biol. Med. 2009, 39, 286–293. [Google Scholar] [CrossRef] [PubMed]
- Ghazizadeh, H.; Maerefat, M.; Azimi, A. Explicit and implicit finite difference schemes for fractional Cattaneo equation. J. Comput. Phys. 2010, 229, 7042–7057. [Google Scholar] [CrossRef]


| CGIM | DPL | |||
|---|---|---|---|---|
| α | τ | τT | τq | |
| Sample A | 0.985707860045904 | 9.88794652835751 | 0.005 | 10 |
| Sample B | 0.982593197834287 | 9.82287275801926 | 0.05 | 10 |
| Sample C | 0.958513945252591 | 9.87599109128811 | 0.1 | 10 |
| Sample D | 0.933915800219504 | 0.57923029381419 | 0.05 | 1 |
| Sample E | 0.982593197834287 | 9.82287275801926 | 0.05 | 10 |
| Sample F | 0.989762788979733 | 14.8313003950098 | 0.05 | 15 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Goudarzi, P.; Abidi, A.; Mehryan, S.A.M.; Ghalambaz, M.; Sheremet, M.A. Estimating Relaxation Time and Fractionality Order Parameters in Fractional Non-Fourier Heat Conduction Using Conjugate Gradient Inverse Approach in Single and Three-Layer Skin Tissues. Processes 2021, 9, 1877. https://doi.org/10.3390/pr9111877
Goudarzi P, Abidi A, Mehryan SAM, Ghalambaz M, Sheremet MA. Estimating Relaxation Time and Fractionality Order Parameters in Fractional Non-Fourier Heat Conduction Using Conjugate Gradient Inverse Approach in Single and Three-Layer Skin Tissues. Processes. 2021; 9(11):1877. https://doi.org/10.3390/pr9111877
Chicago/Turabian StyleGoudarzi, Piran, Awatef Abidi, Seyed Abdollah Mansouri Mehryan, Mohammad Ghalambaz, and Mikhail A. Sheremet. 2021. "Estimating Relaxation Time and Fractionality Order Parameters in Fractional Non-Fourier Heat Conduction Using Conjugate Gradient Inverse Approach in Single and Three-Layer Skin Tissues" Processes 9, no. 11: 1877. https://doi.org/10.3390/pr9111877
APA StyleGoudarzi, P., Abidi, A., Mehryan, S. A. M., Ghalambaz, M., & Sheremet, M. A. (2021). Estimating Relaxation Time and Fractionality Order Parameters in Fractional Non-Fourier Heat Conduction Using Conjugate Gradient Inverse Approach in Single and Three-Layer Skin Tissues. Processes, 9(11), 1877. https://doi.org/10.3390/pr9111877







