Global Stability Analysis of a Bioreactor Model for Phenol and Cresol Mixture Degradation
Abstract
1. Introduction
2. Model Description
3. Existence of Equilibrium Points
4. Local Stability of the Equilibrium Points
- (i)
- If then the equilibrium point (with ) is locally asymptotically unstable (a saddle).
- (ii)
- If then is locally asymptotically stable (a stable node).
- (iii)
- At the equilibrium is neither stable, nor unstable: possesses a zero eigenvalue, , thus is a bifurcation parameter value.
- (iv)
- The equilibrium point , (see (13)), is locally asymptotically unstable.
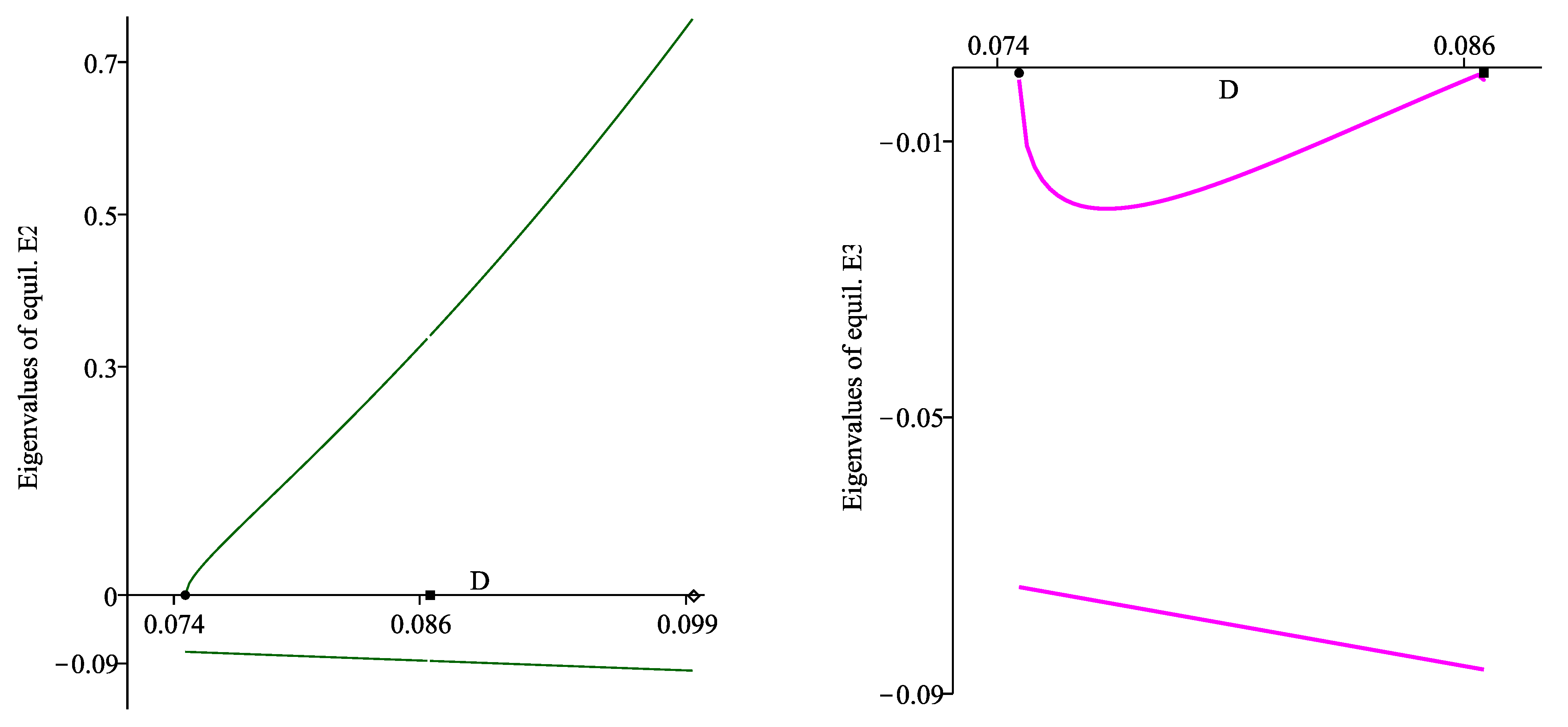
- The equilibrium , defined for , is locally asymptotically unstable (a saddle).
- The equilibrium , defined for , is locally asymptotically stable (a stable node).
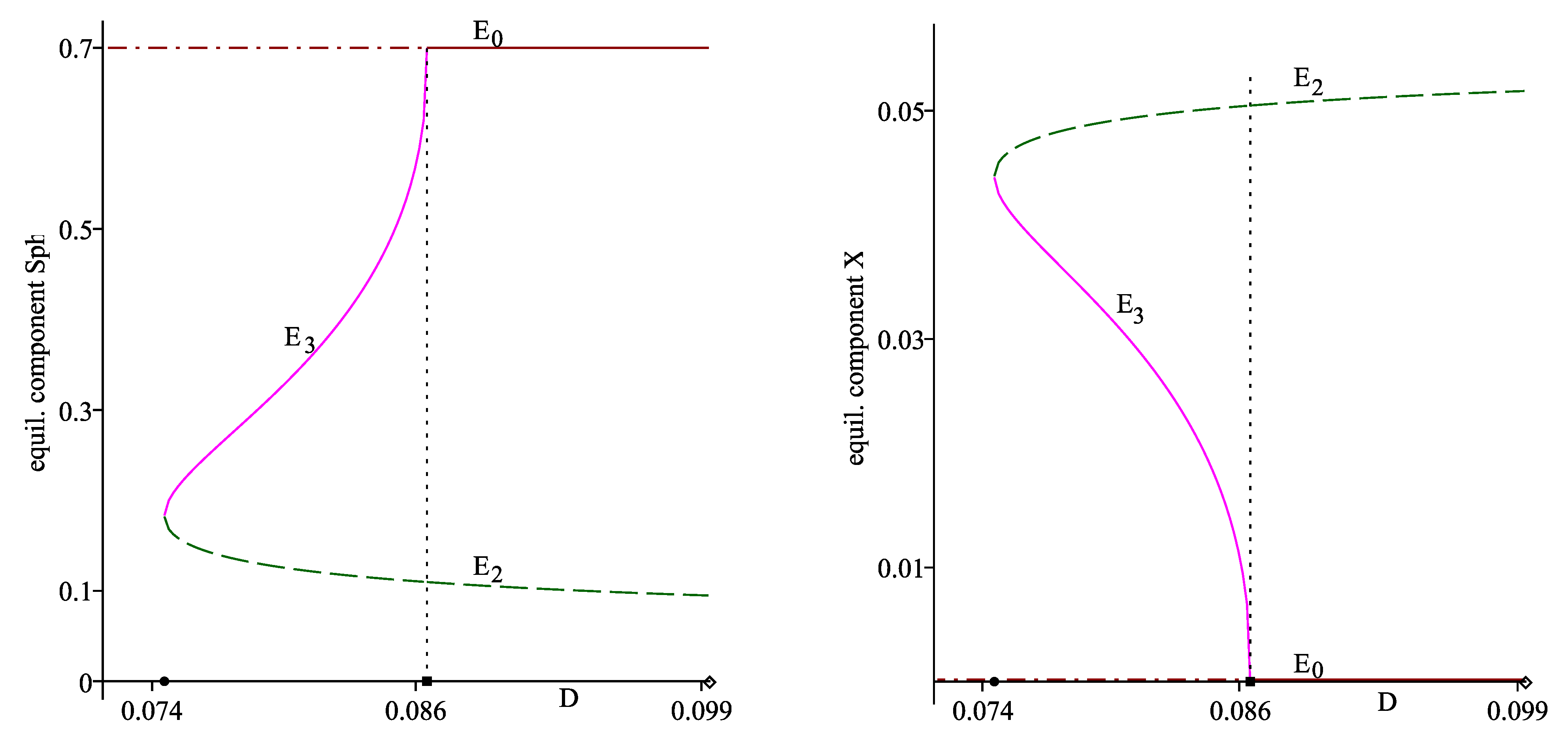
- At , the two interior equilibrium points, and , are ’born’, thus is a bifurcation value of the parameter D. At the steady states and undergo a saddle-node bifurcation.
- At the equilibrium points and coalesce and exchange stability for . Thus, at the steady states and undergo a transcritical bifurcation.
5. Global Stabilizability of the Model Dynamics
- (i)
- If then all model solutions tend to the equilibrium point .
- (ii)
- If then , , for all .
- (iii)
- All solutions are uniformly bounded for all .
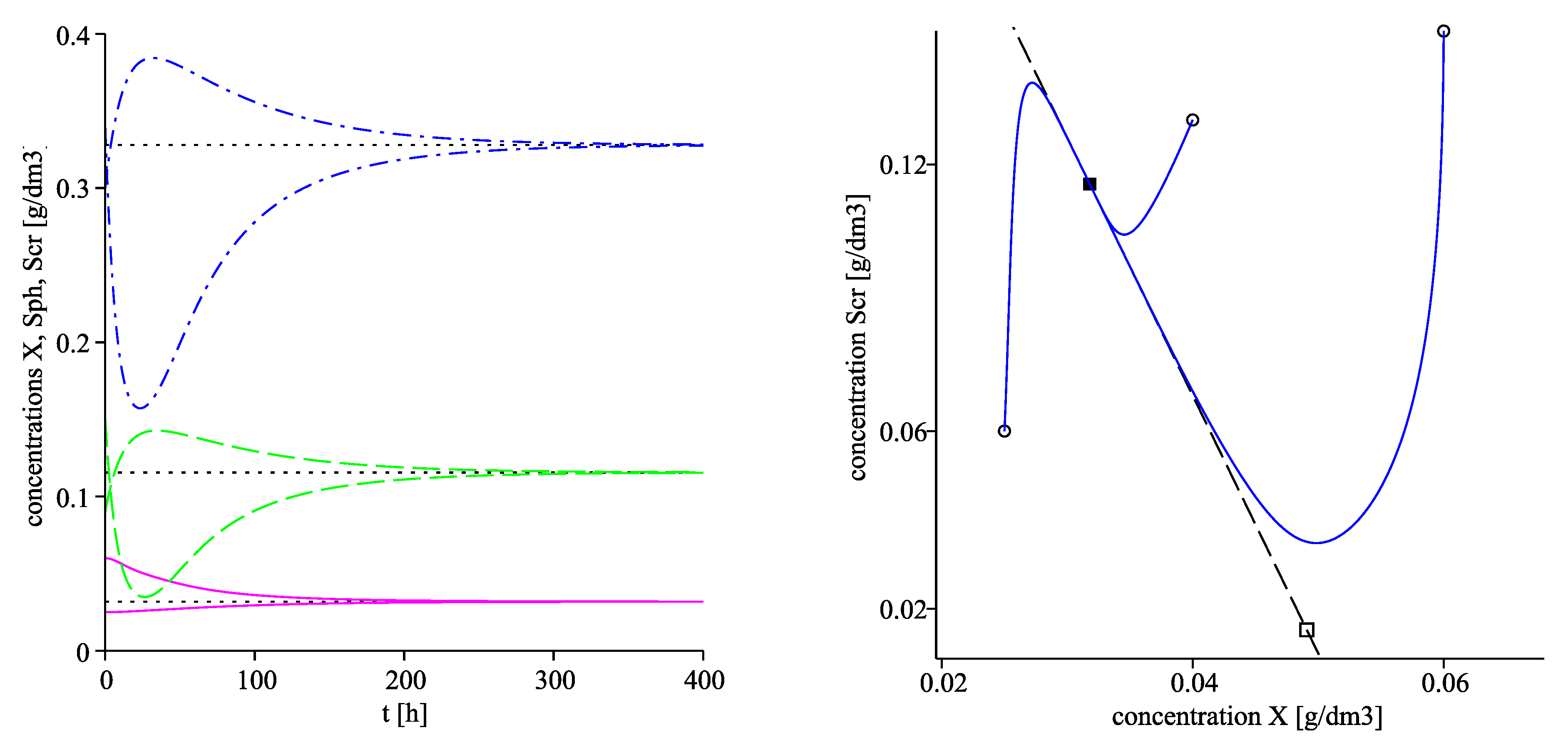
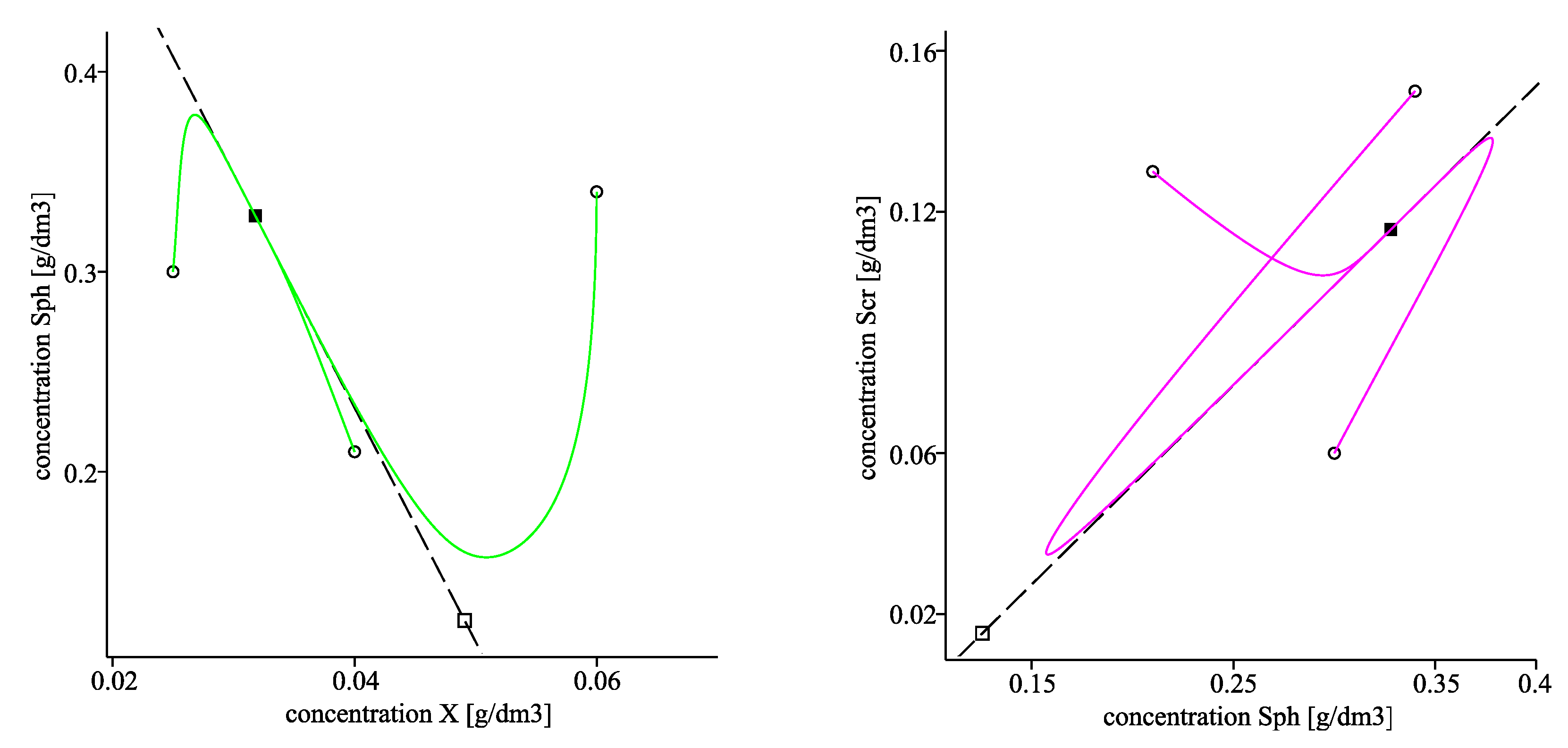
6. Dynamic Behavior of the Model Solutions: Numerical Simulation
7. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Singh, P.; Jain, R.; Srivastava, N.; Borthakur, A.; Pal, D.B.; Singh, R.; Madhav, S.; Srivastava, P.; Tiwary, D.; Mishra, P.K. Current and emerging trends in bioremediation of petrochemical waste: A review. Crit. Rev. Environ. Sci. Technol. 2017, 47, 155–201. [Google Scholar] [CrossRef]
- Seo, J.-S.; Keum, Y.-S.; Li, Q.X. Bacterial degradation of aromatic compounds. Int. J. Environ. Res. Public Health 2009, 6, 278–309. [Google Scholar] [CrossRef] [PubMed]
- Sharma, N.K.; Philip, L.; Bhallamudi, S.M. Aerobic degradation of phenolics and aromatic hydrocarbons in presence of cyanide. Bioresour. Technol. 2012, 121, 263–273. [Google Scholar] [CrossRef] [PubMed]
- Tomei, M.C.; Annesini, M.C. Biodegradation of phenolic mixtures in a sequencing batch reactor: A kinetic study. Environ. Sci. Pollut. Res. 2008, 15, 188–195. [Google Scholar] [CrossRef] [PubMed]
- Yemendzhiev, H.; Zlateva, P.; Alexieva, Z. Comparison of the biodegradation capacity of two fungal strains toward a mixture of phenol and cresol by mathematical modeling. Biotechnol. Biotechnol. Equip. 2012, 26, 3278–3281. [Google Scholar] [CrossRef][Green Version]
- Kietkwanboot, A.; Chaiprapat, S.; Müller, R.; Suttinun, O. Biodegradation of phenolic compounds present in palm oil mill effluent as single and mixed substrates by Trameteshirsuta AK04. J. Environ. Sci. Heal. Part A Toxic/Hazard. Subst. Environ. Eng. 2020, 55, 989–1002. [Google Scholar] [CrossRef]
- Liu, J.; Jia, X.; Wen, J.; Zhou, Z. Substrate interactions and kinetics study of phenolic compounds biodegradation by Pseudomonas sp. cbp1-3. Biochem. Eng. J. 2012, 67, 156–166. [Google Scholar] [CrossRef]
- Kumar, S.; Arya, D.; Malhotra, A.; Kumar, S.; Kumar, B. Biodegradation of dual phenolic substrates in simulated wastewater by Gliomastixindicus MTCC 3869. J. Environ. Chem. Eng. 2013, 1, 865–874. [Google Scholar] [CrossRef]
- Angelucci, D.M.; Annesini, M.C.; Tomei, M.C. Modelling of biodegradation kinetics for binary mixtures of substituted phenols in sequential bioreactors. Chem. Eng. Trans. 2013, 32, 1081–1086. [Google Scholar] [CrossRef]
- Lepik, R.; Tenno, T. Biodegradability of phenol, resorcinol and 5-methylresorcinol as single and mixed substrates by activated sludge. Oil Shale 2011, 28, 425–446. [Google Scholar] [CrossRef]
- Yoon, H.; Klinzing, G.; Blanch, H.W. Competition for mixed substrate by microbial populations. Biotechnol. Bioeng. 1977, 19, 1193–1210. [Google Scholar] [CrossRef] [PubMed]
- Reardon, K.F.; Mosteller, D.C.; Rogers, J.D.B. Biodegradation kinetics of benzene, toluene, and phenol as single and mixed substrates for Pseudomonas Putida F1. Biotechnol. Bioeng. 2000, 69, 385–400. [Google Scholar] [CrossRef]
- Reardon, K.F.; Mosteller, D.C.; Rogers, J.B.; DuTeau, N.M.; Kim, K.-H. Biodegradation kinetics of aromatic hydrocarbon mixtures by pure and mixed bacterial cultures. Environ. Health 2002, 110, 1005–1011. [Google Scholar] [CrossRef] [PubMed]
- Abuhamed, T.; Bayraktar, E.; Mehmetoǧlu, T.; Mehmetoǧlu, Ü. Kinetics model for growth of Pseudomonas Putida F1 Benzene, Toluene Phenol Biodegrad. Process Biochem. 2004, 39, 983–988. [Google Scholar] [CrossRef]
- Datta, A.; Philip, L.; Bhallamudi, S.M. Modeling the biodegradation kinetics of aromatic and aliphatic volatile pollutant mixture in liquid phase. Chem. Eng. J. 2014, 241, 288–300. [Google Scholar] [CrossRef]
- Hazrati, H.; Shayegan, J.; Seyedi, S.M. Biodegradation kinetics and interactions of styrene and ethylbenzene as single and dual substrates for a mixed bacterial culture. J. Environ. Health Sci. Eng. 2015, 13, 72. [Google Scholar] [CrossRef] [PubMed]
- Yemendzhiev, H.; Gerginova, M.; Zlateva, P.; Stoilova, I.; Krastanov, A.; Alexieva, Z. Phenol and cresol mixture degradation by Aspergillus awamori strain: Biochemical and kinetic substrate interactions. Proc. ECOpole 2008, 2, 153–159. Available online: https://ecesociety.com/proceedings-of-ecopole-peco (accessed on 7 January 2021).
- Dimitrova, N.; Zlateva, P. Stability Analysis of a Model for Phenol and Cresol Mixture Degradation. In Proceedings of the IOP Conference Series: Earth and Environmental Science (EES), Xiamen, China, 7–9 June 2019; Volume 356, p. 012209. [Google Scholar] [CrossRef]
- Wiggins, S. Introduction to Applied Nonlinear Dynamical Systems and Chaos; Springer: New York, NY, USA, 1990. [Google Scholar] [CrossRef]
- Smith, H.L.; Waltman, P. The Theory of the Chemostat: Dynamics of Microbial Competition; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar] [CrossRef]
- Thieme, H.R. Convergence results and a Poincaré–Bendixon trichotomy for asymptotically autonomous differential equations. J. Math. Biol. 1992, 30, 755–763. [Google Scholar] [CrossRef]
- Harmand, J.; Lobry, C.; Rapaport, A.; Sari, T. The Chemostat: Mathematical Theory of Microorganism Cultures; Volume 1 of Chemical Engineering Series; Wiley: Hoboken, NJ, USA, 2017. [Google Scholar] [CrossRef]
- Hsu, S.B. Limiting behavior of competing species. SIAM J. Appl. Math. 1978, 34, 760–763. [Google Scholar] [CrossRef]
- Wolkowicz, G.S.K.; Lu, Z. Global dynamics of a mathematical model of competition in the chemostat: General response function and differential death rates. SIAM J. Appl. Math. 1992, 52, 222–233. [Google Scholar] [CrossRef]
- Dimitrova, N.S.; Krastanov, M.I. Model-based optimization of biogas production in an anaerobic biodegradation process. Comput. Math. Appl. 2014, 68, 986–993. [Google Scholar] [CrossRef]
- Gopalsamy, K. Stability and Oscillations in Delay Differential Equations of Population Dynamics; Kluwer Academic Publishers: Dordrecht, The Netherlands, 1992. [Google Scholar] [CrossRef]
- Grognard, F.; Bernard, O. Stability analysis of a wastewater treatment plant with saturated control. Water Sci. Technol. 2006, 53, 149–157. [Google Scholar] [CrossRef] [PubMed][Green Version]



| Definitions | Values | |
|---|---|---|
| X | biomass concentration [g/dm3] | - |
| phenol concentration [g/dm3] | - | |
| p-cresol concentration [g/dm3] | - | |
| D | dilution rate | - |
| influent phenol concentration [g/dm3] | 0.7 | |
| influent p-cresol concentration [g/dm3] | 0.3 | |
| metabolic coefficient | 11.7 | |
| metabolic coefficient | 5.8 | |
| inhibition constant for cell growth on phenol [g/dm3] | 0.61 | |
| inhibition constant for cell growth on cresol [g/dm3] | 0.45 | |
| interaction coefficient indicating the degree to which phenol affects the p-cresol biodegradation | 0.3 | |
| interaction coefficient indicating the degree to which p-cresol affects the phenol biodegradation | 8.6 | |
| maximum specific growth rate on phenol as a single substrate | 0.23 | |
| maximum specific growth rate on p-cresol as a single substrate | 0.17 | |
| saturation constant for cell growth on phenol [g/dm3] | 0.11 | |
| saturation constant for cell growth on p-cresol [g/dm3] | 0.35 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dimitrova, N.; Zlateva, P. Global Stability Analysis of a Bioreactor Model for Phenol and Cresol Mixture Degradation. Processes 2021, 9, 124. https://doi.org/10.3390/pr9010124
Dimitrova N, Zlateva P. Global Stability Analysis of a Bioreactor Model for Phenol and Cresol Mixture Degradation. Processes. 2021; 9(1):124. https://doi.org/10.3390/pr9010124
Chicago/Turabian StyleDimitrova, Neli, and Plamena Zlateva. 2021. "Global Stability Analysis of a Bioreactor Model for Phenol and Cresol Mixture Degradation" Processes 9, no. 1: 124. https://doi.org/10.3390/pr9010124
APA StyleDimitrova, N., & Zlateva, P. (2021). Global Stability Analysis of a Bioreactor Model for Phenol and Cresol Mixture Degradation. Processes, 9(1), 124. https://doi.org/10.3390/pr9010124






