The Minimum Safe Thickness and Catastrophe Process for Water Inrush of a Karst Tunnel Face with Multi Fractures
Abstract
:1. Introduction
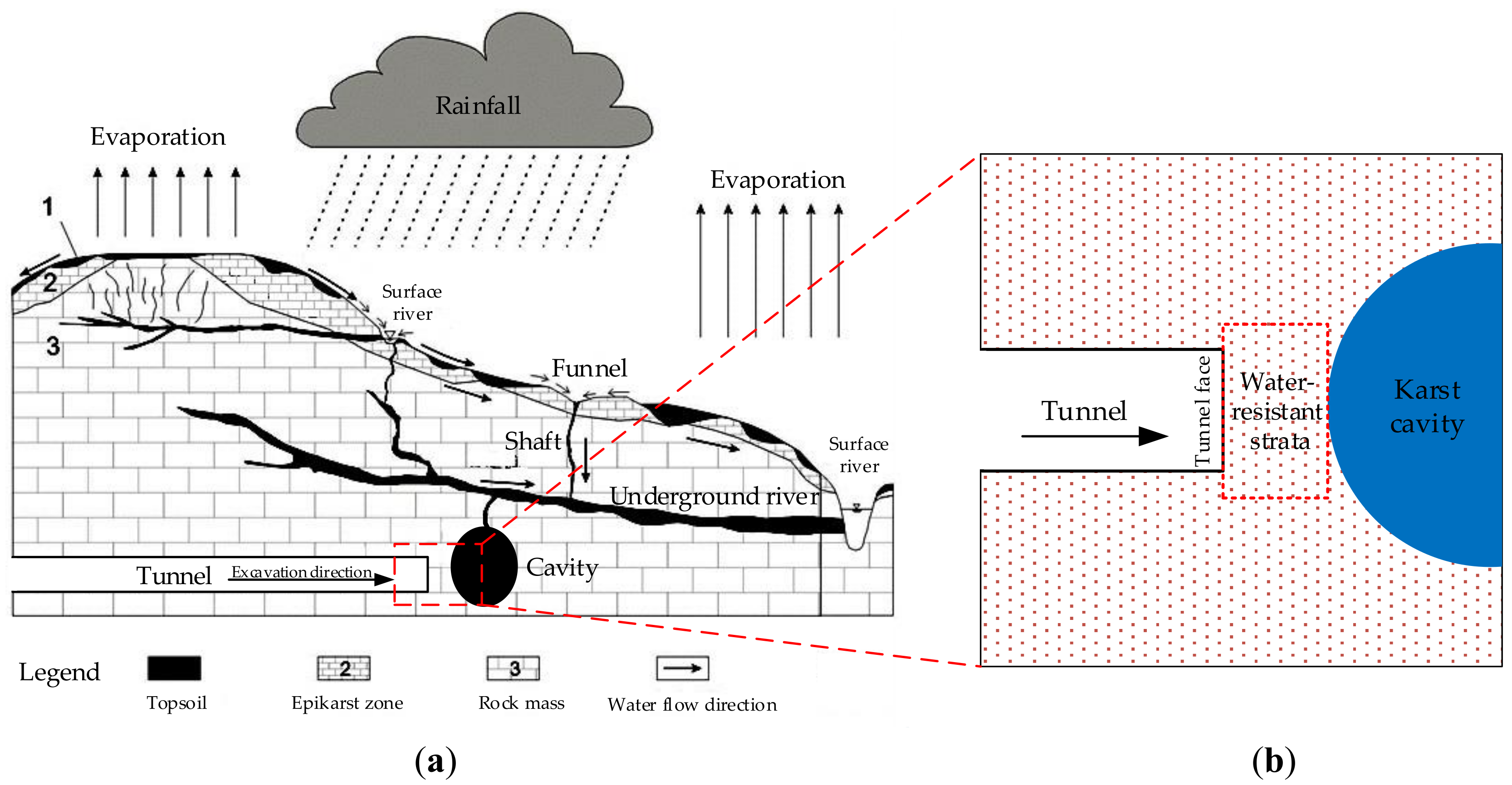
2. Structure of Water-Resistant Strata and Its Hydraulic Failure Mode
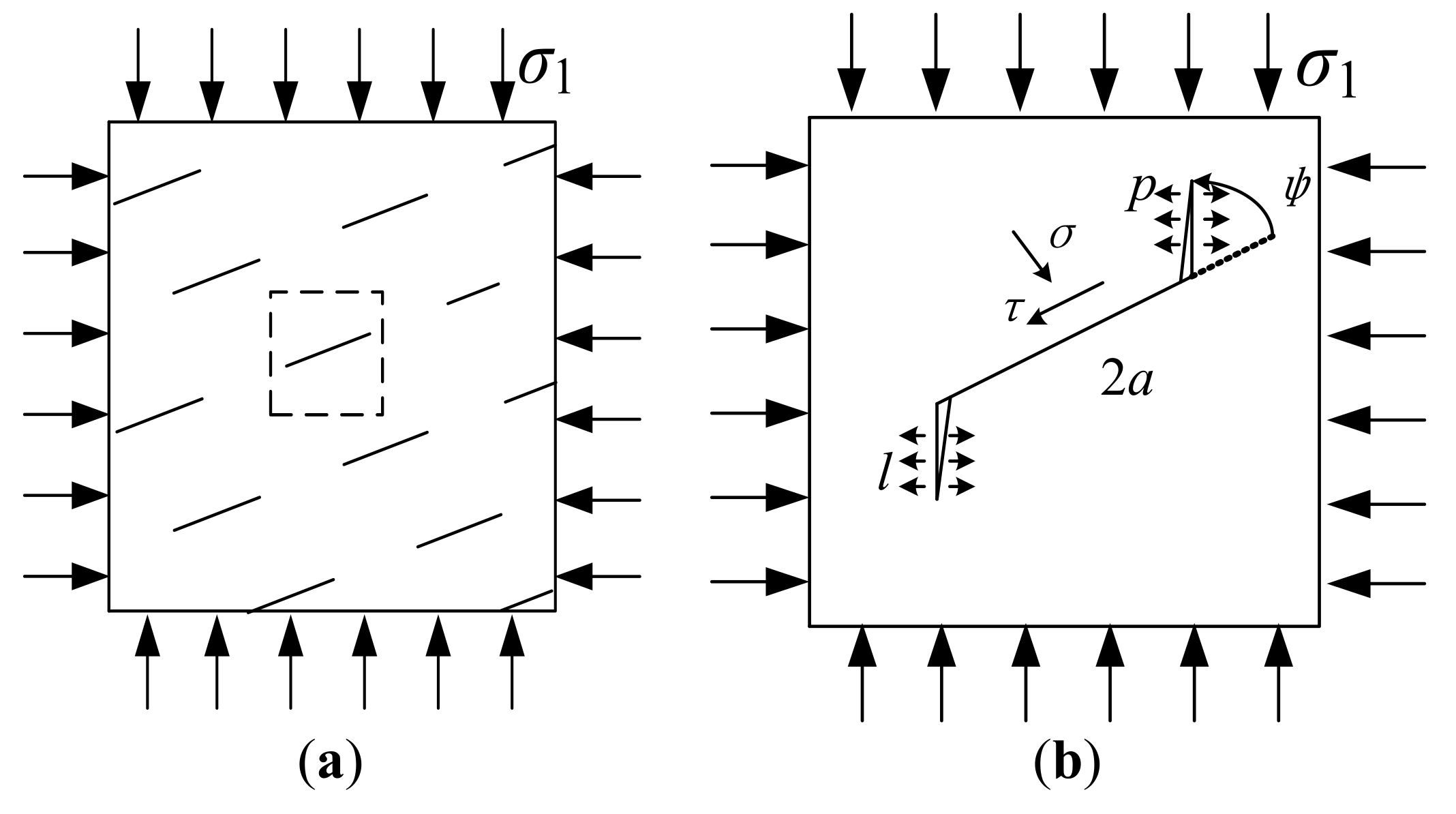
2.1. Failure Mechanics Mode of Fracture with High Water Pressure
2.2. Critical Water Pressure of Hydraulic Fracturing for Rock Mass with Multi Fractures
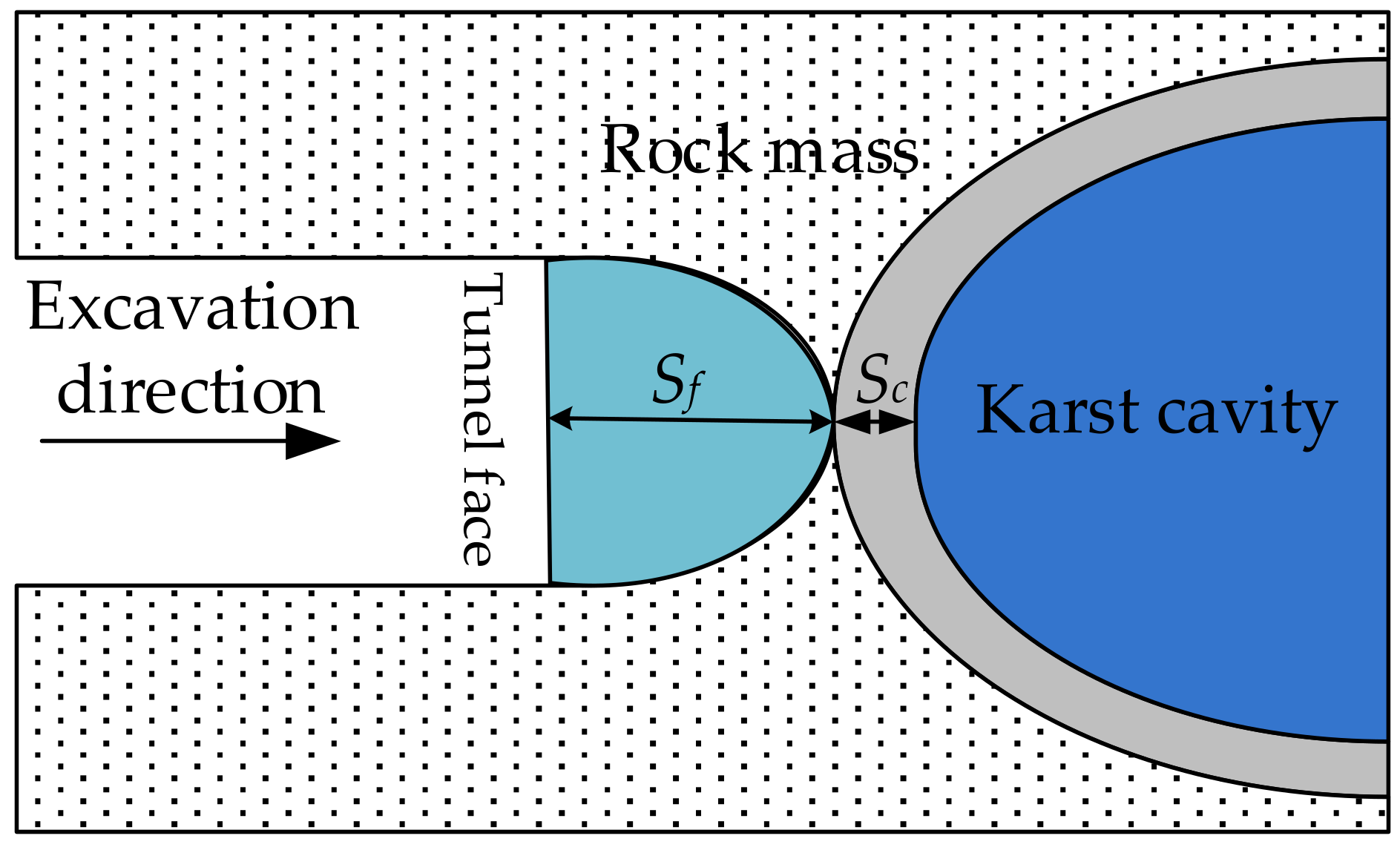
3. Safety Thickness of Water-Resistant Strata with Multi Fractures
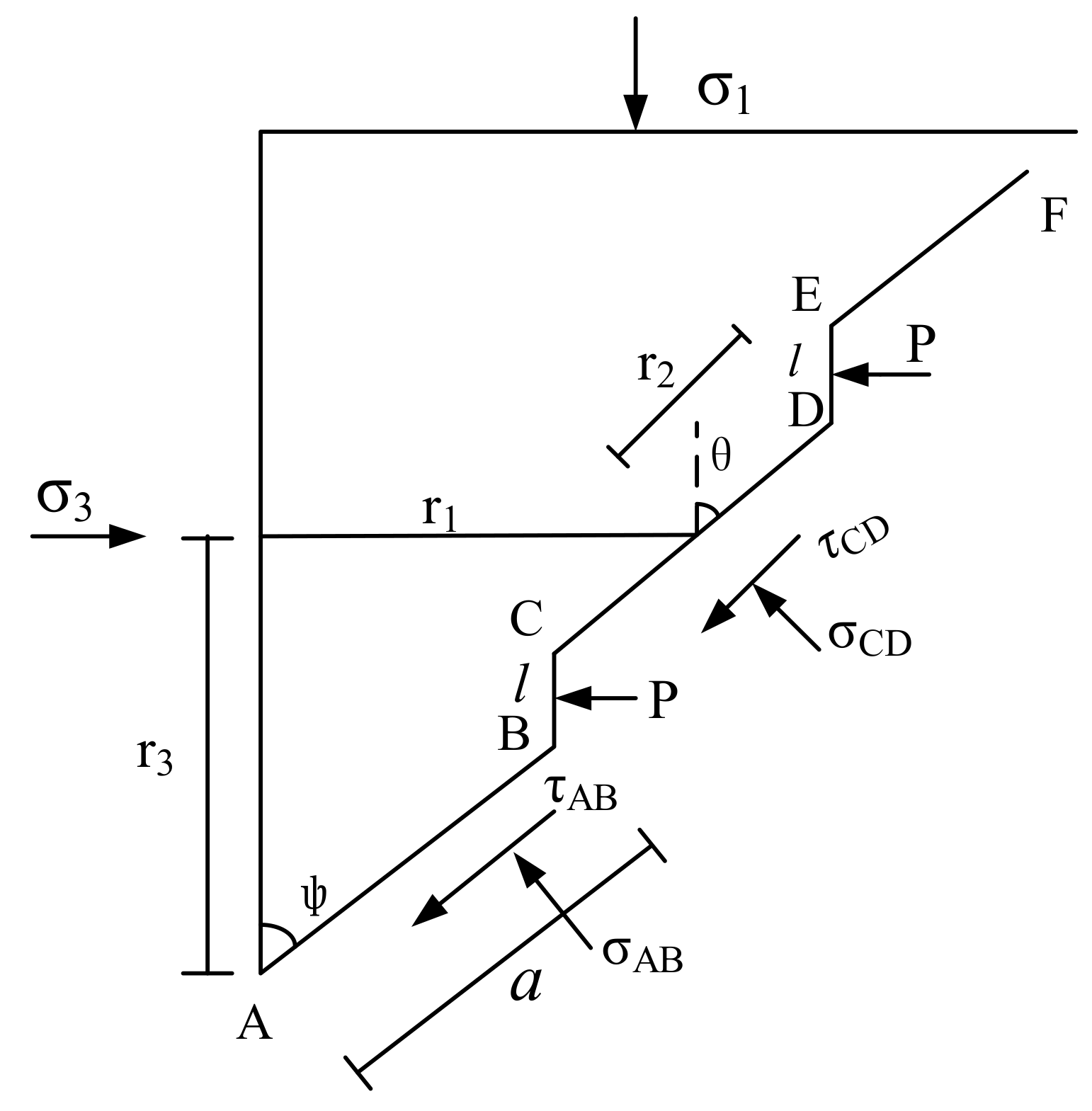
3.1. Two Band Theory
- (1)
- Karst initial fissure zone—Under the coupling effect of stress, chemistry, hydraulic scouring and seasonal variation of karst water pressure, the initial fracture zone caused by trenchless factors around the cavity filled water is formed. The strength of rock mass in this zone is relatively low, and the structure of rock mass is different from rock away from the cavity. At the moment, there is no mature theory to determine the range of the initial fracture zone due to the complex of formation process of the initial fracture zone around the high-pressure cavity. Now most scholars rely on field measurement or replace it with the plastic zone of the rock mass around the cavity [8,27]. In practical engineering activities, engineers often use Ground Penetrating Radar and other geophysical methods to detect the thickness of initial fracture zone and verification by drilling. According to practical engineering experience, it is generally believed that the thickness of initial fracture zone is 1~2 m [24].
- (2)
- Karst hydraulic fracturing zone—By substituting the far-field stress σ3 = λσ1 = λγH into the critical water pressure calculate formula (Equation (6)), we can know that:
3.2. Minimum Safety Thickness of Water-Resistant Strata with Multi Fractures
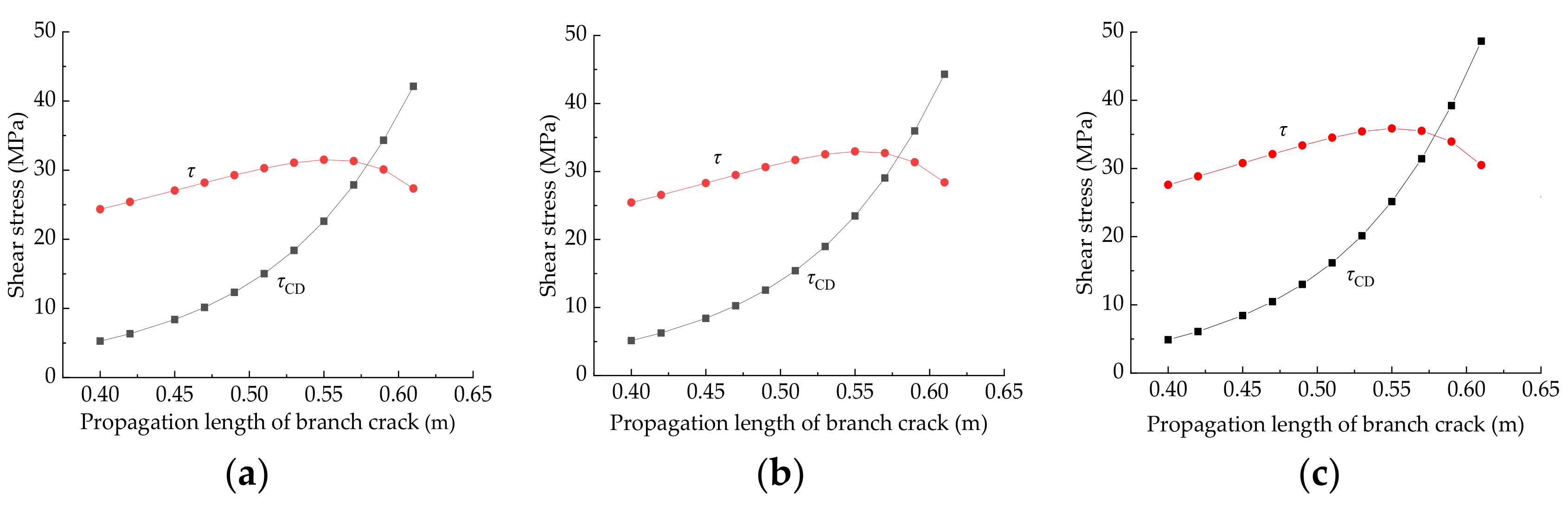
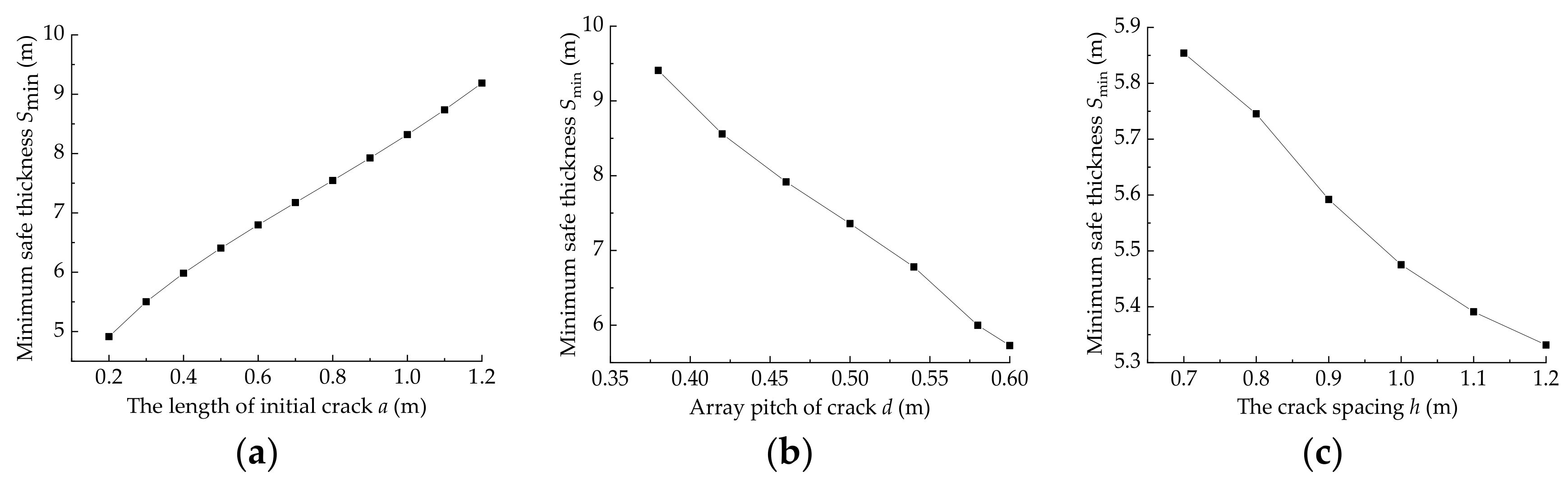
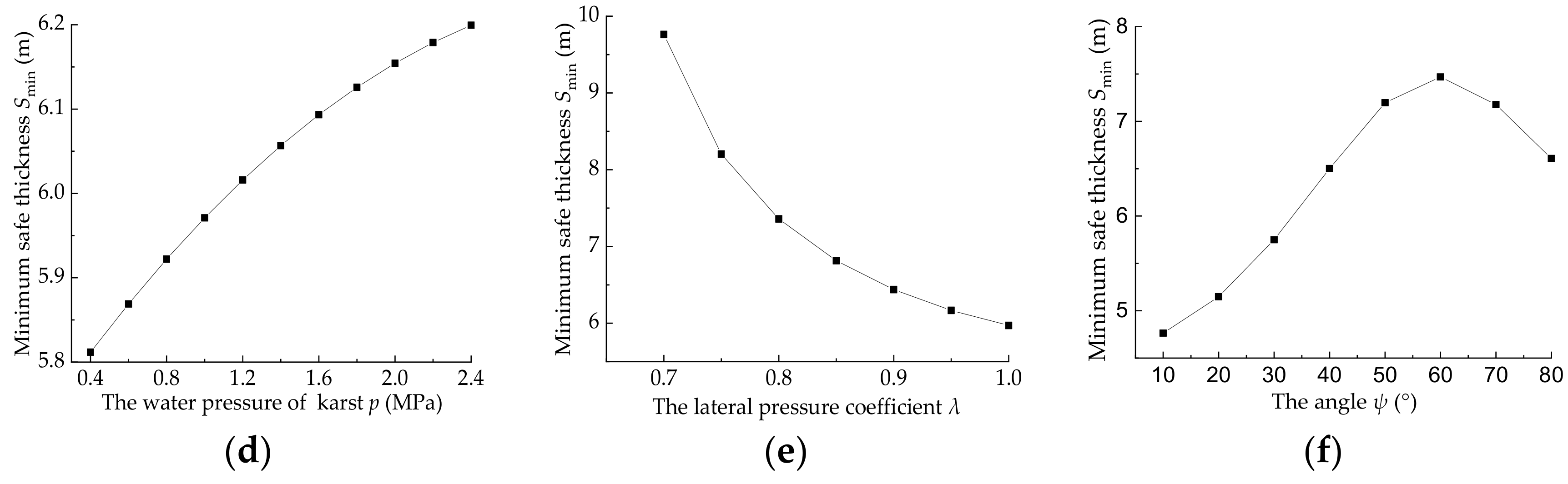
3.3. Parametric Analysis of the Minimum Safe Thickness
4. Numerical Analysis of Water Inrush Evolutionary Process
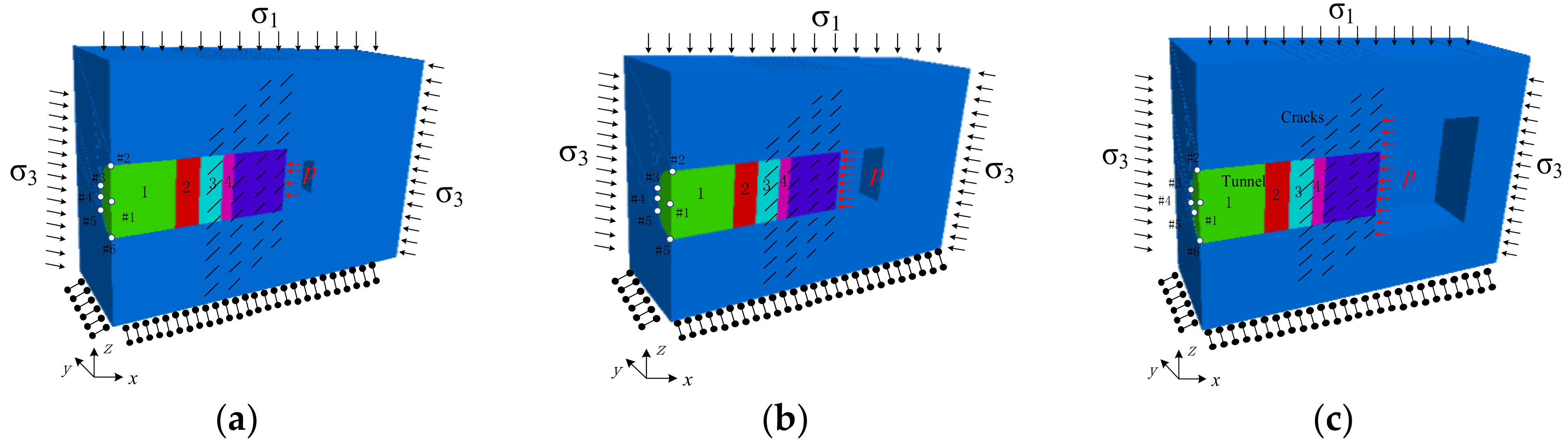
4.1. Numerical Calculation Model
4.2. Numerical Results and Discussions
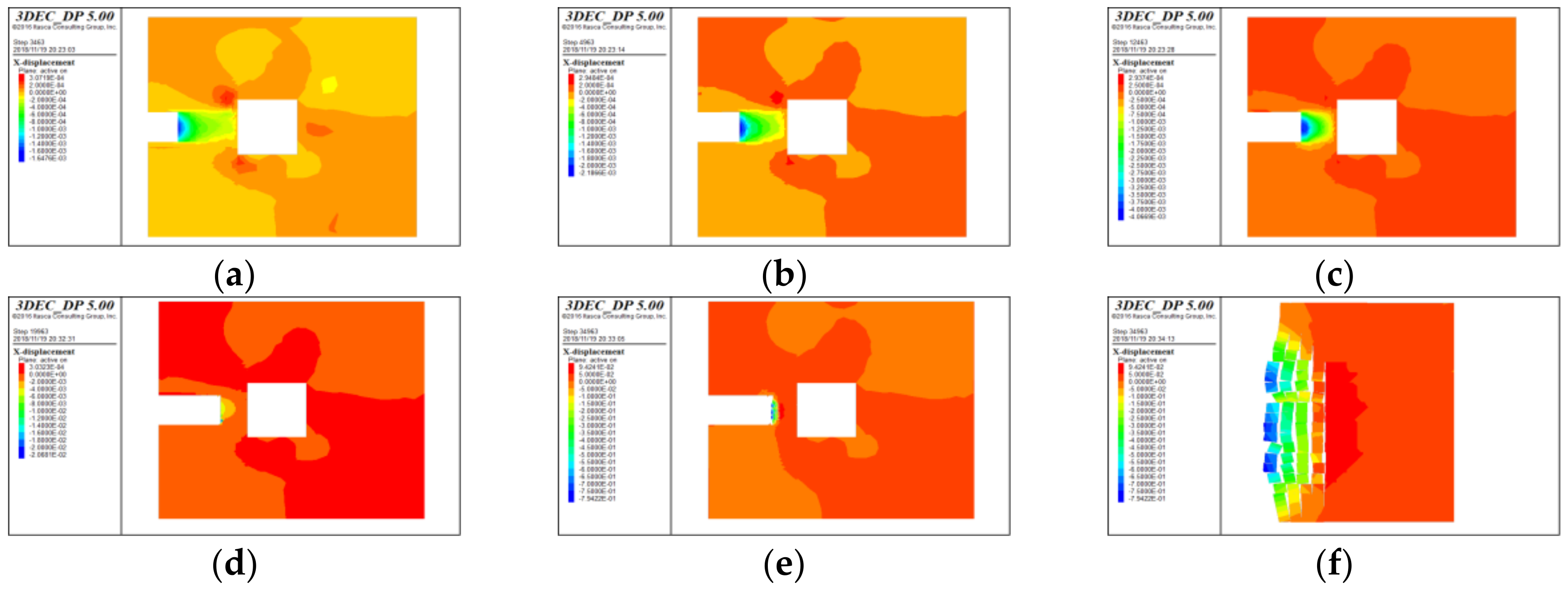
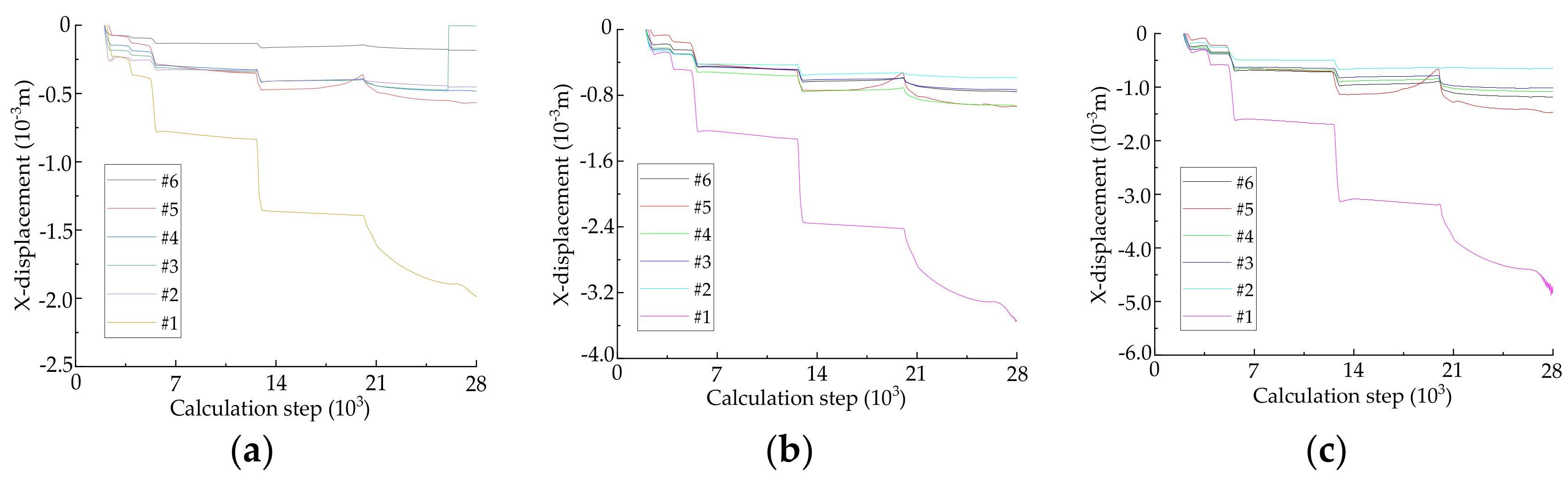
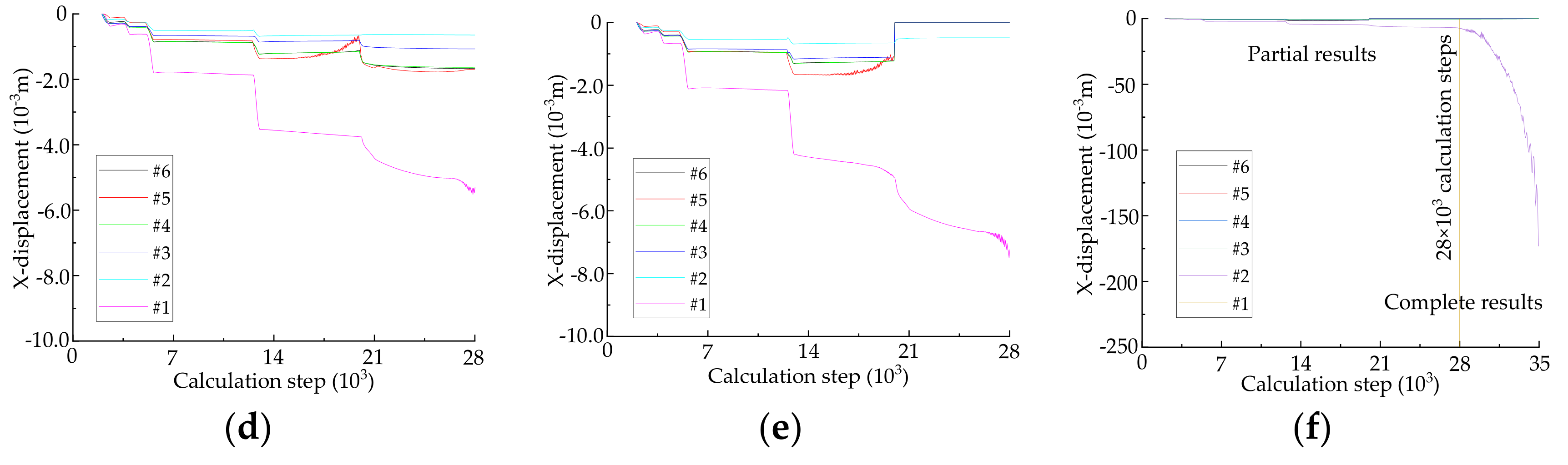
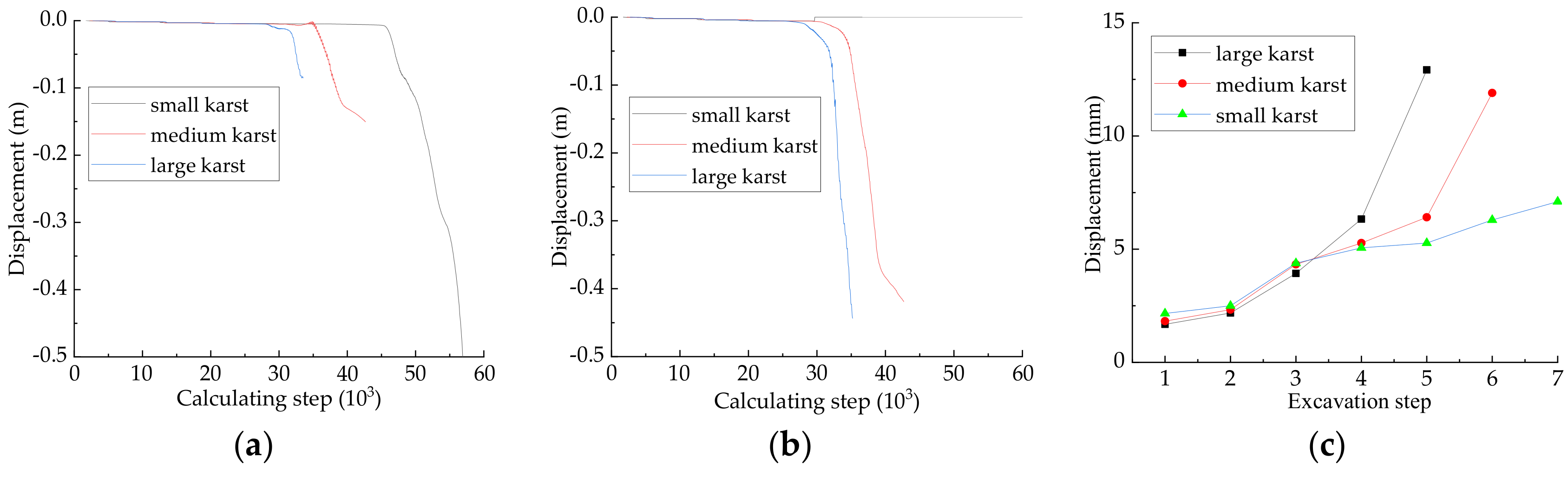
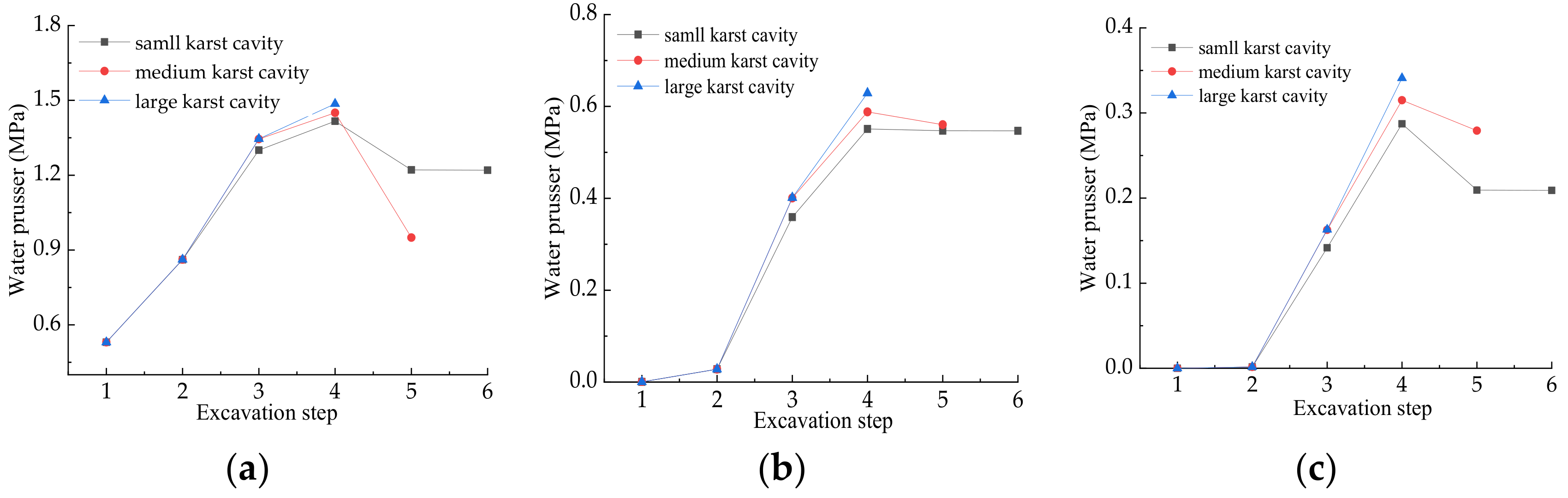
4.2.1. Analysis of Catastrophic Process of Water Inrush
4.2.2. Influence of Karst Cavity Scale on Water Inrush
5. Conclusions
- (1)
- The rock mass with non-persistent joints is a common type of tunnel surrounding rock mass. The water inrush model for the fractured rock mass is usually hydraulic fracturing, and the model of hydraulic fracturing for rock mass with multi fractures is compression shear failure under karst water pressure and geo-stress. With the increase of the crack propagated length, the shear stress of rock bridge increases. Based on the Mohr-Coulomb strength criterion and the shear stress condition of rock bridge, the formula of critical water pressure for rock mass with multi fractures when it occurs the tension-shear combined fracturing failure is deduced.
- (2)
- The water-resistant strata ahead of karst tunnel face can be divided into karst initial fissure zone and karst hydraulic fracturing zone. Based on the two-band theory and the critical water pressure, the formula to determine the minimum safe thickness of water-resistant strata with multi fractures is established. The minimum safety thickness increases with the increase of initial crack length and karst water pressure, and decreases with the increase of crack distance (d and h), lateral pressure coefficient. As the angle between the initial crack and the maximum principal stress increases, the minimum safety thickness increases firstly and then decreases.
- (3)
- 3D discrete element method is used to study the catastrophe process of water inrush in the water-resistant strata with multi fractures during tunnel excavation. The change from the single effect of unloading on the extrusion displacement of karst tunnel face to combined action of unloading and karst water pressure occurs in process of tunnel face close to karst cavity; The extrusion displacement and water flow velocity in tunnel face suddenly rise before the water inrush pathway forms, which is the important precursory information for water inrush disaster. With the increase of karst cavity scale, the water pressure of same monitoring point increase at the same time, and the minimum safe thickness of water-resistant strata also increases.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Fraldi, M.; Guarracino, F. Limit analysis of collapse mechanisms in cavities and tunnels according to the Hoek-Brown failure criterion. Int. J. Rock Mech. Min. Sci. 2009, 46, 665–673. [Google Scholar] [CrossRef]
- Fraldi, M.; Guarracino, F. Analytical solutions for collapse mechanisms in tunnels with arbitrary cross sections. Int. J. Solids Struct. 2010, 47, 216–223. [Google Scholar] [CrossRef]
- Yang, Z.H.; Zhang, J.H. Minimum safe thickness of rock plug in karst tunnel according to upper bound theorem. J. Cent. South Univ. 2016, 23, 2346–2353. [Google Scholar] [CrossRef]
- Guo, J.Q.; Chen, J.X.; Chen, F.; Huang, S.X.; Wang, H.Y. Using the Schwarz alternating method to identify critical water-resistant thickness between tunnel and concealed cavity. Adv. Civ. Eng. 2018, 2018, 8401482. [Google Scholar] [CrossRef]
- Xu, Z.H.; Wu, J.; Li, S.C.; Zhang, B.; Huang, X. Semianalytical solution to determine minimum safety thickness of rock resisting water inrush from filling-type karst caves. Int. J. Geomech. 2018, 18, 4017152. [Google Scholar] [CrossRef]
- Gan, K.R.; Yang, Y.; Jiang-She, L.I. Analysis on karst water inflow mechanisms and determination of thickness of safe rock walls: Case study on a tunnel. Tunn. Constr. 2007, 28, 13–16. [Google Scholar] [CrossRef]
- Li-Ping, L.I.; Wei, L.U.; Shu-Cai, L.I.; Zhang, Q.S.; Zhen-Hao, X.U.; Shi, S.S. Research status and developing trend analysis of the water inrush mechanism for underground engineering construction. J. Shandong Univ. 2010, 40, 104–112. [Google Scholar]
- Guo, J.Q.; Qiao, C.S. Study on water-inrush mechanism and safe thickness of rock wall of karst tunnel face. J. Chin. Railw. Soc. 2012, 34, 105–111. [Google Scholar] [CrossRef]
- Wu, Q.; Wang, M.; Wu, X. Investigations of groundwater bursting into coal mine seam floors from fault zones. Int. J. Rock Mech. Min. Sci. 2004, 41, 557–571. [Google Scholar] [CrossRef]
- Li, S.C.; Wu, J.; Xu, Z.H.; Zhou, L.; Zhang, B. A possible prediction method to determine the top concealed karst cave based on displacement monitoring during tunnel construction. Bull. Eng. Geol. Environ. 2019, 78, 341–355. [Google Scholar] [CrossRef]
- Wang, C. Study on Risk Identification and Warning of Karst Water Bursting Disaster of Railway Tunnel. Ph.D. Thesis, Beijing Jiaotong University, Beijing, China, 1 July 2015. [Google Scholar]
- Sterpi, D.; Cividini, A. A physical and numerical investigation on the stability of shallow tunnels in strain softening media. Rock Mech. Rock Eng. 2004, 37, 277–298. [Google Scholar] [CrossRef]
- Jiang, C.X.; Shi, H.P.; Li, Y.; Yu, H.M. Numerical simulation of groundwater under complex karst conditions and the prediction of roadway gushing in a coal mine: A case study in the Guang’an Longtan reservoir in Sichuan province, China. Acta Geochim. 2016, 35, 72–84. [Google Scholar] [CrossRef]
- Parise, M.; Lollino, P. A preliminary analysis of failure mechanisms in karst and man-made underground caves in Southern Italy. Geomorphology 2011, 134, 132–143. [Google Scholar] [CrossRef]
- Geng, P.; Quan, Q.; Wang, S. Study of the formation process of mud and water bursts during tunnel construction and the influence of fault dip angles. Mod. Tunn. Technol. 2015, 52, 102–109. [Google Scholar] [CrossRef]
- Liu, S.; Liu, H.; Wang, S.; Hu, B.; Zhang, X. Direct shear tests and PFC2D numerical simulation of intermittent joints. Chin. J. Rock Mech. Eng. 2008, 27, 1828–1836. [Google Scholar] [CrossRef]
- Cai, M. Influence of intermediate principal stress on rock fracturing and strength near excavation boundaries—Insight from numerical modeling. Int. J. Rock Mech. Min. Sci. 2008, 45, 763–772. [Google Scholar] [CrossRef]
- Yang, S.Q. Crack coalescence behavior of brittle sandstone samples containing two coplanar fissures in the process of deformation failure. Eng. Fract. Mech. 2011, 78, 3059–3081. [Google Scholar] [CrossRef]
- Kemeny, J.M. A model for non-linear rock deformation under compression due to sub-critical crack growth. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1991, 28, 459–467. [Google Scholar] [CrossRef]
- Li, Y.P.; Chen, L.Z.; Wang, Y.H. Experimental research on pre-cracked marble under compression. Int. J. Solids Struct. 2005, 42, 2505–2516. [Google Scholar] [CrossRef]
- Kachanov, M. On the problems of crack interactions and crack coalescence. Int. J. Fract. 2003, 120, 537–543. [Google Scholar] [CrossRef]
- Bobet, A.; Einstein, H.H. Fracture coalescence in rock-type materials under uniaxial and biaxial compression. Int. J. Rock Mech. Min. Sci. 1998, 35, 863–888. [Google Scholar] [CrossRef]
- Liu, T.; Cao, P.; Lin, H. Damage and fracture evolution of hydraulic fracturing in compression-shear rock cracks. Theor. Appl. Fract. Mech. 2014, 74, 55–63. [Google Scholar] [CrossRef]
- Li, S.C.; Yuan, Y.C.; Li, L.P.; Ye, Z.H.; Zhang, Q.Q.; Lei, T. Water inrush mechanism and minimum safe thickness of rock wall of karst tunnel face under blast excavation. Chin. J. Geotech. Eng. 2015, 37, 313–320. [Google Scholar] [CrossRef]
- Wang, X.; Wang, M. Analysis of mechanism of water inrush in Karst tunnels. In Proceedings of the American Society of Civil Engineers GeoShanghai International Conference 2006, Shanghai, China, 6–8 June 2006; pp. 66–72. [Google Scholar] [CrossRef]
- Li, L.P.; Li, S.C.; Zhang, Q.-S. Study of mechanism of water inrush induced by hydraulic fracturing in karst tunnels. Rock Soil Mech. 2010, 31, 523–528. [Google Scholar]
- Zhong, X.U.; Deng, H.; Deng, S.; Guoxiang, T.U.; Wan, K. Study on formation mechanism of water gushing in karst tunnel and safety thickness of rock wall. Yangtze River 2018, 49, 61–66. [Google Scholar] [CrossRef]
- Wang, J.X.; Zhu, H.H.; Tang, Y.Q.; Yang, L.Z. Fracture mechanical model and hydrochemical-hydraulic coupled damage evolution equation of limestone. J. Tongji Univ. 2004, 32, 1320–1324. [Google Scholar] [CrossRef]
- Olson, J.E.; Taleghani, A.; DahiTaleghani, M. Modeling simultaneous growth of multiple hydraulic fractures and their interaction with natural fractures. In Proceedings of the SPE hydraulic fracturing technology conference, The Woodlands, TX, USA, 19–21 January 2009; pp. 1–7. [Google Scholar] [CrossRef]
- Wu, K.; Olson, J.E. A simplified three-dimensional displacement discontinuity method for multiple fracture simulations. Int. J. Fract. 2015, 193, 191–204. [Google Scholar] [CrossRef]
- Vahab, M.; Khalili, N. X-fem modeling of multizone hydraulic fracturing treatments within saturated porous media. Rock Mech. Rock Eng. 2018, 51, 3219–3239. [Google Scholar] [CrossRef]
- Itasca Consulting Group Inc. 3UEDC (3 Dimension Distinct Element Code); Itasca: Minneapolis, MN, USA, 2011. [Google Scholar]



| Material Property | Bulk Modulus (GPa) | Shear Modulus (GPa) | Density (kg/m3) | Friction (°) | Cohesion (MPa) | Tensile Strength (MPa) | Joint Normal Stiffness (GPa/m) | Joint Shear Stiffness (GPa/m) |
|---|---|---|---|---|---|---|---|---|
| Rock | 22.6 | 11.1 | 26.6 | 42 | 0.86 | 0.45 | ||
| Joint | - | - | - | 30 | 0.86 | 0.0 | 18.6 | 6.2 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, J.; Qian, Y.; Chen, J.; Chen, F. The Minimum Safe Thickness and Catastrophe Process for Water Inrush of a Karst Tunnel Face with Multi Fractures. Processes 2019, 7, 686. https://doi.org/10.3390/pr7100686
Guo J, Qian Y, Chen J, Chen F. The Minimum Safe Thickness and Catastrophe Process for Water Inrush of a Karst Tunnel Face with Multi Fractures. Processes. 2019; 7(10):686. https://doi.org/10.3390/pr7100686
Chicago/Turabian StyleGuo, Jiaqi, Yuan Qian, Jianxun Chen, and Fan Chen. 2019. "The Minimum Safe Thickness and Catastrophe Process for Water Inrush of a Karst Tunnel Face with Multi Fractures" Processes 7, no. 10: 686. https://doi.org/10.3390/pr7100686
APA StyleGuo, J., Qian, Y., Chen, J., & Chen, F. (2019). The Minimum Safe Thickness and Catastrophe Process for Water Inrush of a Karst Tunnel Face with Multi Fractures. Processes, 7(10), 686. https://doi.org/10.3390/pr7100686





