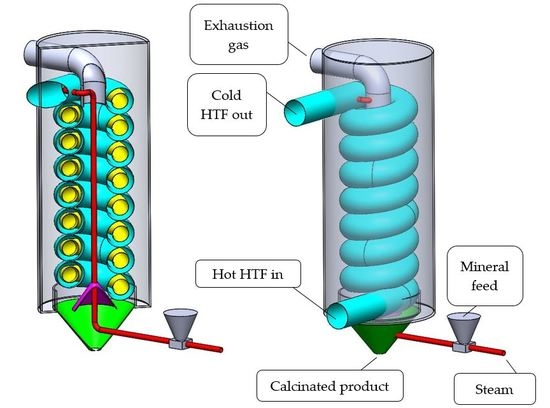
Stop Smoking—Tube-In-Tube Helical System for Flameless Calcination of Minerals
Abstract
1. Introduction
2. Methodology
2.1. Heat Transfer
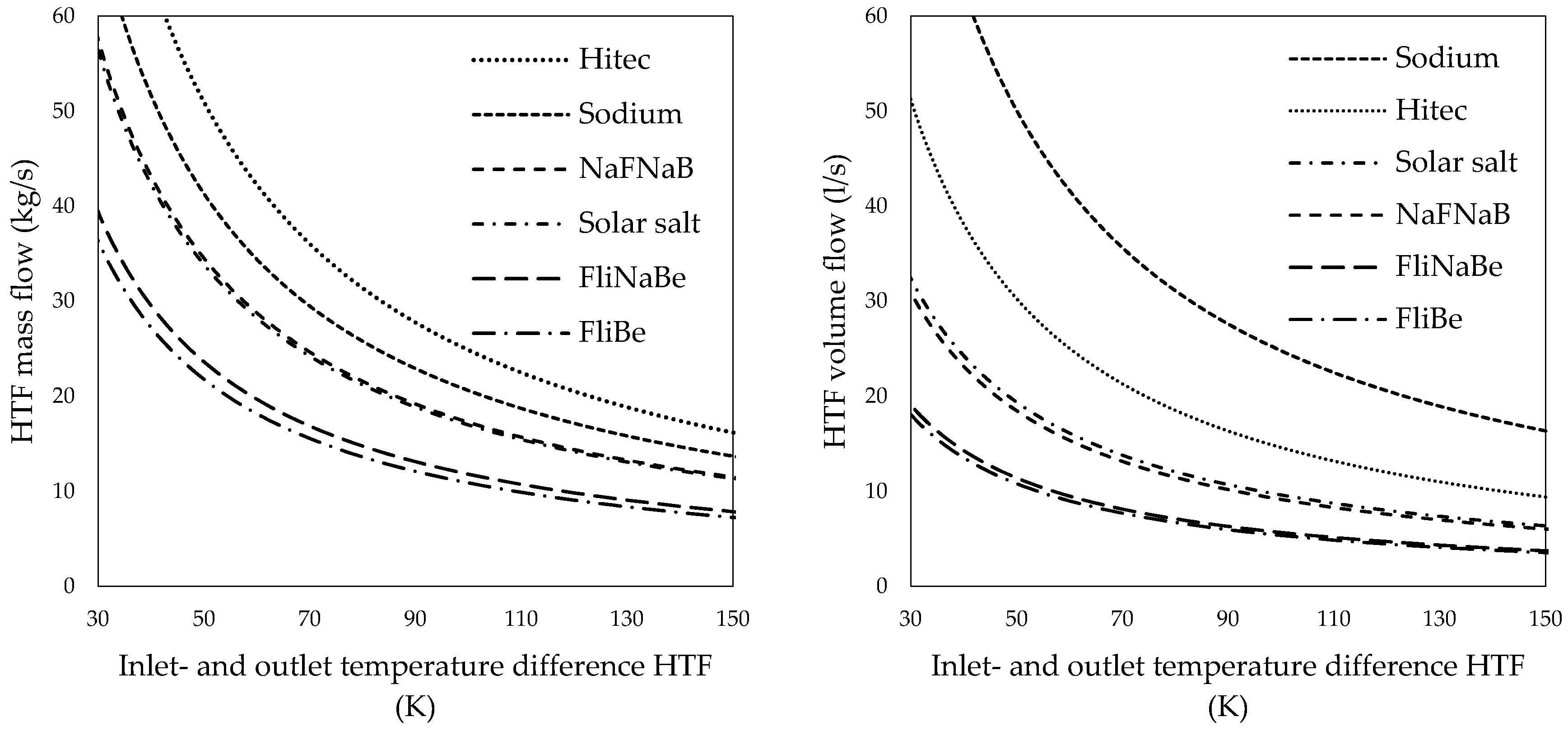
2.2. Outer Tube Mass and Volume Flows
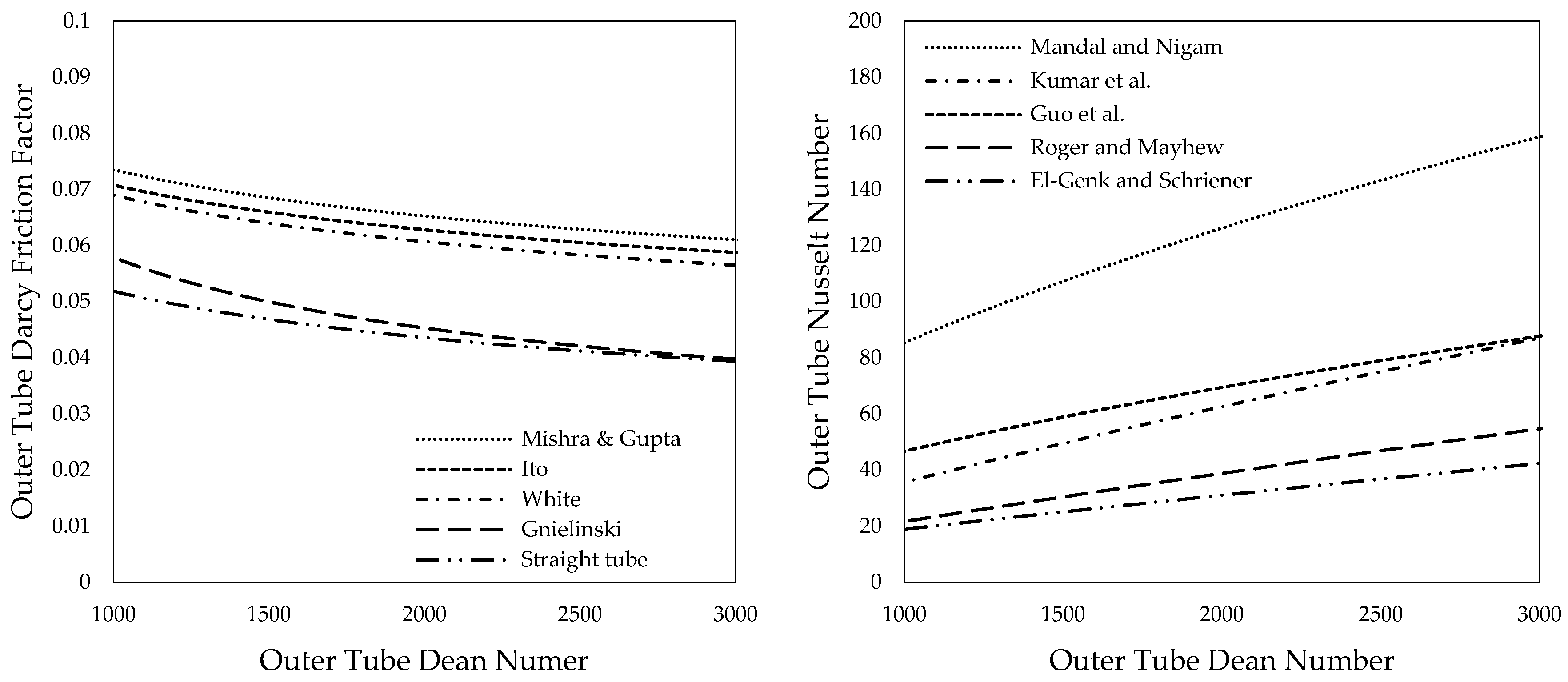
2.3. Outer Tube Friction Factors
2.4. Outer Tube Friction Factors
3. Results and Discussion
3.1. Total Heat Transfer Coefficient
3.2. Outer Tube Mass and Volume Flows
3.3. Outer Tube Friction Factors
3.4. Outer Tube Nusselt Numbers
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Nomenclature
| A | area (m2) |
| d | tube diameter (m) |
| D | Coil diameter (m) |
| fD | Darcy friction factor |
| Nu | Nusselt number |
| q | heat flow (W) |
| t | time (s) |
| U | Overall heat transfer coefficient (W/(m2K)) |
| Y | degree of calcination |
| cp | heat capacity (J/K) |
| dh | hydraulic diameter (m2) |
| De | Dean number (Re ) |
| mass flow (kg/s) | |
| Pr | Prandtl Number |
| Re | Reynolds number |
| T | Temperature (K) |
| v | speed (m/s) |
Greek symbols
| degree of reaction | |
| η | dynamic viscosity (Ps s) |
| difference operator | |
| density (kg/m3) |
References
- Worrell, E.; Price, L.; Martin, N.; Hendriks, C.; Meida, L.O. Carbon Dioxide Emission from the Global Cement Industry. Annu. Rev. Energy Environ. 2001, 26, 303–329. [Google Scholar] [CrossRef]
- Ali, M.B.; Saidur, R.; Hossain, M.S. A review on emission analysis in cement industries. Renew. Sustain. Energy Rev. 2011, 15, 2252–2261. [Google Scholar] [CrossRef]
- Wang, Q.; Luo, J.; Zhong, Z.; Borgna, A. CO2 capture by solid adsorbents and their applications: Current status and new trends. Energy Environ. Sci. 2011, 4, 42–55. [Google Scholar] [CrossRef]
- Yu, C.H.; Huang, C.H.; Tan, C.S. A review of CO2 capture by absorption and adsorption. Aerosol Air Qual. Res. 2012, 12, 745–769. [Google Scholar] [CrossRef]
- Leung, D.Y.C.; Caramanna, G.; Maroto-Valer, M.M. An overview of current status of carbon dioxide capture and storage technologies. Renew. Sustain. Energy Rev. 2014, 39, 426–443. [Google Scholar] [CrossRef]
- Atsonios, K.; Grammelis, P.; Antiohos, S.K.; Nikolopoulos, N.; Kakaras, E. Integration of calcium looping technology in existing cement plant for CO2 capture: Process modeling and technical considerations. Fuel 2015, 153, 210–223. [Google Scholar] [CrossRef]
- Reich, L.; Yue, L.; Bader, R.; Lipiński, W. Towards solar thermochemical carbon dioxide capture via calcium oxide looping: A review. Aerosol Air Qual. Res. 2014, 14, 500–514. [Google Scholar] [CrossRef]
- Benhelal, E.; Zahedi, G.; Shamsaei, E.; Bahadori, A. Global strategies and potentials to curb CO2 emissions in cement industry. J. Clean. Prod. 2013, 51, 142–161. [Google Scholar] [CrossRef]
- Flamant, G.; Hernandez, D.; Bonet, C.; Traverse, J.P. Experimental aspects of the thermochemical conversion of solar energy; Decarbonation of CaCO3. Sol. Energy 1980, 24, 385–395. [Google Scholar] [CrossRef]
- Flamant, G.; Gauthier, D.; Boudhari, C.; Flitris, Y. A 50 kW fluidized bed high temperature solar receiver: Heat transfer analysis. J. Sol. Energy Eng. 1988, 110, 313–320. [Google Scholar] [CrossRef]
- Licht, S.; Wu, H.; Hettige, C.; Wang, B.; Asercion, J.; Lau, J.; Stuart, J. STEP cement: Solar Thermal Electrochemical Production of CaO without CO2 emission. Chem. Commun. 2012, 48, 6019–6021. [Google Scholar] [CrossRef] [PubMed]
- Meier, A.; Bonaldi, E.; Cella, G.M.; Lipinski, W.; Wuillemin, D. Solar chemical reactor technology for industrial production of lime. Sol. Energy 2006, 80, 1355–1362. [Google Scholar] [CrossRef]
- Meier, A.; Bonaldi, E.; Cella, G.M.; Lipinski, W.; Wuillemin, D.; Palumbo, R. Design and experimental investigation of a horizontal rotary reactor for the solar thermal production of lime. Energy 2004, 29, 811–821. [Google Scholar] [CrossRef]
- Meier, A.; Gremaud, N.; Steinfeld, A. Economic evaluation of the industrial solar production of lime. Energy Convers. Manag. 2005, 46, 905–926. [Google Scholar] [CrossRef]
- Meier, A.; Bonaldi, E.; Cella, G.M.; Lipinski, W. Multitube Rotary Kiln for the Industrial Solar Production of Lime. J. Sol. Energy Eng. 2005, 127, 386–395. [Google Scholar] [CrossRef]
- Salman, O.A.; Kraishi, N. Thermal decomposition of limestone and gypsum by solar energy. Sol. Energy 1988, 41, 305–308. [Google Scholar] [CrossRef]
- Sceats, M.G.; Horley, C.J.; Richardson, P. System and Method for the Calcination of Minerals. U.S. Patent US 8,807,993, 19 August 2014. [Google Scholar]
- Haneklaus, N.; Reitsma, F.; Tulsidas, H. High Temperature Reactors for a new IAEA Coordinated Research Project on energy neutral mineral development processes. Nucl. Eng. Des. 2016, 306, 198–202. [Google Scholar] [CrossRef]
- Fütterer, M.A.; Li, F.; Sink, C.; de Groot, S.; Pouchon, M.; Kim, Y.W.; Carré, F.; Tachibana, Y. Status of the very high temperature reactor system. Prog. Nucl. Energy 2014, 77, 266–281. [Google Scholar] [CrossRef]
- FEECO. The Rotary Kiln Handbook; FEECO International Inc.: Green Bay, WI, USA, 2017. [Google Scholar]
- Abanades, J.C.; Anthony, E.J.; Wang, J.; Oakey, J.E. Fluidized Bed Combustion Systems Integrating CO2 Capture with CaO. Environ. Sci. Technol. 2005, 39, 2861–2866. [Google Scholar] [CrossRef] [PubMed]
- Höftberger, D.; Karl, J. Self-Fluidization in an Indirectly Heated Calciner. Chem. Eng. Technol. 2013, 36, 1533–1538. [Google Scholar] [CrossRef]
- Höftberger, D.; Karl, J. The Indirectly Heated Carbonate Looping Process for CO2 Capture—A Concept With Heat Pipe Heat Exchanger. J. Energy Ressour. Technol. 2016, 138, 042211. [Google Scholar] [CrossRef]
- Junk, M.; Reitz, M.; Ströhle, J.; Epple, B. Technical and Economical Assessment of the Indirectly Heated Carbonate Looping Process. J. Energy Ressour. Technol. 2016, 138, 042210. [Google Scholar] [CrossRef]
- Reitz, M.; Junk, M.; Ströhle, J.; Epple, B. Design and operation of a 300 kWth indirectly heated carbonate looping pilot plant. Int. J. Greenh. Gas Control 2016, 54, 272–281. [Google Scholar] [CrossRef]
- Moon, H.; Yoo, H.; Seo, H.; Park, Y.-K.; Cho, H.H. Thermal design of heat-exchangeable reactors using a dry-sorbent CO2 capture multi-step process. Energy 2015, 84, 704–713. [Google Scholar] [CrossRef]
- Aly, W.I.A. Numerical study on turbulent heat transfer and pressure drop of nanofluid in coiled tube-in-tube heat exchangers. Energy Convers. Manag. 2014, 79, 304–316. [Google Scholar] [CrossRef]
- Haneklaus, N.; Schröders, S.; Zheng, Y.; Allelein, H.-J. Economic evaluation of flameless phosphate rock calcination using concentrated solar power and high temperature reactors. Energy 2017, 140, 1148–1157. [Google Scholar] [CrossRef]
- Maclntire, W.H.; Stansel, T.B. Steam Catalysis in Calcinations of Dolomite and Limestone Fines. Ind. Eng. Chem. 1953, 45, 1548–1555. [Google Scholar] [CrossRef]
- Donat, F.; Florin, N.H.; Anthony, E.J.; Fennell, P.S. Influence of high-temperature steam on the reactivity of CaO sorbent for CO2 capture. Environ. Sci. Technol. 2012, 46, 1262–1269. [Google Scholar] [CrossRef] [PubMed]
- Li, Z.H.; Wang, Y.; Xu, K.; Yang, J.Z.; Niu, S.B.; Yao, H. Effect of steam on CaO regeneration, carbonation and hydration reactions for CO2 capture. Fuel Process. Technol. 2016, 151, 101–106. [Google Scholar] [CrossRef]
- Liu, W.; An, H.; Qin, C.; Yin, J.; Wang, G.; Feng, B.; Xu, M. Performance enhancement of calcium oxide sorbents for cyclic CO2 capture—A review. Energy Fuels 2012, 26, 2751–2767. [Google Scholar] [CrossRef]
- Zarghami, S.; Ghadirian, E.; Arastoopour, H.; Abbasian, J. Effect of Steam on Partial Decomposition of Dolomite. Ind. Eng. Chem. Res. 2015, 54, 5398–5406. [Google Scholar] [CrossRef]
- Abouzeid, A.Z.M. Physical and thermal treatment of phosphate ores—An overview. Int. J. Miner. Process. 2008, 85, 59–84. [Google Scholar] [CrossRef]
- Kearney, D.; Herrmann, U.; Nava, P.; Kelly, B.; Mahoney, R.; Pacheco, J.; Cable, R.; Potrovitza, N.; Blake, D.; Price, H. Assessment of a Molten Salt Heat Transfer Fluid in a Parabolic Trough Solar Field. J. Sol. Energy Eng. 2003, 125, 170–176. [Google Scholar] [CrossRef]
- Goods, S.H.; Bradshaw, R.W. Corrosion of stainless steels and carbon steel by molten mixtures of commercial nitrate salts. J. Mater. Eng. Perform. 2004, 13, 78–87. [Google Scholar] [CrossRef]
- McConohy, G.; Kruizenga, A. Molten nitrate salts at 600 and 680 °C: Thermophysical property changes and corrosion of high-temperature nickel alloys. Sol. Energy 2014, 103, 242–252. [Google Scholar] [CrossRef]
- Zhang, H.L.; Baeyens, J.; Degrève, J.; Cacères, G. Concentrated solar power plants: Review and design methodology. Renew. Sustain. Energy Rev. 2013, 22, 466–481. [Google Scholar] [CrossRef]
- Kolb, G.; Ho, C.; Mancini, T.; Gary, J. Power Tower Technology Roadmap and Cost Reduction Plan; SAND2011-2419; Sandia National Laboratories: Albuquerque, NM, USA, 2011. [CrossRef]
- Romero, M.; Steinfeld, A. Concentrating solar thermal power and thermochemical fuels. Energy Environ. Sci. 2012, 5, 9234–9245. [Google Scholar] [CrossRef]
- Forsberg, C.W.; Peterson, P.F.; Zhao, H. High-Temperature Liquid-Fluoride-Salt Closed-Brayton-Cycle Solar Power Towers. J. Sol. Energy Eng. 2007, 129, 141–146. [Google Scholar] [CrossRef]
- Barlev, D.; Vidu, R.; Stroeve, P. Innovation in concentrated solar power. Sol. Energy Mater. Sol. Cells 2011, 95, 2703–2725. [Google Scholar] [CrossRef]
- Vignarooban, K.; Xu, X.; Arvay, A.; Hsu, K.; Kannan, A.M. Heat transfer fluids for concentrating solar power systems—A review. Appl. Energy 2015, 146, 383–396. [Google Scholar] [CrossRef]
- Wang, Y.; Thomson, W.J. The Effects of Steam and Carbon-Dioxide on Calcite Decomposition Using Dynamic X-Ray-Diffraction. Chem. Eng. Sci. 1995, 50, 1373–1382. [Google Scholar] [CrossRef]
- Beruto, D.; Searcy, A.W. Use of the Langmuir method for kinetic studies of decomposition reactions: Calcite (CaCO3). J. Chem. Soc. Faraday Trans. 1974, 70, 2145–2153. [Google Scholar] [CrossRef]
- Olszak-Humienik, M.; Jablonski, M. Thermal behavior of natural dolomite. J. Therm. Anal. Calorim. 2015, 119, 2239–2248. [Google Scholar] [CrossRef]
- Shah, R.K.; Sekulic, D.P. Fundamentals of Heat Exchanger Design; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2003. [Google Scholar] [CrossRef]
- Mishra, P.; Gupta, S.N. Momentum Transfer in Curved Pipes. 1. Newtonian Fluids. Ind. Eng. Chem. Process. Des. Dev. 1979, 18, 130–137. [Google Scholar] [CrossRef]
- Ito, H. Friction factors for turbulent flow in curved pipes. J. Basic Eng. 1959, 81, 123–134. [Google Scholar]
- White, C.M. Friction factor and its relation to heat transfer. Trans. Inst. Chem. Eng. 1932, 18, 66–86. [Google Scholar]
- Gnielinski, V. Heat Transfer Coefficients for Turbulent Flow in Concentric Annular Ducts. Heat Transf. Eng. 2009, 30, 431–436. [Google Scholar] [CrossRef]
- Gnielinski, V. Turbulent Heat Transfer in Annular Spaces—A New Comprehensive Correlation. Heat Transf. Eng. 2015, 36, 787–789. [Google Scholar] [CrossRef]
- Gnielinski, V. Berechnung des Druckverlustes in Glatten Konzentrischen Ringspalten bei Ausgebildeter Laminarer und Turbulenter Isothermer Strömung. Chem.-Ingenieur-Technik 2007, 79, 91–95. [Google Scholar] [CrossRef]
- Konakov, P.K. A new correlation for the friction coefficient in smooth tubes—Berichte der Akademie der Wissenschaften der UdSSR. In VDI Heat Atlas; Springer: Berlin/Heidelberg, Germany, 1946; Volume 51, pp. 503–506. [Google Scholar]
- El-Genk, M.S.; Schriener, T.M. A Review and Correlations for Convection Heat Transfer and Pressure Losses in Toroidal and Helically Coiled Tubes. Heat Transf. Eng. 2017, 385, 447–474. [Google Scholar] [CrossRef]
- Ghobadi, M.; Muzychka, Y.S. A Review of Heat Transfer and Pressure Drop Correlations for Laminar Flow in Curved Circular Ducts. Heat Transf. Eng. 2016, 37, 815–839. [Google Scholar] [CrossRef]
- Huminic, G.; Huminic, A. Heat transfer and flow characteristics of conventional fluids and nanofluids in curved tubes: A review. Renew. Sustain. Energy Rev. 2016, 58, 1327–1347. [Google Scholar] [CrossRef]
- Spedding, P.L.; Benard, E.; Mcnally, G.M. Fluid Flow through 90 Degree Bends. Dev. Chem. Eng. Miner. Process. 2004, 2, 107–128. [Google Scholar] [CrossRef]
- Mandal, M.M.; Nigam, K.D.P. Experimental Study on Pressure Drop and Heat Transfer of Turbulent Flow in Tube in Tube Helical Heat Exchanger. Ind. Eng. Chem. Res. 2009, 48, 9318–9324. [Google Scholar] [CrossRef]
- Kumar, V.; Faizee, B.; Mridha, M.; Nigam, K.D.P. Numerical studies of a tube-in-tube helically coiled heat exchanger. Chem. Eng. Process. Process. Intensif. 2008, 47, 2287–2295. [Google Scholar] [CrossRef]
- Guo, L.; Chen, X.; Feng, Z.; Bai, B. Transient convective heat transfer in a helical coiled tube with pulsatile fully developed turbulent flow. Int. J. Heat Mass Transf. 1998, 41, 2867–2875. [Google Scholar] [CrossRef]
- Rogers, G.F.C.; Mayhew, Y.R. Heat transfer and pressure loss in helically coiled tubes with turbulent flow. Int. J. Heat Mass Transf. 1964, 7, 1207–1216. [Google Scholar] [CrossRef]
- Roetzel, W.; Spang, B. Typical Values of Overall Heat Transfer Coefficients. In VDI Heat Atlas; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar] [CrossRef]
- Serrano-Lopez, R.; Fradera, J.; Cuesta-Lopez, S. Molten salts database for energy applications. Chem. Eng. Process. Process. Intensif. 2013, 73, 87–102. [Google Scholar] [CrossRef]
- Boerema, N.; Morrison, G.; Taylor, R.; Rosengarten, G. Liquid sodium versus Hitec as a heat transfer fluid in solar thermal central receiver systems. Sol. Energy 2012, 86, 2293–2305. [Google Scholar] [CrossRef]
- Smith, P.G. Development of Fuel- and Coolant-Salt Centrifugal Pumps for the Molten-Salt Reactor Experiment; Oak Ridge National Laboratory: Oak Ridge, TN, USA, 1970.
- Burgaleta, J.I.; Arias, S.; Ramirez, D. Gemasolar: The First Tower Thermosolar Commercial Plant with Molten Salt Storage System. In Proceedings of the 18th SolarPACES International Conference, Marrakech, Morocco, 11–14 September 2012; pp. 11–14. [Google Scholar]
- Dunn, R.I.; Hearps, P.J.; Wright, M.N. Molten-salt power towers: Newly commercial concentrating solar storage. Proc. IEEE 2012, 100, 504–515. [Google Scholar] [CrossRef]
- Relloso, S.; García, E. Tower Technology Cost Reduction Approach after Gemasolar Experience. Energy Procedia 2015, 69, 1660–1666. [Google Scholar] [CrossRef]
- Kumar, V.; Saini, S.; Sharma, M.; Nigam, K.D.P. Pressure drop and heat transfer study in tube-in-tube helical heat exchanger. Chem. Eng. Sci. 2006, 61, 4403–4416. [Google Scholar] [CrossRef]



| Temperature Range (°C) | Mineral | Gas Pressure (Mpa) | Mineral Feed Rate (kg/s) | Steam Feed Rate (kg/s) |
|---|---|---|---|---|
| 850–960 | Limestone/calcite (CaCO3), dolomite (CaCO3 MgCO3), magnesite (MgCO3) | 0.02–0.30 | 1.40 | 0.05–0.50 |
| 500–650 | Dolomite (CaCO3 MgCO3), magnesite (MgCO3 ) | 0.02–0.30 | 1.40 | 0.05–0.50 |
| 200–400 | Hydrated materials | 0.02–0.30 | 1.00 | 0.05–0.50 |
| Authors | Correlation | Conditions |
|---|---|---|
| Mishra and Gupta [48] | 4500 < Re < 105 | |
| Ito [49] | ||
| White [50] | Not specified | |
| Konakov [54] | Not specified |
| Authors | Correlation | Conditions |
|---|---|---|
| Mandal and Nigam [59] | Not specified | |
| Kumar et al. [60] | ||
| Guo et al. [61] | ||
| Roger and Mayhew [62] | ||
| El-Genk and Schriener [55] | Not specified |
| Name | Temperature Range (°C) | |
|---|---|---|
| Flibe | 2LiF-BeF2 | 515–821 |
| FlinaBe | LiF-NaF-BeF2 | 527–752 |
| NaFNaB | NaF-NaBF4 | 400–591 |
| Solar Salt | NaNO3-KNO3 | 300–600 |
| Hitec | NaNO3-NaNO2-KNO3 | 175–500 |
| Sodium | Na | 98–873 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Haneklaus, N.; Zheng, Y.; Allelein, H.-J. Stop Smoking—Tube-In-Tube Helical System for Flameless Calcination of Minerals. Processes 2017, 5, 67. https://doi.org/10.3390/pr5040067
Haneklaus N, Zheng Y, Allelein H-J. Stop Smoking—Tube-In-Tube Helical System for Flameless Calcination of Minerals. Processes. 2017; 5(4):67. https://doi.org/10.3390/pr5040067
Chicago/Turabian StyleHaneklaus, Nils, Yanhua Zheng, and Hans-Josef Allelein. 2017. "Stop Smoking—Tube-In-Tube Helical System for Flameless Calcination of Minerals" Processes 5, no. 4: 67. https://doi.org/10.3390/pr5040067
APA StyleHaneklaus, N., Zheng, Y., & Allelein, H.-J. (2017). Stop Smoking—Tube-In-Tube Helical System for Flameless Calcination of Minerals. Processes, 5(4), 67. https://doi.org/10.3390/pr5040067