Computational Analysis of Entry-Region Flow Dynamics for Giesekus Fluids in Tubes
Abstract
1. Introduction
2. Numerical Methods
2.1. Governing Equations and Boundary Conditions
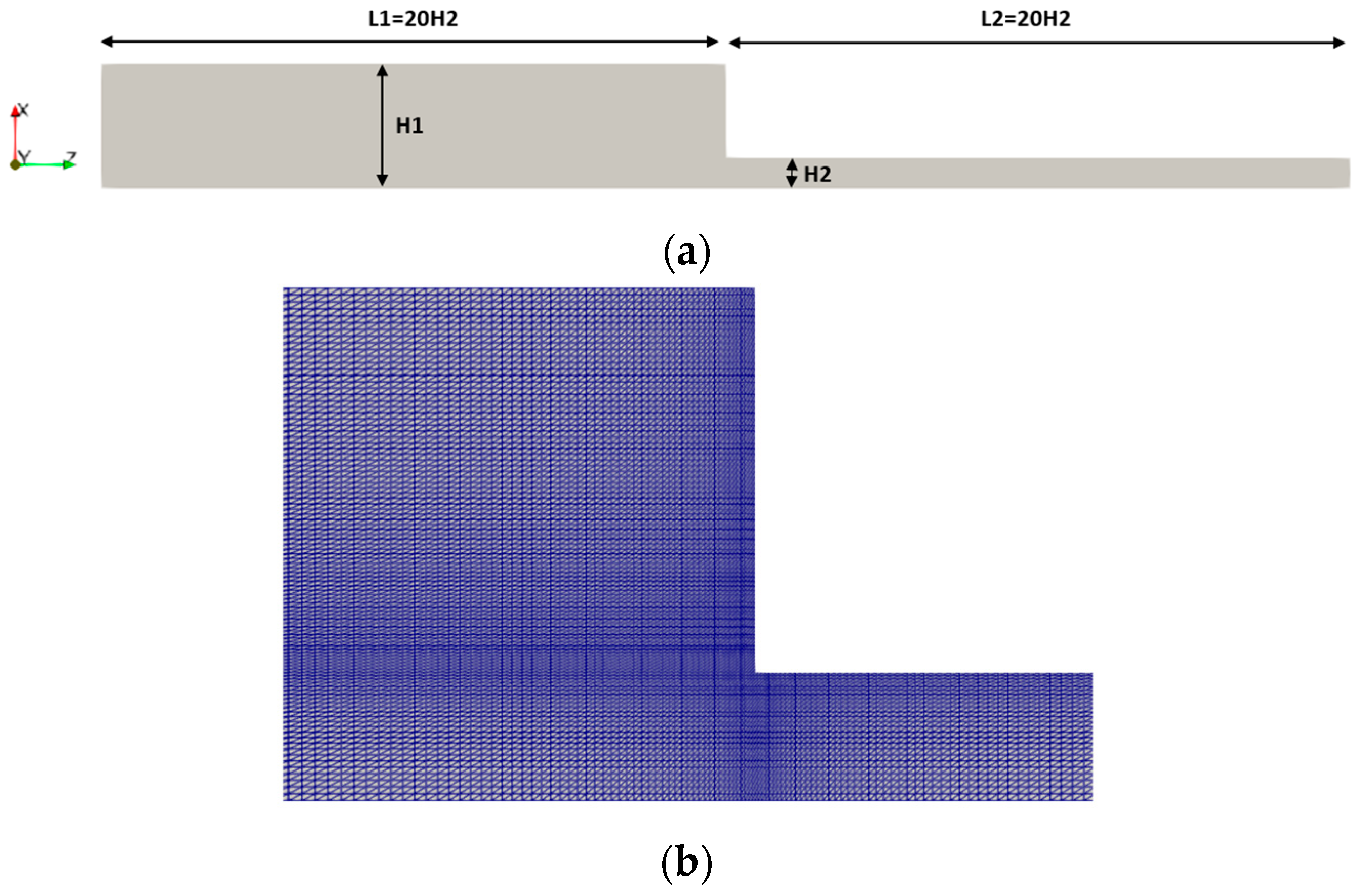
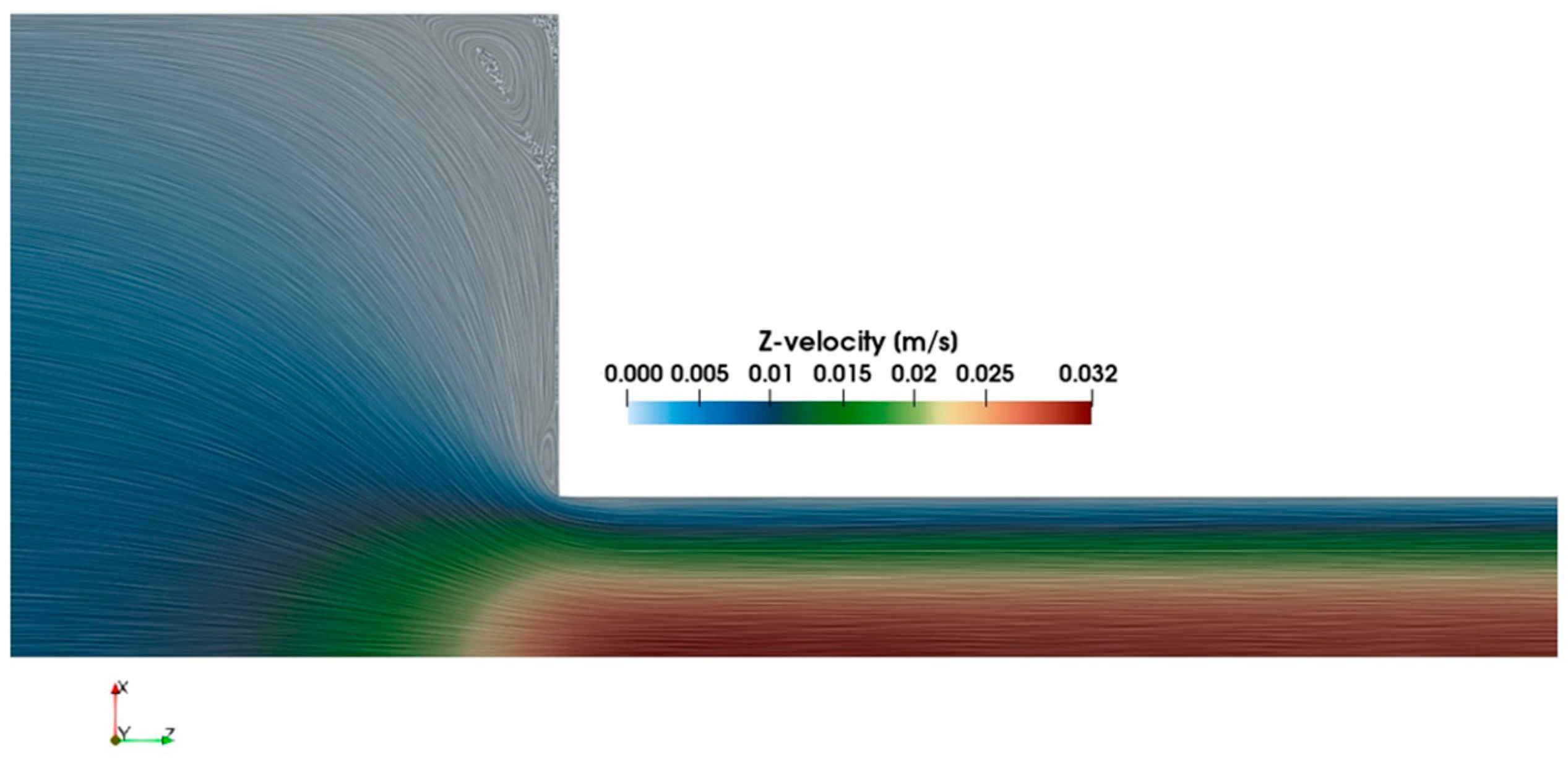
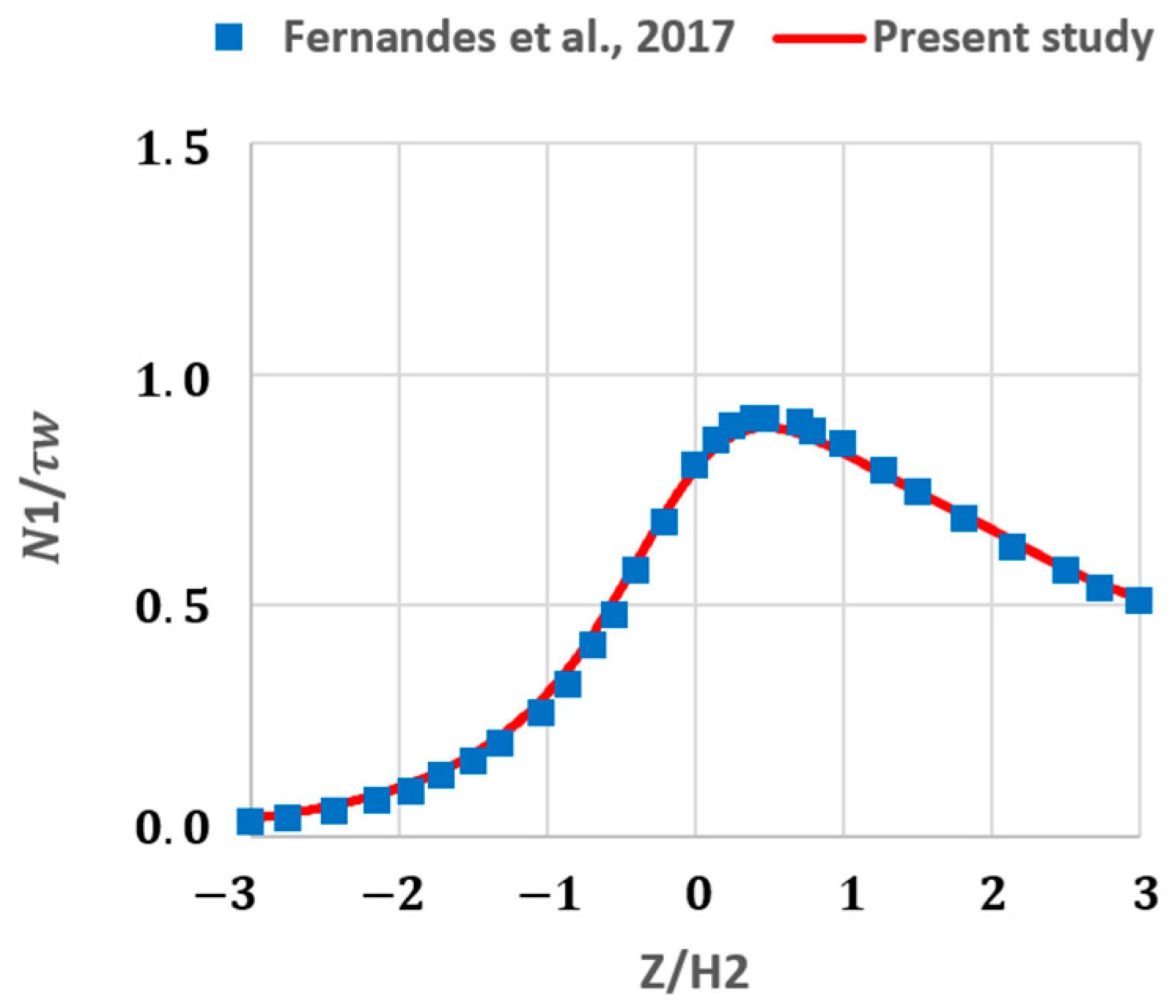
2.2. Validation of the CFD Model
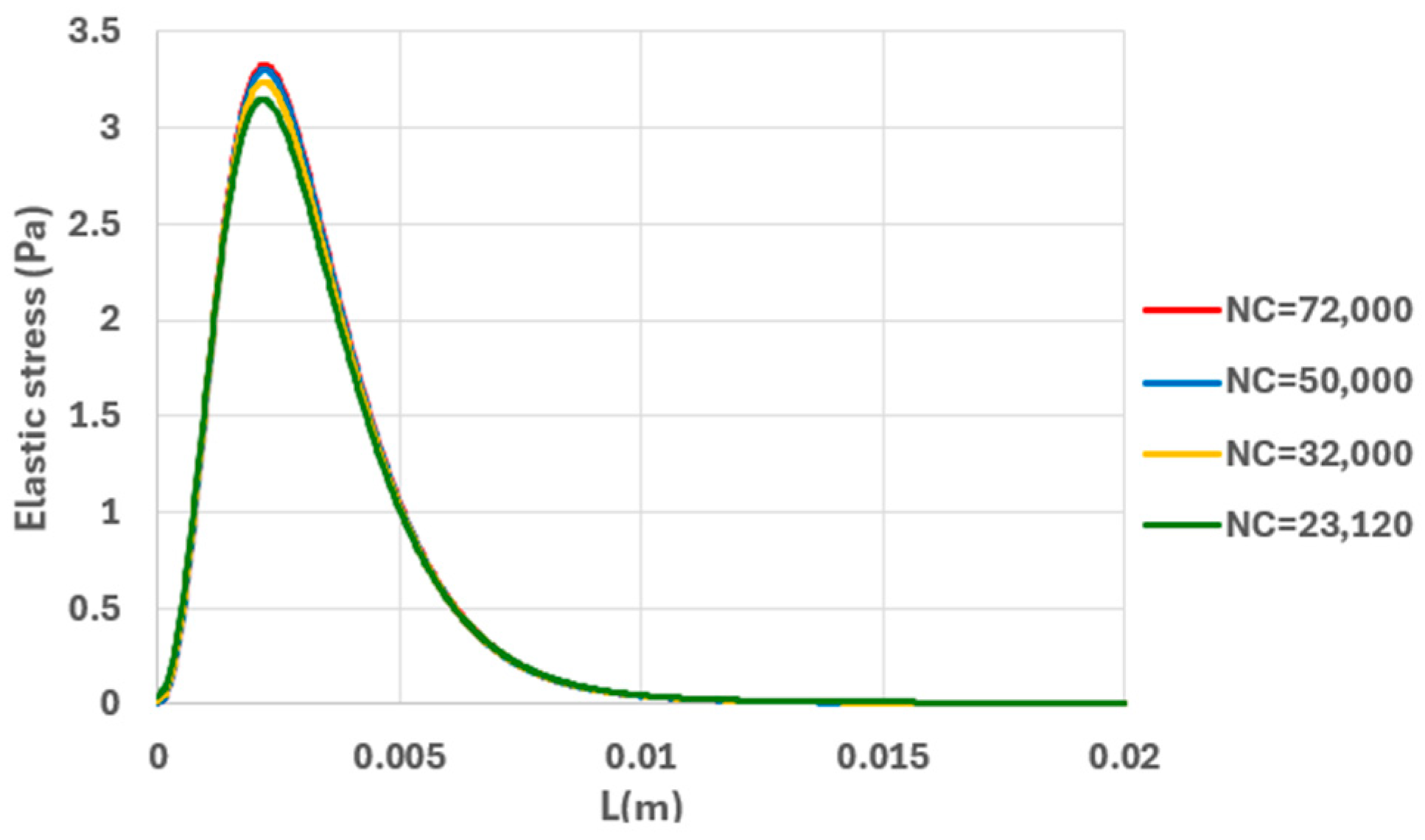
2.3. Mesh Sensitivity Analysis for Axisymmetric Tube Geometry
3. Results and Discussion
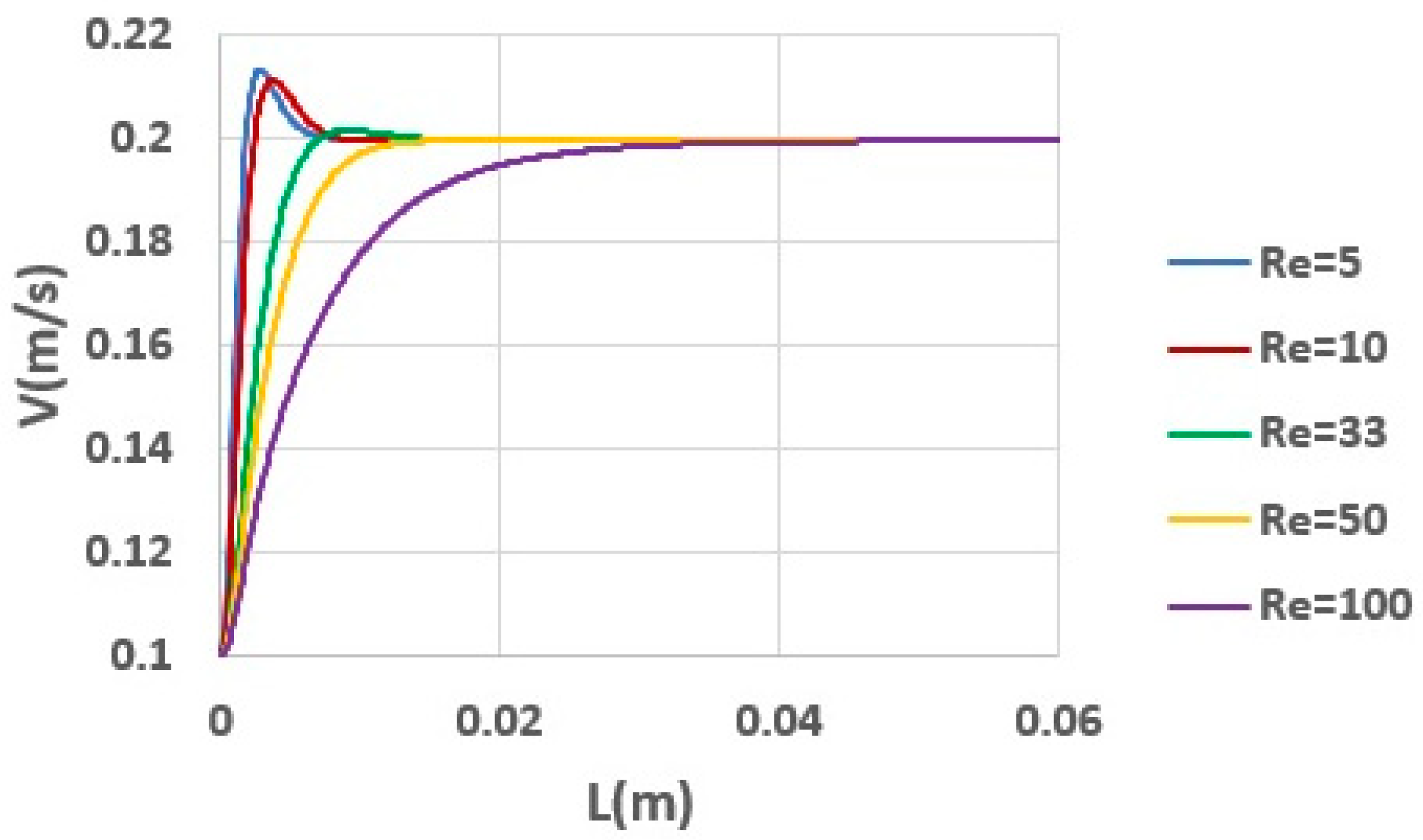
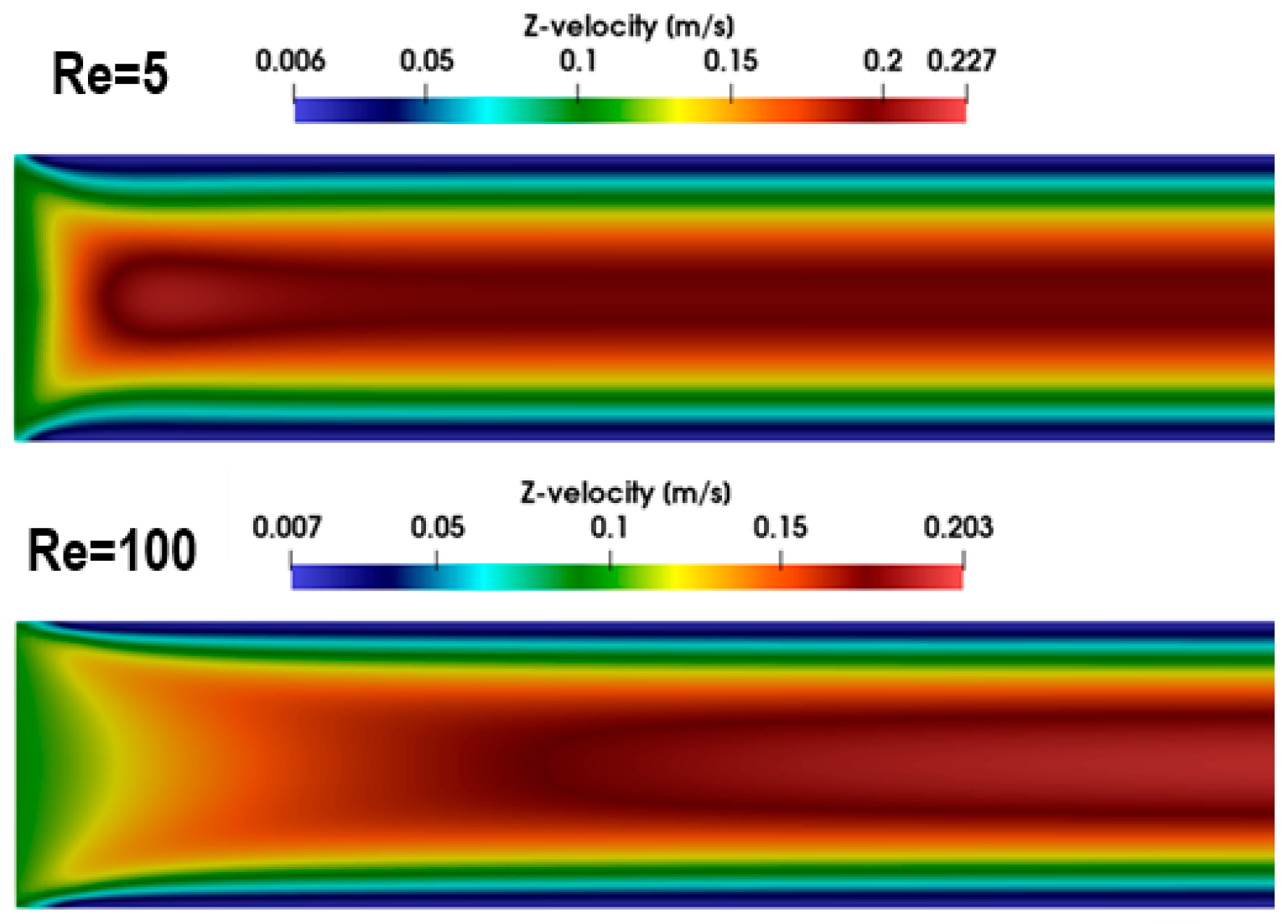
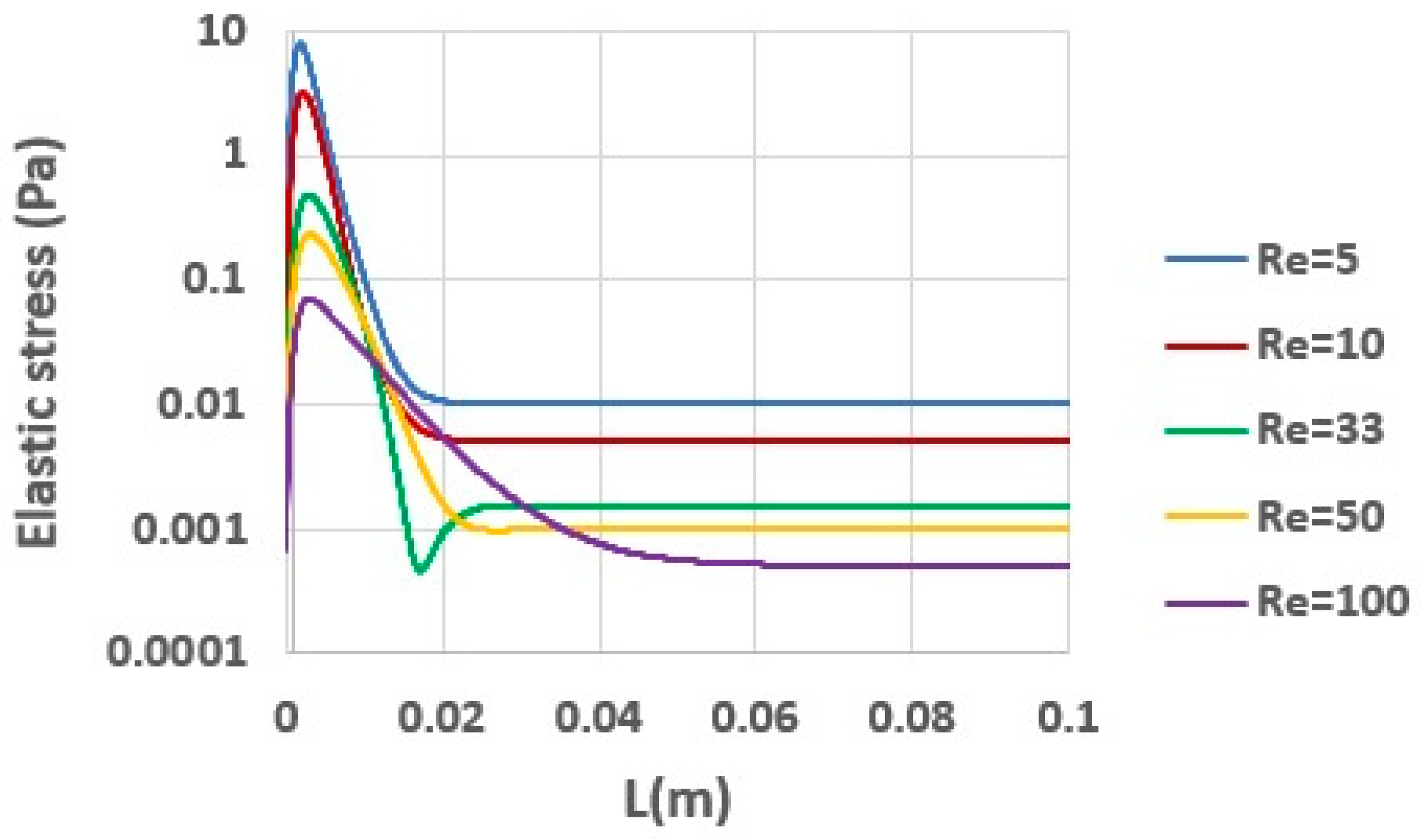
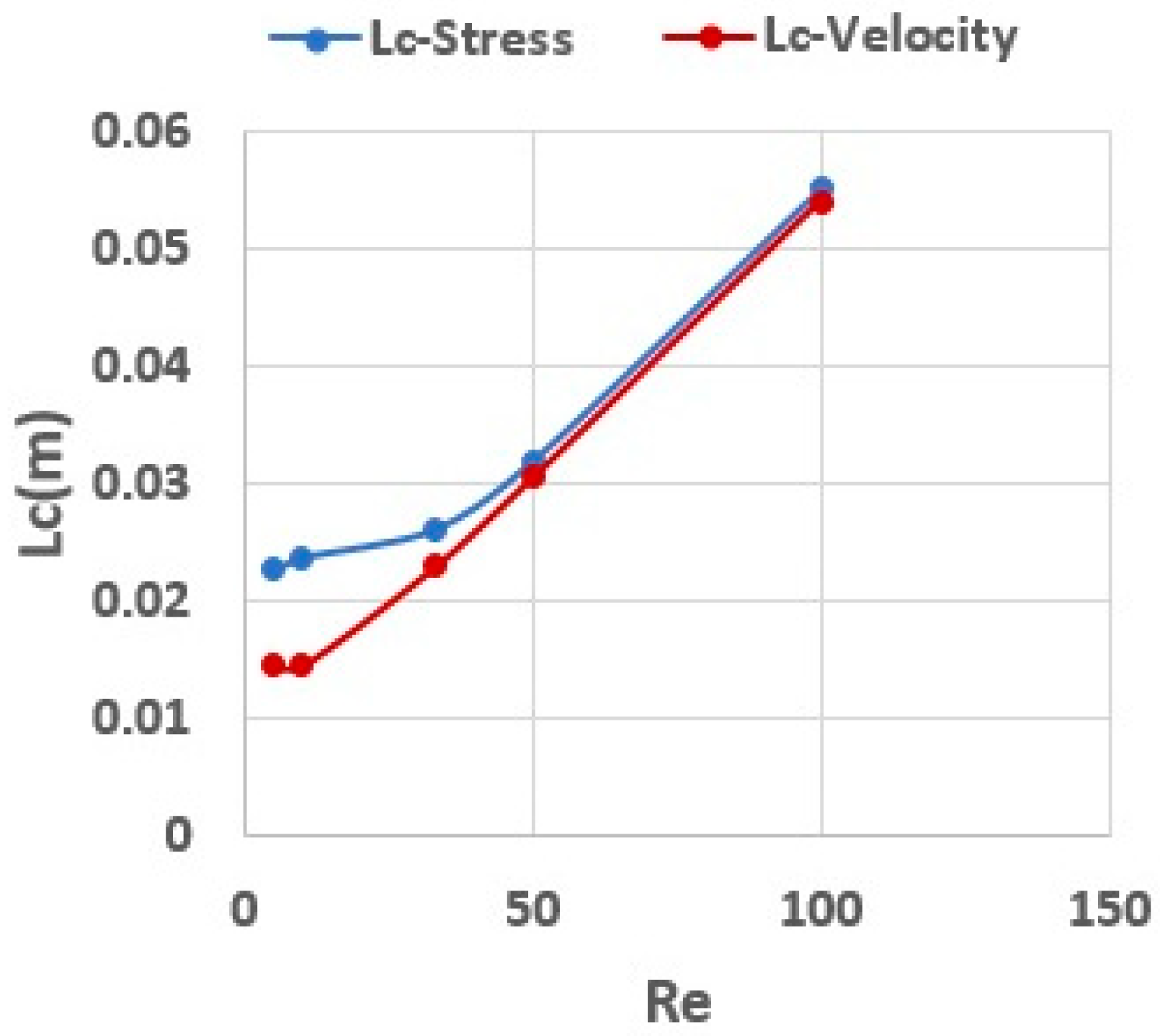
3.1. Influence of Reynolds Number
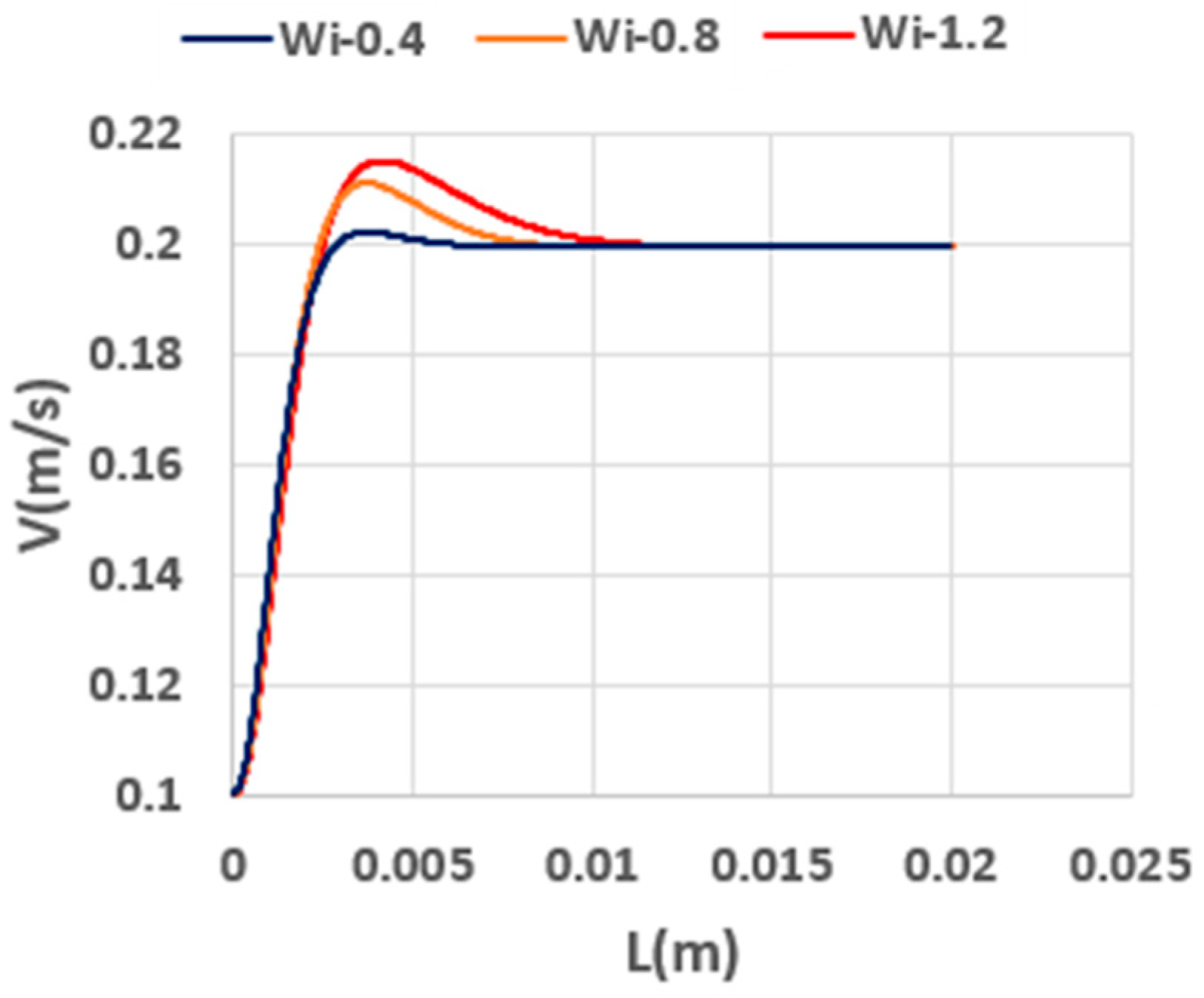
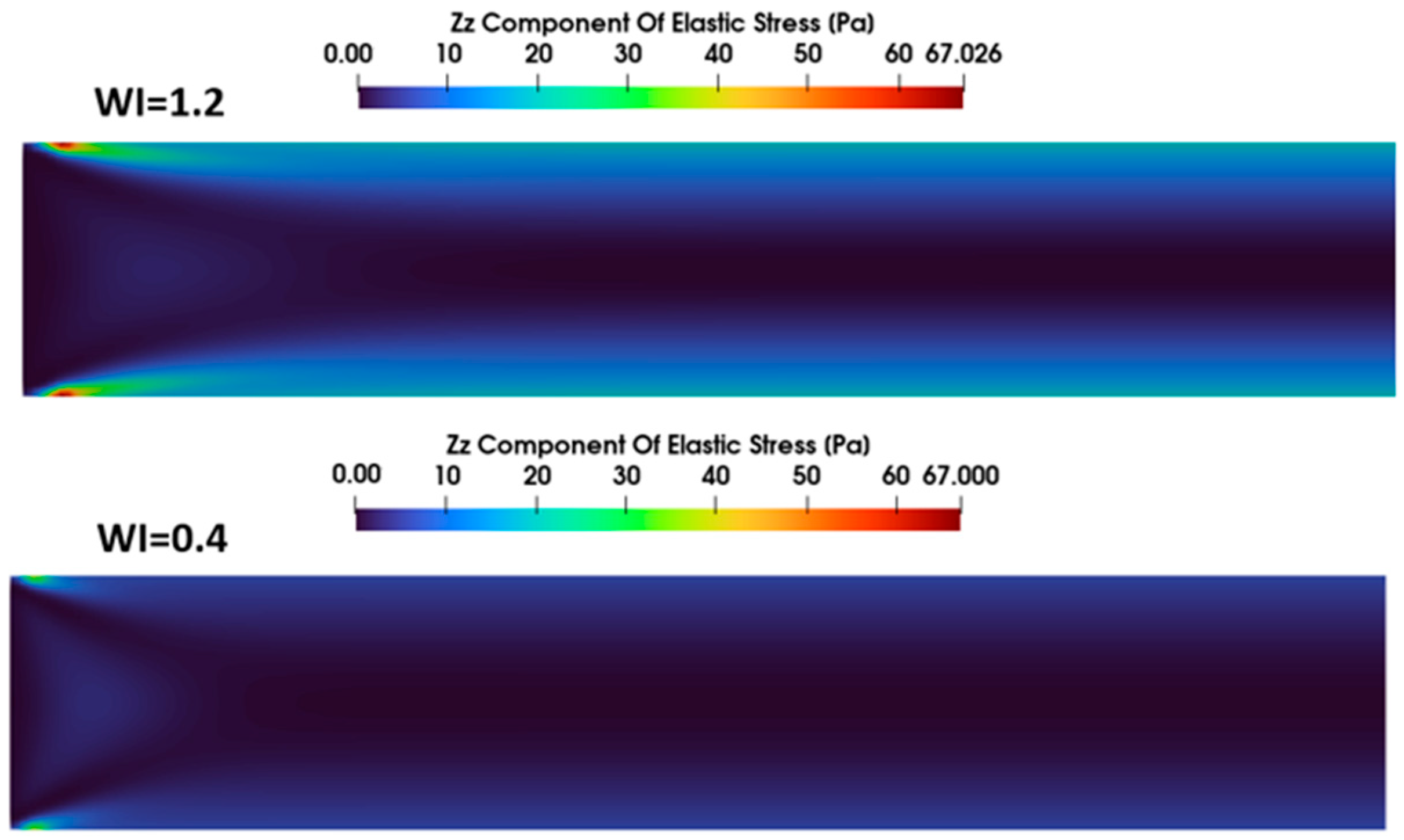
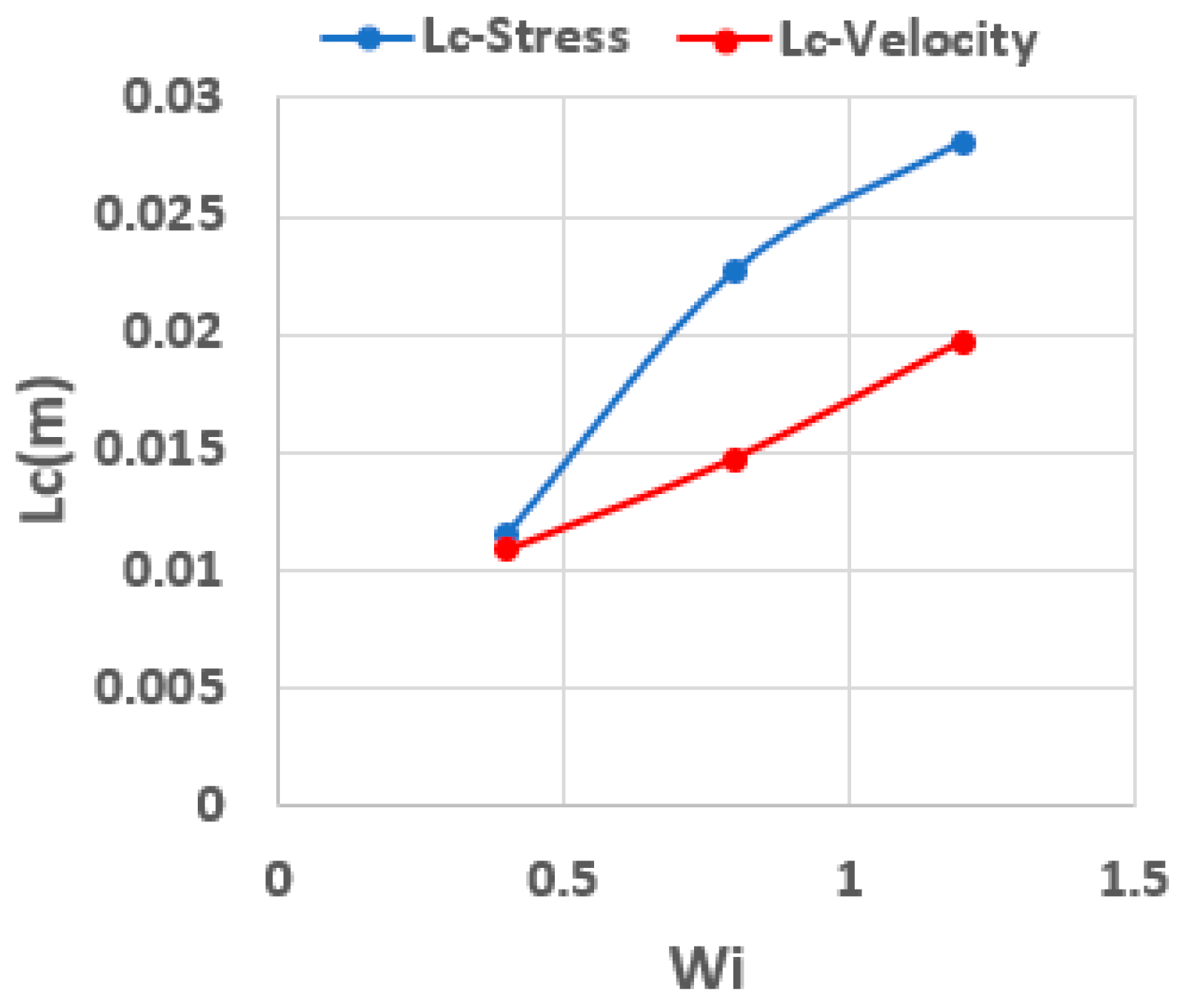
3.2. Influence of the Weissenberg Number
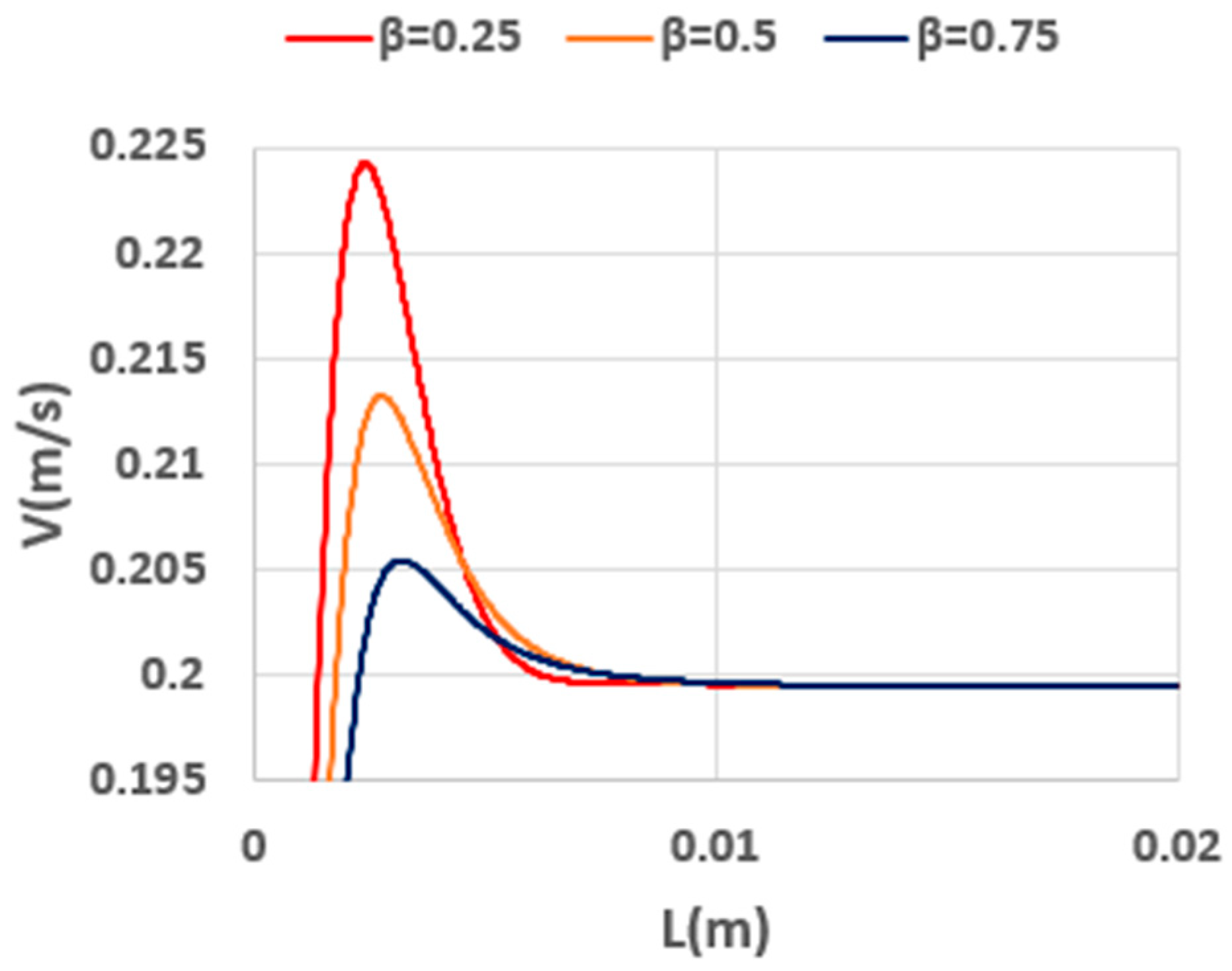
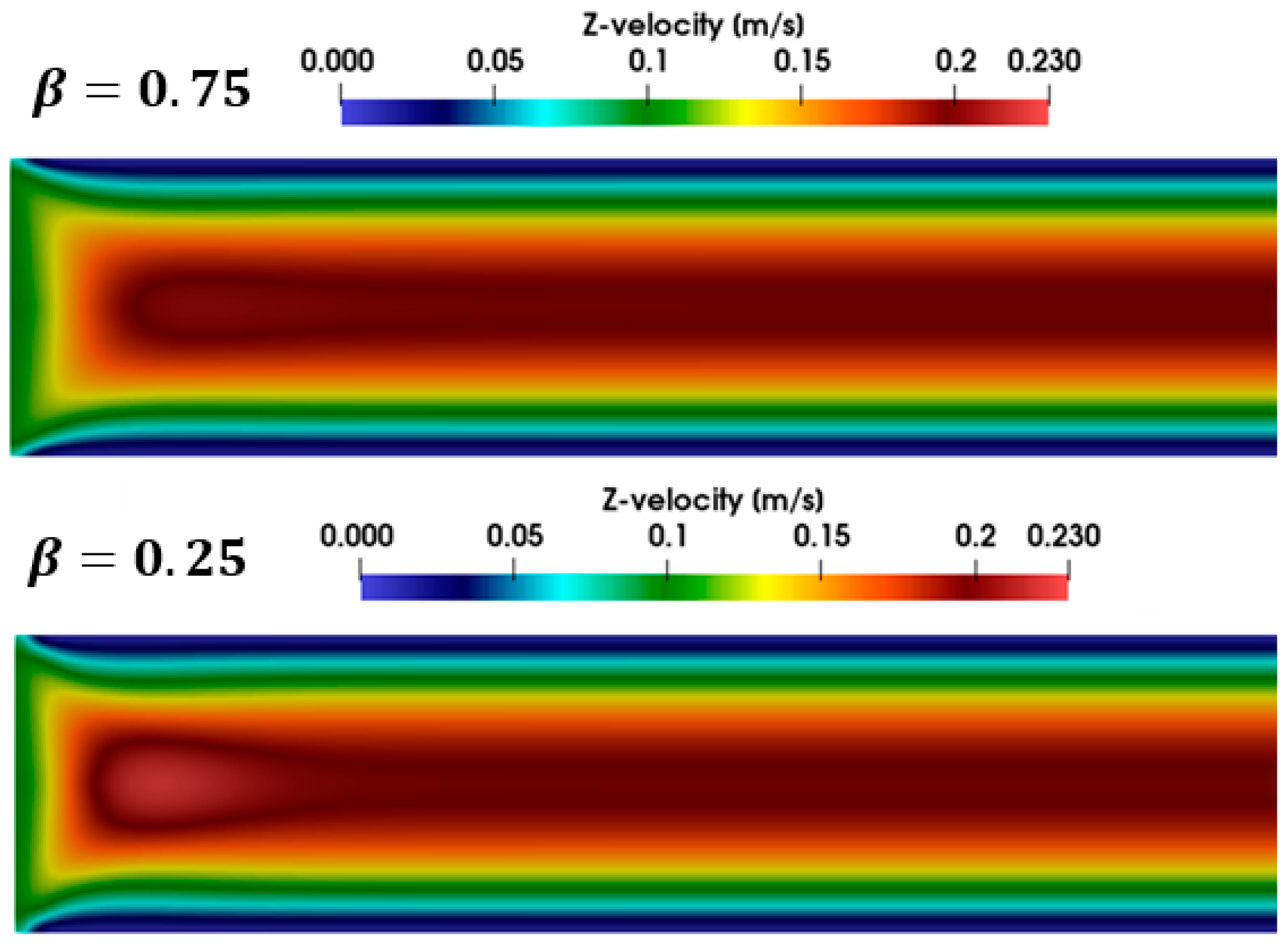
3.3. Influence Solvent Viscosity Ratio
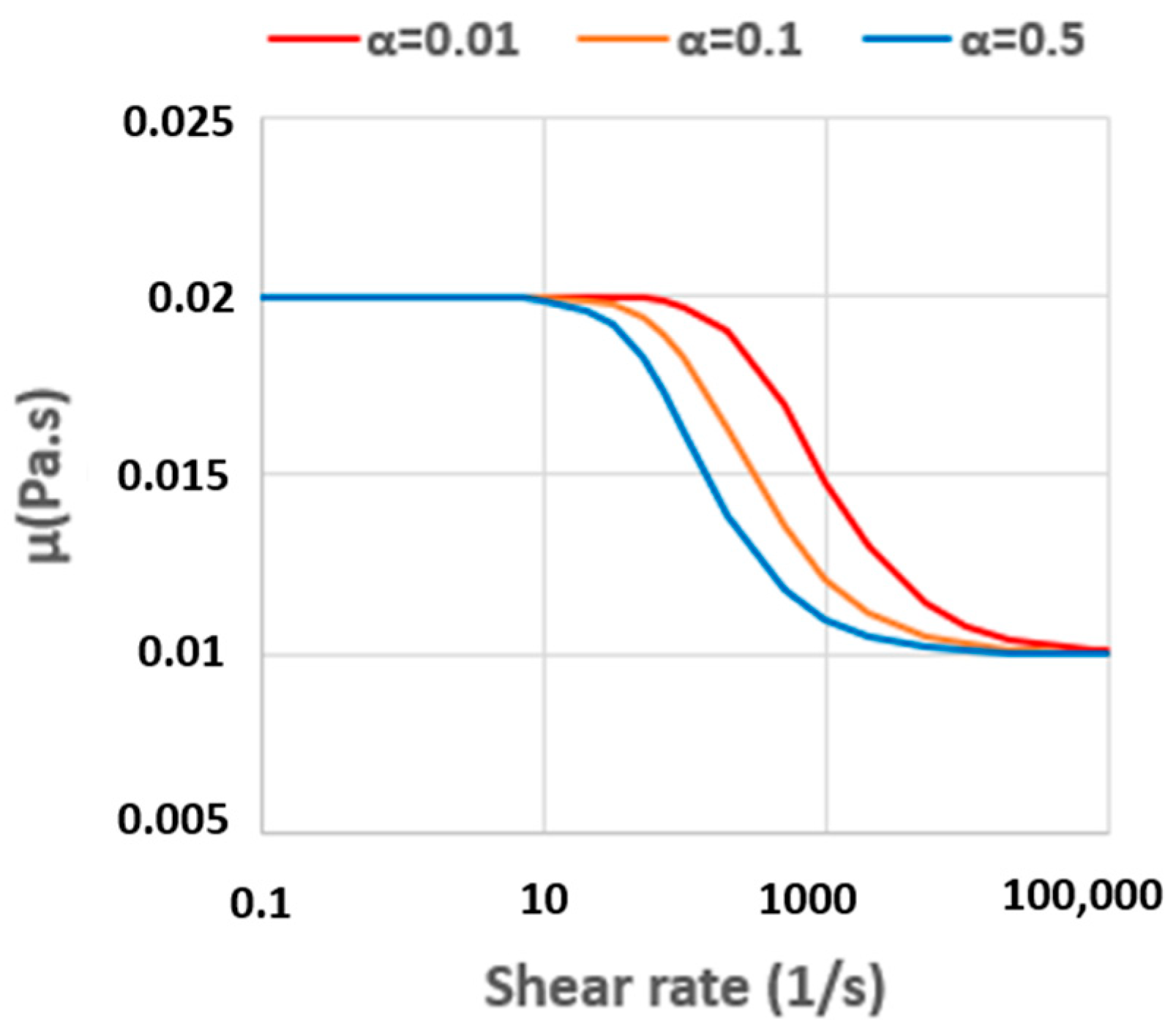
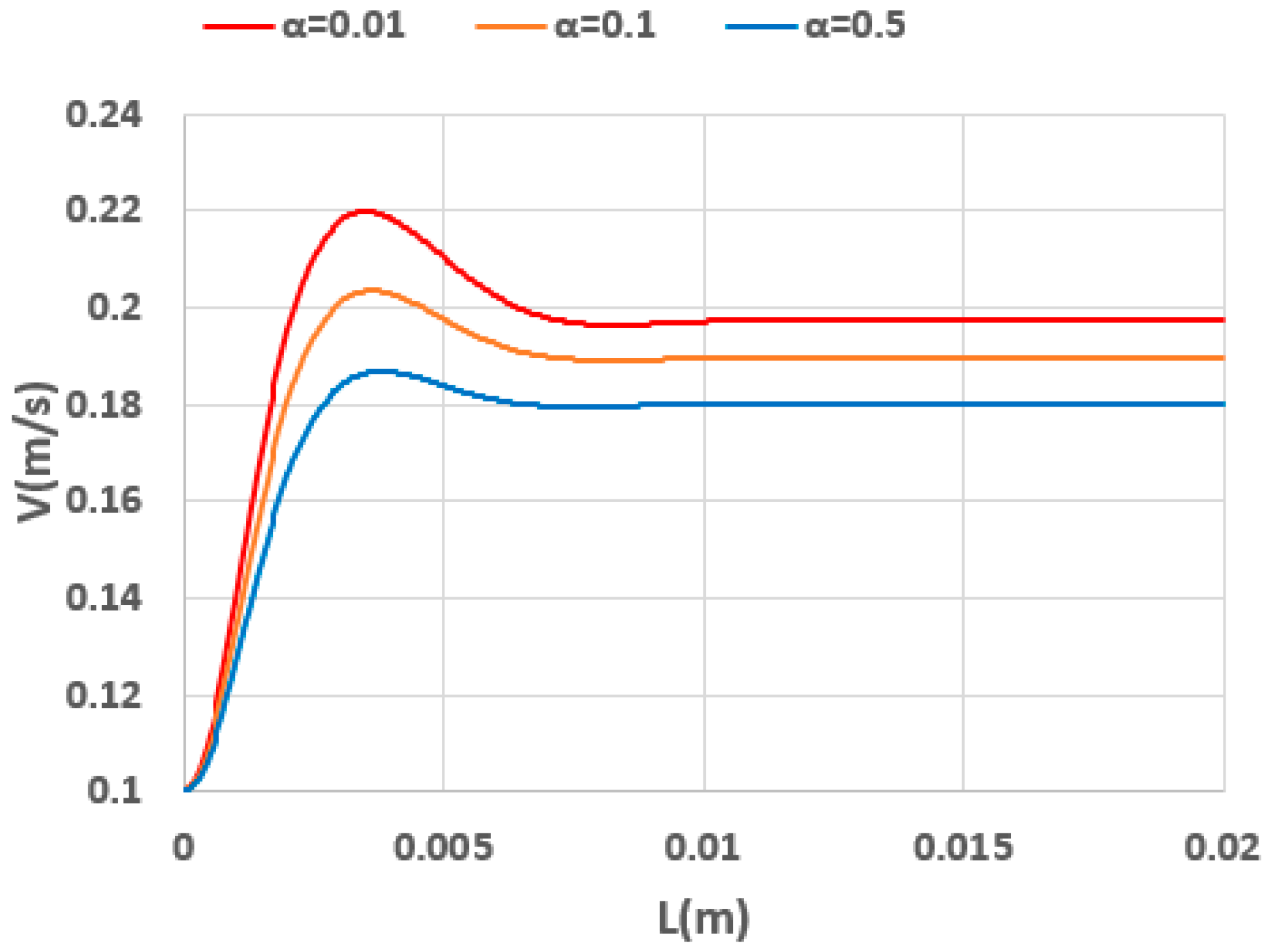
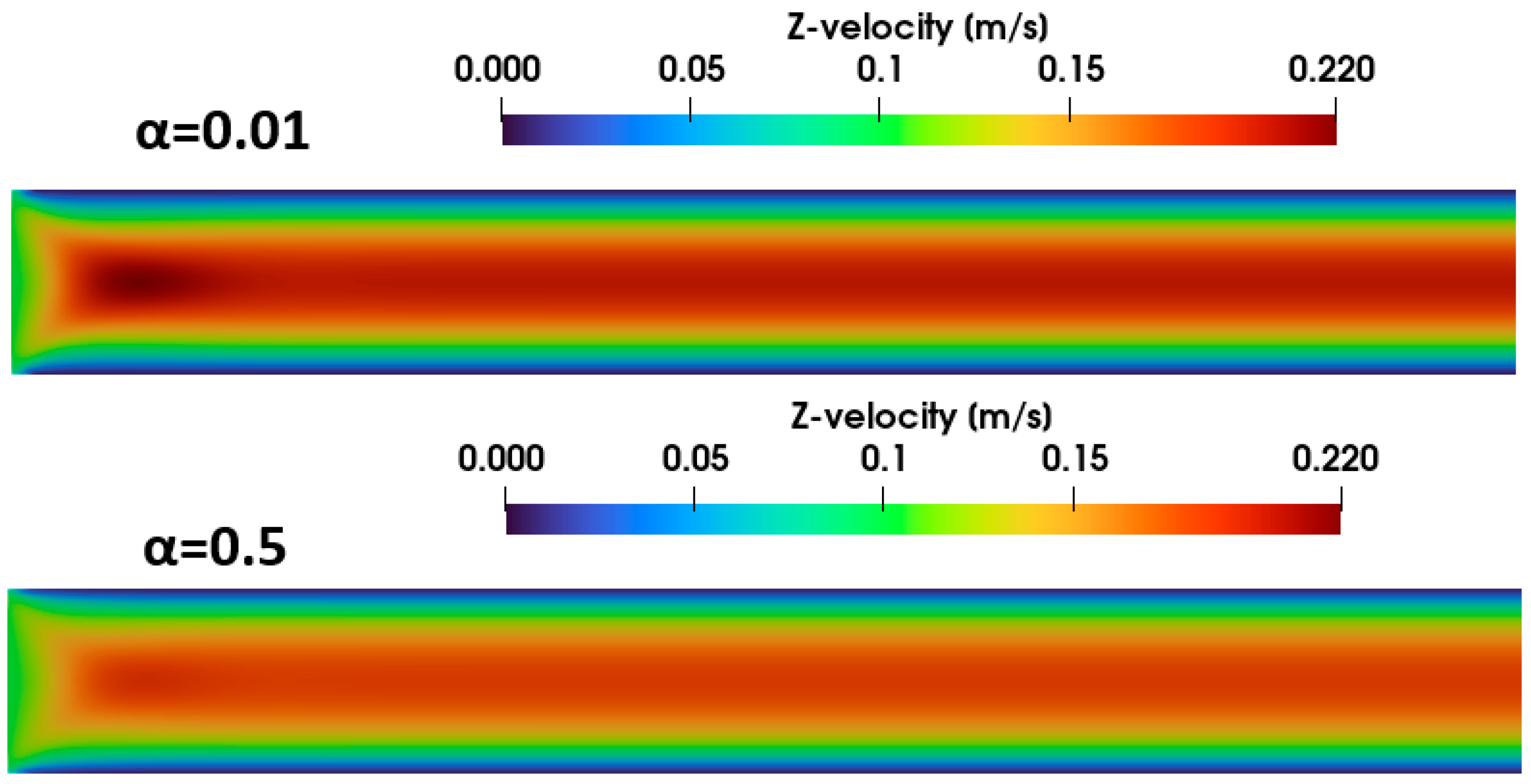
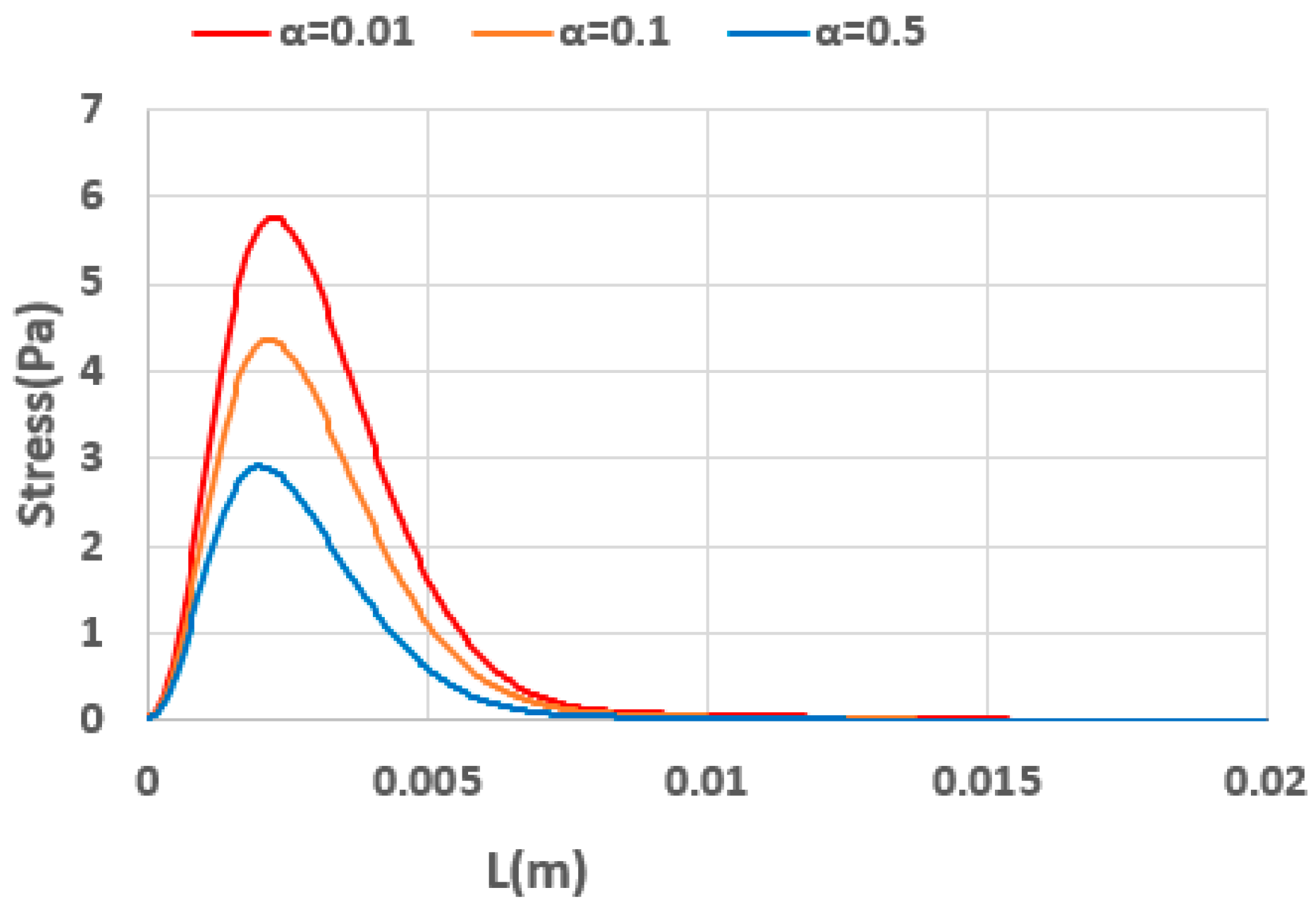
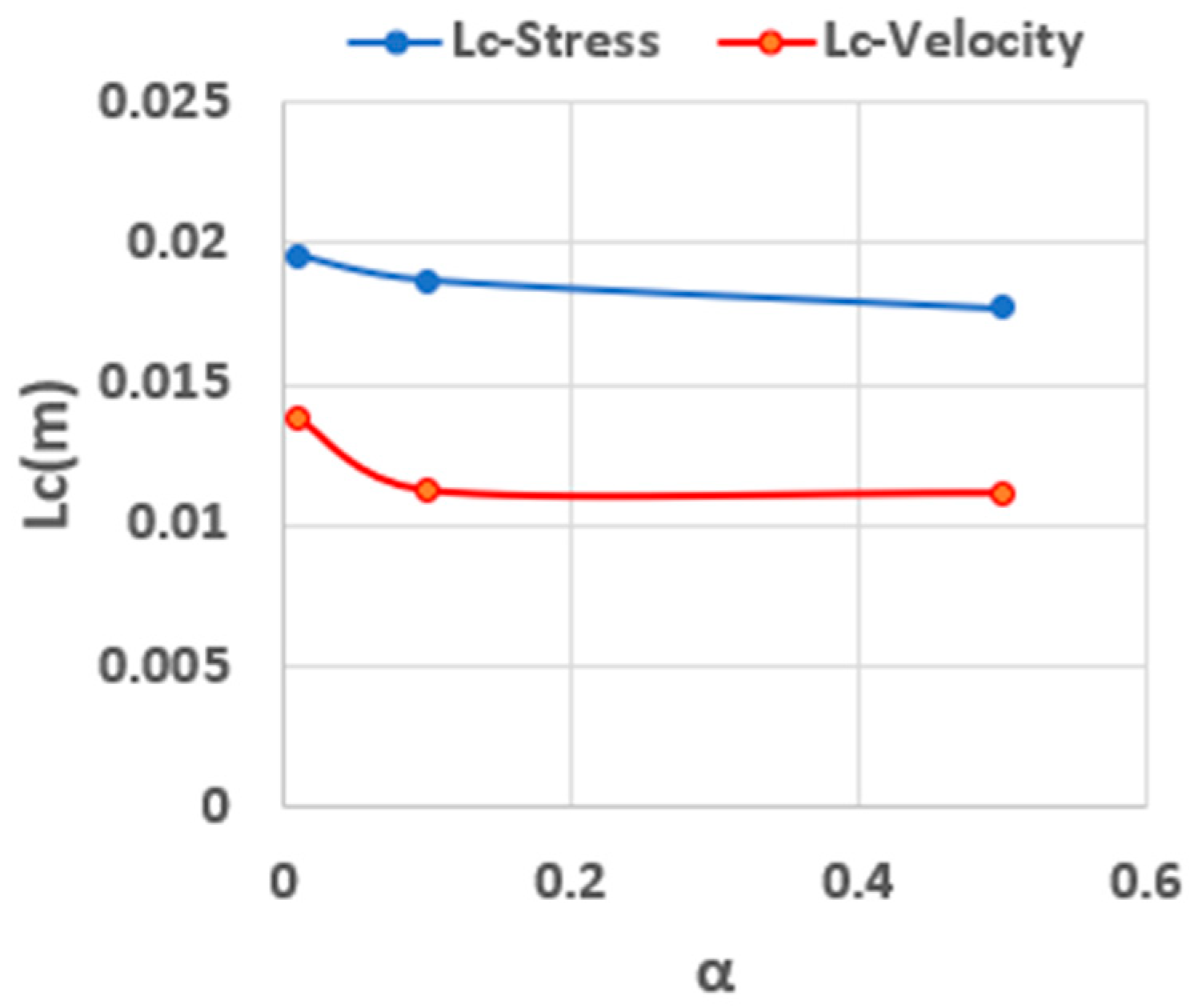
3.4. Influence Shear Thinning Behavior
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Grotberg, J.B. Biofluid Mechanics: Analysis and Applications; Cambridge University Press: Cambridge, UK, 2021. [Google Scholar]
- Clark, A.R.; Lin, M.; Tawhai, M.; Saghian, R.; James, J.L. Multiscale modelling of the feto–placental vasculature. Interface Focus 2015, 5, 20140078. [Google Scholar] [CrossRef] [PubMed]
- Li, Z.; Haward, S.J. Viscoelastic flow development in planar microchannels. Microfluid. Nanofluid. 2015, 19, 1123–1137. [Google Scholar] [CrossRef]
- Kim, B.; Park, S. Study on in-nozzle flow and spray behavior characteristics under various needle positions and length-to-width ratios of nozzle orifice using a transparent acrylic nozzle. Int. J. Heat Mass Transf. 2019, 143, 118478. [Google Scholar] [CrossRef]
- Hatzikiriakos, S.G.; Dealy, J.M. Start-up pressure transients in a capillary rheometer. Polym. Eng. Sci. 1994, 34, 493–499. [Google Scholar] [CrossRef]
- Wang, S.; Gao, D.; Wester, A.; Beaver, K.; Wyke, K. Analytical and computational modeling of relaxation times for non-Newtonian fluids. Fluids 2024, 9, 165. [Google Scholar] [CrossRef]
- Durst, F.; Ray, S.; Ünsal, B.; Bayoumi, O.A. The development lengths of laminar pipe and channel flows. ASME J. Fluids Eng. 2005, 127, 1154–1160. [Google Scholar] [CrossRef]
- Mohanty, A.K.; Asthana, S.B.L. Laminar flow in the entrance region of a smooth pipe. J. Fluid Mech. 1979, 90, 433–447. [Google Scholar] [CrossRef]
- Poole, R.J.; Ridley, B.S. Development-length requirements for fully developed laminar pipe flow of inelastic non-Newtonian liquids. ASME J. Fluids Eng. 2007, 129, 1281–1287. [Google Scholar] [CrossRef]
- Lambride, C.; Syrakos, A.; Georgiou, G.C. Entrance length estimates for flows of power-law fluids in pipes and channels. J. Non-Newton. Fluid Mech. 2023, 317, 105056. [Google Scholar] [CrossRef]
- Rehman, S.; Chebbi, R. Power-law fluid flow in the entrance region between two parallel plates. Results Eng. 2024, 24, 103066. [Google Scholar] [CrossRef]
- Ookawara, S.; Ogawa, K.; Dombrowski, N.; Amooie-Foumeny, E.; Riza, A. Unified entry length correlation for Newtonian, power law and Bingham fluids in laminar pipe flow at low Reynolds number. J. Chem. Eng. Japan. 2000, 33, 675–678. [Google Scholar] [CrossRef]
- Fernandes, C.; Ferrás, L.L.; Araujo, M.S.; Nóbrega, J.M. Development length in planar channel flows of inelastic non-Newtonian fluids. J. Non-Newton. Fluid Mech. 2018, 255, 13–18. [Google Scholar] [CrossRef]
- Syrakos, A.; Gryparis, E.; Georgiou, G.C. A revisit of the development of viscoplastic flow in pipes and channels. arXiv 2024, arXiv:2409.00842. [Google Scholar] [CrossRef]
- Soto, R.J.; Shah, V.L. Entrance flow of a yield-power law fluid. Appl. Sci. Res. 1976, 32, 73–85. [Google Scholar] [CrossRef]
- Poole, R.J.; Chhabra, R.P. Development length requirements for fully developed laminar pipe flow of yield stress fluids. J. Fluids Eng. 2010, 132, 034501. [Google Scholar] [CrossRef]
- Philippou, M.; Kountouriotis, Z.; Georgiou, G.C. Viscoplastic flow development in tubes and channels with wall slip. J. Non-Newton. Fluid Mech. 2016, 234, 69–81. [Google Scholar] [CrossRef]
- Poole, R.J. Inelastic and flow-type parameter models for non-Newtonian fluids. J. Non-Newton. Fluid Mech. 2023, 320, 105106. [Google Scholar] [CrossRef]
- Na, Y.; Yoo, J.Y. A finite volume technique to simulate the flow of a viscoelastic fluid. Comput. Mech. 1991, 8, 43–55. [Google Scholar] [CrossRef]
- Missirlis, K.A.; Assimacopoulos, D.; Mitsoulis, E. A finite volume approach in the simulation of viscoelastic expansion flows. J. Non-Newton. Fluid Mech. 1998, 78, 91–118. [Google Scholar] [CrossRef]
- Al Moatssime, H.; Esselaoui, D.; Hakim, A.; Raghay, S. Finite volume multigrid method of the planar contraction flow of a viscoelastic fluid. Int. J. Numer. Methods Fluids 2001, 36, 885–902. [Google Scholar] [CrossRef]
- Liang, J.Z. Determination of the entry region length of viscoelastic fluid flow in a channel. Chem. Eng. Sci. 1998, 53, 3185–3187. [Google Scholar] [CrossRef]
- Alves, M.A.; Oliveira, P.J.; Pinho, F.T. Benchmark solutions for the flow of Oldroyd-B and PTT fluids in planar contractions. J. Non-Newton. Fluid Mech. 2003, 110, 45–75. [Google Scholar] [CrossRef]
- Yapici, K.; Karasozen, B.; Uludag, Y. Numerical analysis of viscoelastic fluids in steady pressure-driven channel flow. J. Fluids Eng. 2012, 134, 051206. [Google Scholar] [CrossRef]
- Teixeira, D.F.M. Determination of the Entrance Length for Viscoelastic Fluid Flows in Microchannels. Master’s Thesis, Universidade do Porto, Porto, Portugal, 2022. [Google Scholar]
- Bertoco, J.; Leiva, R.T.; Ferrás, L.L.; Afonso, A.M.; Castelo, A. Development length of fluids modelled by the gptt constitutive differential equation. Appl. Sci. 2021, 11, 10352. [Google Scholar] [CrossRef]
- Pouraria, H.; Gao, D.; Wang, S. Numerical investigation of developing flow of viscoelastic fluids in a tube. In ASTFE Digital Library; Begel House Inc.: Danbury, CT, USA, 2025. [Google Scholar] [CrossRef]
- Alves, M.A.; Oliveira, P.J.; Pinho, F.T. Numerical methods for viscoelastic fluid flows. Annu. Rev. Fluid Mech. 2021, 53, 509–541. [Google Scholar] [CrossRef]
- Giesekus, H. A simple constitutive equation for polymer fluids based on the concept of deformation-dependent tensorial mobility. J. Non-Newton. Fluid Mech. 1982, 11, 69–109. [Google Scholar] [CrossRef]
- Bird, R.B.; Armstrong, R.C.; Hassager, O. Dynamics of Polymeric Liquids, Volume 1: Fluid Mechanics; Wiley: Hoboken, NJ, USA, 1987. [Google Scholar]
- Fattal, R.; Kupferman, R. Time-dependent simulation of viscoelastic flows at high Weissenberg number using the log-conformation representation. J. Non-Newton. Fluid Mech. 2005, 126, 23–37. [Google Scholar] [CrossRef]
- Fernandes, C.; Araujo, M.S.B.; Ferrás, L.L.; Nóbrega, J.M. Improved both sides diffusion (iBSD): A new and straightforward stabilization approach for viscoelastic fluid flows. J. Non-Newton. Fluid Mech. 2017, 249, 63–78. [Google Scholar] [CrossRef]
- Flow Science, Inc. FLOW-3D. Version 2023R2. Flow Science, Inc.: Pasadena, CA, USA, 2023. Available online: https://www.flow3d.com/ (accessed on 14 May 2025).
- Dunstan, D.E. The viscosity-radius relationship for concentrated polymer solutions. Sci. Rep. 2019, 9, 543. [Google Scholar] [CrossRef]
- Ananyev, D.V.; Halitova, G.R.; Vachagina, E.K. Hydrodynamics and heat transfer in a laminar flow of viscoelastic fluid in a flat slot channel. Thermo-Phys. Aeromechanics 2015, 22, 49–60. [Google Scholar] [CrossRef]
- Litvinov, S.; Hu, X.; Ellero, M.; Adams, N. Mesoscopic simulation of the transient behavior of semi-diluted polymer solution in a microchannel following extensional flow. Microfluid. Nanofluid. 2014, 16, 257–264. [Google Scholar] [CrossRef]
- Kleßinger, U.A.; Wunderlich, B.K.; Bausch, A.R. Transient flow behavior of complex fluids in microfluidic channels. Microfluid. Nanofluid. 2013, 15, 533–540. [Google Scholar] [CrossRef][Green Version]
- Casanellas, L.; Alves, M.A.; Poole, R.J.; Lerouge, S.; Lindner, A. The stabilizing effect of shear thinning on the onset of purely elastic instabilities in serpentine microflows. Soft Matter 2016, 12, 6167–6175. [Google Scholar] [CrossRef]
- Maklad, O.; Poole, R.J. A review of the second normal-stress difference; its importance in various flows, measurement techniques, results for various complex fluids and theoretical predictions. J. Non-Newton. Fluid Mech. 2021, 292, 104522. [Google Scholar] [CrossRef]
- Li, Y.K.; Zheng, Z.Y.; Zhang, H.N.; Li, F.C.; Qian, S.; Joo, S.W.; Kulagina, L.V. Numerical study on secondary flows of viscoelastic fluids in straight ducts: Origin analysis and parametric effects. Comput. Fluids 2017, 152, 57–73. [Google Scholar] [CrossRef]
- Pouraria, H.; Foudazi, R.; Houston, J.P. Exploitation of elasto-inertial fluid flow for the separation of nano-sized particles: Simulating the isolation of extracellular vesicles. Cytom. Part A 2023, 103, 786–795. [Google Scholar] [CrossRef]
- Wang, P.; Yu, Z.; Lin, J. Numerical simulations of particle migration in rectangular channel flow of Giesekus viscoelastic fluids. J. Non-Newton. Fluid Mech. 2018, 262, 142–148. [Google Scholar] [CrossRef]












Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pouraria, H.; Gao, D.; Wang, S. Computational Analysis of Entry-Region Flow Dynamics for Giesekus Fluids in Tubes. Processes 2025, 13, 1587. https://doi.org/10.3390/pr13051587
Pouraria H, Gao D, Wang S. Computational Analysis of Entry-Region Flow Dynamics for Giesekus Fluids in Tubes. Processes. 2025; 13(5):1587. https://doi.org/10.3390/pr13051587
Chicago/Turabian StylePouraria, Hassan, Dalong Gao, and Sheldon Wang. 2025. "Computational Analysis of Entry-Region Flow Dynamics for Giesekus Fluids in Tubes" Processes 13, no. 5: 1587. https://doi.org/10.3390/pr13051587
APA StylePouraria, H., Gao, D., & Wang, S. (2025). Computational Analysis of Entry-Region Flow Dynamics for Giesekus Fluids in Tubes. Processes, 13(5), 1587. https://doi.org/10.3390/pr13051587






