Optimized Economic Evaluation Model for Intensive Drilling in Unconventional Oil and Gas Development
Abstract
1. Introduction
2. Materials and Methods
2.1. Model Cost Classification
2.2. Quantification of the Economic Benefit Components for Intensive Drilling Design
2.2.1. Pre-Drilling Costs
- Land Acquisition Costs
- Well Construction Costs
- Equipment Installation and Removal Costs
2.2.2. Drilling Costs
- Drilling Operation Costs
- Drilling Material Costs
- Drilling Fluid Environmentally Safe Treatment Costs
2.2.3. Drilling Engineering Costs
2.2.4. Optimized Economic Evaluation Model
- Combined Cost Function
- Pre-Drilling Cost with Synergy Effect
- Drilling Operation Cost with Synergy Effect
- Material Cost with Synergy Effect
- Drilling Fluid Treatment Cost
- Final Integrated Equation
- Decision Timeliness Optimization
3. Results and Discussions
3.1. Validation of the Established Model
3.2. The Impact of Platform Scale on Drilling Investment
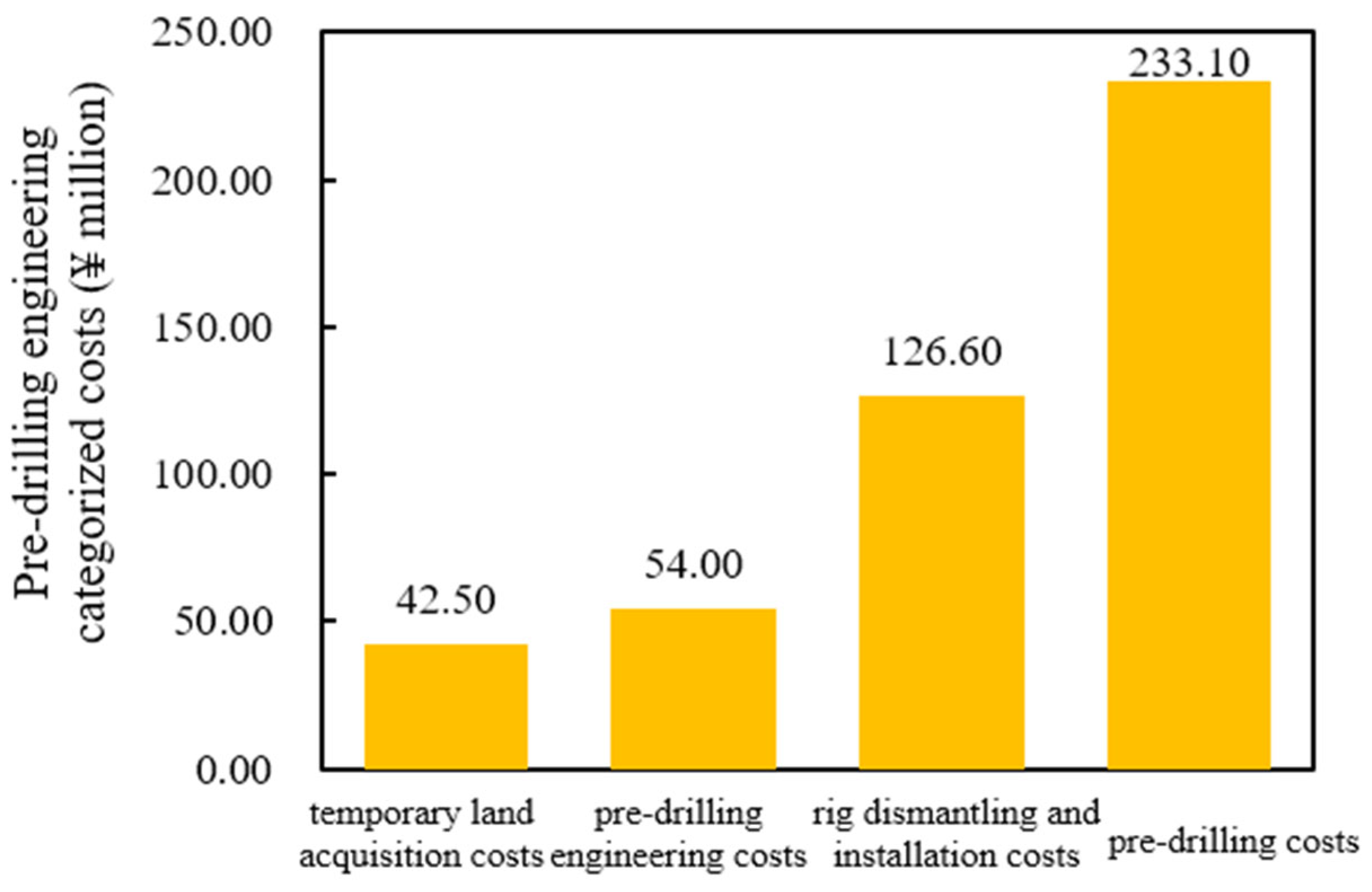
3.2.1. Temporary Land Acquisition Costs
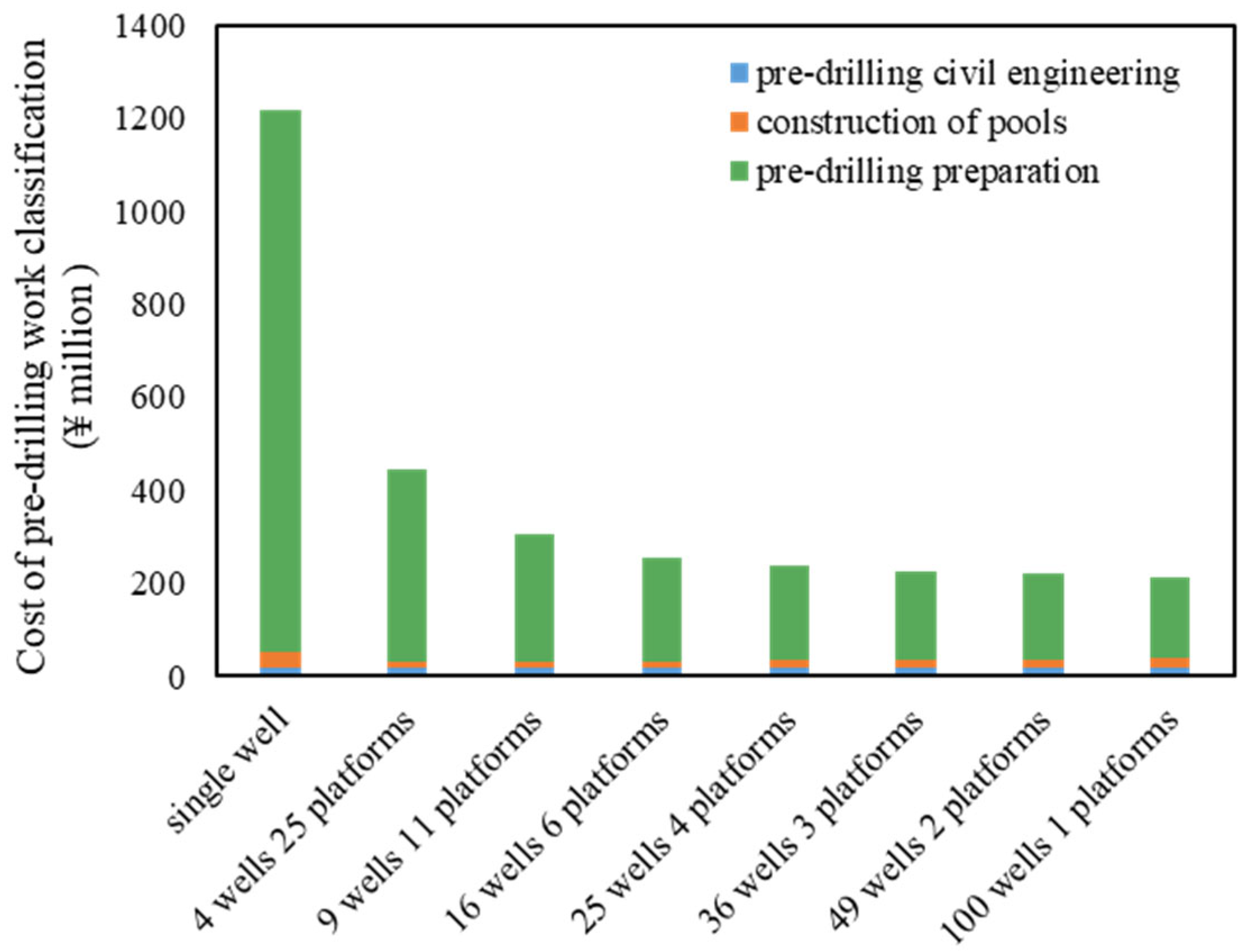
3.2.2. Pre-Drilling Engineering
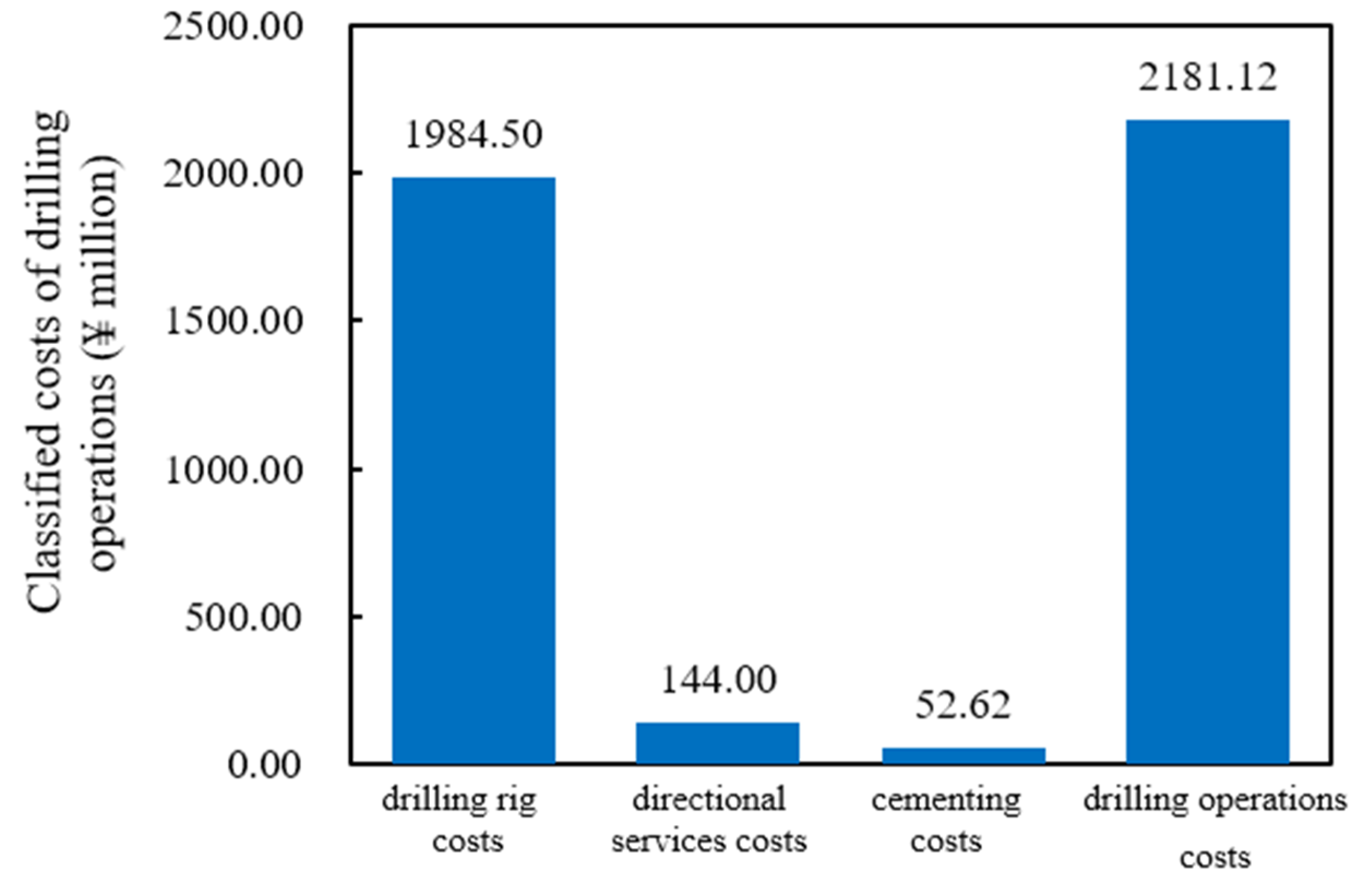
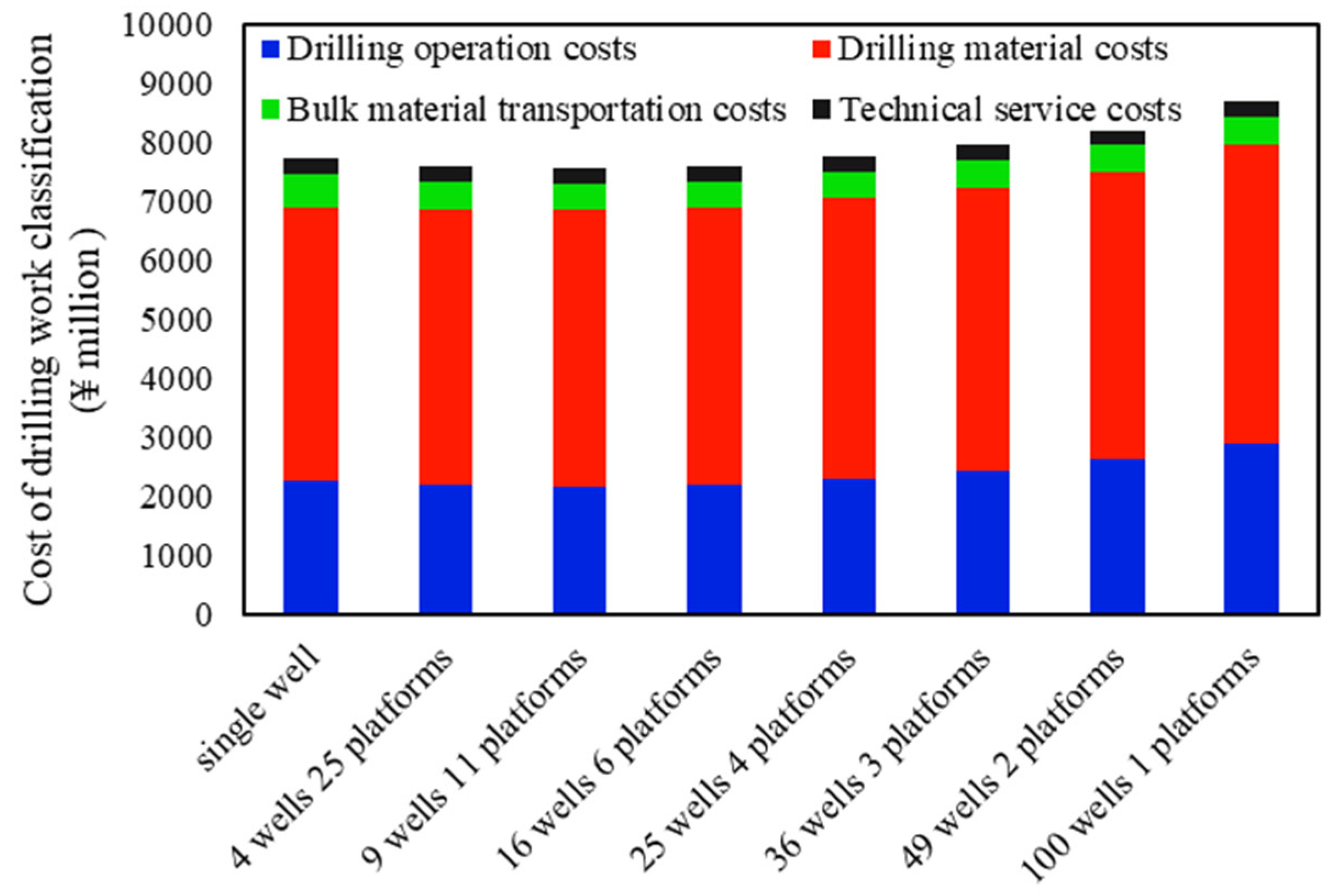
3.2.3. Drilling Engineering
3.2.4. Cementing Engineering
3.2.5. Drilling Fluid Waste Treatment
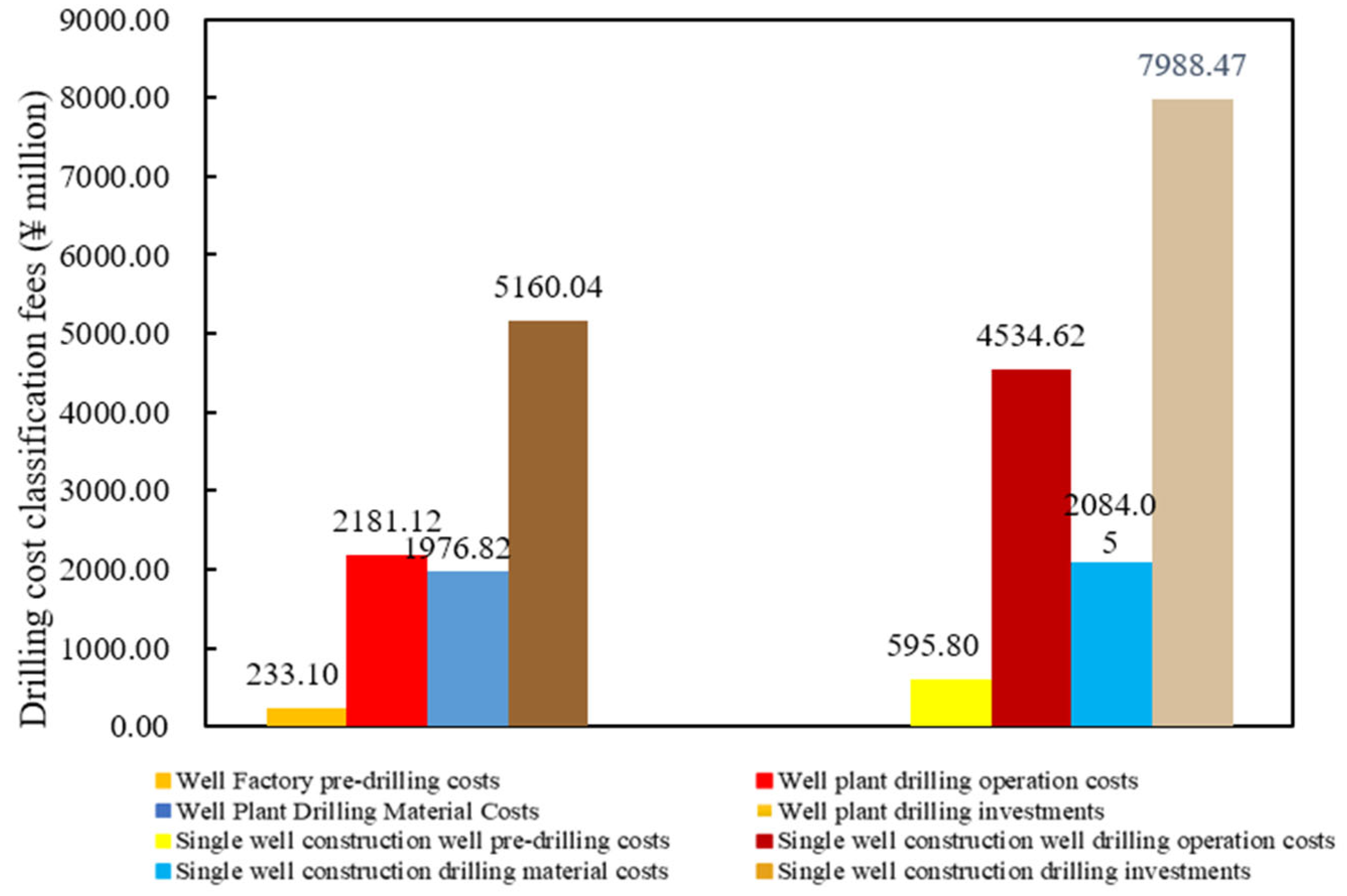
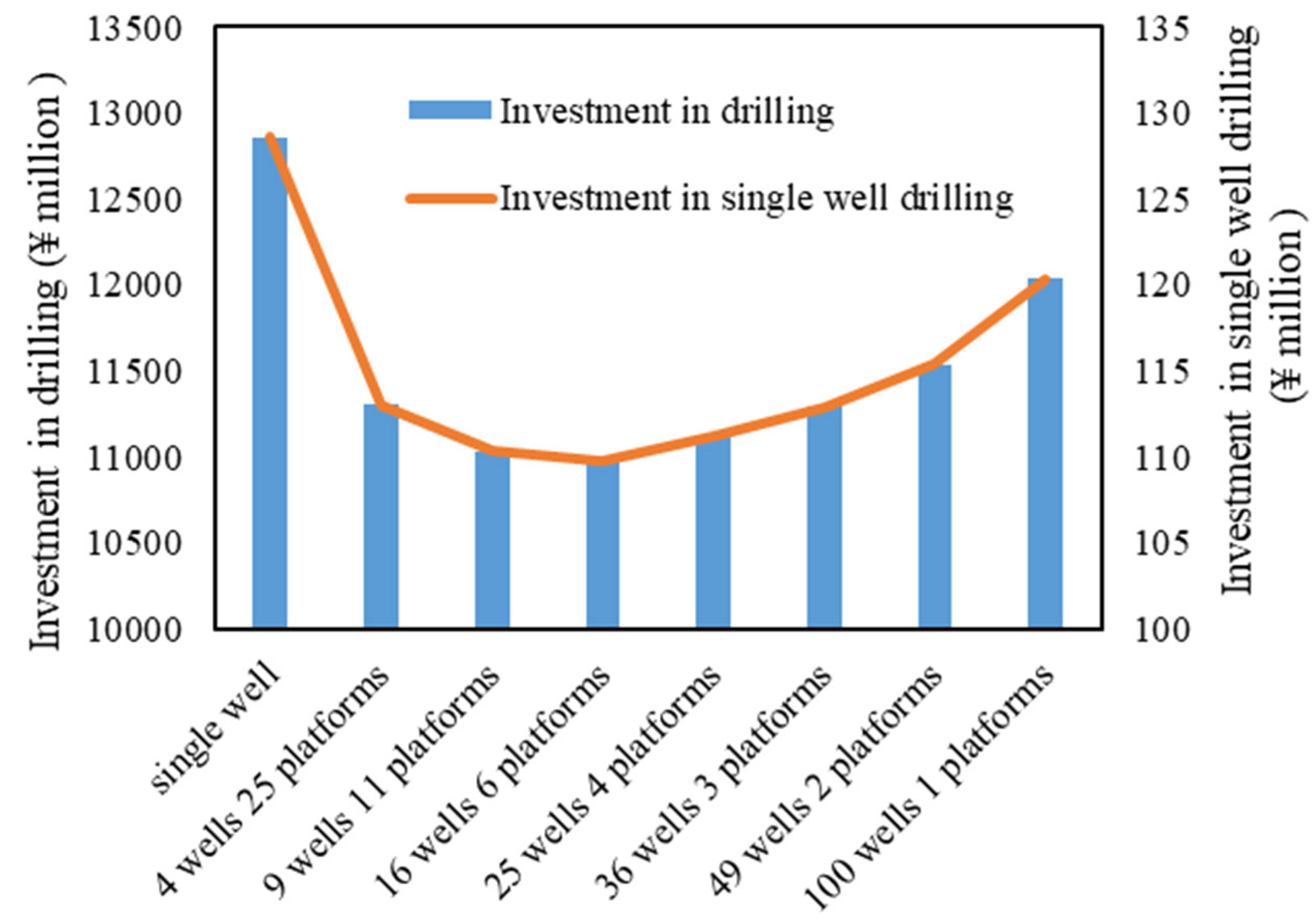
3.2.6. Total Drilling Investment and Drilling Investment per Well
4. Conclusions
- (1)
- The optimized model incorporates synergy factors—Φpre, Φdrill, and Φmat—to capture the cost-saving effects of intensive drilling, such as reduced land acquisition costs, optimized rig sharing, and enhanced reuse of drilling fluids. Moreover, by explicitly incorporating decision response time into the objective function, the model can be dynamically adjusted to meet varying requirements in the balance between cost minimization and decision efficiency, thus fulfilling the high timeliness demands of economic evaluation methods in unconventional oil and gas development.
- (2)
- Compared with the conventional single-well drilling approach, the “well factory” operation mode significantly reduces drilling costs. Notably, there are substantial reductions in both pre-drilling costs and drilling operation costs. Within the pre-drilling category, costs associated with temporary land acquisition and pre-drilling engineering are considerably lower, while in the drilling operations category, rig costs are dramatically reduced.
- (3)
- The primary advantage of the intensive well construction method lies in its ability to save considerable land expenses and lower rig mobilization and installation costs, thereby reducing the overall investment. However, as well depth increases, the cost of drilling materials rises. When the number of wells per platform reaches a certain threshold, the incremental increase in drilling investment due to greater well depths can outweigh the savings in land and rig-related expenses, causing overall costs to rise gradually. Therefore, it is essential to analyze and determine an optimal platform scale.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
- 1.
- Pre-Drilling Cost Block (Equations (1)–(4))
- 2.
- Drilling Cost Block (Equations (5)–(12))
- 3.
- Drilling-Engineering Aggregates (Equations (13) and (14))
- 4.
- Synergistic and Optimization Model (Equations (15)–(24))
- -
- Equation (16) scales pre-drilling by Φpre (from Equation (17)).
- -
- Equation (18) scales drilling ops by Φdrill (from Equation (19)).
- -
- Equation (20) scales materials by Φmat (from Equation (21)).
- -
- Equation (22) retains Equation (10)’s treatment cost (synergy minor).
- 5.
- Decision-Timeliness Objective
Appendix B
| Symbol | Unit | Description |
|---|---|---|
| Cpre | Yuan | Total pre-drilling cost (land + site + mobilization) across all platforms |
| Czji | Yuan | Rig operation cost for platform i (daily rig + labor rate × drilling days) |
| Cdxi | Yuan | Directional drilling service cost (daily directional rate × days) |
| Cgji | Yuan | Cementing cost (sum of individual cement job costs) |
| Ccli | Yuan | Drilling material cost (unit cost per meter for bits, fluids, casing, slurry × drilled length) |
| Δq2nj | Yuan | Fluid-reuse savings (fluid unit price × reused volume) |
| Cmci | Yuan | Waste treatment cost (treatment fee per m3 × waste volume) |
| Cdri | Yuan | Platform-level total drilling cost |
| Cdrill | Yuan | Sum of all platform drilling costs |
| Ctotal | Yuan | Synergy-adjusted total cost under factory-mode optimization |
| S1, S2 | m2 | First-well and additional-well pad area |
| q | Yuan/m2 | Land cost per square meter |
| qr1, qr2,qr3 | Yuan/move | Unit costs for major rig move, full relocation, pad-to-pad move |
| Tznj, Tdxnj | Day | Drilling and directional-drilling durations |
| Hnj | m | Depth drilled in phase j of well n |
| Qnj | Yuan/m3 | Drilling fluid unit price |
| ΔVnj | m3 | Volume of fluid reused |
| Bn | Yuan/m3 | Unit treatment cost per cubic meter of waste |
| Vn | m3 | Total waste volume (cuttings + spent fluid) |
| α | – | Coefficient controlling pre-drilling synergy |
| β | – | Coefficient controlling drilling synergies |
| γ | – | Coefficient governing material-reuse efficiency |
| λ | Yuan/day | Weight for decision-timeliness penalty in the objective function |
| Tdecision | Day | Estimated decision-making time |
References
- Chukwunweike, J.N. Leveraging AI and Principal Component Analysis (PCA) For In-Depth Analysis in Drilling Engineering: Optimizing Production Metrics through Well Logs and Reservoir Data. Int. J. Comput. Appl. Technol. Res. 2024, 13, 40–52. [Google Scholar] [CrossRef]
- Hansen, P.; de Luna Pedrosa Filho, E.; Carneiro Ribeiro, C. Location and Sizing of Offshore Platforms for Oil Exploration. Eur. J. Oper. Res. 1992, 58, 202–214. [Google Scholar] [CrossRef]
- Jiang, P.; Li, L.; Fu, Y. Research and Application of Drilling and Completion Technology for Cluster Wells. J. Phys. Conf. Ser. 2024, 2834, 012033. [Google Scholar] [CrossRef]
- Zou, C.; Dong, D.; Wang, Y.; Li, X.; Huang, J.; Wang, S.; Guan, Q.; Zhang, C.; Wang, H.; Liu, H.; et al. Shale Gas in China: Characteristics, Challenges and Prospects (II). Pet. Explor. Dev. 2016, 43, 182–196. [Google Scholar] [CrossRef]
- Dou, Z.; Liu, Y.; Zhang, J.; Xu, X.; Zhang, W.; Zhu, J. Optimization of Well Factory Platform Mode Considering Optimal Allocation of Water Resources. Arab. J. Sci. Eng. 2022, 47, 11159–11170. [Google Scholar] [CrossRef]
- Li, Y.; Zhou, D.H.; Wang, W.H.; Jiang, T.X.; Xue, Z.J. Development of Unconventional Gas and Technologies Adopted in China. Energy Geosci. 2020, 1, 55–68. [Google Scholar] [CrossRef]
- Liang, X.; Shan, C.; Wang, W.; Li, Z.; Zhu, D.; Xu, J.; Zhang, Z.; Zhang, Z.; Luo, Y.; Yuan, X. Exploration and Development in the Zhaotong National Shale Gas Demonstration Area: Progress and Prospects. Nat. Gas Ind. B 2023, 10, 44–61. [Google Scholar] [CrossRef]
- Ertekin, T. The Efficacy and Superiority of the Expert Systems in Reservoir Engineering Decision Making Processes. Appl. Sci. 2021, 11, 6347. [Google Scholar] [CrossRef]
- Hu, Q.; Li, Y.; Wu, N.; Jiang, Y.; Sun, X.; Wang, H.; Bu, Q.; Hu, G. Influences of Stress State on Compressional Wave Velocity of Sandy Hydrate-Bearing Sediment: Experiments and Modeling. Geoenergy Sci. Eng. 2024, 234, 212683. [Google Scholar] [CrossRef]
- Guo, X.; Hu, D.; Shu, Z.; Li, Y.; Zheng, A.; Wei, X.; Ni, K.; Zhao, P.; Cai, J. Exploration, Development, and Construction in the Fuling National Shale Gas Demonstration Area in Chongqing: Progress and Prospects. Nat. Gas Ind. B 2023, 10, 62–72. [Google Scholar] [CrossRef]
- Al-Qahtani, G.D.; Elrafie, E.A.; Abbas, R.T.; Ikuku, C.E.; Hogg, M.F.; Rincon, A. Complex Well Modeling Workflow Enabling Full Field Optimization and Forward Decisions. In Proceedings of the SPE Latin America and Caribbean Petroleum Engineering Conference. SPE, Cartagena, Columbia, 31 May–3 June 2009; Volume 1, pp. 28–40. [Google Scholar] [CrossRef]
- Li, W.; Finsa, M.M.; Laskey, K.B.; Houser, P.; Douglas-Bate, R.; Verner, K. Optimizing Well Placement for Sustainable Irrigation: A Two-Stage Stochastic Mixed Integer Programming Approach. Water 2024, 16, 2715. [Google Scholar] [CrossRef]
- Wang, G.; Zhang, W. Study and Application of Wellbore Trajectory Optimization for Dense Cluster Wells. In E3S Web of Conferences; EDP Sciences: Paris, France, 2021; Volume 261, pp. 1–5. [Google Scholar] [CrossRef]
- Ochulor, O.J.; Onita, F.B. Geosteering in Deep Water Wells: A Theoretical Review of Challenges and Solutions. World J. Eng. Technol. Res. 2024, 3, 046–054. [Google Scholar] [CrossRef]
- Li, S.Q.; Tong, Y.S.; Yan, T.; Zhou, Y.C.; Li, W.; Sun, X.F. Numerical Simulation of Rock Breaking by Resonance Coring Based on Laboratory Experiments. SPE J. 2025, 3, 1162–1176. [Google Scholar] [CrossRef]
- Saleem, M. Application of Machine Learning for Optimizing Oil Well Production and Reservoir Management: A Simulation-Based Approach. J. Biol. Allied Heal. Sci. 2022, 05, 3–13. [Google Scholar] [CrossRef]
- Alpak, F.O. Simultaneous Optimization of Well Count and Placement: Algorithm, Validation, and Field Testing. SPE J. 2023, 28, 147–172. [Google Scholar] [CrossRef]
- LIU, Z. Innovation and Practice of Petroleum Engineering Synergetic Management for the Development of Difficult-to-Produce Reserves. Pet. Explor. Dev. 2020, 47, 1316–1324. [Google Scholar] [CrossRef]
- Gu, Y.; Gao, D.; Yang, J.; Wang, Z.; Li, X.; Tan, L. A Model for Platform Location Optimization in Shale Gas with Learning Effect. In SPE Asia Pacific Oil and Gas Conference and Exhibition 2018; SPE: Richardson, TX, USA. [CrossRef]
- Dbouk, H.M.; Ghorayeb, K.; Kassem, H.; Hayek, H.; Torrens, R.; Wells, O. Facility Placement Layout Optimization. J. Pet. Sci. Eng. 2021, 207, 109079. [Google Scholar] [CrossRef]
- Wang, G.; Huang, W.; Gao, D. Optimization Design Method of “Well Factory” Platform Planning and Wellhead Layout for Oil and Gas Development. SPE J. 2024, 29, 5875–5895. [Google Scholar] [CrossRef]
- Egbumokei, P.I.; Dienagha, I.N.; Digitemie, W.N.; Cynthia, E.; Oladipo, O.T. Strategic Contract Management for Drilling Efficiency and Cost Reduction: Insights and Perspectives. Int. J. Multidiscip. Res. Growth Eval. 2024, 05, 1042–1050. [Google Scholar] [CrossRef]
- Li, Y.; Peng, G.; Du, T.; Jiang, L.; Kong, X.Z. Advancing Fractured Geothermal System Modeling with Artificial Neural Network and Bidirectional Gated Recurrent Unit. Appl. Energy 2024, 372, 123826. [Google Scholar] [CrossRef]
- Qiaobo, H.; Xiaofeng, S.; Jingyu, Q.; Yanlong, L. Creep Behaviors of Methane Hydrate-Bearing Sandy Sediments under Load-to-Unload Stress State. Geoenergy Sci. Eng. 2025, 247, 213702. [Google Scholar] [CrossRef]
- Kohler, M.; Vellasco, M.; Silva, E.; Figueiredo, K. SimProxy Decision Support System: A Neural Network Proxy Applied to Reservoir and Surface Integrated Optimization. IEEE Syst. J. 2020, 14, 5111–5120. [Google Scholar] [CrossRef]
- Li, Y.; Peng, G.; Tang, J.; Zhang, J.; Zhao, W.; Liu, B.; Pan, Y. Thermo-Hydro-Mechanical Coupling Simulation for Fracture Propagation in CO2 Fracturing Based on Phase-Field Model. Energy 2023, 284, 128629. [Google Scholar] [CrossRef]
- Gao, D. Some Research Advances in Well Engineering Technology for Unconventional Hydrocarbon. Nat. Gas Ind. B 2022, 9, 41–50. [Google Scholar] [CrossRef]
- Hummes, O.; Bond, P.; Jones, A.; Symons, W.; Bishop, M.; Serdy, A.; Pokrovsky, S.; Polito, N. Using Advanced Drilling Technology to Enable Well Factory Concept in the Marcellus Shale. In SPE/IADC Drilling Conference and Exhibition; SPE: Richardson, TX, USA, 2012; Volume 2, pp. 1089–1105. [Google Scholar] [CrossRef]
- Ranjith, R.; Suhag, A.; Balaji, K.; Putra, D.; Dhannoon, D.; Saracoglu, O.; Hendroyono, A.; Temizel, C.; Aminzadeh, F. Production Optimization through Utilization of Smart Wells in Intelligent Fields. In Proceedings of the SPE Western Regional Meeting, Bakersfield, CA, USA, 23–27 April 2017; pp. 1284–1312. [Google Scholar] [CrossRef]
- Gamal, H.; Albahri, S.; Essa, H.; Srivastava, M.; Alghamdi, N.; Shaikh, W. Driving Operational Excellence: Cost Savings and Performance Optimization Through Tripping and Connection Time Optimization. In Proceedings of the International Petroleum Technology Conference, Damman, Saudi Arabia, 12–14 February 2024. [Google Scholar] [CrossRef]
- Karacali, O.; Ramcharan, N.; Sahadeo, L.; Holub, C.; Julien, J.; Sunarto, H.; Tong, S. Pushing the Limits in Deep Water Data Acquisition for Accelerated Field Development: Industry Record Batch Well Testing. In Proceedings of the Offshore Technology Conference, OTC, Houston, TX, USA, 1–4 May 2023. [Google Scholar] [CrossRef]
- SY/T 5466-2013; Well Site Preparation and Layout Requirements. National Energy Administration: Beijing, China, 2013.
- SY/T 5505-2006; Cluster Well Platform Layout. National Energy Administration: Beijing, China, 2006.
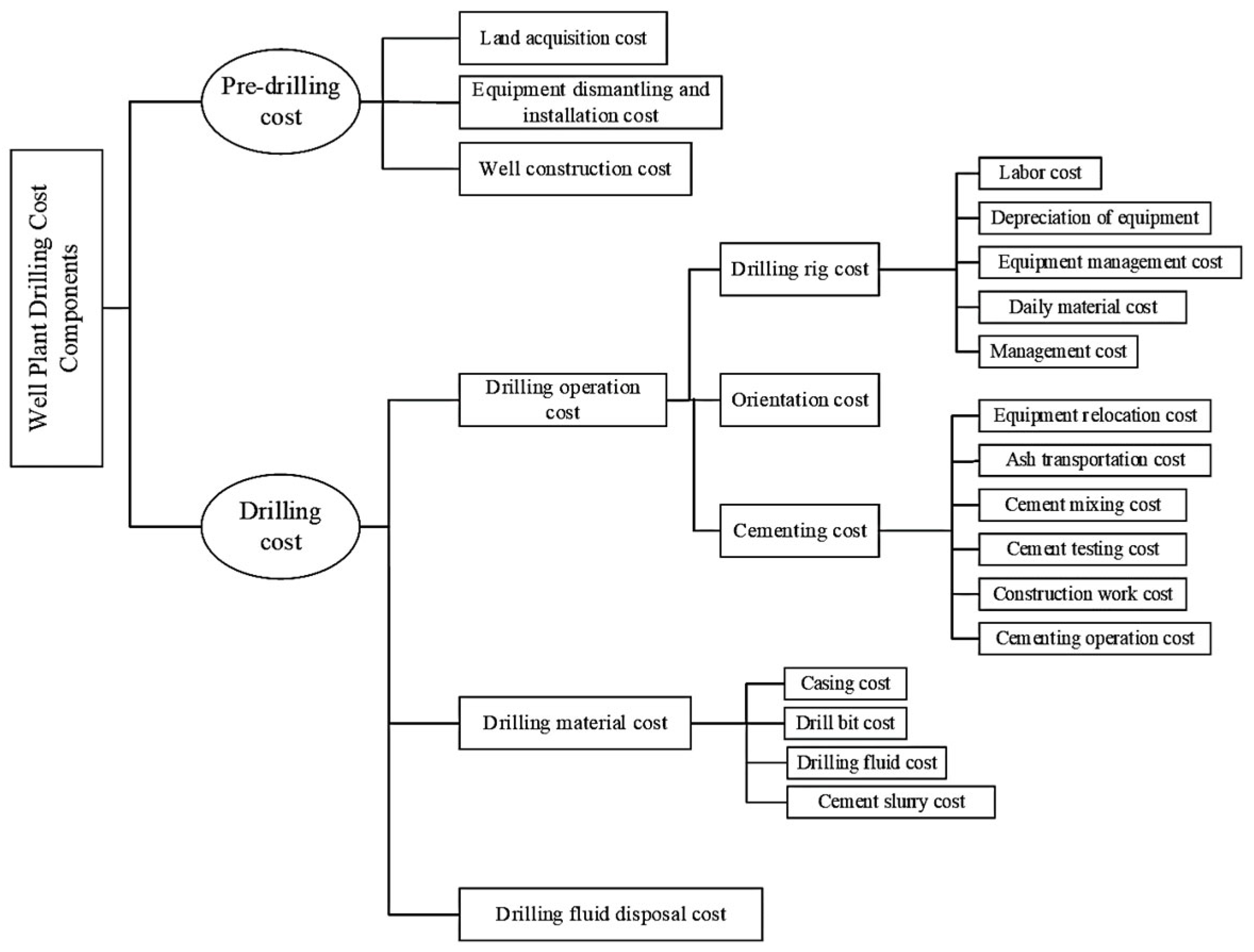





| Category | Sub-Component | Description |
|---|---|---|
| Pre-drilling cost | Land acquisition cost | Temporary land occupation cost for platform I (first-well area + additional well area) |
| Well-site construction cost | Site preparation quota per well, with 10% surcharge for each extra well | |
| Equipment installation/removal cost | Rig mobilization and demobilization (major moves, full relocations, pad-to-pad moves) | |
| Drilling operation cost | Rig operation cost | Daily rig + labor cost × drilling cycle days (including depreciation, management, and materials) |
| Directional drilling service cost | Daily directional service rate × days of directional drilling | |
| Cementing operation cost | Rig mobilization and cost per cement job (equipment, transport, mixing, curing) | |
| Drilling material cost | Drill bit cost | Unit cost per meter of drilling bit used |
| Drilling fluid cost | Fluid unit price × volume used (net of reuse savings) | |
| Casing cost | Unit cost per meter of casing string | |
| Cement slurry cost | Unit cost per meter of cement slurry | |
| Drilling fluid disposal cost | Environmentally safe treatment cost | Treatment fee per m3 × waste volume (water- and oil-based cuttings and fluids) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, S.; Wang, K.; Dong, H.; Tai, Y.; Chen, Z.; Zhou, D.; Sun, S. Optimized Economic Evaluation Model for Intensive Drilling in Unconventional Oil and Gas Development. Processes 2025, 13, 1579. https://doi.org/10.3390/pr13051579
Yu S, Wang K, Dong H, Tai Y, Chen Z, Zhou D, Sun S. Optimized Economic Evaluation Model for Intensive Drilling in Unconventional Oil and Gas Development. Processes. 2025; 13(5):1579. https://doi.org/10.3390/pr13051579
Chicago/Turabian StyleYu, Shaoqing, Kai Wang, Huimin Dong, Yuehua Tai, Zezhou Chen, Dongmei Zhou, and Shihui Sun. 2025. "Optimized Economic Evaluation Model for Intensive Drilling in Unconventional Oil and Gas Development" Processes 13, no. 5: 1579. https://doi.org/10.3390/pr13051579
APA StyleYu, S., Wang, K., Dong, H., Tai, Y., Chen, Z., Zhou, D., & Sun, S. (2025). Optimized Economic Evaluation Model for Intensive Drilling in Unconventional Oil and Gas Development. Processes, 13(5), 1579. https://doi.org/10.3390/pr13051579






