2.1. Theoretical Basis
In pneumatic conveying, the state of particles is categorized into translational and rotational states, both of which adhere to Newton’s second law of motion. For any particle
i, its state can be expressed mathematically, as shown in Equation (1).
In the formula, mi, vi, FDi, FSi, FMi, ki, Fij, Ii, ωi, and Mij are the mass, velocity, drag force, Saffman lift, Magnus lift, collision frequency, resultant force, moment of inertia, angular velocity, and resultant torque of the particles , respectively, and g is the acceleration due to gravity.
In the pneumatic transportation of solid particles, variations in parameters such as the gas velocity, temperature, and pressure are generally minor. As a result, the gas-phase control equation can be accurately represented using only the mass conservation equation and the momentum conservation equation, while the energy conservation equation can be disregarded. However, to better simulate real-world conditions and enhance the precision and reliability of theoretical analyses, it is often necessary to modify and adapt the gas-phase control equations. Consequently, refining these equations is a crucial step in practical applications.
The gas mass conservation equation ensures that the increase in the mass of a gas unit over a given time is equal to the mass inflow during the same period. This relationship is described mathematically in Equation (2).
In the formula, is the gas density, is the gas volume fraction, is the gas flow velocity, and is the Hamiltonian factor.
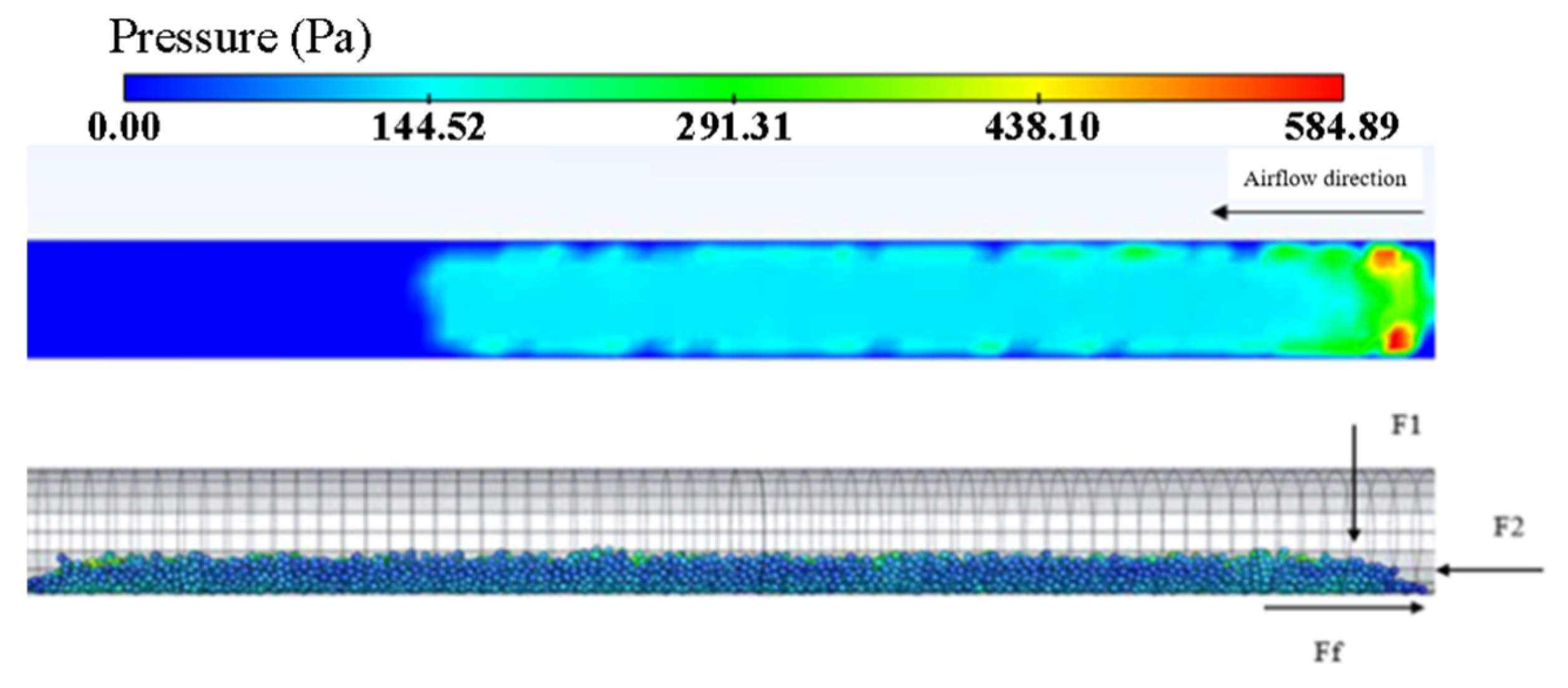
The momentum conservation equation for gas describes the principle of momentum conservation during gas movement. It states that the change in momentum of a gas over time equals the sum of all the external forces acting upon it. When using the Euler–Lagrange bilateral coupling approach, the momentum conservation equation takes the form shown in Equation (3).
In the formula,
is the fluid unit pressure
is the air flow tension, and
S is the interaction force between the gas and solid phases inside the unit.
In the formula, is the volume of the gas unit cell, , , , , , and are the drag force, Saffman lift force, Magnus lift force, Basset force, pressure gradient force, and viscous force exerted on the particles, respectively.
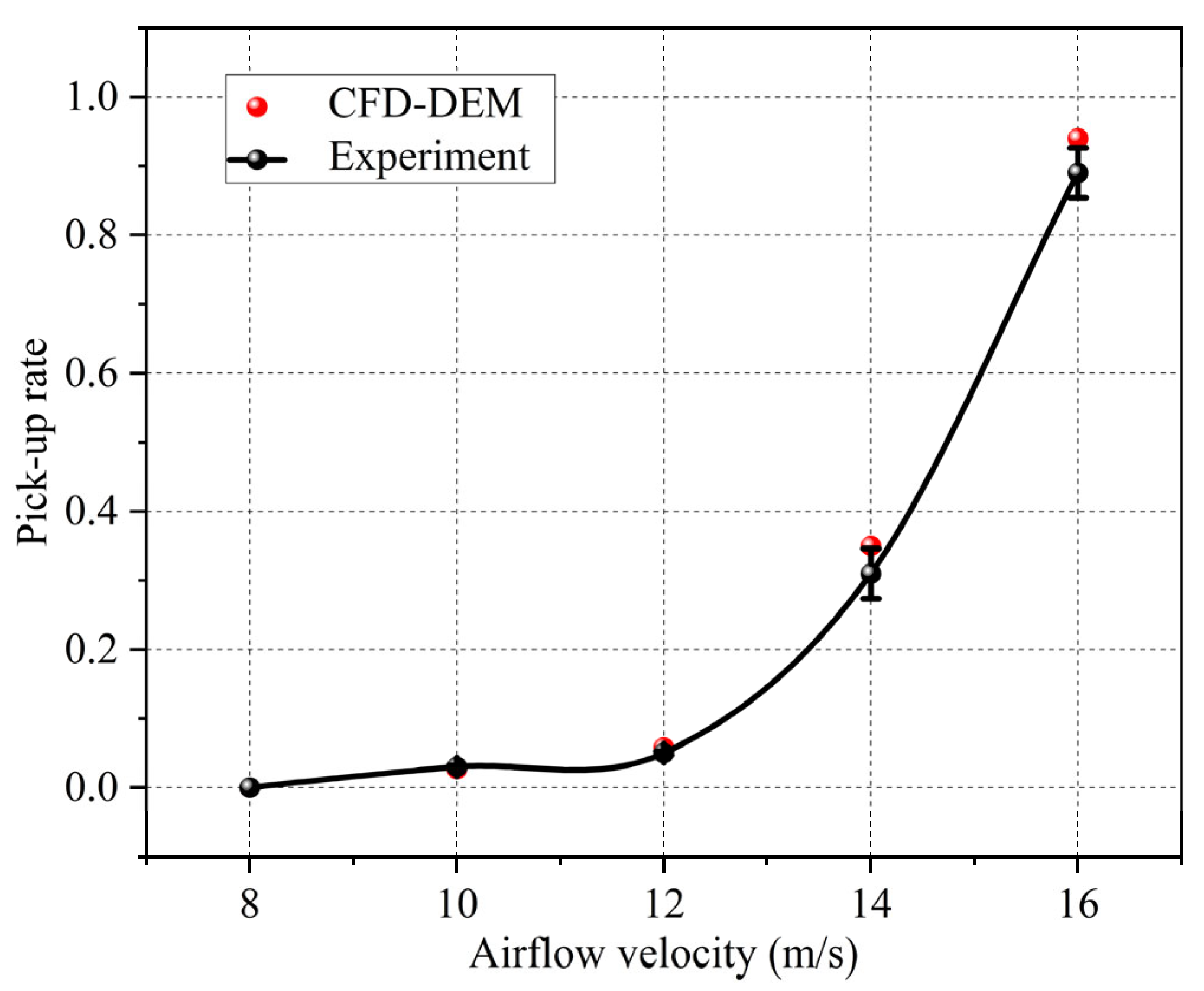
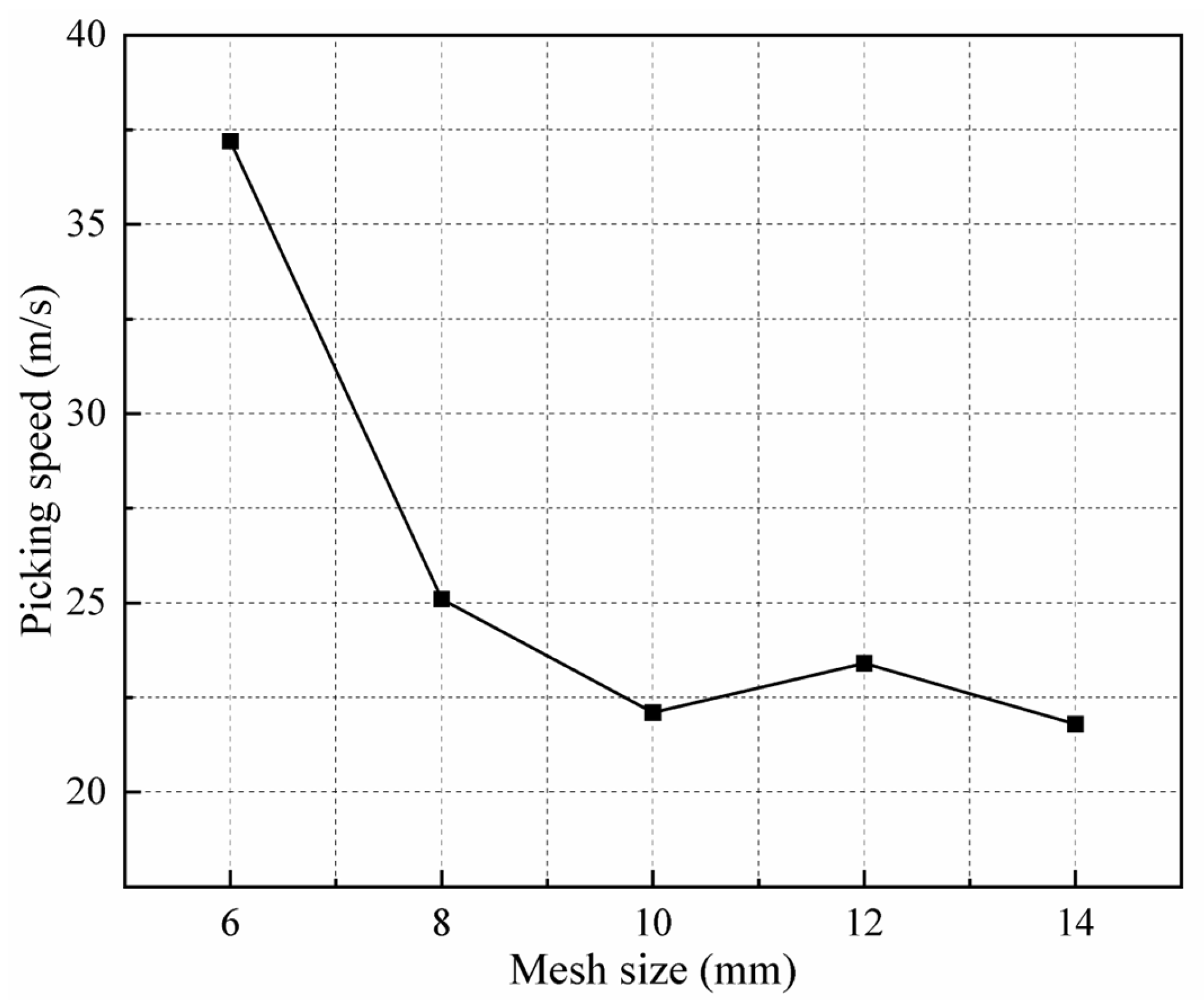
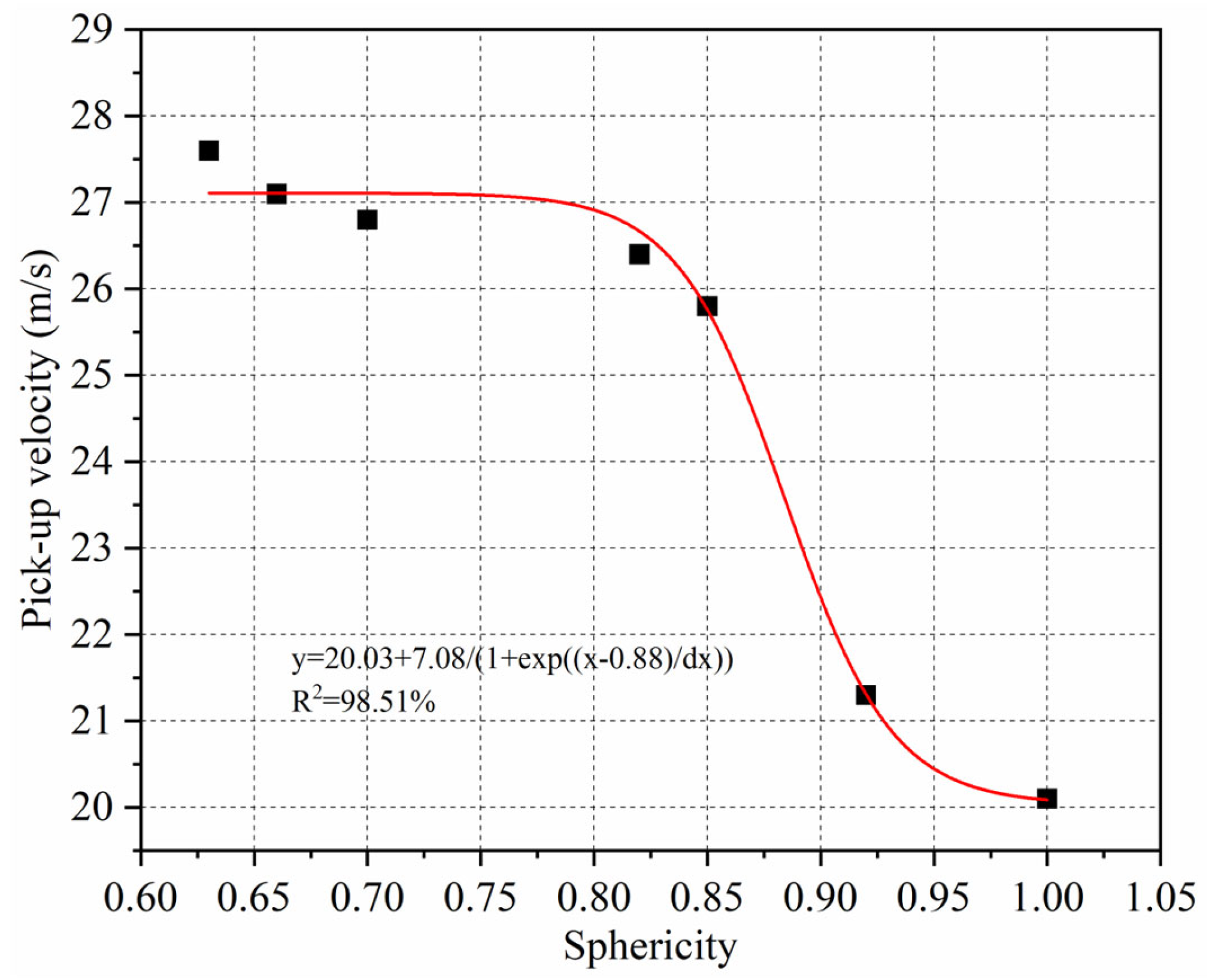
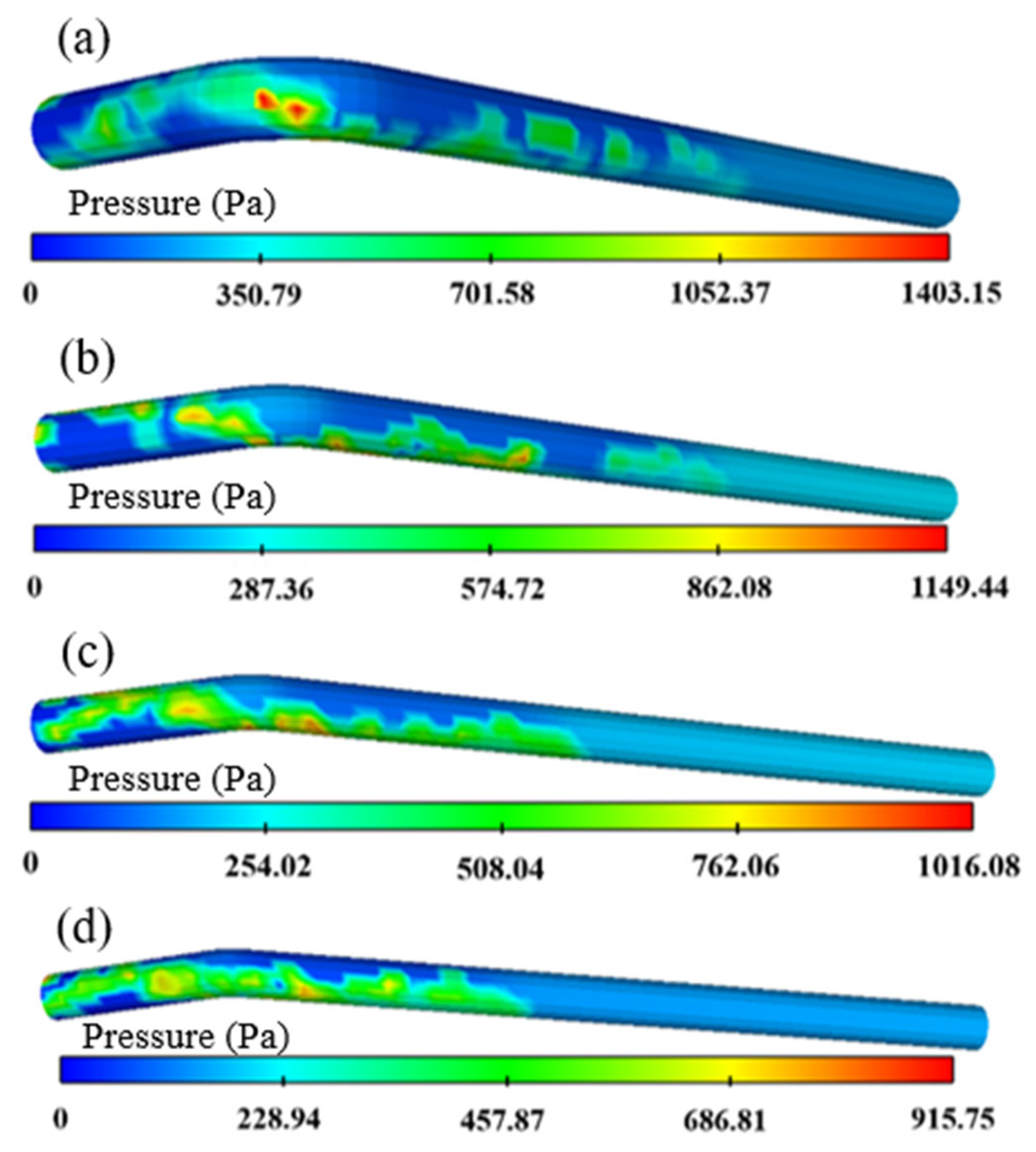
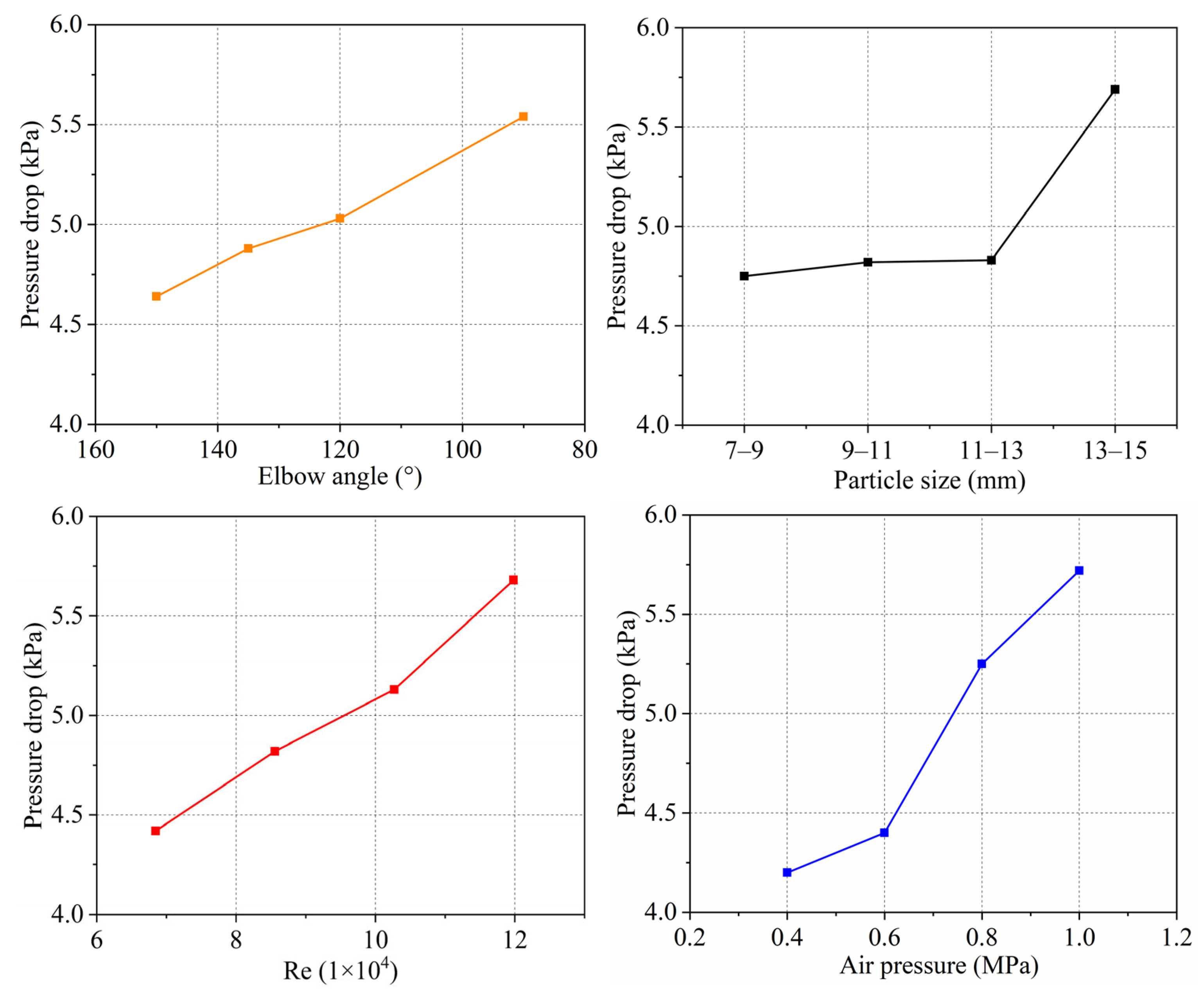
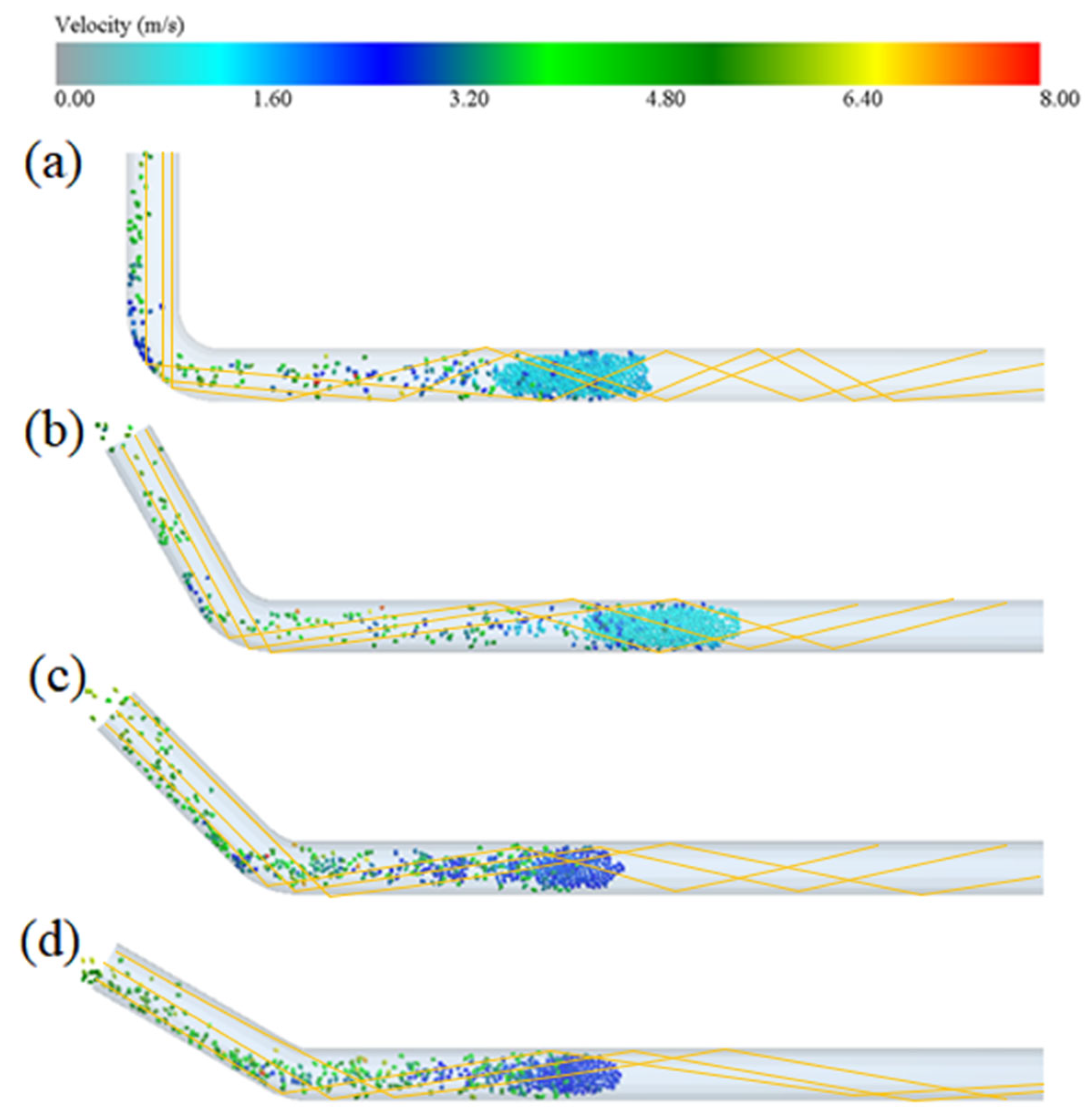
In pneumatic conveying, the airflow velocity significantly influences particle motion. The critical velocity, often referred to as the “picking speed”, is a key metric for assessing the system’s economic efficiency. It is defined as the minimum airflow velocity required for particles to lift and transition from sedimentation to suspension. Studies have shown that factors such as the particle density, particle size, flow field conditions, and pipeline geometry can alter the picking speed.
Kalman et al. investigated various Geldart particles, classifying them into three types based on particle size. They characterized the picking speed of particles with differing materials using modified Reynolds and Archimedes numbers [
26,
27,
28]. For particles of varying sizes, the corrected particle Reynolds number is described in Equation (5).
In the formula,
is the modified particle Reynolds number and
is the modified Archimedes number, where
In the formula, dp is the particle size and μa is the gas dynamic viscosity.
When the particle size is greater than 0.6 mm, the particles belong to Geldart D-class particles, and their picking speed is shown in Equation (7).
In the formula, D is the diameter of the test pipeline, D50 = 50 mm.
Selecting the drag force model is crucial for gas–solid coupling. The calculation method for drag force
is as follows:
where
represents the coefficient of resistance between the gas and solid phases and can be defined as follows:
where
is the particle size and
represents the drag coefficient, determining the magnitude of the drag transfer between the fluid and the particle. For non-spherical particles, this work uses the typical correlation proposed by Haider and Levenspiel [
29]. The concept of sphericity is used in the correlation to quantify the effect of particle shape as follows:
In this formula, the local relative Reynolds number of particle
is represented by
, where
is the equivalent diameter, defined as the diameter of a sphere with the same volume as the corresponding non-spherical particle. The sphericity coefficients A, B, C, and D are given by the following:
In the formula, represent the sphericity.
2.2. Size Characteristics of Non-Spherical Particles
Commonly used particle size and granularity are used to characterize the size of particles. The size of individual particles is represented by the particle size, while the size of particle populations is represented by the particle size.
- (1)
Particle size of non-spherical particles
For particles with regular shapes, the particle size is often represented by their characteristic size. Spherical shapes are the simplest to analyze geometrically and physically. In theoretical studies, non-spherical particles are often approximated as spheres, with the spherical equivalent diameter being the most frequently employed parameter. Depending on the context, this equivalent diameter can be defined in terms of volume, specific surface area, drag, free fall, or other criteria, as detailed in
Table 1.
- (2)
Particle size of non-spherical particles
When analyzing the pneumatic conveying of non-spherical particles, it is essential to consider particle groups with varying sizes and shapes. Describing such groups involves determining the average particle size and size distribution. Various statistical methods can be used to calculate the average particle size, including the geometric mean diameter, arithmetic mean diameter, peak diameter, harmonic mean diameter, average surface area diameter, median diameter, and average volume diameter.
The choice of statistical method may result in different average particle sizes; therefore, the appropriate method should be selected based on the physical characteristics and specific requirements of the study. By dividing the particle population into several levels and designating the size of any given level as
dc, the number of particles in that range
nd can be used to calculate the surface area equivalent particle size and volume equivalent particle size for non-spherical particle groups, as shown in Equations (12) and (13).
In the formula, des is the average surface area equivalent particle size of the non-spherical particle group, and dev is the average volume equivalent particle size of the non-spherical particle group.
2.4. Experimental Apparatus
- (1)
Experimental materials and screening
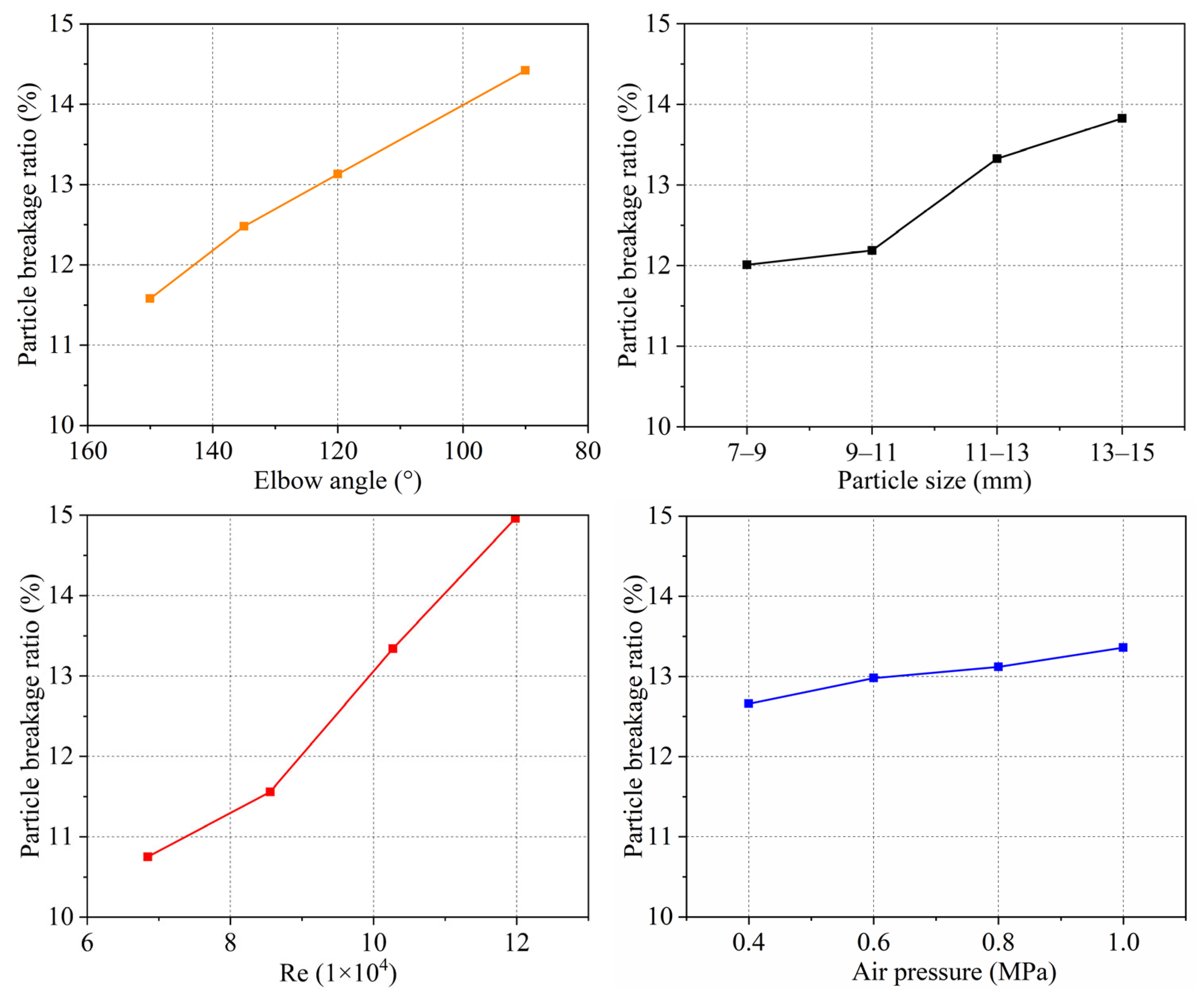
This experiment investigated six different particle sizes of gangue particles, ranging from 3–5 mm, 5–7 mm, 7–9 mm, 9–11 mm, and 11–13 mm to 13–15 mm. The filtering process utilized a three-layer mesh linear vibrating screen.
- (2)
Experimental equipment
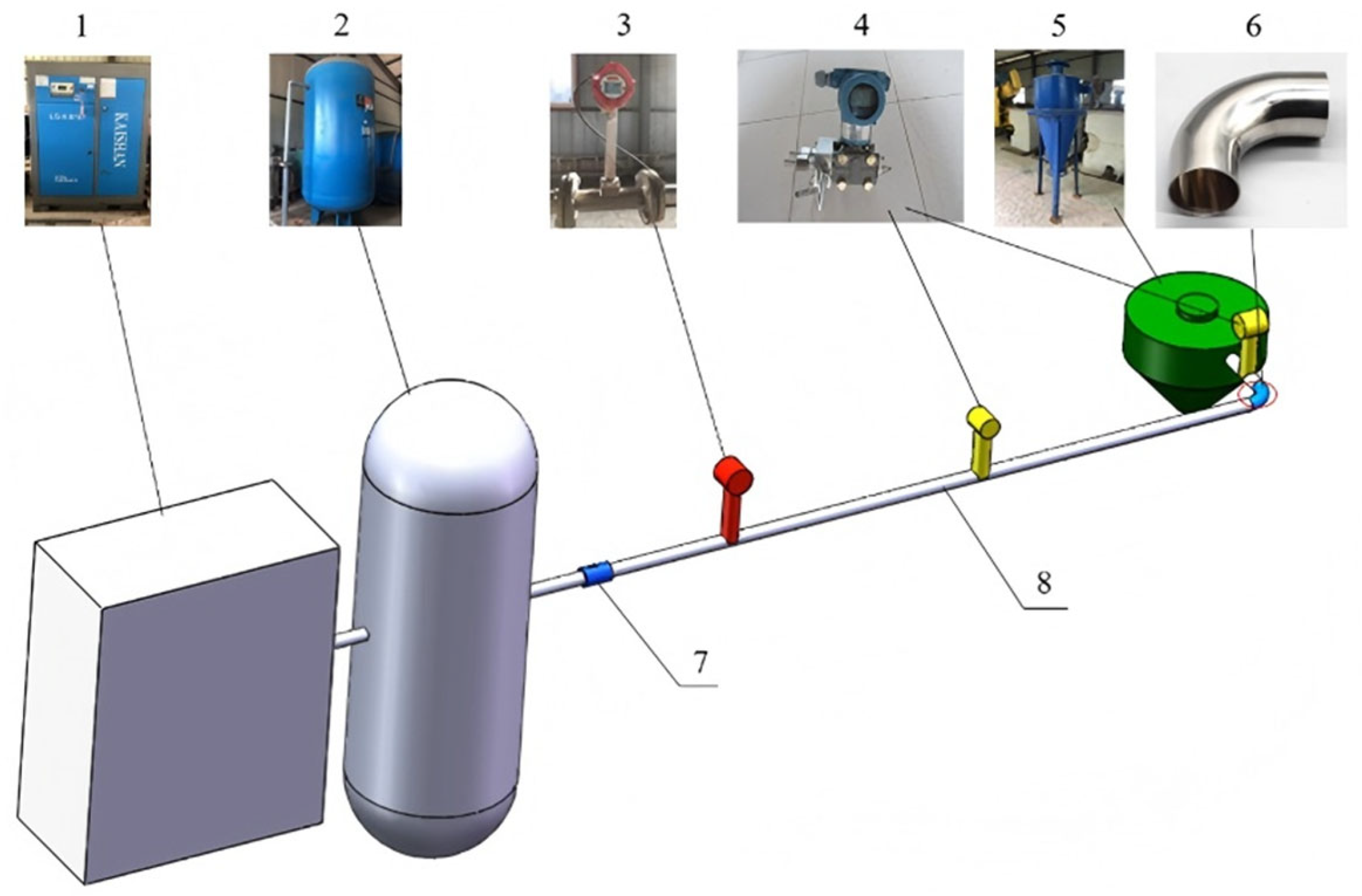
Figure 1 illustrates the pneumatic conveying system employed to study the transport of gangue particles of varying sizes in horizontal pipes. The air source of the experimental system adopts the Kongshan power frequency series screw air compressor, model BK37-8ZG, with a power supply of 37 kW, an air displacement of 6 m
3/min, and a maximum supply pressure of 0.8 MPa. Use explosion-proof steel wire hose to connect the air outlet of the air compressor to the air storage tank, in order to stabilize the air pressure and slow down the airflow oscillation caused by the opening and closing of the air compressor. The design temperature of the gas storage tank is 105 °C, the design pressure is 1.1 MPa, the volume is 3 m
3, and the flow meter range is 30–380 m
3/h. It is equipped with a pressure gauge for measuring the air pressure inside the tank. When its reading is equal to the preset pressure of the air compressor, it represents that the air compressor has completed the current round of inflation and that the airflow has reached stability. The flow meter is SENLOD’s LUGB customized pressure output type, with temperature and pressure compensation. The measurement range is 35–380 m
3/h, and its dial displays the cumulative flow rate and instantaneous flow rate. By reading the instantaneous flow rate, the instantaneous wind speed before particle feeding can be calculated.
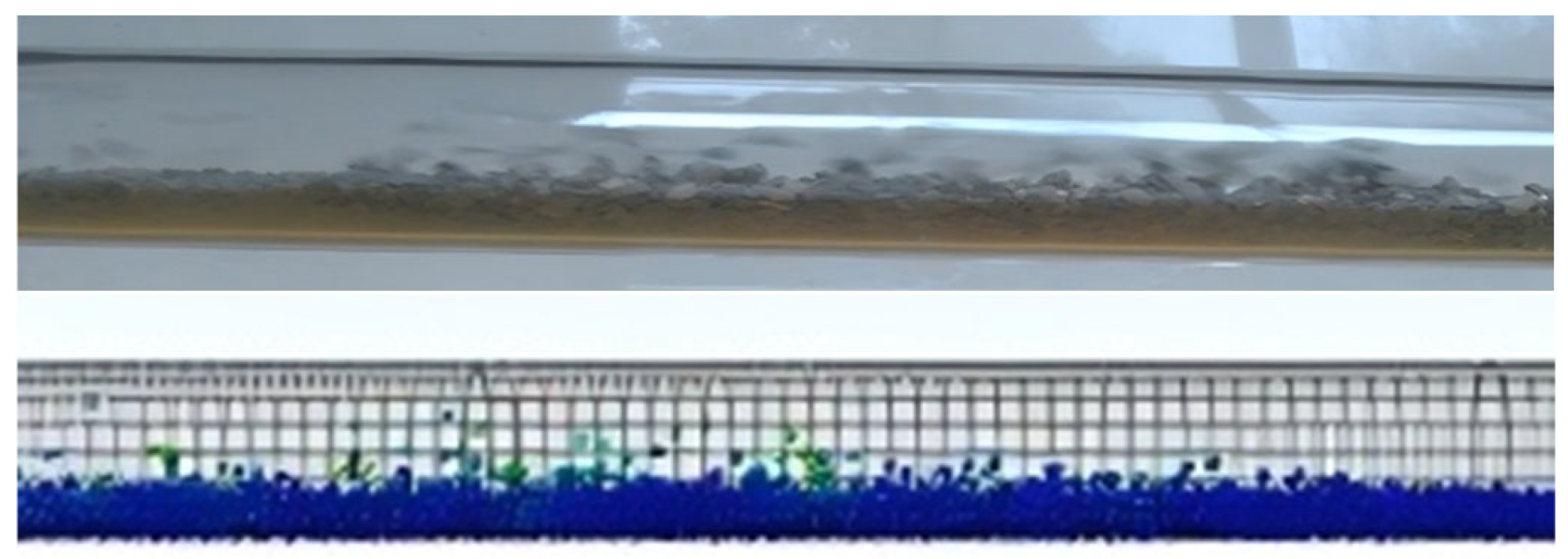
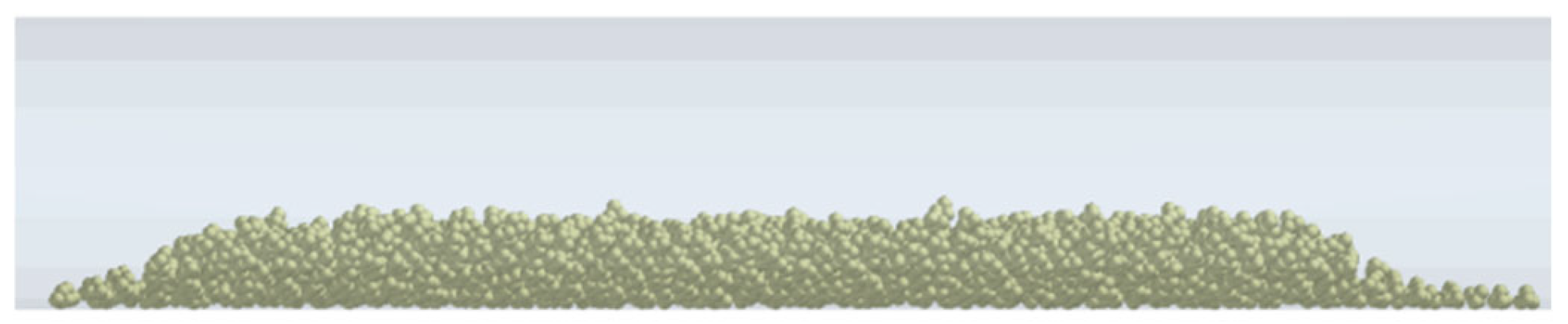
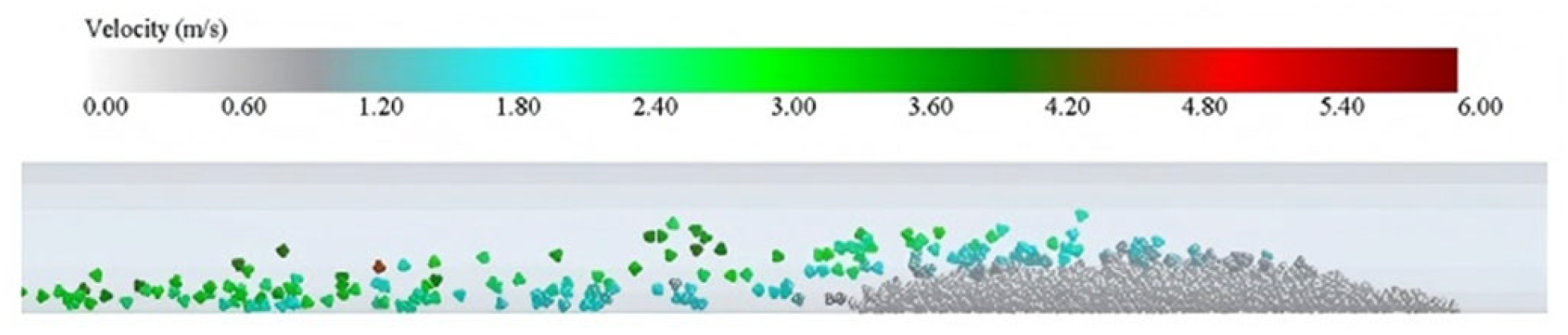
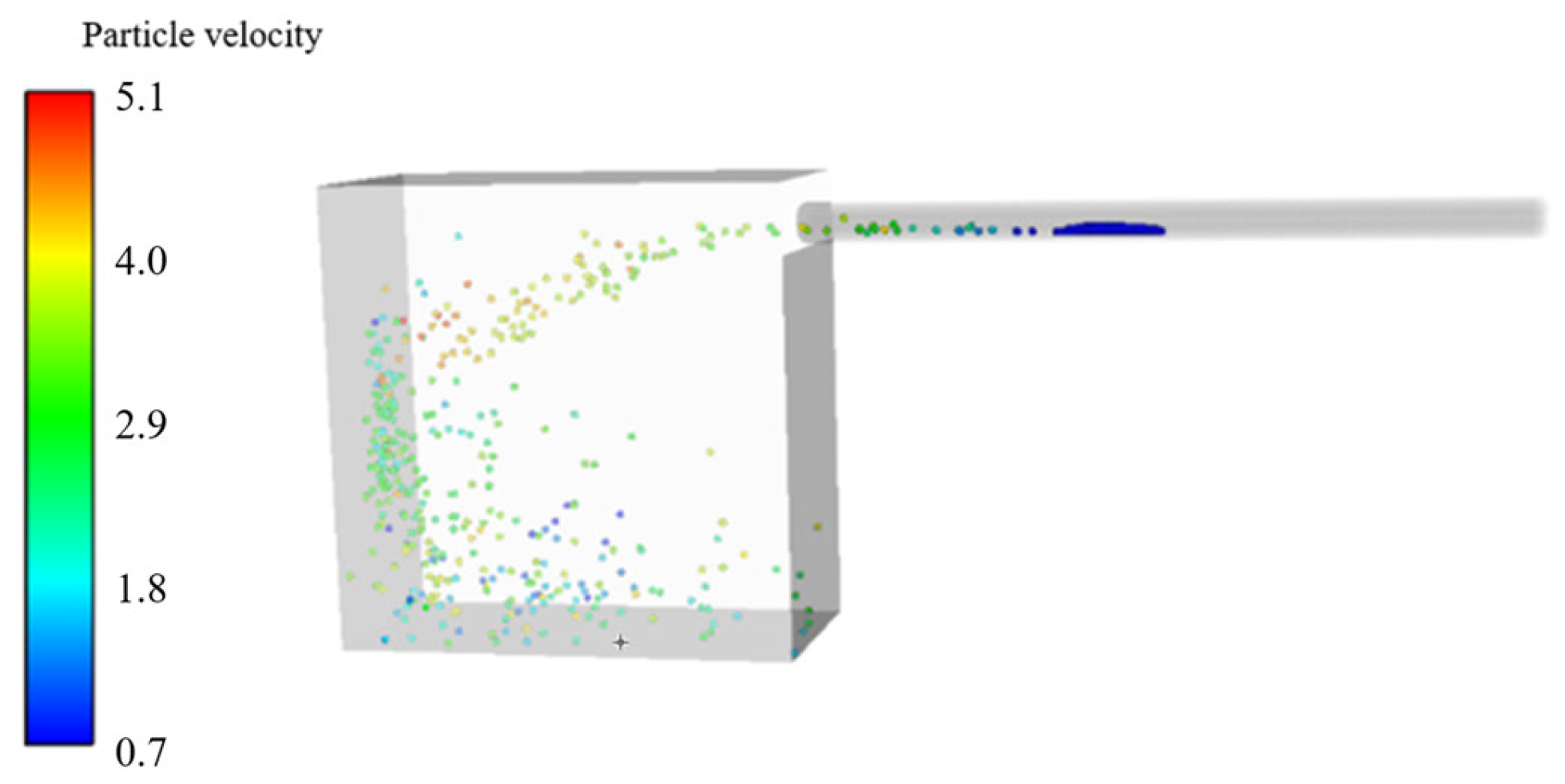
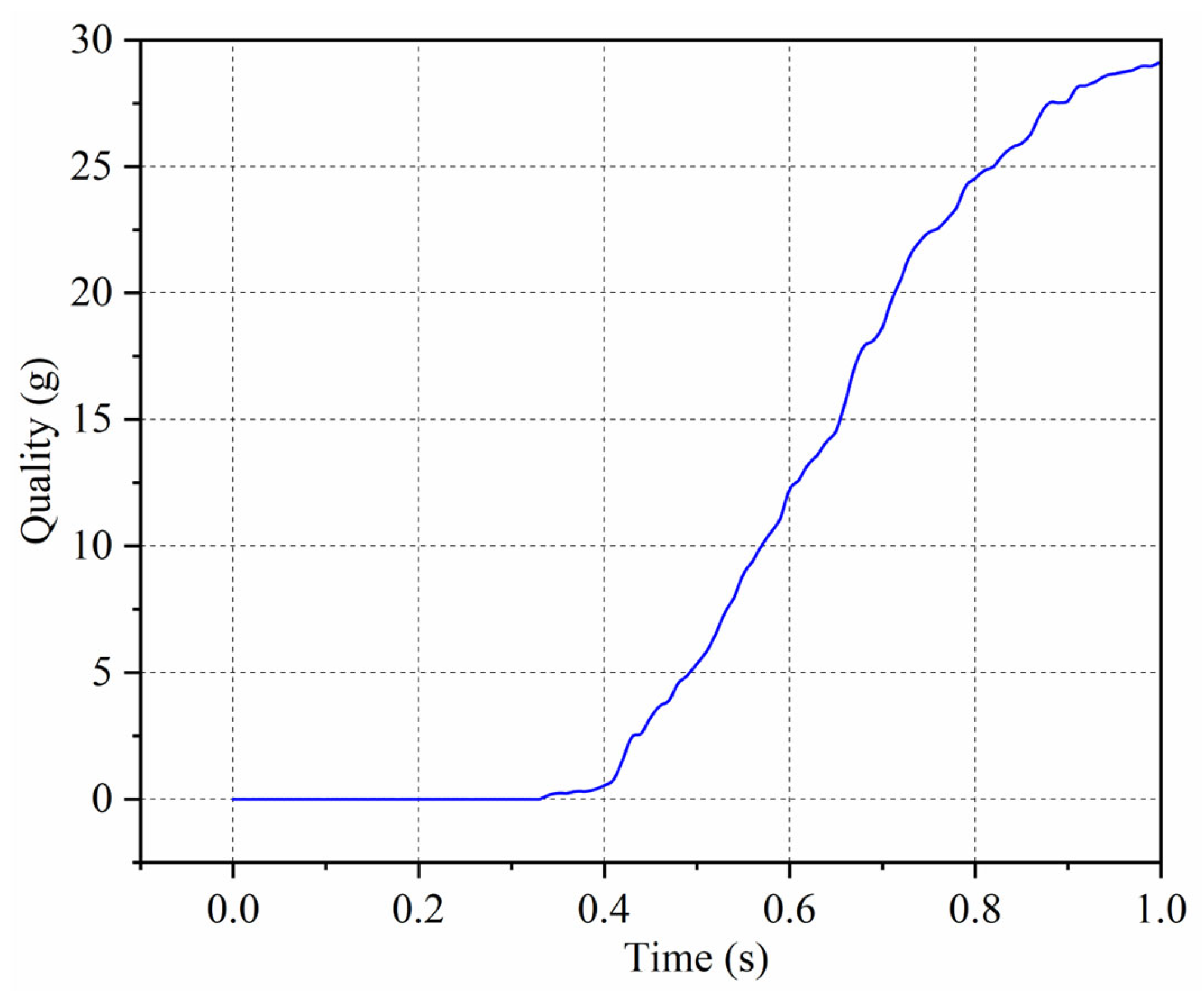
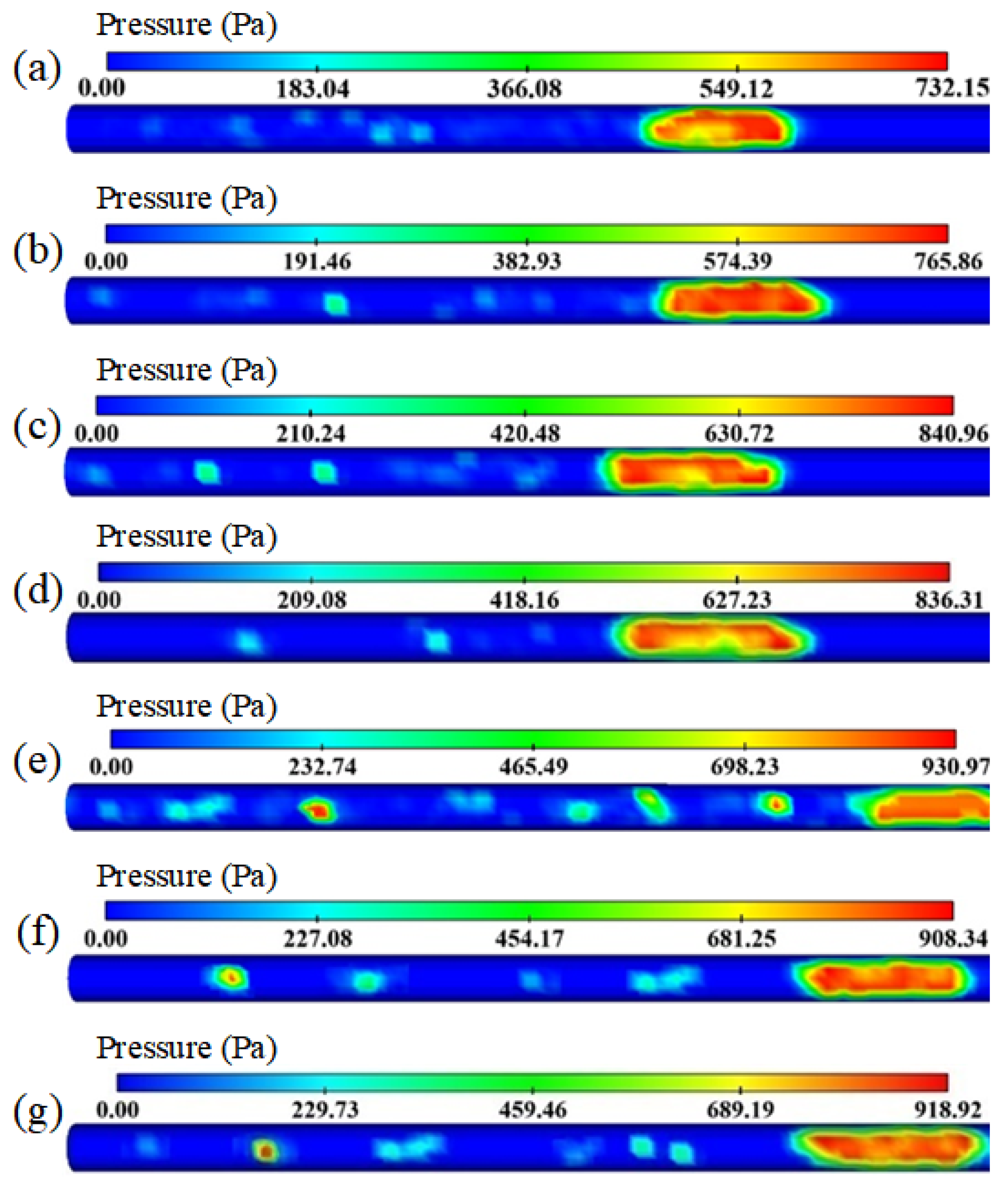
The position between the flowmeter and pressure transmitter is equipped with a Canon EOS R10 camera with a frame rate of 120 fps, used to observe the flow state of particles. A pressure gauge is installed at 20D to record the relationship between particle picking and pressure fluctuations, and to determine the pressure loss pattern of pneumatic conveying. The end of the conveying pipeline is a cyclone separator used to collect particles, weigh them, and calculate the picking rate.
The flowmeter and pressure transmitter are powered by a 24 V DC power supply and both output pressure signals. Their output terminals are connected to the Altay USB 3202N signal collector, which can be driven by the collector and computer to monitor the flow and pressure signals of the flow field in real time.
To prevent pipeline blockages, the pipeline’s inner diameter was chosen to be at least three times the size of the largest non-spherical particles, resulting in a conveying pipe with a 50 mm inner diameter. To stabilize the airflow measurement by the flowmeter, the distance between the flowmeter and the air valve was set to 50 times the pipe diameter (50D). The total length of the acrylic pipe from the flowmeter to the cyclone separator was 60D.