Mechanism and Experimental Research on Rock Breaking by Cutting Teeth of High-Speed PDC Drill Bit
Abstract
1. Introduction
2. Case Analysis of High-Speed PDC Drill Bits
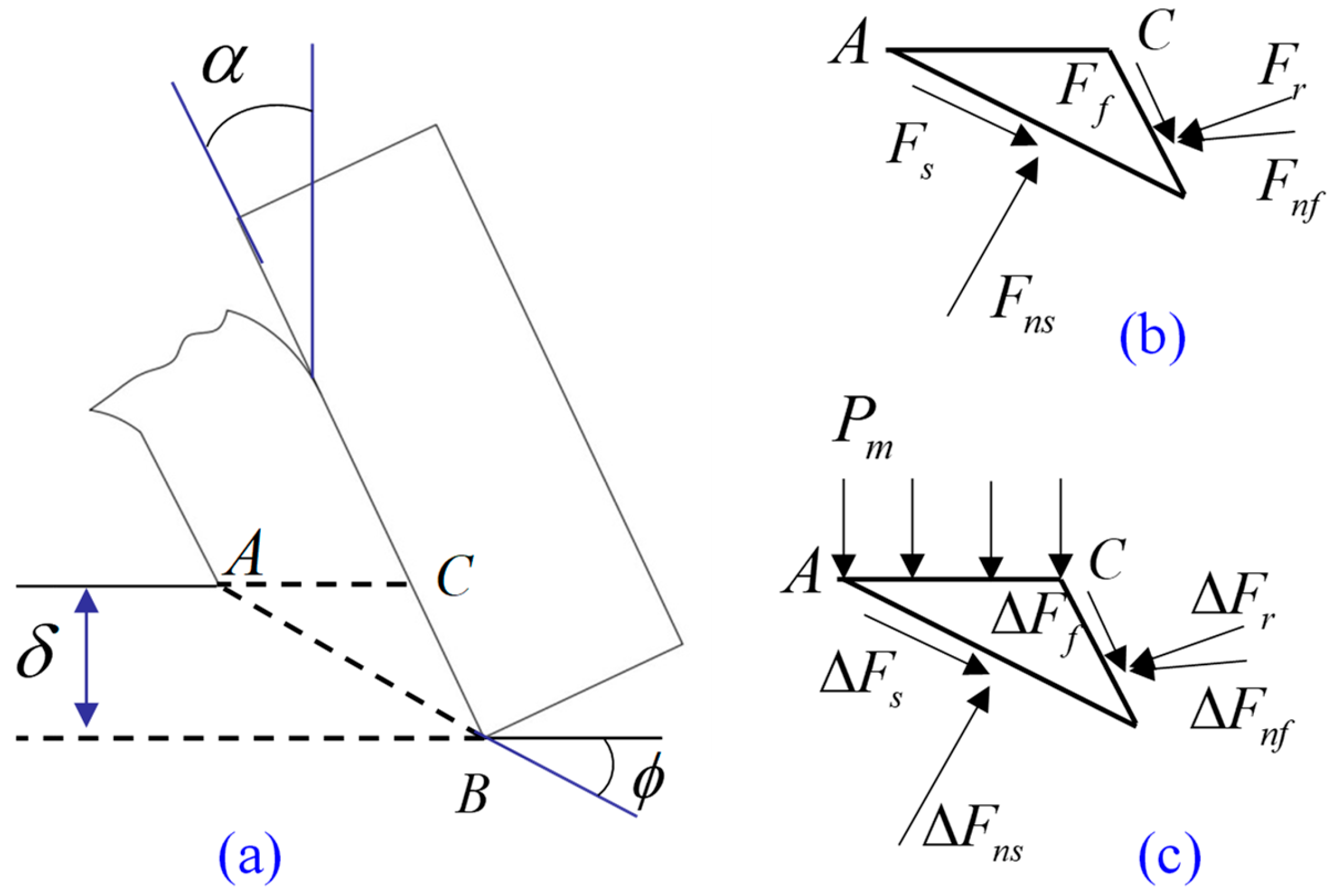
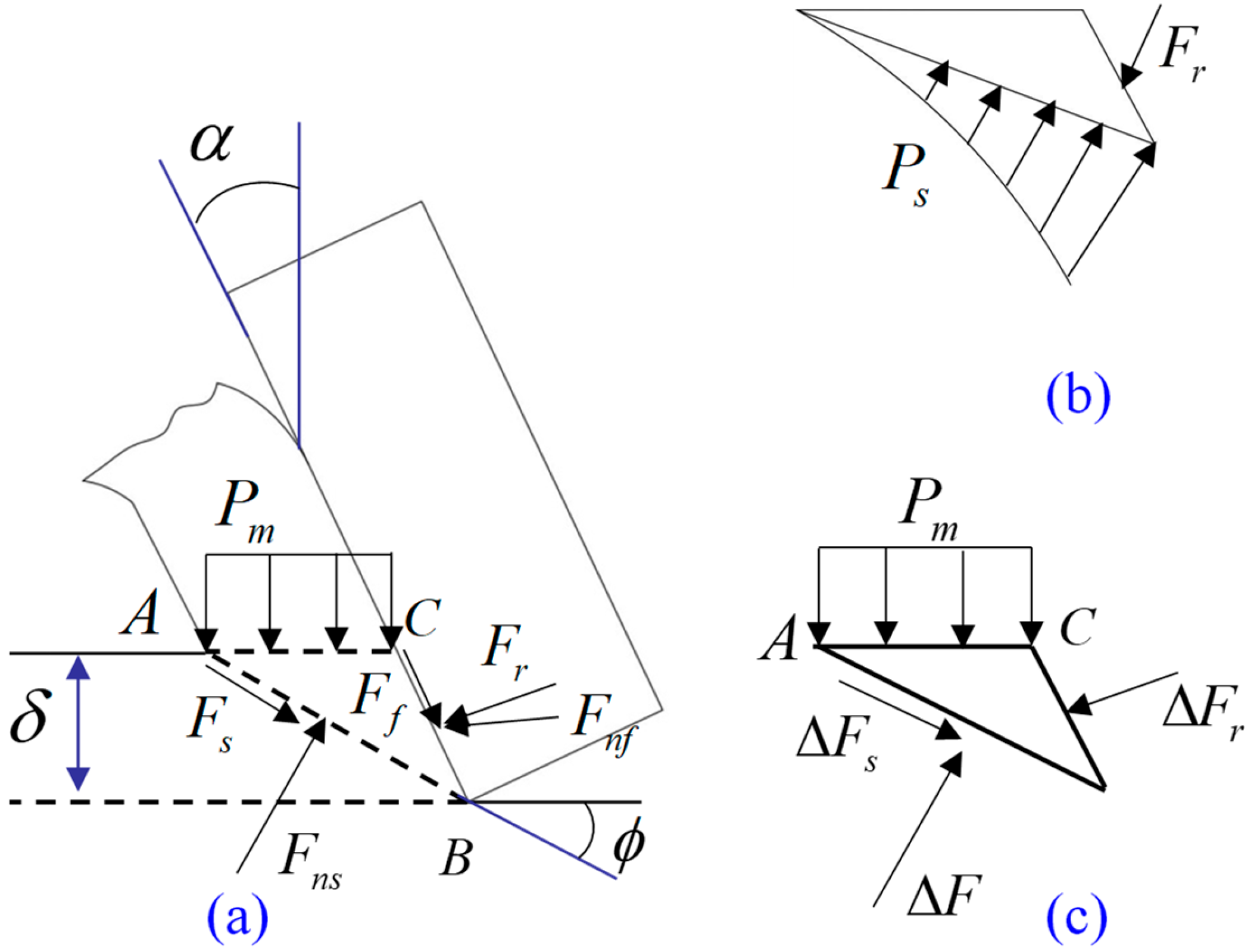
3. Calculation of Single-Tooth Cutting Force
3.1. Cutting Force Under the Condition of Uniform Stress Distribution in the Shear Plane
3.2. Cutting Force Under the Condition of Power Function Distribution of Shear Plane Stress
4. Finite-Element Simulation Study on the Interaction Between Cutting Teeth and Rock
4.1. Three-Dimensional Finite-Element Geometric Modeling
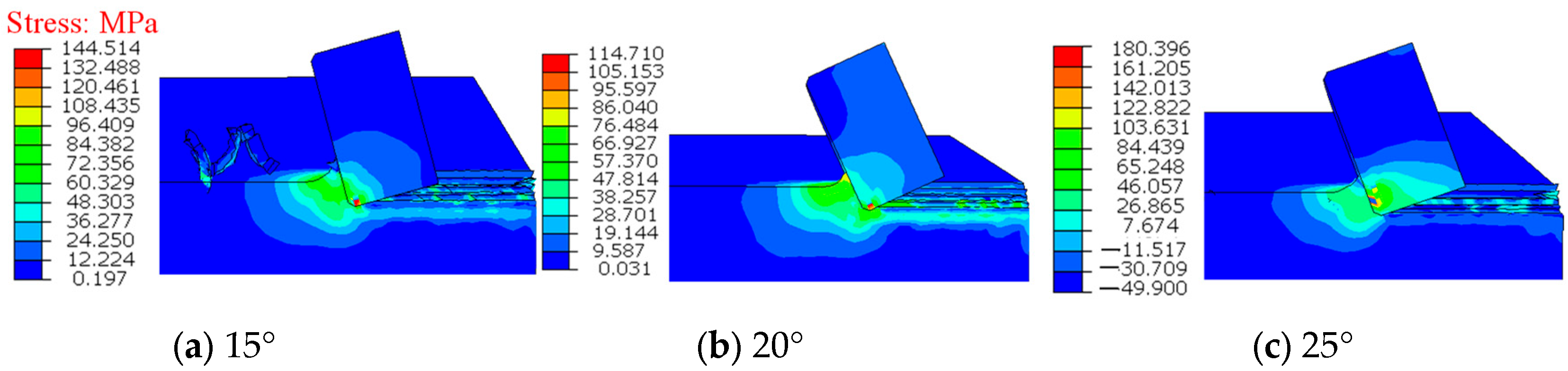
4.2. Three-Dimensional Finite-Element Simulation Results and Analysis
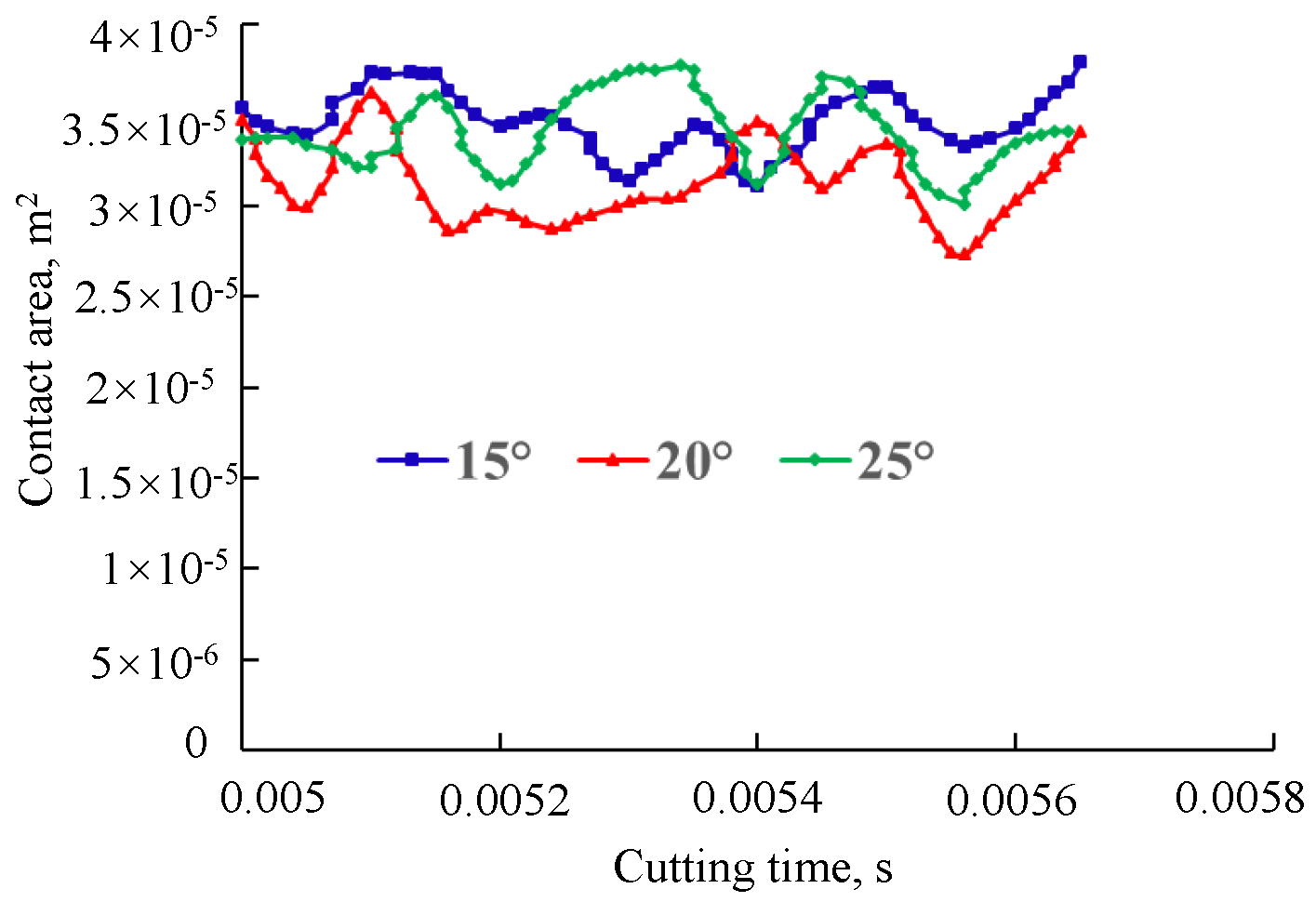
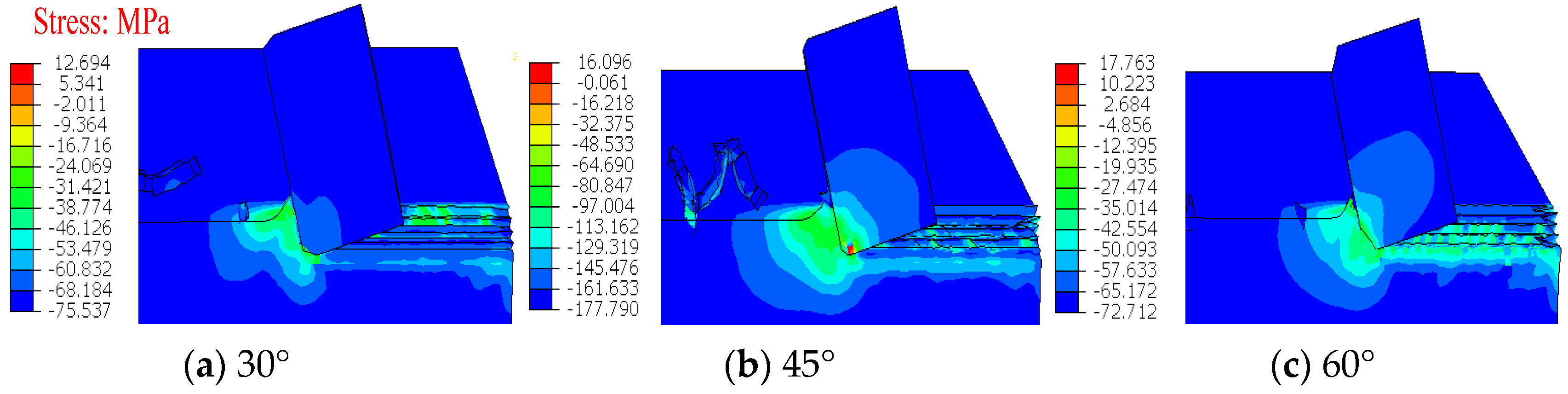
4.2.1. Change the Size of the Rake Angle of the Cutting Teeth
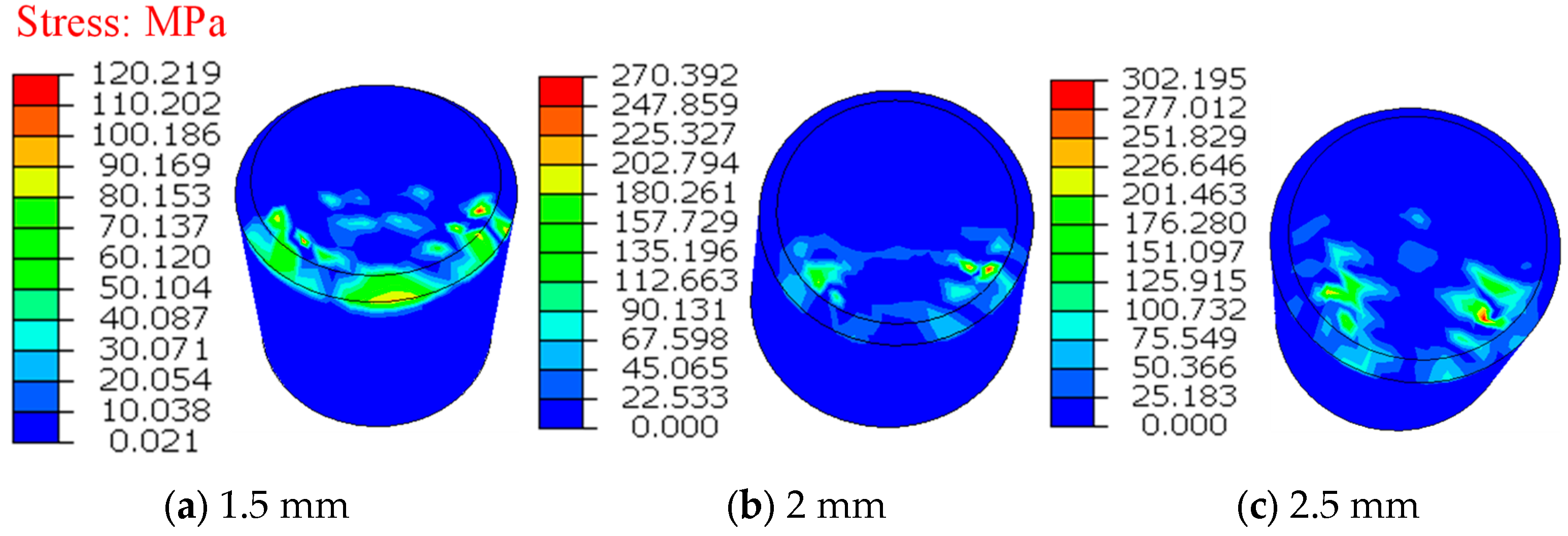
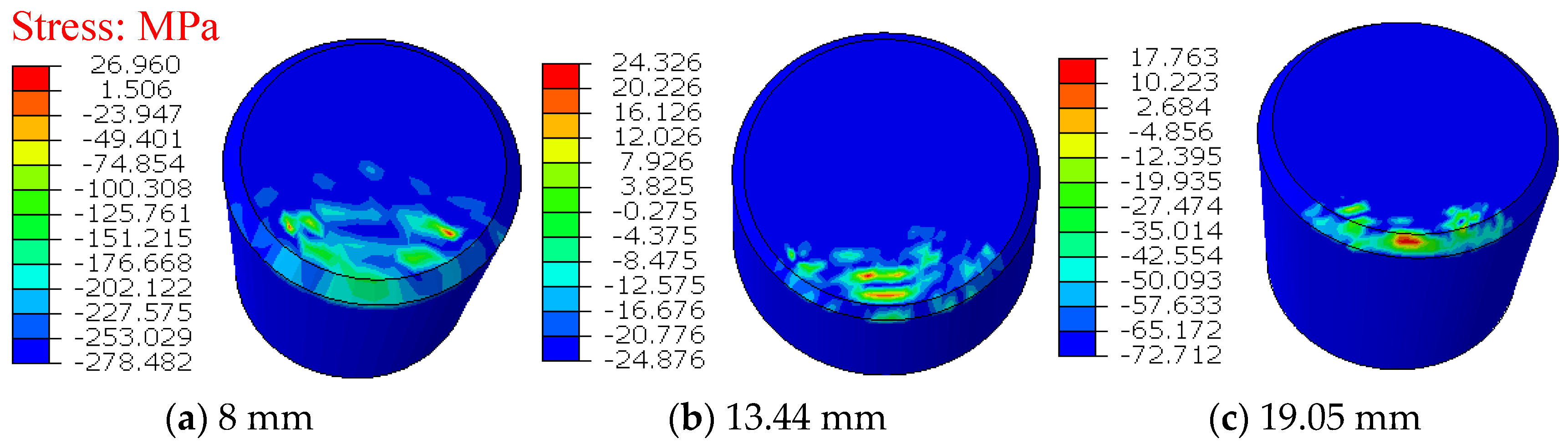
4.2.2. Change the Cutting Depth of the Cutting Teeth
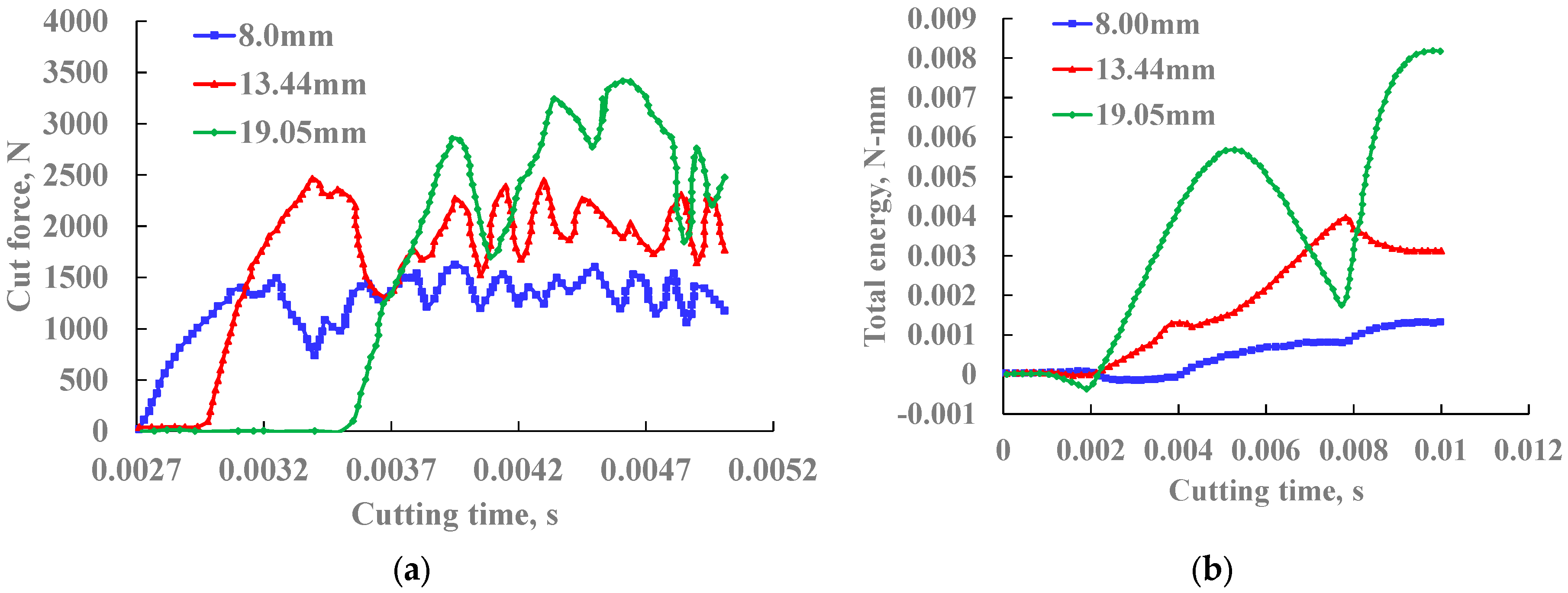
4.2.3. Change the Diameter of the Cutting Tooth
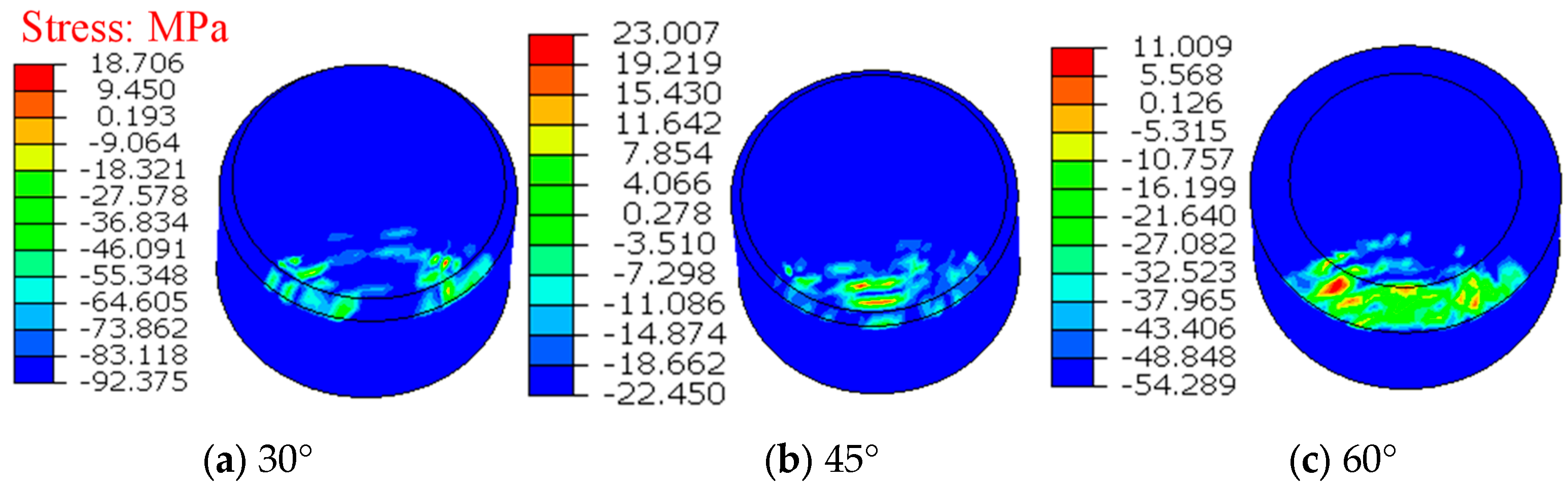
4.2.4. Change the Chamfer Size of the Cutting Teeth
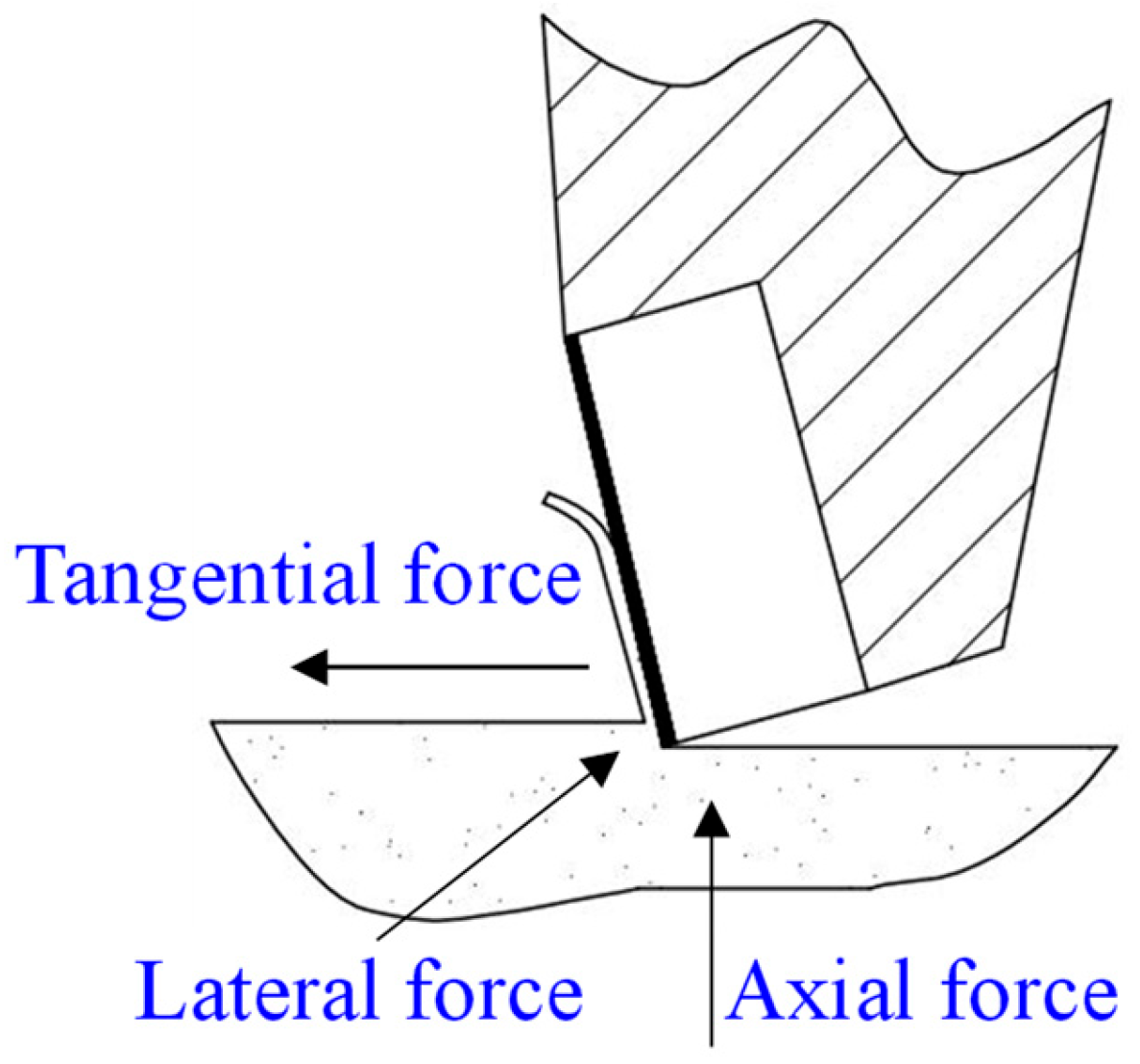
5. Cutting Force Test and Analysis of PDC Tooth
5.1. Purpose and Content of the Experiment
5.2. Experimental Rock Samples and PDC Cutting Teeth
5.3. Cutting Load Test Experimental Process
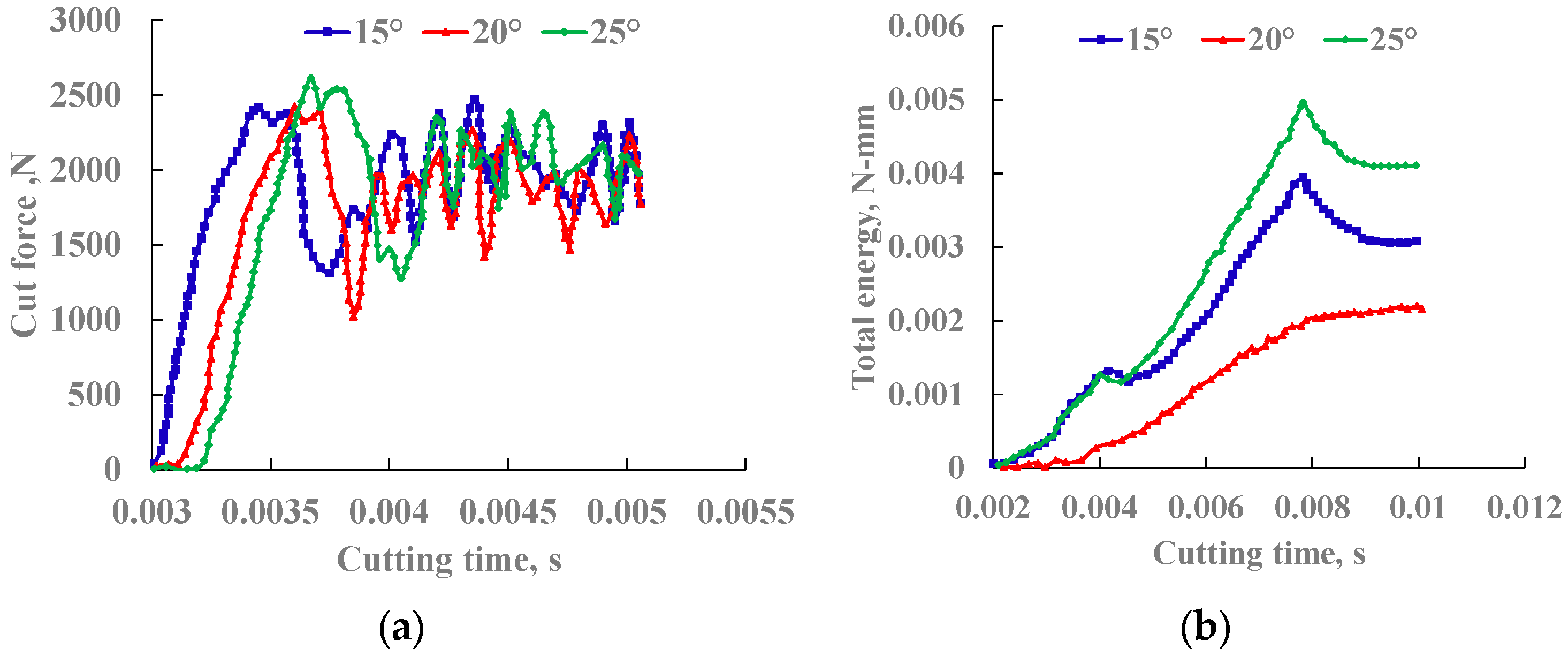
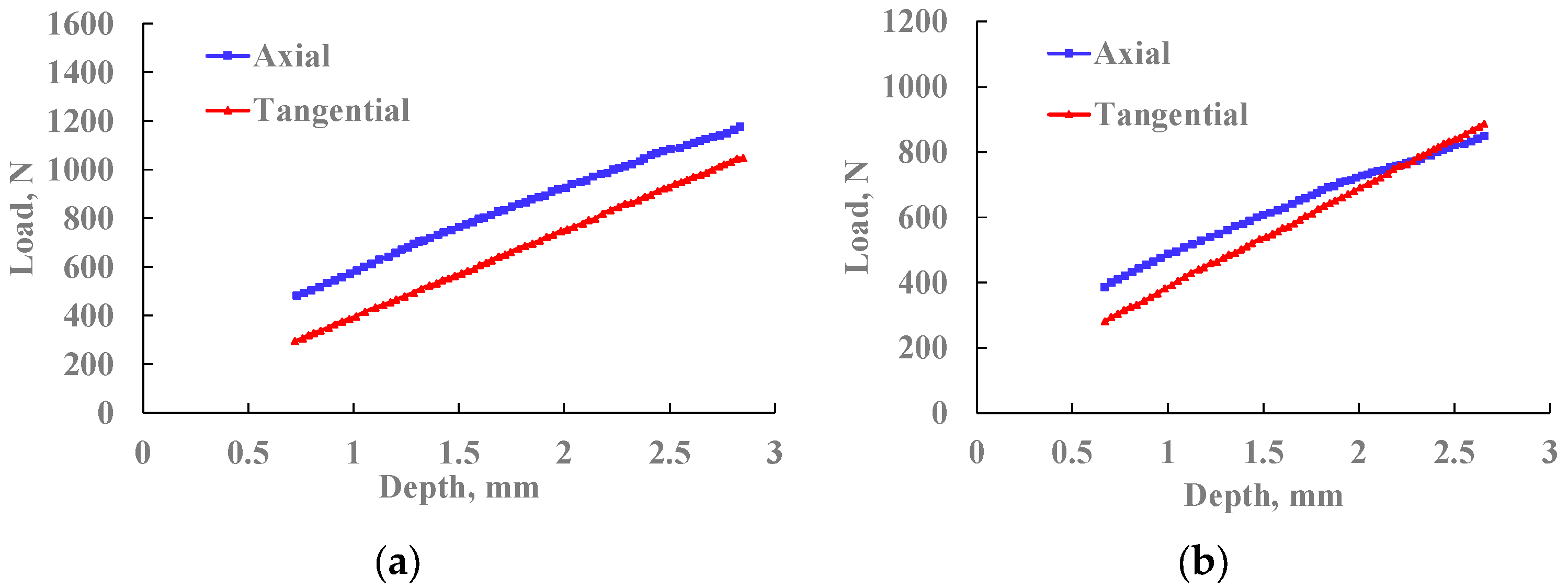
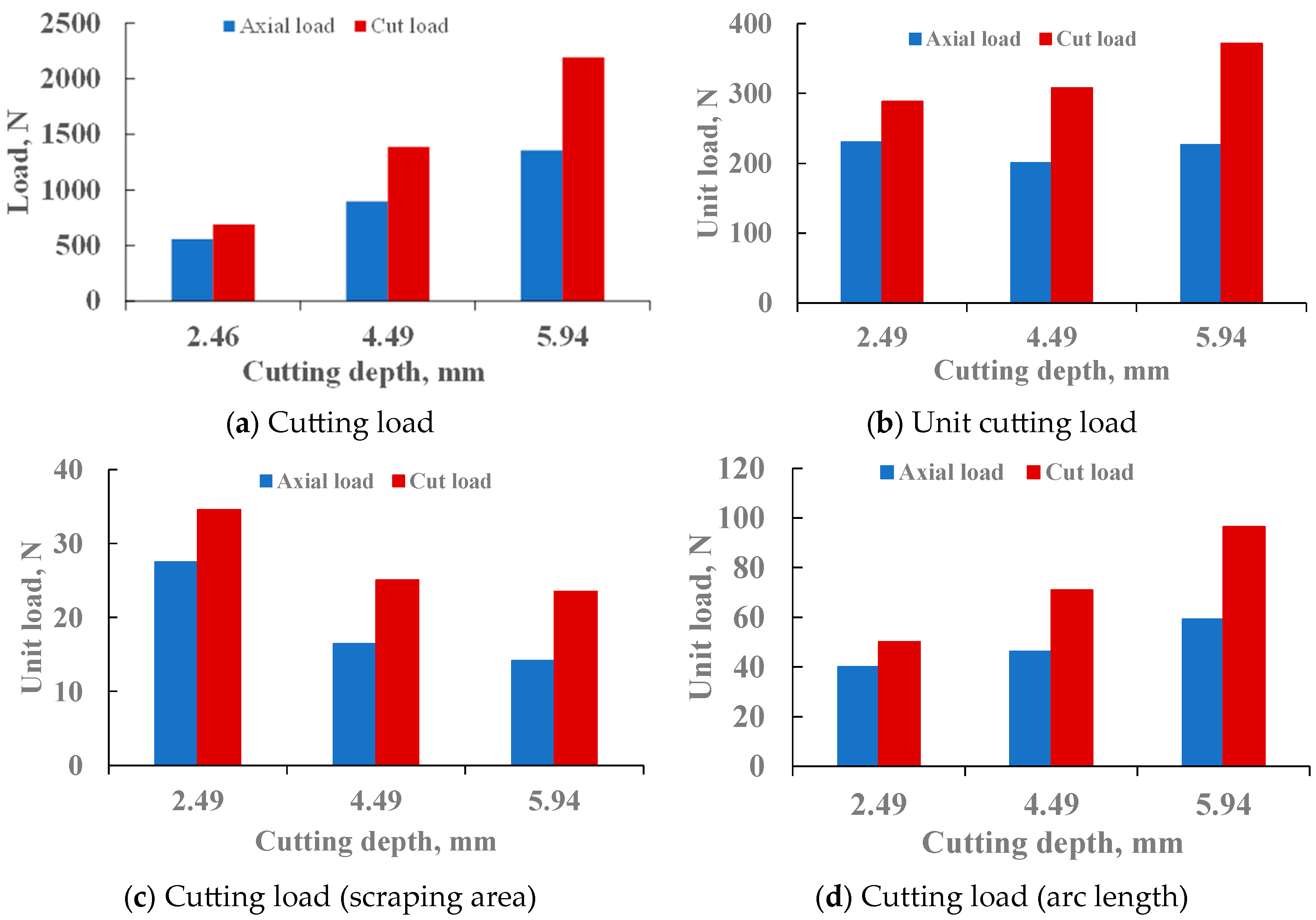
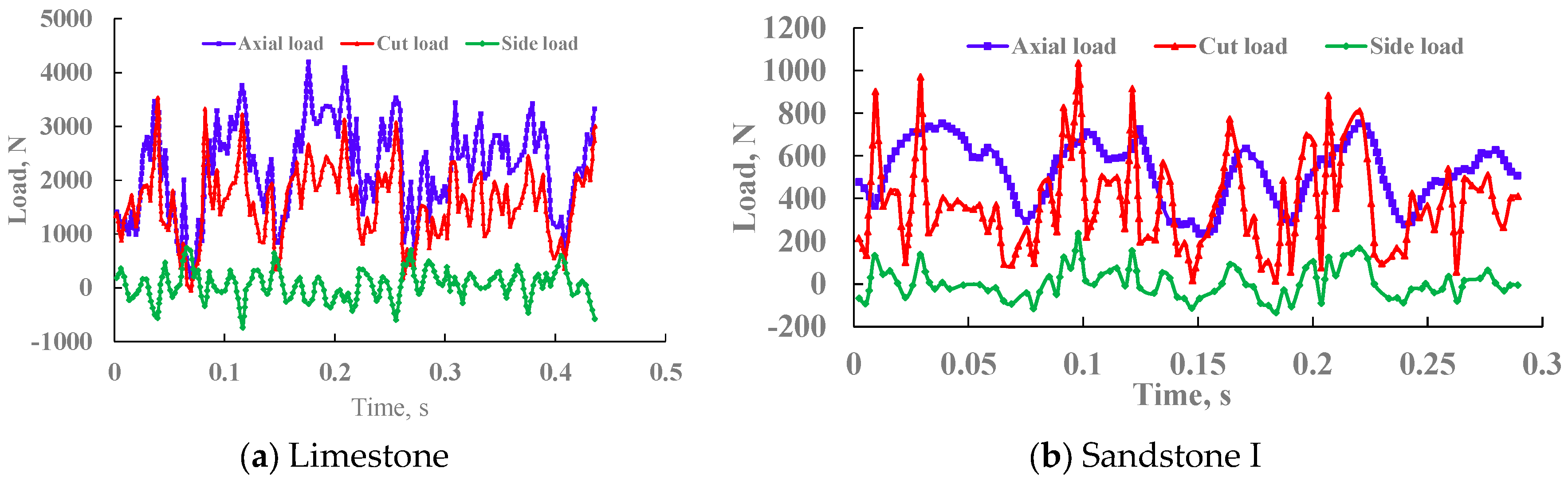
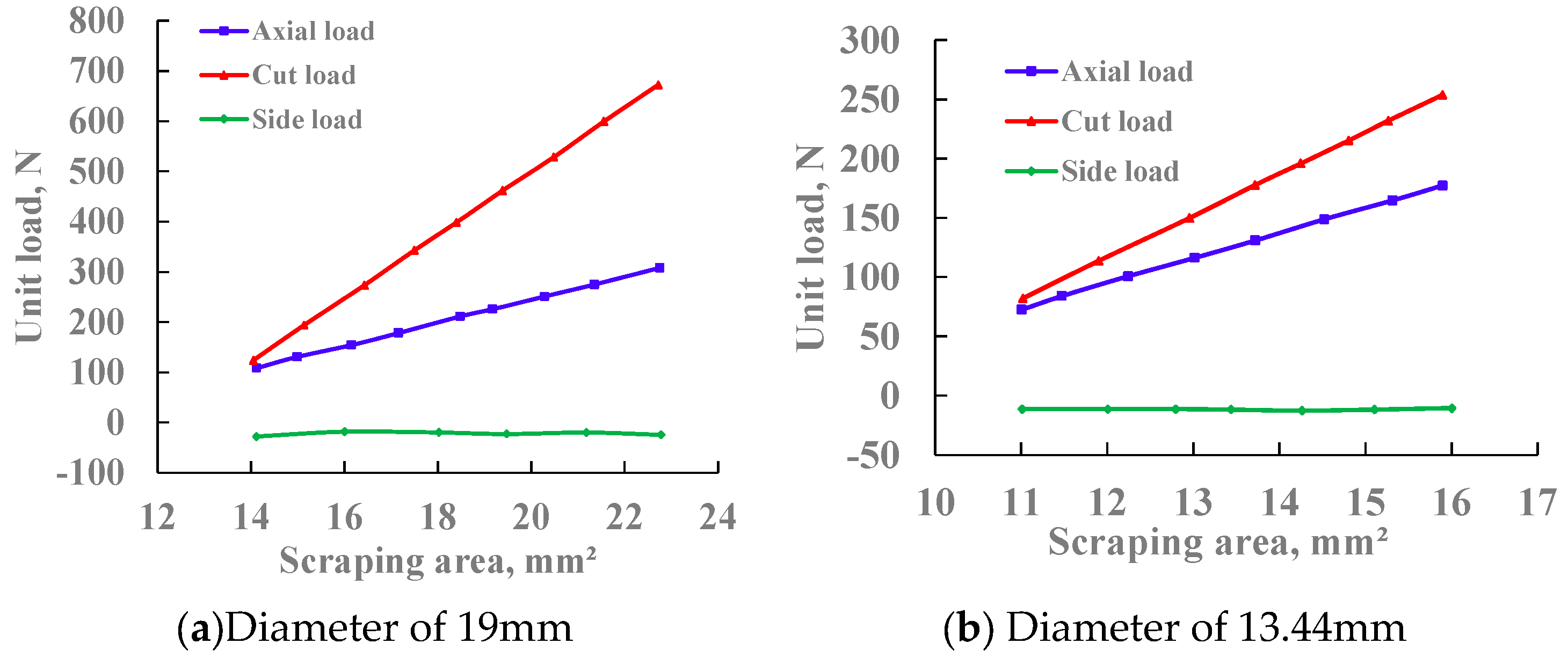
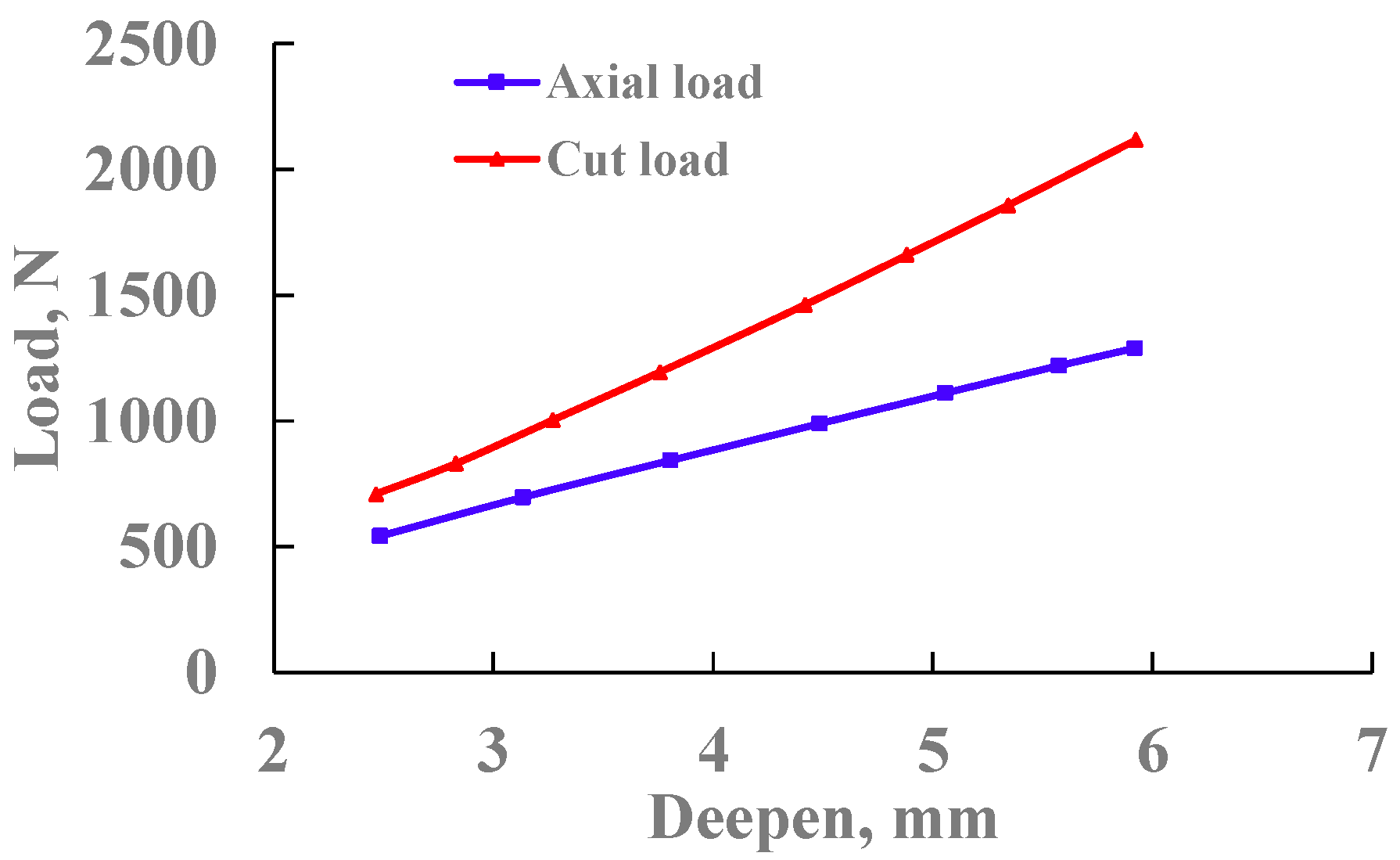
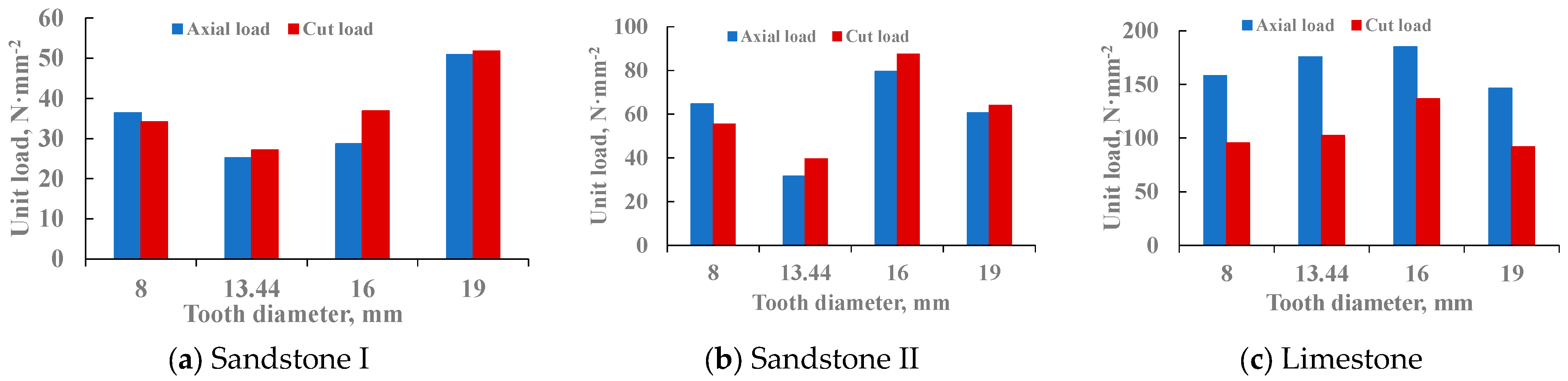
5.4. Experimental Data Processing and Analysis
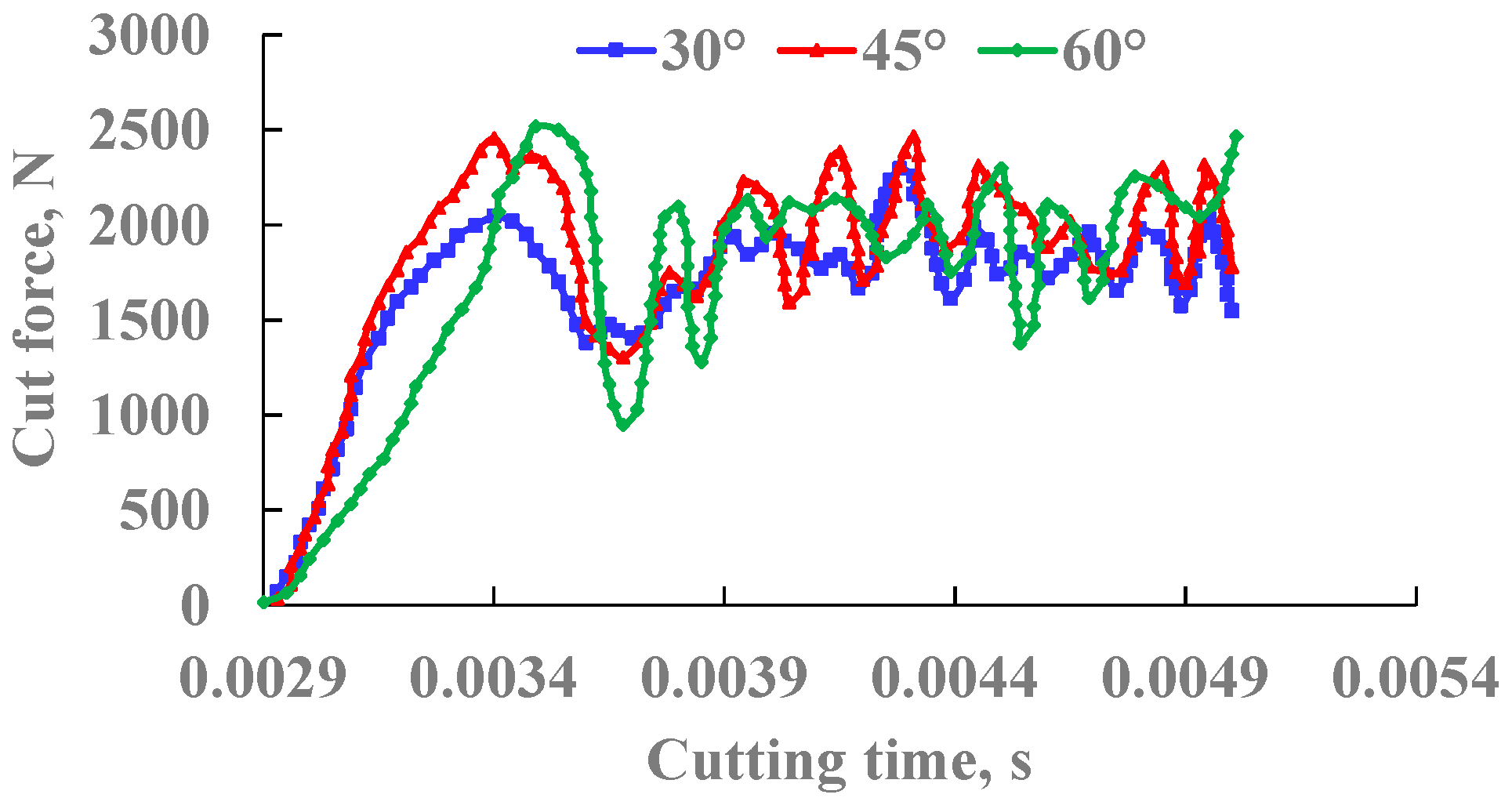
5.5. Analysis of the Variation Law of Cutting Load with Cutting Parameters
6. Discussion and Conclusions
6.1. Discussion
6.2. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Huang, X.; Xu, T.; Liu, Y. Experimental and thermal-structure coupling analysis for oil and water-swellable packer. Energy Sci. Eng. 2023, 11, 3055–3069. [Google Scholar] [CrossRef]
- Liu, W.; Deng, H.; Zhu, X.; Deng, K. The PDC cutter-rock interaction behavior in rock cutting: A review. Geoenergy Sci. Eng. 2023, 229, 212168. [Google Scholar] [CrossRef]
- Liu, Y.; Lian, Z.; Zou, J.; Deng, C.; He, Y. Fracture failure analysis and research on thread joint of drive shaft shell of positive displacement motor. Eng. Fail. Anal. 2020, 118, 104805. [Google Scholar] [CrossRef]
- Ma, Y.; Tao, L.; Rong, Z.; Tang, K.; Huang, Z.; Ke, C.; Wang, G. Research on expression method of PDC bit wear uncertainty and influence of lateral force. Geoenergy Sci. Eng. 2023, 225, 211700. [Google Scholar] [CrossRef]
- Huang, Z.; Li, Q.; Zhou, Y.; Jing, S.; Ma, Y.; Hu, W.; Fan, Y. Experimental research on the surface strengthening technology of roller cone bit bearing based on the failure analysis. Int. J. Rock Mech. Min. Sci. 2018, 105, 11–21. [Google Scholar] [CrossRef]
- Miyazaki, K.; Ohno, T.; Karasawa, H.; Imaizumi, H. Performance of polycrystalline diamond compact bit based on laboratory tests assuming geothermal well drilling. Geothermics 2019, 80, 185–194. [Google Scholar] [CrossRef]
- Dumoulin, S.; Kane, A.; Coudert, T.; Morin, N.; Gerbaud, L.; Velmurugan, N.; Jahangir, E.; Sellami, H.; Latham, J.-P.; Naderi, S.; et al. Three-dimensional numerical study of DTH bit-rock interaction with HPWJ downhole slotting: Influence of bit design and bottom hole geometric conditions on rock breaking efficiency in percussive drilling. Rock Mech. Bull. 2025, 4, 100169. [Google Scholar] [CrossRef]
- Hu, H.; Guan, Z.; Zhang, B.; Xu, Y.; Liu, Y.; Wang, B. Structure design of weight-on-bit self-adjusting PDC bit based on stress field analysis and experiment evaluation. J. Pet. Sci. Eng. 2021, 196, 107692. [Google Scholar] [CrossRef]
- Zhang, Z.; Zhao, D.; Zhao, Y.; Zhou, Y.; Tang, Q.; Han, J. Simulation and experimental study on temperature and stress field of full-sized PDC bits in rock breaking process. J. Pet. Sci. Eng. 2020, 186, 106679. [Google Scholar] [CrossRef]
- Yang, Y.; Yang, Y.-X.; Ren, H.-T.; Qi, Q.-L.; Huang, Z.-Q.; Zhou, C.-X. Experimental study on motion and mechanical characteristics of the vertical wheel in the rock-breaking process. Pet. Sci. 2023, 20, 495–506. [Google Scholar] [CrossRef]
- Cirimello, P.; Otegui, J.; Sanchez, J.M.; Carfi, G. Oil well drill bit failure during pull out: Redesign to reduce its consequences. Eng. Fail. Anal. 2018, 83, 75–87. [Google Scholar] [CrossRef]
- Stoxreiter, T.; Portwood, G.; Gerbaud, L.; Seibel, O.; Essl, S.; Plank, J.; Hofstätter, H. Full-scale experimental investigation of the performance of a jet-assisted rotary drilling system in crystalline rock. Int. J. Rock Mech. Min. Sci. 2019, 115, 87–98. [Google Scholar] [CrossRef]
- He, W.; Zhang, R.; Liu, L.; Chen, Z.; Shi, H.; Huang, Z.; Xiong, C.; Li, X.; Sun, J.; Hu, C. Numerical simulation of rock-breaking mechanisms by triple-ridged PDC cutter in hard rocks. Geoenergy Sci. Eng. 2023, 229, 212148. [Google Scholar] [CrossRef]
- Al-Sudani, J.A. Real-time monitoring of mechanical specific energy and bit wear using control engineering systems. J. Pet. Sci. Eng. 2017, 149, 171–182. [Google Scholar] [CrossRef]
- Shao, F.; Liu, W.; Gao, D. Effects of the chamfer and materials on performance of PDC cutters. J. Pet. Sci. Eng. 2021, 205, 108887. [Google Scholar] [CrossRef]
- Zhang, D.; Yang, Y.; Ren, H.; Niu, S. Failure resistance research of the dual-stage hybrid bit. Eng. Fail. Anal. 2023, 145, 107009. [Google Scholar] [CrossRef]
- Lin, W.; Chávez, J.P.; Liu, Y.; Yang, Y.; Kuang, Y. Stick-slip suppression and speed tuning for a drill-string system via proportional-derivative control. Appl. Math. Model. 2020, 82, 487–502. [Google Scholar] [CrossRef]
- Niu, S.; Li, Y.; Xie, B.; Yang, Y.; Li, G.; Huang, K. Unit experimental and numerical simulation study on rock breaking mechanism of disc-like hybrid bit. Geoenergy Sci. Eng. 2023, 228, 212006. [Google Scholar] [CrossRef]
- Huang, K.; Yang, Y.; Liu, Y.; Niu, Q. Vibration failure and anti-vibration analysis of an annular-grooved PDC bit. Eng. Fail. Anal. 2020, 115, 104658. [Google Scholar] [CrossRef]
- Barnett, L.; Al Dushaishi, M.F.; Khan, M.F.M.H. Experimental investigation of drillstring torsional vibration effect on rate of penetration with PDC bits in hard rock. Geothermics 2022, 103, 102410. [Google Scholar] [CrossRef]
- Zhang, C.L.; Yang, Y.X.; Qi, Q.L.; Ren, H.T.; Wang, J.C. Research on numerical drilling technology of mesh-like cutting PDC bit. Energy Rep. 2021, 7, 2068–2080. [Google Scholar] [CrossRef]
- Li, Y.; Chen, Z.; Ye, Y.; Yang, Y. Combined finite-discrete element method for modeling the interaction between single PDC cutter and brittle rock. J. Pet. Sci. Eng. 2021, 207, 109133. [Google Scholar] [CrossRef]
- Panayirci, H.M. An experimental study on steering response of PDC drill bits. J. Pet. Sci. Eng. 2022, 208, 109440. [Google Scholar] [CrossRef]
- de Moura, J.; Xiao, Y.; Yang, J.; Butt, S.D. An empirical model for the drilling performance prediction for roll-er-cone drill bits. J. Pet. Sci. Eng. 2021, 204, 108791. [Google Scholar] [CrossRef]
- Zhang, C.; Wang, J.; Ke, X.; Yang, Y.; Ren, H.; Niu, S.; Cai, C. Rock-breaking performance analysis of worn polycrystalline diamond compact bit. Geoenergy Sci. Eng. 2023, 221, 211352. [Google Scholar] [CrossRef]
- Song, D.; Ren, Z.; Yang, Y.; Ren, H. Research on cutter surface shapes and rock breaking efficiency under high well temperature. Geoenergy Sci. Eng. 2023, 222, 211422. [Google Scholar] [CrossRef]
- Cai, C.; Kang, Y.; Yang, Y.; Wang, X.; Li, Y.; Huang, M.; Wu, J. The effect of shale bedding on supercritical CO2 jet fracturing: A experimental study. J. Pet. Sci. Eng. 2020, 195, 107798. [Google Scholar] [CrossRef]
- Yang, Y.; Yang, Y.; Ren, H.; Qi, Q.; Chen, X. Research on the working mechanism of the PDC drill bit in compound drilling. J. Pet. Sci. Eng. 2020, 185, 106647. [Google Scholar] [CrossRef]
- Yang, Y.; Yang, Y.; Liu, X.; Huang, K.; Ren, H. Optimized design and application of a directional reaming-while-drilling polycrystalline diamond compact bit. Eng. Fail. Anal. 2019, 105, 699–707. [Google Scholar] [CrossRef]
- Mostafa, G.; Behzad, E. Real time prediction and detection of drilling bit issues during drilling with the focus on bit status evaluation using along string measurement (ASM). Geoenergy Sci. Eng. 2023, 224, 211612. [Google Scholar]
- Moazzeni, A.R.; Khamehchi, E. Rain optimization algorithm (ROA): A new metaheuristic method for drilling opti-mization solutions. J. Pet. Sci. Eng. 2020, 195, 107512. [Google Scholar] [CrossRef]
- Elkatatny, S. Real-time prediction of rate of penetration while drilling complex lithologies using artificial intelligence techniques. Ain Shams Eng. J. 2021, 12, 917–926. [Google Scholar] [CrossRef]
- Boukredera, F.S.; Youcefi, M.R.; Hadjadj, A.; Ezenkwu, C.P.; Vaziri, V.; Aphale, S.S. Enhancing the drilling efficiency through the appli-cation of machine learning and optimization algorithm. Eng. Appl. Artif. Intell. 2023, 126, 107035. [Google Scholar] [CrossRef]
- Afebu, K.O.; Liu, Y.; Papatheou, E. Feature-based intelligent models for optimisation of percussive drilling. Neural Netw. 2022, 148, 266–284. [Google Scholar] [CrossRef]
- Dougherty, P.S.; Mpagazehe, J.; Shelton, J.; Iii, C.F.H. Elucidating PDC rock cutting behavior in dry and aqueous conditions using tribometry. J. Pet. Sci. Eng. 2015, 133, 529–542. [Google Scholar] [CrossRef]
- Kamel, J.M.; Yigit, A.S. Modeling and analysis of stick-slip and bit bounce in oil well drillstrings equipped with drag bits. J. Sound Vib. 2014, 333, 6885–6899. [Google Scholar] [CrossRef]
- Khoshouei, M.; Bagherpour, R. Measurement, prediction, and modeling of the drilling specific energy by soft rock properties during the drilling operation. Measurement 2023, 222, 113679. [Google Scholar] [CrossRef]
- Mazen, A.Z.; Mujtaba, I.M.; Hassanpour, A.; Rahmanian, N. Mathematical modelling of performance and wear prediction of PDC drill bits: Impact of bit profile, bit hydraulic, and rock strength. J. Pet. Sci. Eng. 2020, 188, 106849. [Google Scholar] [CrossRef]
- Iwata, K.; Osakada, K.; Terasaka, T. Process Modeling of Orthogonal Cutting by the Rigid-plastic Finite Element Method. Tran. ASME J. Eng. Mater. Technol. 1984, 106, 132–138. [Google Scholar] [CrossRef]










| Value | X1 | X2 | Ev | |
|---|---|---|---|---|
| Depth | ||||
| 1.5 mm | 565.6 | 96.3 | 5.87 | |
| 2.0 mm | 344.1 | 48.5 | 7.19 | |
| 2.5 mm | 335.4 | 62.75 | 5.36 | |
| Value | X1 | X2 | Ev | |
|---|---|---|---|---|
| Chamfers | ||||
| 30° | 264.0 | 93.38 | 2.83 | |
| 45° | 219.5 | 129.7 | 1.69 | |
| 60° | 251.0 | 118.0 | 2.13 | |
| Mean Value (N) | Variance | Maximum Value (N) | Minimum Value (N) | |
|---|---|---|---|---|
| Axial load | 1512.4 | 349,472.5 | 3004.3 | 441.6 |
| Tangential load | 2045.1 | 912,048.8 | 4523.4 | 318.3 |
| Lateral load | 24.2 | 3812.3 | 107.5 | −130.5 |
| Anterior angle α (°) | 0 | 5 | 10 | 15 | 20 | 25 | 30 |
| Critical roll angle β′ (°) | 0 | 0 | 0 | 5 | 10 | 15 | 20 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Z.; Wang, C.; Feng, S.; Li, N.; Zhang, Z.; Fan, Y. Mechanism and Experimental Research on Rock Breaking by Cutting Teeth of High-Speed PDC Drill Bit. Processes 2025, 13, 1213. https://doi.org/10.3390/pr13041213
Wang Z, Wang C, Feng S, Li N, Zhang Z, Fan Y. Mechanism and Experimental Research on Rock Breaking by Cutting Teeth of High-Speed PDC Drill Bit. Processes. 2025; 13(4):1213. https://doi.org/10.3390/pr13041213
Chicago/Turabian StyleWang, Zhe, Chunsheng Wang, Shaobo Feng, Ning Li, Zhi Zhang, and Yuguang Fan. 2025. "Mechanism and Experimental Research on Rock Breaking by Cutting Teeth of High-Speed PDC Drill Bit" Processes 13, no. 4: 1213. https://doi.org/10.3390/pr13041213
APA StyleWang, Z., Wang, C., Feng, S., Li, N., Zhang, Z., & Fan, Y. (2025). Mechanism and Experimental Research on Rock Breaking by Cutting Teeth of High-Speed PDC Drill Bit. Processes, 13(4), 1213. https://doi.org/10.3390/pr13041213





