Enhancement of Geothermal Exploitation in Hot Dry Rock Reservoir Through Multilateral Horizontal Well Systems: A Case Study in Qiabuqia Geothermal Field, Gonghe Basin
Abstract
1. Introduction
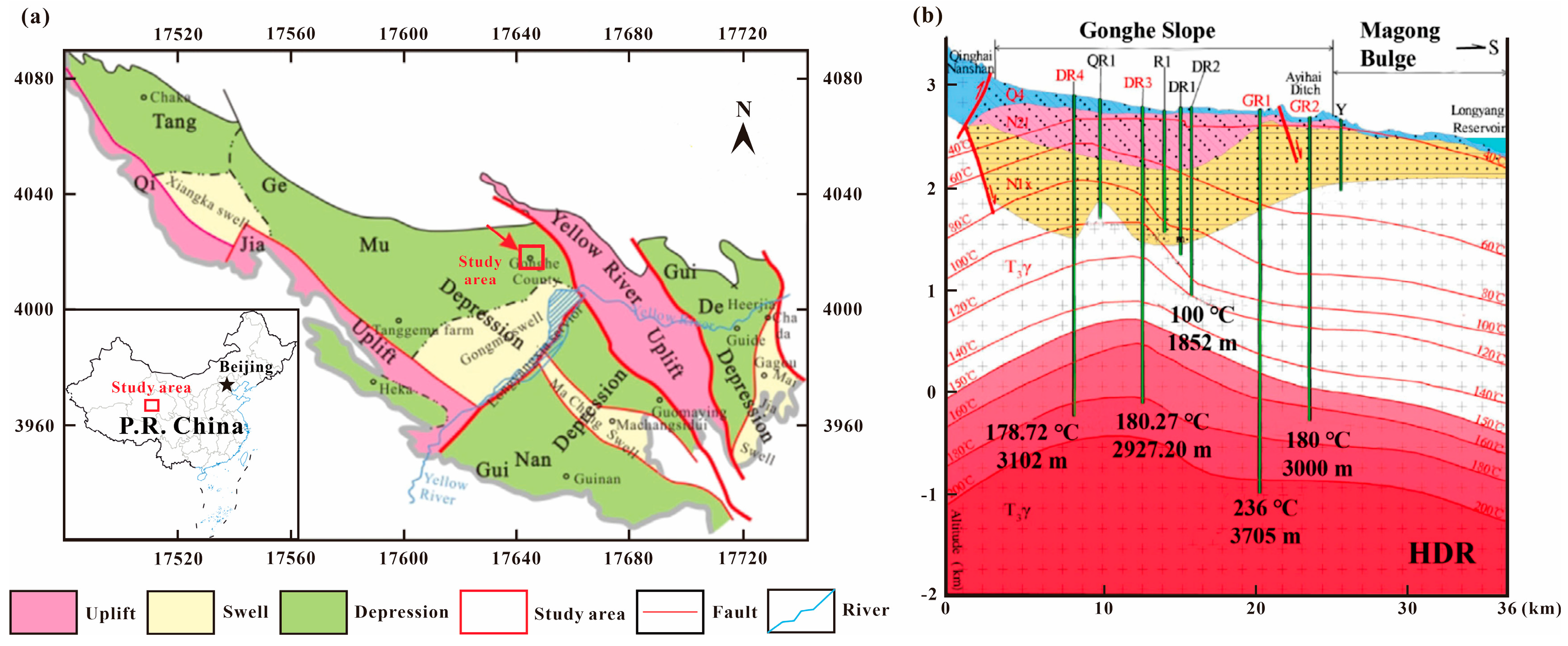
2. Characteristics of the Research Field
3. Model Description
3.1. Model Geometry
3.2. Computational Model and Grid Discretization
3.3. System Properties
3.4. Initial and Boundary Conditions
3.5. Simulation Cases
4. Results and Discussion
4.1. The Base Case
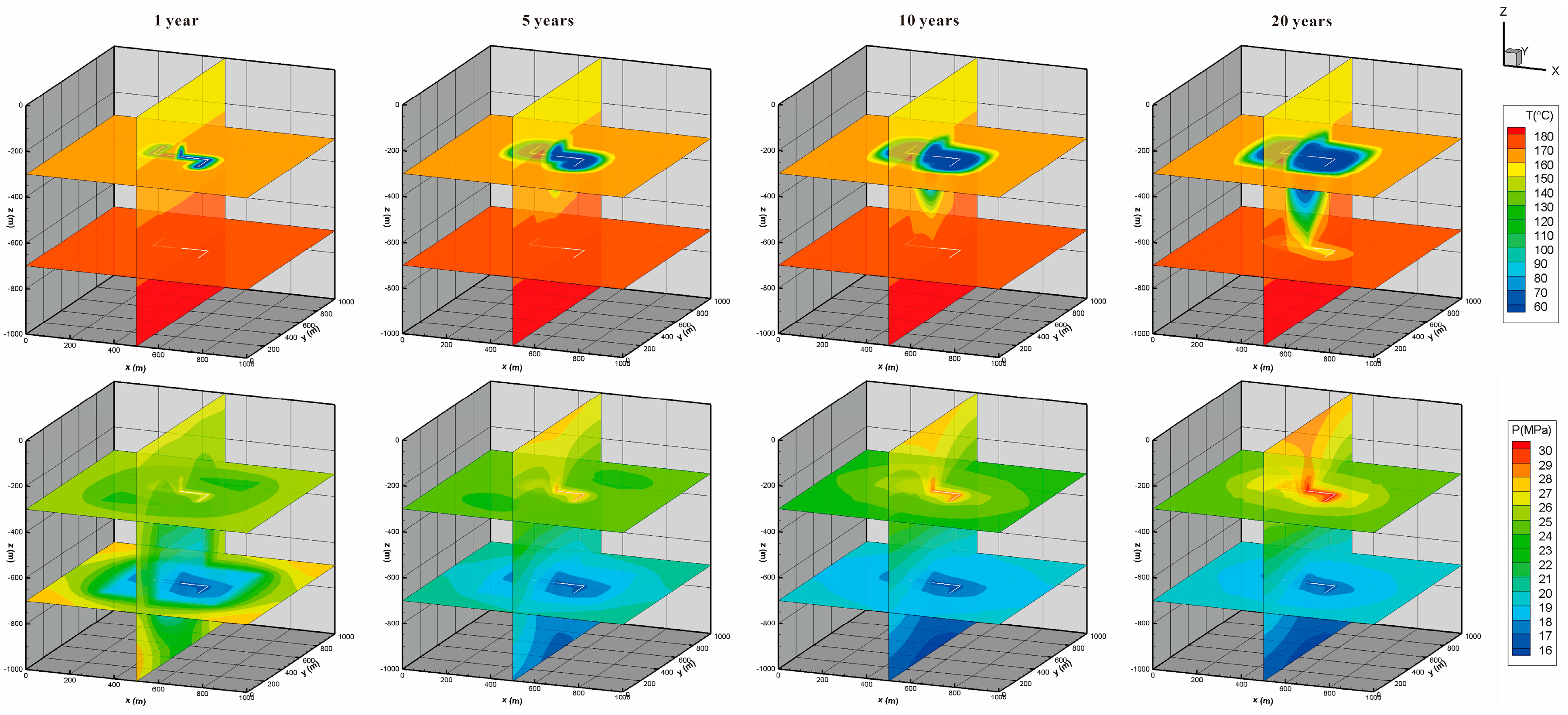
4.1.1. Production Temperature
4.1.2. Thermal Production and Electricity Generation
4.1.3. Injection Pressure and Flow Impedance
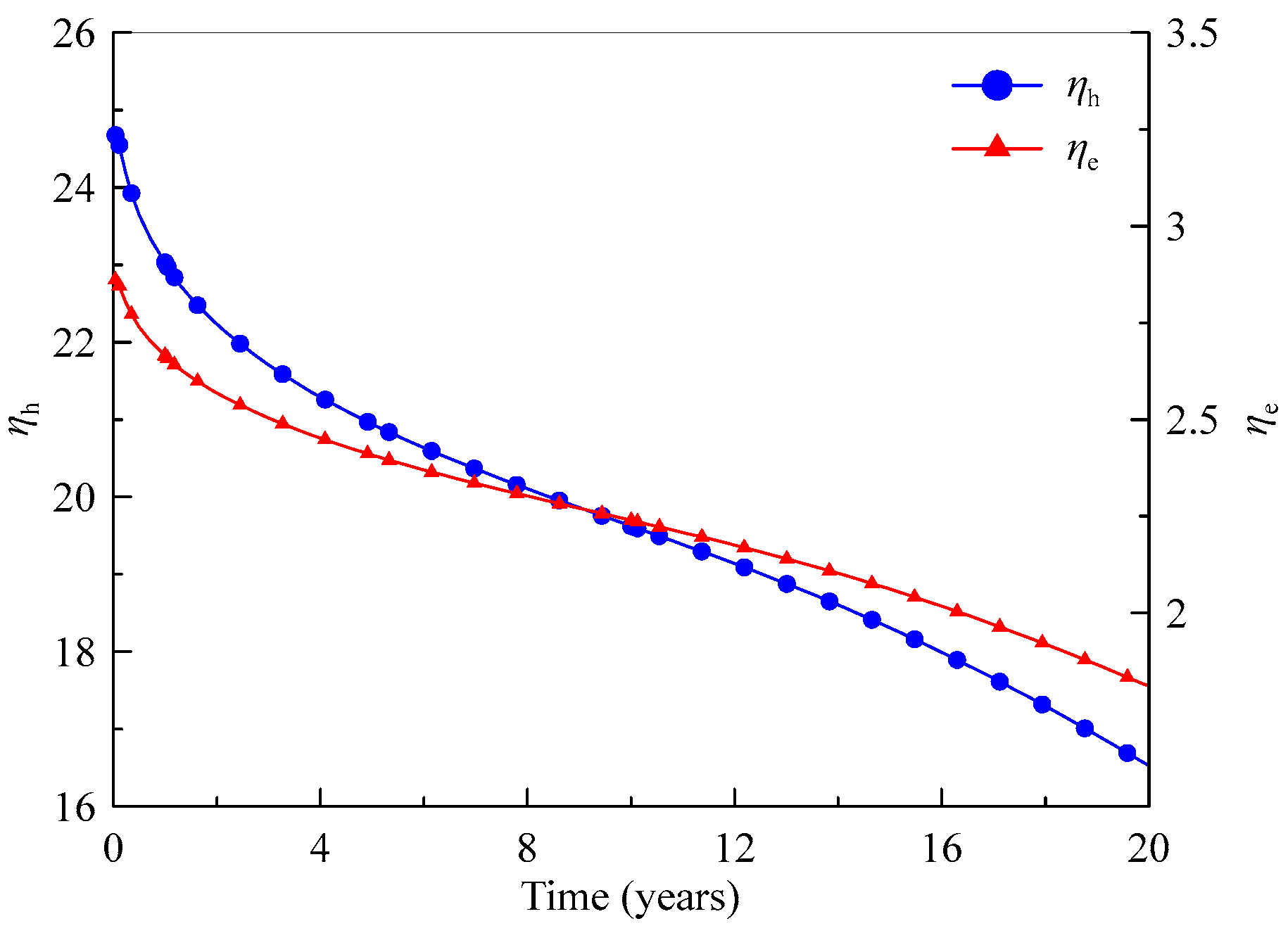
4.1.4. Energy Efficiency
4.1.5. Economic Assessment and Environmental Benefits
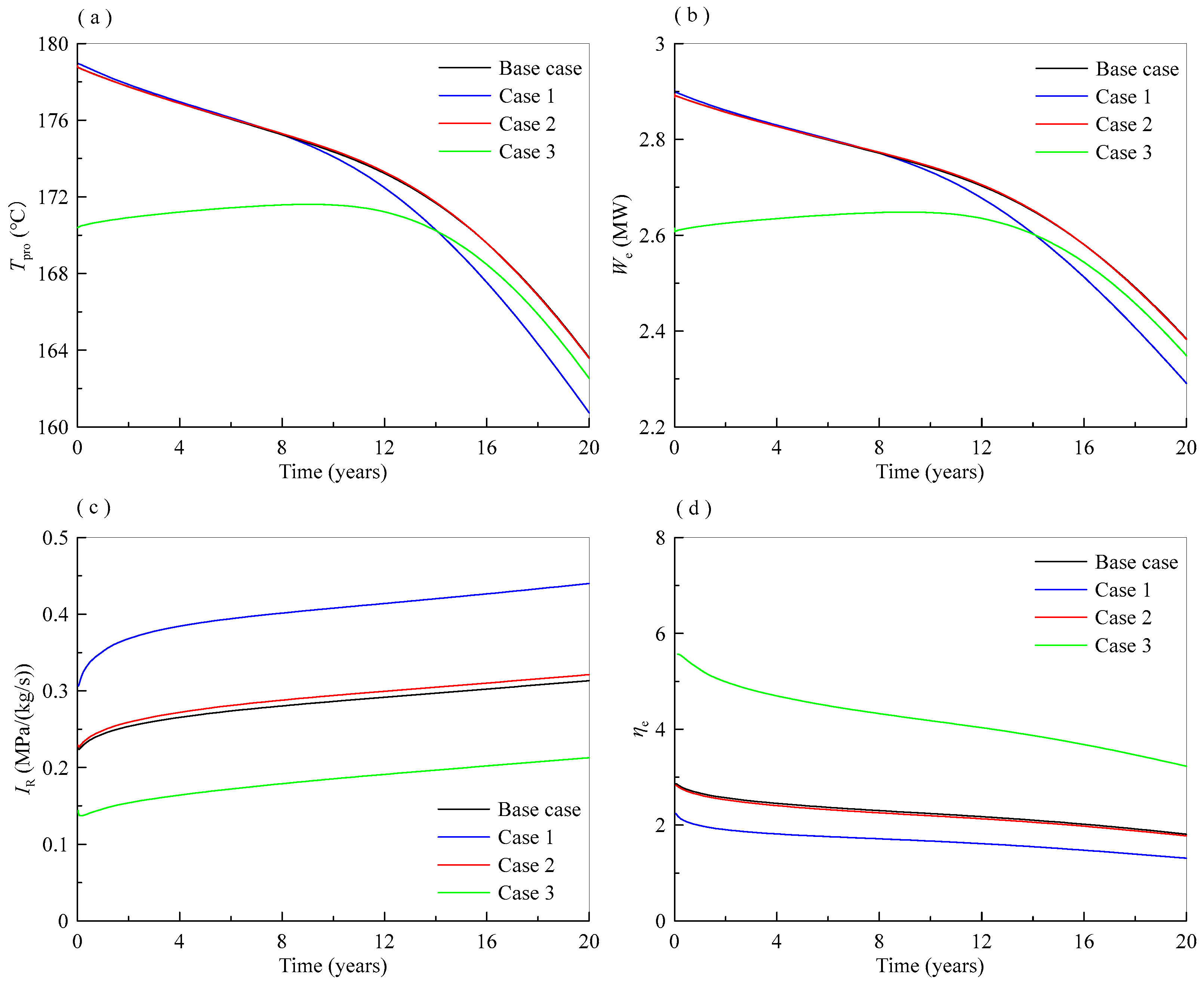
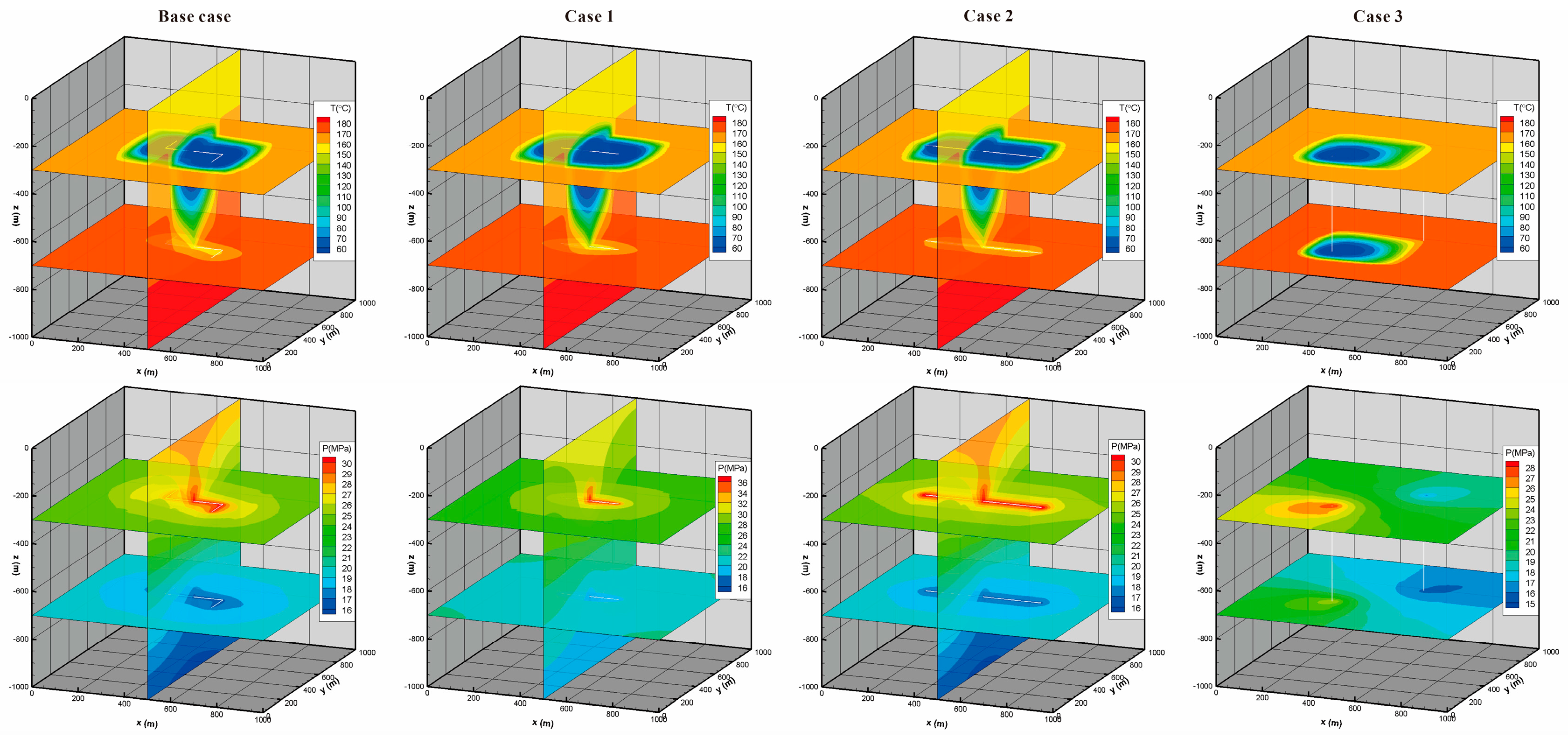
4.2. Comparisons of Base Case and Cases of Classic Double-Well EGS
4.3. Sensitivity Analysis
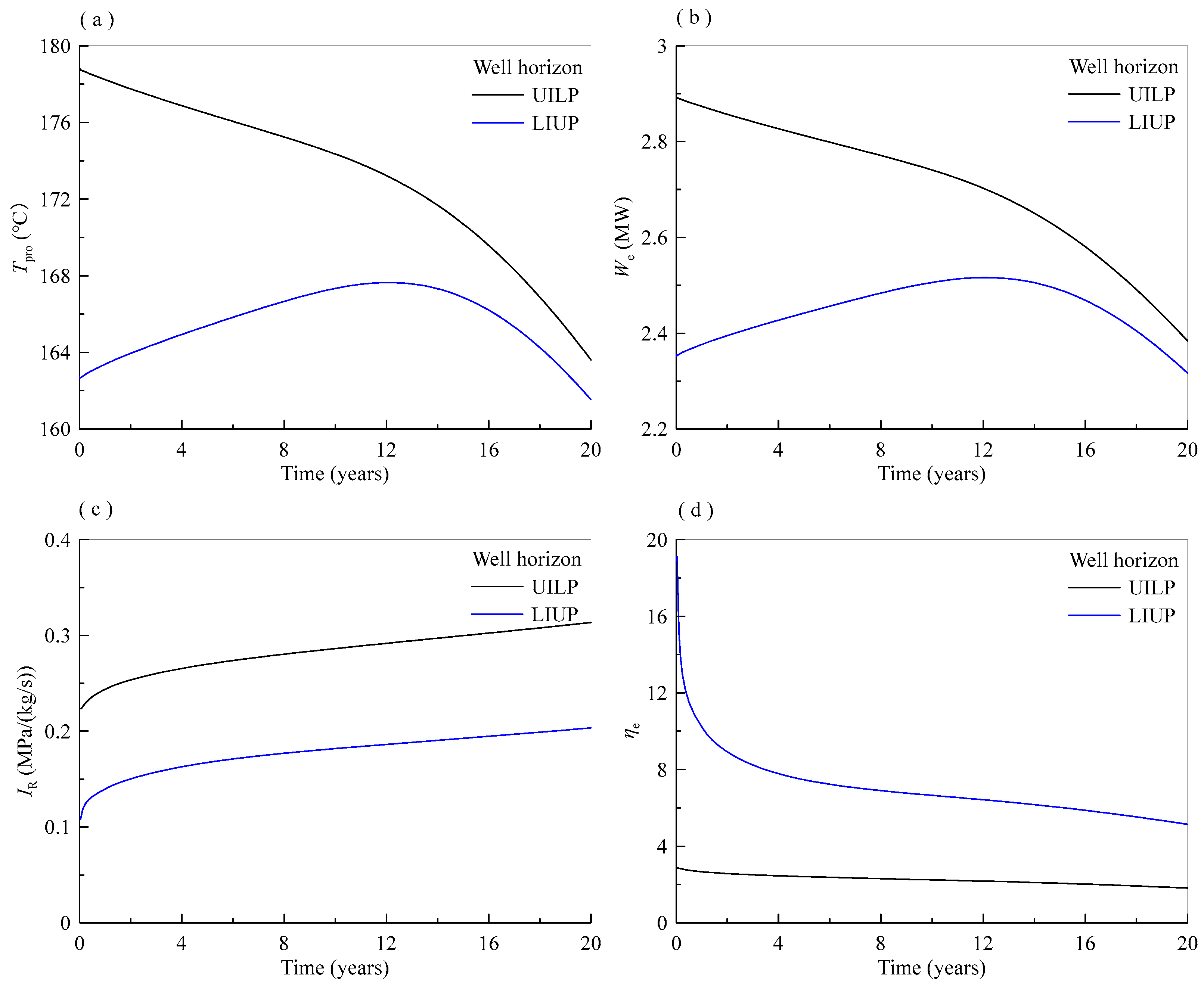
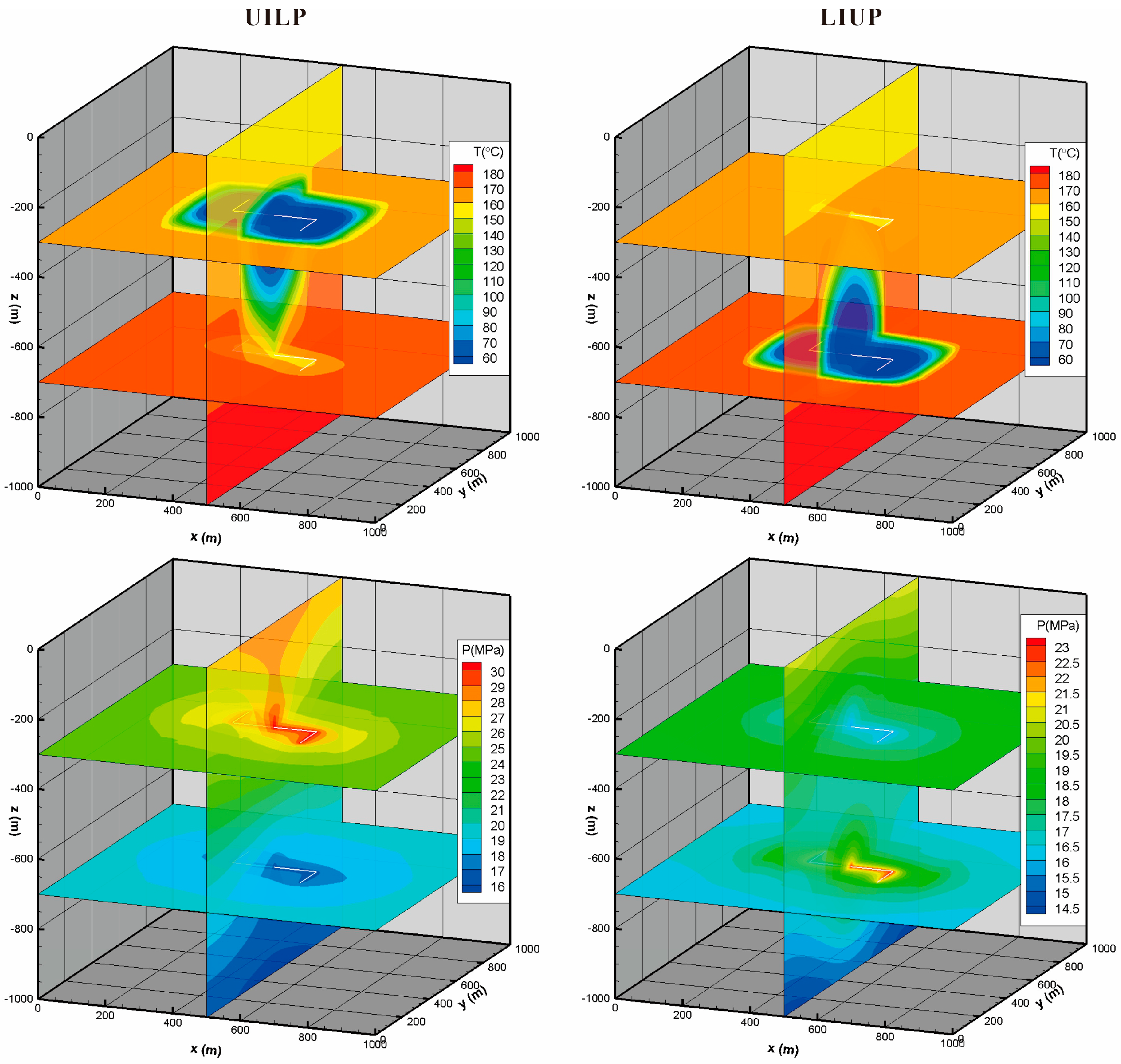
4.3.1. Influence of Well Depth
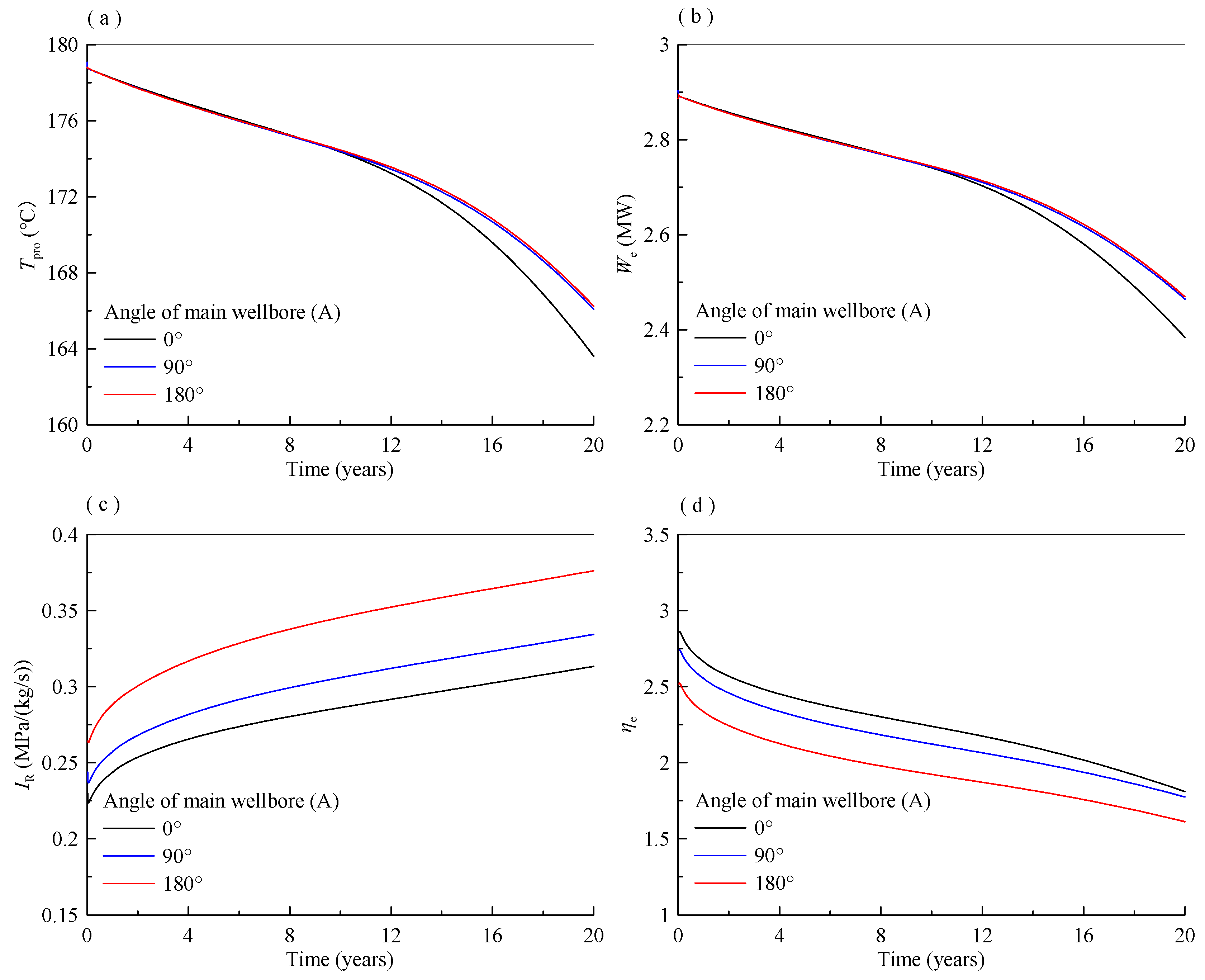
4.3.2. Influence of Angle of Main Wellbore
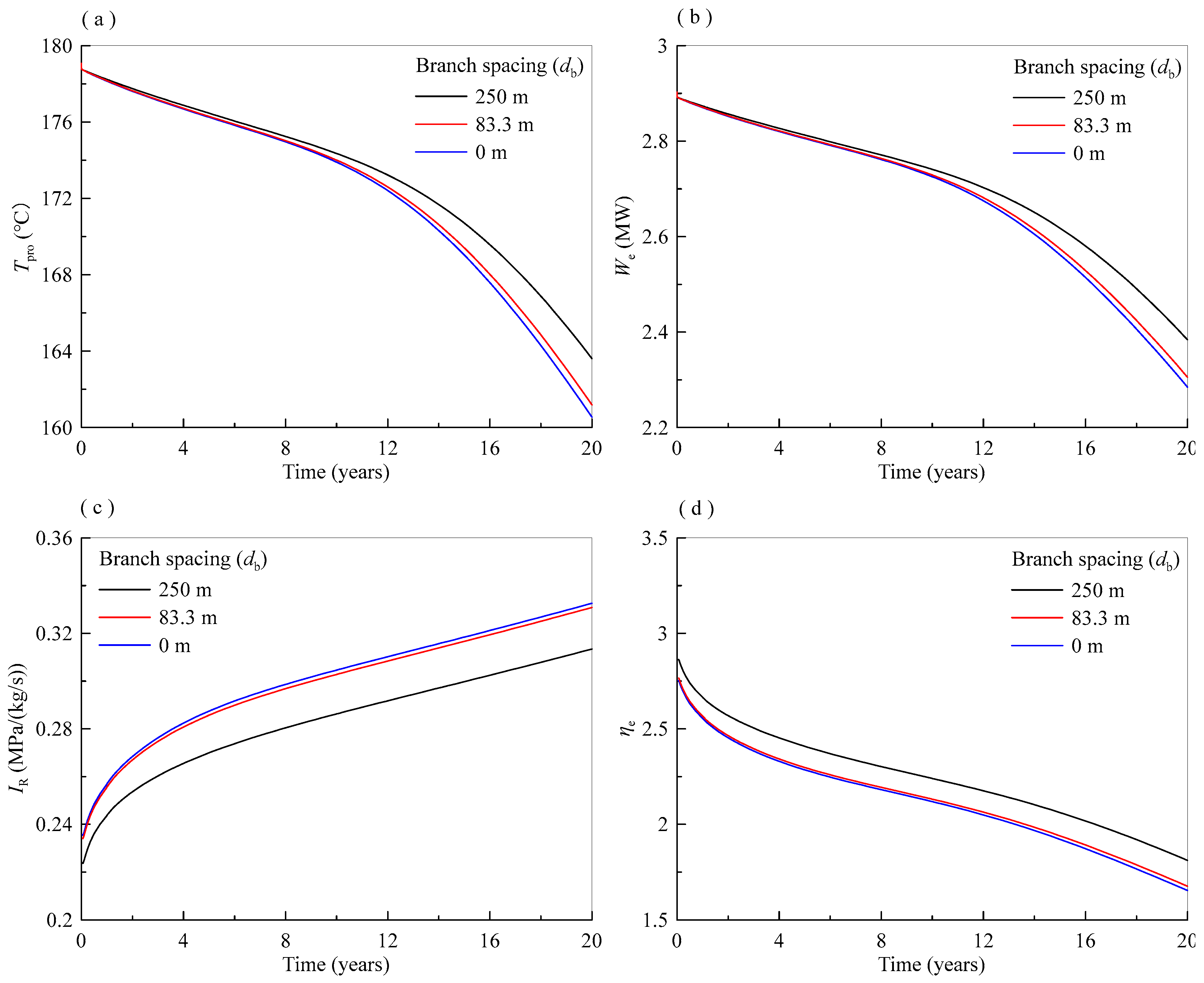
4.3.3. Influence of Branch Spacing
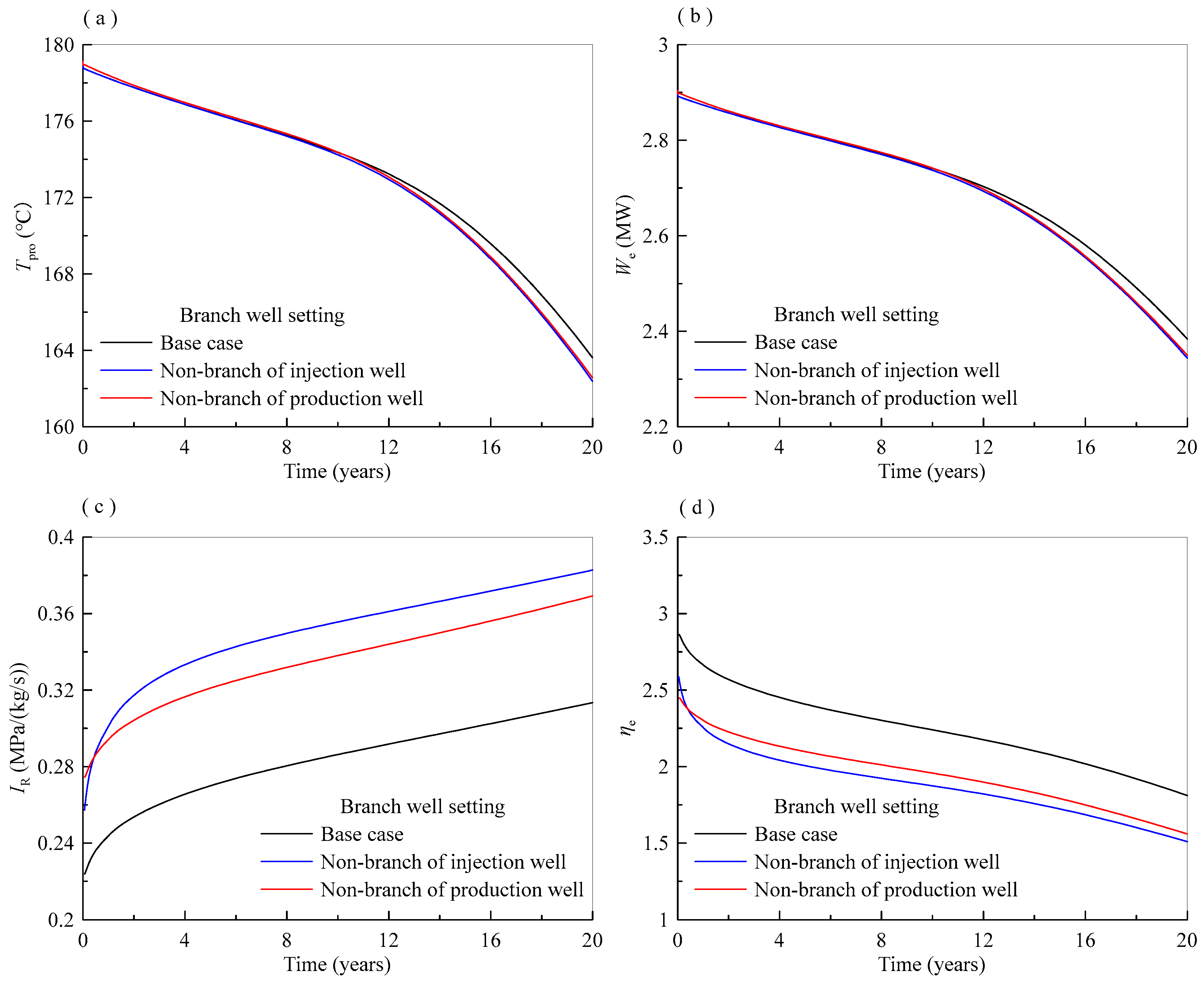
4.3.4. Influence of Branch Well Setting
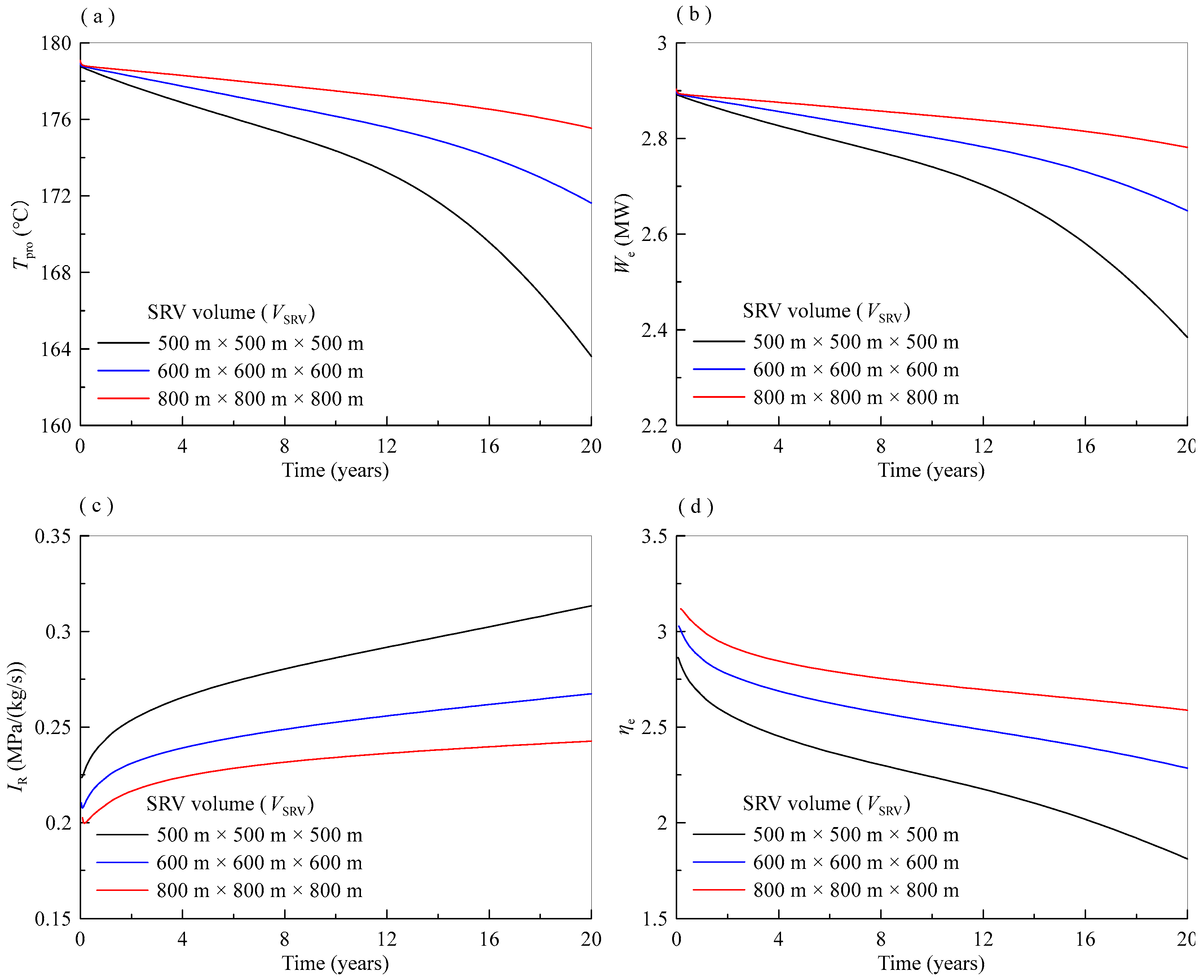
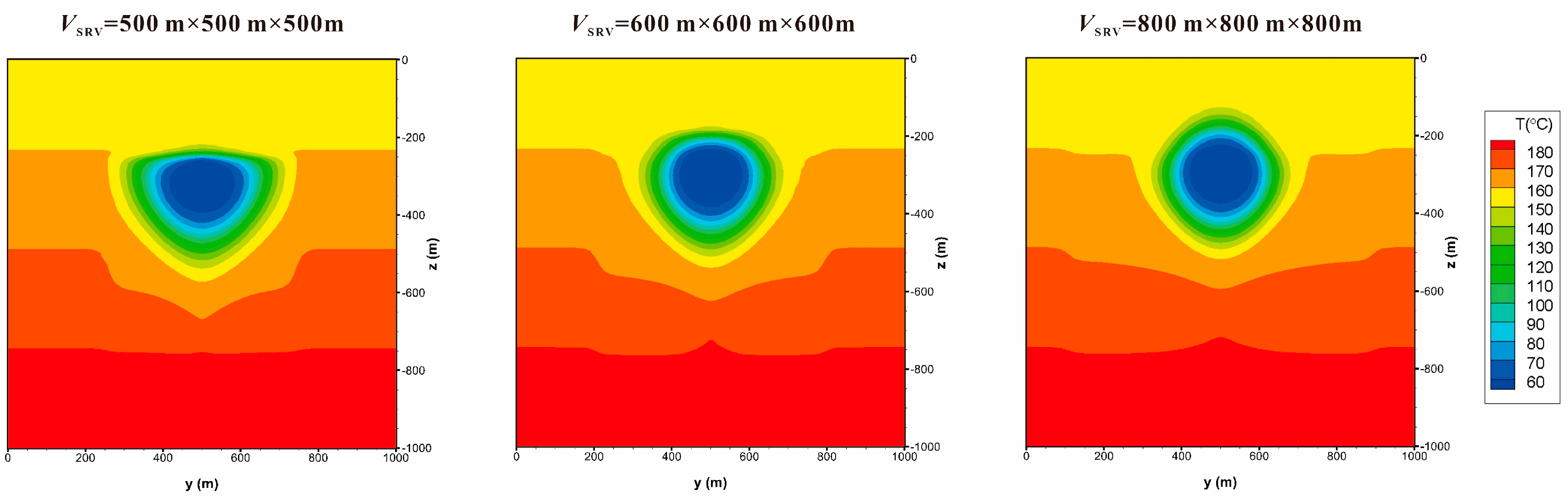
4.3.5. Influence of SRV Volume
4.4. Economic Analysis
5. Conclusions
- (1)
- For the basic multilateral horizontal well system, the initial production temperature and electric power reach 178.4 °C and 2.90 MW and decrease by 8.3% and 17.9% over 20 years of production, respectively, satisfying the thermal sustainability requirements for EGS development. Throughout the 20-year period, the system achieves an electric power of 2.38–2.90 MW, a flow impedance of 0.207–0.313 MPa/(kg/s), and an electric energy efficiency of 1.8–2.9.
- (2)
- Based on the simulation outcomes, the proposed configuration has an installed power capacity of 2.7 MW. The designed power plant is projected to produce around 432.48 GWh of electricity, with total costs estimated at USD 14.715 million. The LCOE is calculated as 0.062 USD/kWh. Additionally, the GHG emissions reduction is expected to range from 0.15 to 0.51 Mt over 20 years.
- (3)
- The multilateral horizontal well EGS achieves higher production temperature and electric power than the configurations of non-branch horizontal well and conventional vertical double-well EGS. The multilateral horizontal well EGS only required one vertical wellbore, significantly reducing drilling costs.
- (4)
- The heat production of geothermal reservoirs highly depends on the well depth and branch well arrangement. The layout of upper injection and lower production wells could result in a high production temperature but low energy efficiency. Meanwhile, the layout of lower injection and upper production wells leads to low production temperature but high energy efficiency. The layout featuring a lower injection and an upper production well is more favorable.
- (5)
- Increasing the angle of the main wellbore results in higher electric power, but the pump power consumption also increases. Reducing the branch spacing leads to a decrease in electric power and an increase in pump power consumption. Non-branching in the injection well and production well has little effect on electric power, but the pump power consumption increases significantly.
- (6)
- A large volume of stimulating reservoir can significantly reduce flow impedance, lower internal energy consumption, and enhance economic performance.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zheng, B.; Xu, J.; Ni, T.; Li, M. Geothermal energy utilization trends from a technological paradigm perspective. Renew. Energy 2015, 77, 430–441. [Google Scholar]
- International Energy Agency (nd) China Energy Data, IEA. Available online: https://www.iea.org/countries/china (accessed on 25 July 2024).
- Breede, K.; Dzebisashvili, K.; Liu, X.; Falcone, G. A systematic review of enhanced (or engineered) geothermal systems: Past, present and future. Geotherm. Energy 2013, 1, 4. [Google Scholar]
- Lukawski, M.Z.; Silverman, R.L.; Tester, J.W. Uncertainty analysis of geothermal well drilling and completion costs. Geothermics 2016, 64, 382–391. [Google Scholar] [CrossRef]
- Zeng, Y.; Zhan, J.; Wu, N.; Luo, Y.; Cai, W. Numerical investigation of electricity generation potential from fractured granite reservoir by water circulating through three horizontal wells at Yangbajing geothermal field. Appl. Therm. Eng. 2016, 104, 1–15. [Google Scholar] [CrossRef]
- Wang, H.; Guo, J.; Zhang, L. A semi-analytical model for multilateral horizontal wells in low-permeability naturally fractured reservoirs. J. Pet. Sci. Eng. 2017, 149, 564–578. [Google Scholar] [CrossRef]
- Cinelli, S.D.; Kamel, A.H. Novel Technique to Drill Horizontal Laterals Revitalizes Aging Field. Conference Novel Technique to Drill Horizontal Laterals Revitalizes Aging Field. In Proceedings of the SPE/IADC Drilling Conference, Amsterdam, The Netherlands, 5–7 March 2013. [Google Scholar]
- Zander, D.; Czehura, M.; Snyder, D.J.; Seale, R. Horizontal Drilling and Well Completion Optimization in a North Dakota Bakken Oilfield. In Proceedings of the SPE Annual Technical Conference and Exhibition, Florence, Italy, 19–22 September 2010. [Google Scholar]
- Rankin, R.; Thibodeau, M.; Vincent, M.C.; Palisch, T.T. Improved Production and Profitability Achieved with Superior Completions in Horizontal Wells: A Bakken/Three Forks Case History. In Proceedings of the SPE Annual Technical Conference and Exhibition, Florence, Italy, 19–22 September 2010. [Google Scholar]
- Mao, P.; Wan, Y.; Sun, J.; Li, Y.; Hu, G.; Ning, F.; Wu, N. Numerical study of gas production from fine-grained hydrate reservoirs using a multilateral horizontal well system. Appl. Energy 2021, 301, 117450. [Google Scholar]
- Zhang, B.; Li, X.; Zhao, Y.; Chang, C.; Zheng, J. A Review of Gas Flow and Its Mathematical Models in Shale Gas Reservoirs. Geofluids 2020, 2020, 8877777. [Google Scholar] [CrossRef]
- Ye, J.; Qin, X.; Xie, W.; Lu, H.; Ma, B.; Qiu, H.; Jinqiang, L.; Jing’an, L.; Zenggui, K.; Cheng, L.; et al. Main progress of the second gas hydrate trial production in the South China Sea. Geol. China 2020, 47, 557–568. (In Chinese) [Google Scholar]
- Ren, X.; Zhou, L.; Zhou, J.; Lu, Z.; Su, X. Numerical analysis of heat extraction efficiency in a multilateral-well enhanced geothermal system considering hydraulic fracture propagation and configuration. Geothermics 2020, 87, 101834. [Google Scholar] [CrossRef]
- Daryasafar, A.; Shahbazi, K.; Ghadiri, M.; Marjani, A. Simultaneous geological CO2 sequestration and gas production from shale gas reservoirs: Brief review on technology, feasibility, and numerical modeling. Energy Sources Part A Recovery Util. Environ. Eff. 2020, 47, 1472–1489. [Google Scholar]
- Ragab, A.M.; Kamel, A.M. Radial Drilling Technique for Improving Well Productivity in Petrobel-Egypt. In Proceedings of the SPE North Africa Technical Conference and Exhibition, Cairo, Egypt, 15–17 April 2013. [Google Scholar]
- Nair, R.; Peters, E.; Sliaupa, S.; Valickas, R.; Petrauskas, S. A case study of radial jetting technology for enhancing geothermal energy systems at Klaipeda geothermal demonstration plant. In Proceedings of the 42nd Workshop on Geothermal Reservoir Engineering Stanford University, Stanford, CA, USA, 13–15 February 2017; pp. 1–11. [Google Scholar]
- Xie, J.; Wang, J.; Liu, X. Performance analysis of pinnate horizontal well in enhanced geothermal system. Appl. Therm. Eng. 2022, 201, 117799. [Google Scholar]
- Song, X.; Shi, Y.; Li, G.; Yang, R.; Wang, G.; Zheng, R.; Li, J.; Lyu, Z. Numerical simulation of heat extraction performance in enhanced geothermal system with multilateral wells. Appl. Energy 2018, 218, 325–337. [Google Scholar]
- Zhang, X.; Huang, Z.; Li, G.; Wu, X.; Wang, T.; Zhou, X. Enhancing reservoir stimulation and heat extraction performance for fractured geothermal reservoirs: Utilization of novel multilateral wells. Energy 2024, 291, 130410. [Google Scholar]
- Zhang, W.; Wang, M.; Wei, Z.; Yu, H.; Wang, C.; Wang, D.; Guo, T. Study of the fracture propagation and the corresponding heat mining performance of multi-branch radial wells geothermal system based on the THM-D coupling model. Geoenergy Sci. Eng. 2024, 243, 213302. [Google Scholar]
- Wang, C.; Lv, Y. Gonghe Basin: A new and worth-researching basin. Xinjiang Pet. Geol. 2004, 25, 471–473. (In Chinese) [Google Scholar]
- Zhang, C.; Zhang, S.; Li, S.; Jia, X.; Jiang, G.; Gao, P.; Wang, Y.; Hu, S. The characteristics of geothermal field of the Qiabuqia geothermal area in the Gonghe basin, northeastern Tibetan Plateau. Chin. J. Geophys. 2018, 61, 4545–4557. (In Chinese) [Google Scholar]
- Zhang, C.; Jiang, G.; Shi, Y.; Wang, Z.; Wang, Y.; Li, S.; Jia, X.; Hu, S. Terrestrial heat flow and crustal thermal structure of the Gonghe-Guide area, northeastern Qinghai-Tibetan plateau. Geothermics 2018, 72, 182–192. [Google Scholar]
- Lei, Z.; Zhang, Y.; Zhang, S.; Fu, L.; Hu, Z.; Yu, Z.; Li, L.; Zhou, J. Electricity generation from a three-horizontal-well enhanced geothermal system in the Qiabuqia geothermal field, China: Slickwater fracturing treatments for different reservoir scenarios. Renew. Energy 2020, 145, 65–83. [Google Scholar] [CrossRef]
- Lei, Z.; Zhang, Y.; Yu, Z.; Hu, Z.; Li, L.; Zhang, S.; Fu, L.; Zhou, L.; Xie, Y. Exploratory research into the enhanced geothermal system power generation project: The Qiabuqia geothermal field, Northwest China. Renew. Energy 2019, 139, 52–70. [Google Scholar]
- Xu, T.; Yuan, Y.; Jia, X.; Lei, Y.; Li, S.; Feng, B.; Hou, Z.; Jiang, Z. Prospects of power generation from an enhanced geothermal system by water circulation through two horizontal wells: A case study in the Gonghe Basin, Qinghai Province, China. Energy 2018, 148, 196–207. [Google Scholar]
- Zhang, J.; Xie, J.; Zhang, H. Production capacity and mining plan optimization of fault/fracture-controlled EGS model in Gonghe Basin. Energy Sci. Eng. 2019, 7, 2966–2983. [Google Scholar] [CrossRef]
- Zhai, H.; Jin, G.; Liu, L.; Su, Z.; Zeng, Y.; Liu, J.; Li, G.; Feng, C.; Wu, N. Parametric study of the geothermal exploitation performance from a HDR reservoir through multilateral horizontal wells: The Qiabuqia geothermal area, Gonghe Basin. Energy 2023, 275, 127370. [Google Scholar] [CrossRef]
- Zhong, C.; Xu, T.; Gherardi, F.; Yuan, Y. Comparison of CO2 and water as working fluids for an enhanced geothermal system in the Gonghe Basin, northwest China. Gondwana Res. 2023, 122, 199–214. [Google Scholar] [CrossRef]
- Borgia, A.; Cattaneo, L.; Marconi, D.; Delcroix, C.; Rossi, E.L.; Clemente, G.; Amoroso, C.; Re, F.L.; Tozzato, E. Using a MODFLOW grid, generated with GMS, to solve a transport problem with TOUGH2 in complex geological environments: The intertidal deposits of the Venetian Lagoon. Comput. Geosci. 2011, 37, 783–790. [Google Scholar] [CrossRef]
- Pruess, K.; Oldenburg, C.M.; Moridis, G.J. TOUGH2 User’s Guide Version 2; Lawrence Berkeley National Lab. (LBNL): Berkeley, CA, USA, 1999; 204p. [Google Scholar]
- Yue, G.; Deng, X.; Xing, L.; Lin, W.; Liu, F.; Liu, Y.; Wang, G. Numerical simulation of hot dry rock exploitation using enhanced geothermal systems in Gonghe Basin. Sci. Technol. Rev. 2015, 33, 62–67. (In Chinese) [Google Scholar]
- Zhang, C.; Jiang, G.; Jia, X.; Li, S.; Zhang, S.; Hu, D.; Hu, S.; Wang, Y. Parametric study of the production performance of an enhanced geothermal system: A case study at the Qiabuqia geothermal area, northeast Tibetan plateau. Renew. Energy 2019, 132, 959–978. [Google Scholar] [CrossRef]
- Popov, Y.A.; Pribnow, D.F.C.; Sass, J.H.; Williams, C.F.; Burkhardt, H. Characterization of rock thermal conductivity by high-resolution optical scanning. Geothermics 1999, 28, 253–276. [Google Scholar] [CrossRef]
- Zhuang, Y.; Zhang, C.; Zhu, M.; Zhu, Y.; Luo, J.; Guo, Q. Laboratory study of improving granite permeability by using mud acid as the chemical stimulus. Saf. Environ. Eng. 2017, 24, 16–21+45. (In Chinese) [Google Scholar]
- Evans, K. Enhanced/engineered geothermal system: An introduction with overviews of deep systems built and circulated to date. In Proceedings of the China Geothermal Development Forum Beijing, Beijing, China, 6–7 November 2010; pp. 410–433. [Google Scholar]
- Sanyal, S.; Butler, S. An Analysis of Power Generation Prospects from Enhanced Geothermal Systems. Geotherm. Resour. Counc. Trans. 2005, 29, 131–138. [Google Scholar]
- Garnish, J.D. Hot Dry Rock—A European Perspective. Geotherm. Resour. Counc. Trans. 1985, 9, 329–337. [Google Scholar]
- Liu, B.; Jin, H.; Sun, L.; Sun, Z.; Su, Z.; Zhang, C. Holocene climatic change revealed by aeolian deposits from the Gonghe Basin, northeastern Qinghai–Tibetan Plateau. Quat. Int. 2013, 296, 231–240. [Google Scholar] [CrossRef]
- Zeng, Y.; Zhan, J.; Wu, N.; Luo, Y.; Cai, W. Numerical simulation of electricity generation potential from fractured granite reservoir through vertical wells at Yangbajing geothermal field. Energy 2016, 103, 290–304. [Google Scholar]
- Tester, J.W.; Anderson, B.J.; Batchelor, A.S.; Blackwell, D.D.; DiPippo, R. The Future of Geothermal Energy. Mass. Inst. Technol. 2006, 358, 1–3. [Google Scholar]
- Murphy, H.; Brown, D.; Jung, R.; Matsunaga, I.; Parker, R. Hydraulics and well testing of engineered geothermal reservoirs. Geothermics 1999, 28, 491–506. [Google Scholar]
- Zeng, Y.; Su, Z.; Wu, N. Numerical simulation of heat production potential from hot dry rock by water circulating through two horizontal wells at Desert Peak geothermal field. Energy 2013, 56, 92–107. [Google Scholar]
- Feng, Z.; Zhao, Y.; Zhou, A.; Zhang, N. Development program of hot dry rock geothermal resource in the Yangbajing Basin of China. Renew. Energy 2012, 39, 490–495. [Google Scholar]
- Zhang, Y.; Li, Z.; Guo, L.; Gao, P.; Jin, X.; Xu, T. Electricity generation from enhanced geothermal systems by oilfield produced water circulating through reservoir stimulated by staged fracturing technology for horizontal wells: A case study in Xujiaweizi area in Daqing Oilfield, China. Energy 2014, 78, 788–805. [Google Scholar] [CrossRef]
- Chamorro, C.R.; Mondéjar, M.E.; Ramos, R.; Segovia, J.J.; Martín, M.C.; Villamañán, M.A. World geothermal power production status: Energy, environmental and economic study of high enthalpy technologies. Energy 2012, 42, 10–18. [Google Scholar] [CrossRef]
- Bertani, R.; Thain, I. Geothermal power generating plant CO2 emission survey. IGA News 2002, 49, 1–3. [Google Scholar]
- Kristmannsdóttir, H.; Ármannsson, H. Environmental aspects of geothermal energy utilization. Geothermics 2003, 32, 451–461. [Google Scholar]
- Yin, Y.; Jiang, Z.; Liu, Y.; Yu, Z. Factors Affecting Carbon Emission Trading Price: Evidence from China. Emerg. Mark. Financ. Trade 2019, 55, 3433–3451. [Google Scholar] [CrossRef]

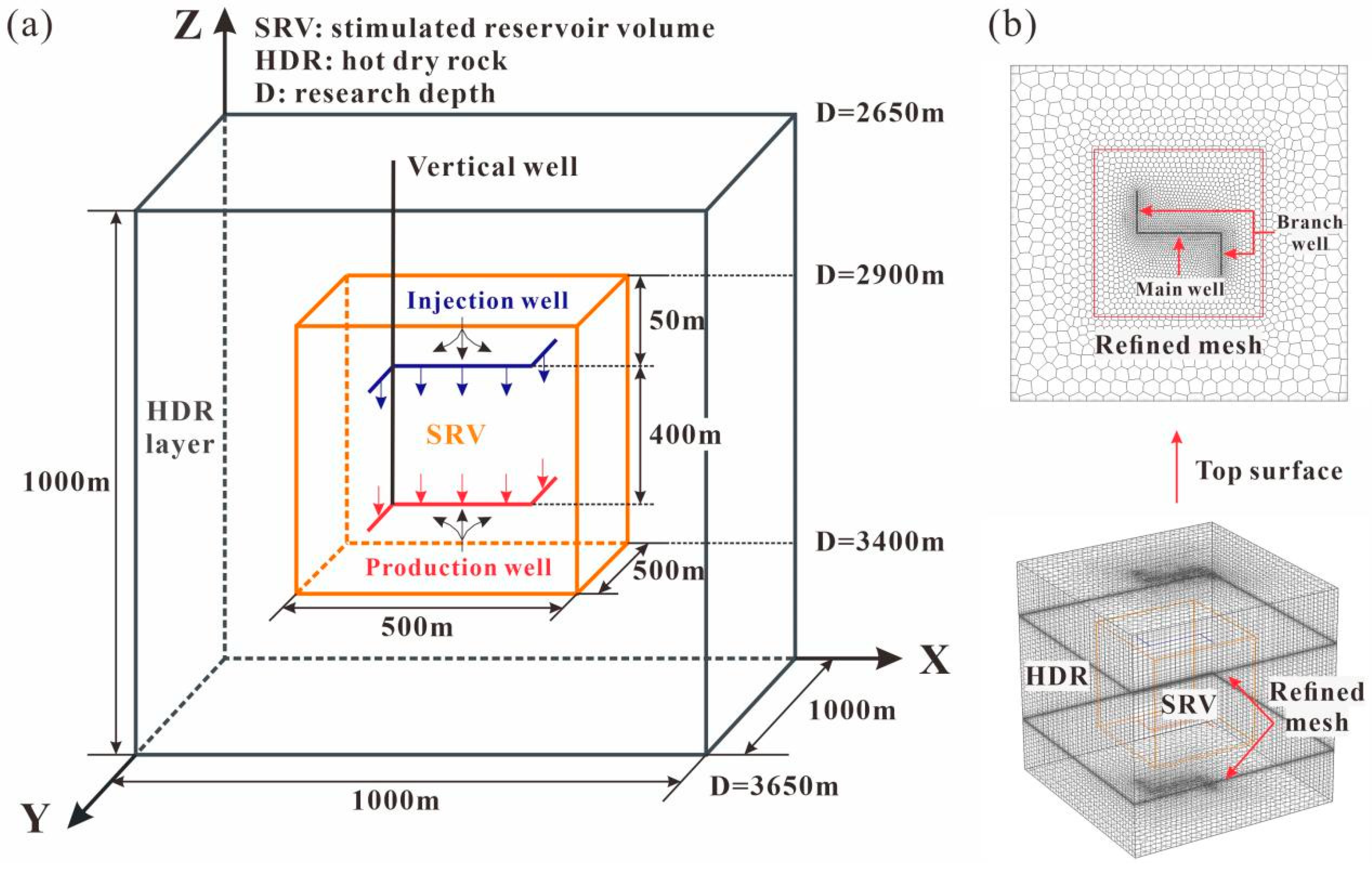







| Parameter | Value |
|---|---|
| Computational dimensions | 1000 m × 1000 m × 1000 m |
| SRV dimensions | 500 m × 500 m × 500 m |
| Length of main horizontal wellbore | 250 m |
| Total length of horizontal lateral wells | 500 m |
| Number of branch wells | 2 |
| Branch well length | 125 m |
| Branch well spacing | 250 m |
| Well radius | 0.1 m |
| Injection and production well spacing | 400 m |
| Density of HDR layer | 2652 kg/m3 |
| Thermal conductivity of HDR layer | 2.43 W/(m·K) |
| Heat capacity of HDR layer | 1100 J/(kg·K) |
| Porosity of HDR layer | 3% |
| Permeability of HDR layer | 2.8 × 10−18 m2 |
| Density of SRV | 2500 kg/m3 |
| Thermal conductivity of SRV | 2.81 W/(m·K) |
| Heat capacity of SRV | 900 J/(kg·K) |
| Porosity of SRV | 10% |
| Permeability of SRV | 5.0 × 10−15 m2 |
| Productivity index, PI | 8.18 × 10−12 m3 |
| Production pressure | 15 MPa |
| Case ID | Well Type | Main Wellbore Length | Well Depth | Branch Number | Branch Length | Branch Spacing | Main Horizontal Well Angle | Volume of SRV | Remark |
|---|---|---|---|---|---|---|---|---|---|
| Base case | Horizontal | 250 m | UILP | 2 | 125 m | 250 m | 0° | 500 m × 500 m × 500 m | Base case |
| Case 1 | - | - | - | - | Classic double-well EGS | ||||
| Case 2 | 500 m | - | - | - | - | ||||
| Case 3 | Vertical | 500 m | - | - | - | - | - | ||
| Case 4 | Horizontal | 250 m | LIUP | 2 | 125 m | 250 m | 0° | Well depth | |
| Case 5 | UILP | 2 | 125 m | 250 m | 90° | Angle of the main horizontal well | |||
| Case 6 | 180° | ||||||||
| Case 7 | 83.3 m | 0° | Branch spacing | ||||||
| Case 8 | 0 m | ||||||||
| Case 9 | Non-branch for injection well | Branch well setting | |||||||
| Case 10 | Non-branch for production well | ||||||||
| Case 11 | 125 m | 250 m | 600 m × 600 m × 600 m | Volume of SRV | |||||
| Case 12 | 800 m × 800 m × 800 m | ||||||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhai, H.; Jin, G.; Liu, L.; Lu, J.; Su, Z.; Zhang, J.; Feng, C.; Zeng, Y.; Wu, N. Enhancement of Geothermal Exploitation in Hot Dry Rock Reservoir Through Multilateral Horizontal Well Systems: A Case Study in Qiabuqia Geothermal Field, Gonghe Basin. Processes 2025, 13, 1020. https://doi.org/10.3390/pr13041020
Zhai H, Jin G, Liu L, Lu J, Su Z, Zhang J, Feng C, Zeng Y, Wu N. Enhancement of Geothermal Exploitation in Hot Dry Rock Reservoir Through Multilateral Horizontal Well Systems: A Case Study in Qiabuqia Geothermal Field, Gonghe Basin. Processes. 2025; 13(4):1020. https://doi.org/10.3390/pr13041020
Chicago/Turabian StyleZhai, Haizhen, Guangrong Jin, Lihua Liu, Jingsheng Lu, Zheng Su, Jie Zhang, Chuangji Feng, Yuchao Zeng, and Nengyou Wu. 2025. "Enhancement of Geothermal Exploitation in Hot Dry Rock Reservoir Through Multilateral Horizontal Well Systems: A Case Study in Qiabuqia Geothermal Field, Gonghe Basin" Processes 13, no. 4: 1020. https://doi.org/10.3390/pr13041020
APA StyleZhai, H., Jin, G., Liu, L., Lu, J., Su, Z., Zhang, J., Feng, C., Zeng, Y., & Wu, N. (2025). Enhancement of Geothermal Exploitation in Hot Dry Rock Reservoir Through Multilateral Horizontal Well Systems: A Case Study in Qiabuqia Geothermal Field, Gonghe Basin. Processes, 13(4), 1020. https://doi.org/10.3390/pr13041020







